A Novel Fairness-Based Cost Model for Adopting Smart Charging at Fast Charging Stations
Abstract
:1. Introduction
1.1. Background
1.2. Aim of the Work
1.3. Contribution
2. Characteristics of Smart Charging at FCSs
2.1. Smart Charging Power
- Premium;
- Regular;
- Economic.
- Premium charging power: can provide an EV with an output power () ranging from to of the maximum output power .
- Regular charging power: can provide an EV with an output power () ranging from to of the maximum output power .
- Economic charging power: can provide an EV with an output power () ranging from to of the maximum output power .
2.2. Smart Charging Constraints
3. Cost of Smart Charging at FCSs
3.1. Cost of Flicker Mitigation Technologies
- : the per unit cost of the unified power quality conditioner,
- the per unit cost of the distribution static compensator,
- the per unit cost of the thyristor switched capacitor,
- the per unit cost of the fixed capacitors/thyristor-controlled reactors,
- : the per unit cost of the dynamic voltage restorer,
- the per unit cost of the fixed series capacitor,
- the operating range of the unified power quality conditioner,
- the operating range of the distribution static compensator,
- the operating range of the thyristor switched capacitor,
- the operating range of the fixed capacitors/thyristor-controlled reactors,
- the operating range of the dynamic voltage restorer,
- the operating range of the fixed series capacitor,
3.2. Comparison of Costs of Flicker Mitigation Technologies
3.3. Determining the per Unit Time of the Smart Charging
3.4. Determining the per Unit Cost of the Smart Charging
3.4.1. The per Unit Cost of the Premium Charging Power
3.4.2. The per Unit Cost of the Regular Charging Power
3.4.3. The per Unit Cost of the Economic Charging Power
3.5. Determining the Maximum Charging Cost
3.6. Determining the Maximum Charging Duration
4. FCS Annual Energy Profile
4.1. FCS Data
4.2. Modelling of Per Event Energy Demand from the FCS
4.3. Modelling of Annual Energy Demand from the FCS
5. Computing the Cost of Smart Charging
5.1. Computing the Rebates
5.2. Computing the Revenue
- The percent of vehicles that use premium charging power is ,
- The percent of vehicles that use regular charging power is ,
- The percent of vehicles that use premium charging power is .
- The deterministic factor is penetration level , which is varied from 0% up to 100% by 10%.
- The stochastic factor is , which is generated using a uniform distribution.
- The annual charging energy () is another stochastic factor which represents the vehicle demand from the FCS per year.
5.3. Comparison of Rebate and Revenue
6. Results and Discussions
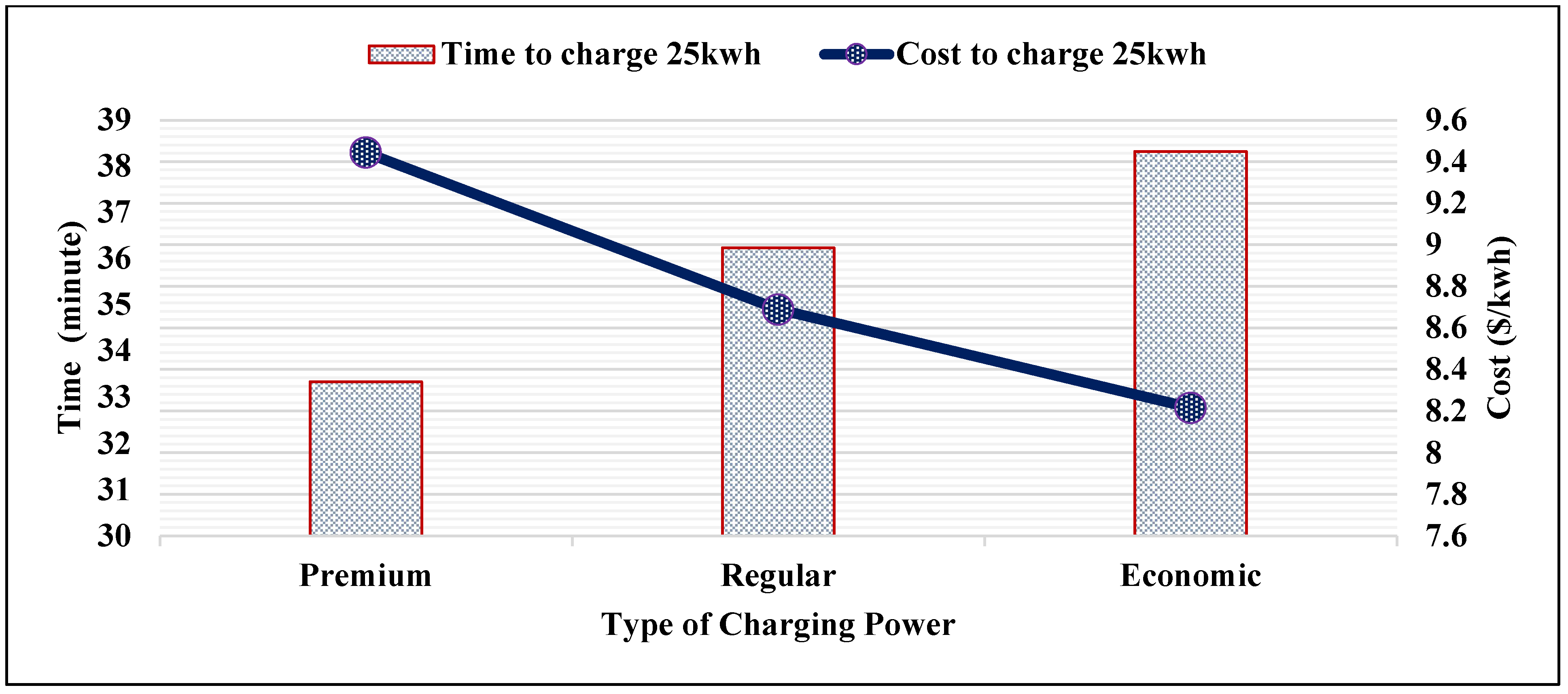
6.1. Per Unit Time and per Unit Cost of the Smart Charging
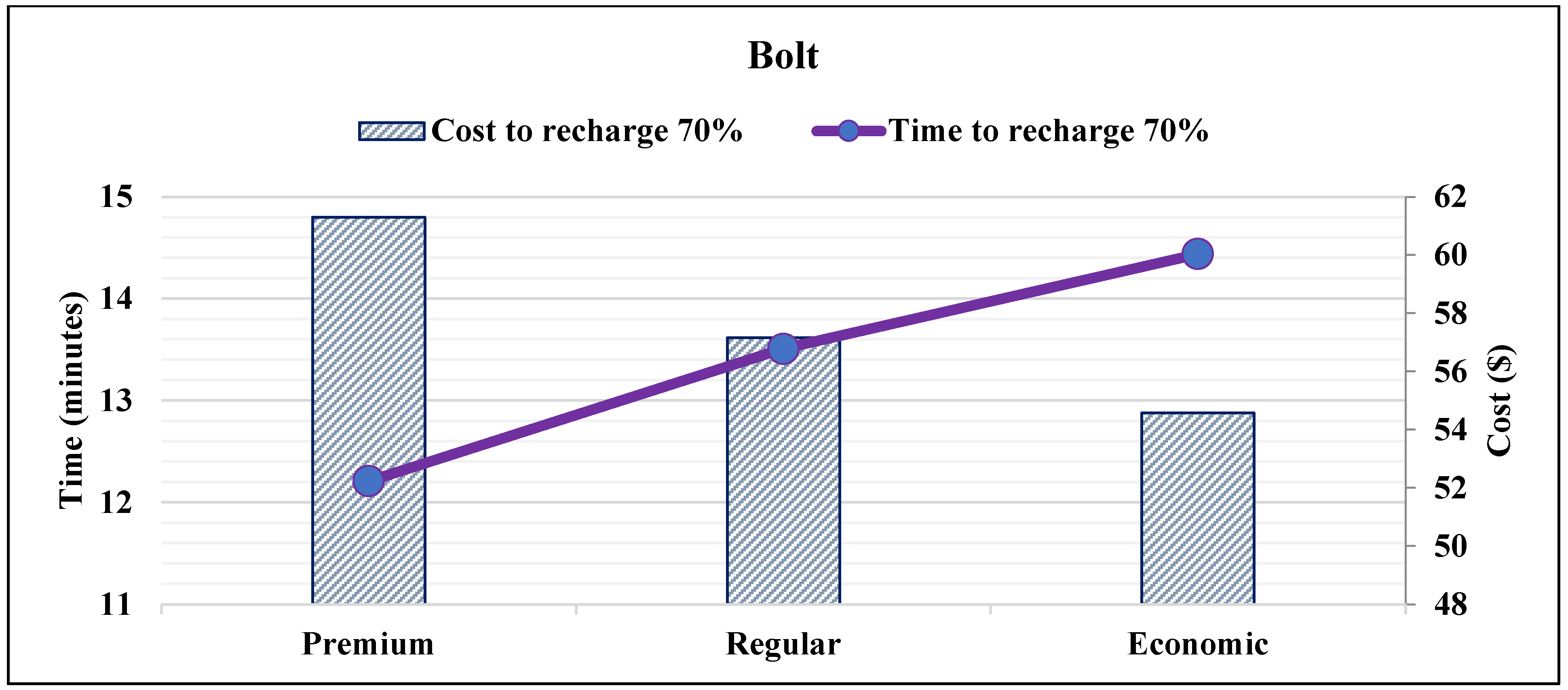
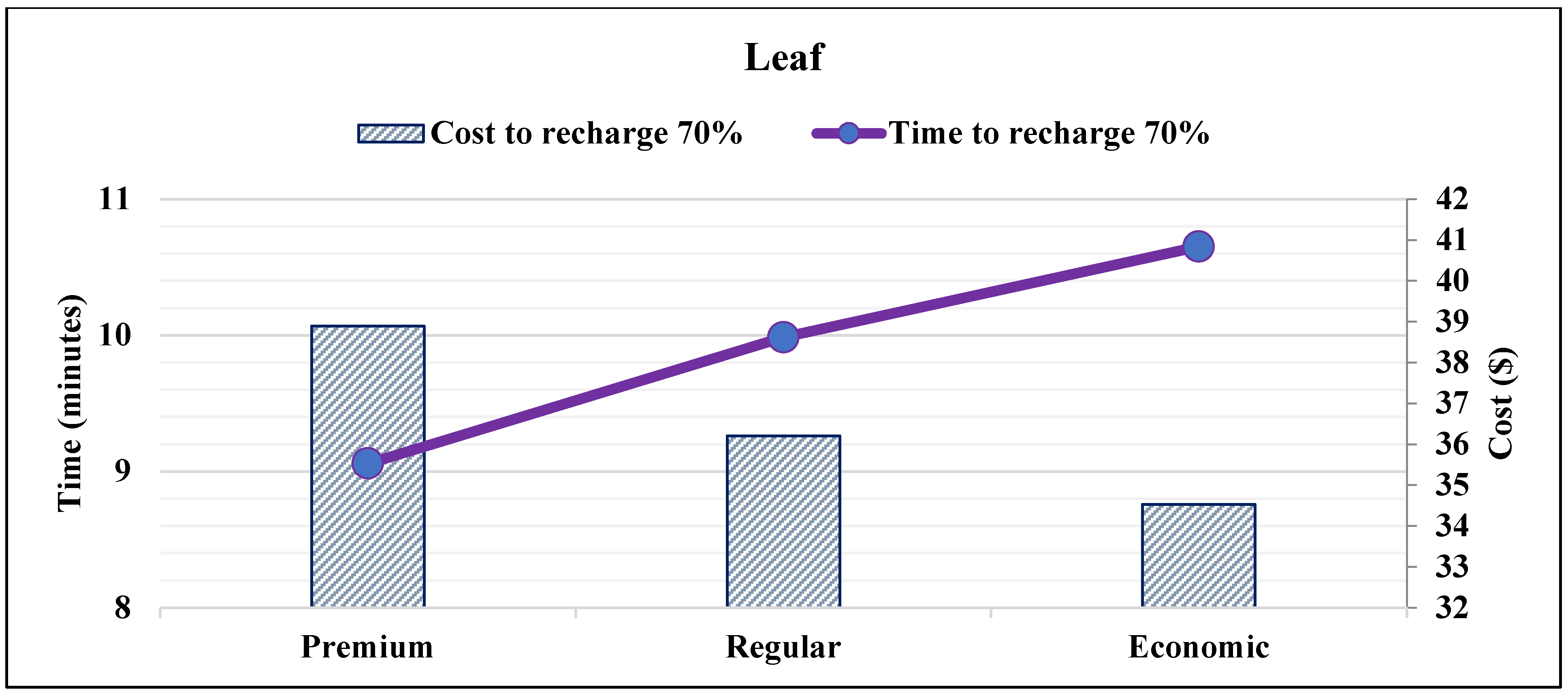
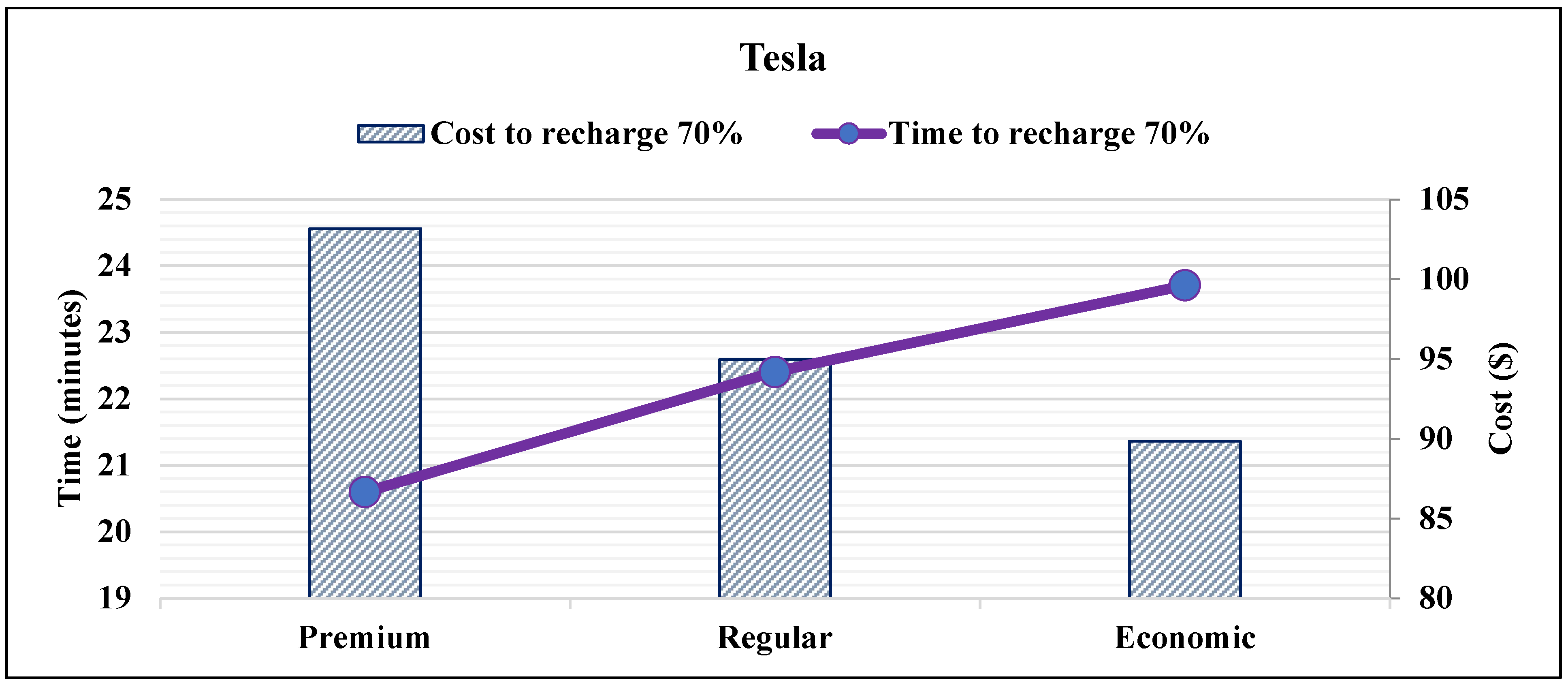
6.2. Effect of Charging Power on Charging Duration and Charging Cost
- Given the battery safety considerations, the maximum state-of-charge using FCS is , as in [47].
- Given and , the maximum charging duration is determined as in Equation (37).
- The Nissan Leaf is utilized to represent a vehicle with a small battery capacity, .
- The Chevy Bolt is utilized to represent a vehicle with a small battery capacity, .
- The Tesla Model S is utilized to represent a vehicle with a small battery capacity, .
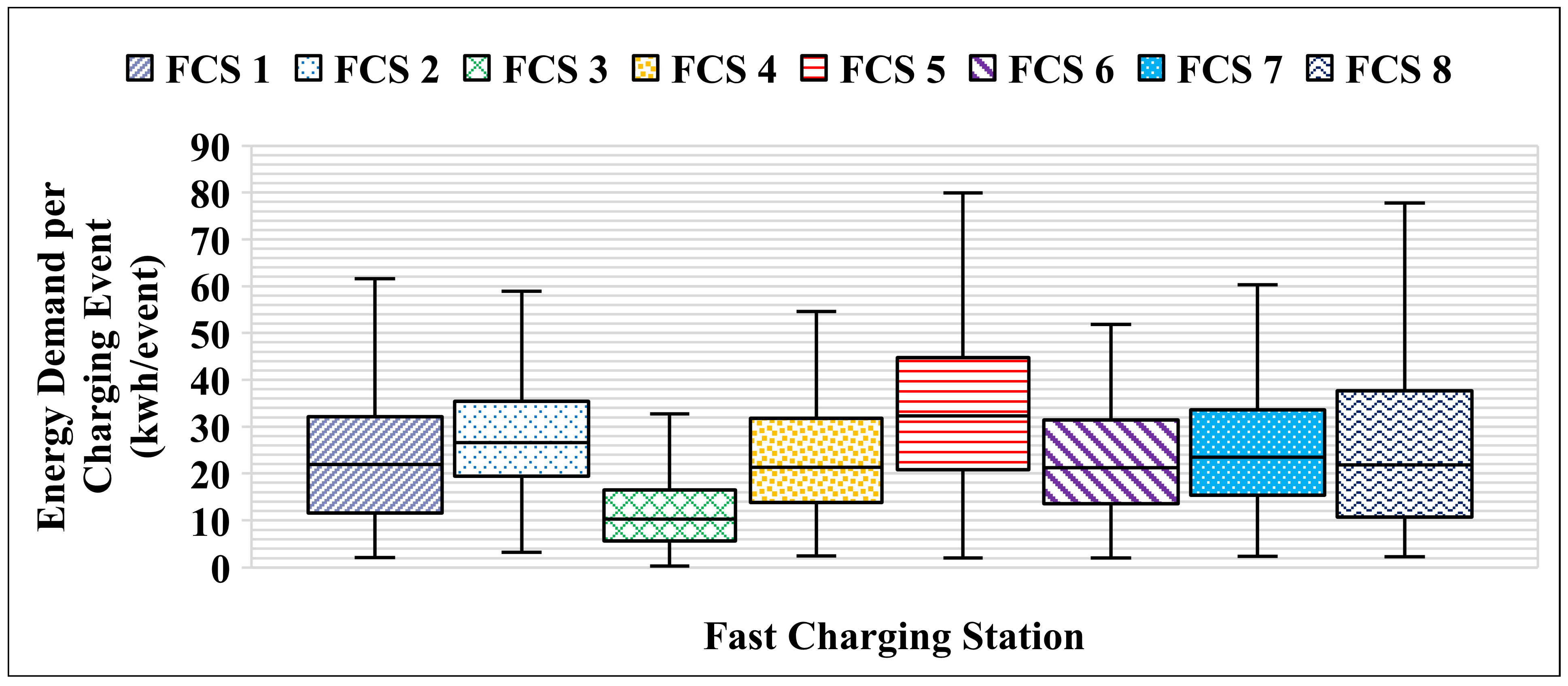
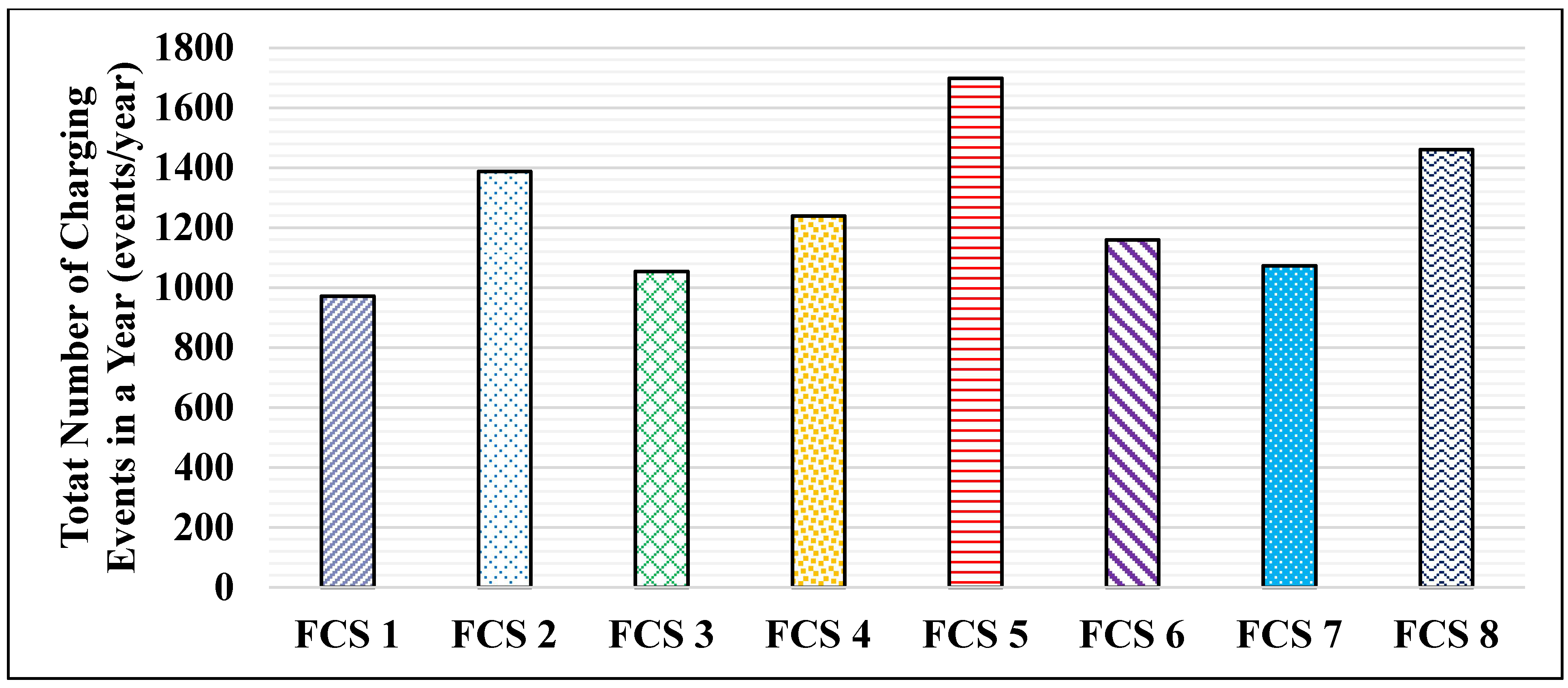
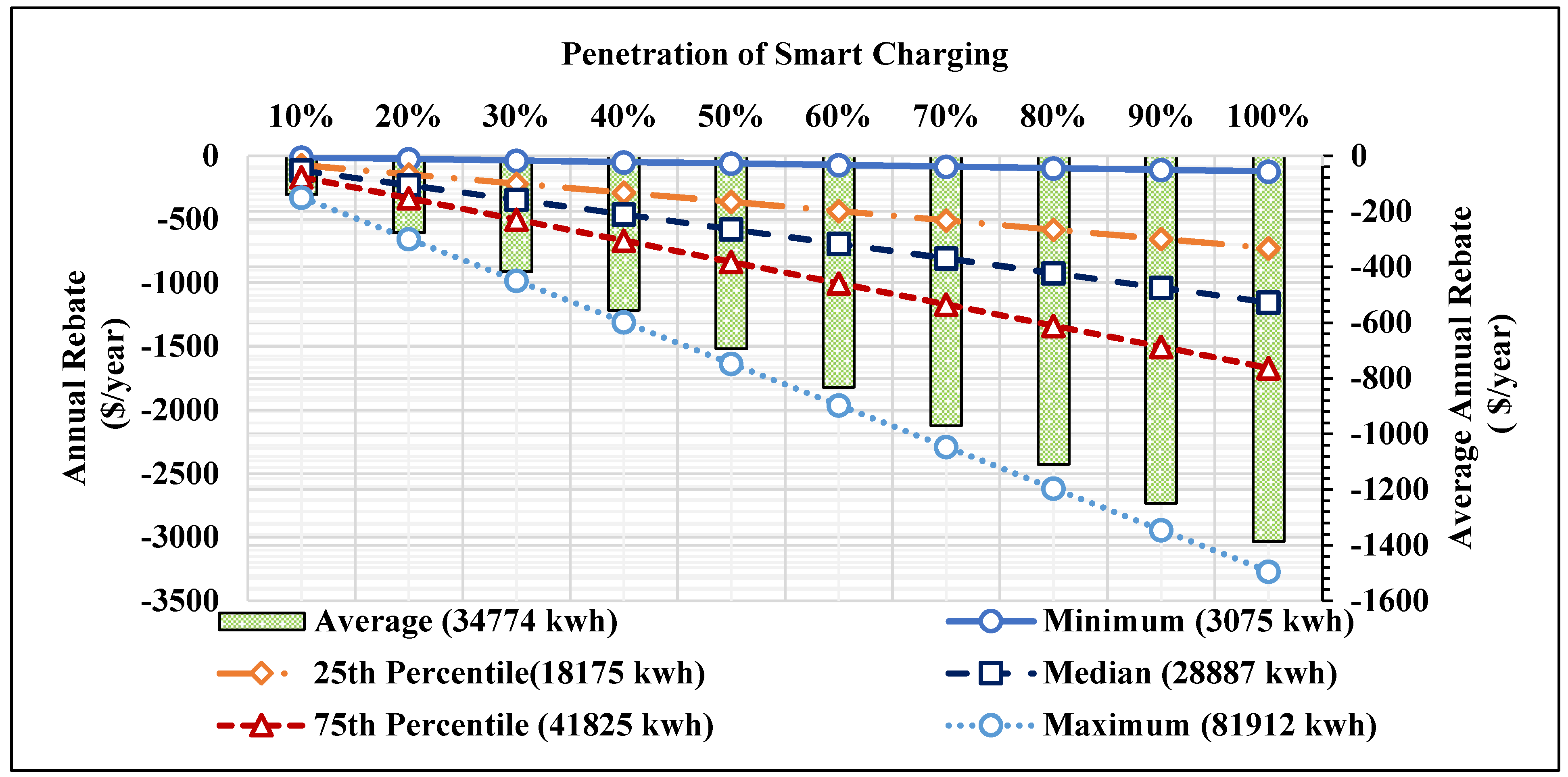
6.3. Descriptive Statistics of Annual Data for Multiple FCSs
6.4. Average Annual Energy Required from FCS
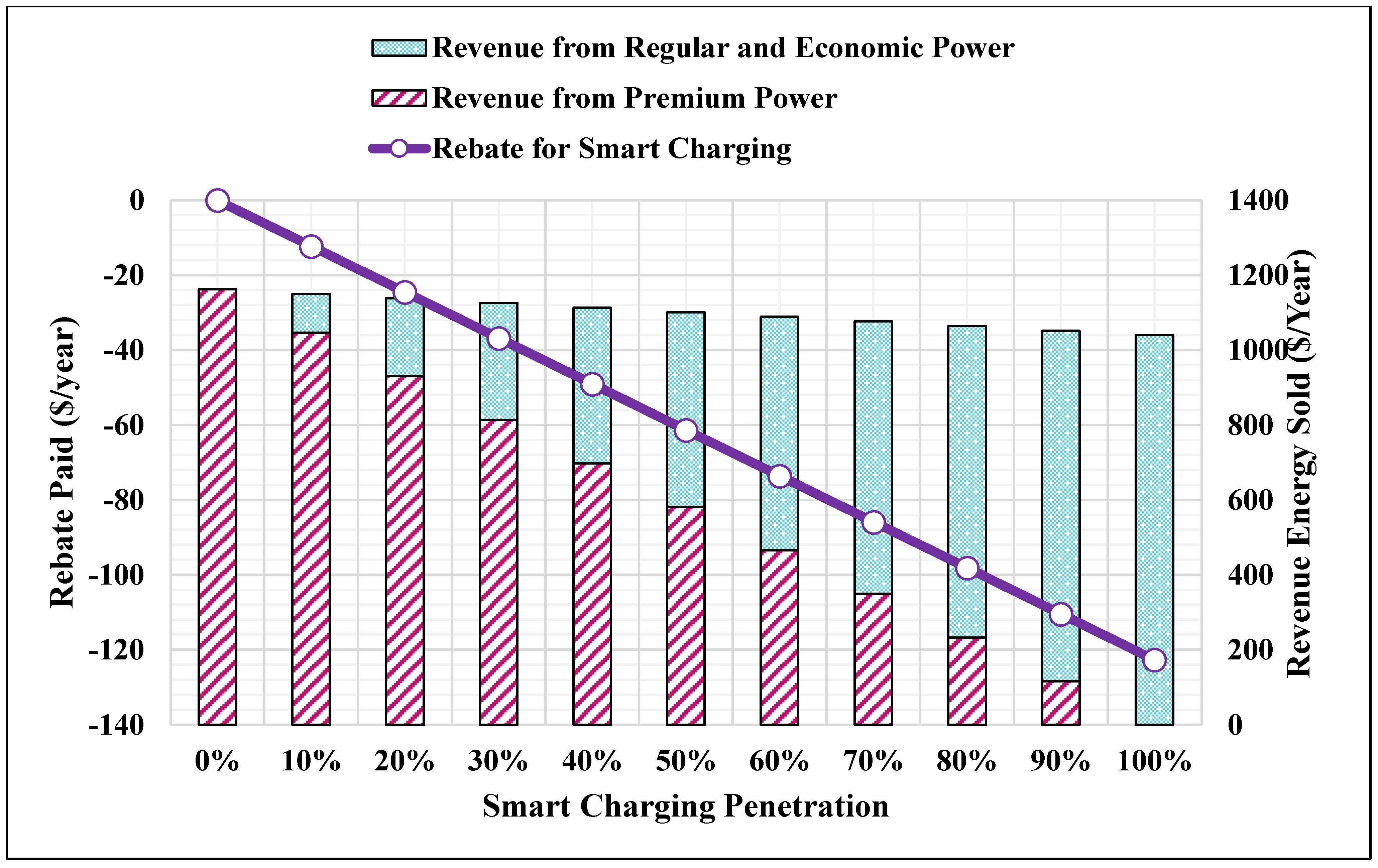
6.5. Fast Charging Station Annual Revenue and Rebate
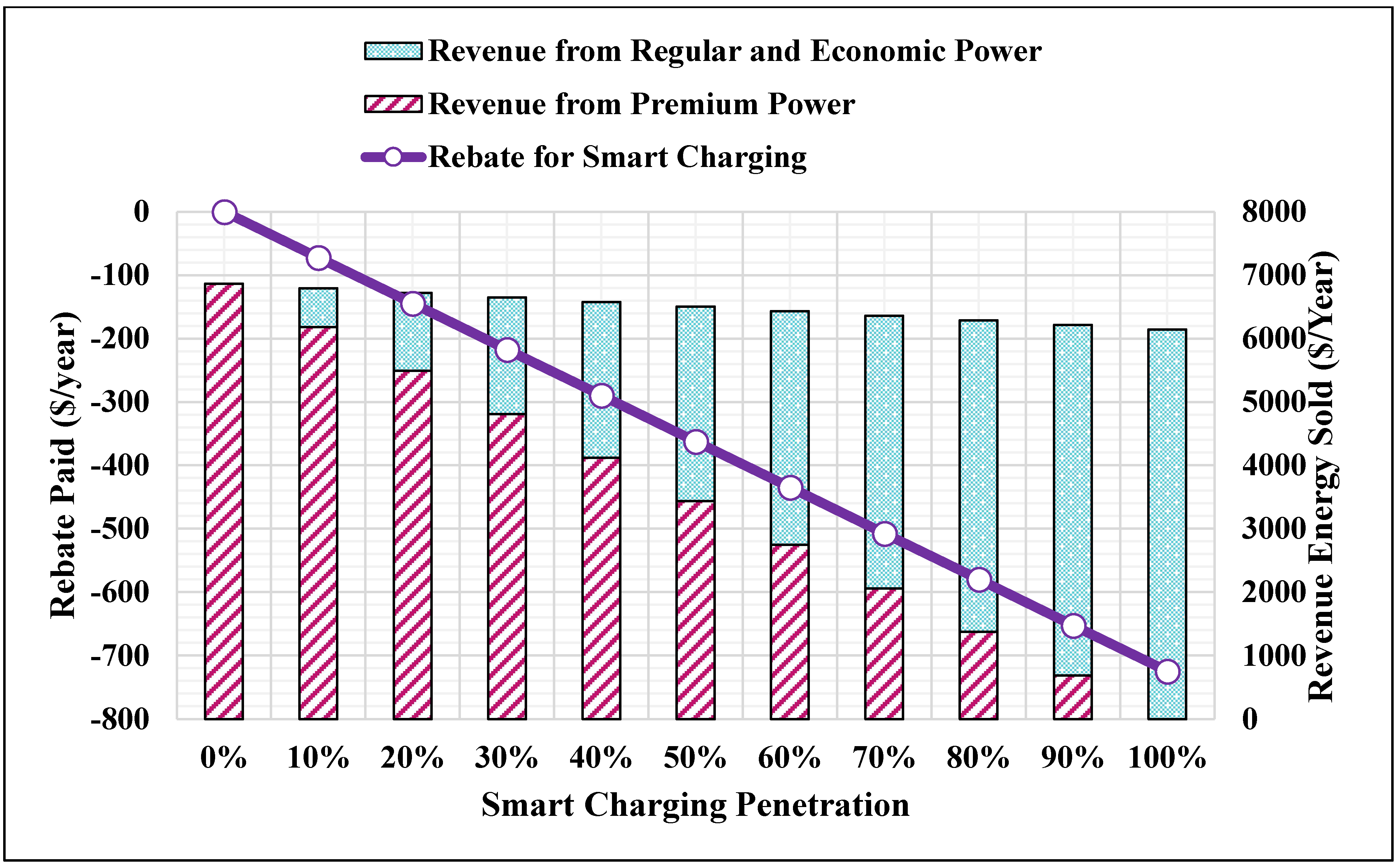
- The minimum annual charging energy (Figure 9) obtained as in (54);
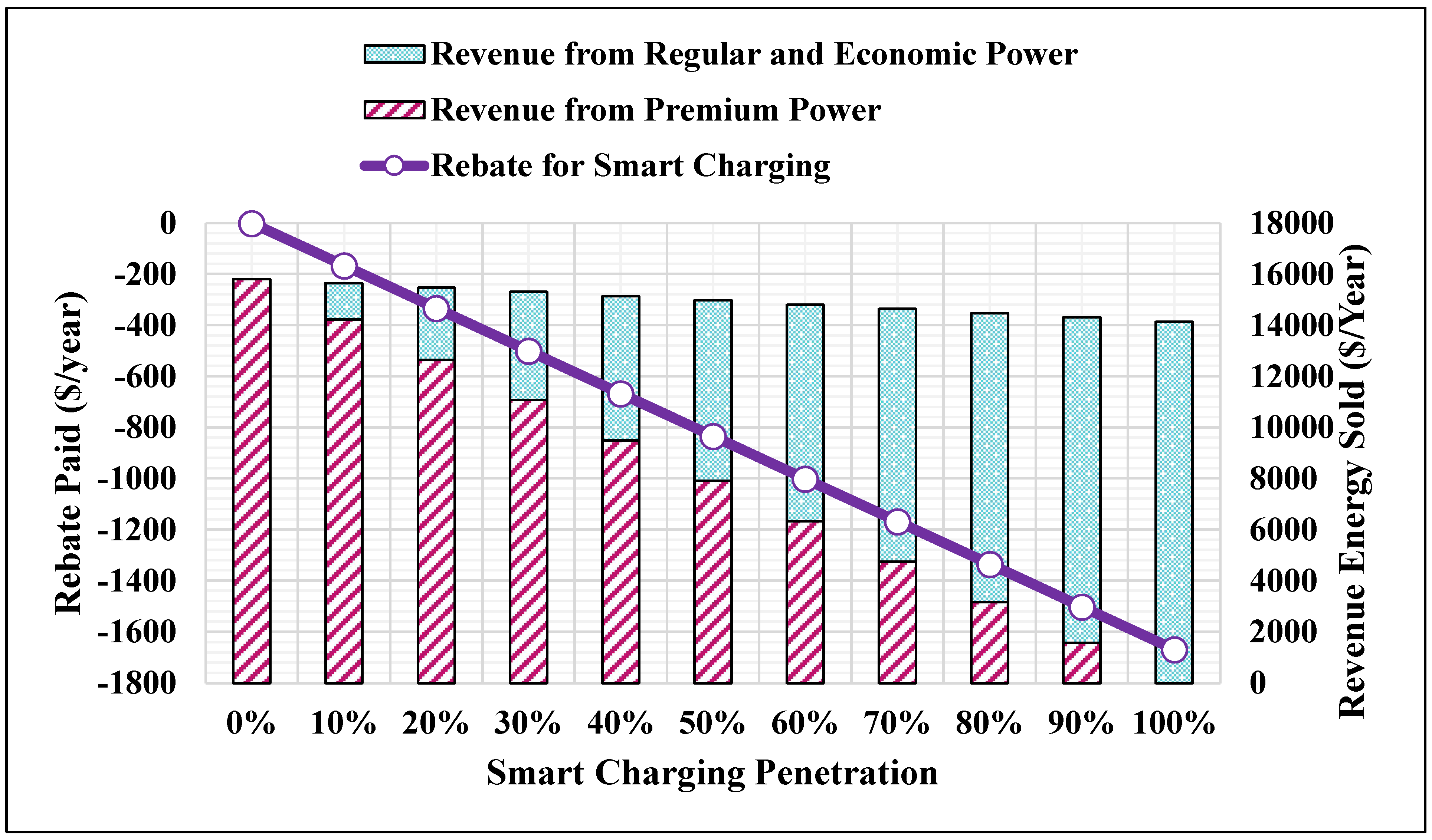
- The 25th percentile annual charging energy (Figure 10) obtained as in (50);
- The 50th percentile annual charging energy (Figure 11) obtained as in (51);
- The 75th percentile annual charging energy (Figure 12) obtained as in (52);
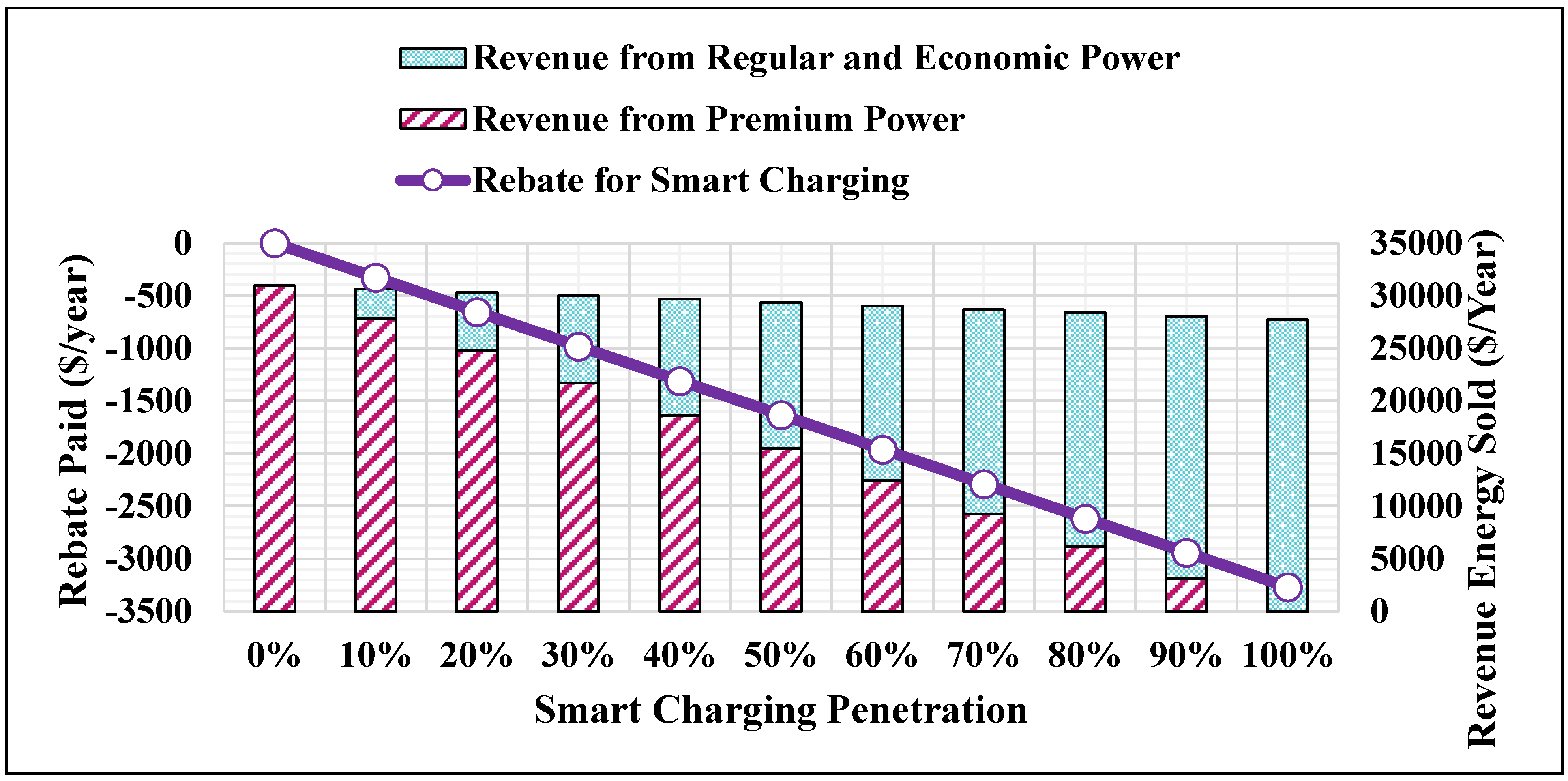
- The maximum charging energy (Figure 13) obtained as in (53).
- Smart charging penetration is
- The costs of premium, regular, and economic charging powers as shown in Figure 1 are , , and ;
- The uniform random number determines the share of regular and economic power for each penetration level;
- The optimal factors for the premium , regular , and economic power are 1, 0.925 and 0.875.
- the average minimum annual charging energy required from the FCS is ,
- the annual rebate and revenue are calculated as in Equations (55) and (65),
- the revenue of the smart charging is zero because , as well as the rebate ,
- thus, the revenue is calculated from selling the energy from using the premium power only, ,
- the ratio of the revenue to the rebate of using the smart charging is 9 and obtained by averaging optimal factors, and , and substituting the average into Equations (95) or (98).
- The annual revenue changes slightly as the smart charging penetration levels increases. This means that as the revenue of using the premium power decreases; it is compensated by using the regular and economic charging powers. Therefore, the proposed smart charging method preserves the annual revenue.
- The rebate is increased as the smart charging penetration levels is increased (i.e., increased considering the same in Figure 9).
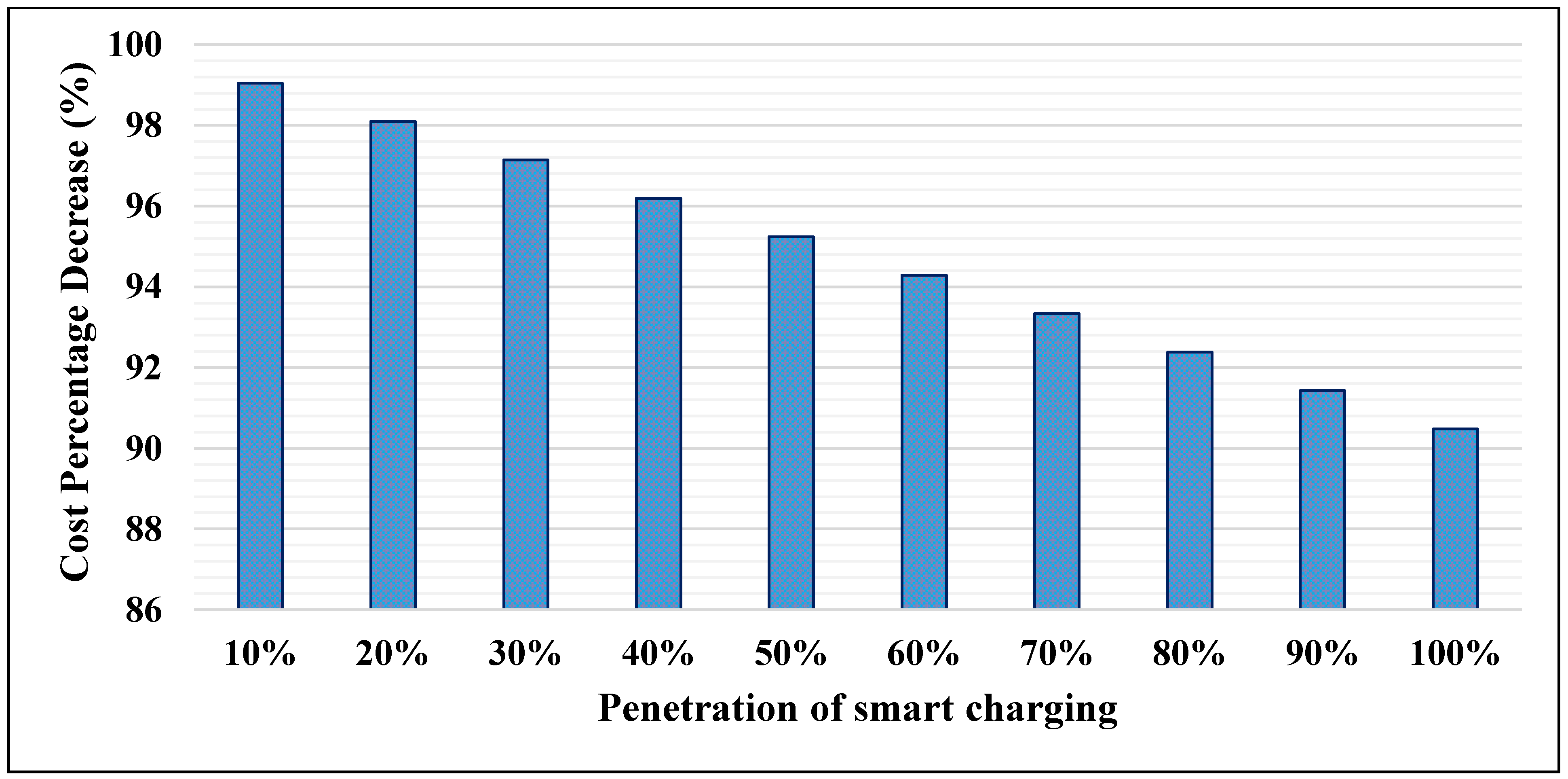
6.6. Cost Comparison of the Proposed Smart Charging Method and DSTATCOM
7. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| The total annual equivalent cost, | |
| The annual equivalent cost of capital invested, | |
| The annual equivalent cost of maintenance, | |
| The first cost of installed flicker mitigation device, | |
| The estimated salvage value at the end of the device useful life, | |
| The capital recovery factor | |
| The single-payment discount factor | |
| The fixed charge rate, % | |
| The useful life in years (flicker mitigation device lifetime), years | |
| The cost per unit of installed flicker mitigation device, | |
| The salvage value per kvar at the end of , | |
| The operating range of the flicker mitigation device, | |
| The per unit cost of the unified power quality conditioner, | |
| The per unit cost of the distribution static compensator, | |
| The per unit cost of the thyristor switched capacitor, | |
| The per unit cost of the fixed capacitors/thyristor controlled reactors, | |
| The per unit cost of the dynamic voltage restorer, | |
| The per unit cost of the fixed series capacitor, | |
| The operating range of the unified power quality conditioner, | |
| The operating range of the distribution static compensator, | |
| The operating range of the thyristor switched capacitor, | |
| The operating range of the fixed capacitors/thyristor controlled reactors, | |
| The operating range of the dynamic voltage restorer, | |
| The operating range of the fixed series capacitor, | |
| The maintenance cost in percent of the first cost, (%) | |
| Number of electric vehicles in the system | |
| The maintenance cost of the unified power quality conditioner in % of first cost | |
| The maintenance cost of the distribution static compensator in % of first cost | |
| The maintenance cost of the thyristor switched capacitor in % of first cost | |
| The maintenance cost of the fixed capacitors/thyristor controlled reactors in % of first cost | |
| The maintenance cost of the dynamic voltage restorer in % of first cost | |
| The maintenance cost of the fixed series capacitor in % of first cost | |
| Efficiency of the fast charger, % | |
| Factor to convert hour into minutes | |
| Maximum charging power per port, kw | |
| Premium charging power, kw | |
| Regular charging power, kw | |
| Economic charging power, kw | |
| Factor to set the upper limits of premium charging power, % | |
| Factor to set the lower limits of premium charging power, % | |
| Factor to set the upper limits of regular charging power, % | |
| Factor to set the lower limits of regular charging power, % | |
| Factor to set the upper limits of economic charging power, % | |
| Factor to set the lower limits of economic charging power, % | |
| The time it takes to charge 1 kwh by premium power, | |
| The time it takes to charge 1 kwh by regular power, | |
| The time it takes to charge 1 kwh by economic power, | |
| The per unit cost of the premium charging, | |
| The per unit cost of the regular charging, | |
| The per unit cost of the economic charging, | |
| The per hour PBEV fast charging cost, | |
| The per minutes PBEV fast charging cost, | |
| The maximum cost of charging a BPEV from FCS, | |
| The per unit cost of fast charging, | |
| The maximum allowable SOC for any BPEV, | |
| The minimum allowable SOC for any BPEV, | |
| A percent to determine the maximum SOC, | |
| A percent to determine the minimum SOC, | |
| Capacity of a PBEV battery, | |
| Capacity of a small battery, | |
| Capacity of a medium battery, | |
| Capacity of a large battery, | |
| A set of PEVs with small battery capacity uses the FCS | |
| A set of PEVs with medium battery capacity uses the FCS | |
| A set of PEVs with large battery capacity uses the FCS | |
| The maximum time it takes a BPEV to be charged, from its minimum to its maximum state-of-charge, using FCS, | |
| The per unit time it takes to charge a BPEV, from its minimum to its maximum state-of-charge, using FCS, | |
| The 25th percentile of charging energy per charging event of FCS in a year, | |
| The 50th percentile of charging energy per charging event of FCS in a year, | |
| The 75th percentile of charging energy per charging event of FCS in a year, | |
| The maximum of charging energy per charging event of FCS in a year, | |
| The minimum of charging energy per charging event of FCS in a year, | |
| The average value of the first quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the second quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the third quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the maximum of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the minimum of charging energy per charging event in a year, for number of FCSs, | |
| The maximum cost of charging a BPEV from FCS, | |
| The per unit cost of fast charging, | |
| The maximum allowable SOC for any BPEV, | |
| The minimum allowable SOC for any BPEV, | |
| A percent to determine the maximum SOC, | |
| A percent to determine the minimum SOC, | |
| Capacity of a PBEV battery, | |
| Capacity of a small battery, | |
| Capacity of a medium battery, | |
| Capacity of a large battery, | |
| A set of PEVs with small battery capacity uses the FCS | |
| A set of PEVs with medium battery capacity uses the FCS | |
| A set of PEVs with large battery capacity uses the FCS | |
| The maximum time it takes a BPEV to be charged, from its minimum to its maximum state-of-charge, using FCS, | |
| The per unit time it takes to charge a BPEV, from its minimum to its maximum state-of-charge, using FCS, | |
| The 25th percentile of charging energy per charging event of FCS in a year, | |
| The 50th percentile of charging energy per charging event of FCS in a year, | |
| The 75th percentile of charging energy per charging event of FCS in a year, | |
| The maximum of charging energy per charging event of FCS in a year, | |
| The minimum of charging energy per charging event of FCS in a year, | |
| The average value of the first quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the second quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the third quartile of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the maximum of charging energy per charging event in a year, for number of FCSs, | |
| The average value of the minimum of charging energy per charging event in a year, for number of FCSs, | |
| The maximum cost of charging a BPEV from FCS, | |
| The per unit cost of fast charging, | |
| The average number of annual charging events in number of FCSs, | |
| The number of annual charging events in FCS | |
| The estimated 25th percentile value of annual charging energy for a FCS, | |
| The estimated 50th percentile value of annual charging energy for a FCS, | |
| The estimated 75th percentile value of annual charging energy for a FCS, | |
| The estimated maximum value of annual charging energy for a FCS, | |
| The estimated minimum value of annual charging energy for a FCS, | |
| The 25th percentile annual rebate paid to customers for using smart charging power, | |
| The median annual rebate paid to customers for using smart charging power, | |
| The 75th percentile annual rebate paid to customers for using smart charging power, | |
| The maximum annual rebate paid to customers for using smart charging power, | |
| The minimum annual rebate paid to customers for using smart charging power, | |
| A uniform random number | |
| Share of PBEV that utilizes smart charging power at an FCS, | |
| The 25th percentile annual revenue from customers for using smart charging power, | |
| The median annual revenue from customers for using smart charging power, | |
| The 75th percentile annual revenue from customers for using smart charging power, | |
| The maximum annual revenue from customers for using smart charging power, | |
| The minimum annual revenue from customers for using smart charging power, | |
| Revenue from a customer when the regular charging power is utilized, | |
| Rebate paid to a customer when the regular charging power is utilized, | |
| Optimal factor to set the limit of premium charging power, | |
| Optimal factor to set the limit of regular charging power, | |
| Revenue from a customer when the economic charging power is utilized, | |
| Rebate paid to a customer when the economic charging power is utilized, |
References
- McKerracher, C. Electric Vehicle Outlook 2018. Bloomberg New Energy Finance. Available online: https://about.bnef.com/electric-vehicle-outlook/ (accessed on 4 April 2019).
- Sharma, I.; Canizares, C.; Bhattacharya, K. Smart Charging of PEVs Penetrating into Residential Distribution Systems. IEEE Trans. Smart Grid 2014, 5, 1196–1209. [Google Scholar] [CrossRef]
- Humayd, A.S.B.; Bhattacharya, K. Design of Optimal Incentives for Smart Charging Considering Utility-Customer Interactions and Distribution Systems Impact. IEEE Trans. Smart Grid 2017, 10, 1521–1531. [Google Scholar] [CrossRef]
- Moghaddam, Z.; Ahmad, I.; Habibi, D.; Phung, Q.V. Smart Charging Strategy for Electric Vehicle Charging Stations. IEEE Trans. Transp. Electrif. 2018, 4, 13. [Google Scholar] [CrossRef]
- van der Kam, M.; van Sark, W. Smart Charging of Electric Vehicles with Photovoltaic Power and Vehicle-to-Grid Technology in a Microgrid: A Case Study. Appl. Energy 2015, 152, 20–30. [Google Scholar] [CrossRef] [Green Version]
- Bin Humayd, A.S.; Lami, B.; Bhattacharya, K. The Effect of PEV Uncontrolled and Smart Charging on Distribution System Planning. In Proceedings of the IEEE Electrical Power and Energy Conference, Ottawa, ON, Canada, 12–14 October 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Ma, T.-Y.; Xie, S. Optimal Fast Charging Station Locations for Electric Ridesharing with Vehicle-Charging Station Assignment. Transp. Res. Part D Transp. Environ. 2021, 90, 102682. [Google Scholar] [CrossRef]
- Mahfouz, M.M.; Iravani, R. Autonomous Operation of the DC Fast-Charging Station. IEEE Trans. Ind. Electron. 2022, 69, 3787–3797. [Google Scholar] [CrossRef]
- Zhang, W.; Zhao, H.; Song, Z. Integrating Transit Route Network Design and Fast Charging Station Planning for Battery Electric Buses. IEEE Access 2021, 9, 51604–51617. [Google Scholar] [CrossRef]
- Ding, X.; Zhang, W.; Wei, S.; Wang, Z. Optimization of an Energy Storage System for Electric Bus Fast-Charging Station. Energies 2021, 14, 4143. [Google Scholar] [CrossRef]
- Liu, X. Dynamic Response Characteristics of Fast Charging Station-EVs on Interaction of Multiple Vehicles. IEEE Access 2020, 8, 42404–42421. [Google Scholar] [CrossRef]
- Ardeshiri, A.; Rashidi, T.H. Willingness to Pay for Fast Charging Station for Electric Vehicles with Limited Market Penetration Making. Energy Policy 2020, 147, 111822. [Google Scholar] [CrossRef]
- Elma, O. A Dynamic Charging Strategy with Hybrid Fast Charging Station for Electric Vehicles. Energy 2020, 202, 117680. [Google Scholar] [CrossRef]
- Sun, B.; Dragičević, T.; Freijedo, F.D.; Vasquez, J.C.; Guerrero, J.M. A Control Algorithm for Electric Vehicle Fast Charging Stations Equipped With Flywheel Energy Storage Systems. IEEE Trans. Power Electron. 2016, 31, 6674–6685. [Google Scholar] [CrossRef] [Green Version]
- Gjelaj, M.; Traholt, C.; Hashemi, S.; Andersen, P.B. Cost-Benefit Analysis of a Novel DC Fast-Charging Station with a Local Battery Storage for EVs. In Proceedings of the 2017 52nd International Universities Power Engineering Conference (UPEC), Heraklion, Greece, 28–31 August 2017; pp. 1–6. [Google Scholar]
- Chen, H.; Hu, Z.; Zhang, H.; Luo, H. Coordinated Charging and Discharging Strategies for Plug-in Electric Bus Fast Charging Station with Energy Storage System. IET Gener. Transm. Distrib. 2018, 12, 2019–2028. [Google Scholar] [CrossRef] [Green Version]
- Deng, J.; Shi, J.; Liu, Y.; Tang, Y. Application of a Hybrid Energy Storage System in the Fast Charging Station of Electric Vehicles. IET Gener. Transm. Distrib. 2016, 10, 1092–1097. [Google Scholar] [CrossRef]
- Malik, F.H.; Lehtonen, M. Analysis of power network loading due to fast charging of Electric Vehicles on highways. In Proceedings of the 2016 Electric Power Quality and Supply Reliability (PQ), Tallinn, Estonia, 29–31 August 2016; pp. 101–106. [Google Scholar] [CrossRef]
- Amanor-Boadu, J.M.; Abouzied, M.A.; Sanchez-Sinencio, E. An Efficient and Fast Li-Ion Battery Charging System Using Energy Harvesting or Conventional Sources. IEEE Trans. Ind. Electron. 2018, 65, 7383–7394. [Google Scholar] [CrossRef]
- Celli, G.; Soma, G.G.; Pilo, F.; Lacu, F.; Mocci, S.; Natale, N. Aggregated Electric Vehicles Load Profiles with Fast Charging Stations. In Proceedings of the 2014 Power Systems Computation Conference, Wrocław, Poland, 18–22 August 2014; pp. 1–7. [Google Scholar] [CrossRef]
- Febriwijaya, Y.H.; Purwadi, A.; Rizqiawan, A.; Heryana, N. A Study on the Impacts of DC Fast Charging Stations on Power Distribution System. In Proceedings of the 2014 International Conference on Electrical Engineering and Computer Science (ICEECS), Kuta, Bali, Indonesia, 24–25 November 2014; pp. 136–140. [Google Scholar] [CrossRef]
- Alshareef, S.M. A Novel Smart Charging Method to Mitigate Voltage Fluctuation at Fast Charging Stations. Energies 2022, 15, 1746. [Google Scholar] [CrossRef]
- IRENA. Innovation Landscape Brief: Electric-Vehicle Smart Charging. 2019. Available online: https://www.irena.org/-/media/Files/IRENA/Agency/Publication/2019/Sep/IRENA_EV_Smart_Charging_2019.pdf?la=en&hash=E77FAB7422226D29931E8469698C709EFC13EDB2 (accessed on 7 June 2020).
- Pinto, R.J.C.; Pombo, J.; Calado, M.R.A.; Mariano, S.J.S. An Electric Vehicle Charging Station: Monitoring and Analysis of Power Quality. In Proceedings of the 2015 9th International Conference on Compatibility and Power Electronics (CPE), Costa da Caparica, Portugal, 24–26 June 2015; pp. 37–42. [Google Scholar] [CrossRef]
- Yunus, K.; Reza, M. Distribution Grid Impact of Plug-In Electric Vehicles Charging at Fast Charging Stations Using Stochastic Charging Model. In Proceedings of the 14th European Conference on Power Electronics and Applications, Birmingham, UK, 30 August–1 September 2011; p. 11. [Google Scholar]
- Li, Q.; Tao, S.; Xiao, X.; Wen, J. Monitoring and Analysis of Power Quality in Electric Vehicle Charging Stations. In Proceedings of the 2013 1st International Future Energy Electronics Conference (IFEEC), Tainan, Taiwan, 3–6 November 2013; pp. 277–282. [Google Scholar] [CrossRef]
- Alshareef, S.M.; Morsi, W.G. Impact of Fast Charging Stations on the Voltage Flicker in the Electric Power Distribution Systems. In Proceedings of the 2017 IEEE Electrical Power and Energy Conference (EPEC), Saskatoon, SK, Canada, 22–25 October 2017; pp. 1–6. [Google Scholar]
- Marei, M.I.; El-Saadany, E.F.; Salama, M.M. Envelope Tracking Techniques for FlickerMitigation and Voltage Regulation. IEEE Trans. Power Deliv. 2004, 19, 1854–1861. [Google Scholar] [CrossRef]
- Hock, R.T.; De Novaes, Y.R.; Batschauer, A.L. A Voltage Regulator for Power Quality Improvement in Low-Voltage Distribution Grids. IEEE Trans. Power Electron. 2018, 33, 2050–2060. [Google Scholar] [CrossRef]
- Molina, M.G.; Mercado, P.E. Control Design and Simulation of DSTATCOM with Energy Storage for Power Quality Improvements. In Proceedings of the 2006 IEEE/PES Transmission & Distribution Conference and Exposition, Latin America, Caracas, Venezuela, 15–18 August 2006; pp. 1–7. [Google Scholar] [CrossRef]
- Molina, M.G.; Mercado, P.E. Dynamic Modeling and Control Design of DSTATCOM with Ultra-Capacitor Energy Storage for Power Quality Improvements. In Proceedings of the 2008 IEEE/PES Transmission and Distribution Conference and Exposition, Latin America, Bogota, Colombia, 13–15 August 2008; pp. 1–8. [Google Scholar] [CrossRef]
- Electric Power Research Institute (EPRI). Guidebook on Custom Power Devices. 2000. Available online: https://www.epri.com/research/products/000000000001000340 (accessed on 4 January 2020).
- Mathur, R.M.; Varma, R.K. Thyristor-Based FACTS Controllers for Electrical Transmission Systems; Wiley-IEEE Press: New York, NY, USA, 2002. [Google Scholar] [CrossRef]
- Taher, S.A.; Afsari, S.A. Optimal Location and Sizing of UPQC in Distribution Networks Using Differential Evolution Algorithm. Math. Probl. Eng. 2012, 2012, 838629. [Google Scholar] [CrossRef]
- Marjani, S.R.; Talavat, V.; Galvani, S. Optimal Allocation of D-STATCOM and Reconfiguration in Radial Distribution Network using MOPSO Algorithm in TOPSIS Framework. Int. Trans. Electr. Energy Syst. 2019, 29, e2723. [Google Scholar] [CrossRef]
- Saravanan, M.; Slochanal, S.M.R.; Venkatesh, P.; Abraham, J.P.S. Application of Particle Swarm Optimization Technique for Optimal Location of FACTS Devices Considering Cost of Installation and System Loadability. Electr. Power Syst. Res. 2007, 77, 276–283. [Google Scholar] [CrossRef]
- Moghadasi, A.; Sarwat, A.; Guerrero, J. A Comprehensive Review of Low-Voltage-Ride-through Methods for Fixed-Speed Wind Power Generators. Renew. Sustain. Energy Rev. 2016, 55, 823–839. [Google Scholar] [CrossRef] [Green Version]
- Hosseinpour, M.; Yazdian, A.; Hohamadian, M.; Kazempour, J. Desing and Simulation of UPQC to Improve Power Quality and Transfer Wind Energy to Grid. J. Appl. Sci. 2008, 8, 3770–3782. [Google Scholar] [CrossRef]
- Somsai, K.; Kulworawanichpong, T. Cost Estimation for Reactive Power Compensation in Distribution Power System by using D-STATCOM. Available online: http://www.i-asem.org/publication_conf/anbre13/M3D.4.ER655_1199F.pdf (accessed on 7 January 2020).
- Milanovic, J.; Zhang, Y. Global Minimization of Financial Losses Due to Voltage Sags With FACTS Based Devices. IEEE Trans. Power Deliv. 2010, 25, 298–306. [Google Scholar] [CrossRef]
- McGranaghan, M.; Roettger, B. Economic Evaluation of Power Quality. IEEE Power Eng. Rev. 2002, 22, 8–12. [Google Scholar] [CrossRef]
- Arrillaga, J.; Liu, Y.H.; Watson, N.R. Flexible Power Transmission; John Wiley & Sons: Chichester, UK, 2007. [Google Scholar] [CrossRef]
- C. Electrique Inc. 50 kW Fast-Charge Station. The Electric Circuit. Available online: https://lecircuitelectrique.com/en/cost/ (accessed on 20 April 2022).
- evCloud | FleetCarma. Available online: https://www.fleetcarma.com/evCloud (accessed on 19 December 2018).
- Hajimiragha, A.; Canizares, C.A.; Fowler, M.W.; Elkamel, A. Optimal Transition to Plug-in Hybrid Electric Vehicles in Ontario, Canada, Considering the Electricity-Grid Limitations. IEEE Trans. Ind. Electron. 2009, 57, 690–701. [Google Scholar] [CrossRef]
- Bin Humayd, A. Distribution System Planning in Smart Grids to Accommodate Distributed Energy Resources and Electric Vehicles. University of Waterloo, 2017. Available online: https://uwspace.uwaterloo.ca/bitstream/handle/10012/12049/BinHumayd_Abdullah.pdf?sequence=3&isAllowed=y (accessed on 11 November 2019).
- Gjelaj, M.; Traeholt, C.; Hashemi, S.; Andersen, P.B. DC Fast-Charging Stations for EVs Controlled by a Local Battery Storage in Low Voltage Grids. In Proceedings of the 2017 IEEE, Manchester PowerTech, Manchester, UK, 18–22 June 2017; pp. 1–6. [Google Scholar]
- Genovese, A.; Ortenzi, F.; Villante, C. On the Energy Efficiency of Quick DC Vehicle Battery Charging. World Electr. Veh. J. 2015, 7, 570–576. [Google Scholar] [CrossRef] [Green Version]
- Saberbari, E.; Saboori, H. Evaluating PHEV Impacts on Domestic Distribution Grid in Terms of Power Losses and Voltage Drop. In Proceedings of the 2014 19th Conference on Electrical Power Distribution Networks (EPDC), Tehran, Iran, 6–7 May 2014; pp. 52–58. [Google Scholar] [CrossRef]

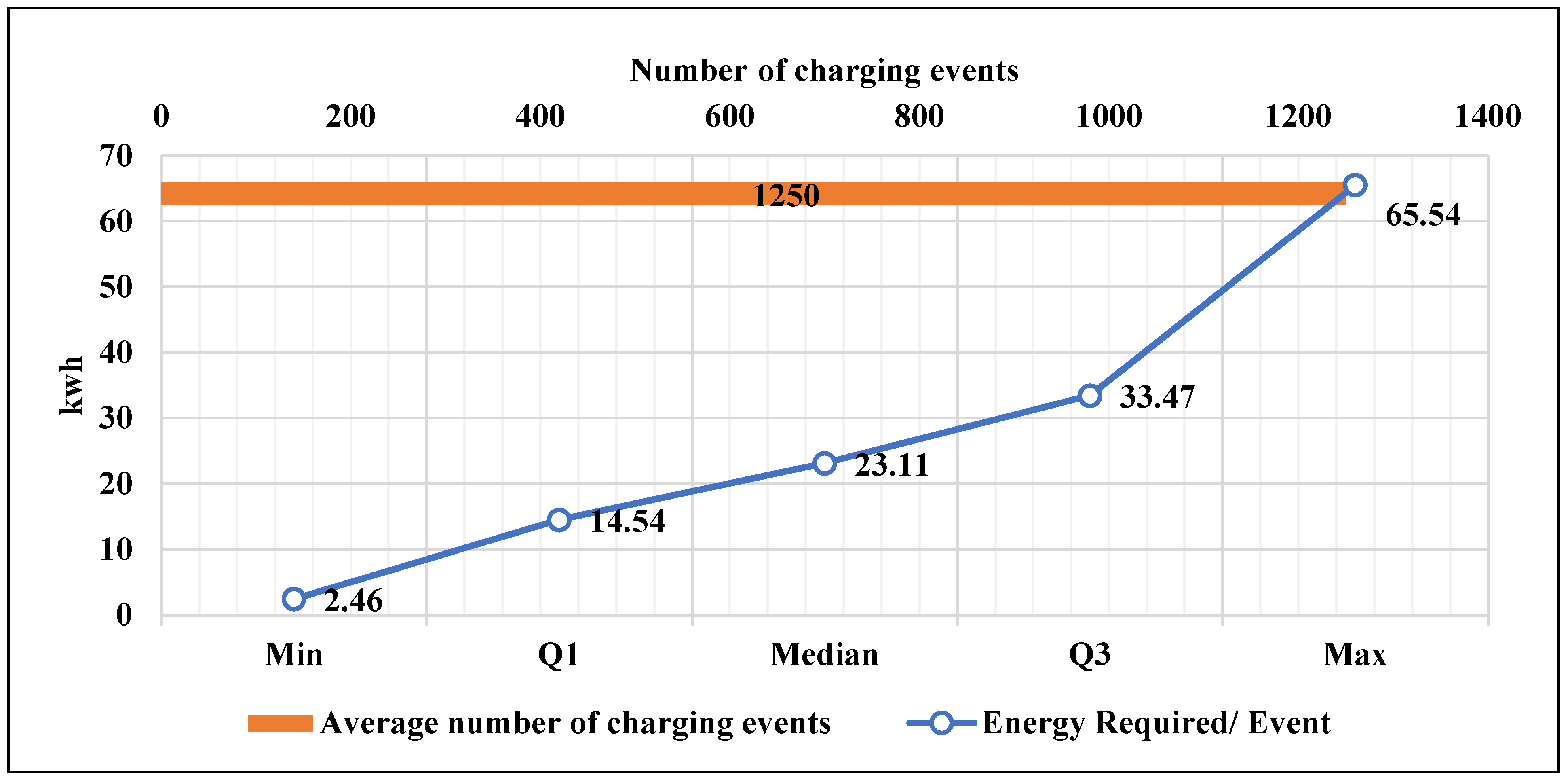

| Mitigation Device | % of the First Cost | The Source |
|---|---|---|
| 10 | [38] | |
| 5 | [39] | |
| 10 | [40] | |
| 10 | [40] | |
| 5 | [41] | |
| 1 | [42] |
| Mitigation Techniques | ||||||
|---|---|---|---|---|---|---|
| Parameters | UPQC | DSTATCOM | TSC | FC-TCR | DVR | FSC |
| Lifetime , (years) | 20 | 20 | 20 | 20 | 20 | 20 |
| Charge rate , (%) | 6 | 6 | 6 | 6 | 6 | 6 |
| Maintenance cost in % of first cost , (%) | 10 | 5 | 10 | 10 | 5 | 1 |
| Reactive power range , (MVAr) | 2 | 2 | 2 | 2 | 2 | 2 |
| The per kVAr cost , ($/kVAr) | ||||||
| Salvage value per kVAr , ($/kVAr) | 0 | 0 | 0 | 0 | 0 | 0 |
| Cost of installation, | ||||||
| Salvage at end of device lifetime, | 0 | 0 | 0 | 0 | 0 | 0 |
| capital recovery factor, | 0.0872 | 0.0872 | 0.0872 | 0.0872 | 0.0872 | 0.0872 |
| Single-payment discount factor, | 0.0272 | 0.0272 | 0.0272 | 0.0272 | 0.0272 | 0.0272 |
| Capital recovery cost, , | 32,728 | 13,874 | 22,114 | 22,114 | 26,735 | 15,691 |
| Annual maintenance cost, , ( | 3272 | 694 | 2211 | 2211 | 1337 | 157 |
| Total annual equivalent cost, | 36,000 | 14,568 | 24,325 | 24,325 | 28,072 | 15,848 |
| Smart Charging Penetration Levels | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | ||
| Annual Energy Required in kwh | 0 | 312.5 | 625 | 937.5 | 1250 | 1562.5 | 1875 | 2187.5 | 2500 | 2812.5 | 3125 | |
| 0 | 1812.5 | 3625 | 5437.5 | 7250 | 9062.5 | 10,875 | 12,687 | 14,500 | 16,312 | 18,125 | ||
| 0 | 2887.5 | 5775 | 8662.5 | 11,550 | 14,437 | 17,325 | 20,212 | 23,100 | 25,987 | 28,875 | ||
| 0 | 4187.5 | 8375 | 12,562 | 16,750 | 20,937 | 25,125 | 29,312 | 33,500 | 37,687 | 41,875 | ||
| 0 | 8187.5 | 16,375 | 24,562 | 32,750 | 40,937 | 49,125 | 57,312 | 65,500 | 73,687 | 81,875 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alshareef, S.M. A Novel Fairness-Based Cost Model for Adopting Smart Charging at Fast Charging Stations. Sustainability 2022, 14, 6450. https://doi.org/10.3390/su14116450
Alshareef SM. A Novel Fairness-Based Cost Model for Adopting Smart Charging at Fast Charging Stations. Sustainability. 2022; 14(11):6450. https://doi.org/10.3390/su14116450
Chicago/Turabian StyleAlshareef, Sami M. 2022. "A Novel Fairness-Based Cost Model for Adopting Smart Charging at Fast Charging Stations" Sustainability 14, no. 11: 6450. https://doi.org/10.3390/su14116450
APA StyleAlshareef, S. M. (2022). A Novel Fairness-Based Cost Model for Adopting Smart Charging at Fast Charging Stations. Sustainability, 14(11), 6450. https://doi.org/10.3390/su14116450






