Numerical Analysis of Enhanced Conductive Deep Borehole Heat Exchangers
Abstract
1. Introduction
2. Materials and Methods
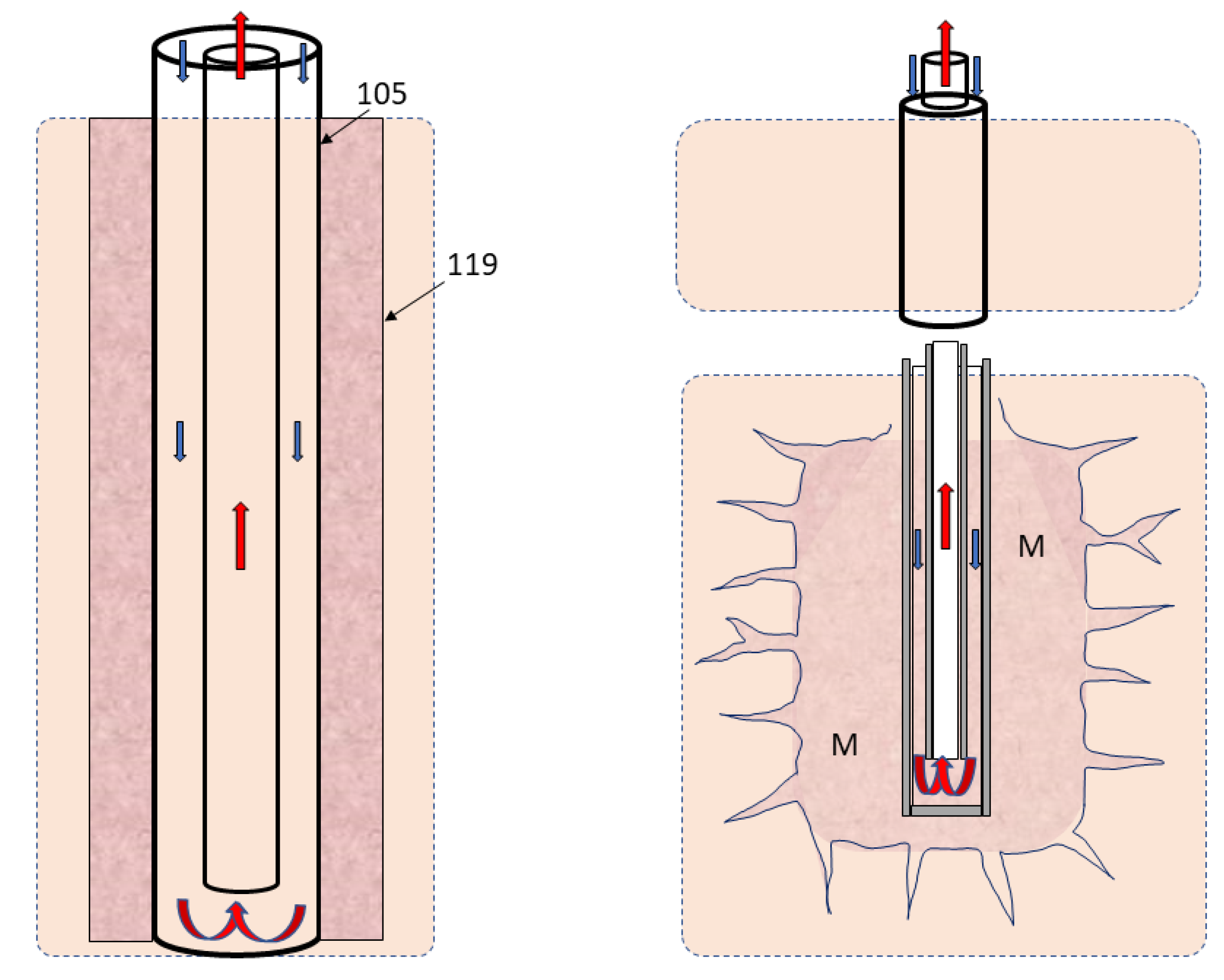
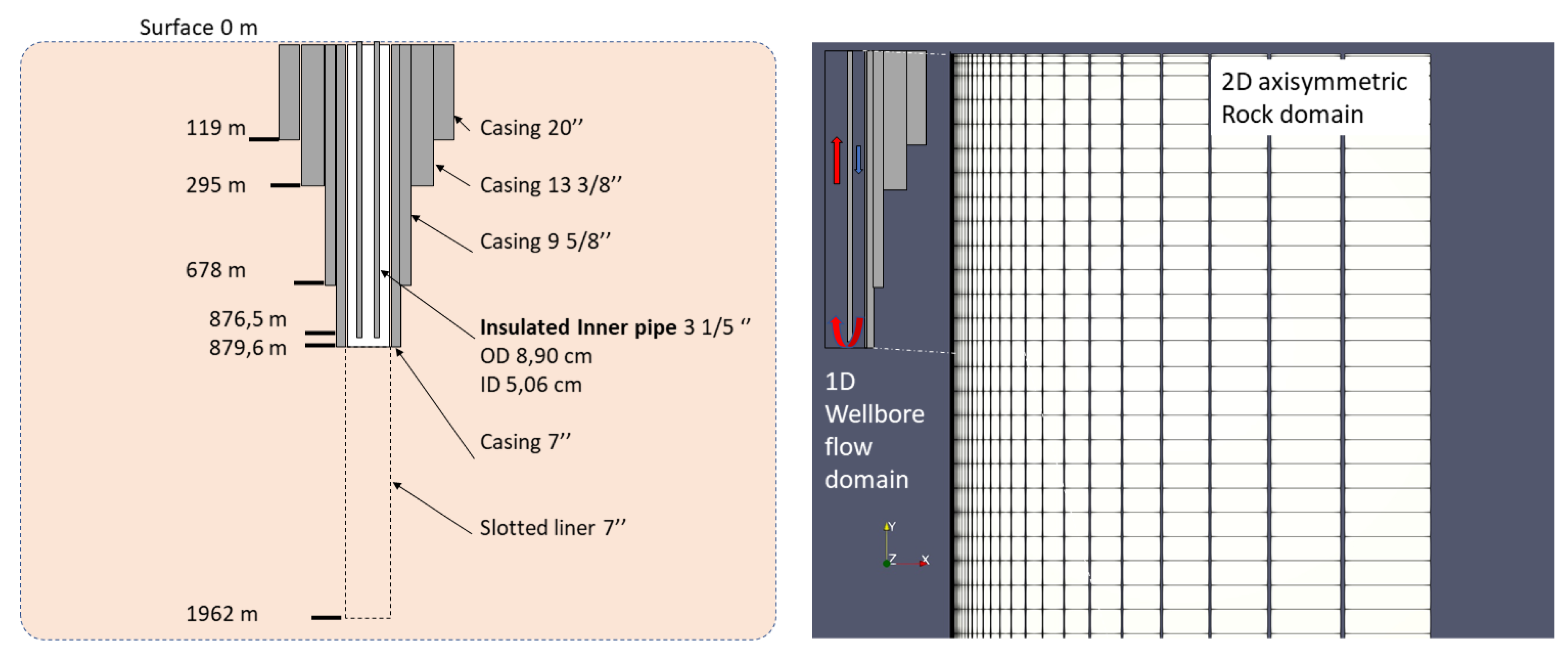
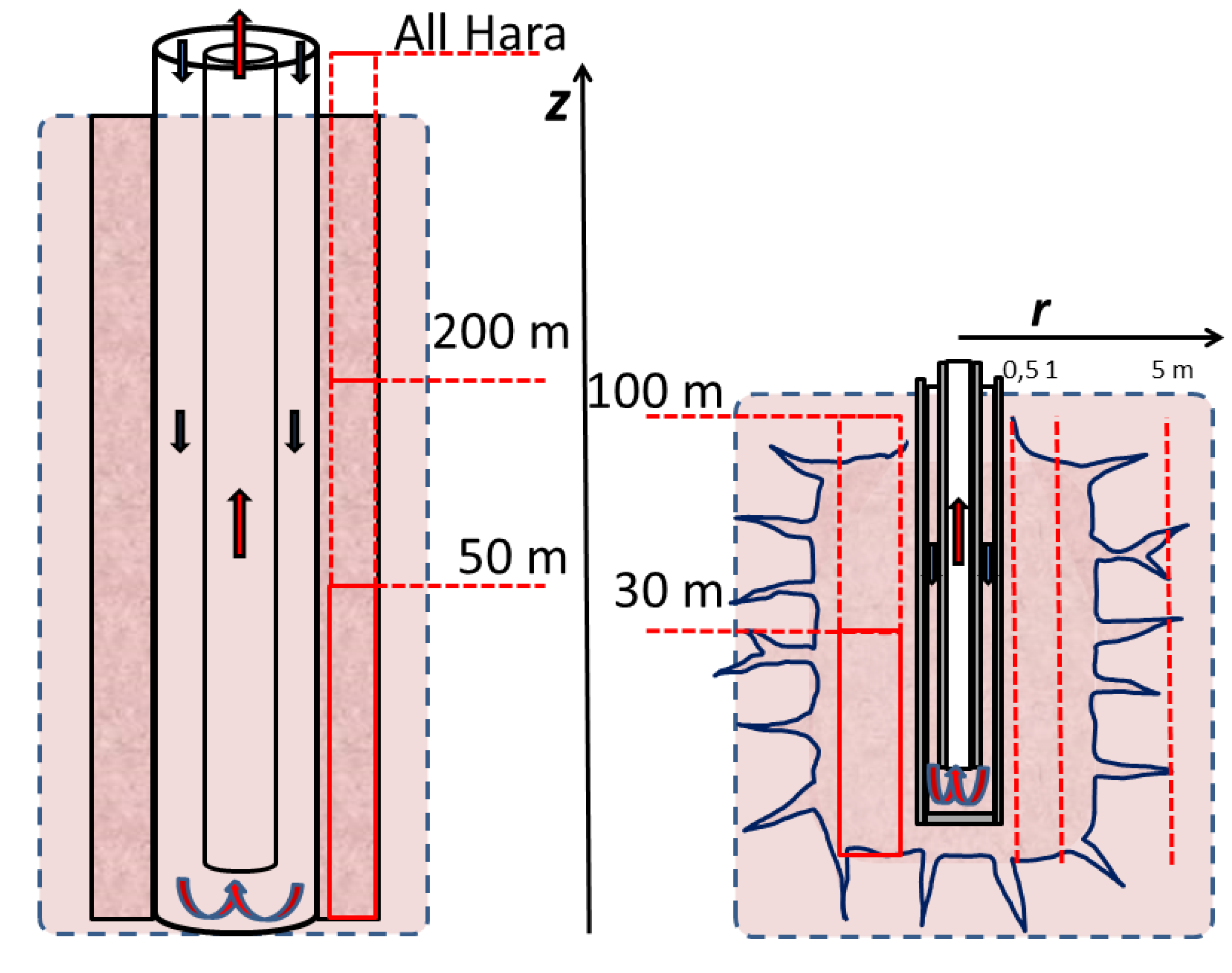
2.1. Model Description
2.2. Mathematical Formulation
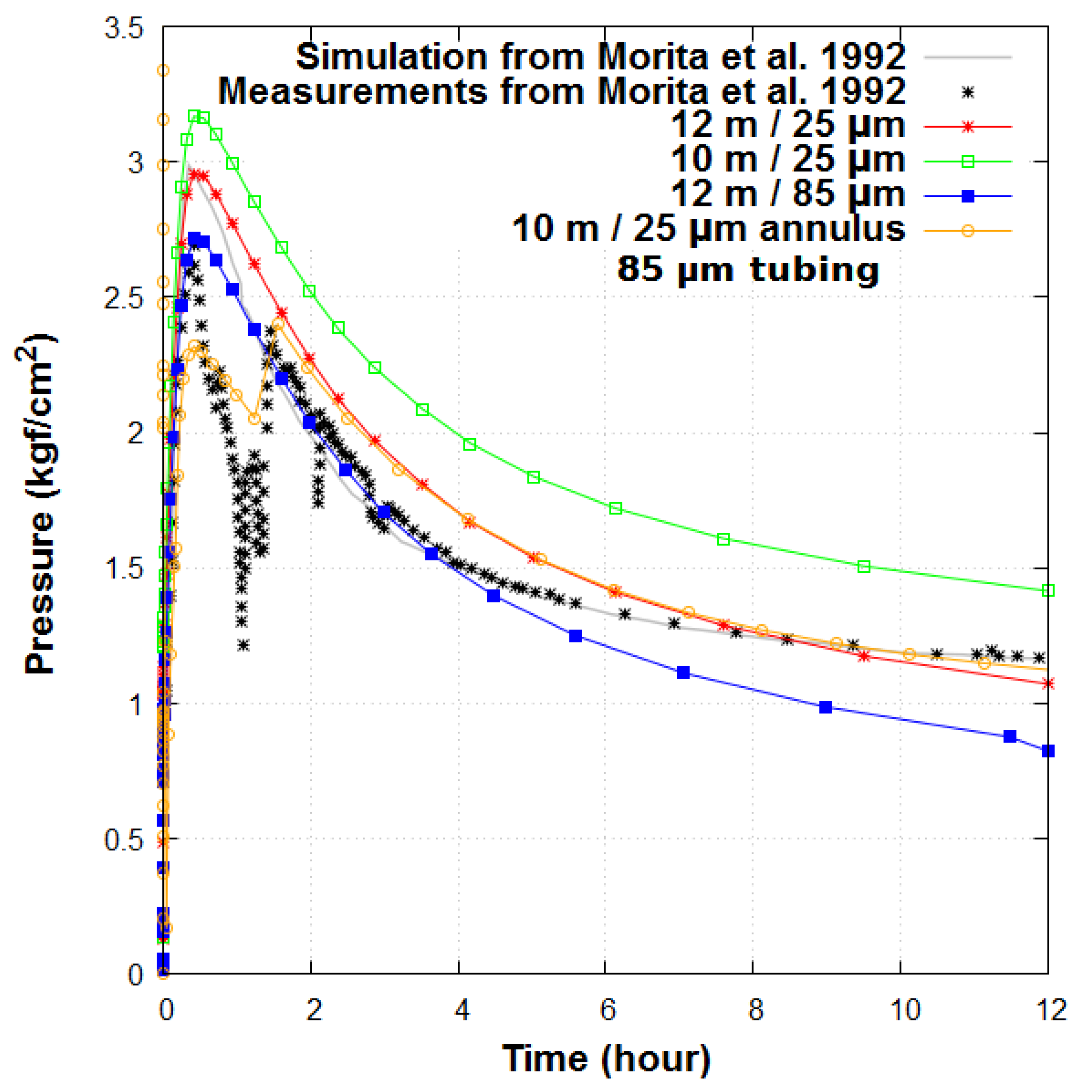
2.3. Model Validation
2.4. Theoretical Analysis of Enhanced DCHEs
2.5. Case Study: Implementation of a DBHE in an EGS System
3. Results
3.1. T2Well DCHE Model
3.1.1. Parameter Analysis
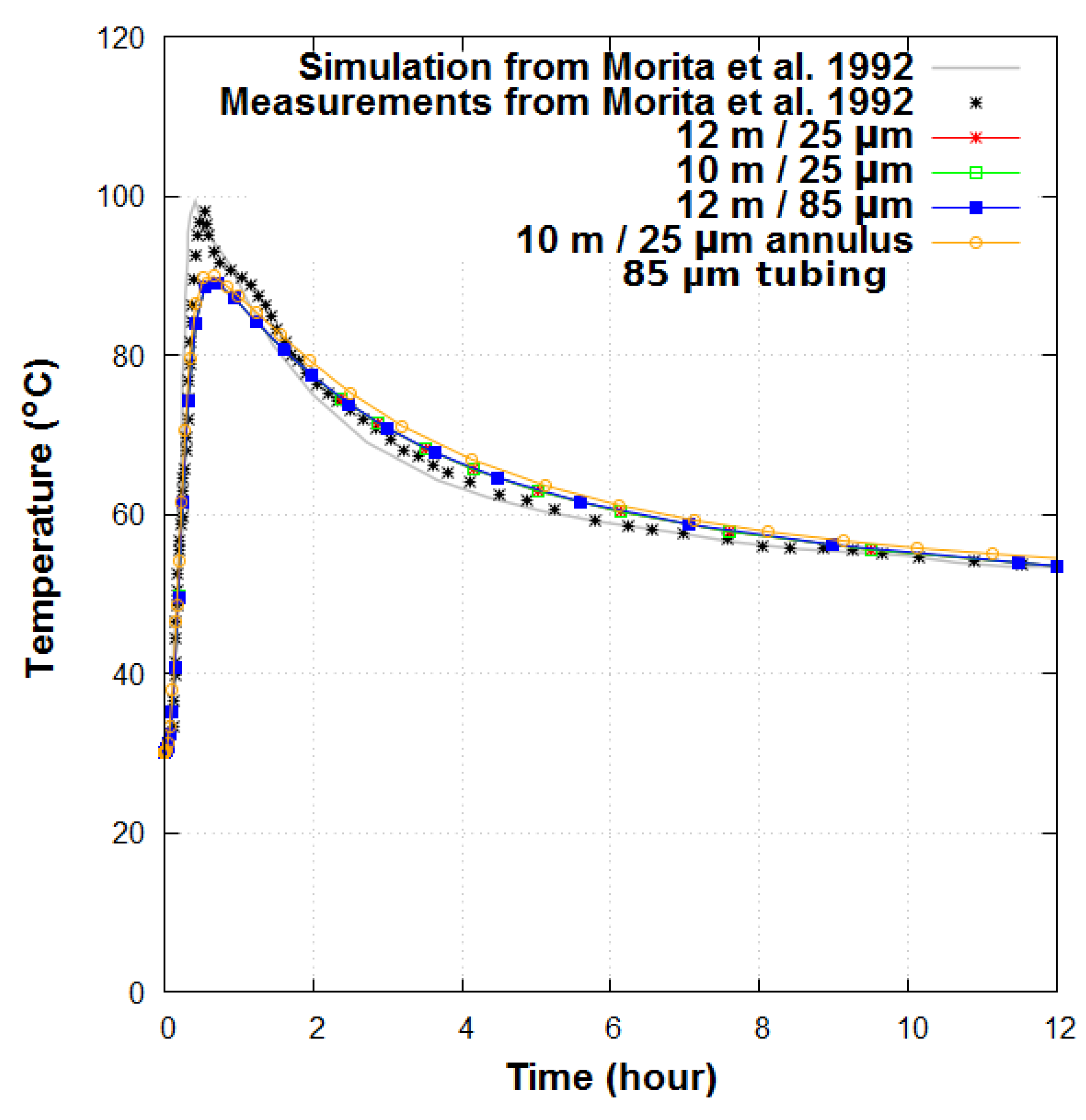
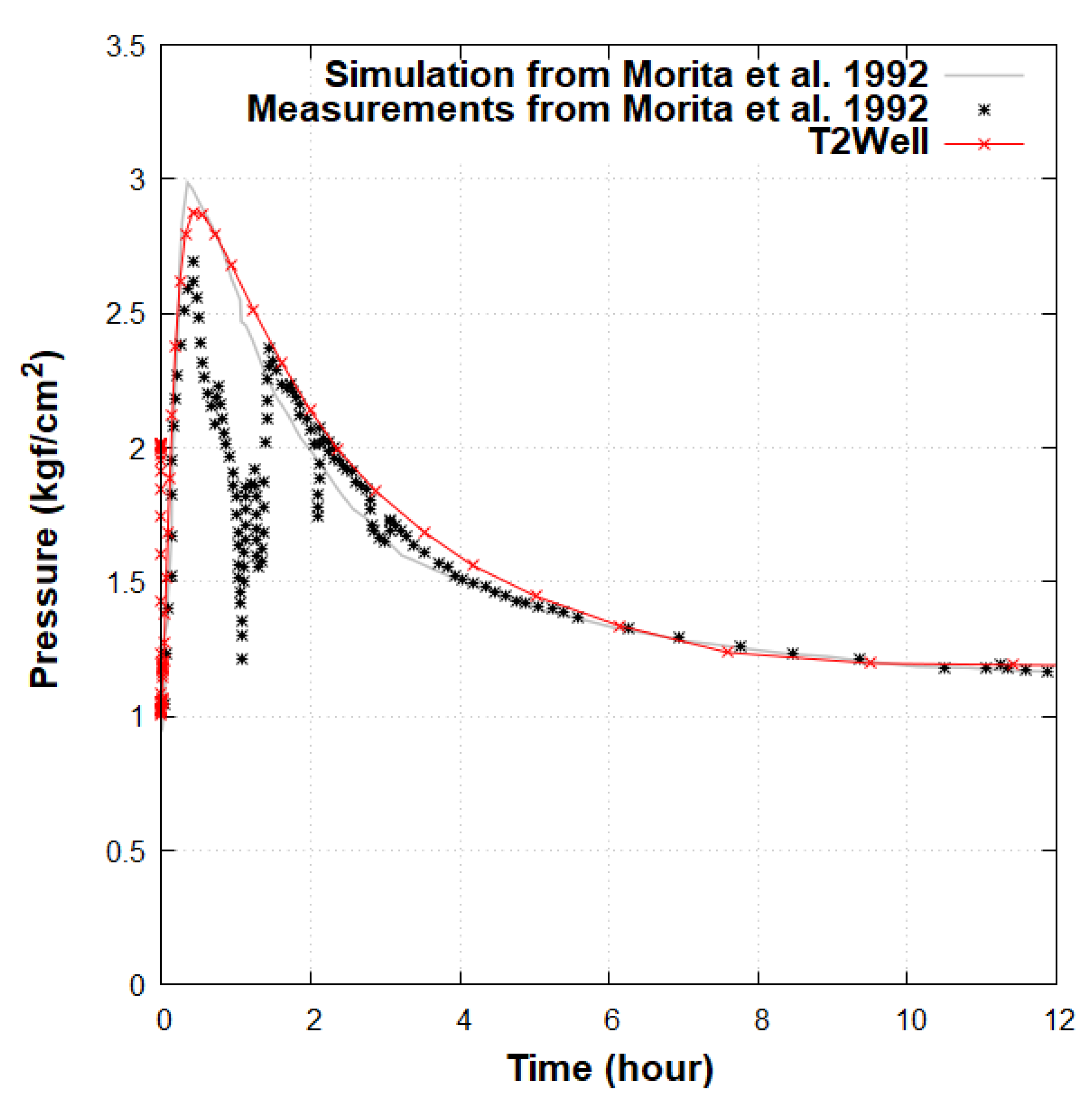
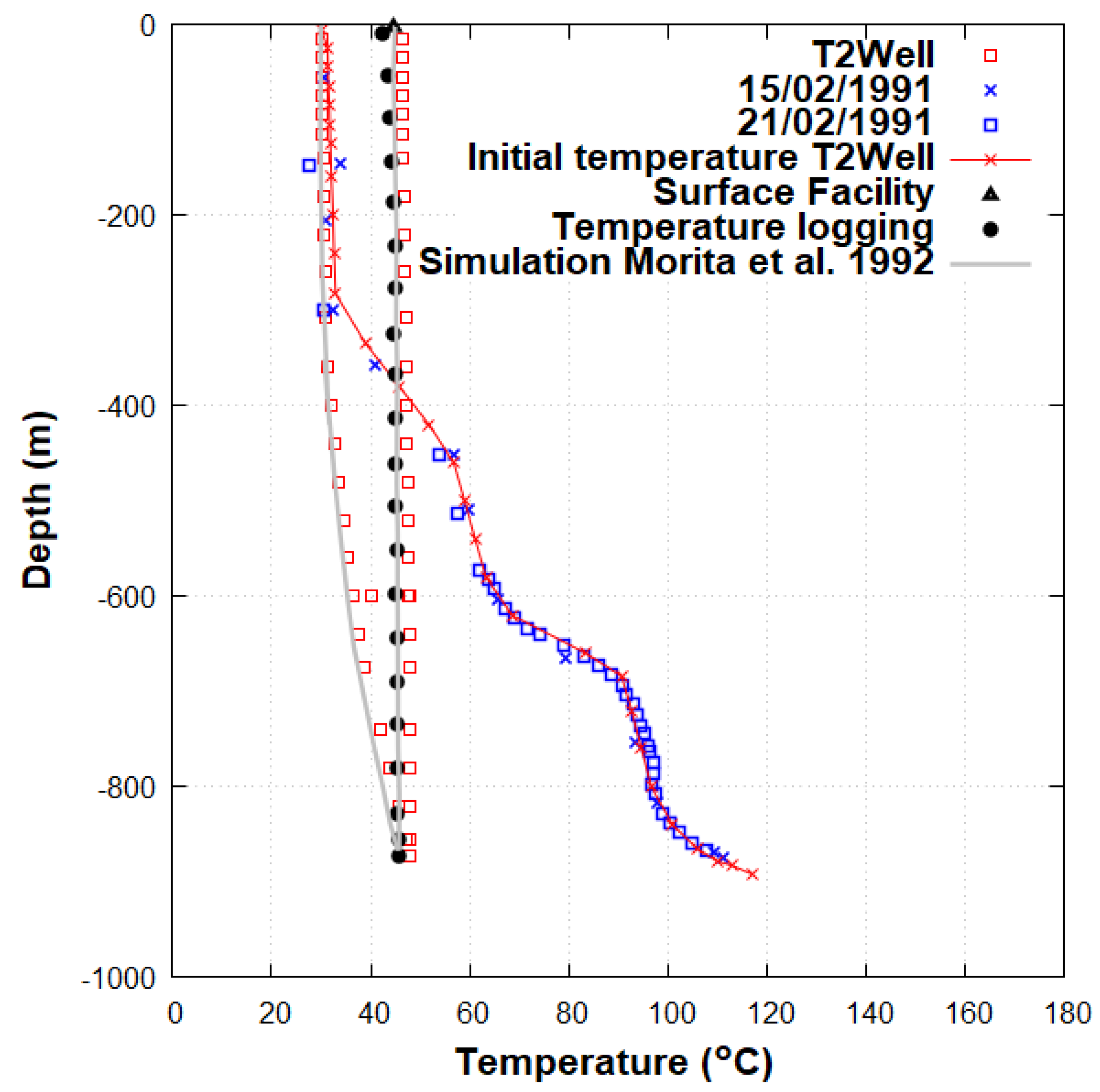
3.1.2. Model Validation
3.2. DBHE Patent Analysis
3.2.1. Thermal Performance Comparison
3.2.2. Energy Production
3.3. Newberry Case Study
3.3.1. Analysis of a DBHE with Graphite
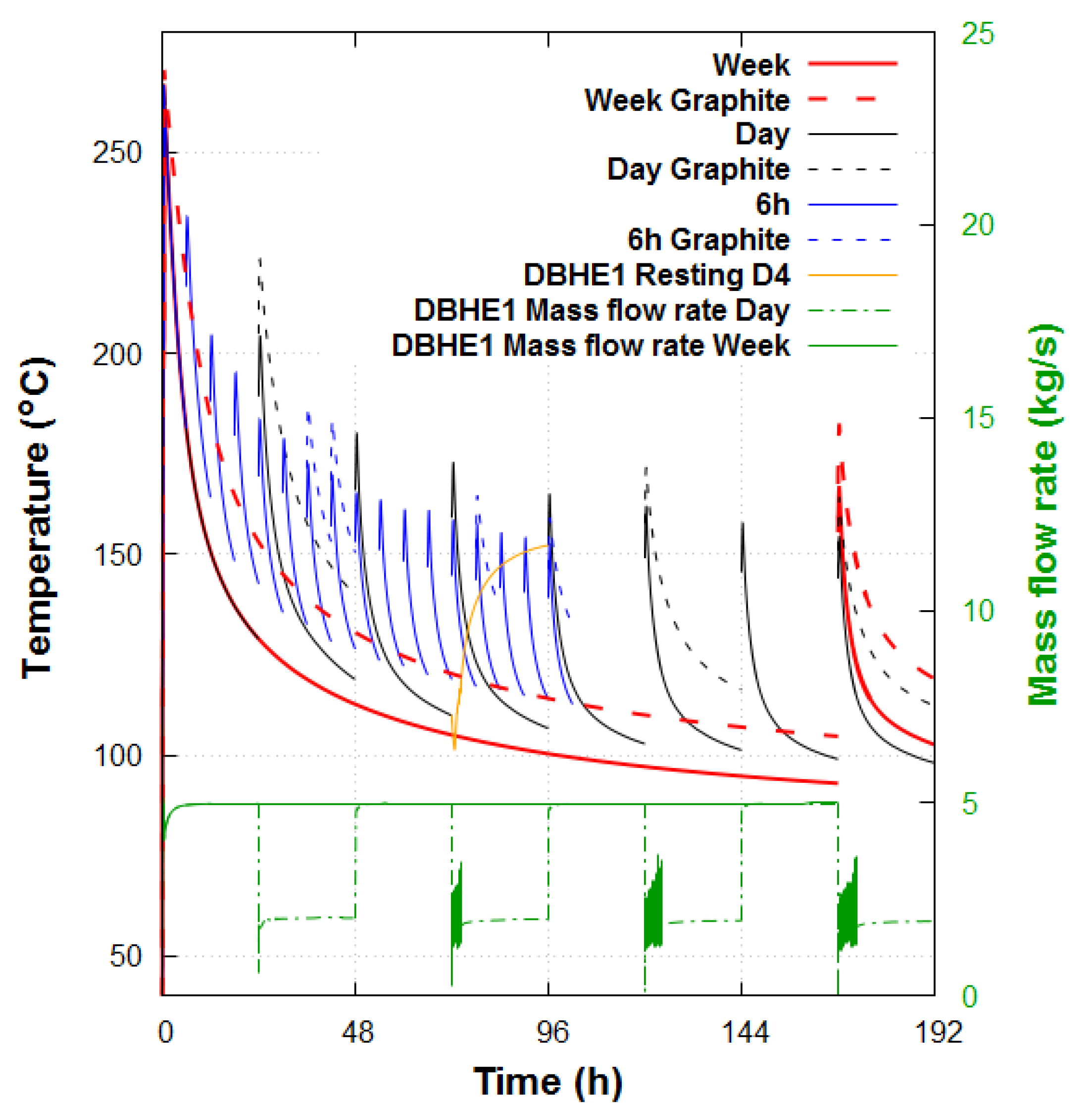
3.3.2. Alternating Heat Recovery with a DBHE Doublet
4. Discussion
5. Conclusions
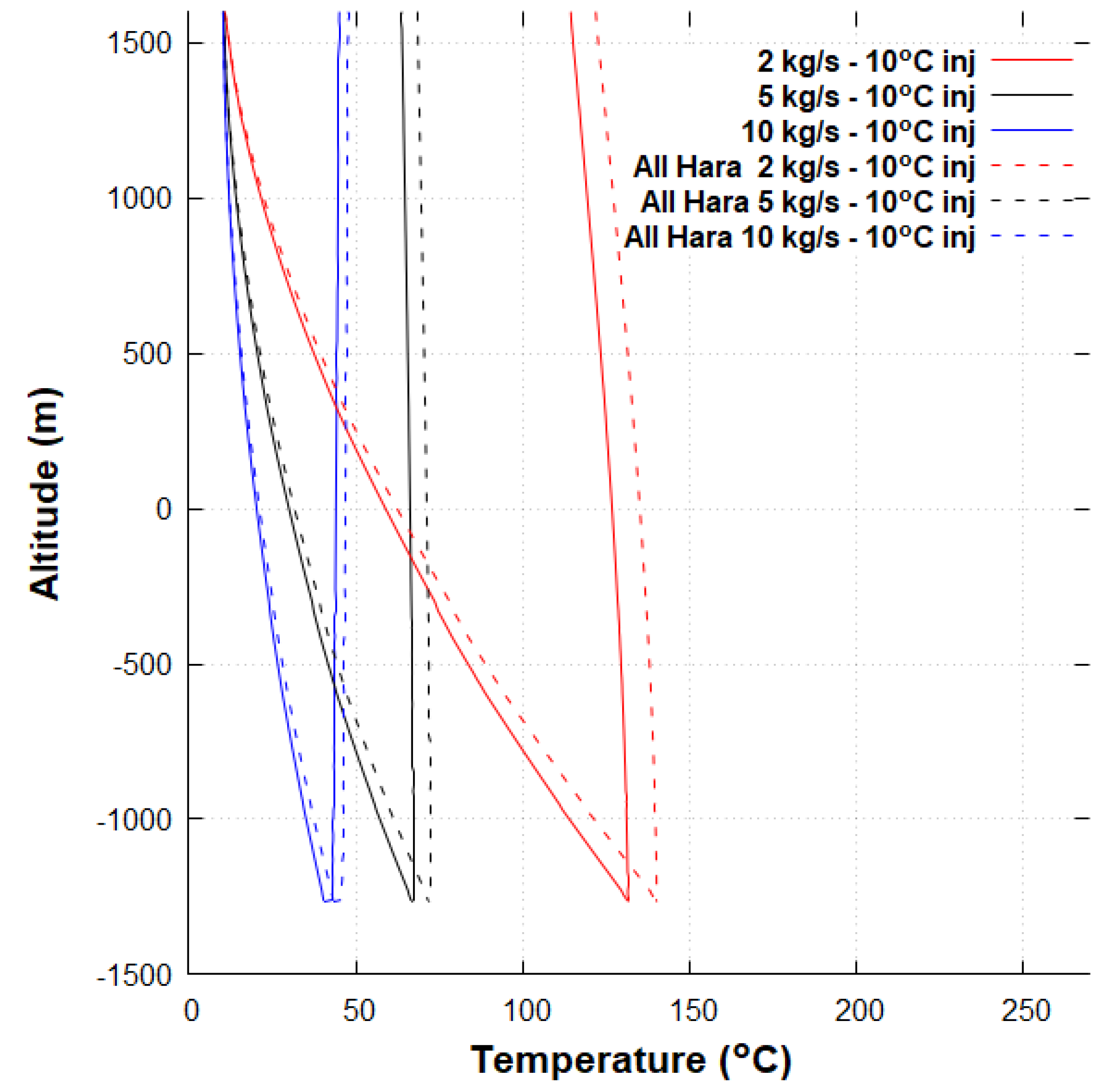
- T2Well can be used to investigate DBHEs, showing a good agreement with experimental data. The user-specific perimeter of the bottomhole and the well roughness play a major role in the pressure response of the system.
- A natural density-driven flow in the tubing is firstly observed before the arrival of the water injected from the annulus. A 1D description of the flow remains challenging to account for the pressure return flow when the water injection starts. T2Well can therefore help to investigate further unconventional geothermal wells and unlock the long-term potential of deep geothermal energy.
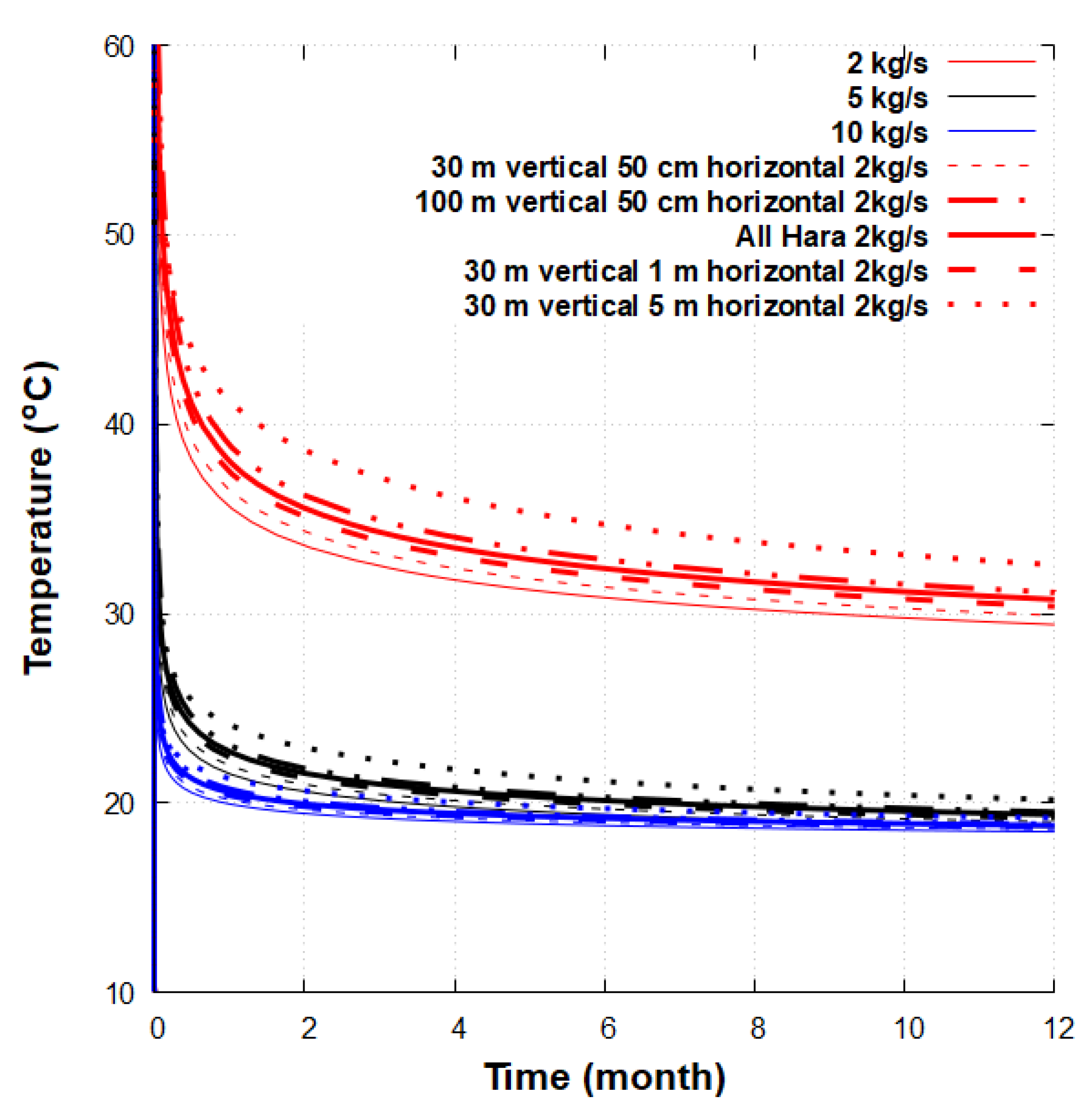
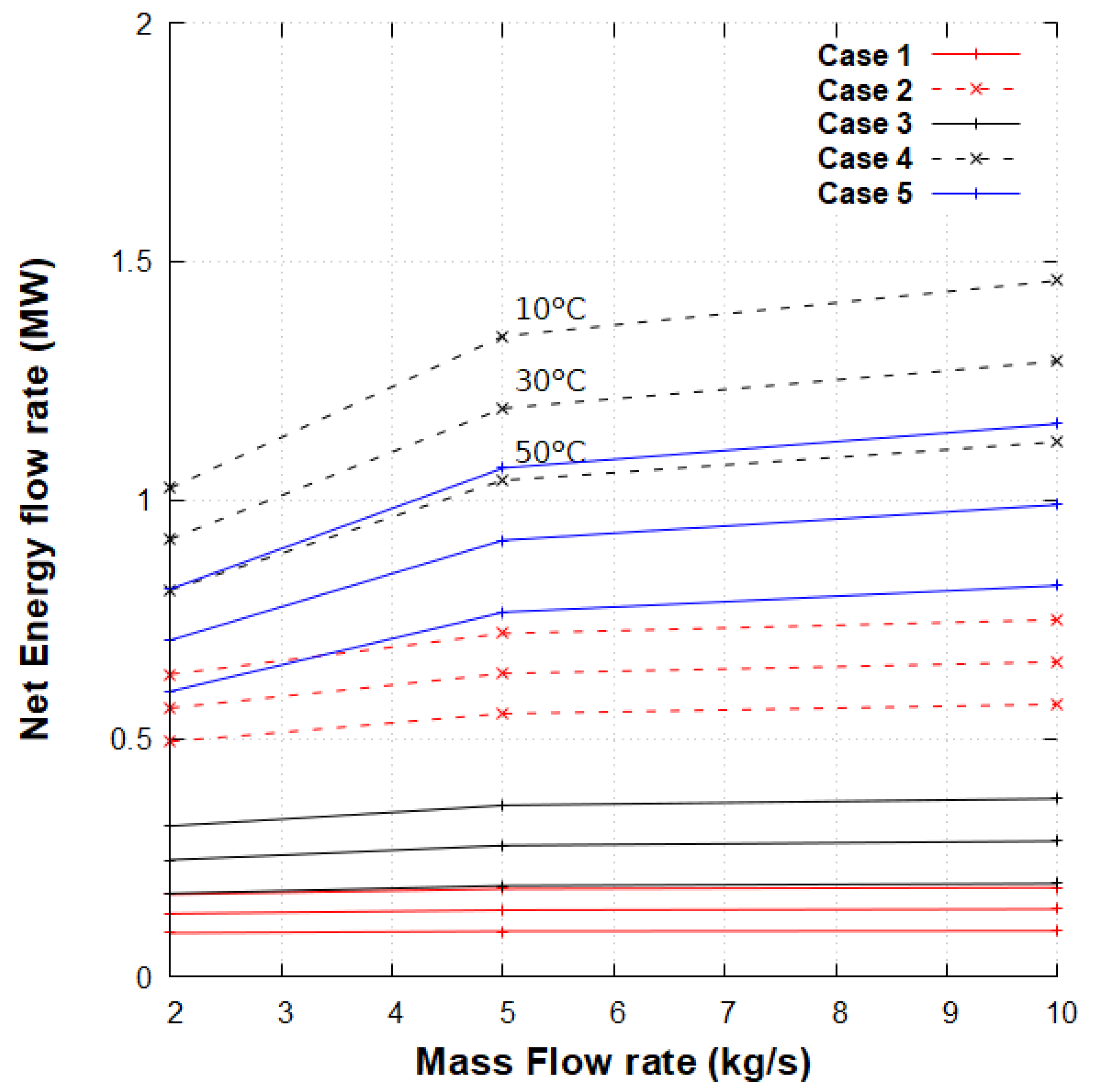
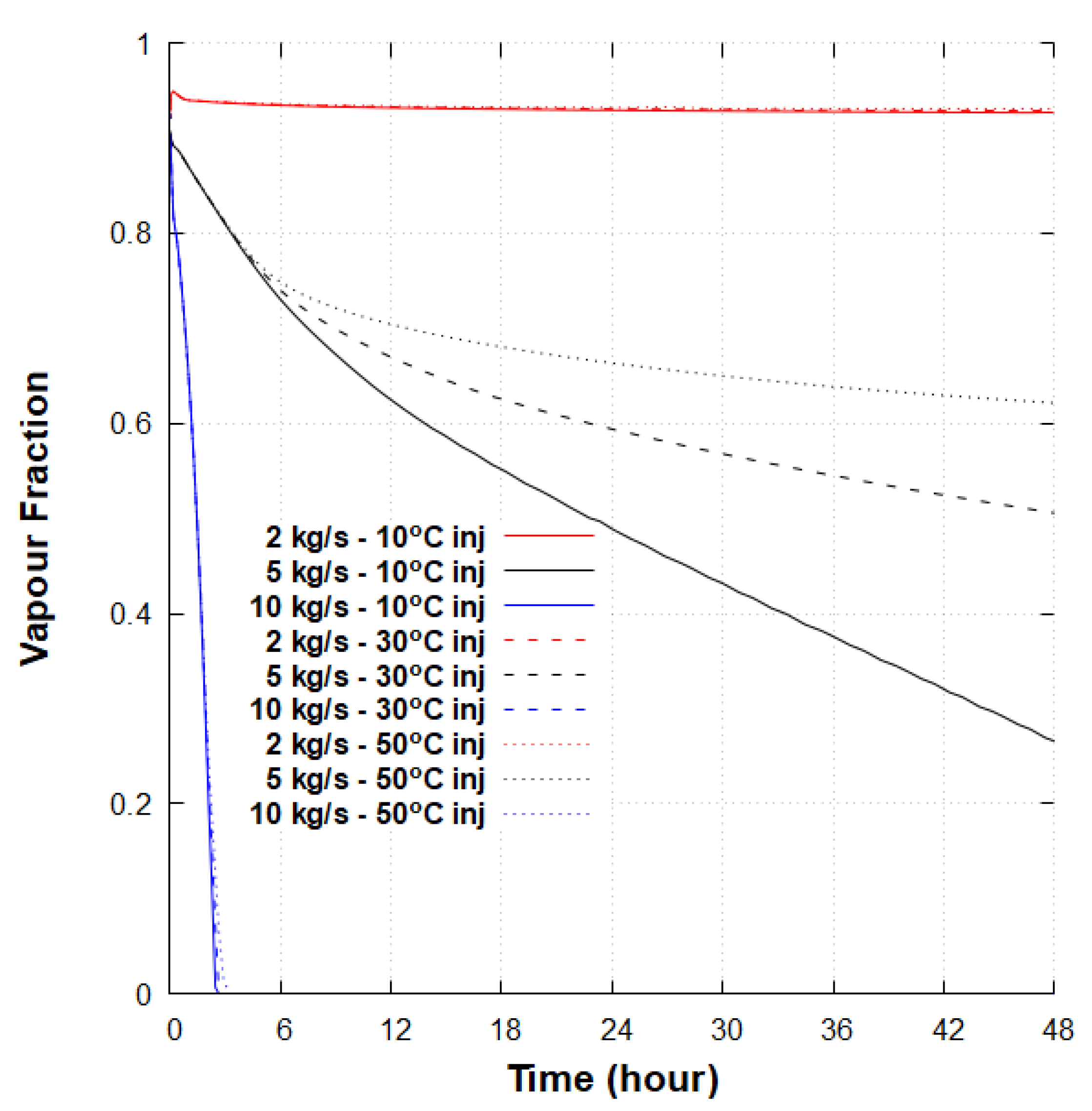
- Regarding efficiency and technical issues, installing graphite along the well casing appears to be the best option for enhancing the formations’ thermal properties and improves the economics of DBHE/DCHE systems. Graphite placed along the wellbore can improve the energy recovery in the range of 5.4 to 8.4% compared to a standard DBHE. From the cases investigated here, a maximum net energy flow rate of 1.45 MW could be extracted after one year, constrained by additional power pumping if needed. The depth of the well, the flow rate, the injection temperature, the insulation and the thermal properties of cement are the main parameters that influence the overall thermal performance of a DCHE. In some cases, an early fraction of vapour in the well can be observed, giving opportunities to further investigate the phase change in single well systems.
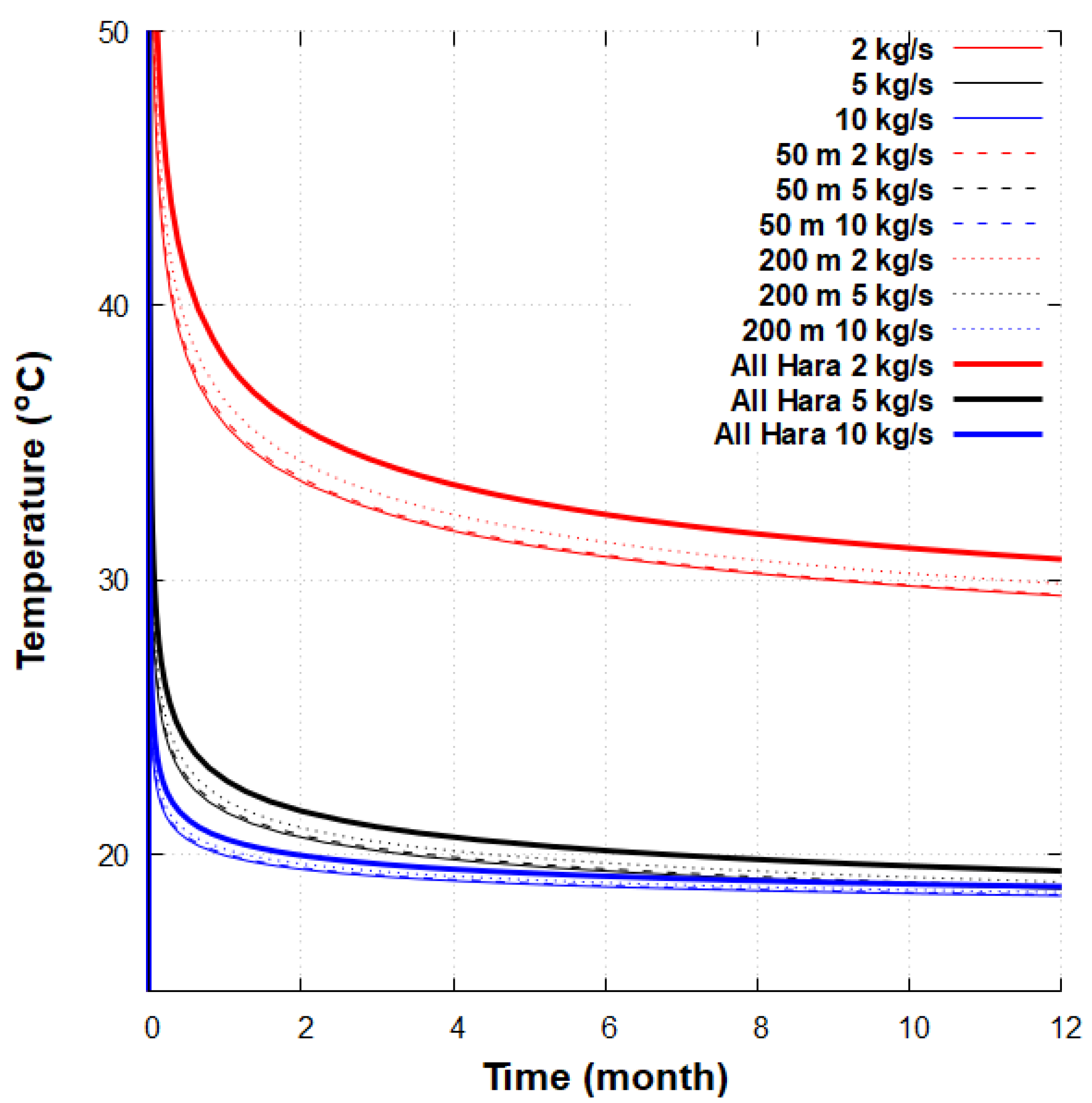
- The theoretical implementation of a DBHE with graphite in the Newberry volcano field shows the potential to recover 1 MW for and an injection flow rate of 2 kg/s with a thermosiphon effect. The analysis of a DBHE doublet injecting water alternately at 5 kg/s shows the possibility of increasing the total flow rate. The energy output variability can also be reduced by shortening the circulating period. The loading periods enable the system to recover heat and sustain a higher temperature in the system. While EGSs are facing technical and economic limitations, developing efficient single well DBHEs with innovative technology could bypass site-specific dependency of geothermal energy, without the need for hydraulic enhancement techniques.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Surface area of the subdomain V | [m] | |
| Thermal conductivity | [W/m·K] | |
| Phase viscosity | [Pa·s] | |
| Porosity | ||
| Density of the phase | [kg·m] | |
| Angle between the well section and the vertical axis | [] | |
| A | Cross sectional area of the well | [m] |
| c | Specific heat | [J·kg.C] |
| g | Gravitational acceleration | [9.81 m·s] |
| h | Specific enthalpy of the phase | [J·kg] |
| k | Absolute permeability | [m] |
| n | Outward normal vector | |
| P | Pressure | [Pa] |
| q | Source term | [kg·m·s, W·m] |
| r | Radial, horizontal component in the well | |
| S | Local saturation of the rock with phase | |
| T | Temperature | [C] |
| t | Time | [s] |
| u | Phase velocity | [m·s] |
| U | Specific internal energy | [J·kg] |
| V | Volume | [m] |
| X | Mass fraction of water | |
| z | Elevation, vertical component in the well | [m] |
| Subscripts | ||
| E | Energy | |
| g | Gas phase | |
| l | Liquid phase | |
| M | Mass | |
| m | Mixture | |
| n | Normal vector on a surface element | |
| R | Rock | |
| r | Relative permeability |
Appendix A
References
- Tester, J.W.; Morris, G.E.; Cummings, R.G.; Bivins, R.L. Electricity from Hot Dry Rock Geothermal Energy: Technical and Economic Issues; Technical Report; United States Department of Energy: Washington, DC, USA, 1979.
- Lu, S.M. A global review of enhanced geothermal system (EGS). Renew. Sustain. Energy Rev. 2017, 81, 2902–2921. [Google Scholar] [CrossRef]
- Cladouhos, T.T.; Petty, S.; Swyer, M.W.; Uddenberg, M.E.; Grasso, K.; Nordin, Y. Results from Newberry Volcano EGS Demonstration, 2010–2014. Geothermics 2015, 63, 44–61. [Google Scholar] [CrossRef]
- Grigoli, F.; Cesca, S.; Rinaldi, A.P. The November 2017 Mw 5.5 Pohang earthquake: A possible case of induced seismicity in South Korea. Science 2018, 10, 1126. [Google Scholar]
- Wilberforce, T.; Baroutaji, A.; El Hassan, Z.; Thompson, J.; Soundan, B.; Oladi, A.G. Prospects and challenges of concentrated solar photovoltaics and enhanced geothermal energy technologies. Sci. Total Environ. 2019, 659, 851–861. [Google Scholar] [CrossRef] [PubMed]
- Alimonti, C.; Soldo, E.; Bocchetti, D.; Berardi, D. The wellbore heat exchangers: A technical review. Renew. Energy 2018, 123, 353–381. [Google Scholar] [CrossRef]
- Wang, Z.; Mcclure, M.W.; Horne, R.N. Modeling Study of Single-Well EGS Configurations. In Proceedings of the World Geothermal Congress, Bali, Indonesia, 25–29 April 2010. [Google Scholar]
- Feng, Y.; Tyagi, M.; White, C.D. A downhole heat exchanger for horizontal wells in low-enthalpy geopressured geothermal brine reservoirs. Geothermics 2015, 53, 368–378. [Google Scholar] [CrossRef]
- Huang, W.; Cao, W.; Jiang, F. A novel single-well geothermal system for hot dry rock geothermal energy exploitation. Energy 2018, 162, 630–644. [Google Scholar] [CrossRef]
- Holmberg, H.; Acuna, J.; Naess, E.; Sønju, O.K. Thermal evaluation of coaxial deep borehole heat exchangers. Renew. Energy 2016, 97, 65–76. [Google Scholar] [CrossRef]
- Beier, R.A.; Acuña, J.; Mogensen, P.; Palm, B. Transient heat transfer in a coaxial borehole heat exchanger. Geothermics 2014, 51, 470–482. [Google Scholar] [CrossRef]
- Lund, J.W.; Boyd, T.L. Direct utilization of geothermal energy 2015 worldwide review. Geothermics 2016, 60, 66–93. [Google Scholar] [CrossRef]
- Kohl, T.; Brenni, R.; Eugester, W. System Performance of a Deep Borehole Heat Exchanger. Geothermics 2002, 31, 687–708. [Google Scholar] [CrossRef]
- Morita, K.; Bollmeier, W.S.; Mizogami, H. An Experiment to Prove the Concept of the Downhole Coaxial Heat Exchanger (DCHE) in Hawaii; Transactions-Geothermal Resources Council; Geothermal Resources Council: Davis, CA, USA, 1992; Volume 16, pp. 9–16. [Google Scholar]
- Song, X.; Shi, Y.; Li, G.; Yang, R.; Xu, Z.; Zheng, R.; Wang, G.; Lyu, Z. Heat extraction performance simulation for various configurations of a downhole heat exchanger geothermal system. Energy 2017, 141, 1489–1503. [Google Scholar] [CrossRef]
- Gharibi, S.; Mortezazadeh, E.; Bodi, S.J.H.A.; Vatani, A. Feasibility study of geothermal heat extraction from abandoned oil wells using a U-tube heat exchanger. Energy 2018, 153, 554–567. [Google Scholar] [CrossRef]
- Song, X.; Shi, Y.; Li, G.; Zhonghou, S.; Xiaodong, H.; Zehao, L.; Rui, Z.; Gaosheng, W. Numerical analysis of the heat production performance of a closed loop geothermal system. Renew. Energy 2018, 120, 365–378. [Google Scholar] [CrossRef]
- Oldenburg, C.M.; Pan, L.; Muir, M.P.; Eastman, A.D.; Higgins, B.S. Numerical Simulation of Critical Factors Controlling Heat Extraction from Geothermal Systems Using a Closed-Loop Heat Exchange Method. In Proceedings of the 41st Workshop on Geothermal Reservoir Engineering, Stanford University, Stanford, CA, USA, 22–24 February 2016. [Google Scholar]
- Zhao, Y.; Ma, Z.; Pang, Z. A Fast Simulation Approach to the Thermal Recovery Characteristics of Deep Borehole Heat Exchanger after Heat Extraction. Sustainability 2020, 12, 2021. [Google Scholar] [CrossRef]
- Cheng, W.L.; Li, T.T.; Nian, Y.L. Evaluation of working fluids for geothermal power generation from abandoned oil wells. Appl. Energy 2014, 118, 238–245. [Google Scholar] [CrossRef]
- Le Lous, M.; Larroque, F.; Dupuy, A. Thermal performance of a deep borehole heat exchanger: Insights from a synthetic coupled heat and flow model. Geothermics 2015, 57, 157–172. [Google Scholar] [CrossRef]
- Tomasz, S.; Kruszewski, M.; Zare, A.; Assadi, M.; Sapinska-Sliwa, A. Potential application of vacuum insulated tubing for deep borehole heat exchangers. Geothermics 2018, 75, 58–67. [Google Scholar]
- Tang, H.; Xu, B.; Hasan, A.R.; Sun, Z.; Killough, J. Modeling wellbore heat exchangers: Fully numerical to fully analytical solutions. Renew. Energy 2019, 133, 1124–1135. [Google Scholar] [CrossRef]
- Chen, C.; Shao, H.; Naumov, D.; Kong, Y.; Tu, K.; Kolditz, O. Numerical investigation on the performance, sustainability, and efficiency of the deep borehole heat exchanger system for building heating. Geotherm. Energy 2019, 7, 18. [Google Scholar] [CrossRef]
- Alimonti, C.; Soldo, E. Study of geothermal power generation from a very deep oil well with a wellbore heat exchanger. Renew. Energy 2016, 86, 292–301. [Google Scholar] [CrossRef]
- Hu, X.; Banks, J.; Wu, L.; Liu, W.V. Numerical modeling of a coaxial borehole heat exchanger to exploit geothermal energy from abandoned petroleum wells in Hinton, Alberta. Renew. Energy 2019, 148, 1110–1123. [Google Scholar] [CrossRef]
- Sui, D.; Wiktorski, E.; Røksl, M.; Basmoen, T.A. Review and investigations on geothermal energy extraction from abandoned petroleum wells. J. Pet. Explor. Prod. Technol. 2018, 9, 1135–1147. [Google Scholar] [CrossRef]
- Renaud, T.; Verdin, P.; Falcone, G. Numerical simulation of a Deep Borehole Heat Exchanger in the Krafla geothermal system. Int. J. Heat Mass Transf. 2018, 143, 58–67. [Google Scholar] [CrossRef]
- Hara, H. Geothermal Well Using Graphite as Solid Conductor. U.S. Patent 2011/0232858 A1, 29 September 2011. [Google Scholar]
- Buchi, H.F. Method and Apparatus for Extracting and Utilizing Geothermal Energy. U.S. Patent 4,912,941, 3 April 1990. [Google Scholar]
- Song, X.; Zheng, R.; Li, G.; Shi, Y.; Wang, G.; Li, J. Heat extraction performance of a downhole coaxial heat exchanger geothermal system by considering fluid flow in the reservoir. Geothermics 2018, 76, 190–200. [Google Scholar] [CrossRef]
- Falcone, G.; Liu, X.; Okech, R.R.; Seyidov, F.; Teodoriu, C. Assessment of Deep Geothermal Energy Exploitation Methods: The Need for Novel Single-Well Solutions. Energy 2018, 160, 54–63. [Google Scholar] [CrossRef]
- Pan, L.; Oldenburg, C.M. T2Well-An integrated wellbore-reservoir simulator. Comput. Geosci. 2014, 65, 46–55. [Google Scholar] [CrossRef]
- Morita, K.; Bollmeier, W.S.; Mizogami, H. Analysis of the Results from the Downhole Coaxial Heat Exchanger (DCHE) Experiment in Hawaii; Transactions-Geothermal Resources Council; Geothermal Resources Council: Davis, CA, USA, 1992. [Google Scholar]
- Pan, L.; Oldenburg, C.M.; Wu, Y.S.; Pruess, K. T2Well/ECO2N Version 1.0: Multiphase and Non-Isothermal Model for Coupled Wellbore-Reservoir Flow of Carbon Dioxide and Variable Salinity Water, LBN-4291E; Earth Sciences Division, Lawrence Berkeley National Laboratory, Univeristy of California: Berkeley, CA, USA, 2011. [Google Scholar]
- Wagner, W.; Cooper, J.R.; Dittmann, A.; Kijima, J.; Kretzschmar, H.J.; Kruse, A.; Mares, R.; Oguchi, K.; Sato, H.; Stocker, I.; et al. The IAPWS Industrial Formulation 1997 for the Thermodynamic Properties of Water and Steam. J. Eng. Gas Turbines Power 2000, 7, 150. [Google Scholar] [CrossRef]
- Pruess, K.; Oldenburg, C.; Moridis, G. TOUGH2 User’s Guide; Earth Sciences Division, Lawrence Berkeley National Laboratory University of California: Berkeley, CA, USA, 2012. [Google Scholar]
- Sonnenthal, E.; Spycher, N.; Callahan, O.; Cladouhos, T.; Petty, S.A. Thermal-Hydrological-Chemical Model for the Enhanced Geothermal System Demonstration Project At Newberry Volcano; Oregon. In Proceedings of the 37th Workshop on Geothermal Reservoir Engineering; Stanford University, Stanford University, Stanford, CA, USA, 30 January–1 February 2012. [Google Scholar]
- Doran, H.; Renaud, T.; Falcone, G.; Pan, L.; Verdin, P.G. Modelling an unconventional closed-loop deep borehole heat exchanger (DBHE): Sensitivity analysis on the Newberry volcanic setting. Geotherm. Energy 2021, 9, 1–24. [Google Scholar] [CrossRef]
- Farshad, F.F.; Rieke, H.H. Surface-Roughness Design Values for Modern Pipes. SPE Drill. Complet. 2006, 21, 212–215. [Google Scholar] [CrossRef]
- Gelet, R.; Loret, B.; Khalili, N. A thermo-hydro-mechanical coupled model in local thermal non-equilibrium for fractured HDR reservoir with double porosity. J. Geophys. Res. Solid Earth 2012, 117, 1–23. [Google Scholar] [CrossRef]
- Noorollahi, Y.; Pourarshad, M.; Jalilinasrabady, S.; Yousefi, H. Numerical simulation of power production from abandoned oil wells in Ahwaz oil field in southern Iran. Geothermics 2015, 55, 16–23. [Google Scholar] [CrossRef]
- Bu, X.; Ma, W.; Li, H. Geothermal energy production utilizing abandoned oil and gas wells. Renew. Energy 2012, 41, 80–85. [Google Scholar] [CrossRef]
- Palsson, B.; Holmgeirsson, S.; Gudmundsson, A.; Boasson, H.A.; Ingason, K.; Sverrisson, H.; Thorhallsson, S. Drilling of the well IDDP-1. Geothermics 2014, 49, 23–30. [Google Scholar] [CrossRef]
- Elders, W.A.; Fridleifsson, G.O.; Albertsson, A. Drilling into magma and the implications of the Iceland Deep Drilling Project (IDDP) for high-temperature geothermal systems worldwide. Geothermics 2014, 49, 111–118. [Google Scholar] [CrossRef]
- Boehm, R.F.; Berg, D.L.; Ortega, A. Modeling of a Magma Energy Geothermal Open Cycle Power. J. Energy Res. Technol. 1989, 111, 39–245. [Google Scholar] [CrossRef]
- Bonneville, A.; Cladouhos, T.T.; Petty, S.; Schultz, A.; Sorlie, C. Toward Super Hot EGS: The Newberry Deep Drilling Project. In Proceedings of the GRC Transactions, Reno, NV, USA, 14–17 October 2018. [Google Scholar]
- Bertani, R.; Büsing, H.; Buske, S.; Dini, A.; Hjelstuen, M.; Luchini, M.; Manzella, A. The First Results of the DESCRAMBLE Project. In Proceedings of the 43rd Workshop on Geothermal Reservoir Engineering 2018, Stanford, CA, USA, 12–14 February 2018. [Google Scholar]
- Eichelberger, J.; Ingolfsson, H.P.; Carrigan, C.; Lavallee, Y.; Tester, J.W.; Markusson, S.H. Krafla Magma Test bed: Understanding and Using the Magma-Hydrothermal Connection. In Procedings of the GRC Transactions, Reno, NV, USA, 14–17 October 2018. [Google Scholar]
- Shi, H.; Holmes, J.A.; Durlofsky, L.J.; Aziz, K.; Diaz Teran Ortegon, L.R.; Alkaya, B.; Oddie, G. Drift-flux modeling of two-phase flow in wellbores. Soc. Pet. Eng. J. 2005, 10, 24–33. [Google Scholar] [CrossRef]


| Materials | [kg/m] | [W/m·K] | c [J/kg·K] |
|---|---|---|---|
| Formation | 3050 | 1.6 | 870 |
| Cement | 1830 | 0.99 | 1900 |
| Casing | 7850 | 46.1 | 470 |
| Inner Casing | 7850 | 0.06 | 470 |
| Case | DCHE Depth [m] | Geothermal Gradient [C/km] |
|---|---|---|
| 1 | 1000 | 170 |
| 2 | 1990 | 170 |
| 3 | 1990 | 85 |
| 4 | 3990 | 85 |
| 5 | 3990 | 56 |
| Formations | Site Altitude | Thermodynamic |
|---|---|---|
| Range [m] | Properties | |
| Newberry–Deschutes | +1600 to +1400 | = 0.2 |
| (upper 300 m) | k = 0.15 × 10 | |
| c = 1000 | ||
| = 1.7 | ||
| Newberry–Deschutes | +1400 to +300 | = 0.1 |
| k = 0.1 × 10 | ||
| = 1.8 | ||
| John Day | +300 to −800 | = 0.05 |
| k = 2.6 × 10 | ||
| = 2.15 | ||
| Intruded John Day | −800 to −1500 | = 0.03 |
| k = 0.5 × 10 | ||
| = 2.2 |
| (m) | (m) | z (m) | Case | V [m] | |
|---|---|---|---|---|---|
| Hara’s patent | 0.11119 | 0.0889 | 1000 | 1 | 14 |
| (All Hara) | 0.11119 | 0.0889 | 1990 | 2, 3 | 27.86 |
| 0.11119 | 0.0889 | 3990 | 4, 5 | 55.87 | |
| Buchi’s patent | 0.5 | 0.0889 | 30 | 1 | 22.8 |
| (30 m vertical) | 1 | 0.0889 | 30 | 1 | 93.45 |
| 5 | 0.0889 | 30 | 1 | 2354.25 |
| Net Energy Flow Rate | Net % of Increase | |||||
|---|---|---|---|---|---|---|
| 2 kg/s | 5 kg/s | 10 kg/s | 2 kg/s | 5 kg/s | 10 kg/s | |
| Case 1 | 0.17/0.13/0.09 | 0.18/0.14/0.09 | 0.18/0.14/0.09 | 7.5/7.9/8.0 | 7.9/7.9/8.1 | 8.0/8.1/8.4 |
| Case 2 | 0.63/0.56/0.49 | 0.71/0.63/0.55 | 0.74/0.66/0.57 | 7.0/7.6/7.9 | 7.6/7.7/7.7 | 7.9/8.0/8.0 |
| Case 3 | 0.31/0.24/0.17 | 0.35/0.27/0.19 | 0.27/0.28/0.19 | 6.8/7.0/7.2 | 7.7/7.8/7.8 | 8.0/8.1/8.0 |
| Case 4 | 1.02/0.91/0.81 | 1.34/1.19/1.04 | 1.45/1.29/1.12 | 5.6/5.7/5.9 | 7.2/7.3/7.4 | 7.8/7.9/8.0 |
| Case 5 | 0.81/0.70/0.59 | 1.06/0.91/0.76 | 1.15/0.98/0.82 | 5.4/5.5/5.7 | 7.0/7.1/7.2 | 7.6/7.7/7.8 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Renaud, T.; Pan, L.; Doran, H.; Falcone, G.; Verdin, P.G. Numerical Analysis of Enhanced Conductive Deep Borehole Heat Exchangers. Sustainability 2021, 13, 6918. https://doi.org/10.3390/su13126918
Renaud T, Pan L, Doran H, Falcone G, Verdin PG. Numerical Analysis of Enhanced Conductive Deep Borehole Heat Exchangers. Sustainability. 2021; 13(12):6918. https://doi.org/10.3390/su13126918
Chicago/Turabian StyleRenaud, Theo, Lehua Pan, Hannah Doran, Gioia Falcone, and Patrick G. Verdin. 2021. "Numerical Analysis of Enhanced Conductive Deep Borehole Heat Exchangers" Sustainability 13, no. 12: 6918. https://doi.org/10.3390/su13126918
APA StyleRenaud, T., Pan, L., Doran, H., Falcone, G., & Verdin, P. G. (2021). Numerical Analysis of Enhanced Conductive Deep Borehole Heat Exchangers. Sustainability, 13(12), 6918. https://doi.org/10.3390/su13126918







