Optimization of Two Soil–Structure Interaction Parameters Using Dynamic Centrifuge Tests and an Analytical Approach
Abstract
1. Introduction
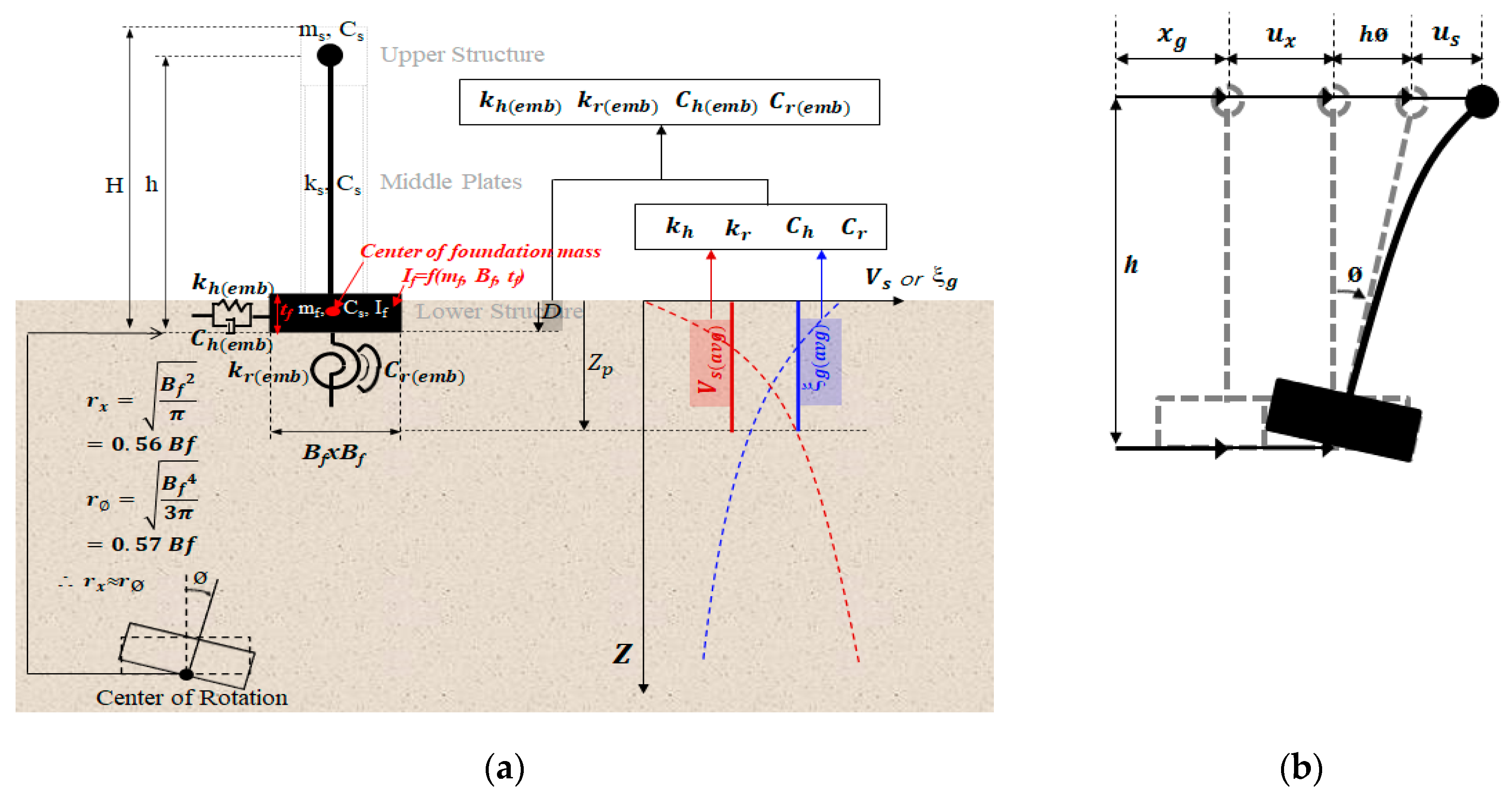
2. Soil–Structure Interaction (SSI) Parameters and Optimum SSI Parameter Selection Procedure
2.1. Soil–Spring Constants and Damping Coefficients
2.1.1. Strain-Dependent Soil Properties
2.1.2. Depth-Dependent Soil Properties and Effective Profile Depth ()
2.1.3. Embedment Correction for Spring Constant and Radiation Damping Coefficient
2.1.4. Determination of Soil Damping Based on Radiation and Material Damping Considerations
2.2. Effective Height of Structure
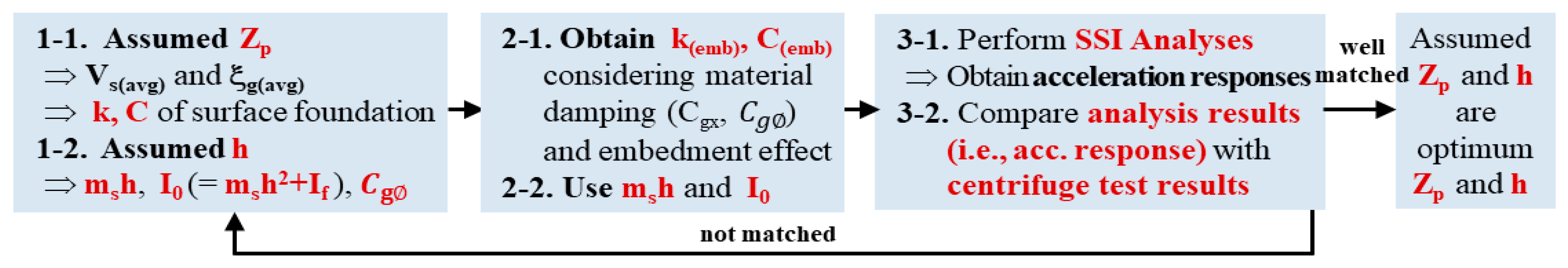
2.3. Procedure Used to Identify the Optimum and of the Structure
3. Testing and Analysis Programs
3.1. Dynamic Centrifuge Tests
3.2. Analytical Approach Based on State Space Equation (SSE)
3.3. Properties of Structure in Tests and Analytical Approach
3.4. Soil Properties and Ground Condition in Tests and Analytical Approaches
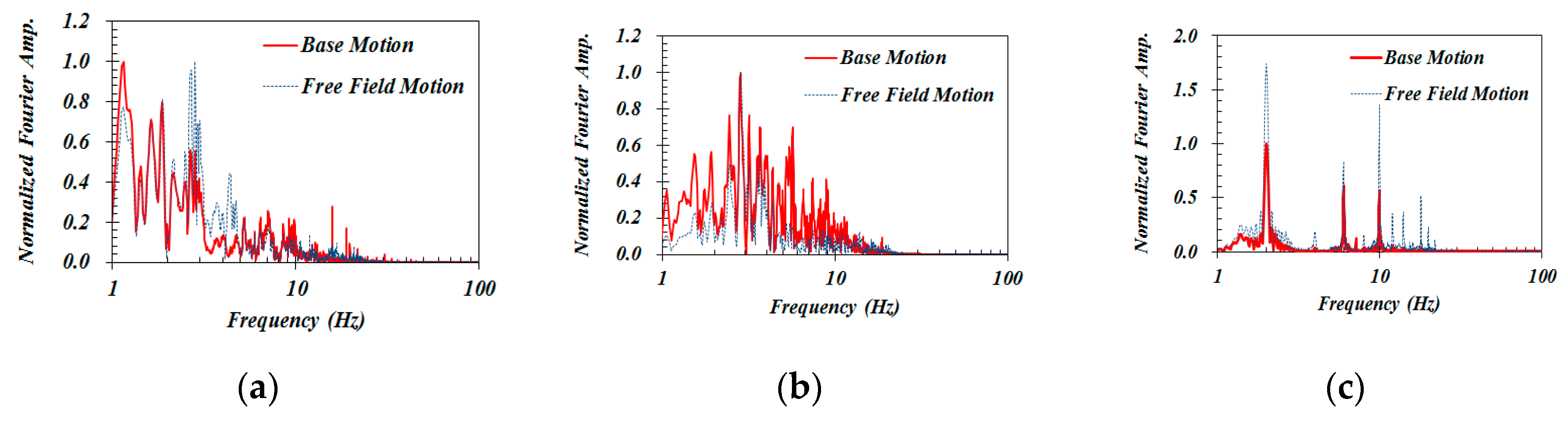
3.5. Input Motions in Tests and Analyses
4. Determination of Soil–Spring Constant and Damping Coefficient
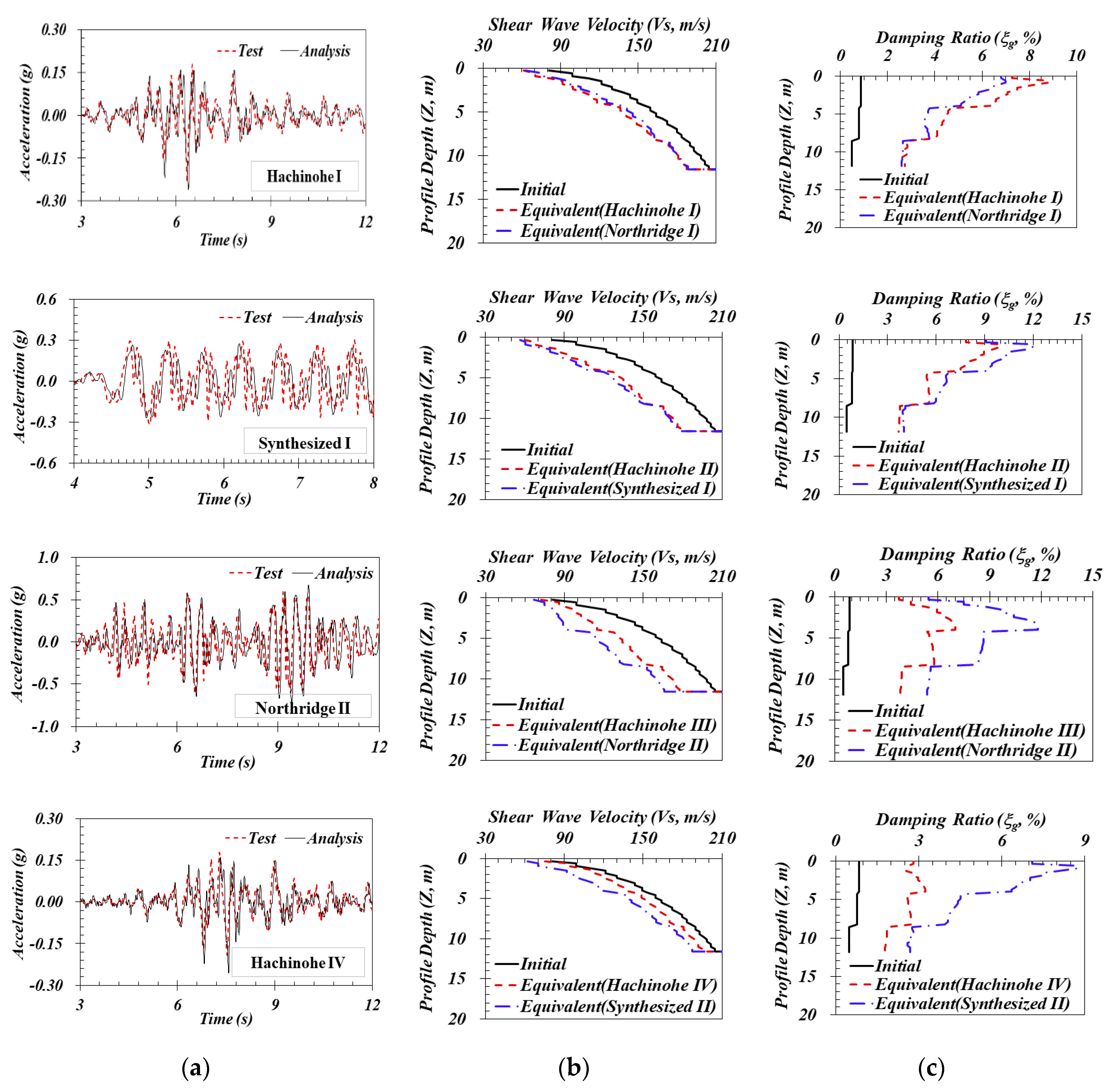
4.1. Site-Response Analyses (SRAs) and Strain-Independent Soil Properties
4.2. Depth-Independent Soil Properties
5. Evaluation of Two Optimum SSI Parameters and
5.1. Determination of Analysis CASES Considering the SSI Parameters
- In the case of the optimum value: 24 soil-spring constants and damping coefficients = one formula × eight input motions × three effective heights × one effective profile depth
- In the case of the optimum value: 48 soil-spring constants and damping coefficients = two formulas × eight input motions × one effective height × three effective profile depths
5.2. Quantification of Differences between Test and Analysis Results
5.3. Evaluation of the Optimum h
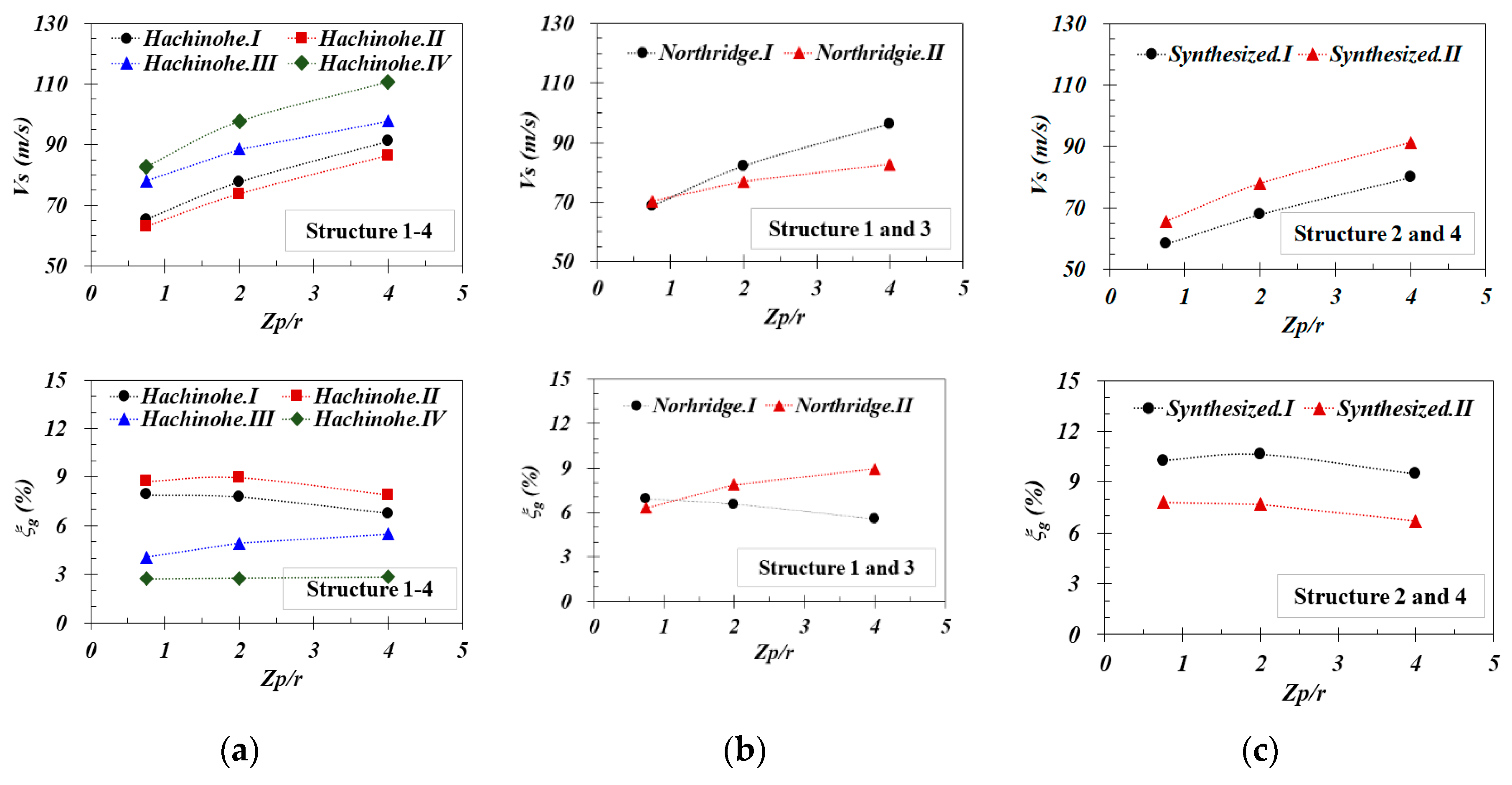
5.4. Evaluation of the Optimum Effective Profile Depth
6. Conclusions
- In this study, the applicability of the SSI parameters suggested by various standards and studies was discussed, and the optimum SSI parameter selection procedure that (a) comprehensively considered the SSI parameters, (b) adopted an analytical approach and a physical model test, was suggested. Based on the established procedure, the optimum values of two controversial SSI parameters (i.e., the effective height and effective profile depth) were determined
- Unlike the conventional standards that apply a simplified reduction factor for the initial shear wave velocity profile and do not apply any corrections in initial damping ratio profile, one dimensional equivalent linear site response analyses were performed to accurately obtain the equivalent strain-independent shear wave velocity ( ) and damping ratio () of the soil. The equivalent and values that varied with depth obtained herein were converted to depth independent and values based on considerations of the effective profile depth ().
- Unlike the conventional research efforts that ignored soil material damping and indirectly determined soil damping based on the effective period lengthening ratio, the total soil damping was obtained directly by the addition of soil material damping to soil radiation damping. In addition, the stiffness and total damping of soil were determined based on embedded foundation conditions.
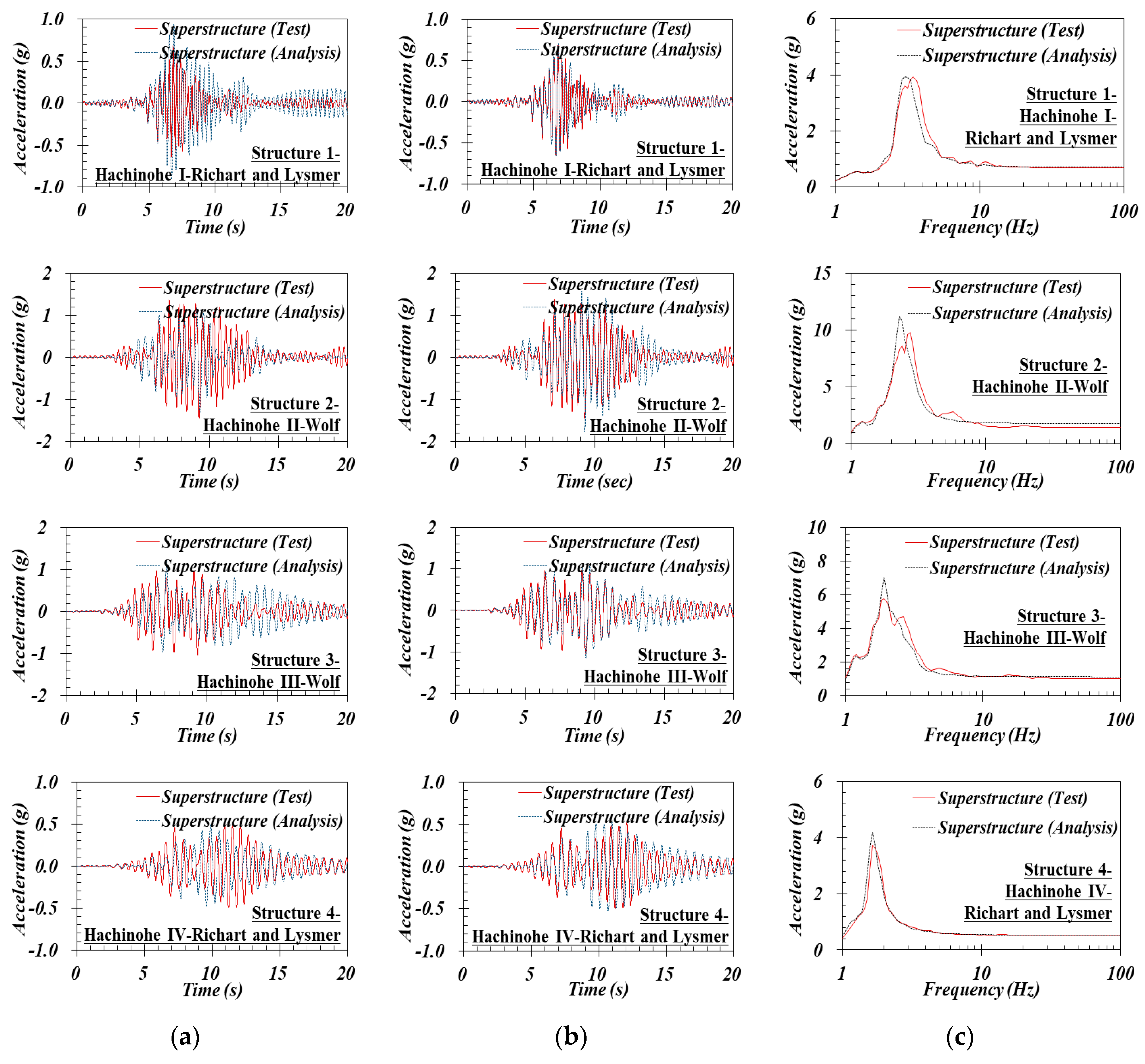
- Unlike the conventional SDOF SSI analysis that was based on the RS, this study adopted a 3DOF time domain SSI analysis based on structural translation, foundation translation, and rocking behavior considerations to accurately obtain structural responses.
- The effective height of the structure affected the rocking behavior of the soil–structure system (i.e., and ). In this study, applicability of the following three effective height scenarios were evaluated based on the following test and analysis results: (1) Height from the bottom of the foundation to the center of the mass of the superstructure, and (2) height compatible to the total mass moment of inertia, and (3) height to satisfy moment equilibrium. As the effective height increased within the effective height range used in the analysis, the differences between test and analysis results increased. Consequently, in all the cases, the height from the bottom of the foundation to the center of the mass of the superstructure was the optimum effective height with the lowest value.
- The optimum effective profile depth () was determined. This was used to average the dynamic soil property that varied as a function of depth. Three scenarios were considered in the analyses: (1) /r = 0.75, (2) /r = 2, and (3) /r = 4. As a result, 4 r was found to be the optimum effective profile depth with the lowest difference between the test and analysis results. In addition, the results associated with earthquake motions yielded higher errors than those of synthesized motions that consisted of sinusoidal waves at the frequencies of 2, 6, and 10 Hz. The maximum acceleration responses by Richart and Lysmer’s formula was more conservative than those obtained with Wolf’s formula.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Wolf, J. Dynamic Soil-Structure Interaction; Section 3.4 Introductory Example; Prentice Hall: Englewood Cliffs, NJ, USA, 1985; pp. 38–50. [Google Scholar]
- Richart, F.; Hall, J.; Woods, R. Vibrations of Soils and Foundations; Prentice-Hall: Englewood Cliffs, NJ, USA, 1970. [Google Scholar]
- Gazetas, G. Foundation Engineering Handbook, 2nd ed.; Fang, H.Y., Ed.; Chapter 15 Foundation Vibrations; Springer Science Business Media: New York, NY, USA, 1991. [Google Scholar]
- Stewart, J.; Seed, R.; Fenves, G. PEER-98/07: Empirical Evaluation of Inertial Soil Structure Interaction; Pacific Earthquake Engineering Research (PEER) Center: Berkeley, CA, USA, 1998. [Google Scholar]
- Wong, H.; Luco, J. Tables of impedance functions for square foundations on layered media. Soil Dyn. Earthq. Eng. 1985, 4, 64–81. [Google Scholar] [CrossRef]
- Whitman, R. Soil Publications No-300: Analysis of Soil-Structure Interaction: A State-of-the-Art Review; Massachusetts Institute of Technology: Cambridge, MA, USA, 1972. [Google Scholar]
- Stewart, J.; Kim, S.; Bielak, J.; Dobry, R.; Power, M. Revisions to soil-structure interaction procedures in NEHRP design provisions. Earthq. Spectra 2003, 19, 677–696. [Google Scholar] [CrossRef]
- Kim, D.; Lee, S.; Kim, D.; Choo, Y.; Park, H. Rocking effect of a mat foundation on the earthquake response of structures. J. Geotech. Geoenviron. Eng. 2015, 141, 10:04014085. [Google Scholar] [CrossRef]
- Ko, K.; Ha, J.; Park, H.; Kim, D. Comparison between cyclic and dynamic rocking behavior for embedded shallow foundation using centrifuge tests. Bull. Earthq. Eng. 2018, 16, 5171–5193. [Google Scholar] [CrossRef]
- Gavras, A.; Kutter, B.; Hakhamaneshi, M.; Gajan, S.; Tsatsis, A.; Sharma, K.; Kohno, T.; Deng, L.; Anastasopoulos, I.; Gazetas, G. Database of rocking shallow foundation performance: Dynamic shaking. Earthq. Spectra 2020, 36, 960–982. [Google Scholar] [CrossRef]
- Kim, D.; Park, H.; Kim, D.; Lee, H. Nonlinear system identification on shallow foundation using Extended Kalman Filter. Soil Dyn. Earthq. Eng. 2020, 128, 105857. [Google Scholar] [CrossRef]
- FEMA. Prestandard and Commentary for the Seismic Rehabilitation of Buildings (FEMA 356); Federal Emergency Management Agency: Washington, DC, USA, 2000.
- ATC. Seismic Evaluation and Retrofit of Concrete Buildings (ATC-40); Applied Technology Council: Redwood City, CA, USA, 1996. [Google Scholar]
- FEMA. Improvement of Nonlinear Static Seismic Analysis Procedures (FEMA 440); Federal Emergency Management Agency: Washington, DC, USA, 2004.
- ASCE. Seismic Evaluation and Retrofit of Existing Buildings (ASCE 41-13); Chapter 8 Foundations and Geologic Site Hazards; American Society of Civil Engineers: Reston, VA, USA, 2014. [Google Scholar]
- BSSC. NEHRP Recommended Provisions for Seismic Regulation for New Buildings and Other Structures; Federal Emergency Management Agency: Washington, DC, USA, 1998.
- Stewart, J.; Fenves, G.; Seed, R. Seismic Soil-Structure Interaction in Buildings. I: Analytical Methods. J. Geotech. Geoenviron. Eng. 1999, 125, 26–37. [Google Scholar] [CrossRef]
- BSSC. NEHRP Recommended Provisions for Seismic Regulations for New Buildings and Other Structures (FEMA 450-1 2003 Edition); Federal Emergency Management Agency: Washington, DC, USA, 2004.
- EPRI. 1010808: Engineering Technical Training Modules for Nuclear Plant Engineers-Civil/Structural Series Module #5-Soil Structure Interaction; EPRI: Palo Alto, CA, USA, 2005. [Google Scholar]
- Mikami, A.; Sawada, T. Time-domain identification system of dynamic soil-structure interaction. In Proceedings of the 13th World Conference on Earthquake Engineering, Vancouver, BC, Canada, 1–6 August 2004. Paper No. 747. [Google Scholar]
- ASCE. Seismic Analysis of Safety-Related Nuclear Structures (ASCE 4-16); Chapter 5 Soil-Structure Interaction Modeling and Analysis; American Society of Civil Engineers: Reston, VA, USA, 2017; p. 24. [Google Scholar]
- Chowdhury, I.; Dasgupta, S. Dynamics of Structure and Foundation-A Unified Approach 2. APPLICATIONS; Chapter 2, Analysis and Design of Machine Foundation—2.4 Effect of Embedment on Foundation; Taylor & Francis Ltd.: London, UK, 2009. [Google Scholar]
- Lee, S.; Choo, Y.; Kim, D. Performance of an equivalent shear beam (ESB) model container for dynamic geotechnical centrifuge tests. Soil Dyn. Earthq. Eng. 2013, 44, 102–114. [Google Scholar] [CrossRef]
- Taylor, R. Geotechnical Centrifuge Technology; Centrifuge in Modeling: Principle and Scale Effects; Taylor & Francis Ltd.: London, UK, 1995; pp. 19–33. [Google Scholar]
- Chang, S.; Kim, D.; Kim, D.; Kang, K. Earthquake Response Reduction of Building Structures Using Learning-Based Lattice Pattern Active Controller. J. Earthq. Eng. 2012, 16, 317–328. [Google Scholar] [CrossRef]
- Nica, G.; Calofir, V.; Corâci, I. A State Space Formulation for the Evaluation of the Pounding Forces During Earthquake. Math. Model. Civ. Eng. 2018, 14, 37–49. [Google Scholar] [CrossRef]
- Karris, S. Introduction to Simulink with Engineering Applications; Chapter 5 The Discrete Blocks Library; Orchard Publications: Fremont, CA, USA, 2006; pp. 5–12. [Google Scholar]
- Kalechman, M. Practical MATLAB Basics for Engineers; Chapter 7 Polynomials and Calculus, a Numerical and Symbolic Approac; Taylor & Francis Group LLC: Boca Raton, FL, USA, 2009; pp. 474–481. [Google Scholar]
- Ross, P. The handbook of Software for Engineers and Scientists; Section V Engineering Tools, 55 MATLAB in Systems and Controls; Taylor & Francis Group: Boca Raton, FL, USA, 1996. [Google Scholar]
- Chopra, A. Dynamics of Structures; Section 2.2.4 Free Vibration Tests; Prentice Hall: Upper Saddle River, NJ, USA, 2007. [Google Scholar]
- Kim, J.; Choo, Y.; Kim, D. Correlation between the Shear-Wave Velocity and Tip Resistance of Quartz Sand in a Centrifuge. J. Geotech. Geoenviron. Eng. 2017, 143, 04017083. [Google Scholar] [CrossRef]
- Cho, H.; Kim, N.; Park, H.; Kim, D. Settlement Prediction of Footings Using VS. Appl. Sci. 2017, 7, 1105. [Google Scholar] [CrossRef]
- Rathje, E.; Abrahamson, N.; Bray, J. Simplified frequency content estimates of earthquake ground motions. J. Geotech. Geoenviron. Eng. 1998, 124, 150–159. [Google Scholar] [CrossRef]
- Kottke, A.; Rathje, E. PEER-2008/10: Technical Manual for Strata; Pacific Earthquake Engineering Research (PEER) Center: Berkeley, CA, USA, 2009. [Google Scholar]




| Type of Formula | Spring Constant | Radiation Damping Coefficient | ||
|---|---|---|---|---|
| Kx (=kh) | Kø (=kr) | Cx | Cø | |
| Wolf [1] FEMA440 [14] | ||||
| Richart and Lysmer [2], EPRI [19], ASCE4-16 [21] | ||||
| Property | Mode | Correction Factor for Embedment | Corrected Stiffness and Damping Ratio |
|---|---|---|---|
| stiffness | |||
| radiation damping | swaying | ||
| rocking |
| Property | Mode | Soil–Spring Constant and Damping Coefficient Formula |
|---|---|---|
| soil spring constant | swaying () | |
| rocking () | ||
| soil total damping coefficient | swaying | |
| rocking |
| Structure Models | Structure 1 | Structure 2 | Structure 3 | Structure 4 |
|---|---|---|---|---|
| dimensions (m) | 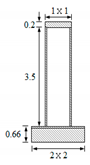 |  |  | 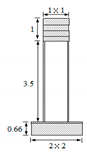 |
| mass of upper structure (, kg) | 1580 | 3634 | 5530 | 7900 |
| mass of middle plates (, kg) | 1991 | |||
| mass of lower structure (, kg) | 20,856 | |||
| natural frequency (, Hz) | 3615 | 2540 | 2097 | 1773 |
| effective mass of superstructure (, kg) | 2003 | 4057 | 5953 | 8323 |
| effective mass of foundation. (, kg) | 22,424 | |||
| effective lateral stiffness (, N/m) | 1,033,191 | |||
| damping ratio (, %) | 1.406 | 1.674 | 2.379 | 2.252 |
| General Properties [32] | Ground Condition | |||||||
|---|---|---|---|---|---|---|---|---|
| USCS | ||||||||
| SP | 0.22 | 2.65 | 1.130 | 0.611 | 55 | 14.092 | 37 | 0.398 |
| Case | Input Motion | Base Motions | Free Field Motions | ||
|---|---|---|---|---|---|
| PGA (g) | PGA (g) | ||||
| structure 1 | Hachinohe I | 0.112 | 1.842 | 0.227 | 2.268 |
| structure 2 | Hachinohe II | 0.245 | 1.685 | 0.573 | 2.215 |
| structure 3 | Hachinohe III | 0.283 | 1.637 | 0.585 | 2.157 |
| structure 4 | Hachinohe IV | 0.113 | 1.797 | 0.193 | 2.194 |
| Average value | 0.188 | 1.740 | 0.395 | 2.209 | |
| structure 1 | Northridge I | 0.119 | 3.355 | 0.285 | 3.564 |
| structure 3 | Northridge II | 0.315 | 3.353 | 0.609 | 3.166 |
| Average value | 0.217 | 3.354 | 0.447 | 3.365 | |
| structure 2 | Synthesized I | 0.188 | 2.814 | 0.317 | 3.394 |
| structure 4 | Synthesized II | 0.189 | 2.887 | 0.345 | 3.167 |
| Average value | 0.189 | 2.851 | 0.331 | 3.281 | |
| Structure | |||||
|---|---|---|---|---|---|
| structure 1 | Hachinohe I/0.227 Northridge I/0.285 | base to | 4.260 | 47.072 | 8.531 |
| Mass Moment of Inertia compatible | 4.561 | 52.388 | 9.134 | ||
| moment equilibrium | 5.499 | 71.276 | 11.011 | ||
| structure 2 | Hachinohe II/0.573 Synthesized I/0.317 | base to | 4.390 | 88.909 | 17.808 |
| Mass Moment of Inertia compatible | 4.530 | 93.979 | 18.377 | ||
| moment equilibrium | 4.988 | 111.653 | 20.234 | ||
| structure 3 | Hachinohe III/0.585 Northridge II/0.609 | base to | 4.510 | 131.806 | 26.846 |
| Mass Moment of Inertia compatible | 4.601 | 136.745 | 27.388 | ||
| moment equilibrium | 4.909 | 154.172 | 29.221 | ||
| Structure 4 | Hachinohe IV/0.193 Synthesized II/0.345 | base to | 4.660 | 191.46 | 38.783 |
| Mass Moment of Inertia compatible | 4.724 | 196.448 | 39.315 | ||
| moment equilibrium | 4.938 | 213.642 | 41.094 |
| Structure | Input Motions/PGA (g) | h (m) | Formulas on k and C of Soil | |
|---|---|---|---|---|
| structure 1 | Hachinohe I/0.227 Northridge I/0.285 | 4.26 | 0.85 (0.75 r) 2.3 (2 r) 4.5 (4 r) | Wolf [1] Richart and Lysmer [2] embedment correction factor–Whitman [6] |
| structure 2 | Hachinohe II/0.573 Synthesized I/0.317 | 4.39 | ||
| structure 3 | Hachinohe III/0.585 Northridge II/0.609 | 4.51 | ||
| structure 4 | Hachinohe IV/0.193 Synthesized II/0.345 | 4.66 |
| Structure | Input Motion | Test | ||||||
|---|---|---|---|---|---|---|---|---|
| Wolf’s Formula | Richart and Lysmer’s Formula | |||||||
| structure 1 | Hachinohe I | 0.685 | 0.885 | 0.785 | 0.694 | 0.941 | 0.812 | 0.707 |
| Northridge I | 1.088 | 1.163 | 1.400 | 1.124 | 1.255 | 1.490 | 1.136 | |
| structure 2 | Hachinohe II | 1.454 | 1.304 | 1.725 | 1.772 | 1.352 | 1.798 | 1.803 |
| Synthesized I | 1.100 | 1.707 | 1.383 | 1.173 | 1.919 | 1.449 | 1.213 | |
| structure 3 | Hachinohe III | 1.039 | 1.189 | 1.088 | 1.149 | 1.221 | 1.108 | 1.180 |
| Northridge II | 1.150 | 0.991 | 1.040 | 1.072 | 0.999 | 1.051 | 1.097 | |
| structure 4 | Hachinohe IV | 0.521 | 0.469 | 0.517 | 0.515 | 0.488 | 0.529 | 0.526 |
| Synthesized II | 0.702 | 0.540 | 0.589 | 0.687 | 0.562 | 0.603 | 0.695 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, H.-U.; Ha, J.-G.; Ko, K.-W.; Kim, D.-S. Optimization of Two Soil–Structure Interaction Parameters Using Dynamic Centrifuge Tests and an Analytical Approach. Sustainability 2020, 12, 7113. https://doi.org/10.3390/su12177113
Kim H-U, Ha J-G, Ko K-W, Kim D-S. Optimization of Two Soil–Structure Interaction Parameters Using Dynamic Centrifuge Tests and an Analytical Approach. Sustainability. 2020; 12(17):7113. https://doi.org/10.3390/su12177113
Chicago/Turabian StyleKim, Hyun-Uk, Jeong-Gon Ha, Kil-Wan Ko, and Dong-Soo Kim. 2020. "Optimization of Two Soil–Structure Interaction Parameters Using Dynamic Centrifuge Tests and an Analytical Approach" Sustainability 12, no. 17: 7113. https://doi.org/10.3390/su12177113
APA StyleKim, H.-U., Ha, J.-G., Ko, K.-W., & Kim, D.-S. (2020). Optimization of Two Soil–Structure Interaction Parameters Using Dynamic Centrifuge Tests and an Analytical Approach. Sustainability, 12(17), 7113. https://doi.org/10.3390/su12177113






