Experimental and Numerical Study on the Characteristics of the Thermal Design of a Large-Area Hot Plate for Nanoimprint Equipment
Abstract
1. Introduction
2. Experimental and Analysis Method
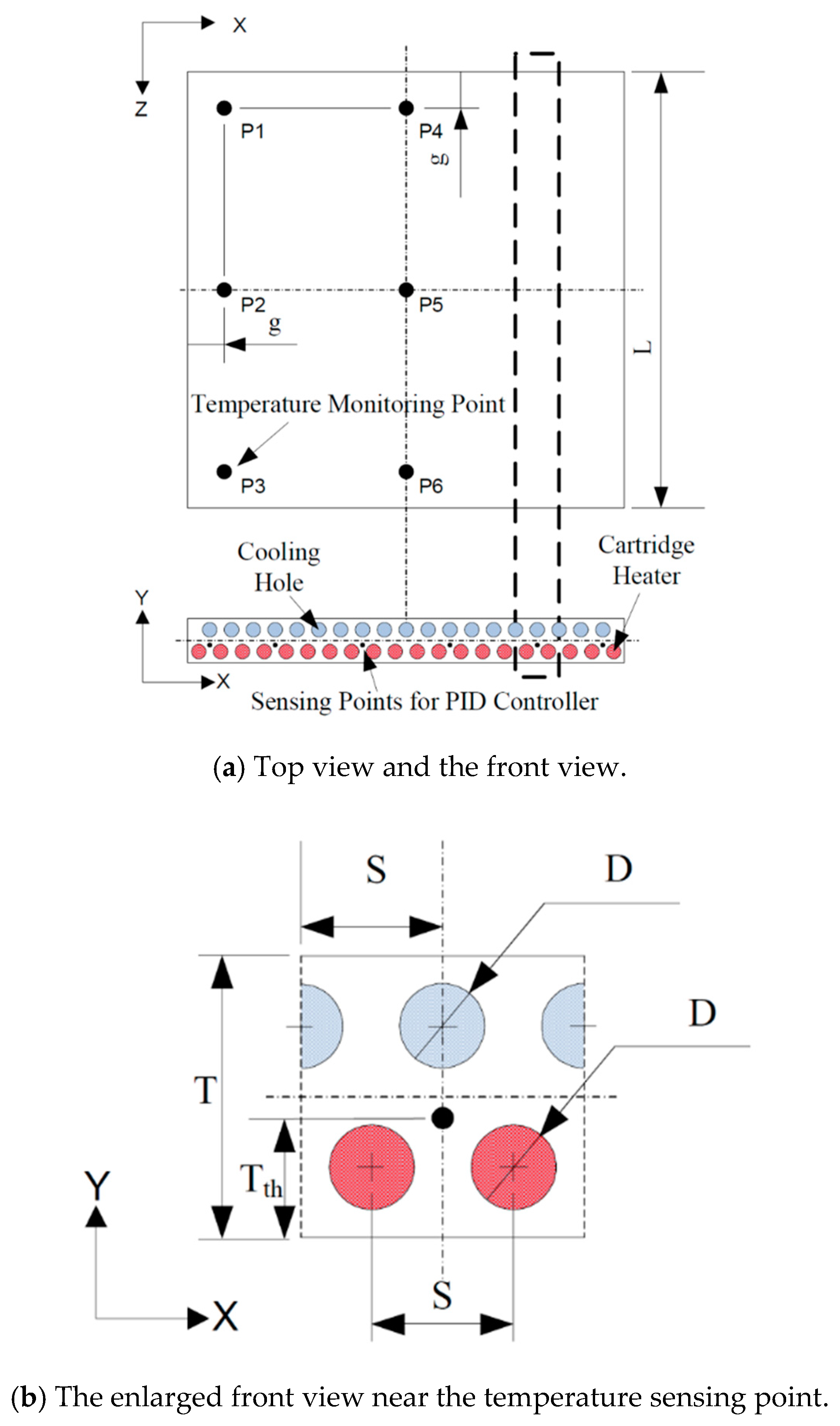
2.1. Experimental Setup and Method
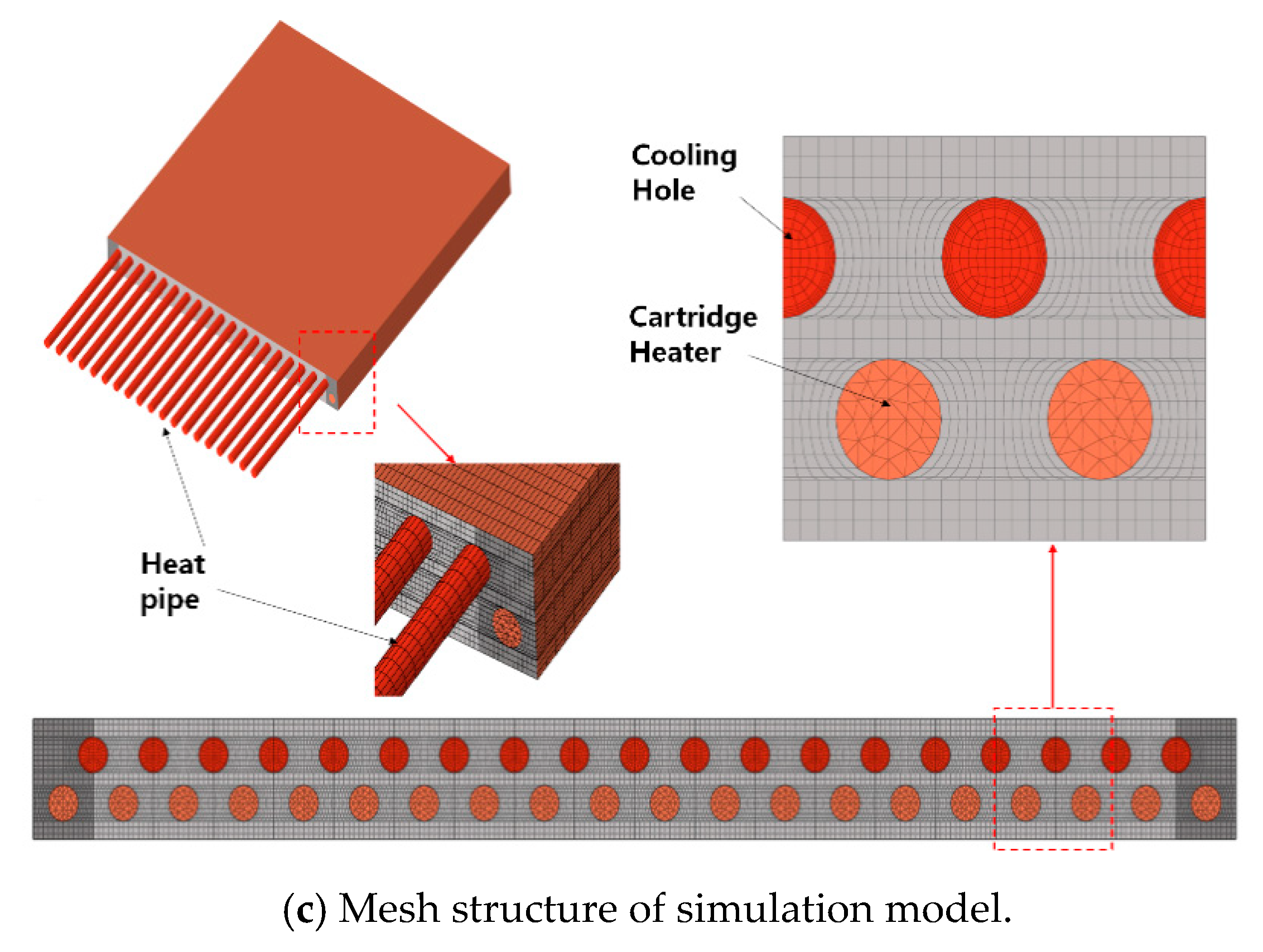
2.2. Numerical Method and Analytical Model
2.3. Control Variables Setting
3. Results and Analysis
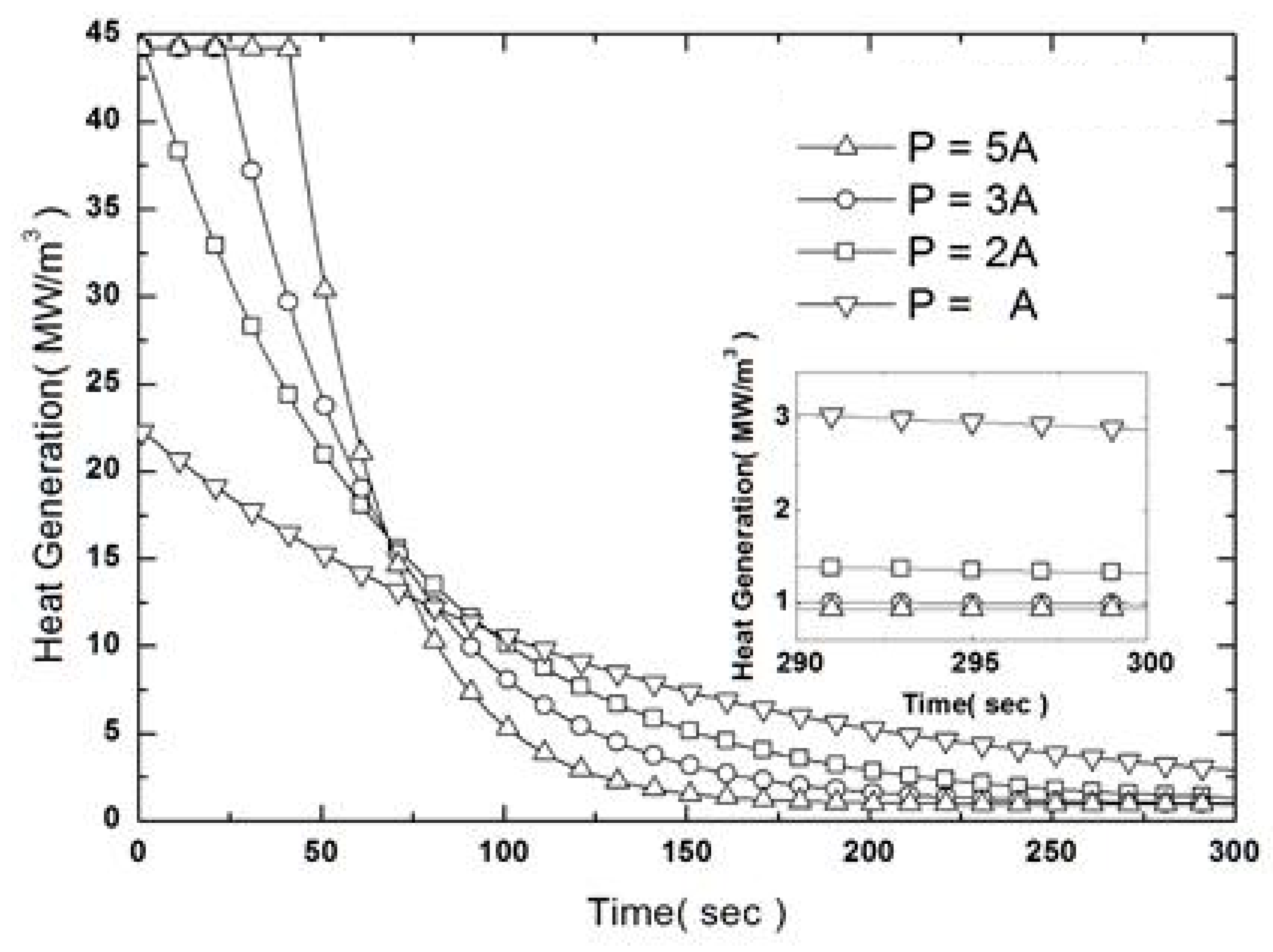
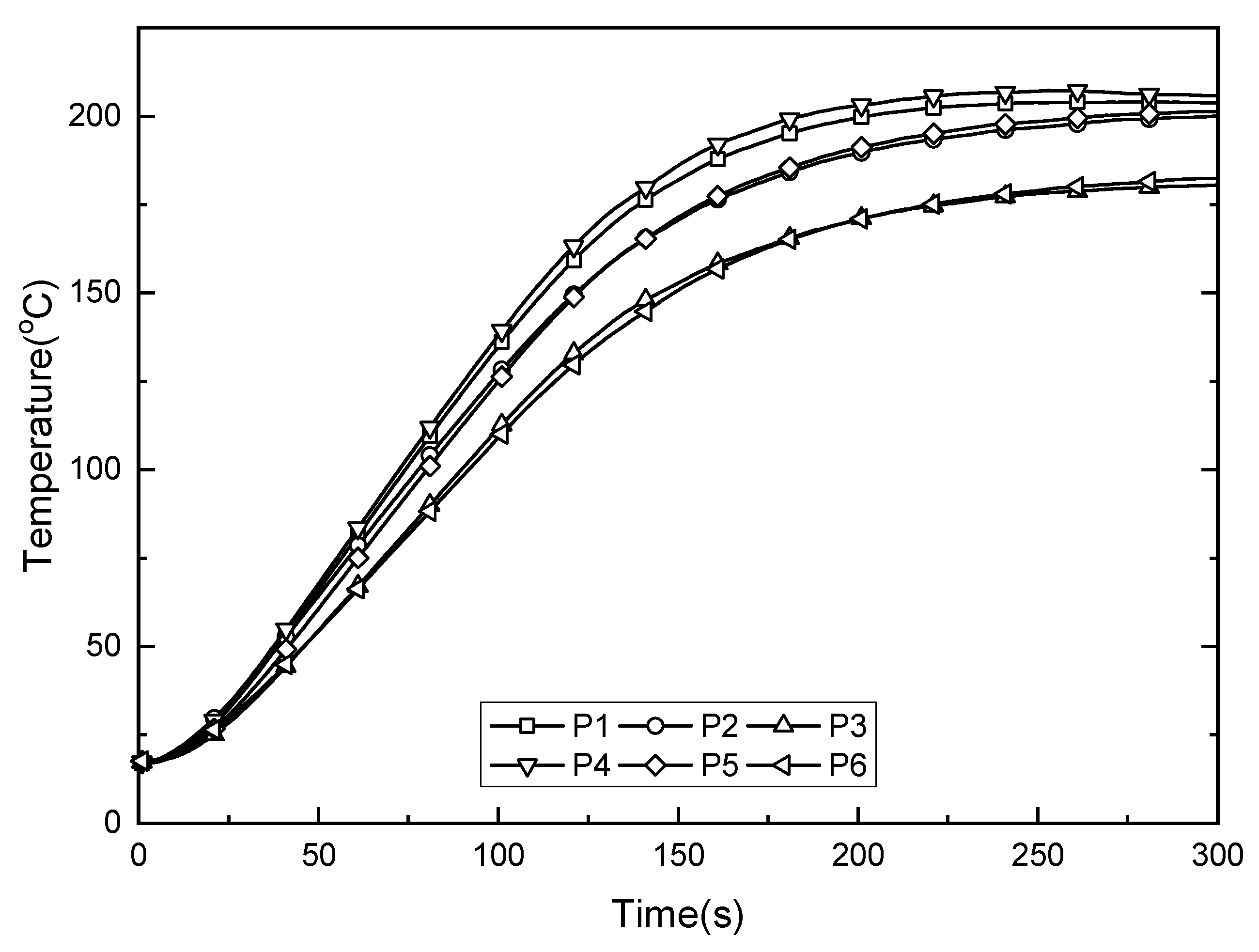
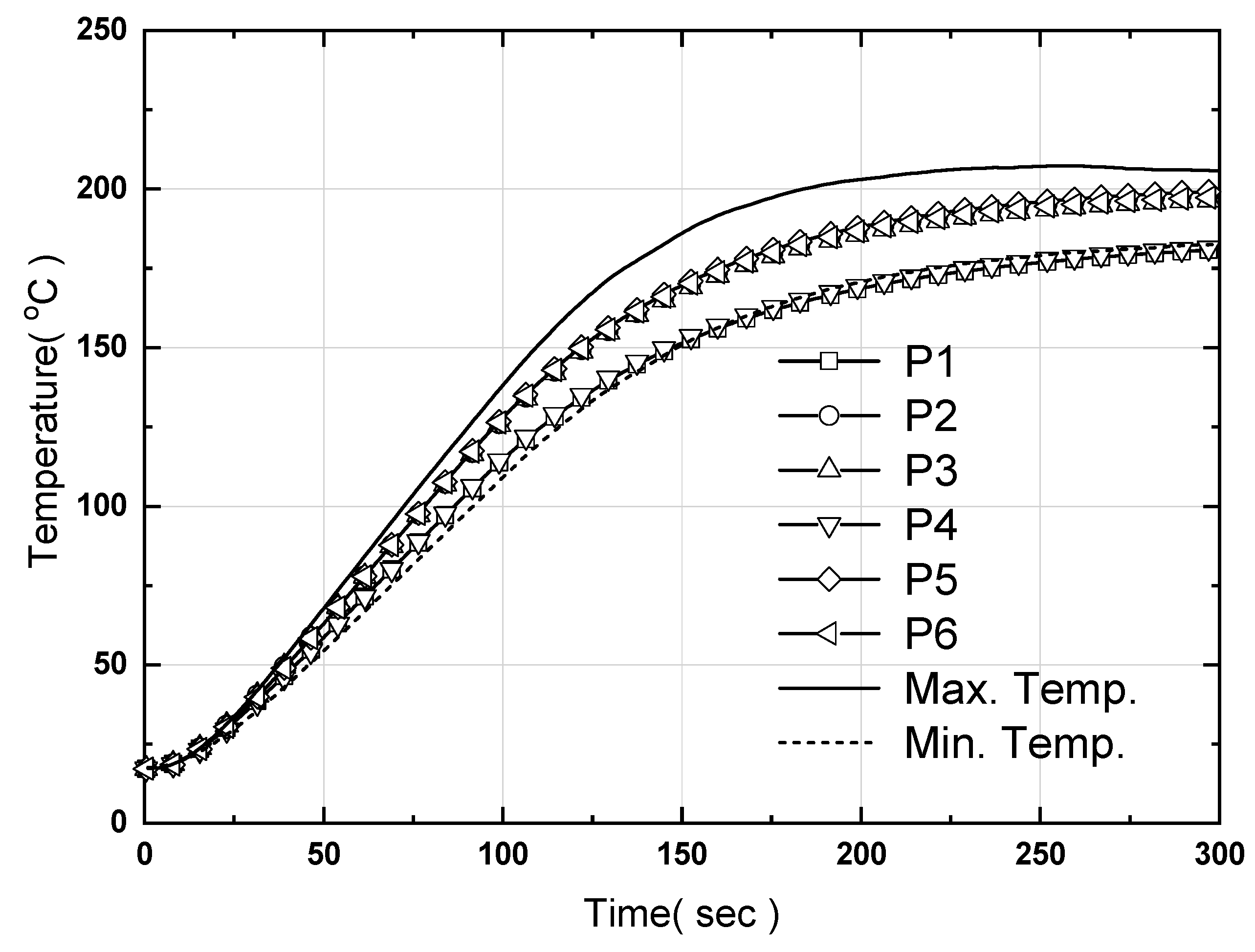
3.1. Heating Numerical Analysis Characteristics
3.2. Cooling Numerical Analysis Characteristics
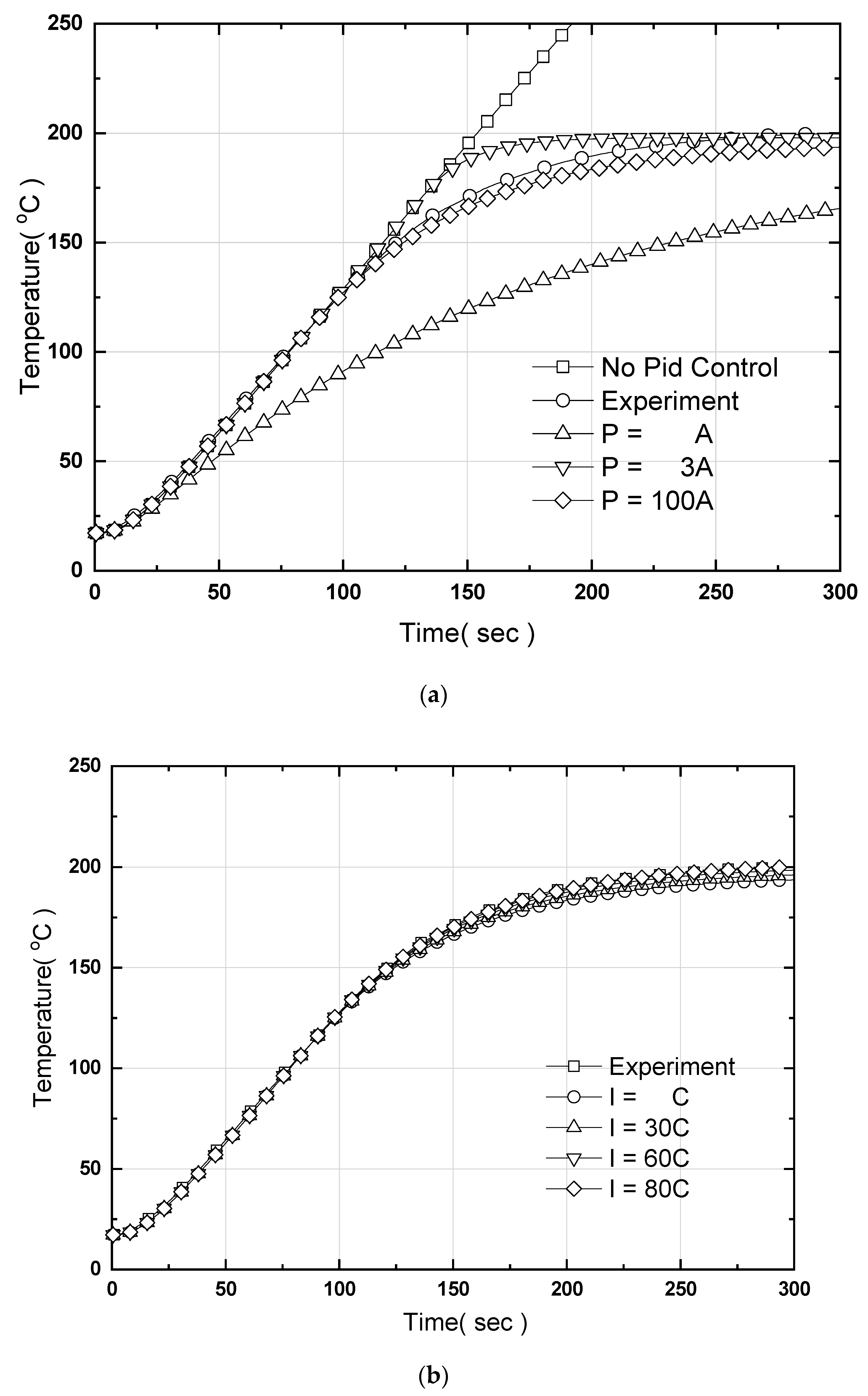
3.3. Comparison of Numerical and Experimental Results
4. Conclusions
- (1)
- Based on the conceptual design, we developed a heating model with a controller to realize the unsteady temperature behavior. The PID algorithm was applied to the controller and its operability was evaluated by changing the values of Pgain, Igain, and Dgain. For cooling, hot plate cooling models incorporating and not incorporating heat pipes were developed in the same manner as the study model. When heat pipes are applied, indirect cooling is performed by cooling their ends.
- (2)
- A computational analysis was performed using a heating model including a controller that applies a stainless steel (STS304) material for the large-area expansion of the hot plate. The results demonstrated that the 2 cm ends of the cartridge heaters were not heated, and the thermal conductivity of the stainless steel was low. Thus, the maximum temperature difference at nine measurement points of the hot plate was 20 °C. For cooling, cooling holes were formed; when using nitrogen and water as the coolants and applying counter-flow, the temperature difference was 20 °C and 32.5 °C, respectively. These results confirmed issues with heating and cooling through conventional methods. To address the above problems, a new heating method was proposed applying heat pipes, and for the cooling method, an indirect cooling method was proposed in which the heat pipe ends are cooled.
- (3)
- When using a stainless steel (STS304) hot plate for large-area hot plate expansion, the heat pipes were inserted in the direction of the cartridge heaters to address the problems that may occur when expanding the hot plate into a large area. As a result, the heating rate was 40 °C/min and the temperature uniformity was less than 1% of the maximum working temperature of 200 °C. For cooling, when considering pressure and using air as the coolant for the ends, a cooling rate of 20 °C/min and thermal performance of less than 13.2 °C (less than 7%) based on the maximum temperature were obtained. These results were similar to the experimental results.
Author Contributions
Funding
Conflicts of Interest
References
- May, G.S.; Spanos, C.J. Fundamentals of Semiconductor Fabrication; John Wily & Sons Publishing: New York, NY, USA, 2003. [Google Scholar]
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Nanoimprint Lithography. J. Vac. Sci. Technol. 1996, 14, 129–4133. [Google Scholar] [CrossRef]
- Becker, H.; Heim, U. Hot Embossing as a Method for the Fabrication of Polymer High Aspect Ratio Structures. Sens. Actuators A Phys. 2000, 84, 130–135. [Google Scholar] [CrossRef]
- Lee, J.; Park, S.; Choi, K.; Kim, G. Nano-scale patterning using the roll typed UVnanoimprint lithography tool. Microelectron. Eng. 2008, 85, 861–865. [Google Scholar] [CrossRef]
- Ahn, S.H.; Guo, L.J. Large-area roll-to-roll and roll-to-plate Nanoimprint Lithography: A step toward high-throughput application of continuous nanoimprinting. ACS Nano. 2009, 3, 2304–2310. [Google Scholar] [CrossRef] [PubMed]
- Yoshikawa, H.; Taniguchi, J.; Tazaki, G.; Zento, T. Fabrication of high-aspect-ratio pattern via high throughput roll-to-roll ultraviolet nanoimprint lithography. Microelectron. Eng. 2013, 112, 273–277. [Google Scholar] [CrossRef]
- Hua, F.; Sun, Y.; Gaur, A.; Meitl, M.A.; Bilhaut, L.; Rotkina, L.; Wang, J.; Geil, P.; Shim, M.; Rogers, J.A.; et al. Polymer imprint lithography with molecular-scale resolution. Nano Lett. 2004, 4, 2467–2471. [Google Scholar] [CrossRef]
- Belligundu, S.; Shiakolas, P.S. Study on two-stage hot embossing microreplication: Silicon to polymer to polymer. J. Microlithogr. Microfabr. Microsyst. 2006, 5, 021103. [Google Scholar] [CrossRef]
- Jaszewski, R.W.; Schift, H.; Schnyder, B.; Schneuwly, A.; Gröning, P. Deposition of anti-adhesive ultra-thin teflon-like films and their interaction with polymers during hot embossing. Appl. Surf. Sci. 1999, 143, 301–308. [Google Scholar] [CrossRef]
- Chou, S.Y.; Krauss, P.R.; Renstrom, P.J. Imprint of sub-25 nm vias and trenches in polymers. Appl. Phy. Lett. 1995, 67, 3114. [Google Scholar] [CrossRef]
- Youn, S.W.; Ogiwara, M.; Goto, H.; Takahashi, M.; Maeda, R. Prototype development of a roller imprint system and its application to large area polymer replication for a microstructured optical device. J. Mater. Process. Technol. 2008, 202, 76–85. [Google Scholar] [CrossRef]
- Xia, Q.; Keimel, C.; Ge, H.; Yu, Z.; Wu, W.; Chou, S.Y. Ultrafast patterning of nanostructures in polymers using laser assisted nanoimprint lithography. Appl. Phy. Lett. 2003, 83, 4417–4419. [Google Scholar] [CrossRef]
- Hsu, Q.C.; Lin, Y.T.; Chou, D.C.; Wu, C.D. Study on Nanoimprint Formability Considering the Anti-adhesion Layer for (CH2) n Polymer by Molecular Dynamics Simulation. Curr. Nanosci. 2012, 8, 424–431. [Google Scholar] [CrossRef]
- Maury, P.; Escalante, M.; Reinhoudt, D.N.; Huskens, J. Directed assembly of nanoparticles onto polymer-imprinted or chemically patterned templates fabricated by nanoimprint lithography. Adv. Mater. 2005, 17, 2718–2723. [Google Scholar] [CrossRef]
- Retolaza, A.; Juarros, A.; Ramiro, J.; Merino, S. Thermal roll to roll nanoimprint lithography for micropillars fabrication on thermoplastics. Microelectron. Eng. 2018, 193, 54–61. [Google Scholar] [CrossRef]
- Andrews, D.L.; Lipson, R.H.; Nann, T.; Mohamed, K. Nanoimprint Lithography for Nanomanufacturing. In Comprehensive Nanoscience and Nanotechnology, 2nd ed.; Academic Press: Cambridge, MA, USA, 2019; pp. 357–386. [Google Scholar]
- Shan, X.; Liu, Y.C.; Lam, C. Studies of polymer deformation and recoveryin micro hot embossing. Microsyst. Technol. 2008, 14, 1055–1060. [Google Scholar] [CrossRef]
- Tan, H.; Gilbertson, A.; Chou, S.Y. Roller nanoimprint lithography. J. Vac. Sci. Technol. B Microelectron. Nanometer Struct. 1998, 16, 3926. [Google Scholar] [CrossRef]
- Striegel, A.; Schneider, M.; Schneider, N.; Benkel, C.; Worgull, M. Seamless tool fabrication for Roll-to-Roll microreplication. Microelectron. Eng. 2018, 194, 8–14. [Google Scholar] [CrossRef]
- Ahn, S.; Cha, J.; Myung, H.; Kim, S.M.; Kang, S. Continuous ultraviolet roll nanoimprinting process for replicating large-scale nano-and micropatterns. Appl. Phys. Lett. 2006, 89, 12–15. [Google Scholar] [CrossRef]
- Moro, M.; Taniguchi, J.; Hiwasa, S. Fabrication of antireflection structure film by roll-to-roll ultraviolet nanoimprint lithography. J. Vac. Sci. Technol. B Nanotechnol. Microelectron. Mater. Process. Meas. Phenom. 2014, 32, 06FG09. [Google Scholar] [CrossRef]
- Chou, S.Y.; Keimel, C.; Gu, J. Ultrafast and direct imprint of nanostructures in silicon. Nature 2002, 417, 835–837. [Google Scholar] [CrossRef]
- Cui, B.; Keimel, C.; Chou, S.Y. Ultrafast direct imprinting of nanostructures in metals by pulsed laser melting. Nanotechnology 2010, 21, 045303. [Google Scholar] [CrossRef] [PubMed]
- Moore, S.; Gomez, J.; Lek, D.; You, B.H.; Kim, N.; Song, I.H. Experimental study of polymer microlens fabrication using partial-filling hot embossing technique. Microelectron. Eng. 2016, 162, 57–62. [Google Scholar] [CrossRef]
- Pang, S.W.; Tamamura, T.; Nakao, M.; Ozawa, A.; Masuda, H. Direct Nano-Printing on Al Substrate Using a SiC Mold. J. Vac. Sci. Technol. 1998, 16, 1145–1149. [Google Scholar] [CrossRef]
- Hirai, Y.; Fujiwara, M.; Okuno, T.; Tanaka, Y.; Endo, M.; Irie, S.; Nakagawa, K.; Sasago, M. Study of the Resist Deformation in Nanoimprint Lithography. J. Vac. Sci. Technol. 2001, 19, 2811–2815. [Google Scholar] [CrossRef]
- Beck, M.; Graczyk, M.; Maximov, I.; Sarwe, E.L.; Ling, T.G.I.; Keil, M.; Montelius, L. Motelius, Improving Stam4ps or 10 nm Level Wafer Scale Nanoimprint Lithography. Microelectron. Eng. 2002, 61, 441–448. [Google Scholar] [CrossRef]
- Khang, D.Y.; Kang, H.; Kim, T.I.; Lee, H.H. Low-Pressure Nanoimprint Lithography. Nano Lett. 2004, 4, 633–637. [Google Scholar] [CrossRef]
- Kwak, H.S.; Park, G.J.; Son, B.C.; Lee, J.J.; Park, H.C. Design of a Hot plate with Rapid Cooling Capability or Thermal Nanoimprint Lithography. In Proceedings of the SICE-ICASE International Joint Conference, Busan, Korea, 18–21 October 2006; pp. 4897–4901. [Google Scholar]
- Park, G.J.; Kwak, H.S.; Shin, D.W.; Lee, J.J. Numerical Simulation of Thermal Control of a Hot Plate for Thermal Nanoimprint Lithography Machines. In Proceedings of the 3rd International Conference on Heating Cooling Technol, Seoul, Korea, 4 March 2007; pp. 321–327. [Google Scholar]
- Yang, J.H. An Experimental Study on the Thermal Performance of a Hot Plate for Thermal Nanoimprint Lithography. Master’s Thesis, Kumoh National Institute of Technology, Gumi, Korea, 2008. [Google Scholar]
- Faghri, A. Heatpipe Science and Technology; Taylor & Francis Press: Abingdon, UK, 1995. [Google Scholar]
- Wallin, P. Heat Pipe: Selection of Working Fluid; Heat and Mass Trasfer Project Report; MVK160: Lund, Sweden, 7 May 2012; pp. 1–7. [Google Scholar]
- Cengel, Y.A. Heat and Mass Transfer; McGraw-Hill Press: New York, NY, USA, 2012; pp. 521–527. [Google Scholar]
- Yakhot, V.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziale, C.G. Development of turbulence models for shear flows by a double expansion technique. Phys. Fluids A 1992, 4, 1510–1520. [Google Scholar] [CrossRef]
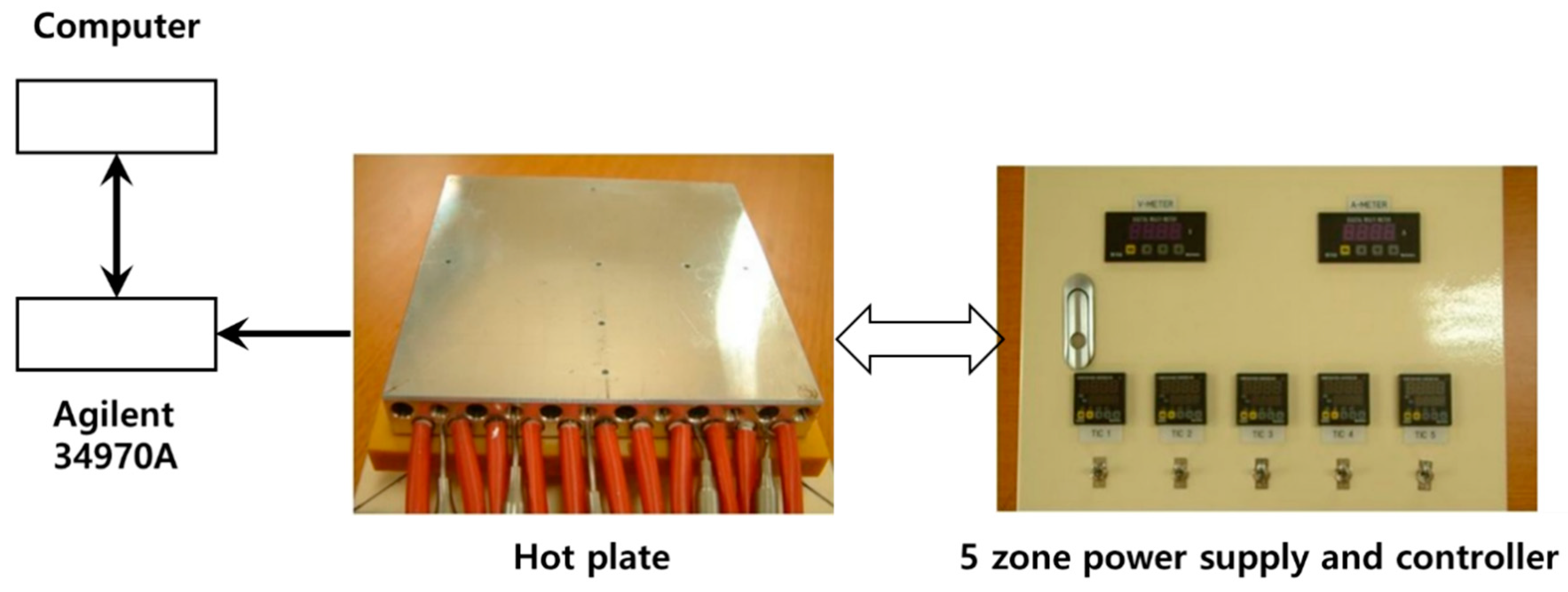



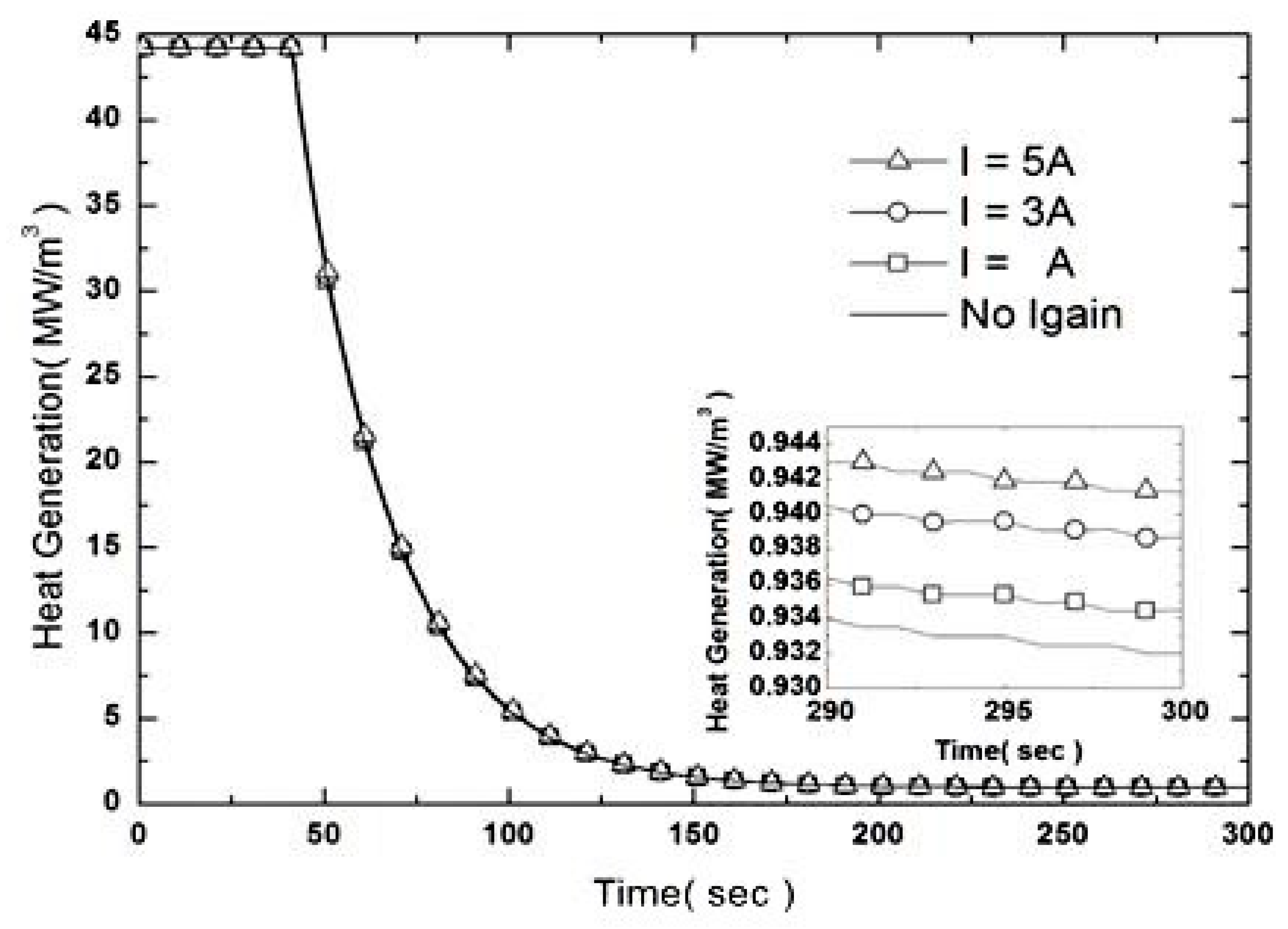
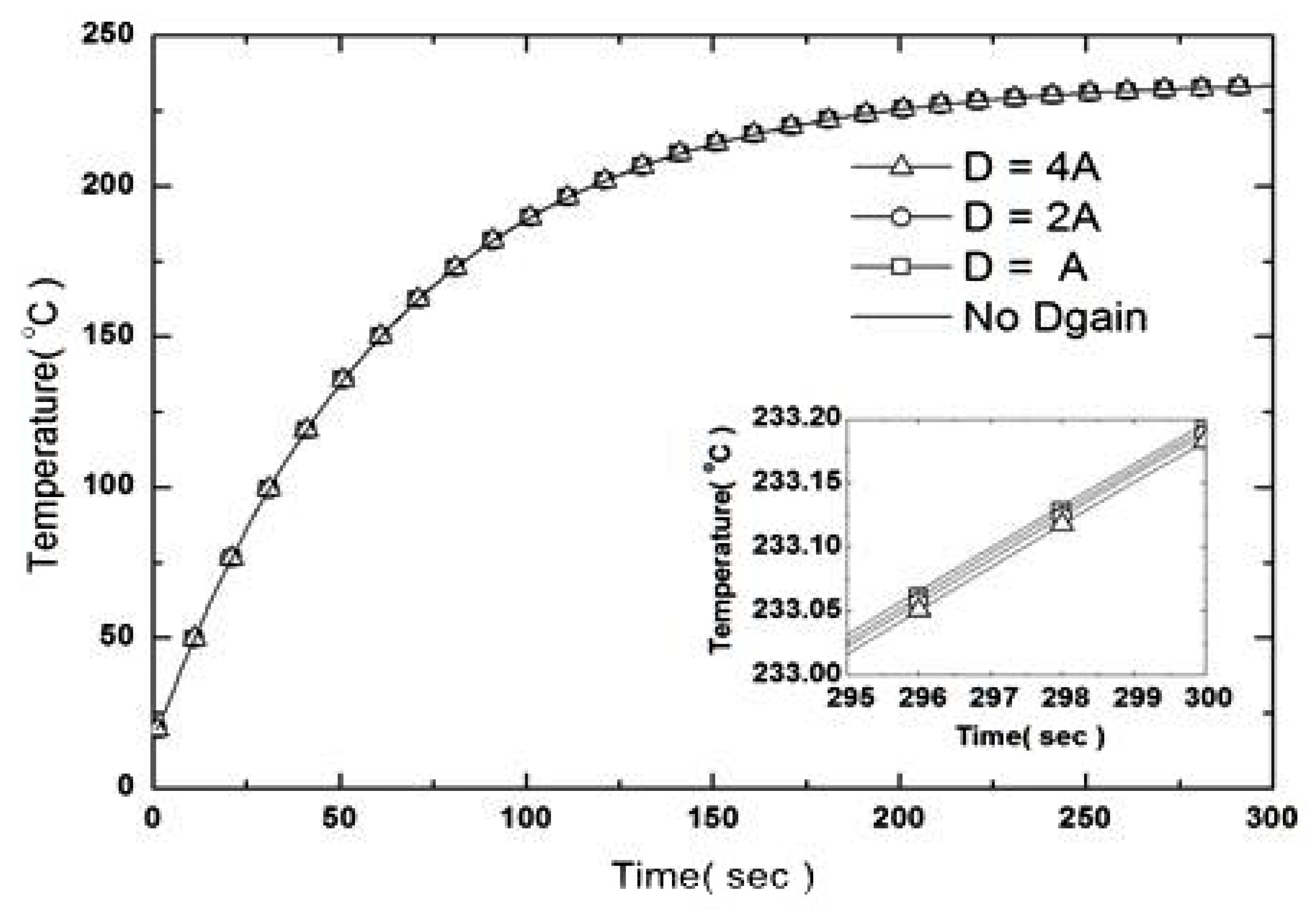
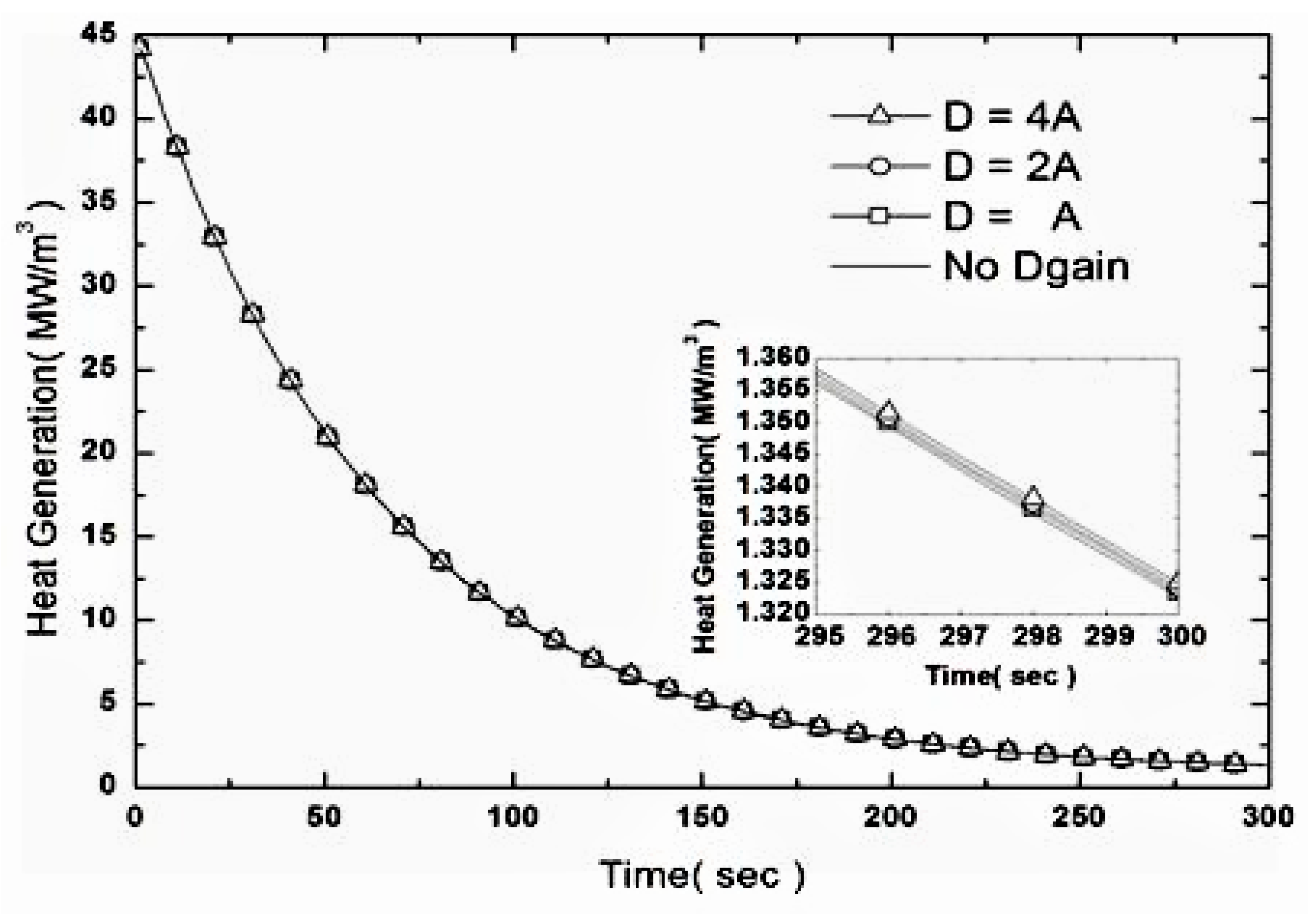

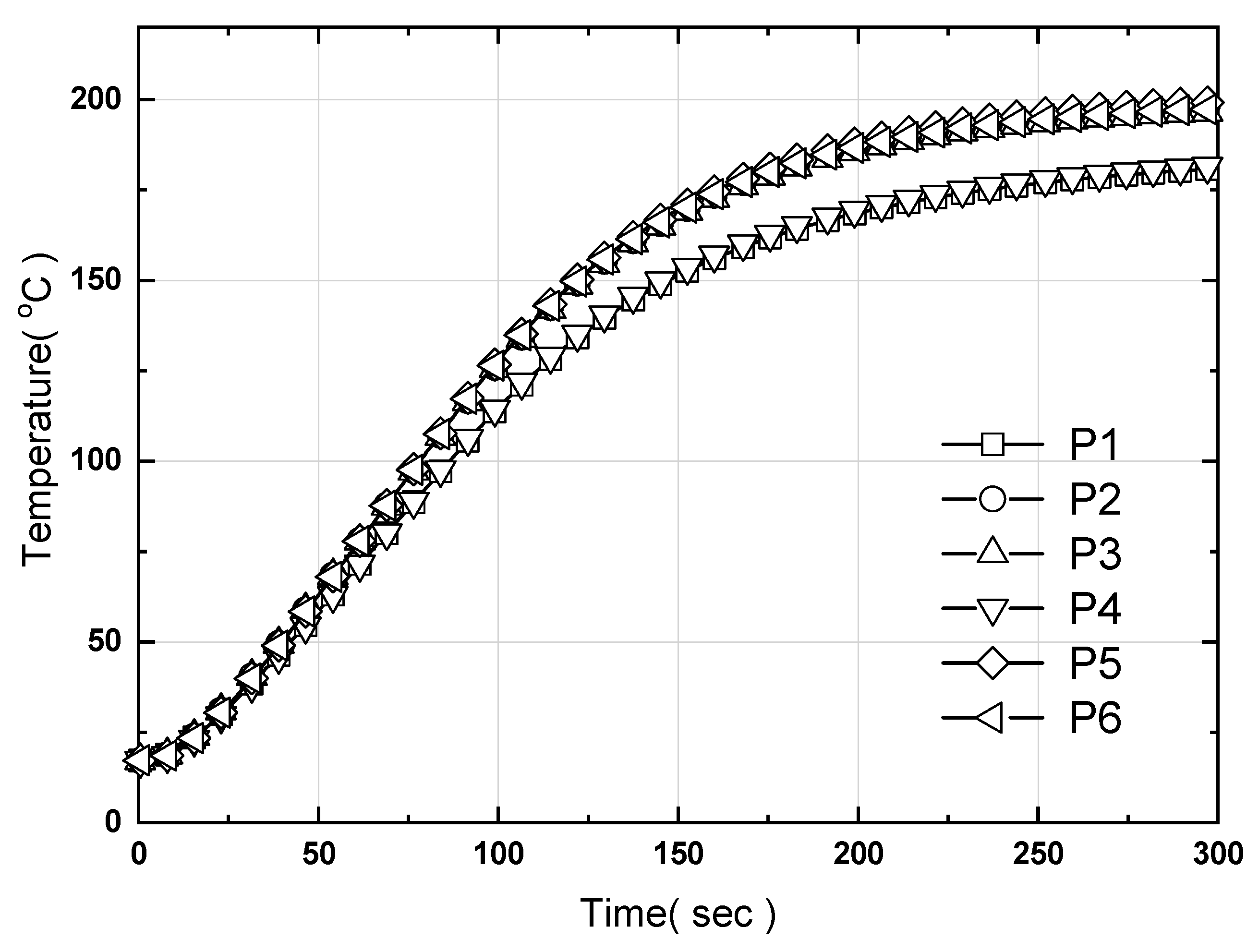
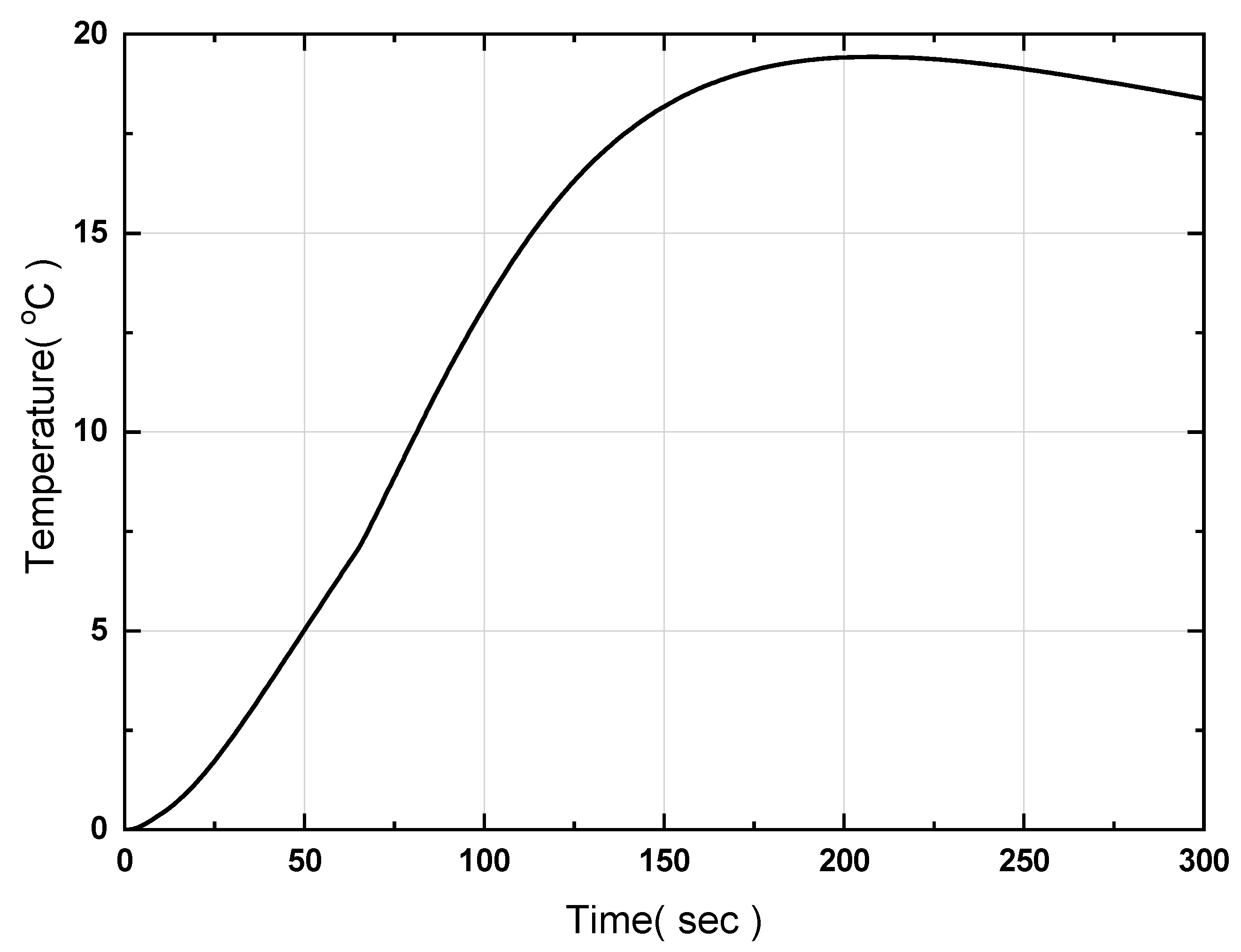

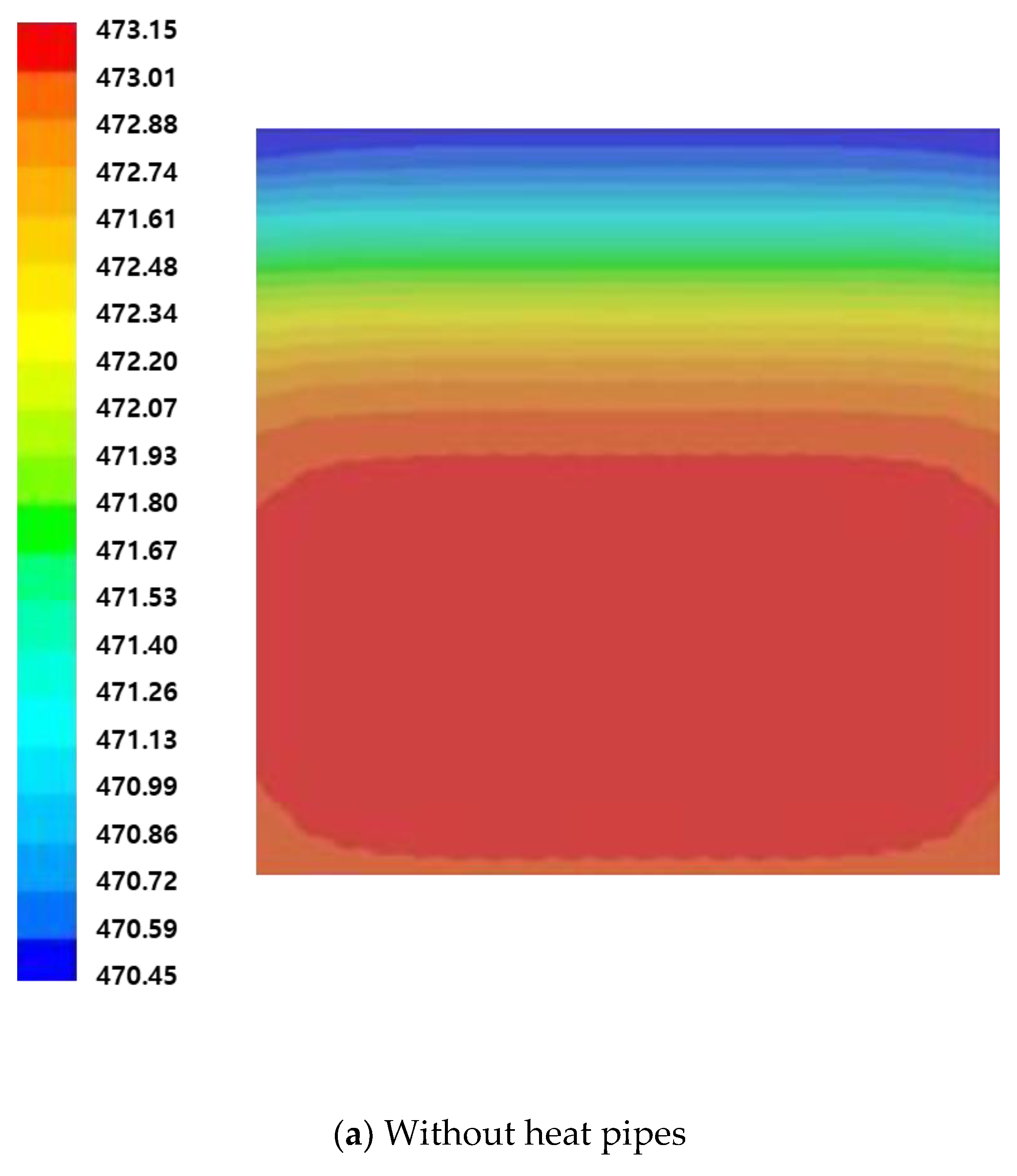
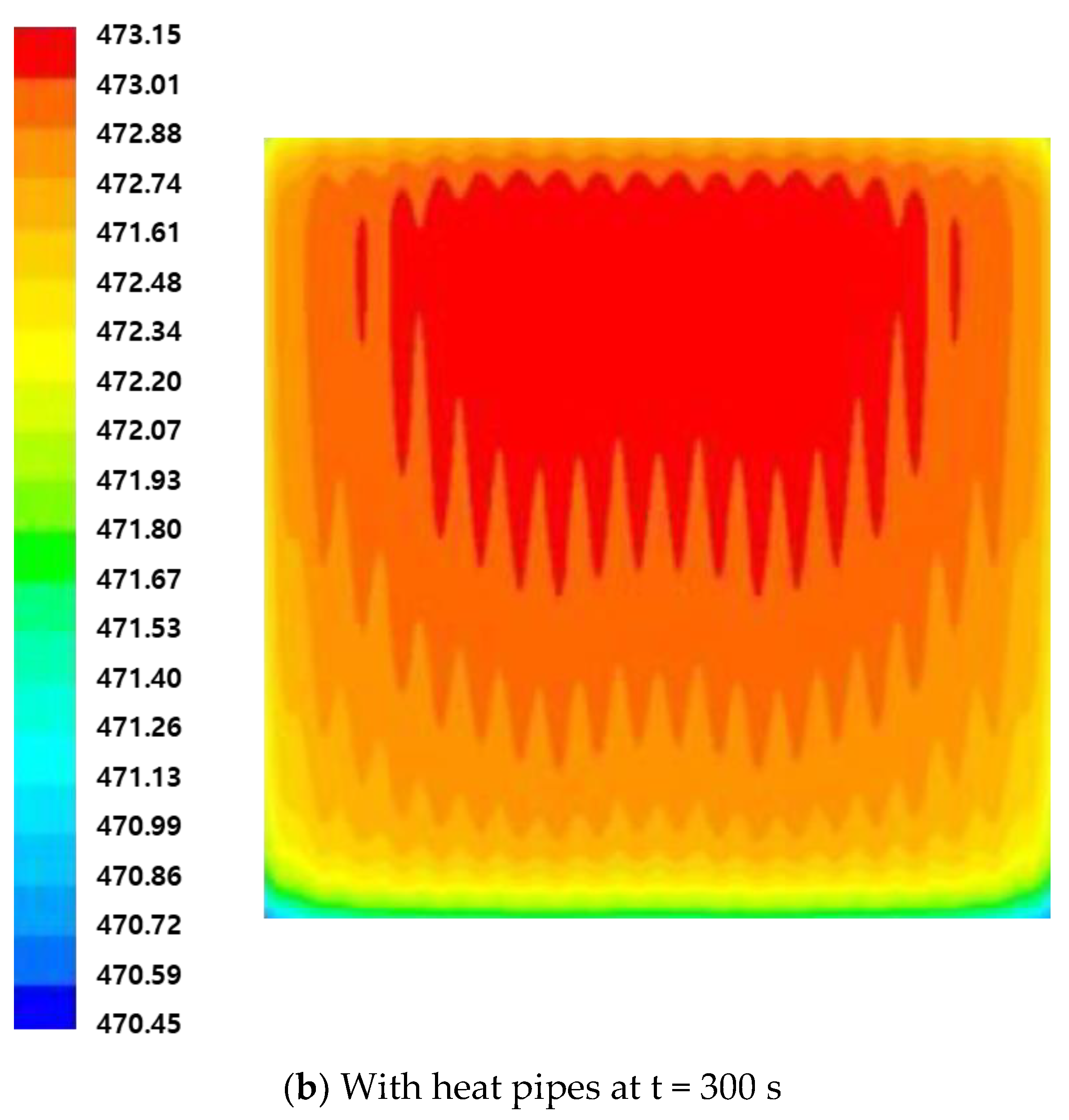

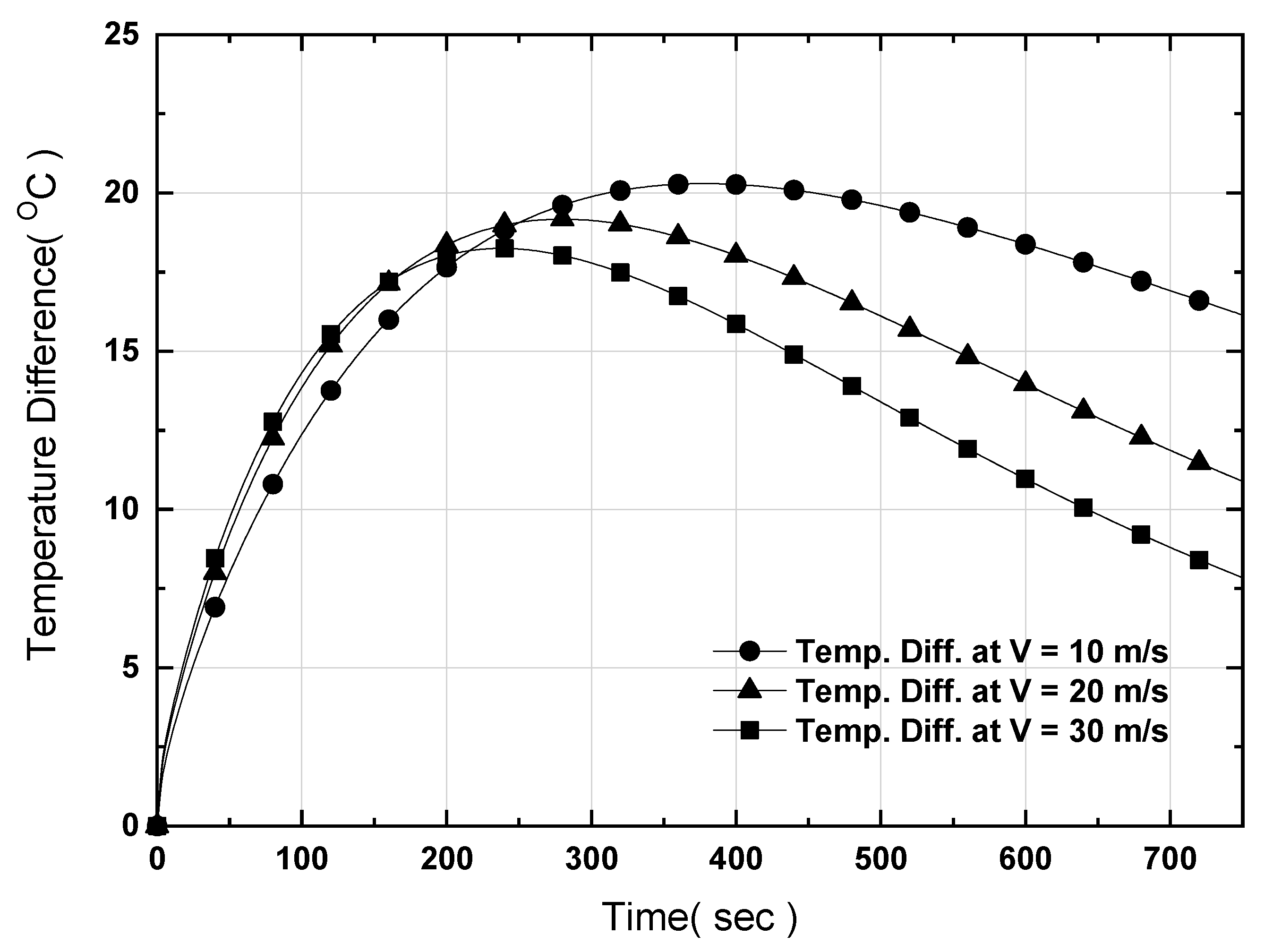



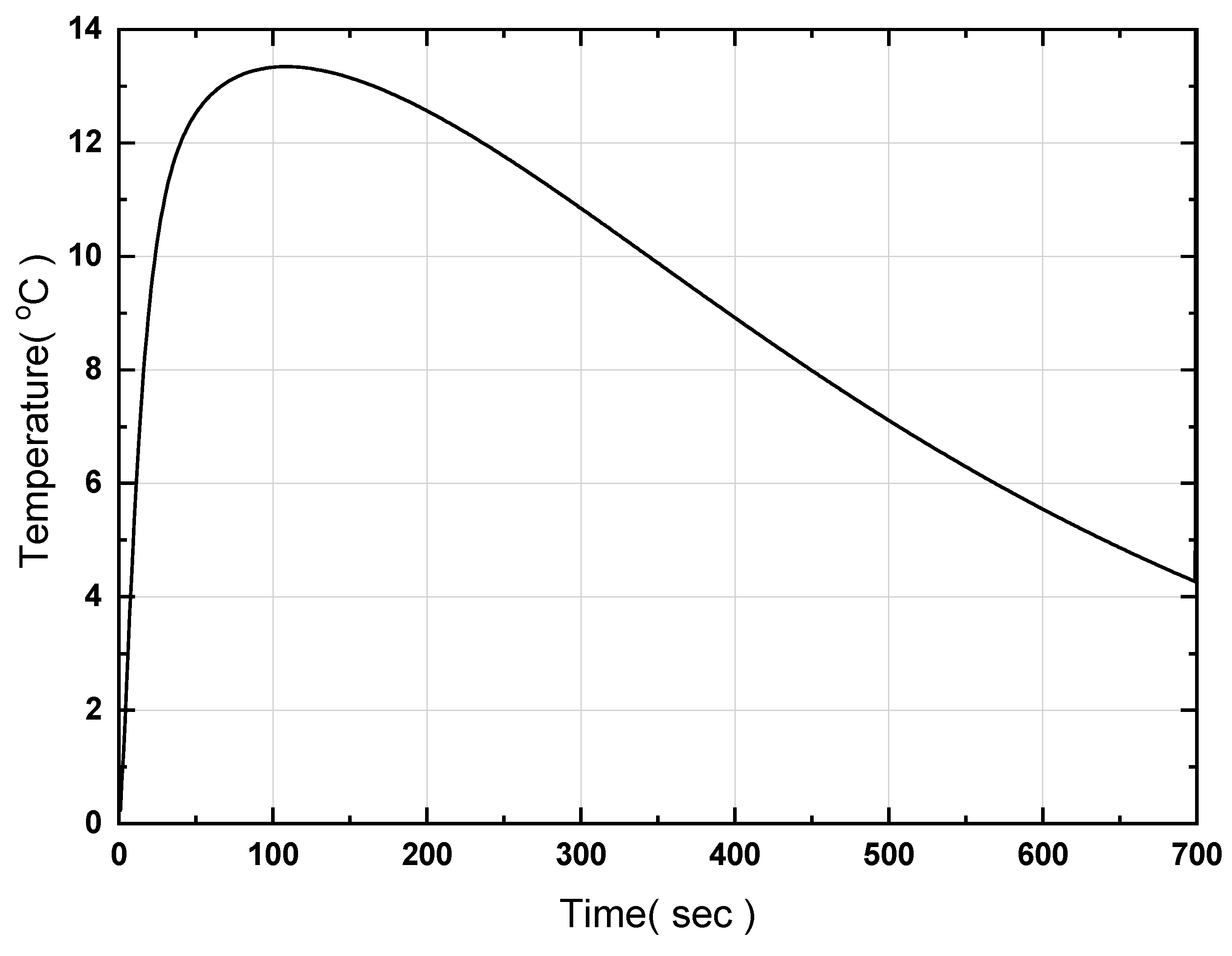

| Area | Temperature difference Max. Temp.–Min. Temp. | Working area (cm2) |
| A | 13.3 | 200 × 200 |
| B | 11.0 | 190 × 190 |
| C | 9.0 | 180 × 180 |
| D | 1.0 | 170 × 170 |
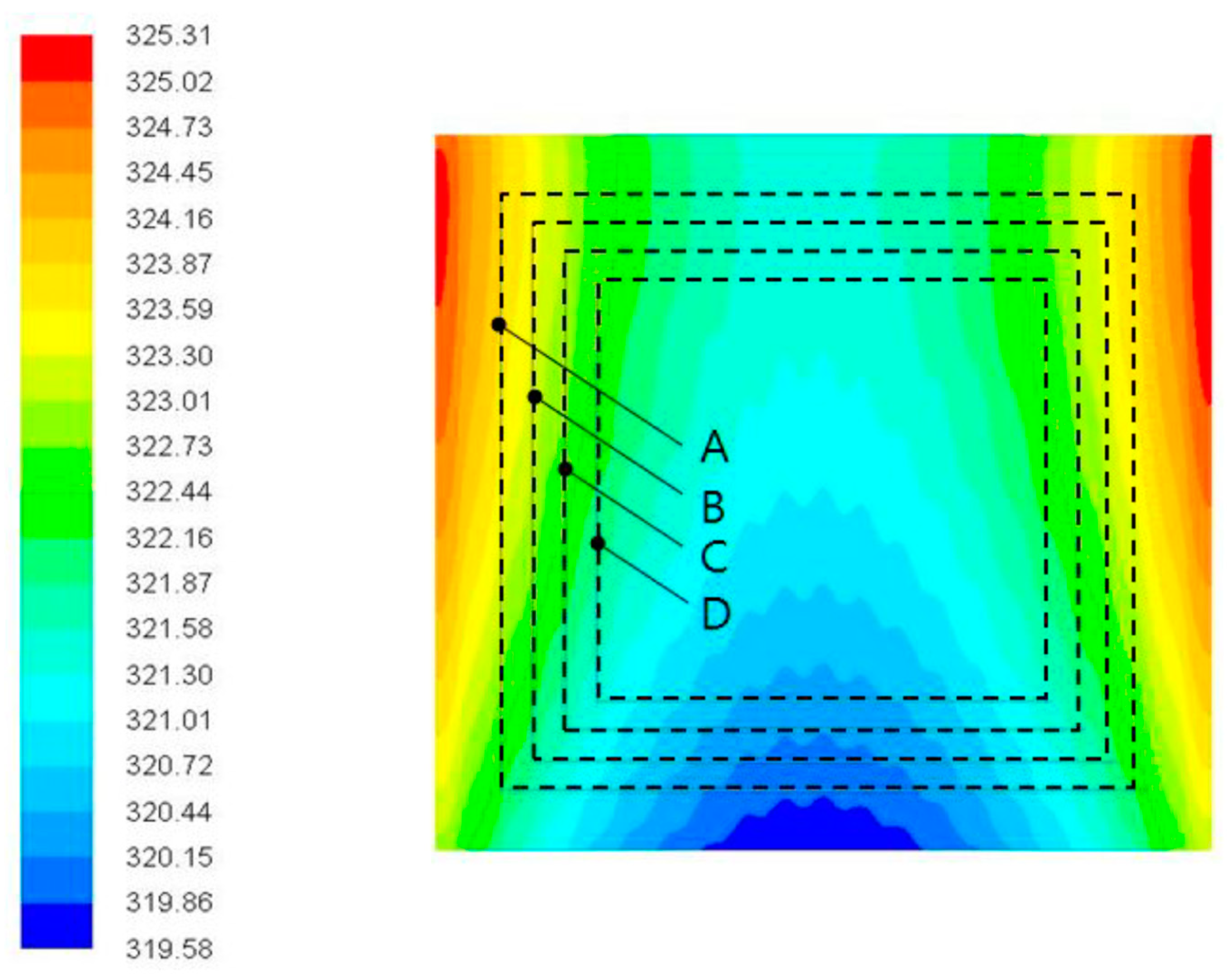
| Area | Temperature difference Max. Temp.–Min. Temp. | Working area (cm2) |
| A | 4.1 | 200 × 200 |
| B | 3.5 | 190 × 190 |
| C | 2.9 | 180 × 180 |
| D | 1.3 | 170 × 170 |



| NIL Method | Process Details | Mold/Substrate | Resist Materials |
|---|---|---|---|
| Thermal NIL [19,20] | Thermal annealing of polymers at temperatures up to 50 °C above the glass transition temperature. | High hardness molds (Young’s modulus should be higher than that of resist): silicon, glasses, quartz, nickel, ceramics, Al oxide Note: thermal expansion coefficient of mold and substrate should match | Only thermoplastic polymers: polystyrene (PS), poly(methyl-methacrylate) (PMMA), polycarbonate (PC), polyethylene terephthalate (PET), siloxane copolymers (PDMS-b-PS, PDMS-g-PMMA), specified spin-on polymers |
| UV NIL at room temperature [4,5,6,21,22] | UV, EUV exposure | UV-transparent materials: quartz glass; soft stamps are more common for UV NIL: polydimethylsiloxane (PDMS), polyvinyl chloride (PVC), PMMA | Low viscosity UV-sensitive materials, ideally with low volume shrinkage after polymerization—usually liquid functionalized monomers or oligomers, CARs (Chemical amplified resists) |
| UV NIL + thermal annealing [23,24] | Simultaneous UV exposure and substrate heating | UV-transparent materials | UV-curable polymers with better surface coverage and lower imprint temperatures as for T-NIL can be used |
| Items | Model | Specifications | Remarks |
|---|---|---|---|
| Data acquisition system | Agilent 34970A | Two 8-bit digital I/O ports, 26-bit Event Counter, Two 16-bit Analog outputs | Data logger of temperature |
| Power supply | WYG 1C 20Z4 | 90~240 VAC | 8.3 ± 1 ms (response time) |
| Feedback controller | TZN4S | PID (Proportional integral derivative) control | Temperature controller |
| Temperature sensor | K-type thermocouple | 2.0 mm diameter | ±1.5 °C |
| Mark | Length [mm] |
|---|---|
| G | 20 |
| L | 240 |
| Lhp | 340 |
| S | 12 |
| Dhp | 6 |
| Dch | 6 |
| H | 20 |
| Hth | 8 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, G.; Lee, C. Experimental and Numerical Study on the Characteristics of the Thermal Design of a Large-Area Hot Plate for Nanoimprint Equipment. Sustainability 2019, 11, 4795. https://doi.org/10.3390/su11174795
Park G, Lee C. Experimental and Numerical Study on the Characteristics of the Thermal Design of a Large-Area Hot Plate for Nanoimprint Equipment. Sustainability. 2019; 11(17):4795. https://doi.org/10.3390/su11174795
Chicago/Turabian StylePark, Gyujin, and Changhee Lee. 2019. "Experimental and Numerical Study on the Characteristics of the Thermal Design of a Large-Area Hot Plate for Nanoimprint Equipment" Sustainability 11, no. 17: 4795. https://doi.org/10.3390/su11174795
APA StylePark, G., & Lee, C. (2019). Experimental and Numerical Study on the Characteristics of the Thermal Design of a Large-Area Hot Plate for Nanoimprint Equipment. Sustainability, 11(17), 4795. https://doi.org/10.3390/su11174795





