State-of-Charge Effects on Standalone Solar-Storage Systems in Hot Climates: A Case Study in Saudi Arabia
Abstract
1. Introduction
2. Review and Motivation
3. Methodology
3.1. Financial Assumptions
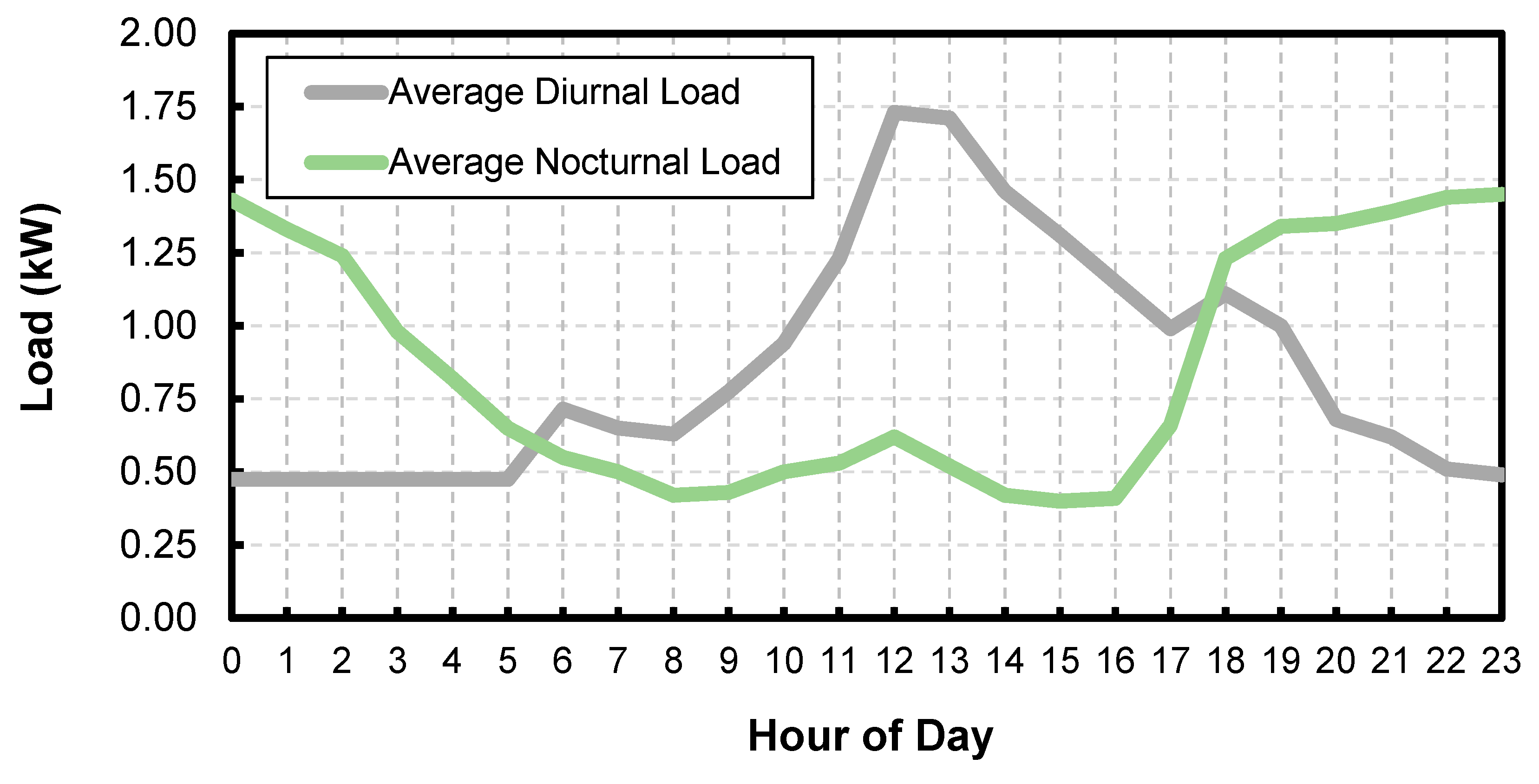
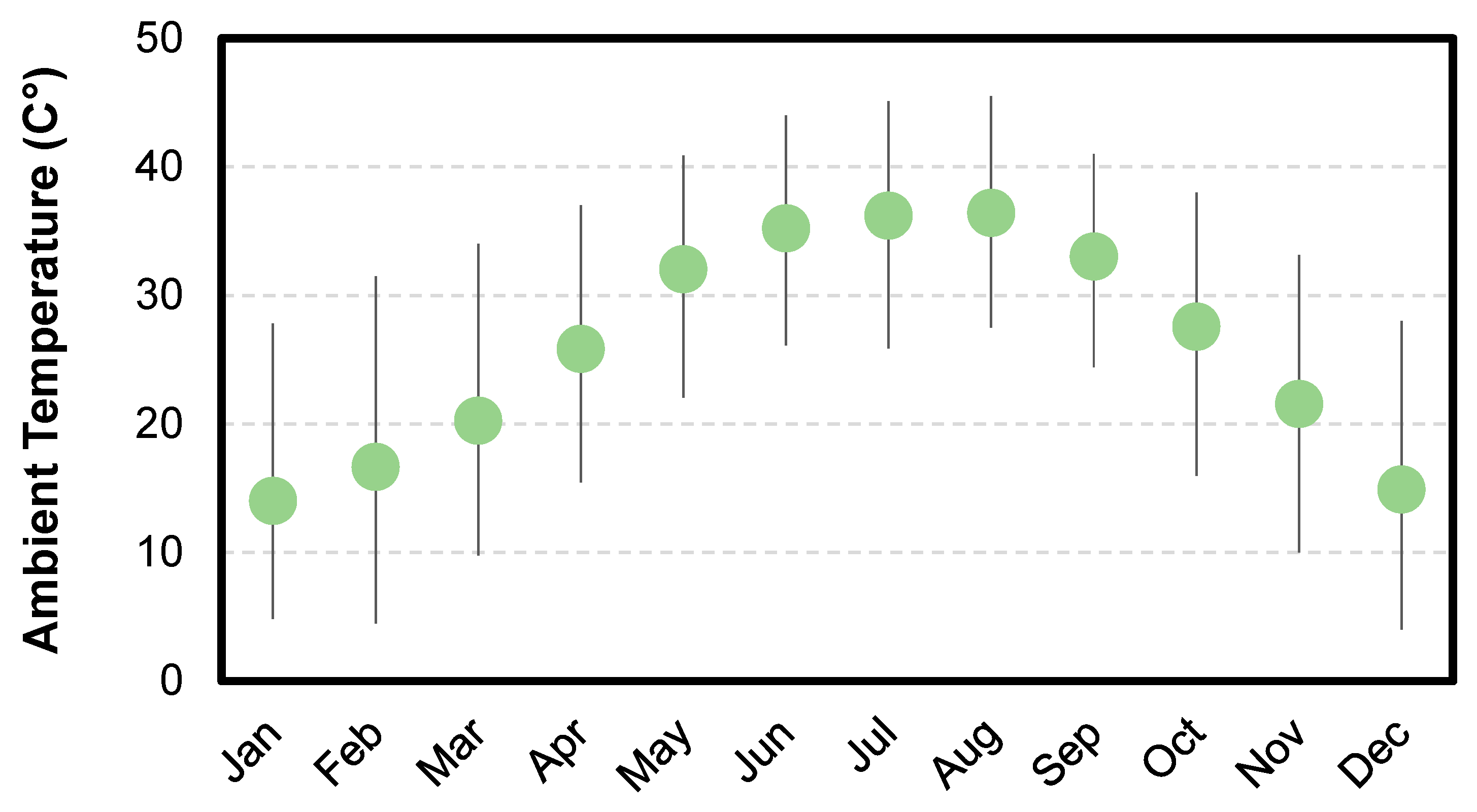
3.2. Load, Irradiation, and Temperature Profiles
3.3. Technical Assumptions
3.3.1. Overall System Assumptions
3.3.2. PV Assumptions
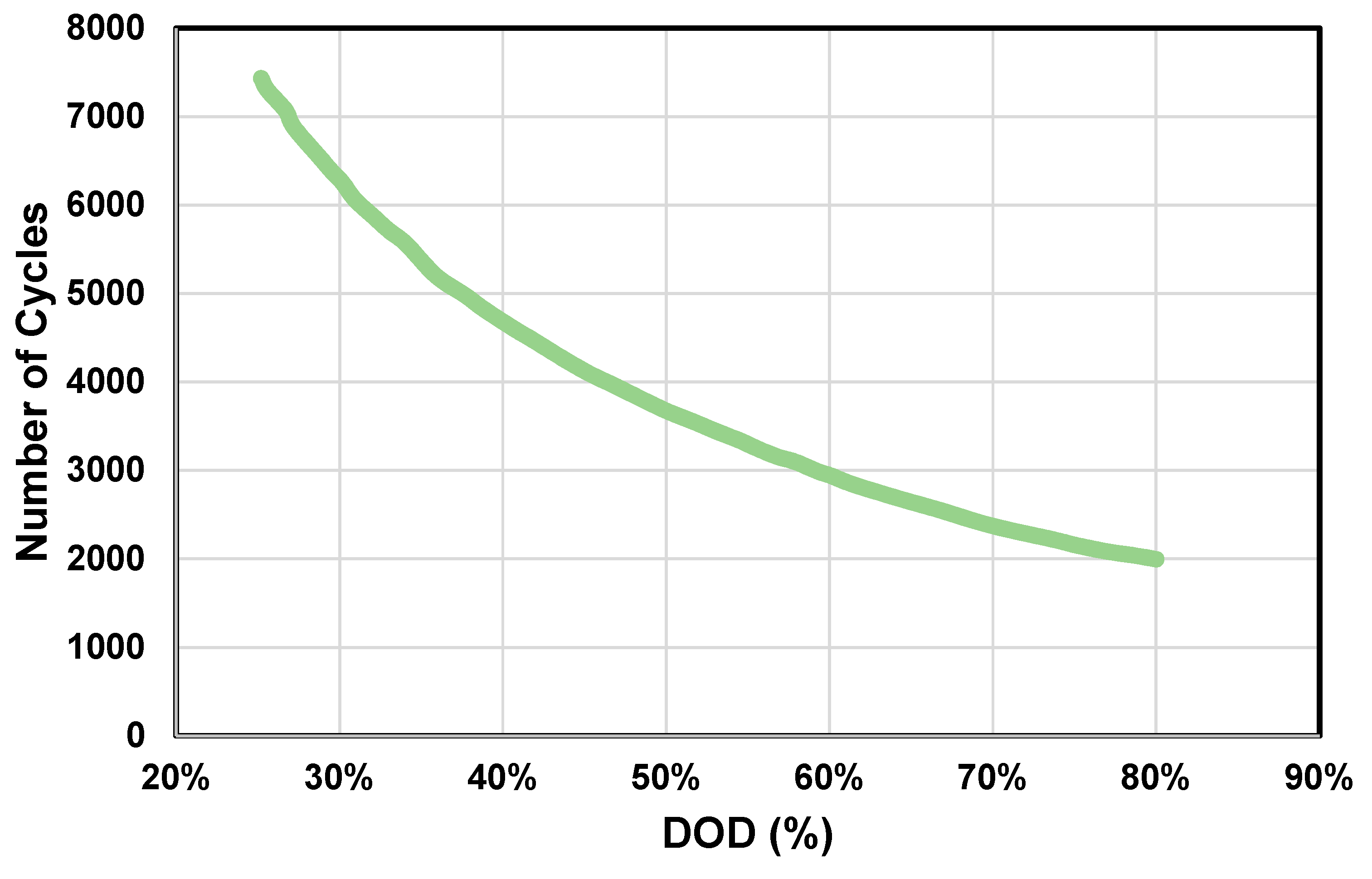
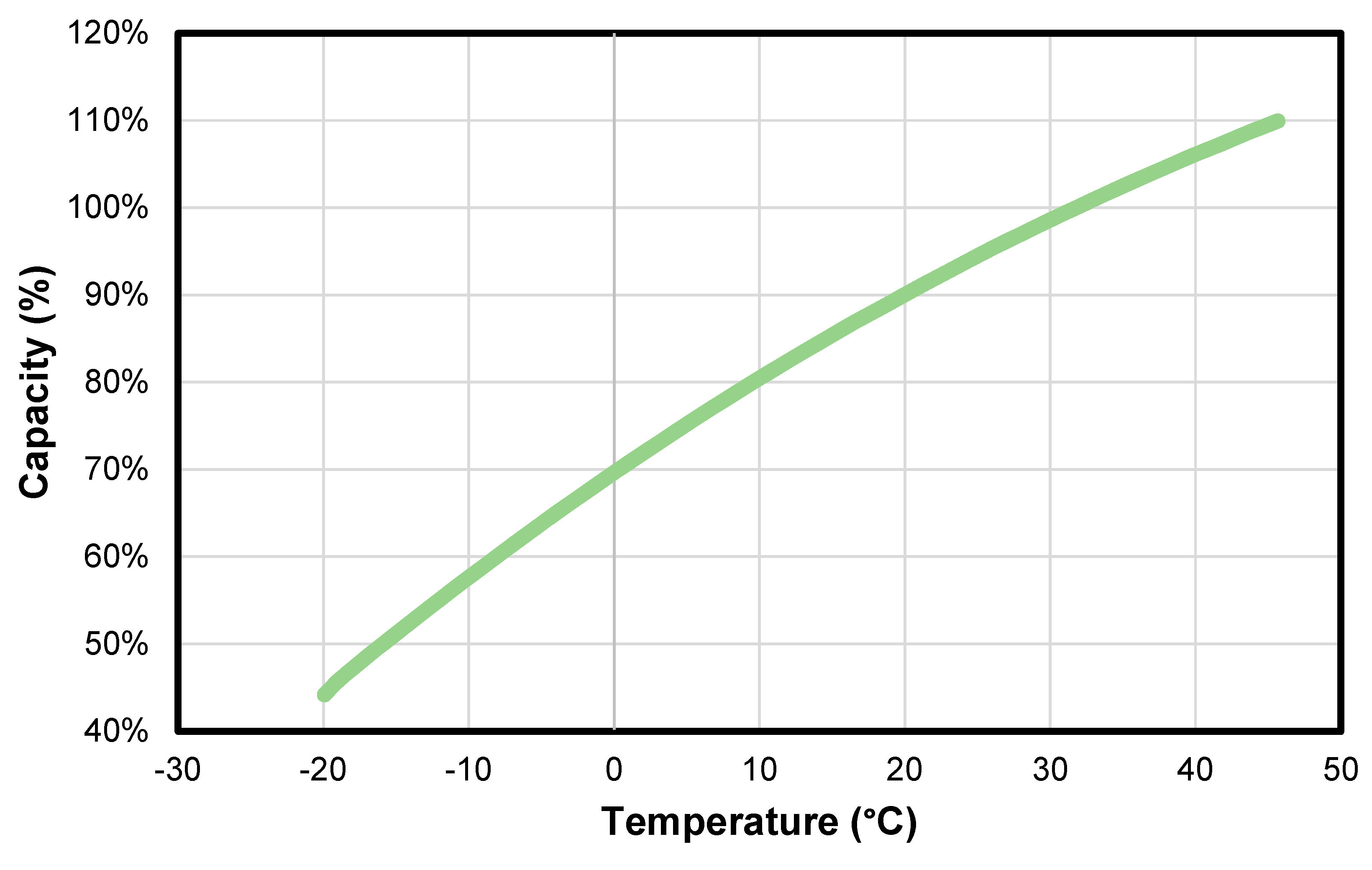
3.3.3. Battery Assumptions
4. Results and Discussion
4.1. Diurnal Load Results
4.2. Nocturnal Load Results
5. Discussion and Policy Implications
5.1. Nocturnal Load Results
5.2. The Diesel Alternative
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Disclaimer
Appendix A
| Appliance/Load | Quantity | AC Watts | Hours On per Day | Wh/Day |
|---|---|---|---|---|
| Coffee maker | 1 | 800 | 0.2 | 160 |
| Microwave | 1 | 1300 | 0.15 | 195 |
| Iron | 1 | 900 | 0.25 | 225 |
| TV | 1 | 130 | 4 | 520 |
| Dishwasher | 1 | 1500 | 0.4 | 600 |
| Washing machine | 1 | 500 | 0.4 | 200 |
| Vacuum cleaner | 1 | 700 | 0.25 | 175 |
| Air conditioner (Room) | 1 | 1500 | 10 | 15,000 |
| Ceiling fan (AC) | 2 | 50 | 5 | 500 |
| Laptop | 1 | 50 | 3 | 150 |
| Lights (25 W) | 8 | 25 | 6 | 1200 |
| Refrigerator (22 ft3) | 1 | 68 | 24 | 1632 |
| Toaster | 1 | 1300 | 0.08 | 104 |
| Blender | 1 | 300 | 0.08 | 24 |
| Total | 20,685 | |||
References
- Rehman, S.; Al-Hadhrami, L. Study of a solar pv-diesel-battery hybrid power system for a remotely located population near Rafha, Saudi Arabia. Energy 2010, 35, 4986–4995. [Google Scholar] [CrossRef]
- Rehman, S.; El-Amin, I. Study of a solar pv/wind/diesel hybrid power system for a remotely located population near Arar, Saudi Arabia. Energy Explor. Exploit. 2015, 33, 591–620. [Google Scholar] [CrossRef]
- Renewable Energy Network for the 21st Century (REN21). Renewable 2017 Global Status Report; REN21: Paris, France, 2017. [Google Scholar]
- Eckhouse, B. Battery Storage Still Needs Solar for Growth. Available online: https://www.bloomberg.com/news/articles/2017-09-21/-new-and-cool-battery-storage-still-leans-on-solar-for-growth (accessed on 16 November 2018).
- Ralon, P.; Taylor, M.; Ilas, A. Electricity Storage and Renewables: Cost and Markets to 2030; IRENA: Abu Dhabi, UAE, 2017. [Google Scholar]
- Curry, C. Lithium-Ion Battery Costs: Squeezed Margins and New Business Models. Available online: https://about.bnef.com/blog/lithium-ion-battery-costs-squeezed-margins-new-business-models/ (accessed on 16 November 2018).
- Soulopoulos, N. Cost Projections: Batteries, Vehicles, and TCO; Bloomberg New Energy Finance (BNEF): New York, NY, USA, 2018. [Google Scholar]
- Mandelli, S.; Barbieri, J.; Mereu, R.; Colombo, E. Off-grid systems for rural electrification in developing countries: Definitions, classification and a comprehensive literature review. Renew. Sustain. Energy Rev. 2016, 58, 1621–1646. [Google Scholar] [CrossRef]
- Ahmad, G. Photovoltaic-powered rural zone family house in Egypt. Renew. Energy 2002, 26, 379–390. [Google Scholar] [CrossRef]
- Bhuiyan, M.; Asgar, M.A. Sizing of a stand-alone photovoltaic power system at Dhaka. Renew. Energy 2003, 28, 929–938. [Google Scholar] [CrossRef]
- Jordan, D.C.; Kurtz, S.R. Photovoltaic degradation rates—An analytical review. Prog. Photovolt. Res. Appl. 2013, 21, 12–29. [Google Scholar] [CrossRef]
- Meydbray, J.; Dross, F. PV Module Reliability Scorecard Report 2016; DNVGL: Høvik, Norway, 2016; pp. 1–18. [Google Scholar]
- Topan, P.A.; Ramadan, M.N.; Fathoni, G.; Cahyadi, A.I.; Wahyunggoro, O. State of charge (soc) and state of health (soh) estimation on lithium polymer battery via kalman filter. In Proceedings of the 2016 2nd International Conference on Science and Technology-Computer (ICST), Yogyakarta, Indonesia, 27–28 October 2016; pp. 93–96. [Google Scholar]
- Alsaidan, I.; Khodaei, A.; Gao, W. Determination of optimal size and depth of discharge for battery energy storage in standalone microgrids. In Proceedings of the North American Power Symposium (NAPS), Denver, CO, USA, 18–20 September 2016; pp. 1–6. [Google Scholar]
- Vidal Faez, A.M.; Ruvalcaba Velarde, S.A. Feasibility Study of Photovoltaic Microgrids and Their Application in Powering Remote Onshore Oil and Gas Surface Instrumentation. In Proceedings of the SPE Saudi Arabia Section Annual Technical Symposium and Exhibition, Al-Khobar, Saudi Arabia, 21–23 April 2015. [Google Scholar]
- Kaabeche, A.; Ibtiouen, R. Techno-economic optimization of hybrid photovoltaic/wind/diesel/battery generation in a stand-alone power system. Sol. Energy 2014, 103, 171–182. [Google Scholar] [CrossRef]
- Dufo-López, R.; Lujano-Rojas, J.M.; Bernal-Agustín, J.L. Comparison of different lead–acid battery lifetime prediction models for use in simulation of stand-alone photovoltaic systems. Appl. Energy 2014, 115, 242–253. [Google Scholar] [CrossRef]
- Jossen, A.; Garche, J.; Sauer, D.U. Operation conditions of batteries in PV applications. Sol. Energy 2004, 76, 759–769. [Google Scholar] [CrossRef]
- Rehman, S.; Alam, M.M.; Meyer, J.P.; Al-Hadhrami, L.M. Feasibility study of a wind–pv–diesel hybrid power system for a village. Renew. Energy 2012, 38, 258–268. [Google Scholar] [CrossRef]
- Shaahid, S.; Al-Hadhrami, L.; Rahman, M. Review of economic assessment of hybrid photovoltaic-diesel-battery power systems for residential loads for different provinces of Saudi Arabia. Renew. Sustain. Energy Rev. 2014, 31, 174–181. [Google Scholar] [CrossRef]
- Shaahid, S.; El-Amin, I. Techno-economic evaluation of off-grid hybrid photovoltaic–diesel–battery power systems for rural electrification in Saudi Arabia—A way forward for sustainable development. Renew. Sustain. Energy Rev. 2009, 13, 625–633. [Google Scholar] [CrossRef]
- Shaahid, S.; Elhadidy, M. Economic analysis of hybrid photovoltaic–diesel–battery power systems for residential loads in hot regions—A step to clean future. Renew. Sustain. Energy Rev. 2008, 12, 488–503. [Google Scholar] [CrossRef]
- Aslam, S.; Javaid, N.; Khan, F.; Alamri, A.; Almogren, A.; Abdul, W. Towards efficient energy management and power trading in a residential area via integrating a grid-connected microgrid. Sustainability 2018, 10, 1245. [Google Scholar] [CrossRef]
- Baker, J. New technology and possible advances in energy storage. Energy Policy 2008, 36, 4368–4373. [Google Scholar] [CrossRef]
- Bahramirad, S.; Reder, W.; Khodaei, A. Reliability-constrained optimal sizing of energy storage system in a microgrid. IEEE Trans. Smart Grid 2012, 3, 2056–2062. [Google Scholar] [CrossRef]
- Ma, T.; Yang, H.; Lu, L. Feasibility study and economic analysis of pumped hydro storage and battery storage for a renewable energy powered island. Energy Convers. Manag. 2014, 79, 387–397. [Google Scholar] [CrossRef]
- Li, X.; Chalvatzis, K.; Stephanides, P. Innovative Energy Islands: Life-Cycle Cost-Benefit Analysis for Battery Energy Storage. Sustainability 2018, 10, 3371. [Google Scholar] [CrossRef]
- Dufo-López, R.; Bernal-Agustín, J.L. Techno-economic analysis of grid-connected battery storage. Energy Convers. Manag. 2015, 91, 394–404. [Google Scholar] [CrossRef]
- Riaz, S.; Chapman, A.C.; Verbic, G. Comparing utility and residential battery storage for increasing flexibility of power systems. In Proceedings of the Power Engineering Conference (AUPEC), Wollongong, NSW, Australia, 27–30 September 2015; pp. 1–6. [Google Scholar]
- Yang, Y.; Ye, Q.; Tung, L.J.; Greenleaf, M.; Li, H. Integrated Size and Energy Management Design of Battery Storage to Enhance Grid Integration of Large-Scale PV Power Plants. IEEE Trans. Ind. Electron. 2018, 65, 394–402. [Google Scholar] [CrossRef]
- Charfi, S.; Atieh, A.; Chaabene, M. Modeling and cost analysis for different PV/battery/diesel operating options driving a load in Tunisia, Jordan and KSA. Sustain. Cities Soc. 2016, 25, 49–56. [Google Scholar] [CrossRef]
- Hossain, M.; Mekhilef, S.; Olatomiwa, L. Performance evaluation of a stand-alone PV-wind-diesel-battery hybrid system feasible for a large resort center in South China Sea, Malaysia. Sustain. Cities Soc. 2017, 28, 358–366. [Google Scholar] [CrossRef]
- Eteiba, M.; Barakat, S.; Samy, M.; Wahba, W.I. Optimization of an off-grid PV/Biomass hybrid system with different battery technologies. Sustain. Cities Soc. 2018, 40, 713–727. [Google Scholar] [CrossRef]
- Yahyaoui, I.; Yahyaoui, A.; Chaabene, M.; Tadeo, F. Energy management for a stand-alone photovoltaic-wind system suitable for rural electrification. Sustain. Cities Soc. 2016, 25, 90–101. [Google Scholar] [CrossRef]
- Ardani, K.; O’Shaughnessy, E.; Fu, R.; McClurg, C.; Huneycutt, J.; Margolis, R. Installed Cost Benchmarks and Deployment Barriers for Residential Solar Photovoltaics with Energy Storage: Q1 2016; National Renewable Energy Laboratory (NREL): Golden, CO, USA, 2016.
- Lai, C.S.; McCulloch, M.D. Levelized cost of electricity for solar photovoltaic and electrical energy storage. Appl. Energy 2017, 190, 191–203. [Google Scholar] [CrossRef]
- Pawel, I. The cost of storage—How to calculate the levelized cost of stored energy (LCOE) and applications to renewable energy generation. Energy Procedia 2014, 46, 68–77. [Google Scholar] [CrossRef]
- Boovaragavan, V.; Methakar, R.N.; Ramadesigan, V.; Subramanian, V.R. A mathematical model of the lead-acid battery to address the effect of corrosion. J. Electrochem. Soc. 2009, 156, A854–A862. [Google Scholar] [CrossRef]
- Jacob, A.S.; Banerjee, R.; Ghosh, P.C. Modelling and simulation of a PV battery grid backup system for various climatic zones of India. In Proceedings of the 2016 IEEE 43rd Photovoltaic Specialists Conference (PVSC), Portland, OR, USA, 5–10 June 2016; pp. 1807–1812. [Google Scholar]
- Khasawneh, H.J.; Mondal, A.; Illindala, M.S.; Schenkman, B.L.; Borneo, D.R. Evaluation and sizing of energy storage systems for microgrids. In Proceedings of the 2015 IEEE/IAS 51st Industrial & Commercial Power Systems Technical Conference (I&CPS), Calgary, AB, Canada, 5–8 May 2015; pp. 1–8. [Google Scholar]
- Arun, P.; Banerjee, R.; Bandyopadhyay, S. Optimum sizing of photovoltaic battery systems incorporating uncertainty through design space approach. Sol. Energy 2009, 83, 1013–1025. [Google Scholar] [CrossRef]
- Kaushika, N.; Gautam, N.K.; Kaushik, K. Simulation model for sizing of stand-alone solar PV system with interconnected array. Sol. Energy Mater. Sol. Cells 2005, 85, 499–519. [Google Scholar] [CrossRef]
- Celik, A.; Muneer, T.; Clarke, P. Optimal sizing and life cycle assessment of residential photovoltaic energy systems with battery storage. Prog. Photovolt. Res. Appl. 2008, 16, 69–85. [Google Scholar] [CrossRef]
- Kaldellis, J. Optimum technoeconomic energy autonomous photovoltaic solution for remote consumers throughout Greece. Energy Convers. Manag. 2004, 45, 2745–2760. [Google Scholar] [CrossRef]
- Meunier, J.; Knittel, D.; Collet, P.; Sturtzer, G.; Carpentier, C.; Rocchia, G.; Wisse, J.; Helfter, M. Sizing of a photovoltaic system with battery storage: Influence of the load profile. In Proceedings of the International Conference CISBAT 2015 Future Buildings and Districts Sustainability from Nano to Urban Scale, Lausanne, Switzerland, 9–11 September 2015; pp. 711–716. [Google Scholar]
- Jülch, V. Comparison of electricity storage options using levelized cost of storage (LCOS) method. Appl. Energy 2016, 183, 1594–1606. [Google Scholar] [CrossRef]
- Mundada, A.S.; Shah, K.K.; Pearce, J.M. Levelized cost of electricity for solar photovoltaic, battery and cogen hybrid systems. Renew. Sustain. Energy Rev. 2016, 57, 692–703. [Google Scholar] [CrossRef]
- Obi, M.; Jensen, S.; Ferris, J.B.; Bass, R.B. Calculation of levelized costs of electricity for various electrical energy storage systems. Renew. Sustain. Energy Rev. 2017, 67, 908–920. [Google Scholar] [CrossRef]
- Belderbos, A.; Delarue, E.; Kessels, K.; D’haeseleer, W. Levelized cost of storage—Introducing novel metrics. Energy Econ. 2017, 67, 287–299. [Google Scholar] [CrossRef]
- Manwell, J.F.; McGowan, J.G.; Abdulwahid, U.; Wu, K. Improvements to the Hybrid2 battery model. In Proceedings of the Windpower 2005 Conference, Denver, CO, USA, 17 May 2005. [Google Scholar]
- PowerThru. Lead Acid Battery Working—Lifetime Study. Available online: http://www.power-thru.com/documents/The%20Truth%20About%20Batteries%20-%20POWERTHRU%20White%20Paper.pdf (accessed on 1 December 2018).
- Feron, S.; Cordero, R.; Labbe, F. Rural electrification efforts based on off-grid photovoltaic systems in the Andean Region: Comparative assessment of their sustainability. Sustainability 2017, 9, 1825. [Google Scholar] [CrossRef]
- Barman, M.; Mahapatra, S.; Palit, D.; Chaudhury, M.K. Performance and impact evaluation of solar home lighting systems on the rural livelihood in Assam, India. Energy Sustain. Dev. 2017, 38, 10–20. [Google Scholar] [CrossRef]
- Upadhyay, T.P.; Shahi, C.; Leitch, M.; Pulkki, R. Economic feasibility of biomass gasification for power generation in three selected communities of northwestern Ontario, Canada. Energy Policy 2012, 44, 235–244. [Google Scholar] [CrossRef]
- Schmidt, O.; Hawkes, A.; Gambhir, A.; Staffell, I. The future cost of electrical energy storage based on experience rates. Nat. Energy 2017, 2, 17110. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, B.; Wang, J.; Begovic, M.M.; Chen, C. Coordinated energy management of networked microgrids in distribution systems. IEEE Trans. Smart Grid 2015, 6, 45–53. [Google Scholar] [CrossRef]
- Noel, L.; Brodie, J.F.; Kempton, W.; Archer, C.L.; Budischak, C. Cost minimization of generation, storage, and new loads, comparing costs with and without externalities. Appl. Energy 2017, 189, 110–121. [Google Scholar] [CrossRef]
- Buchman, A.; Lung, C. State of Charge and State of Health Estimation of Lithium-Ion Batteries. In Proceedings of the 2018 IEEE 24th International Symposium for Design and Technology in Electronic Packaging (SIITME), Iasi, Romania, 25–28 October 2018; pp. 382–385. [Google Scholar]
| Reference | Has the Paper Considered Temperature Effects on Battery Capacity? | Has the Paper Considered Temperature Effects on Lifetime, i.e., Calendar Life and Cyclical Degradation? | Has the Paper Considered Different MSOC Levels for Sizing Optimization? | Has the Paper Tested for Differed Load Profiles? | Was the System Fully Standalone, i.e., No Grid and No Diesel Generation? |
|---|---|---|---|---|---|
| [39] | Yes | Yes | No | No | No |
| [9] | No | No | No | No | Yes |
| [10] | Yes | No | No | No | Yes |
| [40] | Yes | No | No | Yes | No |
| [41] | No | No | No | No | Yes |
| [42] | No | No | No | No | No |
| [1] | No | No | No | No | No |
| [43] | No | Yes | No | No | No |
| [20] | No | No | No | No | No |
| [21] | No | No | No | No | No |
| [44] | No | No | No | No | Yes |
| [15] | No | Yes | No | No | Yes |
| [14] | No | No | Yes | No | No |
| [45] | No | No | No | Yes | No |
| This work | Yes | Yes | Yes | Yes | Yes |
| Parameter | Value |
|---|---|
| Discounting rate | 5 percent |
| Inflation rate | 2 percent |
| System lifetime | 25 years |
| Supply shortage | 0 percent |
| Parameter | Value |
|---|---|
| CAPEX (excluding inverter) ($/W) | 1.8 $/W |
| Inverter CAPEX | 0.2 $/W |
| Module efficiency (%) | 16 percent |
| Annual panel degradation (%) | 0.5 percent |
| Temperature power loss coefficient (%/C) | 0.4%/°C |
| Nominal operating cell temperature | 47 °C |
| Maintenance costs ($/kW/year) | 10 $/kW/year |
| Derating factor (including inverter losses) (%) | 85 percent |
| Panel slope from the horizontal | 25° |
| Azimuth degree from the north | 180° |
| Solar irradiation | Hourly irradiation data for Riyadh |
| Parameter | Value |
|---|---|
| Battery model | Trojan SIND 061225 (lead acid) |
| Battery cost (including installation) | $2205 (300 $/kW h) |
| Nominal voltage | 6 V |
| Energy rating | 7.35 kW h |
| Capacity (current rating) | 1225 Ah |
| Bus voltage | 24 V |
| Days of autonomy | 2 days |
| Allowable throughput | 14,598 kW h (see text for details) |
| Degradation limit | 20 percent |
| Round trip efficiency | 85 percent |
| Temperature effect on capacity | See text for details |
| Temperature effect on calendar lifetime | See text for details |
| Results Incorporating Temperature Effects | |||||||
| MSOC (%) | PV Size (kW) | Total Number of Batteries in the Bank | Average Cost of Energy ($/kW h) | Net Present Cost ($) | Initial Capital ($) | Number of Battery Replacements | Total Number of Batteries Used throughout Project Lifetime |
| 20 | 6.7 | 8 | 0.435 | 69,985 | 31,025 | 3 | 32 |
| 30 | 8.0 | 8 | 0.453 | 72,898 | 33,566 | 3 | 32 |
| 40 | 9.9 | 8 | 0.480 | 77,315 | 37,529 | 3 | 32 |
| 50 | 7.1 | 16 | 0.728 | 117,287 | 49,499 | 2 | 48 |
| 60 | 9.4 | 20 | 0.908 | 146,165 | 62,913 | 2 | 60 |
| 70 | 10.8 | 108 * | 4.208 | 677,471 | 259,781 | 2 | 324 |
| Results without Incorporating Temperature Effects | |||||||
| MSOC (%) | PV Size (kW) | Total Number of Batteries in the Bank | Average Cost of Energy ($/kW h) | Net Present Cost ($) | Initial Capital ($) | Number of Battery Replacements | Total Number of Batteries Used throughout Project Lifetime |
| 20 | 6.2 | 8 | 0.244 | 39,306 | 29,961 | 1 | 16 |
| 30 | 6.9 | 8 | 0.254 | 40,928 | 31,421 | 1 | 16 |
| 40 | 8.5 | 8 | 0.277 | 44,549 | 34,682 | 1 | 16 |
| 50 | 11.7 | 8 | 0.321 | 51,655 | 41,082 | 1 | 16 |
| 60 | 6.6 | 20 | 0.488 | 78,637 | 57,225 | 1 | 40 |
| 70 | 13.9 | 20 | 0.590 | 94,992 | 71,954 | 1 | 40 |
| Results Incorporating Temperature Effects | |||||||
| MSOC (%) | PV Size (kW) | Total Number of Batteries in the Bank | Average Cost of Energy ($/kW h) | Net Present Cost ($) | Initial Capital ($) | Number of Battery Replacements | Total Number of Batteries Used throughout Project Lifetime |
| 20 | 6.8 | 12 | 0.615 | 99,044 | 40,023 | 3 | 48 |
| 30 | 7.2 | 12 | 0.622 | 100,192 | 40,880 | 3 | 48 |
| 40 | 6.9 | 16 | 0.751 | 120,992 | 49,061 | 3 | 64 |
| 50 | 7.8 | 20 | 0.906 | 145,879 | 59,631 | 3 | 80 |
| 60 | 10.2 | 28 * | 1.231 | 198,166 | 82,161 | 2 | 84 |
| 70 | 7.0 | 148 * | 5.653 | 910,114 | 340,321 | 2 | 444 |
| Results without Incorporating Temperature Effects | |||||||
| MSOC (%) | PV Size (kW) | Total Number of Batteries in the Bank | Average Cost of Energy ($/kW h) | Net Present Cost ($) | Initial Capital ($) | Number of Battery Replacements | Total Number of Batteries Used throughout Project Lifetime |
| 20 | 8.8 | 8 | 0.281 | 45,188 | 35,258 | 1 | 16 |
| 30 | 11.1 | 8 | 0.312 | 50,195 | 39,767 | 1 | 16 |
| 40 | 7.8 | 12 | 0.347 | 55,859 | 42,149 | 1 | 24 |
| 50 | 7.5 | 16 | 0.422 | 68,011 | 50,374 | 1 | 32 |
| 60 | 9.4 | 20 | 0.528 | 84,953 | 62,913 | 1 | 40 |
| 70 | 10.7 | 36 * | 0.864 | 139,118 | 100,818 | 1 | 72 |
| Parameter | Value for Independent Generator | Value for Microgrid Generator |
|---|---|---|
| Capital cost | 0.70 $/W | 0.20 $/W |
| Fuel cost | 0.10, 0.50, 1.00, 1.50 $/L | 0.10, 0.50, 1.00, 1.50 $/L |
| Lifetime of generator | 2500 h | 15,000 h |
| Minimum load ratio | 25 percent | 25 percent |
| Maintenance intervals | Every 400 h of operation | Every 400 h of operation |
| Maintenance cost | $25 | $400 |
| Operation cost | 0.10 $/h of operation | 0.45 $/h of operation |
| Scenario | Diesel Price ($/L) | Diesel Consumption Per Year (Million Liters) | Carbon Emissions Per Year (Thousand Tons) | Comments | |||
|---|---|---|---|---|---|---|---|
| 0.10 | 0.50 | 1.00 | 1.50 | ||||
| Individual generator for each home | 111 | 133 | 159 | 185 | 3.03 | 7900 | - No grid investment or maintenance costs required - Model run for two generators each with a capacity of 2 kW |
| Microgrid powering complete community | 91 | 105 | 122 | 139 | 1.94 | 5100 | - Cost of supplying diesel, maintaining and upgrading grid, and externalities not included - Model chooses two generators with capacities of 1 MW and 1.75 MW to meet the load |
| PVB: 30% MSOC with temperature effects incorporated | 99 (maximum) | 0 | - No grid investment or maintenance costs required - Learning curve of battery technology not considered | ||||
| PVB: 30% MSOC with temperature effects NOT incorporated | 50 (maximum) | ||||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elshurafa, A.M.; Aldubyan, M.H. State-of-Charge Effects on Standalone Solar-Storage Systems in Hot Climates: A Case Study in Saudi Arabia. Sustainability 2019, 11, 3471. https://doi.org/10.3390/su11123471
Elshurafa AM, Aldubyan MH. State-of-Charge Effects on Standalone Solar-Storage Systems in Hot Climates: A Case Study in Saudi Arabia. Sustainability. 2019; 11(12):3471. https://doi.org/10.3390/su11123471
Chicago/Turabian StyleElshurafa, Amro M., and Mohammad H. Aldubyan. 2019. "State-of-Charge Effects on Standalone Solar-Storage Systems in Hot Climates: A Case Study in Saudi Arabia" Sustainability 11, no. 12: 3471. https://doi.org/10.3390/su11123471
APA StyleElshurafa, A. M., & Aldubyan, M. H. (2019). State-of-Charge Effects on Standalone Solar-Storage Systems in Hot Climates: A Case Study in Saudi Arabia. Sustainability, 11(12), 3471. https://doi.org/10.3390/su11123471





