Evaluating Power Reliability Dedicated for Sudden Disruptions: Its Application to Determine Capacity on the Basis of Energy Security
Abstract
1. Introduction
2. Materials and Methods
2.1. Development and Evaluation of Electricity Reliability Index Dedicated for the Sudden Disruption
- Analysis of the sustainable duration for the electrical system
- Computation of the sustainable duration analysis to obtain the SESDI for the electrical system.
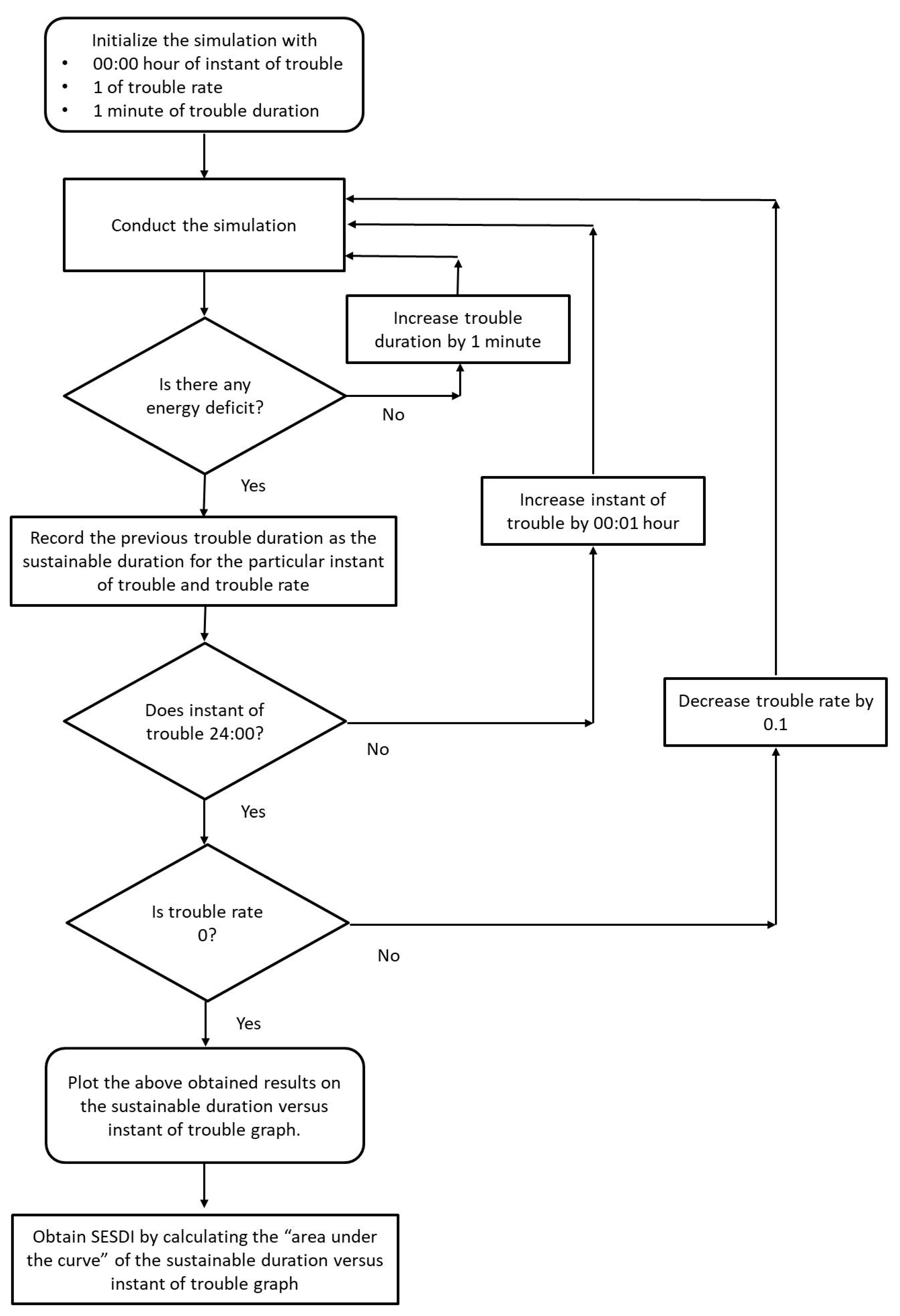
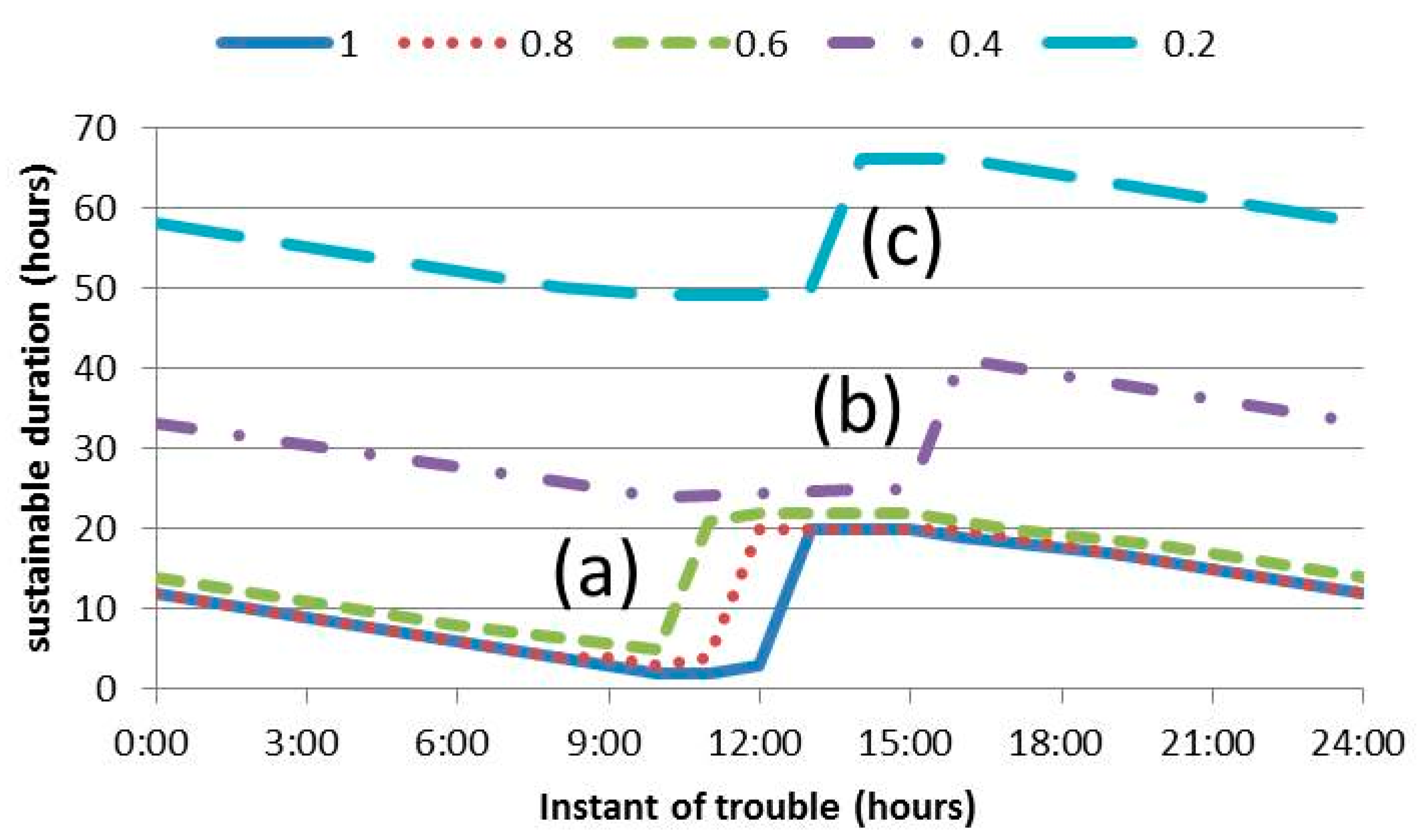
2.1.1. Sustainable Duration Analysis for the Electrical System
- Initialization of system parameters. The instant of trouble is 00:00 h. The trouble rate is 1 (100% failure). The trouble duration is 1 min.
- Run the simulation and check if there is any energy deficit. An energy deficit occurs when all energy sources have been depleted by the consumption of the electrical system.
- If there is no energy deficit, the simulation is repeated with increasing trouble duration in steps of 1 min until the energy deficit is obtained. Once the energy deficit is obtained, the previous trouble duration is recorded as the sustainable duration for the particular instant of trouble and trouble rate.
- Repeat Steps 2 and 3 for the increasing instant of trouble in step 00:01 h until 24:00 h.
- Repeat Steps 2 to 4 for decreasing trouble rate in steps of 0.1 until a trouble rate of 0 is reached.
- The above obtained results are then plotted on the sustainable duration versus instant of trouble graph.
2.1.2. Computation of the Sustainable Duration Analysis to Obtain the SESDI for the Electrical System
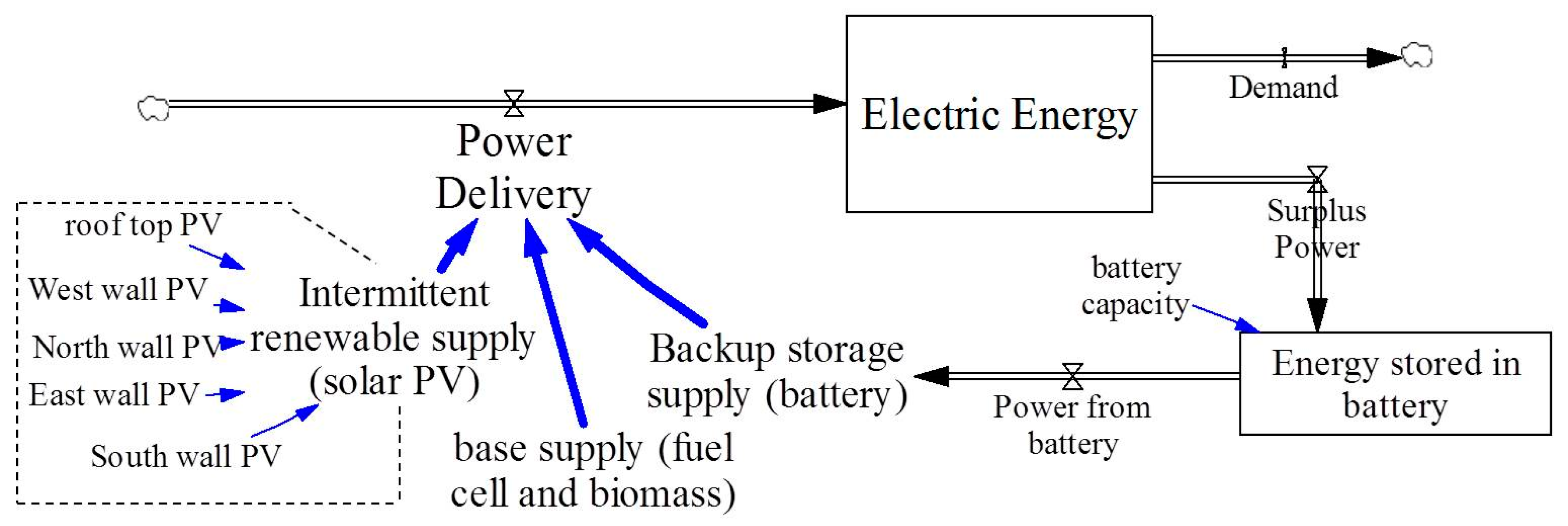
2.2. Site Identification and System Modelling as an Illustrative Study
3. Results
3.1. Calculation of Solar PV Power Delivery Curve from Five Sides of Building
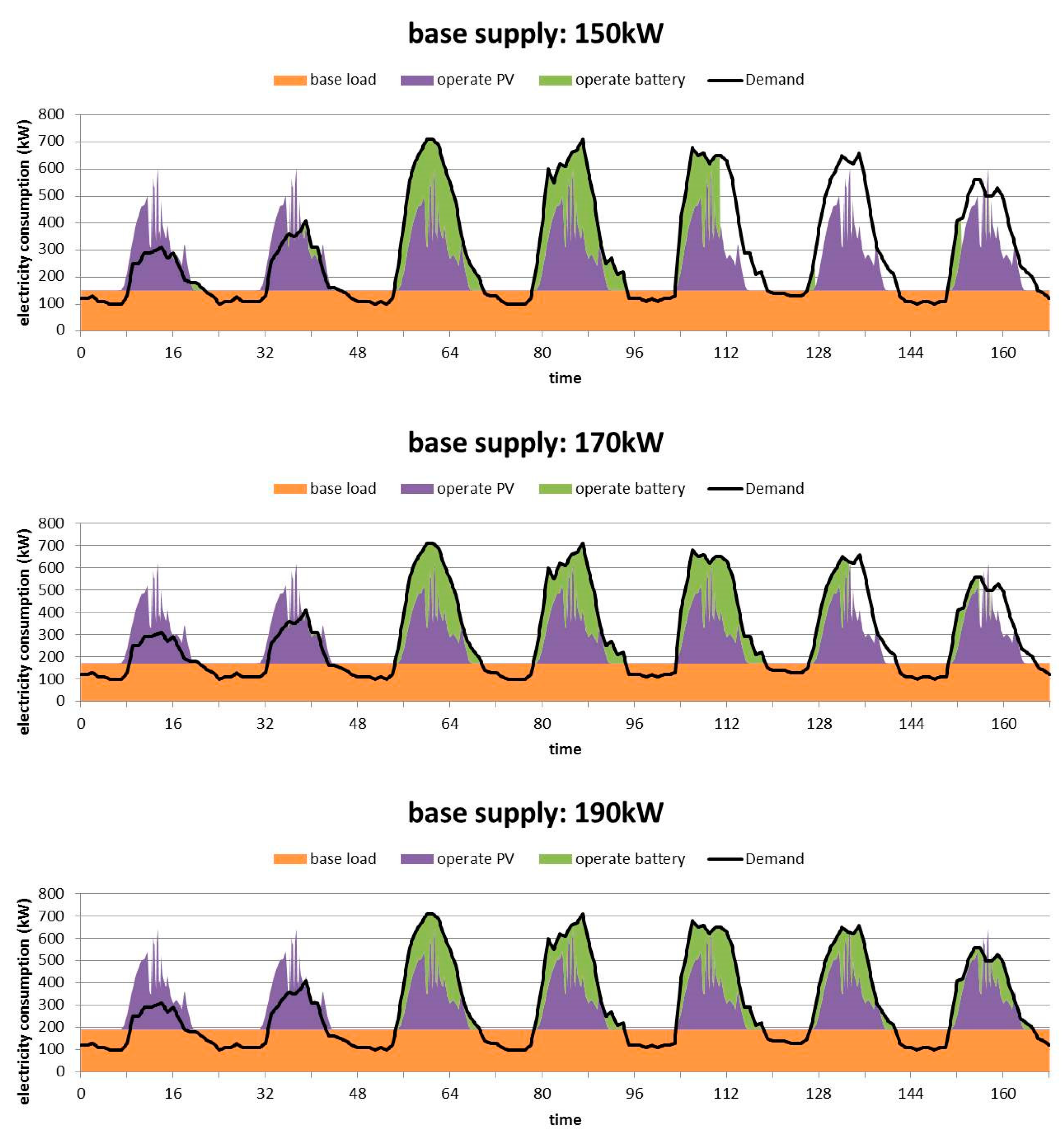
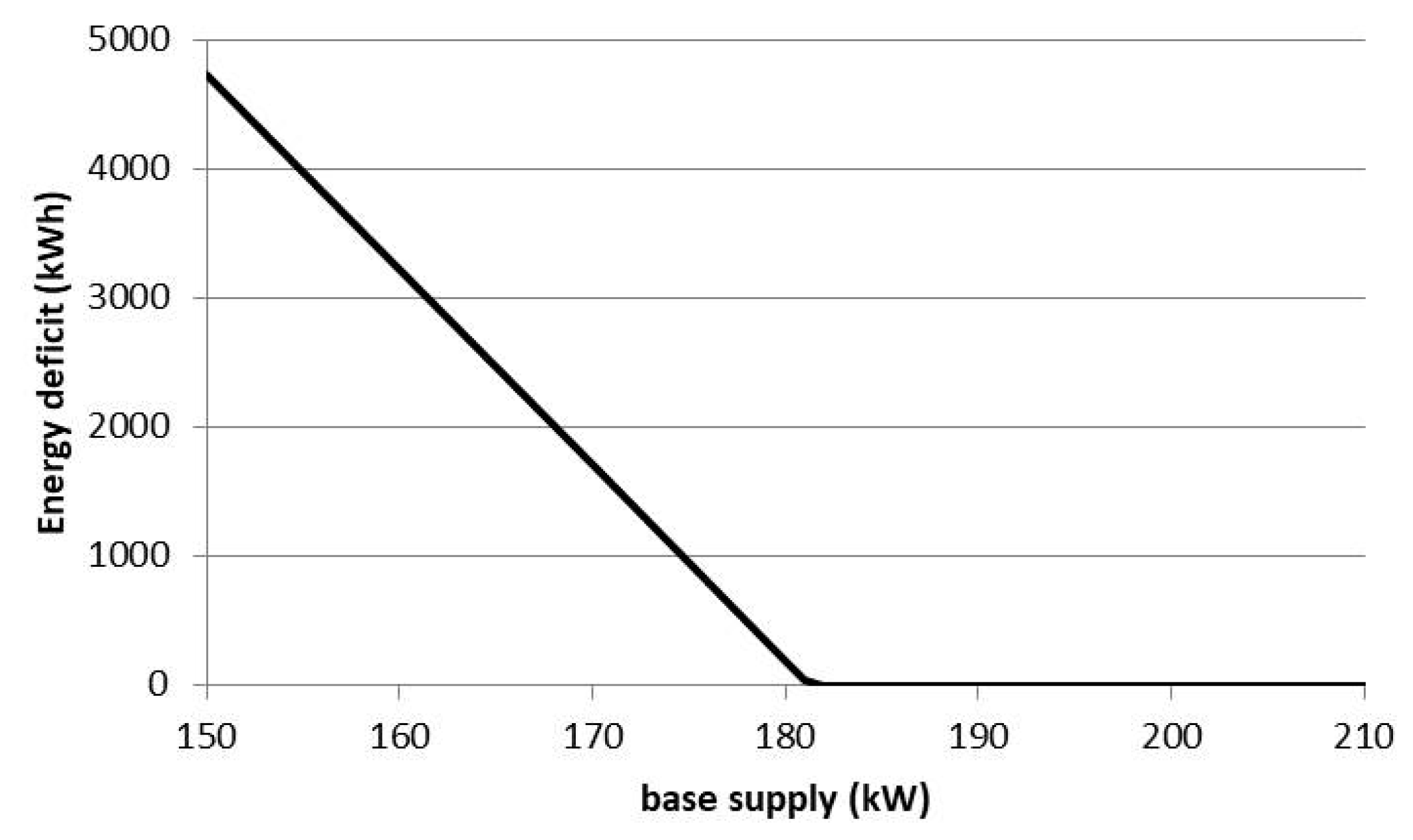
3.2. Identification of the Minimum Required Installed Capacity for Base Supply
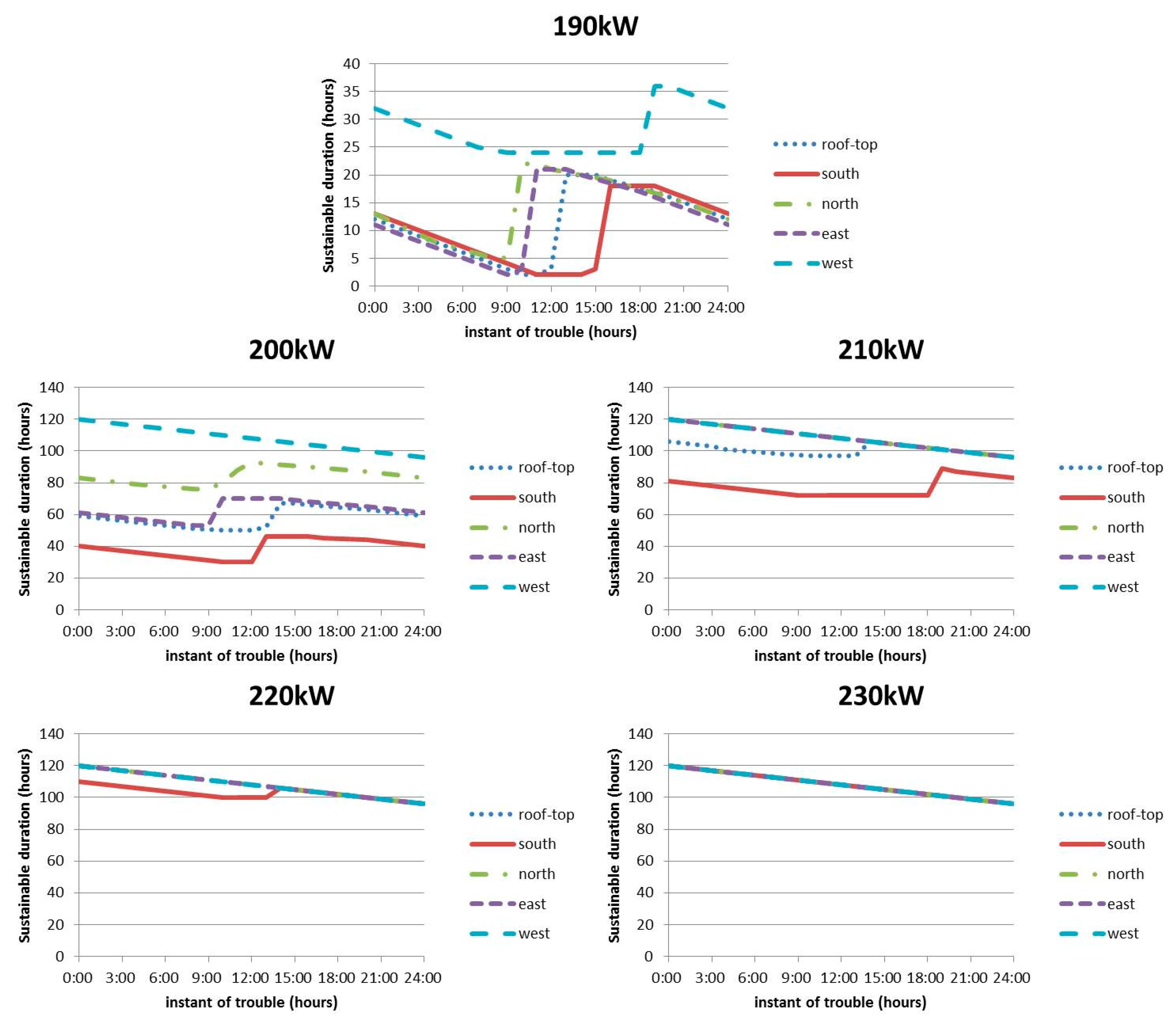
3.3. Sustainable Duration Analysis
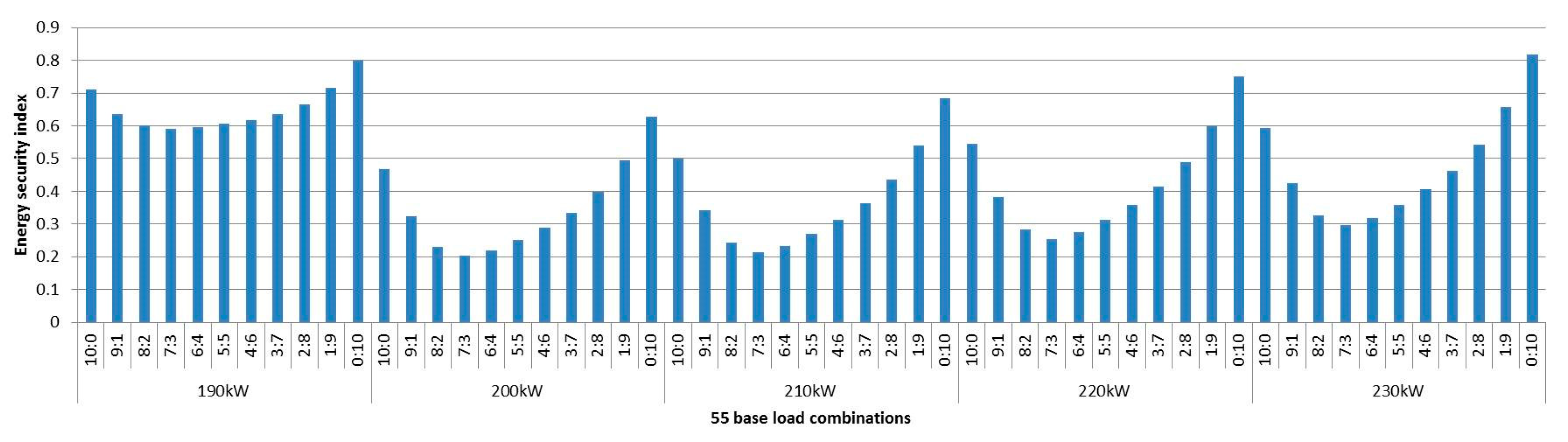
3.4. Evaluation of the SESDI
4. Discussion
- The possible range of the base supply capacity will be identified through analysis of the sustainable duration.
- Three indicators were computed based on the different base supply capacities.
- The computed values were normalized to be expressed in common units and were aggregated to obtain the energy security index.
4.1. Identification of the Possible Range of Installed Capacity for Base Supply
4.2. Computation of the Proposed Three Indicators
4.3. Evaluation of the Energy Security Index
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ministry of Economy, Trade and Industry (METI). The Impact of Electricity Supply Limitation. 2011. Available online: http://www.chusho.meti.go.jp/pamflet/hakusyo/h23/h23/html/k124000.html (accessed on 7 April 2016).
- Ministry of Economy, Trade and Inovation. Energy Strategic Plan. 2014. Available online: http://www.enecho.meti.go.jp/en/category/others/basic_plan/pdf/4th_strategic_energy_plan.pdf (accessed on 20 August 2015).
- Vale, B.; Vale, R. The New Autonomous House: Design and Planning for Sustainability; Thames & Hudson Ltd.: London, UK, 2002. [Google Scholar]
- Feist, W. Life-cycle energy analysis: Comparison of low-energy house, passive house and self-sufficient house. In Proceedings of the International Symposium of CIB W67, Vienna, Austria, 8–10 September 1997. [Google Scholar]
- Kramer, J.; Krothapalli, A.; Greska, B. The Off-grid Zero Emission Building. In Proceedings of the Energy Sustainability Conference, Long Beach, CA, USA, 27–30 July 2007. [Google Scholar]
- Laustsen, J. Energy Efficiency Requirements in Building Codes. In Energy Efficiency Policies for New Building; OECD/IEA: Paris, France, 2008. [Google Scholar]
- Iqbal, M.T. A feasibility study of a zero energy home in Newfoundland. Renew. Energy 2004, 29, 277–289. [Google Scholar] [CrossRef]
- North American Electric Reliability Council (NERC). Glossary of Terms Used in NERC Reliability Standards. 2017. Available online: www.nerc.com/files/glossary_of_terms.pdf (accessed on 1 March 2017).
- Kjølle, G.H.; Utne, I.B.; Gjerde, O. Risk analysis of critical infrastructures emphasizing electricity supply and interdependencies. Reliab. Eng. Syst. Saf. 2012, 105, 80–89. [Google Scholar] [CrossRef]
- Chang, L.; Wu, Z. Performance and reliability of electrical power grids under cascading failures. Electr. Power Energy Syst. 2011, 33, 1410–1419. [Google Scholar] [CrossRef]
- Ren, H.; Dobson, I.; Carreras, B.A. Long-term effect of the n-1 criterion on cascading line outages in an evolving power transmission grid. IEEE Trans. Power Syst. 2008, 23, 1217–1225. [Google Scholar] [CrossRef]
- Haarla, L.; Pulkkinen, U.; Koskinen, M. A method for analysing the reliability of a transmission grid. Reliab. Eng. Syst. Saf. 2006, 93, 277–287. [Google Scholar] [CrossRef]
- Layton, L. Electric System Reliability Indices. 2004. Available online: http://iiee.org.ph/wp-content/uploads/2015/06/Distribution-System-Reliability-Improvement-2015-IIEE-MYC.pdf (accessed on 9 November 2016).
- Čepin, M. Distribution and Transmission System Reliability Measures. In Assessment of Power System Reliability; Springer: Berlin, Germany, 2011; Chapter 14; pp. 215–226. [Google Scholar]
- Celik, N. Effect of different load profiles on the loss-of load probability of stand-alone photovoltaic systems. Renew. Energy 2007, 32, 2096–2115. [Google Scholar] [CrossRef]
- Jakhrani, Q.; Othman, A.-K.; Rigit, A.R.H.; Samo, S.R.; Kamboh, S.A. A novel analytical model for optimal sizing of standalone photovoltaic systems. Energy 2012, 46, 675–682. [Google Scholar] [CrossRef]
- Lucio, J.H.; Valdés, R.; Rodríguez, L.R. Loss-of-load probability model for stand alone photovoltaic systems in Europe. Sol. Energy 2012, 86, 2515–2535. [Google Scholar] [CrossRef]
- Abouzahr, I.; Ramakumar, R. Loss of power supply probability of stand alone photovoltaic systems: A closed form approach. IEEE Trans. Energy Convers. 1991, 6, 1–11. [Google Scholar] [CrossRef]
- Cabral, V.T.; Filho, D.O.; Diniz, A.S.A.C.; Martins, J.H.; Toledo, O.M.; de Vilhena, B.L.; Neto, M. A stochastic method for stand-alone photovoltaic system sizing. Sol. Energy 2010, 84, 1628–1636. [Google Scholar] [CrossRef]
- Kundur, P.; Paserba, J.; Ajjarapu, V.; Andersson, G.; Bose, A.; Canizares, C.; Hatziargyriou, N.; Hill, D.; Stankovic, A.; Taylor, C.; et al. Definition and classification of power system stability. IEEE Trans. Power Syst. 2004, 19, 1387–1401. [Google Scholar]
- Kosai, S.; Yamasue, E. Cost-Security Analysis Dedicated for the Off-grid Electricity System. Renew. Energy 2018, 115, 871–879. [Google Scholar] [CrossRef]
- Hu, F.Y.; Yeung, C.H.; Yang, S.; Wang, W.P.; Zeng, A. Recovery of infrastructure networks after localised attacks. Sci. Rep. 2016, 6, 24522. [Google Scholar] [CrossRef] [PubMed]
- Hazi, G.; Bică, D.; Hazi, A. Considerations on an Indicator Guaranteed in the Electricity Supply to Consumers. Procedia Technol. 2015, 19, 643–650. [Google Scholar] [CrossRef]
- Kosai, S.; Unesaki, H. Quantitative Analysis on the Impact of Nuclear Energy Supply Disruption on Electricity Supply Security. Appl. Energy 2018, 208, 1198–1207. [Google Scholar] [CrossRef]
- Zhang, S.; Huang, P.; Sun, Y. A multi-criterion renewable energy system design optimization for net zero energy buildings under uncertainties. Energy 2016, 94, 654–655. [Google Scholar] [CrossRef]
- Miller, W. What does built environment research have to do with risk mitigation, resilience and disaster recovery? Sustain. Cities Soc. 2015, 19, 91–97. [Google Scholar] [CrossRef]
- Khatib, T.; Mohamed, A.; Sopian, P. A review of photovoltaic systems size optimization techniques. Renew. Sustain. Energy Rev. 2013, 22, 454–465. [Google Scholar] [CrossRef]
- Henneaux, P.; Labeau, P.E.; Maun, J.C. Blackout Probabilistic Risk Assessment and Thermal Effects: Impacts of Changes in Generation. IEEE Trans. Power Syst. 2013, 28, 4722–4731. [Google Scholar] [CrossRef]
- Henneaux, P.; Labeau, P.E.; Maun, J.C. A level-1 probabilistic risk assessment to blackout hazard in transmission power systems. Reliab. Eng. Syst. Saf. 2012, 102, 41–52. [Google Scholar] [CrossRef]
- Kosai, S.; Rahim, N.A. Energy mix with the vulnerability of nuclear power utilization. In Proceedings of the 4th IET International Conference on Clean Energy and Technology CEAT 2016, Kuala Lumpur, Malaysia, 14–15 November 2016; pp. 1–7. [Google Scholar] [CrossRef]
- Ang, W.; Choong, W.L.; Ng, T.S. Energy security: Definitions, dimensions and indexes. Renew. Sustain. Energy Rev. 2015, 42, 1077–1093. [Google Scholar] [CrossRef]
- Sovacool, B.K. Evaluating energy security in the Asia pacific: Towards a more comprehensive approach. Energy Policy 2011, 39, 7472–7479. [Google Scholar] [CrossRef]
- Sovacool, B.K.; Mukherjee, I. Conceptualizing and measuring energy security: A synthesized approach. Energy 2011, 36, 5343–5355. [Google Scholar] [CrossRef]
- Portugal-Pereira, J.; Esteban, M. Implications of paradigm shift in Japan’s electricity security of supply: A multi-dimensional indicator assessment. Appl. Energy 2014, 123, 424–434. [Google Scholar] [CrossRef]
- Jansen, C. Energy Services Security: Concepts and Metrics; Energy Research Centre of the Netherlands (ECN): ZG Petten, The Netherlands, 2009. [Google Scholar]
- Streimikiene, D.; Ciegis, R.; Grundey, D. Energy indicators for sustainable development in Baltic State. Renew. Sustain. Energy Rev. 2007, 11, 877–893. [Google Scholar] [CrossRef]
- Sovacool, B.K.; Brown, M.A. Competing dimensions of energy security: An international perspective. Annu. Rev. Environ. Resour. 2010, 35, 77–108. [Google Scholar] [CrossRef]
- Institute for 21st Century Energy. Energy Index of U.S. Energy Security Risk ed.; Chamber of Commerce: Washington, DC, USA, 2012. [Google Scholar]
- Winzer, C. Conceptualizing energy security. Energy Policy 2012, 46, 36–48. [Google Scholar] [CrossRef]
- Martchamadol, J.; Kumar, S. Thailand’s energy security indicators. Renew. Sustain. Energy Rev. 2012, 16, 6103–6122. [Google Scholar] [CrossRef]
- Cohen, G.; Joutz, F.; Loungani, P. Measuring energy security: Trends in the diversification of oil and natural gas supplies. Energy Policy 2011, 39, 4860–4869. [Google Scholar] [CrossRef]
- Wu, G.; Liu, L.C.; Han, Z.Y.; Wei, Y.M. Climate protection and China’s energy security: Win-win or tradeoff. Appl. Energy 2012, 97, 157–163. [Google Scholar] [CrossRef]
- Cabalu, H. Indicators of security of natural gas supply in Asia. Energy Policy 2010, 38, 218–225. [Google Scholar] [CrossRef]
- International Energy Agency (IEA). Towards Net Zero Energy Solar Buildings—Fact Sheet; SHC Task 40/ECBCS Annex 52 IEA; IEA: Paris, France, 2011. [Google Scholar]
- Clift, R. Climate change and energy policy: The importance of sustainability arguments. Energy 2007, 32, 262–268. [Google Scholar] [CrossRef]
- Wolstenholme, E.F. System Enquiry. A System Dynamics Approach; John Wiley and Sons: Chichester, UK, 1990. [Google Scholar]
- Senge, P. The Fifth Discipline: The Art and Practice of Learning Organization; Random House Business: New York, NY, USA, 1990; p. 424. [Google Scholar]
- Lempert, R.; Popper, S.; Bankes, S. Shaping the Next One Hundred Years: New Methods for Quantitative, Long-Term Policy Analysis; RAND Report MR-1626; RAND Corporation: Santa Monica, CA, USA, 2003. [Google Scholar]
- Kosai, S.; Tan, C. Quantitative analysis on a zero energy building performance from energy trilemma perspective. Sustain. Cities Soc. 2017, 32, 130–141. [Google Scholar] [CrossRef]
- Chartered Institution of Building Services Engineers (CIBSE). Fuel Cells for Buildings. DATASHEET. 2012. Available online: http://www.cibse.org/getmedia/dc463e28-a44d-4072-8d76-8a023815b6c1/Datasheet-4-Fuel-cells.pdf.aspx (accessed on 2 September 2016).
- Torcellini, P.; Pless, S.; Deru, M.; Crawley, D. Zero Energy Buildings: A Criteria Look at the Definition; ACEEE Summer Stud: Pacific Grove, CA, USA, 2006. [Google Scholar]
- Oh, T.H.; Pang, S.Y.; Chua, S.C. Energy policy and alternative energy in Malaysia: Issues and challenges for sustainable growth. Renew. Sustain. Energy Rev. 2010, 14, 1241–1252. [Google Scholar] [CrossRef]
- New Energy and Industrial Technology Development Organization (NEDO). NEDO Irradiation Data Base. 2016. Available online: http://app0.infoc.nedo.go.jp/metpv/metpv.html (accessed on 8 July 2016).
- Chen, H.; Cong, T.N.; Yang, W.; Tan, C.T.; Li, Y.; Ding, D. Progress in electrical energy storage system: A critical review. Prog. Nat. Sci. 2009, 19, 291–312. [Google Scholar] [CrossRef]
- NGK Insulator. Sodium Sulphur Battery, Energy Storage System. 2016. Available online: https://www.ngk.co.jp/nas/specs/#dStructure (accessed on 19 July 2016).
- International Renewable Energy Agency (IRENA). Renewable Energy Technologies: Cost Analysis Series. 2012. Available online: https://www.irena.org/DocumentDownloads/Publications/RE_Technologies_Cost_Analysis-BIOMASS.pdf (accessed on 27 August 2016).
- McPhail, S.J.; Leto, L.; Pitra, M.D.; Cigolotti, V.; Moreno, A. International Status of Molten Carbonate Fuel Cells Technology; Advanced Fuel Cells Implementing Agreement, Annex 23—MCFC; E.N.E.A.: Rome, Italy, 2015. [Google Scholar]
- Bradin, J.; Tunér, M.; Odenbrand, I. Small Scale Gasification: Gas Engine CHP for biofuels. Lund University and Linnaeus University, Växjö/Lund. 2011. Available online: http://www.ieatask33.org/download.php?file=files/file/publications/new/Small%20Small_scale_gasification_overview.pdf (accessed on 31 August 2016).
- Sartori, I.; Napolitano, A.; Voss, K. Net zero energy buildings: A consistent definition framework. Energy Build. 2012, 48, 220–232. [Google Scholar] [CrossRef]
- Nakahara, T.; Kurihara, I. Reliability Criteria for Generation and Transmission System Planning in Foreign Countries. IEEJ Trans. Power Energy 1994, 114, 960–963. [Google Scholar] [CrossRef]
- TEPCO. Tokyo Electronic Company Press Release. 2011. Available online: http://www.tepco.co.jp//cc/press/index1103-j.html (accessed on 15 August 2015).




© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kosai, S.; Tan, C.K.; Yamasue, E. Evaluating Power Reliability Dedicated for Sudden Disruptions: Its Application to Determine Capacity on the Basis of Energy Security. Sustainability 2018, 10, 2059. https://doi.org/10.3390/su10062059
Kosai S, Tan CK, Yamasue E. Evaluating Power Reliability Dedicated for Sudden Disruptions: Its Application to Determine Capacity on the Basis of Energy Security. Sustainability. 2018; 10(6):2059. https://doi.org/10.3390/su10062059
Chicago/Turabian StyleKosai, Shoki, Chia Kwang Tan, and Eiji Yamasue. 2018. "Evaluating Power Reliability Dedicated for Sudden Disruptions: Its Application to Determine Capacity on the Basis of Energy Security" Sustainability 10, no. 6: 2059. https://doi.org/10.3390/su10062059
APA StyleKosai, S., Tan, C. K., & Yamasue, E. (2018). Evaluating Power Reliability Dedicated for Sudden Disruptions: Its Application to Determine Capacity on the Basis of Energy Security. Sustainability, 10(6), 2059. https://doi.org/10.3390/su10062059




