Optimal Design of Fractional-Order Electrical Network for Vehicle Mechatronic ISD Suspension Using the Structure-Immittance Approach
Abstract
1. Introduction
2. Equivalent Realization of Fractional Passive Network Elements
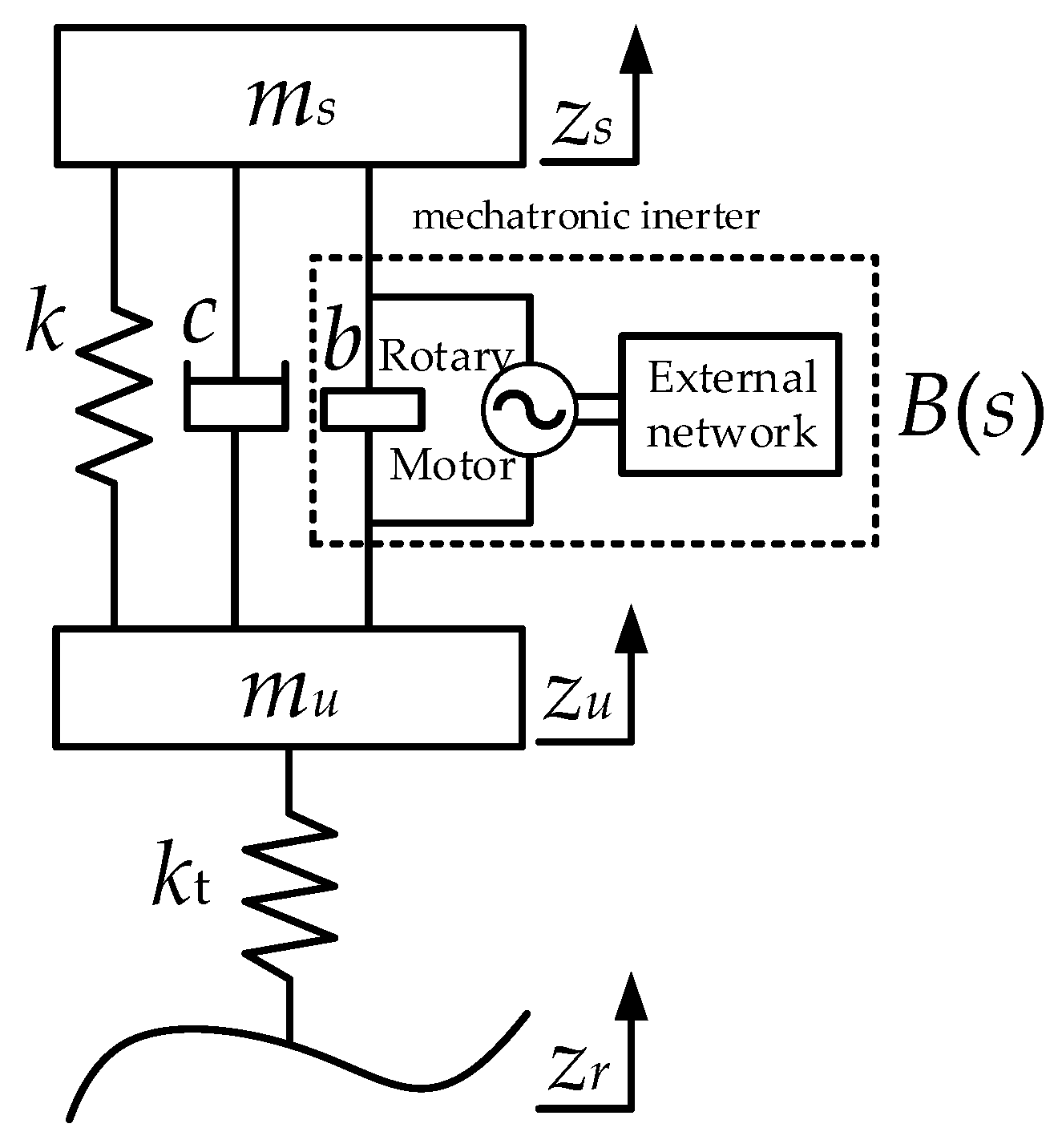
3. Model Construction of Vehicle Mechatronic ISD Suspension System
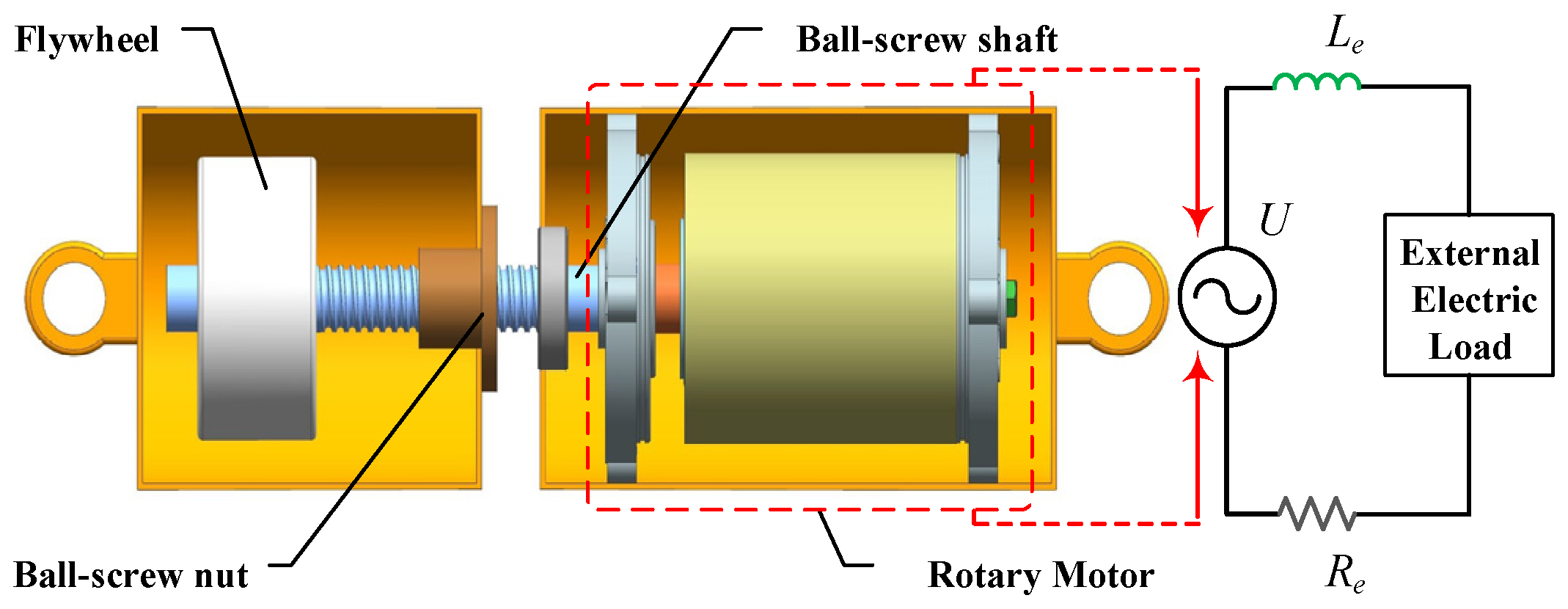
3.1. The Ball-Screw Mechatronic Inerter
3.2. Mechatronic ISD Suspension Structure Layout
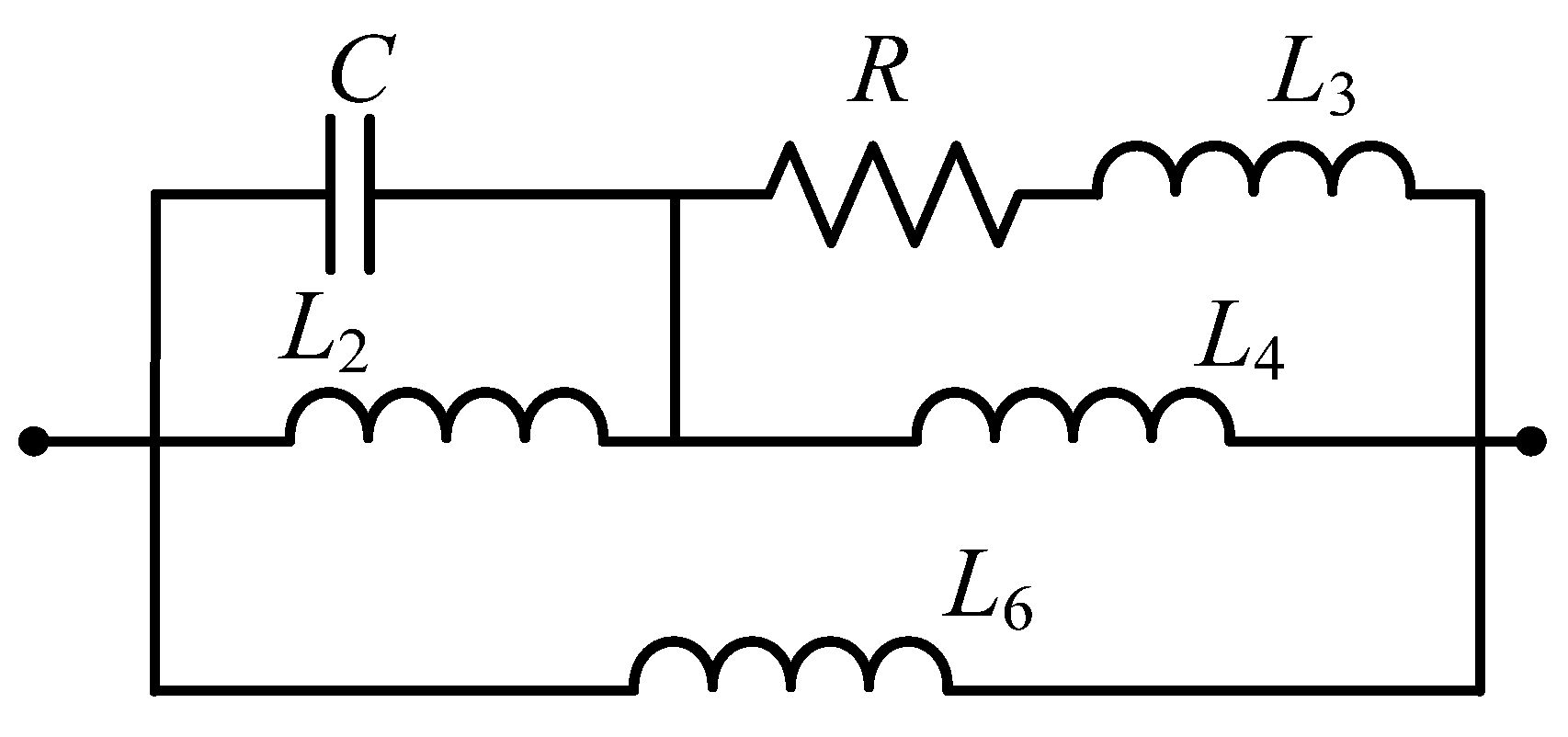
4. Parameter Optimization Design
4.1. Pattern Search Optimization Algorithm
4.2. Optimization Results
5. Simulation Analysis
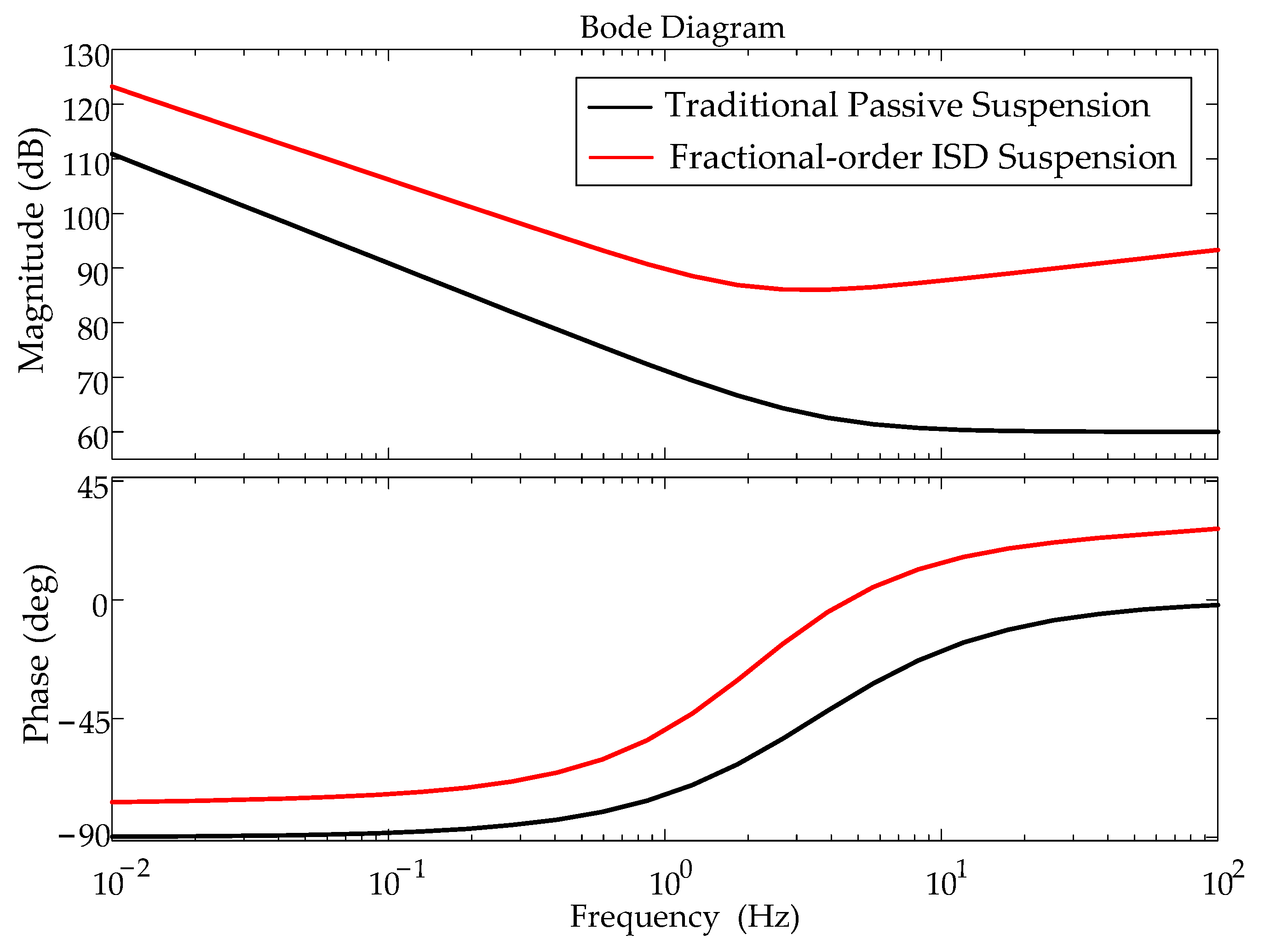
5.1. The Characteristics of Bode Diagram
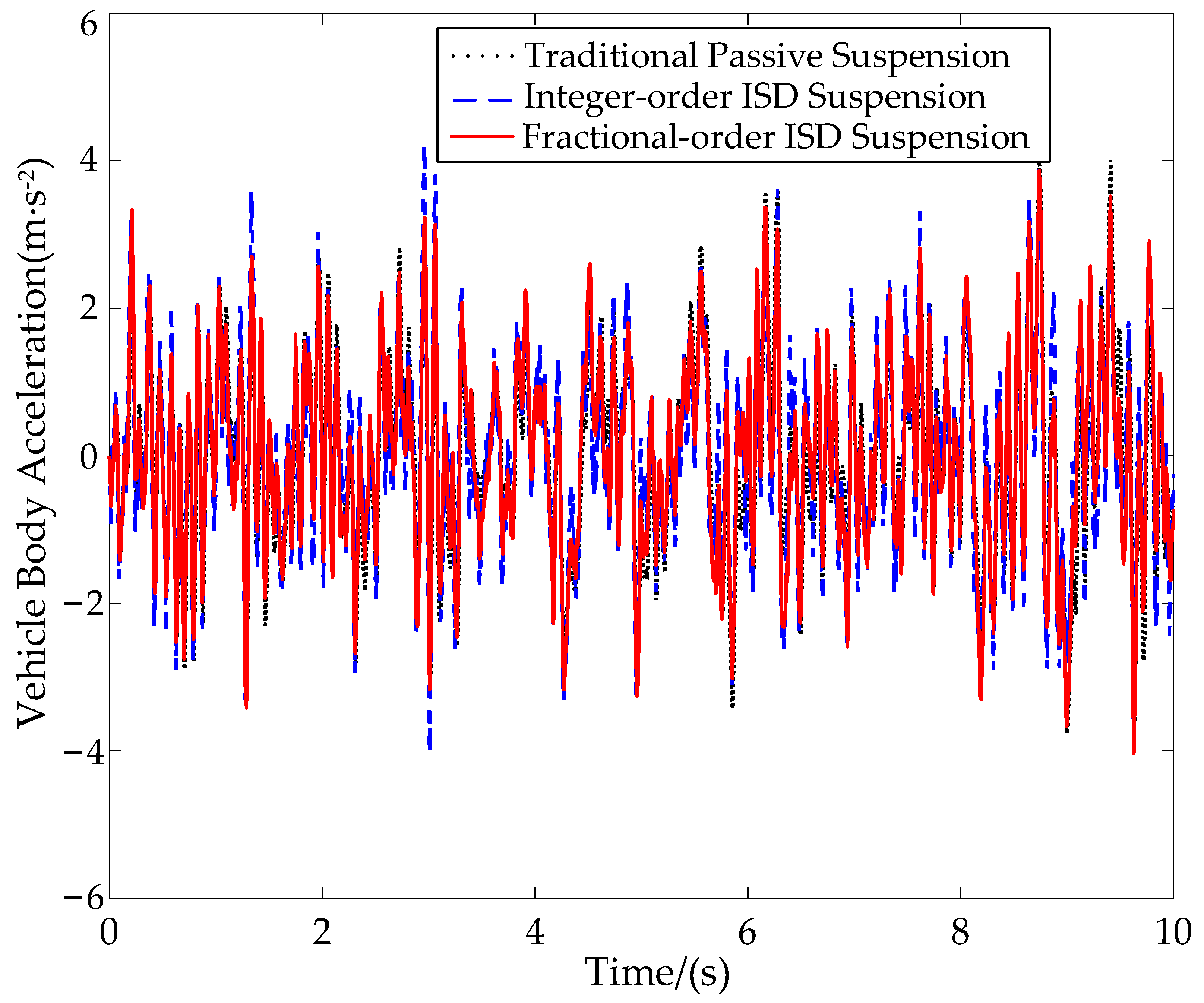
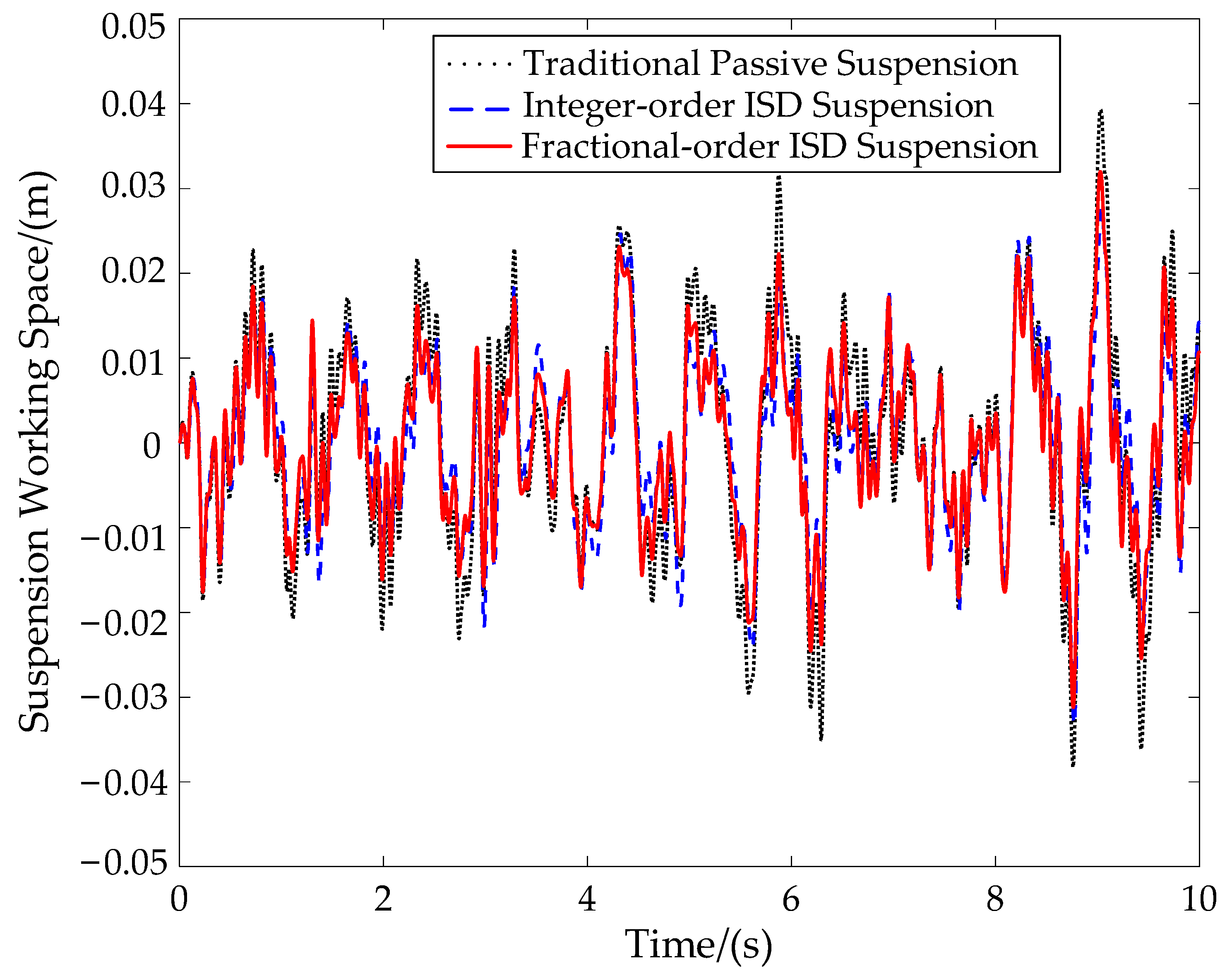
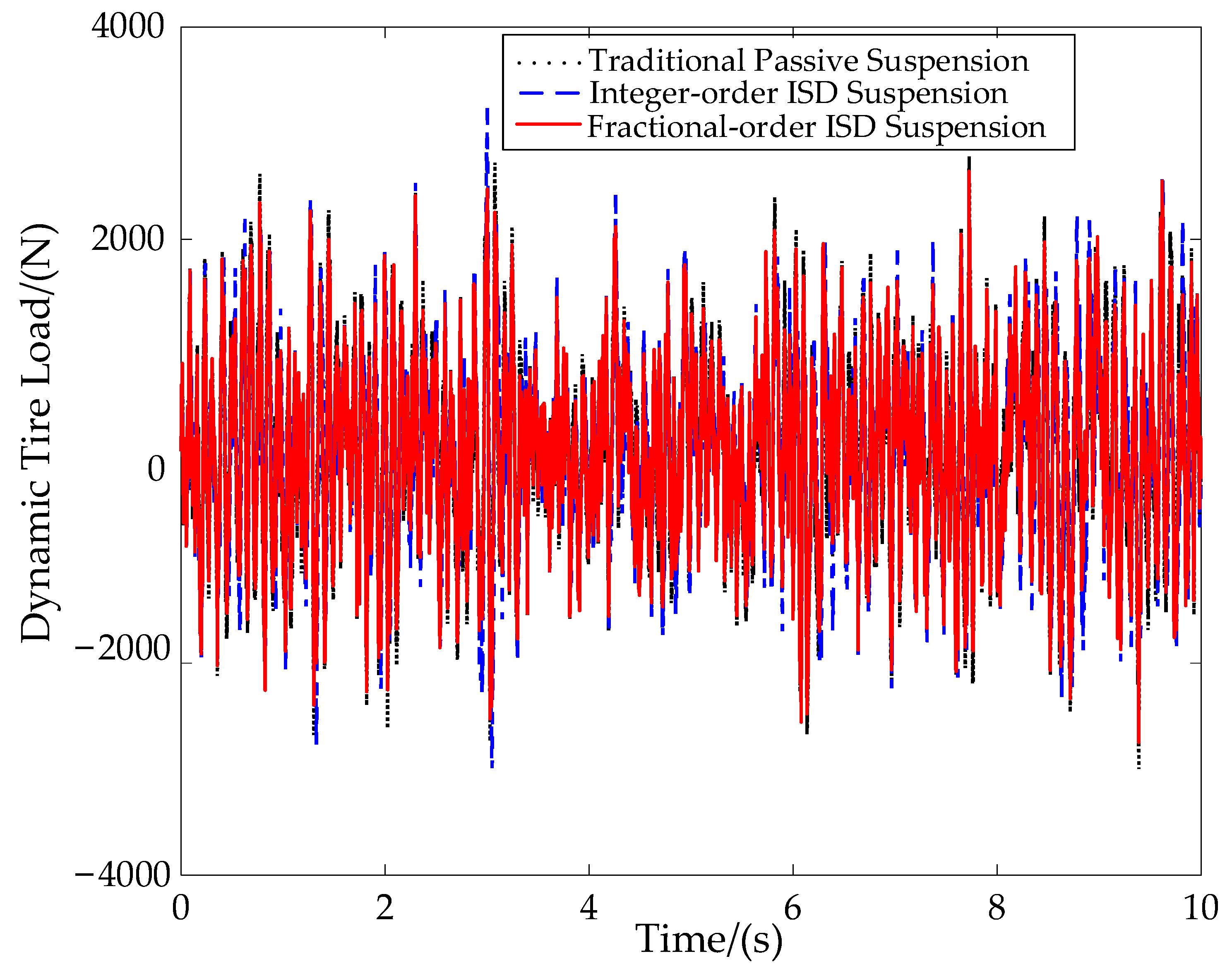
5.2. Random Road Input
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Smith, M.C. Synthesis of mechanical networks: The inerter. IEEE Trans. Autom. Control. 2002, 47, 1648–1662. [Google Scholar] [CrossRef]
- Wen, H.; Guo, J.; Li, Y.; Zhang, K. The transmissibility of a vibration isolation system with ball-screw inerter based on complex mass. J. Low Freq. Noise Vib. Act. Control. 2018, 37, 1097–1108. [Google Scholar] [CrossRef]
- Li, Y.; Cheng, Z.; Hu, N.; Yang, Y.; Xiao, Z. Modeling, design and experiments of a ball-screw inerter with mechanical diodes. J. Sound Vib. 2021, 504, 116121. [Google Scholar] [CrossRef]
- Wang, R.; Meng, X.; Shi, D.; Zhang, X.; Chen, Y.; Chen, L. Design and test of vehicle suspension system with inerters. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2014, 228, 2684–2689. [Google Scholar] [CrossRef]
- Papageorgiou, C.; Houghton, N.E.; Smith, M.C. Experimental Testing and Analysis of Inerter Devices. J. Dyn. Syst. Meas. Control. 2009, 131, 101–116. [Google Scholar] [CrossRef]
- Wang, F.; Hong, M.; Lin, T. Design and testing a hydraulic inerter. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 66–72. [Google Scholar] [CrossRef]
- Shen, Y.; Shi, D.; Chen, L.; Liu, Y.; Yang, X. Modeling and experimental tests of hydraulic electric inerter. Sci. China Technol. Sci. 2019, 62, 2161–2169. [Google Scholar] [CrossRef]
- Domenico, D.D.; Ricciardi, G.; Zhang, R. Optimal design and seismic performance of tuned fluid inerter applied to structures with friction pendulum isolators. Soil Dyn. Earthq. Eng. 2020, 132, 106099. [Google Scholar] [CrossRef]
- Liu, X.; Jiang, J.Z.; Titurus, B.; Harrison, A. Model identification methodology for fluid-based inerters. Mech. Syst. Signal Process. 2018, 106, 479–494. [Google Scholar] [CrossRef]
- Li, Y.; Howcroft, C.; Neild, S.A.; Jiang, J.Z. Using continuation analysis to identify shimmy-suppression devices for an aircraft main landing gear. J. Sound Vib. 2017, 408, 234–251. [Google Scholar] [CrossRef]
- Wang, F.; Liao, M.; Liao, B.; Su, W.; Chan, H. The performance improvements of train suspension systems with mechanical networks employing inerters. Veh. Syst. Dyn. 2009, 47, 805–830. [Google Scholar] [CrossRef]
- De Domenico, D.; Ricciardi, G. An enhanced base isolation system equipped with optimal tuned mass damper inerter (TMDI). Earthq. Eng. Struct. Dyn. 2018, 47, 1169–1192. [Google Scholar] [CrossRef]
- Wang, Q.; Zheng, Z.; Qiao, H.; De Domenico, D. Seismic protection of reinforced concrete continuous girder bridges with inerter-based vibration absorbers. Soil Dyn. Earthq. Eng. 2023, 164, 107526. [Google Scholar] [CrossRef]
- Smith, M.C.; Wang, F. Performance benefits in passive vehicle suspensions employing inerters. Veh. Syst. Dyn. 2004, 42, 235–257. [Google Scholar] [CrossRef]
- Shen, Y.; Jiang, J.Z.; Neild, S.A.; Chen, L. Vehicle vibration suppression using an inerter-based mechatronic device. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2020, 234, 2592–2601. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Sheng, X.; Jiang, J.Z.; Zhou, H.; Ren, W.; Zhang, Z. Vibration suppression of bridges under moving loads using the structure-immittance approach. Int. J. Mech. Sci. 2021, 211, 106792. [Google Scholar] [CrossRef]
- Wang, F.; Chan, H. Vehicle suspensions with a mechatronic network strut. Veh. Syst. Dyn. 2011, 49, 811–830. [Google Scholar] [CrossRef]
- Wang, F.; Hsieh, M.; Chen, H. Stability and performance analysis of a full-train system with inerters. Veh. Syst. Dyn. 2012, 50, 545–571. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Jiang, J.Z.; Wang, H.; Neild, S. Synthesis of essential-regular bicubic impedances. Int. J. Circuit Theory Appl. 2017, 45, 1482–1496. [Google Scholar] [CrossRef]
- You, H.; Shen, Y.; Xing, H.; Yang, S. Optimal control and parameters design for the fractional-order vehicle suspension system. J. Low Freq. Noise Vib. Act. Control. 2018, 37, 456–467. [Google Scholar] [CrossRef]
- Sun, H.; Li, R.; Xu, J.; Xu, F.; Zhang, B.; Dong, X. Fractional Modeling and Characteristic Analysis of Hydro-Pneumatic Suspension for Construction Vehicles. Processes 2021, 9, 1414. [Google Scholar] [CrossRef]
- Chen, E.; Xing, W.; Wang, M.; Ma, W.; Chang, Y. Study on chaos of nonlinear suspension system with fractional-order derivative under random excitation. Chaos Solitons Fractals 2021, 152, 111300. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, J.; Tai, Y.; Xu, X.; Chen, N. Critical damping design method of vibration isolation system with both fractional-order inerter and damper. Mech. Adv. Mater. Struct. 2022, 9, 1348–1359. [Google Scholar] [CrossRef]
- Shah, Z.M.; Khanday, F.A. Analysis of Disordered Dynamics in Polymer Nanocomposite Dielectrics for the Realization of Fractional-Order Capacitor. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 266–273. [Google Scholar] [CrossRef]
- Redman-White, W.; Kennedy, H.; Bodnar, R.; Lee, T. Adaptive Tuning of Large-Signal Resonant Circuits Using Phase-Switched Fractional Capacitance. IEEE Trans. Circuits Syst. II Express Briefs 2017, 64, 1072–1076. [Google Scholar] [CrossRef]
- Xue, D. Definition and Calculation of Fractional Calculus. In Fractional Calculus and Fractional-Order Control; Zhang, Z., Jiang, H., Eds.; Science Press: Beijing, China, 2018; pp. 31–35. ISBN 978-7-03-054398-1. [Google Scholar]
- Xue, D. Fractional Calculus Operators and Approximation of Systems. In Fractional Calculus and Fractional-Order Control; Zhang, Z., Jiang, H., Eds.; Science Press: Beijing, China, 2018; pp. 115–121. ISBN 978-7-03-054398-1. [Google Scholar]
- Shen, Y.; Hua, J.; Fan, W.; Liu, Y.; Yang, X.; Chen, L. Optimal design and dynamic performance analysis of a fractional-order electrical network-based vehicle mechatronic ISD suspension. Mech. Syst. Signal Process. 2023, 184, 109718. [Google Scholar] [CrossRef]
- Gasparo, M.G.; Papini, A.; Pasquali, A. Nonmonotone algorithms for pattern search methods. Numer. Algorithms 2001, 28, 171–186. [Google Scholar] [CrossRef]
- Shen, Y.; Chen, L.; Yang, X.; Shi, D.; Yang, J. Improved design of dynamic vibration absorber by using the inerter and its application in vehicle suspension. J. Sound Vib. 2016, 361, 148–158. [Google Scholar] [CrossRef]
- Sun, X.; Cai, Y.; Chen, L.; Liu, Y.; Wang, S. Vehicle height and posture control of the electronic air suspension system using the hybrid system approach. Veh. Syst. Dyn. 2016, 54, 328–352. [Google Scholar] [CrossRef]


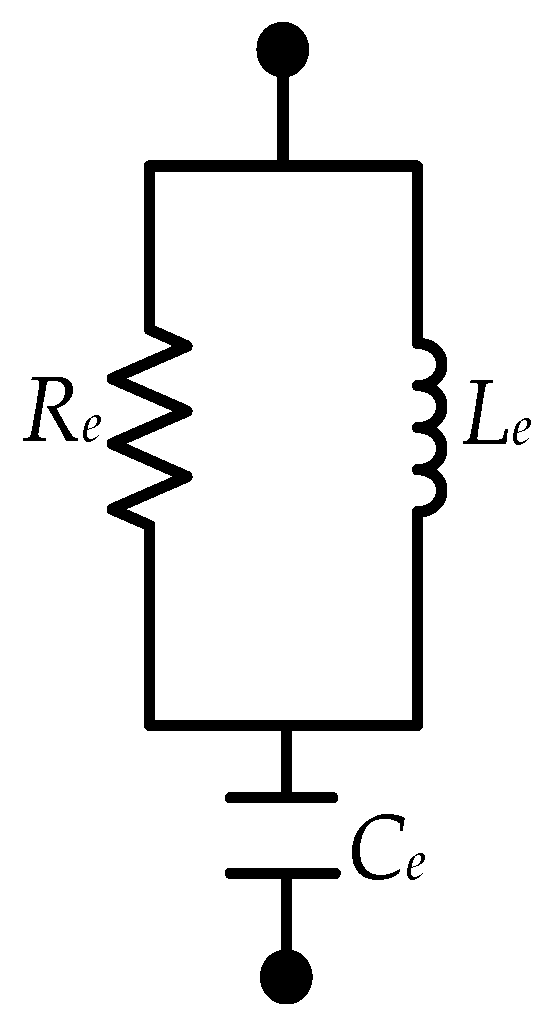
| Mechanical Network Elements | Impedance | Electrical Network Elements | Impedance |
|---|---|---|---|
| Spring | k/sα | Inductor | 1/Lsα |
| Damper | c | Resistor | 1/R |
| Inerter | bsβ | Capacitor | Csβ |
| Parameters | Values |
|---|---|
| Sprung Mass ms/kg | 320 |
| Unsprung Mass mu/kg | 45 |
| Spring Stiffness k/N m−1 | 22,000 |
| Tire Stiffness kt/N m−1 | 190,000 |
| Fractional-Order ISD Suspension | Integer-Order ISD Suspension | ||
|---|---|---|---|
| Parameters | Values | Parameters | Values |
| Inertance b/kg | 5 | Inertance b/kg | 13 |
| Damping coefficient c/N·s·m−1 | 1074 | Damping coefficient c/N·s·m−1 | 232 |
| Fractional-order inductance coefficient Le/H | 1.05 | Inductance coefficient Le/H | 1.34 |
| Fractional-order capacitance coefficient Ce/F | 0.06 | Capacitance coefficient Ce/F | 0.03 |
| Resistance coefficient Re/Ω | 320.73 | Resistance coefficient Re/Ω | 5.56 |
| Fractional-order inductance order α | 0.28 | - | - |
| Fractional-order capacitance order β | 0.81 | - | - |
| Performance Index | Traditional Passive Suspension | Integer-Order Isd Suspension | Improvement | Fractional-Order Isd Suspension | Improvement |
|---|---|---|---|---|---|
| RMS of vehicle body acceleration/(m·s−2) | 1.3096 | 1.3051 | 3.44% | 1.3042 | 4.12% |
| RMS of suspension working space/(m) | 0.0130 | 0.0101 | 22.31% | 0.0100 | 23.08% |
| RMS of dynamic tire load/(N) | 900.4704 | 875.8558 | 2.73% | 852.6704 | 5.31% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hua, J.; Shen, Y.; Yang, X.; Zhang, Y.; Liu, Y. Optimal Design of Fractional-Order Electrical Network for Vehicle Mechatronic ISD Suspension Using the Structure-Immittance Approach. World Electr. Veh. J. 2023, 14, 12. https://doi.org/10.3390/wevj14010012
Hua J, Shen Y, Yang X, Zhang Y, Liu Y. Optimal Design of Fractional-Order Electrical Network for Vehicle Mechatronic ISD Suspension Using the Structure-Immittance Approach. World Electric Vehicle Journal. 2023; 14(1):12. https://doi.org/10.3390/wevj14010012
Chicago/Turabian StyleHua, Jie, Yujie Shen, Xiaofeng Yang, Ying Zhang, and Yanling Liu. 2023. "Optimal Design of Fractional-Order Electrical Network for Vehicle Mechatronic ISD Suspension Using the Structure-Immittance Approach" World Electric Vehicle Journal 14, no. 1: 12. https://doi.org/10.3390/wevj14010012
APA StyleHua, J., Shen, Y., Yang, X., Zhang, Y., & Liu, Y. (2023). Optimal Design of Fractional-Order Electrical Network for Vehicle Mechatronic ISD Suspension Using the Structure-Immittance Approach. World Electric Vehicle Journal, 14(1), 12. https://doi.org/10.3390/wevj14010012









