Analysis of DC Winding Induced Voltage in Wound-Rotor Synchronous Machines by Using the Air-Gap Field Modulation Principle
Abstract
1. Introduction
- (1)
- The analytical MMF-permeance model is established, based on which the spatial–temporal characteristics of the air-gap flux density are analyzed.
- (2)
- The operating mechanism of the DC winding induced voltage in WRSM is analyzed first, and the harmonic order of the DC winding induced voltage is deduced and compared with that predicted by FEA.
- (3)
- Winding configurations of concentrated winding and distributed winding are included in the analysis.
2. Magneto-Motive Force-Permeance Modeling for the DC Winding Induced Voltage in WRSM
2.1. Specifications of the Analyzed WRSMs
2.2. MMF-Permeance Modelling
- The steel permeability is infinite.
- Flux lines are perpendicular to the steel surface.
- The effect of finite stack length is negligible.
2.2.1. Open-circuit condition
2.2.2. Armature Reaction Condition
2.2.3. On-Load Condition
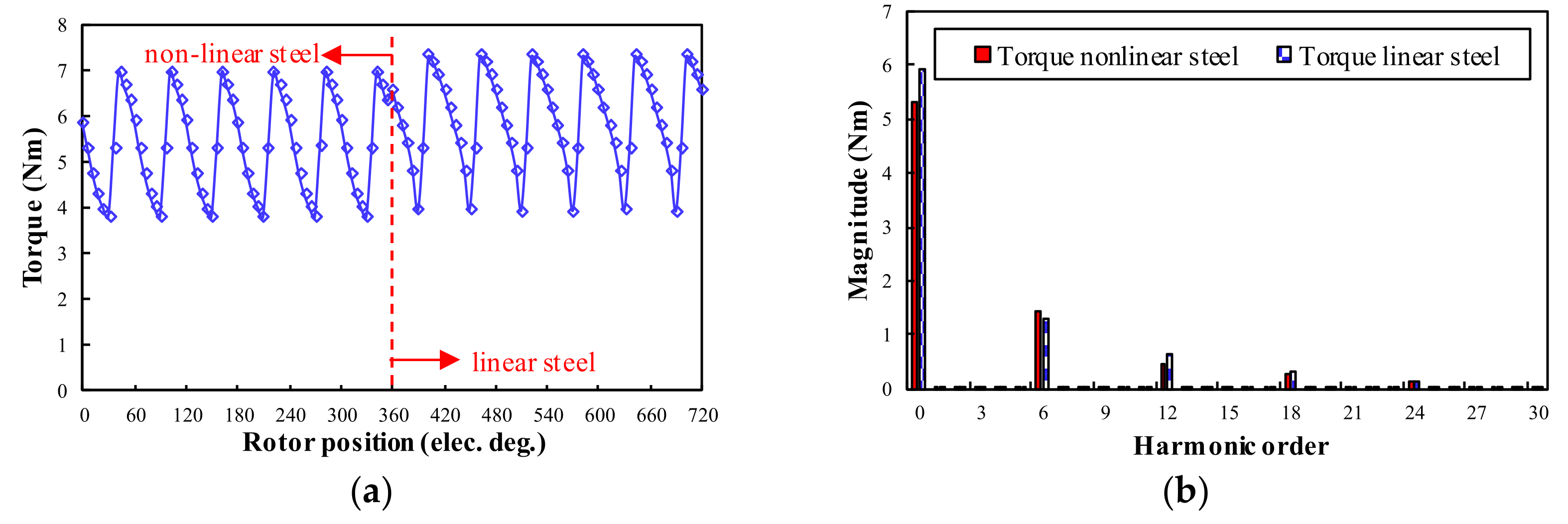
3. FEA Verification
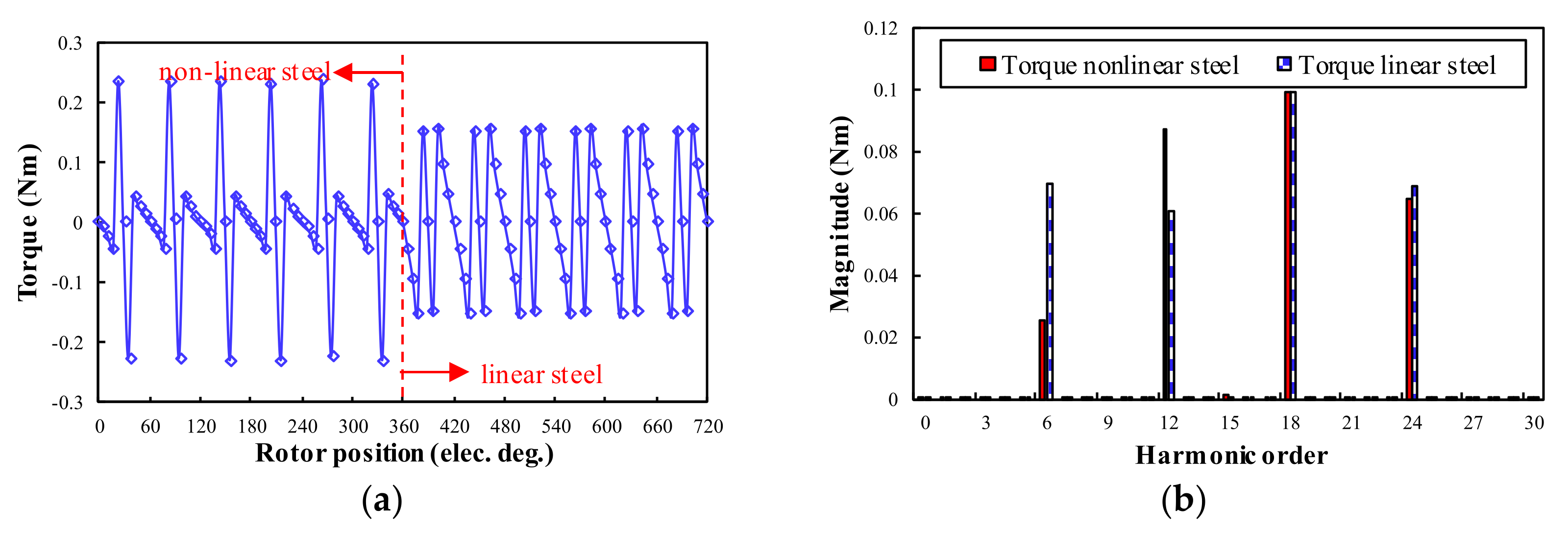
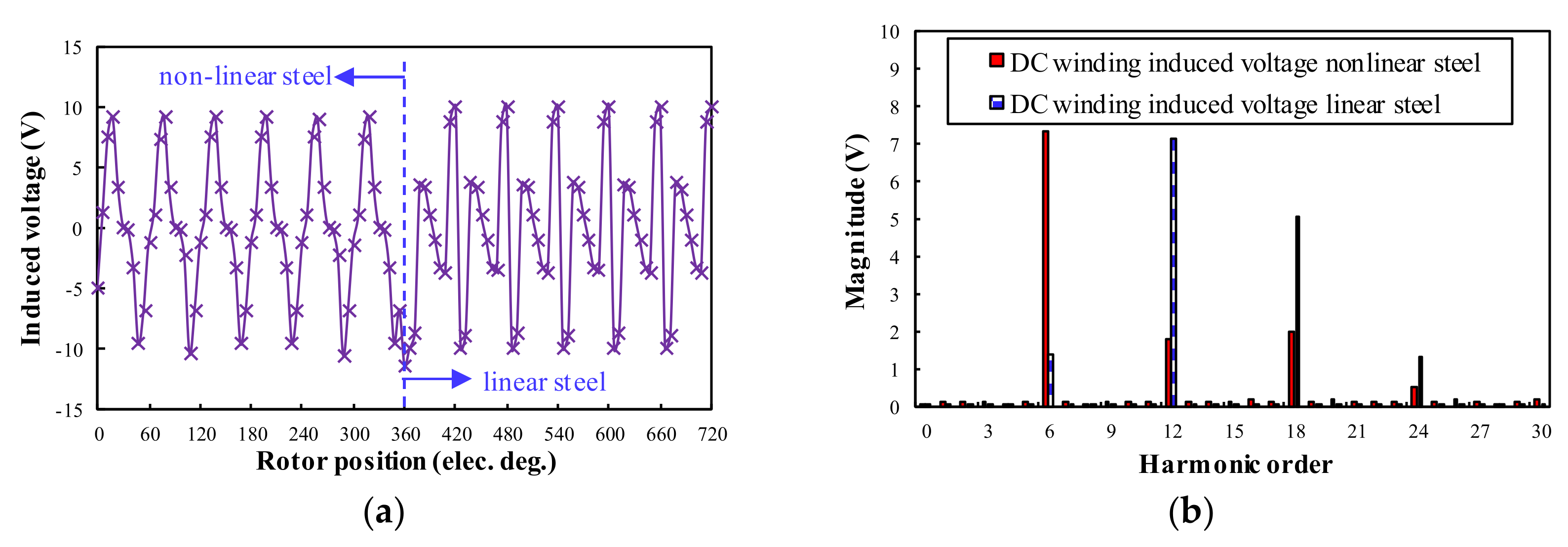
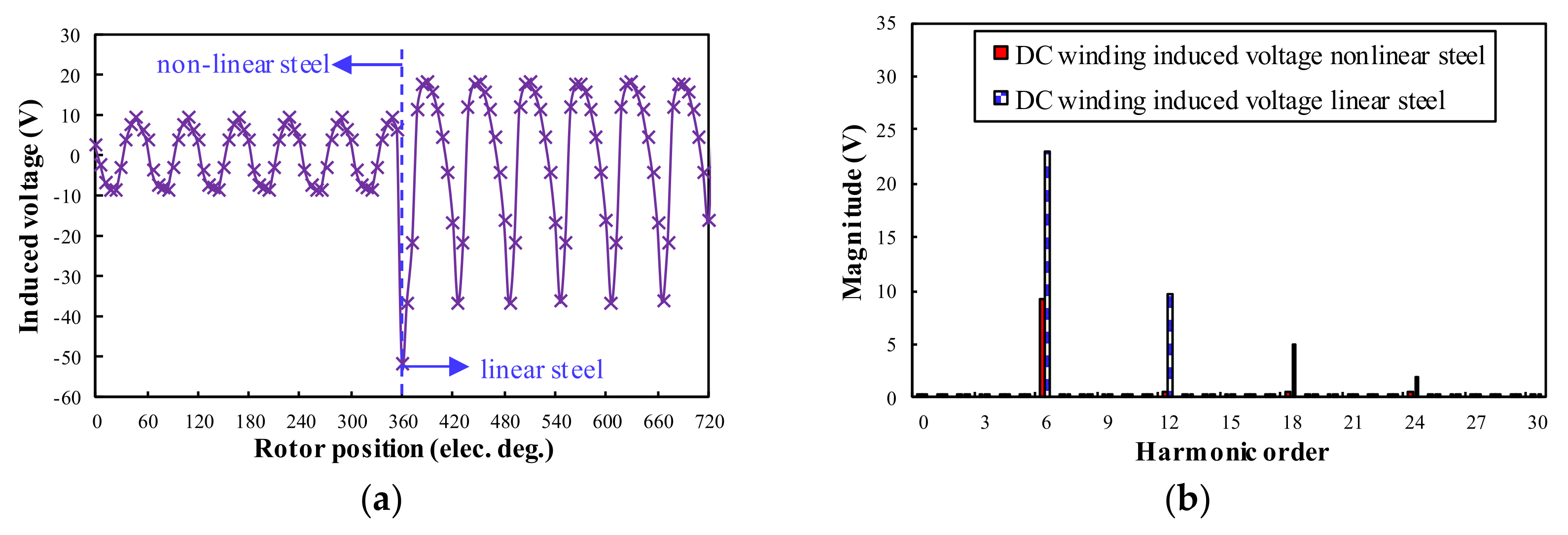
3.1. Open-Circuit Condition
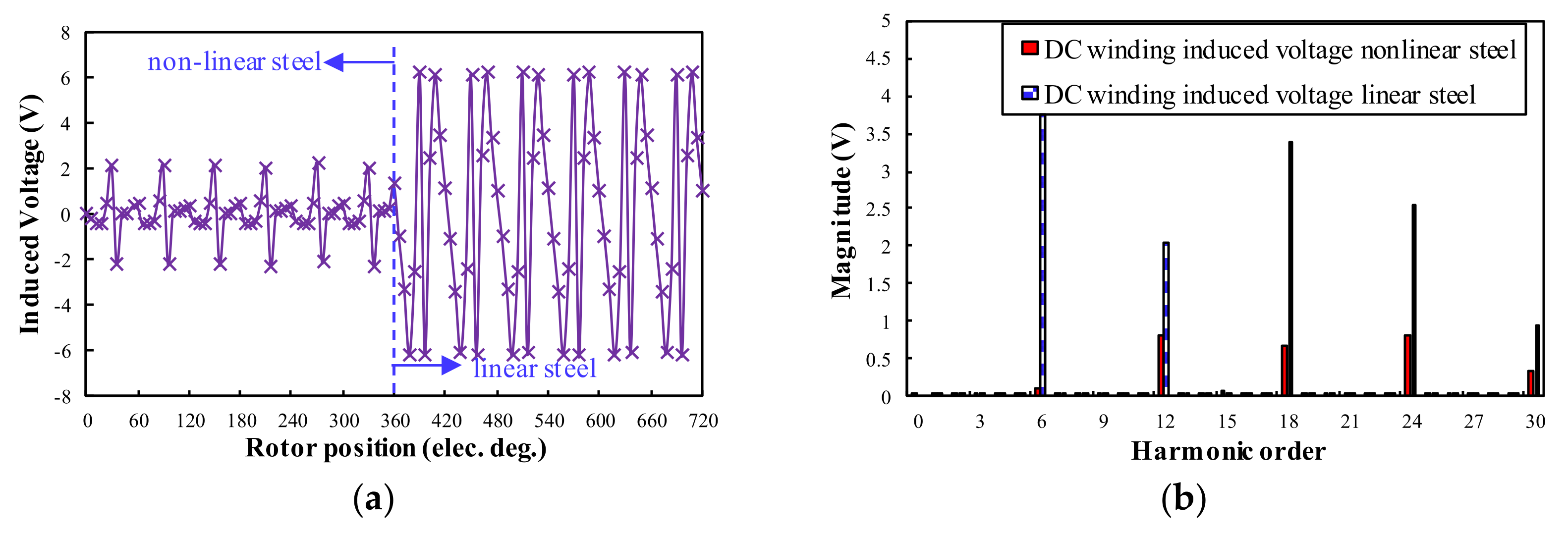
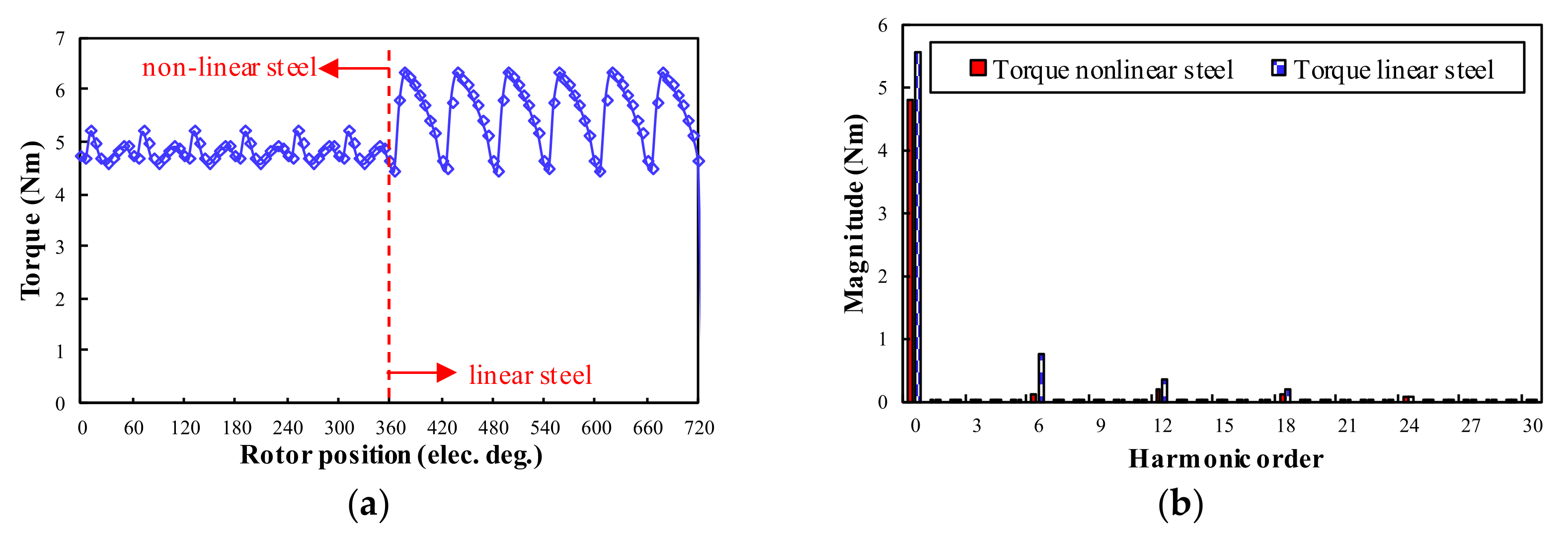
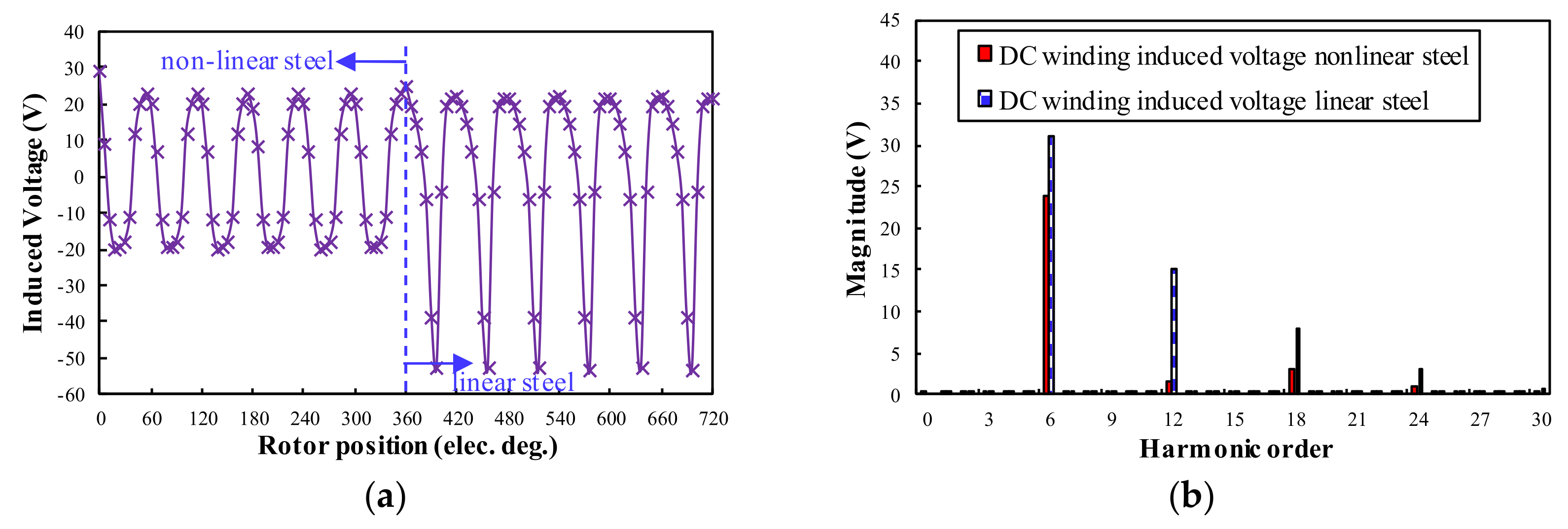
3.2. On-Load Condition
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Raghuraman, B.; Nategh, S.; Sidiropoulos, N.; Petersson, L.; Boglietti, A. Sustainability aspects of electrical machines for E-mobility applications part I: A design with reduced rare-earth elements. In Proceedings of the 47th Annual Conference of the IEEE Industrial Electronics Society, Toronto, ON, Canada, 13–16 October 2021. [Google Scholar]
- Boldea, I.; Tutelea, L.N.; Parsa, L.; Dorrell, D. Automotive Electric Propulsion Systems With Reduced or No Permanent Magnets: An Overview. IEEE Trans. Ind. Electron. 2014, 61, 5696–5711. [Google Scholar] [CrossRef]
- Widmer, J.D.; Martin, R.; Kimiabeigi, M. Electric vehicle traction motors without rare earth magnets. Sustain. Mater. Technol. 2015, 3, 7–13. [Google Scholar] [CrossRef]
- Lipo, T.A.; Du, Z.S. Synchronous motor drives-a forgotten option. In Proceedings of the 2015 Intl Aegean Conference on Electrical Machines & Power Electronics, 2015 Intl Conference on Optimization of Electrical & Electronic Equipment & 2015 Intl Symposium on Advanced Electromechanical Motion Systems, Side, Turkey, 2–4 September 2015; pp. 1–5. [Google Scholar]
- The BMW iX3 between Design and Art. Available online: https://www.bmw.com/en/design/aerodynamic-rims-BMW-iX3-design-meets-art.html (accessed on 9 October 2021).
- Specifications of the Renault ZOE Motor. Available online: https://www.renaultgroup.com/en/news-on-air/news/the-renault-zoe-motor-energy-efficiency-and-power/ (accessed on 9 October 2021).
- MAHLE Develops Highly Efficient Magnet-Free Electric Motor. Available online: https://www.mahle.com/en/news-and-press/press-releases/mahle-develops-highly-efficient-magnet-free-electric-motor--82368 (accessed on 9 October 2021).
- Nie, Y.; Brown, I.P.; Ludois, D.C. Deadbeat-Direct Torque and Flux Control for Wound Field Synchronous Machines. IEEE Trans. Ind. Electron. 2017, 65, 2069–2079. [Google Scholar] [CrossRef]
- Tang, J.; Liu, Y.; Lundberg, S. Estimation Algorithm for Current and Temperature of Field Winding in Electrically Excited Synchronous Machines With High-Frequency Brushless Exciters. IEEE Trans. Power Electron. 2020, 36, 3512–3523. [Google Scholar] [CrossRef]
- Chu, W.Q.; Zhu, Z.Q.; Chen, J.T. Simplified Analytical Optimization and Comparison of Torque Densities Between Electrically Excited and Permanent-Magnet Machines. IEEE Trans. Ind. Electron. 2013, 61, 5000–5011. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, J.; Xiao, Y.; Zhou, L.; Zhu, Z.Q. Two-Level Surrogate-Assisted Transient Parameters Design Optimization of a Wound-Field Synchronous Machine. IEEE Trans. Energy Convers. 2021, 37, 737–747. [Google Scholar] [CrossRef]
- Chai, W.; Kwon, B.-I. Design of an asymmetric rotor pole for wound field synchronous machines. CES Trans. Electr. Mach. Syst. 2021, 5, 321–327. [Google Scholar] [CrossRef]
- Nuzzo, S.; Galea, M.; Bolognesi, P.; Vakil, G.; Fallows, D.; Gerada, C.; Brown, N.L. A Methodology to Remove Stator Skew in Small–Medium Size Synchronous Generators via Innovative Damper Cage Designs. IEEE Trans. Ind. Electron. 2018, 66, 4296–4307. [Google Scholar] [CrossRef]
- Quadri, Q.H.; Nuzzo, S.; Rashed, M.; Gerada, C.; Galea, M. Modeling of Classical Synchronous Generators Using Size-Efficient Lookup Tables With Skewing Effect. IEEE Access 2019, 7, 174551–174561. [Google Scholar] [CrossRef]
- Wang, Y.; Nuzzo, S.; Gerada, C.; Zhang, H.; Zhao, W.; Galea, M. Integrated Damper Cage for THD Improvements of Variable Speed Salient-Pole Synchronous Generators for the More Electric Aircraft. IEEE Trans. Transp. Electrif. 2021, 8, 3618–3629. [Google Scholar] [CrossRef]
- Wang, Y.; Nuzzo, S.; Zhang, H.; Zhao, W.; Gerada, C.; Galea, M. Challenges and Opportunities for Wound Field Synchronous Generators in Future More Electric Aircraft. IEEE Trans. Transp. Electrif. 2020, 6, 1466–1477. [Google Scholar] [CrossRef]
- Fu, X.; Qi, Q.; Tan, L. Design and Analysis of Brushless Wound Field Synchronous Machine With Electro-Magnetic Coupling Resonators. IEEE Access 2019, 7, 173636–173645. [Google Scholar] [CrossRef]
- Ludois, D.C.; Reed, J.K.; Hanson, K. Capacitive Power Transfer for Rotor Field Current in Synchronous Machines. IEEE Trans. Power Electron. 2012, 27, 4638–4645. [Google Scholar] [CrossRef]
- Fallows, D.; Nuzzo, S.; Galea, M. Exciterless Wound-Field Medium-Power Synchronous Machines: Their History and Future. IEEE Ind. Electron. Mag. 2021. [Google Scholar] [CrossRef]
- Wu, Z.Z.; Zhu, Z.Q.; Wang, C.; Mipo, J.C.; Personnaz, S.; Farah, P. Reduction of Open-Circuit DC-Winding-Induced Voltage in Wound Field Switched Flux Machines by Skewing. IEEE Trans. Ind. Electron. 2018, 66, 1715–1726. [Google Scholar] [CrossRef]
- Zulu, A.; Mecrow, B.C.; Armstrong, M. A Wound-Field Three-Phase Flux-Switching Synchronous Motor With All Excitation Sources on the Stator. IEEE Trans. Ind. Appl. 2010, 46, 2363–2371. [Google Scholar] [CrossRef]
- Wu, Z.; Zhu, Z.-Q.; Wang, C.; Mipo, J.-C.; Personnaz, S.; Farah, P. Analysis and Reduction of On-Load DC Winding Induced Voltage in Wound Field Switched Flux Machines. IEEE Trans. Ind. Electron. 2019, 67, 2655–2666. [Google Scholar] [CrossRef]
- Wu, Z.; Zhu, Z.Q.; Hua, W.; Akehurst, S.; Zhu, X.; Zhang, W.; Hu, J.; Li, H.; Zhu, J. Analysis and Suppression of Induced Voltage Pulsation in DC Winding of Five-Phase Wound-Field Switched Flux Machines. IEEE Trans. Energy Convers. 2019, 34, 1890–1905. [Google Scholar] [CrossRef]
- Yu, L.; Zhang, M.; Zhang, Z.; Jiang, B. Reduction of Field-Winding-Induced Voltage in a Doubly Salient Brushless DC Generator With Stator-Damper Winding. IEEE Trans. Ind. Electron. 2021, 69, 7767–7775. [Google Scholar] [CrossRef]
- Sun, X.; Zhu, Z.Q. Investigation of DC Winding Induced Voltage in Hybrid-Excited Switched-Flux Permanent Magnet Machine. IEEE Trans. Ind. Appl. 2020, 56, 3594–3603. [Google Scholar] [CrossRef]
- Sun, X.; Zhu, Z.Q.; Wei, F.R. Voltage Pulsation Induced in DC Field Winding of Different Hybrid Excitation Switched Flux Machines. IEEE Trans. Ind. Appl. 2021, 57, 4815–4830. [Google Scholar] [CrossRef]
- Zhang, W.; Hua, W.; Wu, Z.; Zhao, G.; Wang, Y.; Xia, W. Analysis of DC Winding Induced Voltage in Wound-Field Flux-Switching Machine With Air-Gap Field Modulation Principle. IEEE Trans. Ind. Electron. 2021, 69, 2300–2311. [Google Scholar] [CrossRef]
- Cheng, M.; Han, P.; Hua, W. General Airgap Field Modulation Theory for Electrical Machines. IEEE Trans. Ind. Electron. 2017, 64, 6063–6074. [Google Scholar] [CrossRef]
- Wu, Z.Z.; Zhu, Z.Q. Analysis of Air-Gap Field Modulation and Magnetic Gearing Effects in Switched Flux Permanent Magnet Machines. IEEE Trans. Magn. 2015, 51, 8105012. [Google Scholar] [CrossRef]
- Li, D.; Qu, R.; Li, J.; Xiao, L.; Wu, L.; Xu, W. Analysis of Torque Capability and Quality in Vernier Permanent-Magnet Machines. IEEE Trans. Ind. Appl. 2015, 52, 125–135. [Google Scholar] [CrossRef]
- Zhu, X.; Hua, W.; Wu, Z.; Huang, W.; Zhang, H.; Cheng, M. Analytical Approach for Cogging Torque Reduction in Flux-Switching Permanent Magnet Machines Based on Magnetomotive Force-Permeance Model. IEEE Trans. Ind. Electron. 2017, 65, 1965–1979. [Google Scholar] [CrossRef]
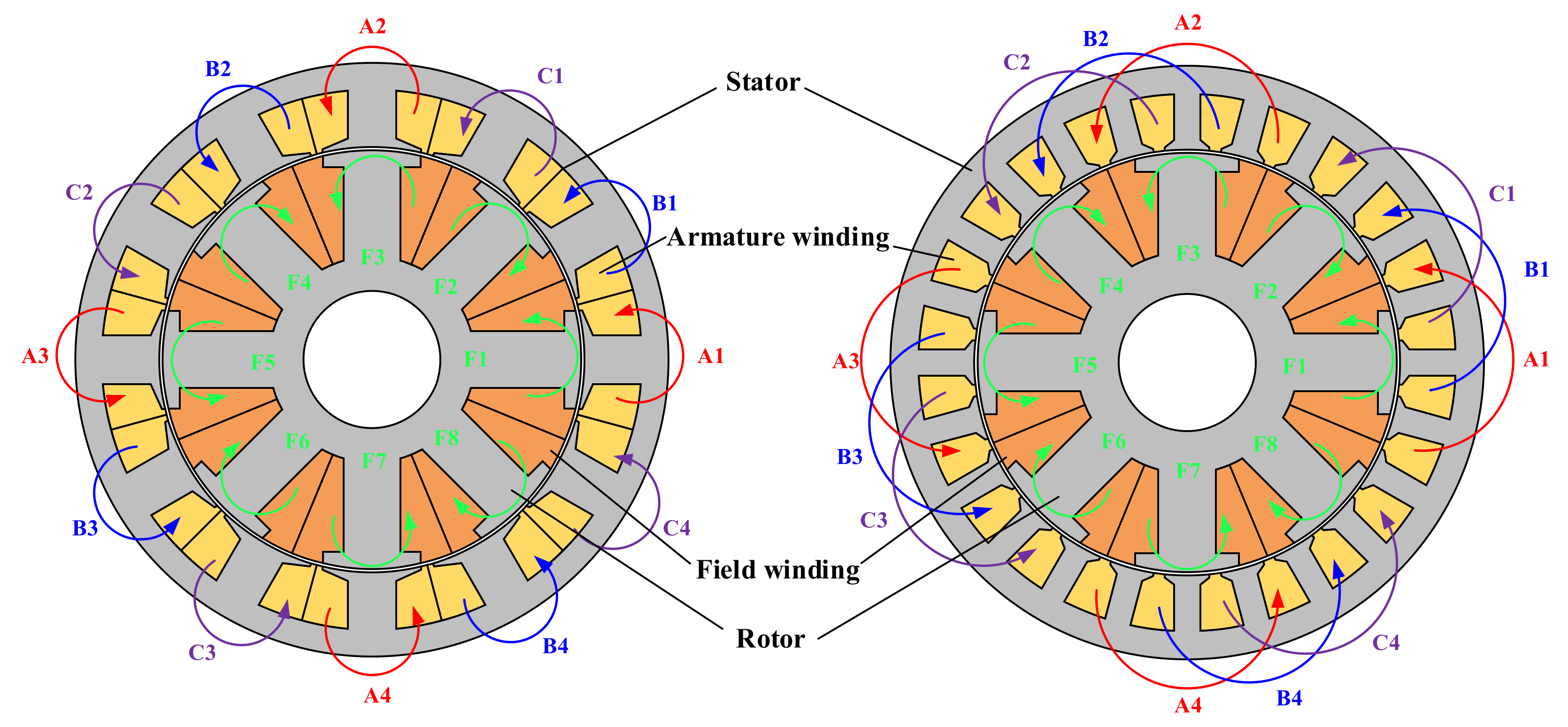
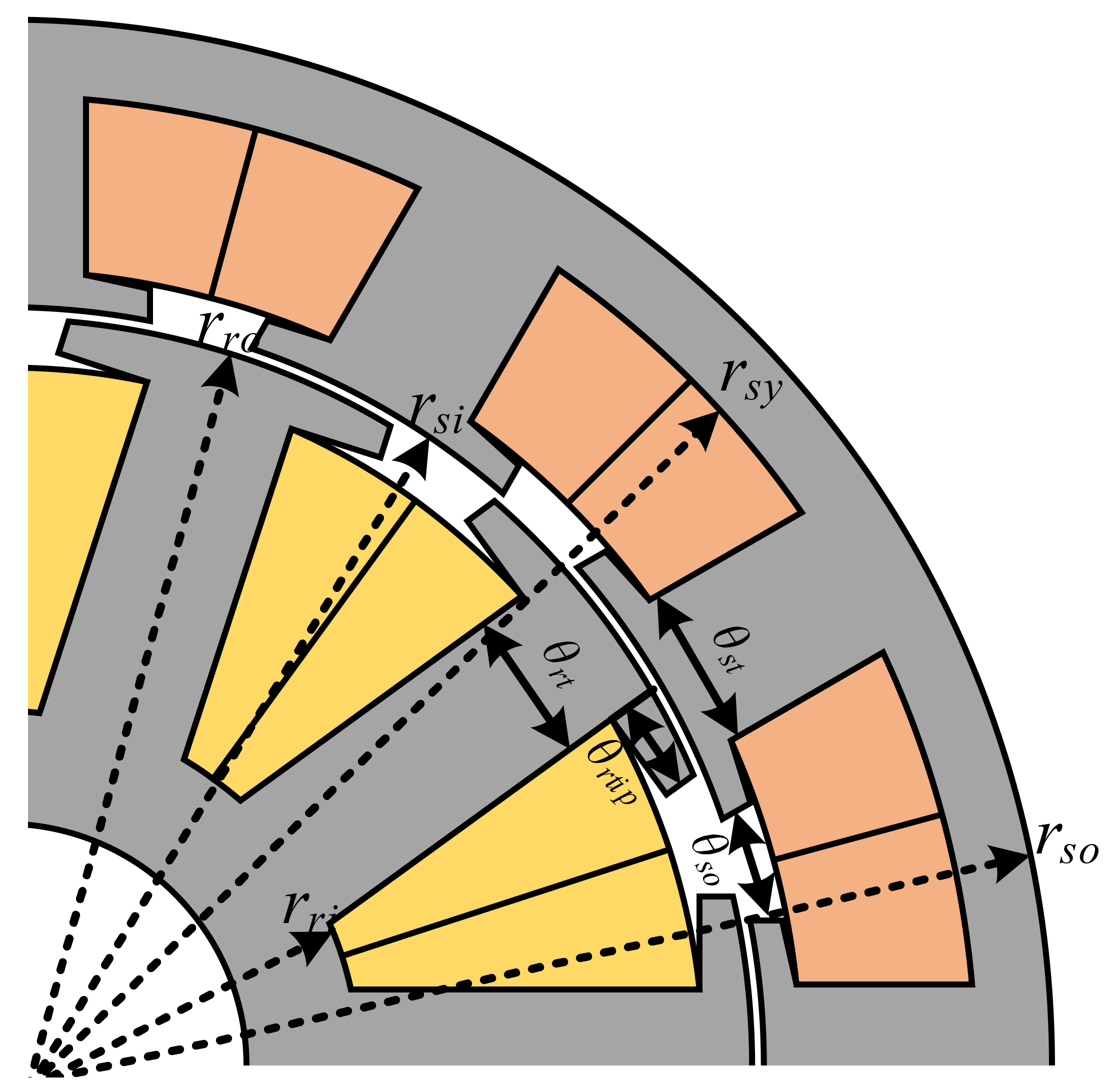
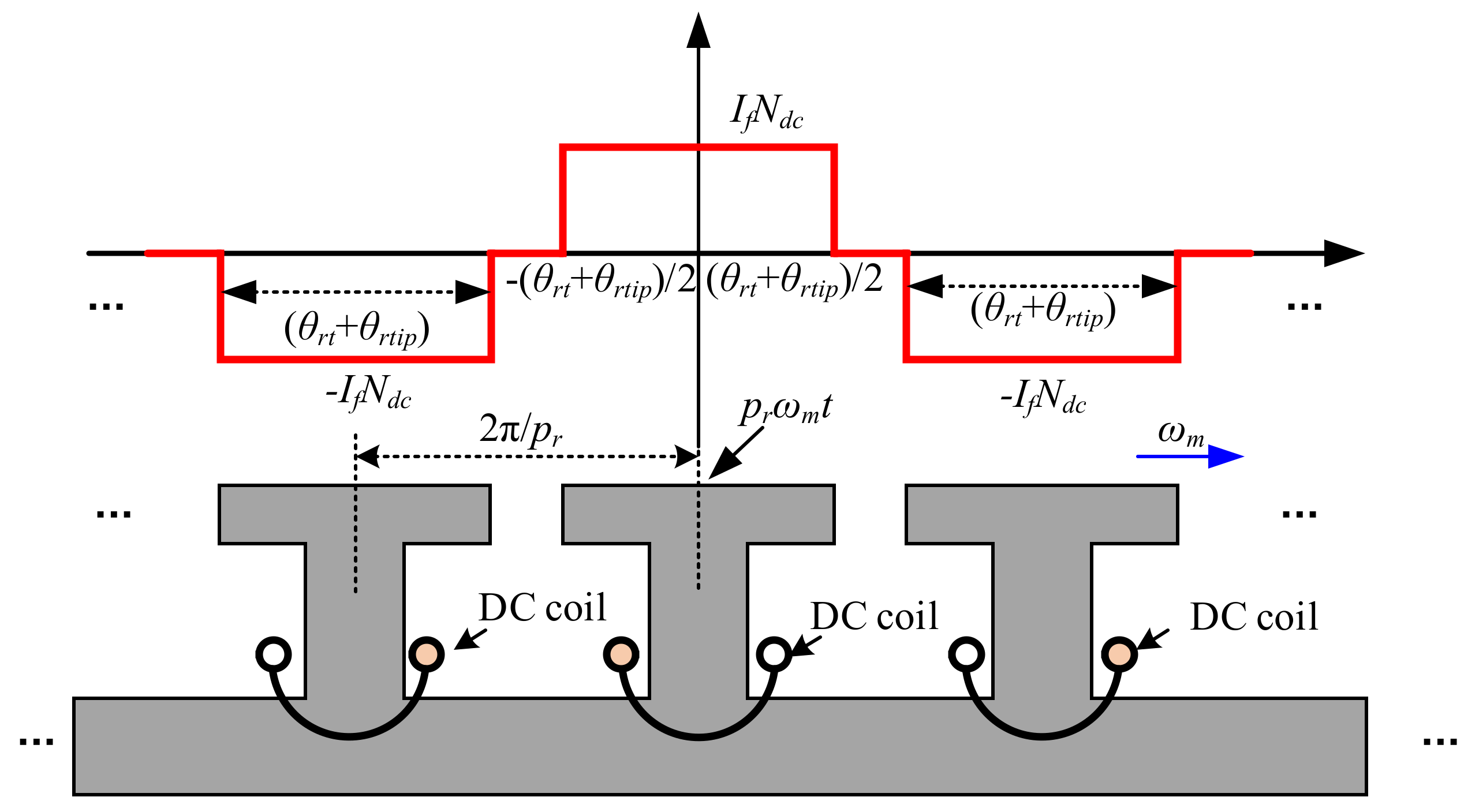
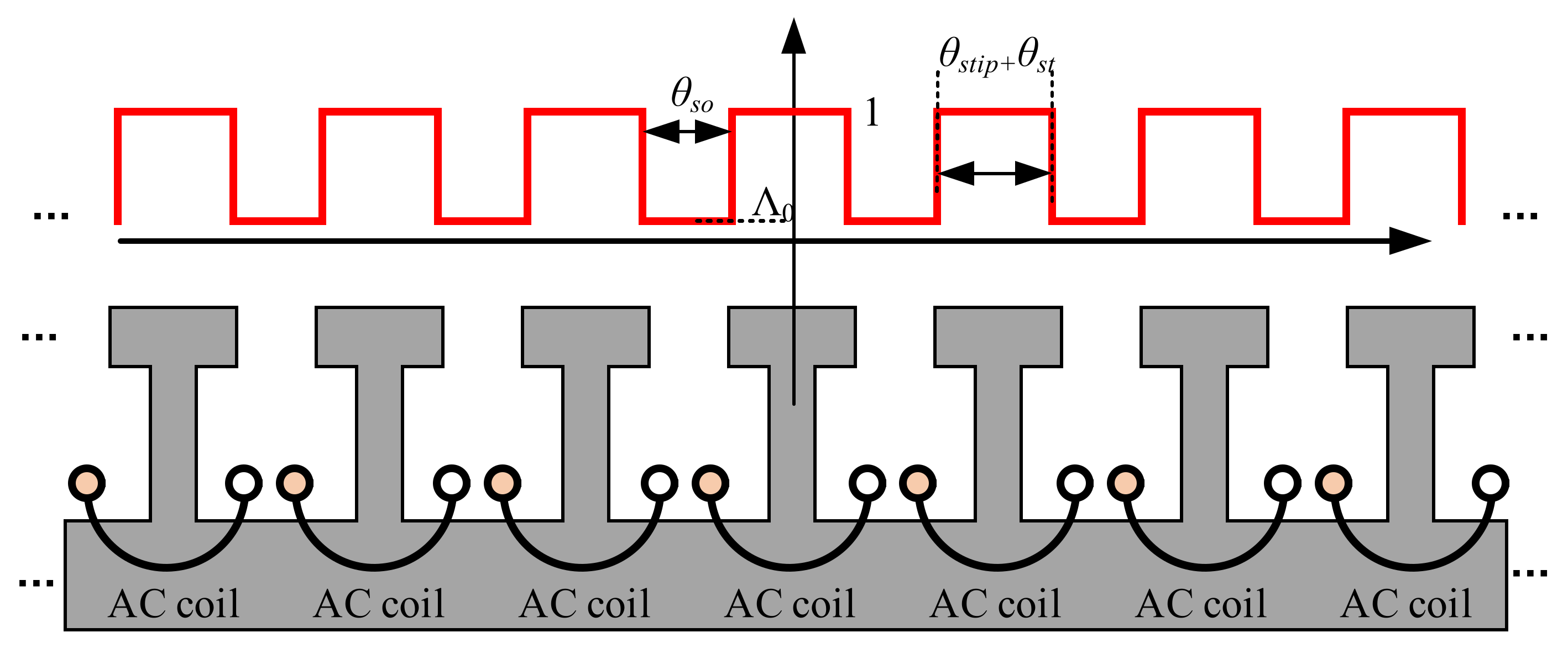

| Parameters | Unit | WRSM | |
|---|---|---|---|
| Stator slot number, Ns | - | 12 | 24 |
| Rotor pole pair number, pr | - | 4 | 4 |
| Stator outer radius, rso | mm | 45 | 45 |
| Stator yoke radius, rsy | mm | 40.97 | 40.75 |
| Stator inner radius, rsi | mm | 32.25 | 32.29 |
| Slot opening arc, θso | deg. | 3.196 | 3.018 |
| Stator tooth arc, θst | deg. | 13.15 | 7.133 |
| Single side air-gap width, g | mm | 0.5 | 0.5 |
| Rotor outer radius, rro | mm | 31.75 | 31.79 |
| Rotor yoke radius, rry | mm | 15.61 | 16.8 |
| Rotor tooth arc, θrt | deg. | 15.54 | 15.85 |
| Rotor tip arc, θrtip | deg. | 5.71 | 6.39 |
| Shaft radius, rsh | mm | 10.4 | 10.4 |
| Stack length, lstk | mm | 50 | 50 |
| Lamination steel type | - | M270-35 | |
| Armature coil turns, Nac | - | 36 | 36 |
| DC coil turns, Ndc | - | 90 | 90 |
| Packing factor, kpf | - | 0.5 | 0.5 |
| Rotor speed, nr | rpm | 1000 | 1000 |
| Armature winding copper loss, pcac | W | 60 | 60 |
| DC winding copper loss, pcdc | W | 60 | 60 |
| Parameters | WRSM | |
|---|---|---|
| Stator-slot/Rotor-pole-pair | 12/4 | 24/4 |
| Npeopen | 6 | 6 |
| Parameters | WRSM | |
|---|---|---|
| Stator slot/Rotor pole pair | 12/4 | 24/4 |
| Npear | 6 | 6 |
| Parameters | WRSM | |
|---|---|---|
| Stator slot/Rotor pole pair | 12/4 | 24/4 |
| Npeol | 6 | 6 |
| Parameters | Linear Steel | Non-Linear Steel | ||
|---|---|---|---|---|
| Stator slot/Rotor pole pair | 12/4 | 24/4 | 12/4 | 24/4 |
| Npeopen | 6 | 6 | 6 | 6 |
| Npeol | 6 | 6 | 6 | 6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Fan, Y.; Zhu, Z.Q.; Wu, Z.; Hua, W.; Cheng, M. Analysis of DC Winding Induced Voltage in Wound-Rotor Synchronous Machines by Using the Air-Gap Field Modulation Principle. World Electr. Veh. J. 2022, 13, 215. https://doi.org/10.3390/wevj13110215
Zhang W, Fan Y, Zhu ZQ, Wu Z, Hua W, Cheng M. Analysis of DC Winding Induced Voltage in Wound-Rotor Synchronous Machines by Using the Air-Gap Field Modulation Principle. World Electric Vehicle Journal. 2022; 13(11):215. https://doi.org/10.3390/wevj13110215
Chicago/Turabian StyleZhang, Wentao, Ying Fan, Z. Q. Zhu, Zhongze Wu, Wei Hua, and Ming Cheng. 2022. "Analysis of DC Winding Induced Voltage in Wound-Rotor Synchronous Machines by Using the Air-Gap Field Modulation Principle" World Electric Vehicle Journal 13, no. 11: 215. https://doi.org/10.3390/wevj13110215
APA StyleZhang, W., Fan, Y., Zhu, Z. Q., Wu, Z., Hua, W., & Cheng, M. (2022). Analysis of DC Winding Induced Voltage in Wound-Rotor Synchronous Machines by Using the Air-Gap Field Modulation Principle. World Electric Vehicle Journal, 13(11), 215. https://doi.org/10.3390/wevj13110215











