A Stator Fault Diagnosis Method Based on the Offline Motor Parameter Measurement for PMSM
Abstract
:1. Introduction
2. Line-to-Line Resistance and Inductance Analysis of a Healthy Motor
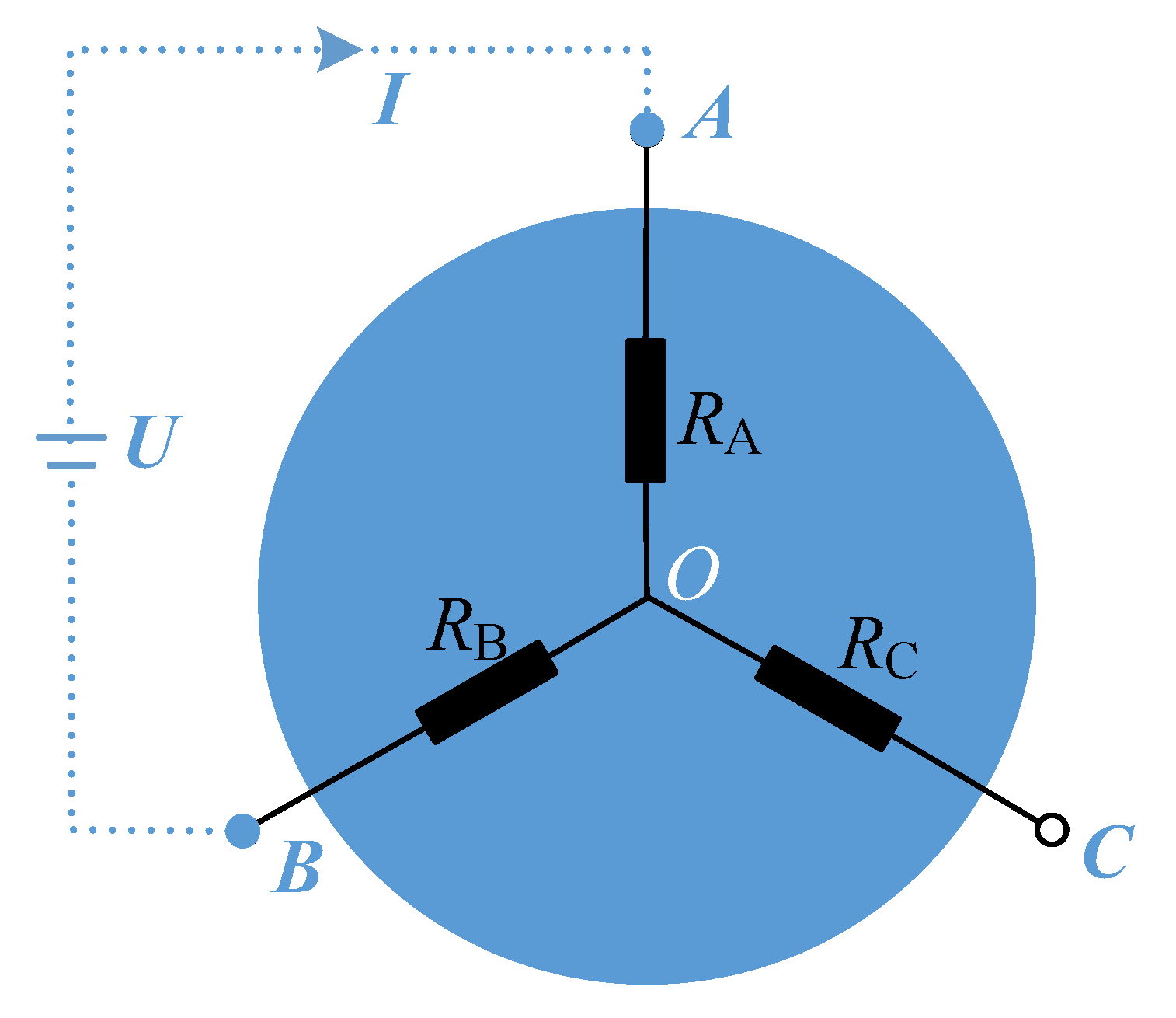
2.1. Line-to-Line Resistance Analysis
2.2. Line-to-Line Inductance Analysis
3. Line-to-Line Resistance and Inductance Analysis for Stator Fault
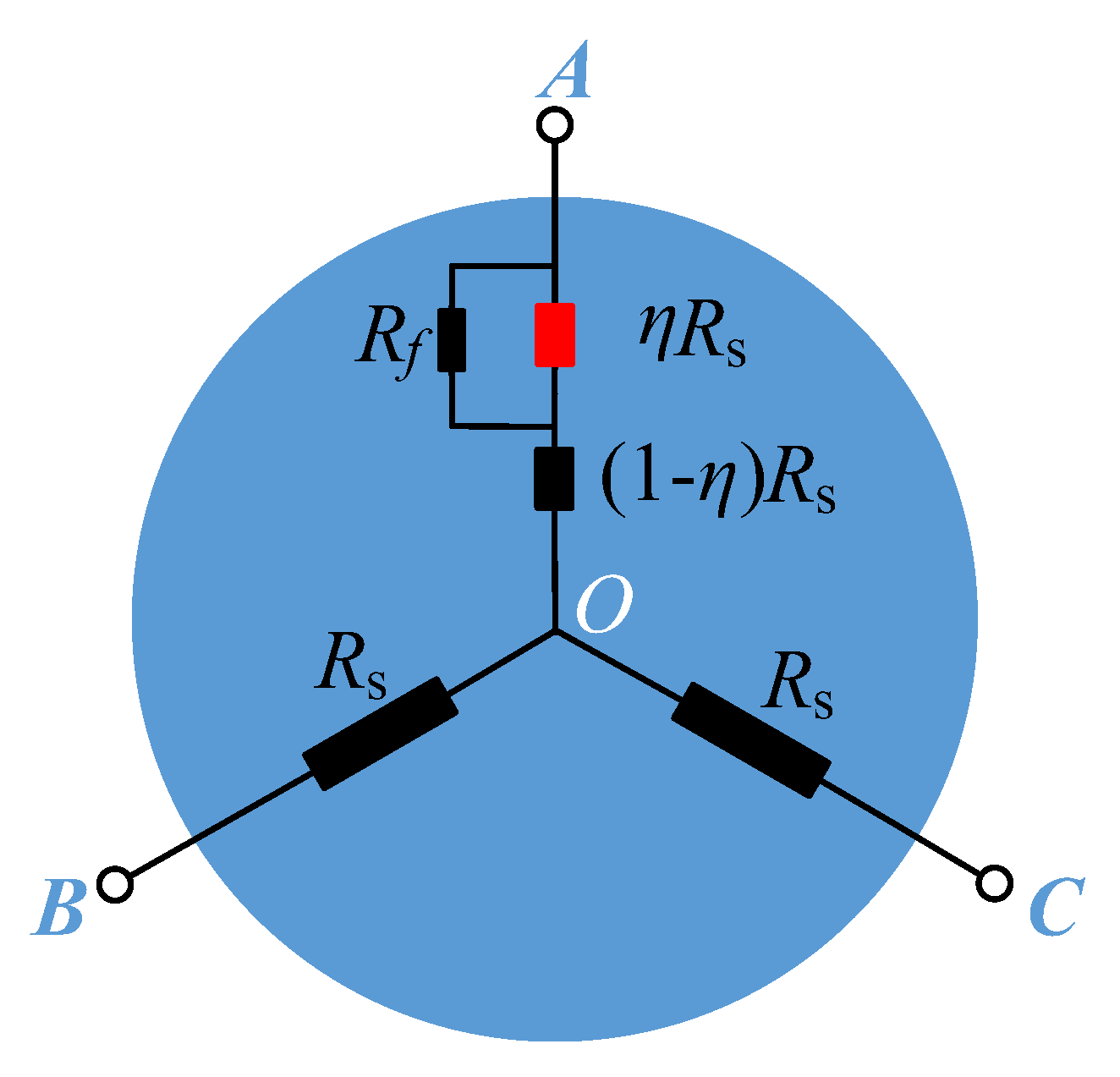
3.1. Line-to-Line Resistance Analysis
3.2. Line-to-Line Inductance Analysis
4. Detection and Evaluation for the Stator Fault
5. Experiment
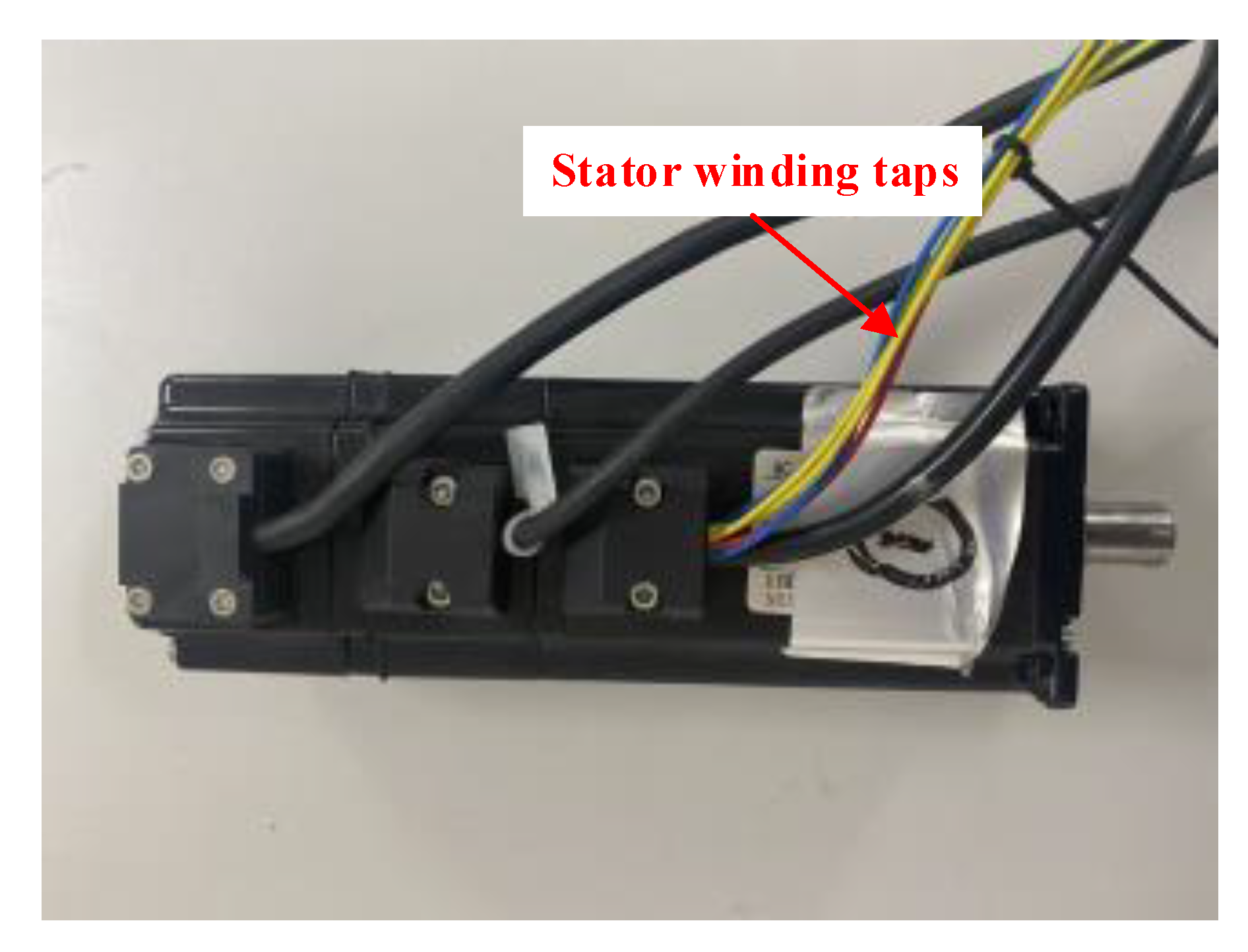
5.1. Tested PMSM with the Stator Fault Injection
5.2. Test Results
6. Conclusions
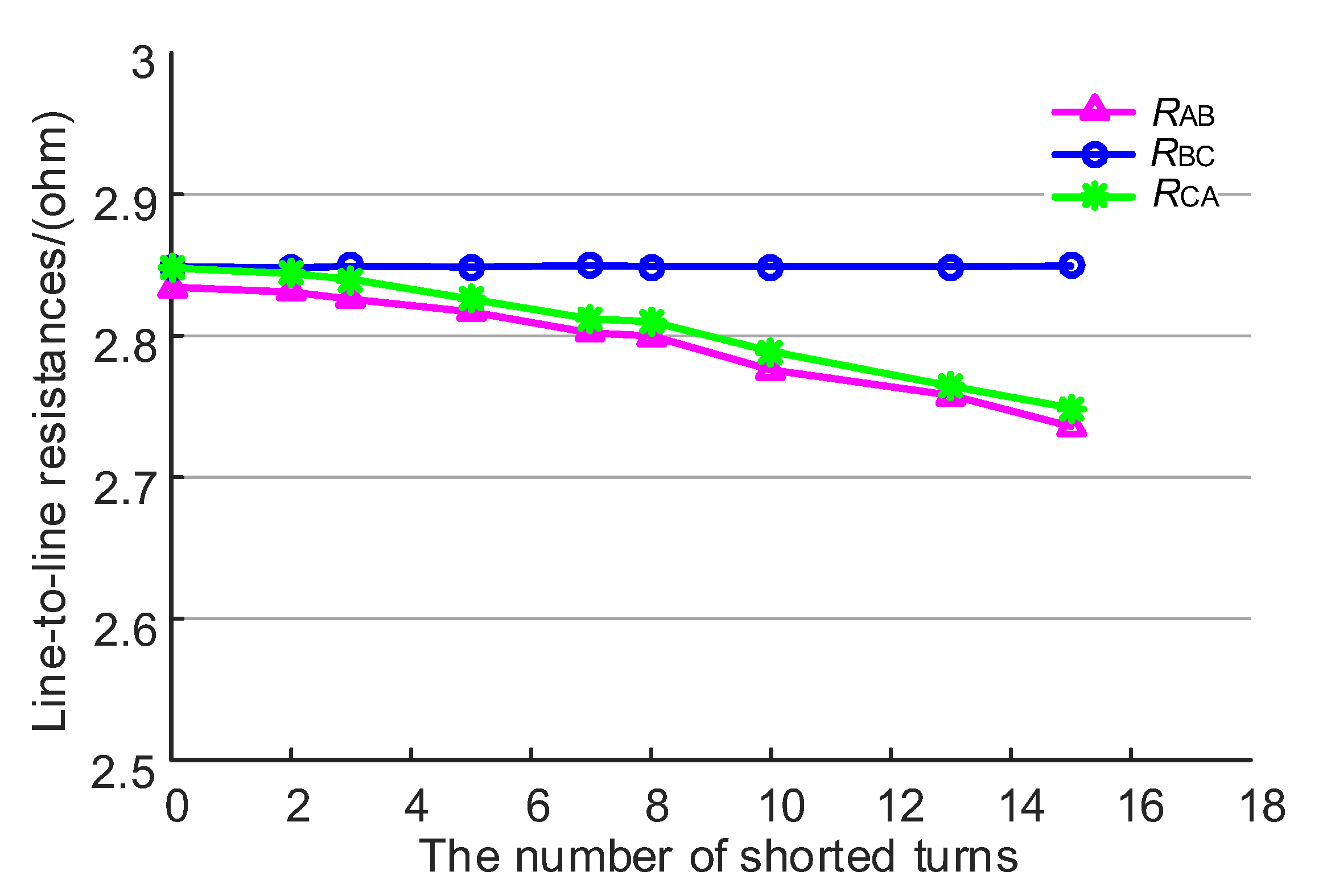
- When a stator fault occurs, the line-to-line resistance and inductance related to the fault phase decrease, whereas the others are unchanged.
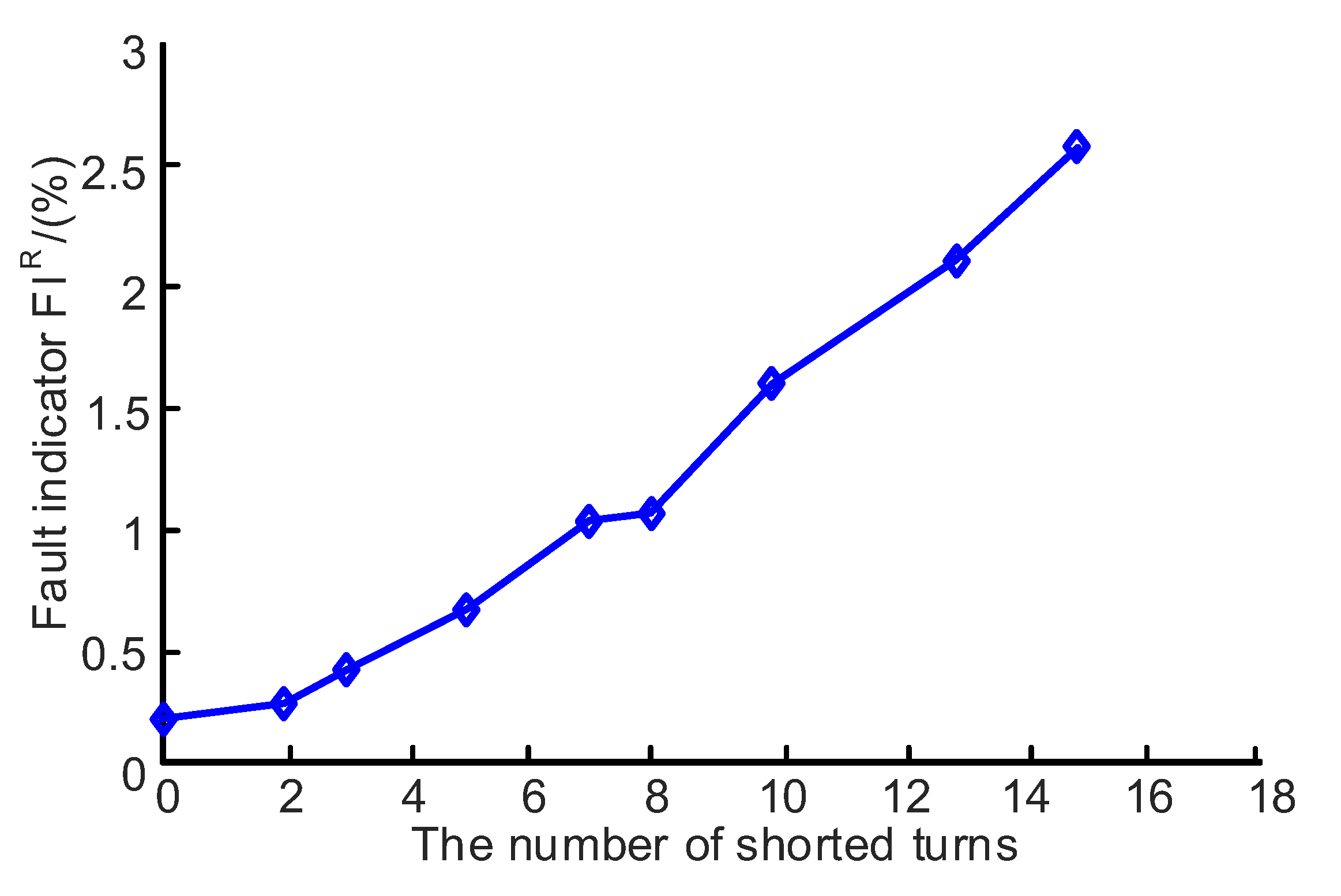
- The fault indicator FIR that represents the imbalance of the resistances increases with the number of shorted turns, which can be used to detect the stator fault.
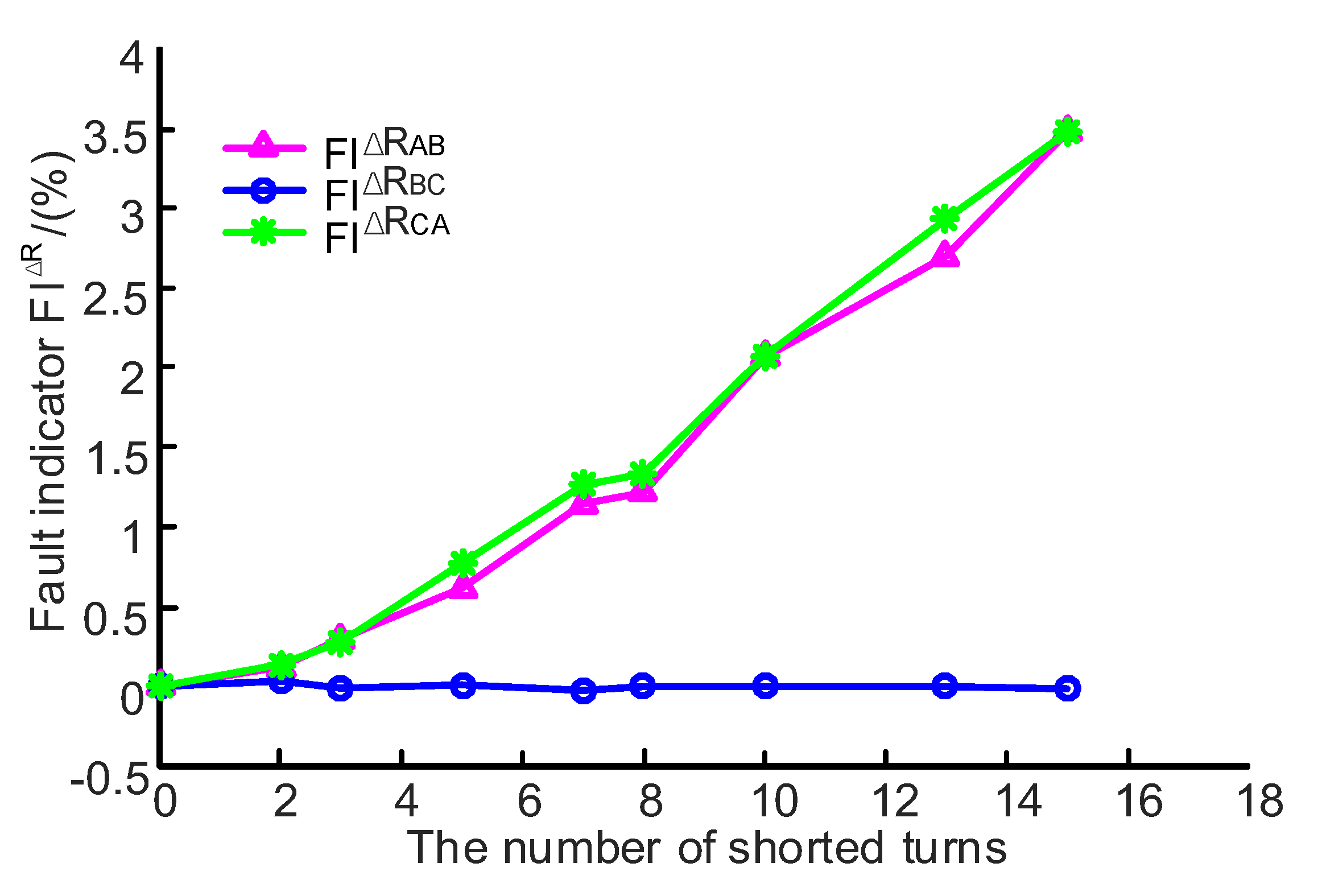
- The fault indicator FI∆R describes the rate of resistance change. When a stator fault occurs, the FI∆R related to the fault phase increases, whereas the other is nearly 0, which can provide a powerful basis to detect and locate the stator fault.
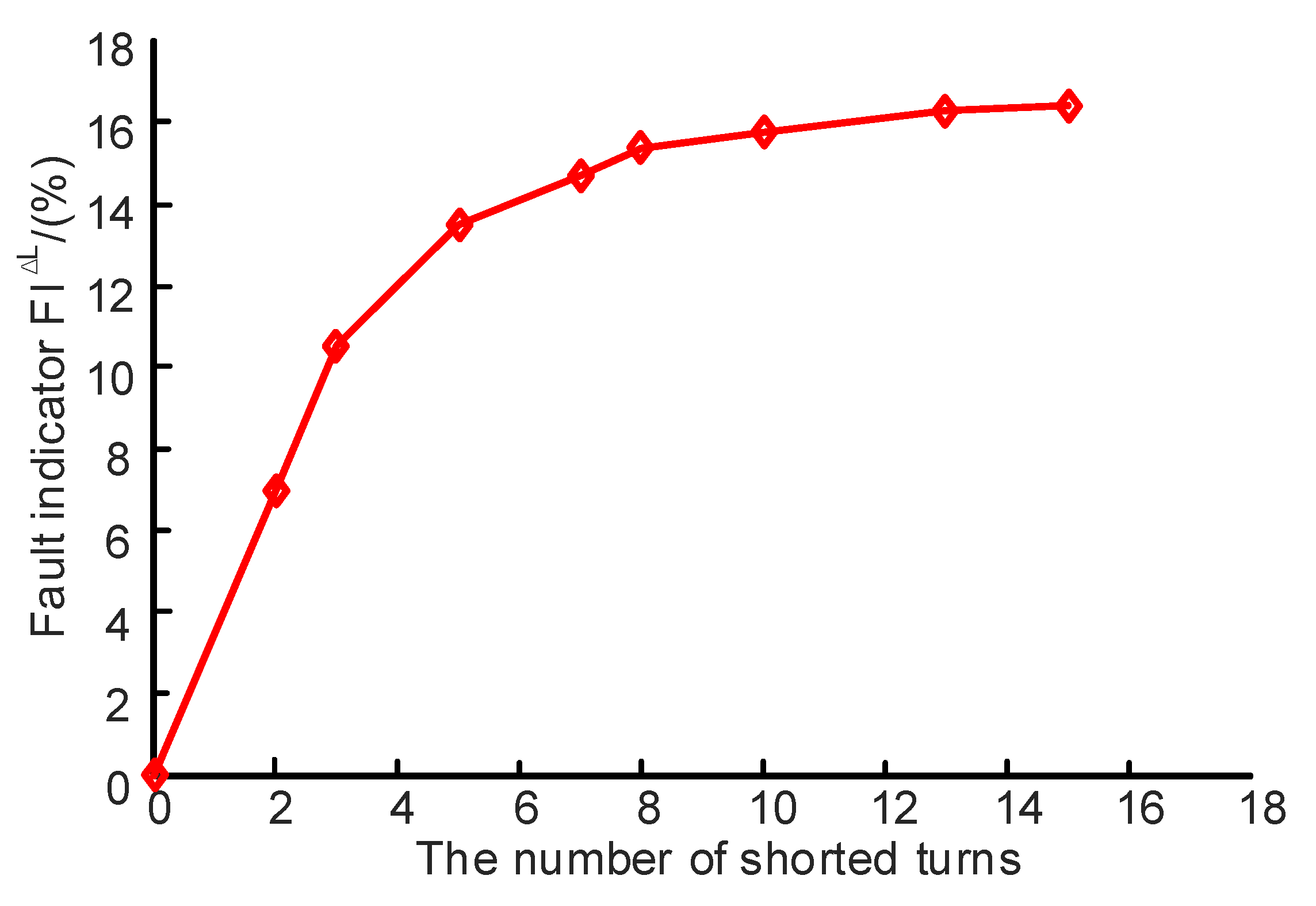
- The fault indicator FI∆L increases significantly when the motor has a stator fault, which is valuable for stator fault diagnosis.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qi, Y.; Bostanci, E.; Zafarani, M.; Akin, B. Severity Estimation of Interturn Short Circuit Fault for PMSM. IEEE Trans. Ind. Electron. 2019, 66, 7260–7269. [Google Scholar] [CrossRef]
- Hang, J.; Zhang, J.; Cheng, M.; Huang, J. Online Interturn Fault Diagnosis of Permanent Magnet Synchronous Machine Using Zero-Sequence Components. IEEE Trans. Power Electron. 2015, 30, 6731–6741. [Google Scholar] [CrossRef]
- Motor Reliability Working Group. Report of large motor reliability survey of industrial and commercial installations: Part I. IEEE Trans. Ind. Appl. 1985, IA-21, 1985. [Google Scholar] [CrossRef]
- Motor Reliability Working Group. Report of large motor reliability survey of industrial and commercial installations: Part II. IEEE Trans. Ind. Appl. 1985, IA-21, 9533. [Google Scholar] [CrossRef]
- Ukil, A.; Chen, S.; Andenna, A. Detection of stator short circuit faults in three-phase induction motors using motor current zero crossing instants. Electr. Power Syst. Res. 2011, 81, 1036–1044. [Google Scholar] [CrossRef]
- Zheng, D.; Zhang, P. An Online Groundwall and Phase-to-Phase Stator Insulation Monitoring Method for Inverter-Fed Machine. IEEE Trans. Ind. Electron. 2021, 68, 5303–5313. [Google Scholar] [CrossRef]
- Liu, T. Research on Stator Winding Interturn Short Circuit Faults Diagnosis of Induction Motor. Ph.D. Thesis, Zhejiang University, Zhejiang, China, 2007. [Google Scholar]
- Briz, F.; Degner, M.W.; Zamarron, A. Online Stator Winding Fault Diagnosis in Inverter-Fed AC Machines using High-Frequency Signal Injection. IEEE Trans. Ind. Appl. 2003, 39, 1109–1117. [Google Scholar] [CrossRef]
- Cheng, S.; Zhang, P.; Habetler, T.G. An Impedance Identification Approach to Sensitive Detection and Location of Stator Turn-to-Turn Faults in a Closed-Loop Multiple-Motor Drive. IEEE Trans. Ind. Electron. 2011, 58, 1545–1554. [Google Scholar] [CrossRef]
- Ghate, V.N.; Dudul, S.V. Cascade Neural-Network-Based Fault Classifier for Three-Phase Induction Motor. IEEE Trans. Ind. Electron. 2011, 58, 1555–1563. [Google Scholar] [CrossRef]
- Devi, N.R.; Sarma, D.V.S.S.S.; Rao, P.V.R. Diagnosis and classification of stator winding insulation faults on a three-phase induction motor using wavelet and MNN. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 2543–2555. [Google Scholar] [CrossRef]
- Seera, M.; Lim, C.P.; Ishak, D. Fault Detection and Diagnosis of Induction Motors Using Motor Current Signature Analysis and a Hybrid FMM–CART Model. IEEE Trans. Neural Netw. Learn. Syst. 2012, 23, 97–108. [Google Scholar] [CrossRef] [PubMed]
- Maitre, J.; Bouchard, B.; Bouzouane, A. Classification Algorithms Comparison for Interturn Short-Circuit Recognition in Induction Machines Using Best-Fit 3-D-Ellipse Method. Can. J. Electr. Comput. Eng. 2017, 40, 255–265. [Google Scholar] [CrossRef]
- Tallam, R.M. A Survey of Methods for Detection of Stator-Related Faults in Induction Machines. IEEE Trans. Ind. Appl. 2007, 43, 920–933. [Google Scholar] [CrossRef]
- Krishnan, R. Permanent Magnet Synchronous and Brushless DC Motor Drives; CRC Press, Taylor & Francis Group LLC: Boca Raton, FL, USA, 2010; ISBN 978-0-8247-5384-9. [Google Scholar]



| Parameters | Values |
|---|---|
| Rated power/(W) | 400 |
| Rated voltage/(V) | 220 |
| Rated current/(A) | 2.8 |
| Rated speed/(rpm) | 3000 |
| Rated torque/(N.m) | 1.27 |
| Line-to-line resistance/(Ω) | 3.43 |
| Line-to-line inductance/(mH) | 6.6 |
| Inertia/(kg/m2 × 10−4) | 0.32 |
| The Number of Shorted Turns | Line-to-Line Resistance/(Ω) | Line-to-Line Inductance/(mH) | ||||
|---|---|---|---|---|---|---|
| RAB | RBC | RCA | ||||
| health | 2.8345 | 2.8490 | 2.8480 | 7.4559 | 7.3719 | 7.3049 |
| 2 turns | 2.8310 | 2.8480 | 2.8440 | 6.5480 | 7.3425 | 6.6970 |
| 3 turns | 2.8260 | 2.8493 | 2.8400 | 6.0690 | 7.3200 | 6.4120 |
| 5 turns | 2.8170 | 2.8487 | 2.8260 | 5.7000 | 7.3067 | 6.1410 |
| 7 turns | 2.8020 | 2.8497 | 2.8120 | 5.5250 | 7.2980 | 6.0580 |
| 8 turns | 2.8000 | 2.8490 | 2.8100 | 5.4370 | 7.2940 | 6.0026 |
| 10 turns | 2.7760 | 2.8490 | 2.7890 | 5.3840 | 7.2919 | 5.9718 |
| 13 turns | 2.7580 | 2.8490 | 2.7645 | 5.3150 | 7.2890 | 5.9254 |
| 15 turns | 2.7360 | 2.8494 | 2.7490 | 5.2970 | 7.2880 | 5.9174 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, J.; Liang, C.; Wang, Y.; Lu, S.; Zhou, J. A Stator Fault Diagnosis Method Based on the Offline Motor Parameter Measurement for PMSM. World Electr. Veh. J. 2021, 12, 248. https://doi.org/10.3390/wevj12040248
Tang J, Liang C, Wang Y, Lu S, Zhou J. A Stator Fault Diagnosis Method Based on the Offline Motor Parameter Measurement for PMSM. World Electric Vehicle Journal. 2021; 12(4):248. https://doi.org/10.3390/wevj12040248
Chicago/Turabian StyleTang, Jing, Chao Liang, Yuanhang Wang, Shuhan Lu, and Jian Zhou. 2021. "A Stator Fault Diagnosis Method Based on the Offline Motor Parameter Measurement for PMSM" World Electric Vehicle Journal 12, no. 4: 248. https://doi.org/10.3390/wevj12040248
APA StyleTang, J., Liang, C., Wang, Y., Lu, S., & Zhou, J. (2021). A Stator Fault Diagnosis Method Based on the Offline Motor Parameter Measurement for PMSM. World Electric Vehicle Journal, 12(4), 248. https://doi.org/10.3390/wevj12040248






