Cost-Effective Scheme for a Brushless Wound Rotor Synchronous Machine
Abstract
:1. Introduction
2. Concept of the Proposed Scheme
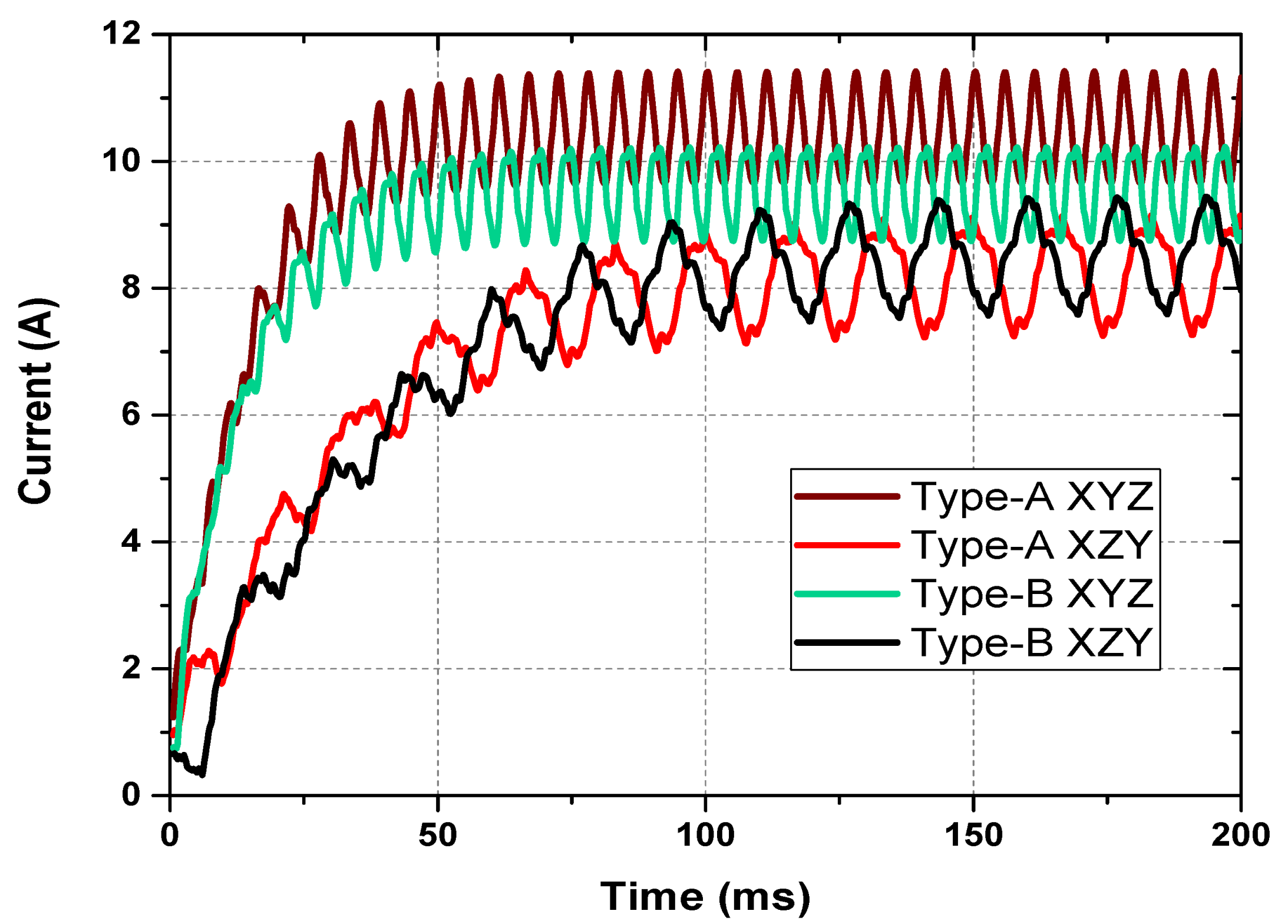
3. Electromagnetic Performance
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Ayub, M.; Hussain, A.; Jawad, G.; Kwon, B. Brushless Operation of a Wound-Field Synchronous Machine Using a Novel Winding Scheme. IEEE Trans. Magn. 2019, 55, 8201104. [Google Scholar] [CrossRef]
- Chakraborty, C.; Basak, S.; Rao, Y.T. Synchronous Generator with Embedded Brushless Synchronous Exciter. IEEE Trans. Energy Convers. 2019, 34, 1242–1254. [Google Scholar] [CrossRef]
- Bukhari, S.S.H.; Memon, A.A.; Madanzadeh, S.; Sirewal, G.J.; Gandoy, J.D.; Ro, J.-S. Novel Single Inverter-Controlled Brushless Wound Field Synchronous Machine Topology. Mathematics 2021, 9, 1739. [Google Scholar] [CrossRef]
- Bukhari, S.S.H.; Ali, Q.; Gandoy, J.D.; Ro, J.-S. High-Efficient Brushless Wound Rotor Synchronous Machine Topology Based on Sub-Harmonic Field-Excitation Technique. Energies 2021, 14, 4427. [Google Scholar] [CrossRef]
- Ayub, M.; Bukhari, S.S.H.; Sirewal, G.J.; Arif, A.; Kwon, B.-I. Utilization of Reluctance Torque for Improvement of the Starting and Average Torques of a Brushless Wound Field Synchronous Machine. Electr. Eng. 2021, 103, 2327–2333. [Google Scholar] [CrossRef]
- Bukhari, S.S.H.; Ahmad, H.; Chachar, F.A.; Ro, J.-S. Brushless Field-Excitation Method for Wound-Rotor Synchronous Machines. Int. Trans. Electr. Energy Syst. 2021, 31, e12961. [Google Scholar] [CrossRef]
- Yao, F.; Sun, D.; Sun, L.; Lipo, T.A. Dual Third-Harmonic-Current Excitation Principle of a Brushless Synchronous Machine Based on Double Three-Phase Armature Windings. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–4. [Google Scholar]
- Ali, Q.; Lipo, T.A.; Kwon, B.I. Design and analysis of a novel brushless wound rotor synchronous machine. IEEE Trans. Magn. 2015, 51, 8109804. [Google Scholar] [CrossRef]
- Jawad, G.; Ali, Q.; Lipo, T.A.; Kwon, B.I. Novel brushless wound rotor synchronous machine with zero-sequence third-harmonic field excitation. IEEE Trans. Magn. 2016, 52, 8106104. [Google Scholar] [CrossRef]
- Pötter, J.; Pfost, M.; Schullerus, G. A Novel Brushless Excitation System for Synchronous Machines with a Rotating Power Converter. In Proceedings of the 2019 CPE-POWERENG, Sonderborg, Denmark, 23–25 April 2019; pp. 1–6. [Google Scholar]
- Pötter, J.; Pfost, M.; Schullerus, G. Design Aspects of a Novel Brushless Excitation System for Synchronous Machines; IEMDC: San Diego, CA, USA, 2019; pp. 1228–1233. [Google Scholar]
- Rebollo, E.; Blanquez, F.R.; Platero, C.A.; Blazquez, F.; Redondo, M. Improved high-speed de-excitation system for brushless synchronous machines tested on a 20 MVA hydro-generator. IET Electr. Power Appl. 2015, 9, 405–411. [Google Scholar] [CrossRef] [Green Version]
- Bukhari, S.S.H.; Sirewal, G.J.; Ayub, M.; Ro, J.-S. A New Small-Scale Self-Excited Wound Rotor Synchronous Motor Topology. IEEE Trans. Magn. 2021, 57, 8200205. [Google Scholar] [CrossRef]
- Jiao, N.; Liu, W.; Zhang, Z.; Meng, T.; Peng, J.; Jiang, Y. Field Current Estimation for Wound-Rotor Synchronous Starter–Generator with Asynchronous Brushless Exciters. IEEE Trans. Energy Convers. 2017, 32, 1554–1561. [Google Scholar] [CrossRef]
- Lipo, T.A. Magnetic Circuits. In Introduction to AC Machine Design; John Wiley & Sons: New York, NY, USA, 2017; pp. 1–50. [Google Scholar]
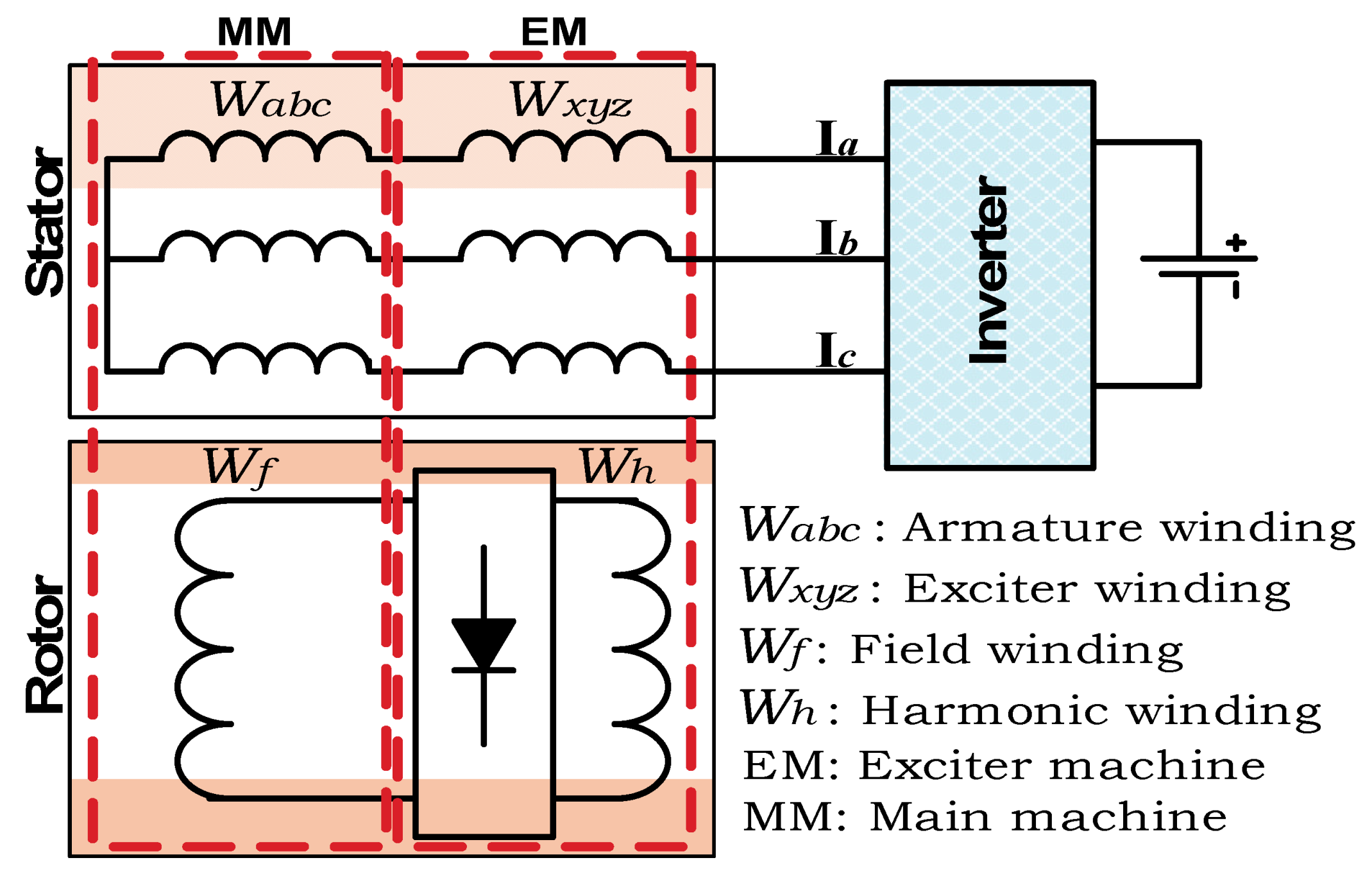
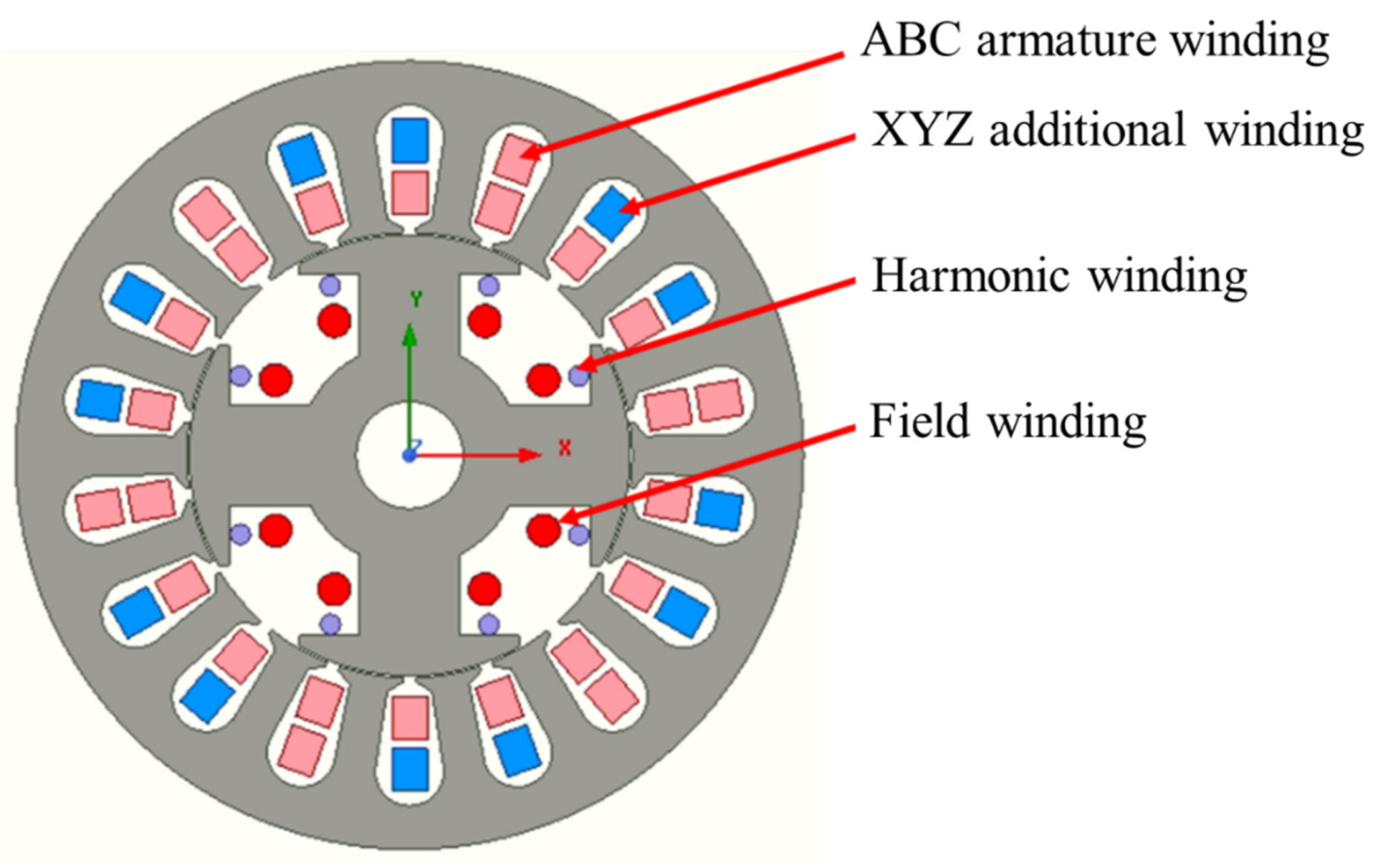

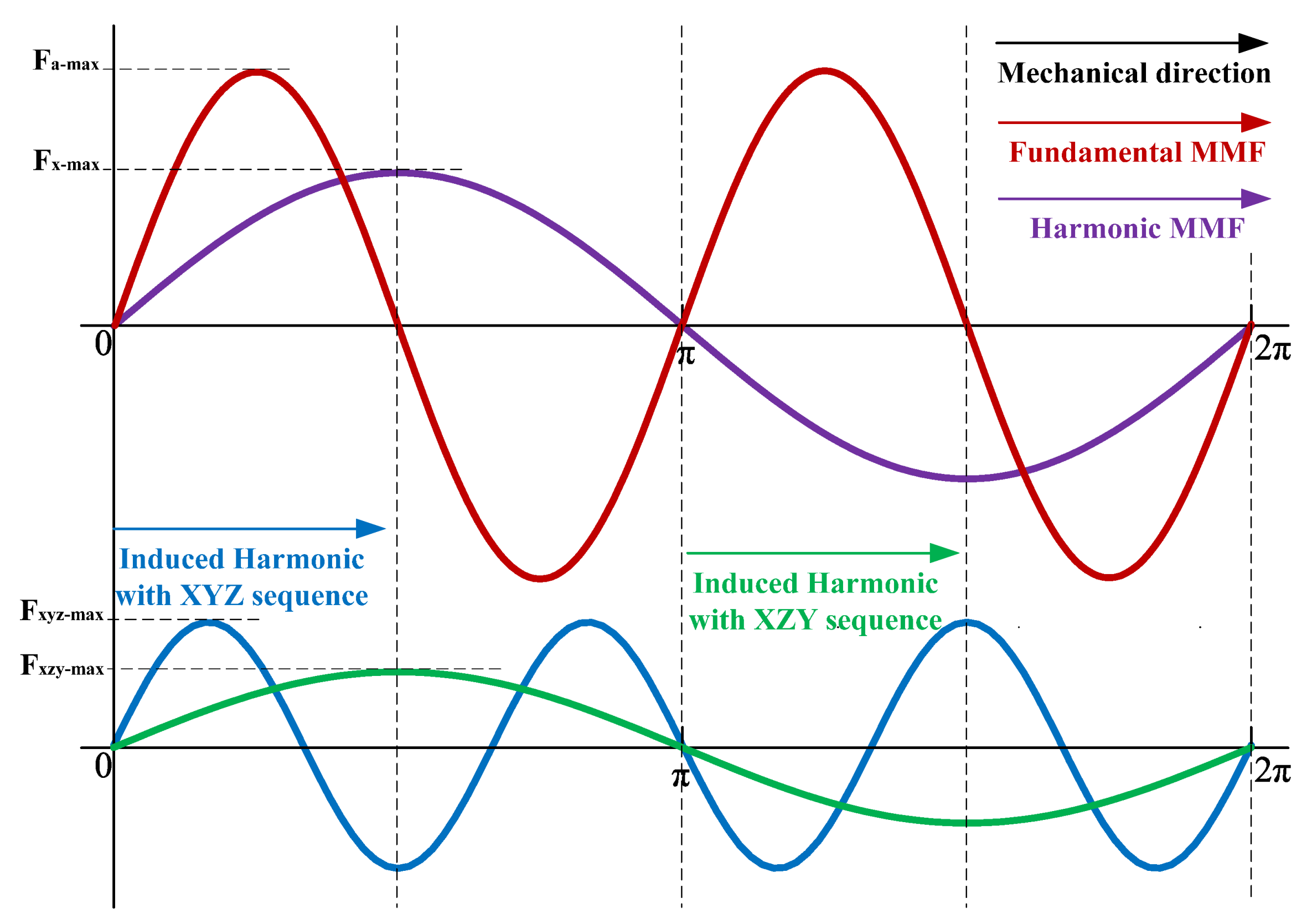
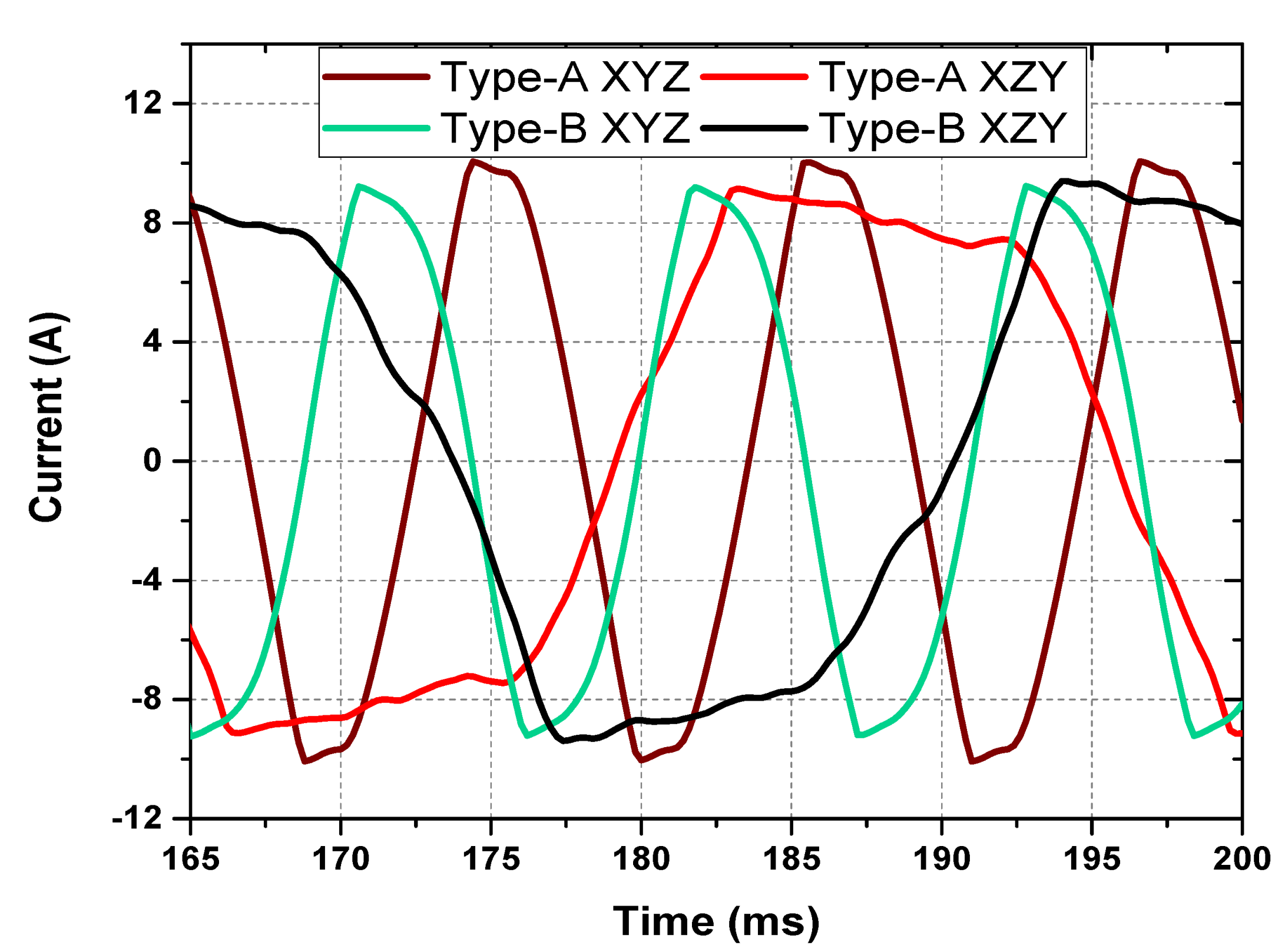

| Parameters | Unit | Value |
|---|---|---|
| Rated speed | rpm | 1800 |
| Rated current | A-peak | 10 |
| Stator outer diameter | mm | 177 |
| Rotor outer diameter | mm | 99 |
| Air-gap length | mm | 0.5 |
| Shaft diameter | mm | 24 |
| Stack length | mm | 65 |
| Number of armature winding poles | - | 4 |
| Number of stator slots | - | 18 |
| Number of harmonic winding poles | - | 6 |
| Armature (ABC) winding turns | - | 300 |
| Harmonic (XYZ) winding turns | - | 150 |
| Field winding turns | - | 200 |
| Harmonic winding turns | - | 50 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sirewal, G.J.; Bukhari, S.S.H. Cost-Effective Scheme for a Brushless Wound Rotor Synchronous Machine. World Electr. Veh. J. 2021, 12, 194. https://doi.org/10.3390/wevj12040194
Sirewal GJ, Bukhari SSH. Cost-Effective Scheme for a Brushless Wound Rotor Synchronous Machine. World Electric Vehicle Journal. 2021; 12(4):194. https://doi.org/10.3390/wevj12040194
Chicago/Turabian StyleSirewal, Ghulam Jawad, and Syed Sabir Hussain Bukhari. 2021. "Cost-Effective Scheme for a Brushless Wound Rotor Synchronous Machine" World Electric Vehicle Journal 12, no. 4: 194. https://doi.org/10.3390/wevj12040194
APA StyleSirewal, G. J., & Bukhari, S. S. H. (2021). Cost-Effective Scheme for a Brushless Wound Rotor Synchronous Machine. World Electric Vehicle Journal, 12(4), 194. https://doi.org/10.3390/wevj12040194







