A Copper Foil Electromagnetic Coupler and Its Wireless Power Transfer System without Compensation
Abstract
:1. Introduction
2. Inductance and Capacitance Integrated Electromagnetic Coupler
3. System Circuit Modelling and Self-Compensating Principle
3.1. System Circuit Model and Its Equivalent Simplification
3.2. Circuit Full Resonance and Parameter Self-Compensating Relation
- (1)
- Equation (10) is satisfied.
- (2)
- Equation (11) is satisfied.
4. Analysis and Design of Circuit Parameters and Electromagnetic Coupler Parameters
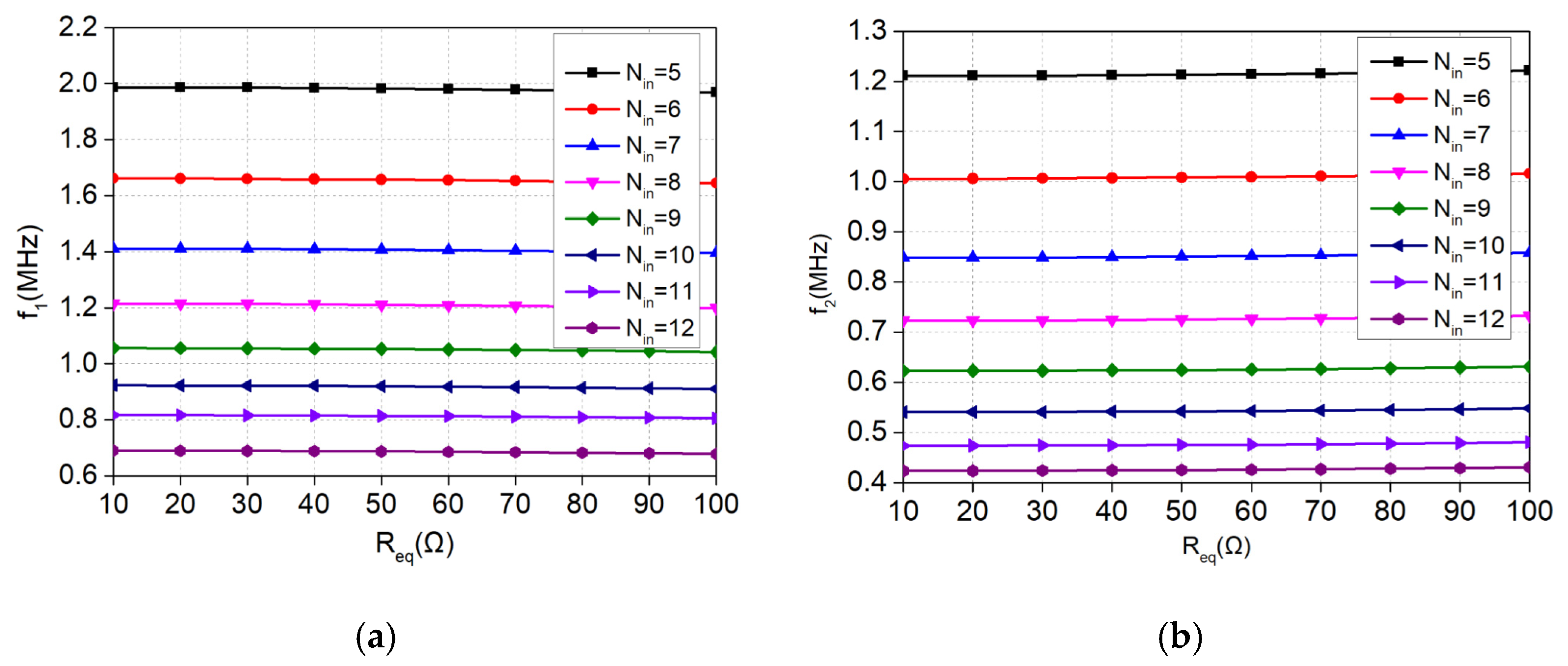
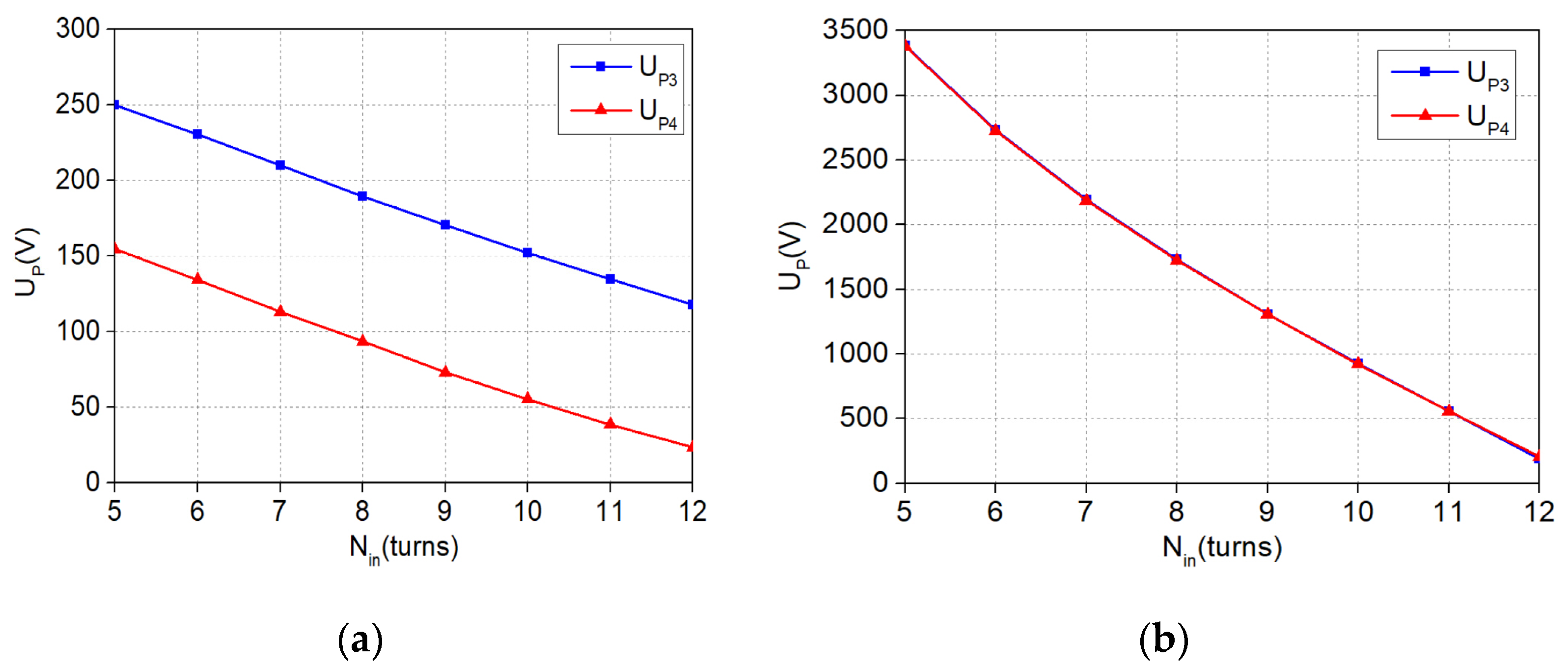
4.1. Selection for System Working Frequency Considering the Influence of Inner Electromagnetic Poles’ Copper foil Turns
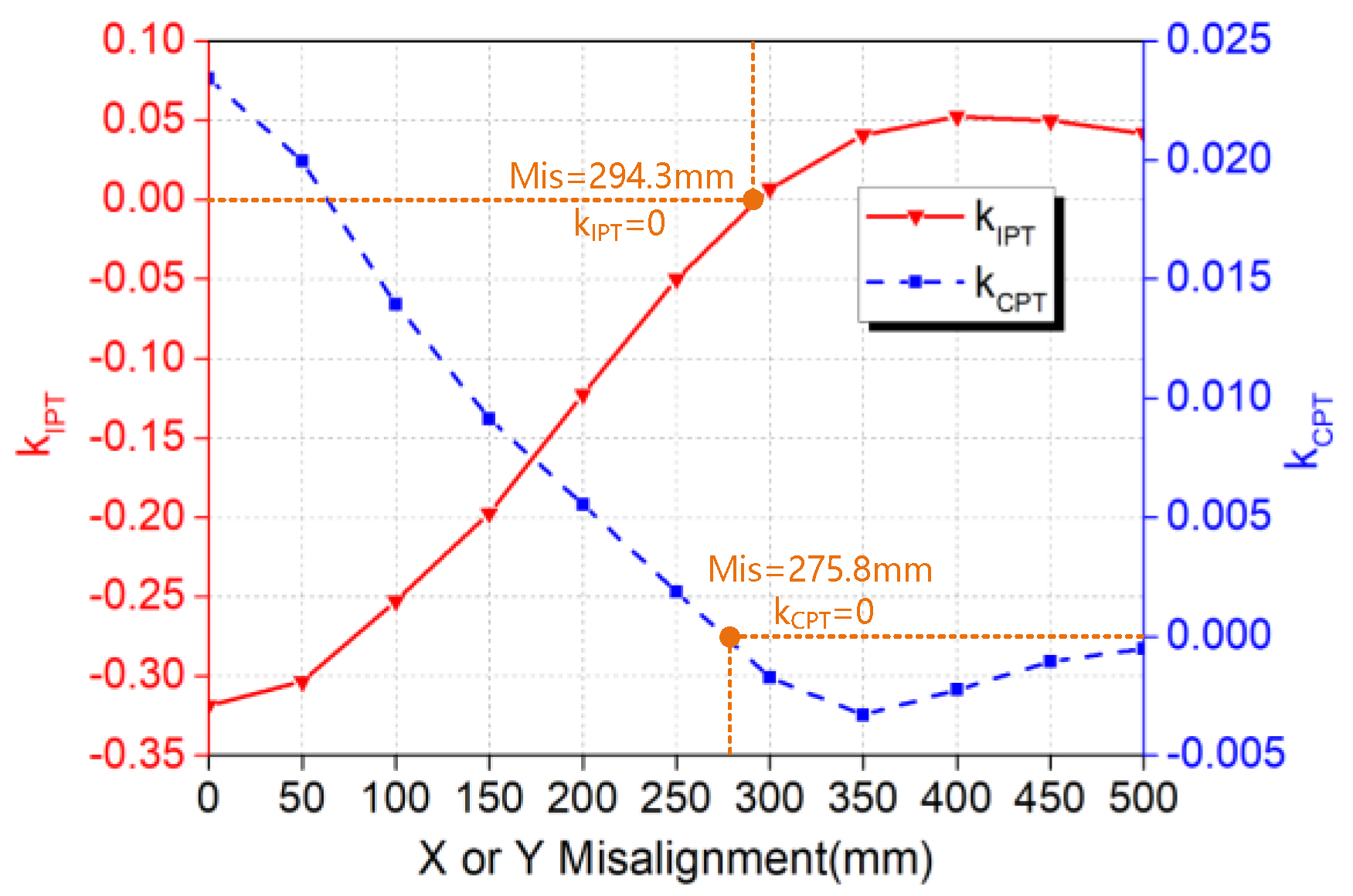
4.2. Influence of Misalignment on Coupling Coefficient
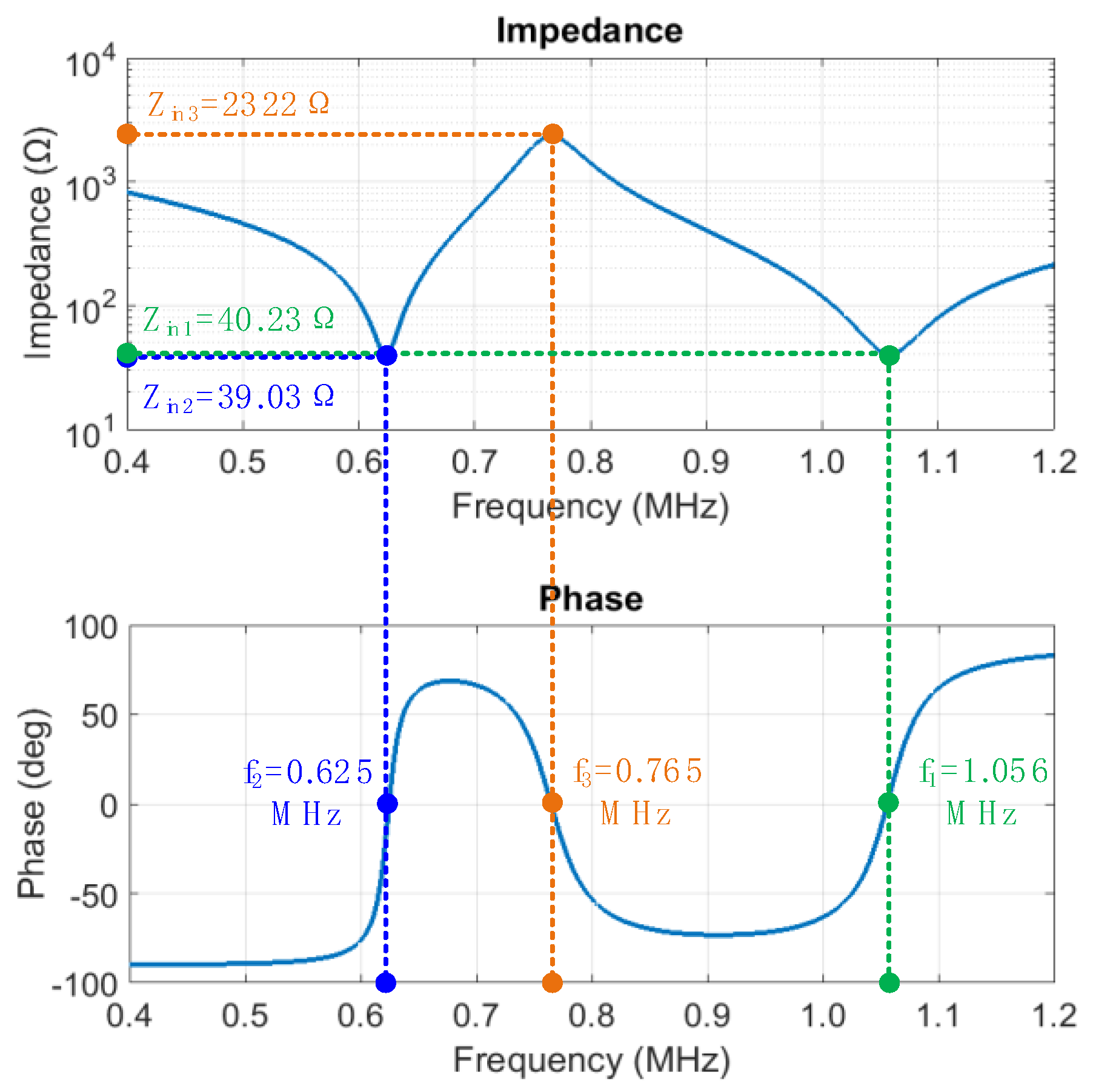
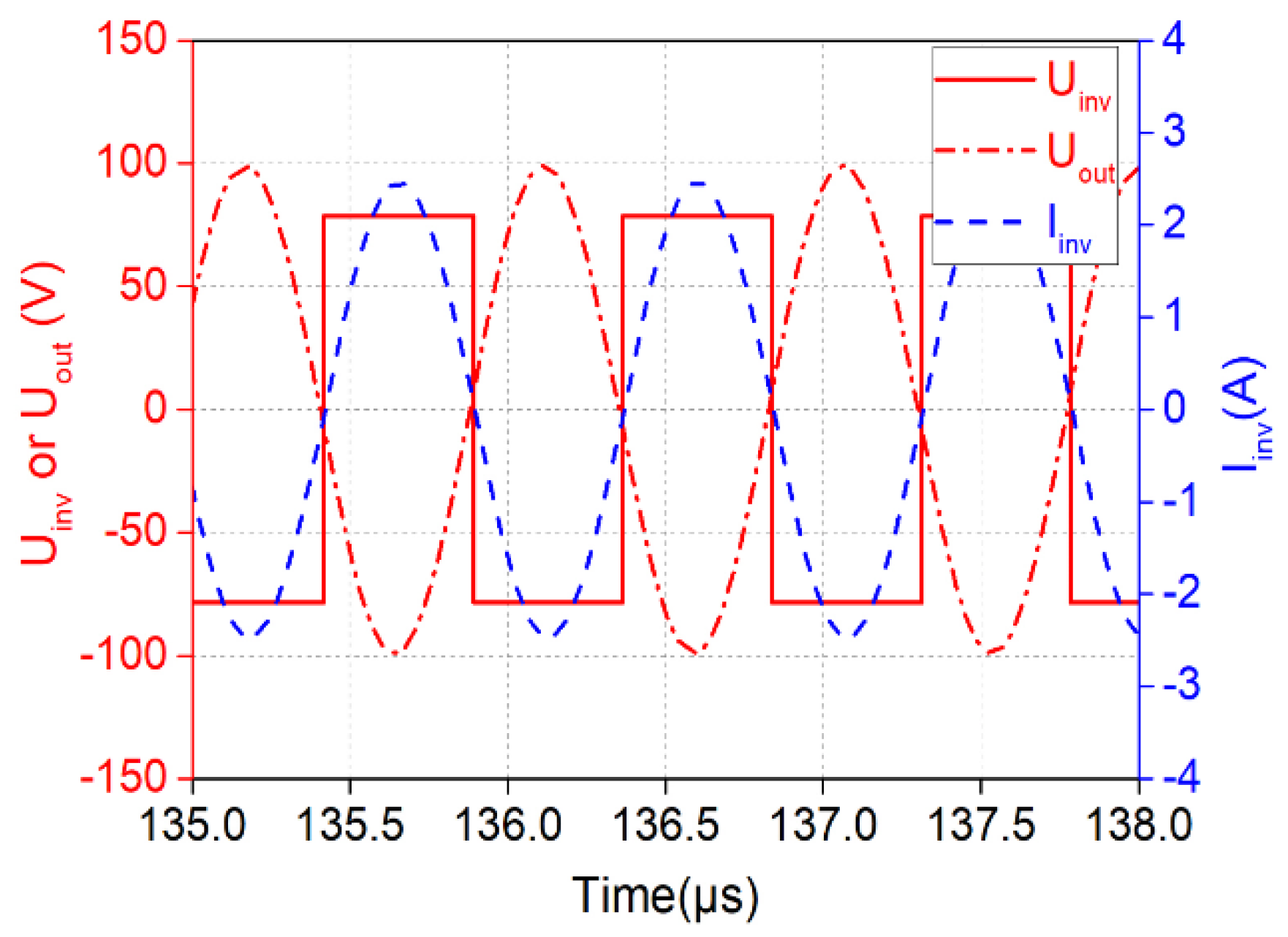
5. Parameters Determination and Simulation Verification
6. Conclusions
- (1)
- Low cost, light weight, simple structure, and high power density;
- (2)
- No additional compensation components and little skin effect, so as to improve system efficiency;
- (3)
- High power factor and ZVS condition.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Hu, A.P.; You, Y.W.; Chen, F.Y.; McCormick, D.; Budgett, D.M. Wireless Power Supply for ICP Devices with Hybrid Supercapacitor and Battery Storage. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 273–279. [Google Scholar] [CrossRef]
- Liu, C.; Hu, A.P.; Covic, G.A.; Nair, N.-K.C. Comparative Study of CCPT Systems with Two Different Inductor Tuning Positions. IEEE Trans. Power Electron. 2012, 27, 294–306. [Google Scholar]
- Lee, K.; Kim, J.; Cha, C. Microwave-based Wireless Power Transfer using Beam Scanning for Wireless Sensors. In Proceedings of the IEEE EUROCON 2019—18th International Conference on Smart Technologies, Novi Sad, Serbia, 1–4 July 2019; pp. 1–5. [Google Scholar]
- Jin, K.; Zhou, W. Wireless Laser Power Transmission: A Review of Recent Progress. IEEE Trans. Power Electron. 2019, 34, 3842–3859. [Google Scholar] [CrossRef]
- Li, S.; Mi, C. Wireless Power Transfer for Electric Vehicle Applications. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 4–17. [Google Scholar]
- Wang, C.-S.; Covic, G.A.; Stielau, O.H. Power Transfer Capability and Bifurcation Phenomena of Loosely Coupled Inductive Power Transfer Systems. IEEE Trans. Ind. Electron. 2004, 51, 148–157. [Google Scholar] [CrossRef]
- Zhang, W.; Wong, S.C.; Tse, C.; Chen, Q. Design for Efficiency Optimization and Voltage Controllability of Series–Series Compensated Inductive Power Transfer Systems. IEEE Trans. Power Electron. 2014, 29, 191–200. [Google Scholar] [CrossRef]
- Hou, J.; Chen, Q.; Yan, K.; Ren, X.; Wong, S.C.; Tse, C. Analysis and control of S/SP compensation contactless resonant converter with constant voltage gain. In Proceedings of the 2013 IEEE Energy Conversion Congress and Exposition, Denver, Colorado, 15–19 September 2013; pp. 2552–2558. [Google Scholar]
- Su, Y.-G.; Zhou, W.; Hu, A.P.; Tang, C.-S.; Xie, S.-Y.; Sun, Y. Full-Duplex Communication on the Shared Channel of a Capacitively Coupled Power Transfer System. IEEE Trans. Power Electron. 2017, 32, 3229–3239. [Google Scholar] [CrossRef]
- Huang, L.; Hu, A. Defining the mutual coupling of capacitive power transfer for wireless power transfer. Electron. Lett. 2015, 51, 1806–1807. [Google Scholar] [CrossRef]
- Dai, J.; Ludois, D.C. Capacitive Power Transfer through a Conformal Bumper for Electric Vehicle Charging. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 1015–1025. [Google Scholar] [CrossRef]
- Yao, Y.; Wang, Y.; Liu, X.; Lin, F.; Xu, D.G. A Novel Parameter Tuning Method for a Double-Sided LCL Compensated WPT System with Better Comprehensive Performance. IEEE Trans. Power Electron. 2017, 33, 8525–8536. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Hofmann, H.; Mi, C. A Double-Sided LCLC-Compensated Capacitive Power Transfer System for Electric Vehicle Charging. IEEE Trans. Power Electron. 2015, 30, 6011–6014. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Hofmann, H.; Mi, C.C. An Inductive and Capacitive Combined Wireless Power Transfer System With LC-Compensated Topology. IEEE Trans. Power Electron. 2016, 31, 8471–8482. [Google Scholar] [CrossRef]
- Luo, B.; Long, T.; Mai, R.; Dai, R.; He, Z.; Li, W. Analysis and design of hybrid inductive and capacitive wireless power transfer for high-power applications. IET Power Electron. 2018, 11, 2263–2270. [Google Scholar] [CrossRef]
- Zhou, W.; Su, Y.-G.; Huang, L.; Qing, X.-D.; Hu, A.P. Wireless Power Transfer Across a Metal Barrier by Combined Capacitive and Inductive Coupling. IEEE Trans. Ind. Electron. 2019, 66, 4031–4041. [Google Scholar] [CrossRef]
- Gao, X.; Liu, C.; Zhou, H.; Hu, W.; Huang, Y.; Xiao, Y.; Lei, Z.; Chen, J. Design and Analysis of a New Hybrid Wireless Power Transfer System with a Space-Saving Coupler Structure. IEEE Trans. Power Electron. 2021, 36, 5069–5081. [Google Scholar] [CrossRef]
- Wu, X.-Y.; Su, Y.-G.; Hu, A.P.; Zou, L.J.; Liu, Z. A Sleeve-Type Capacitive Power Transfer System with Different Coupling Arrangements for Rotary Application. IEEE Access 2020, 8, 69148–69159. [Google Scholar] [CrossRef]
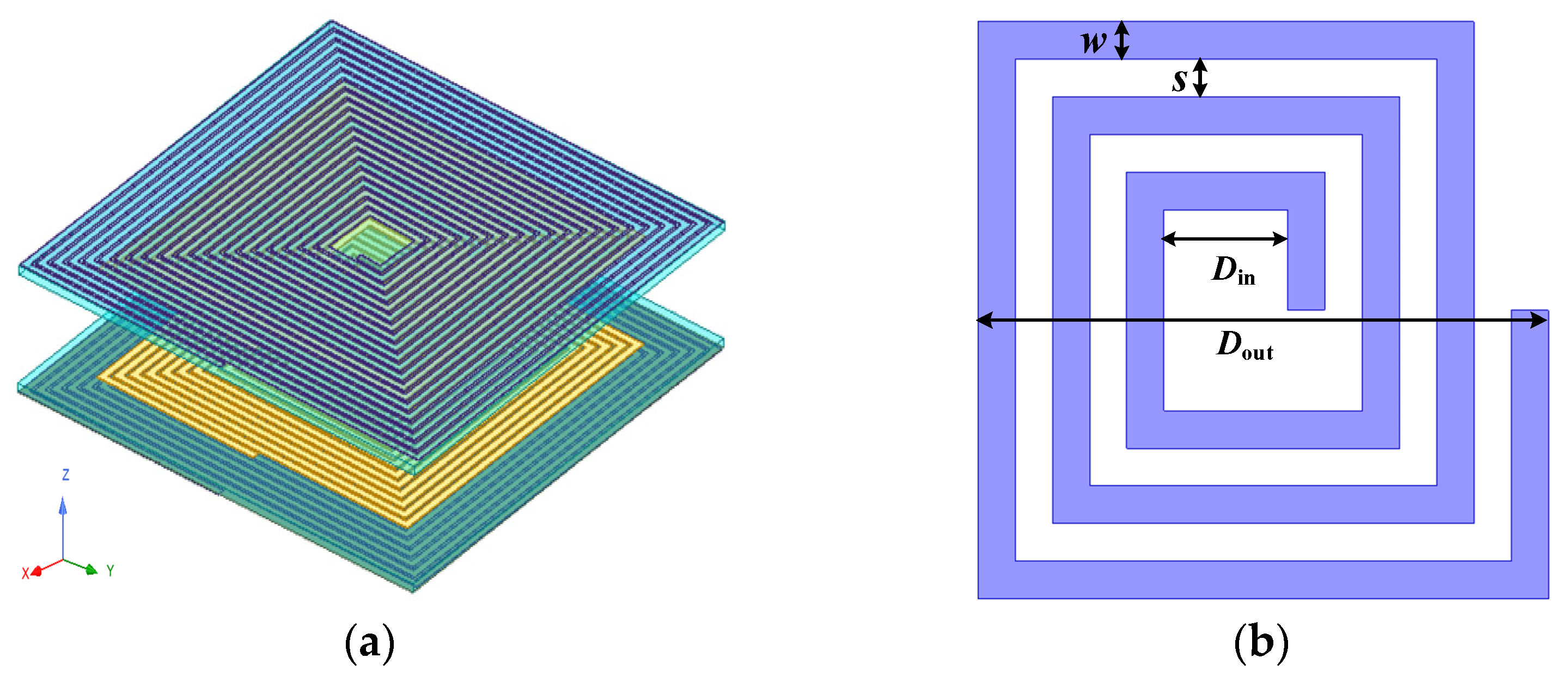
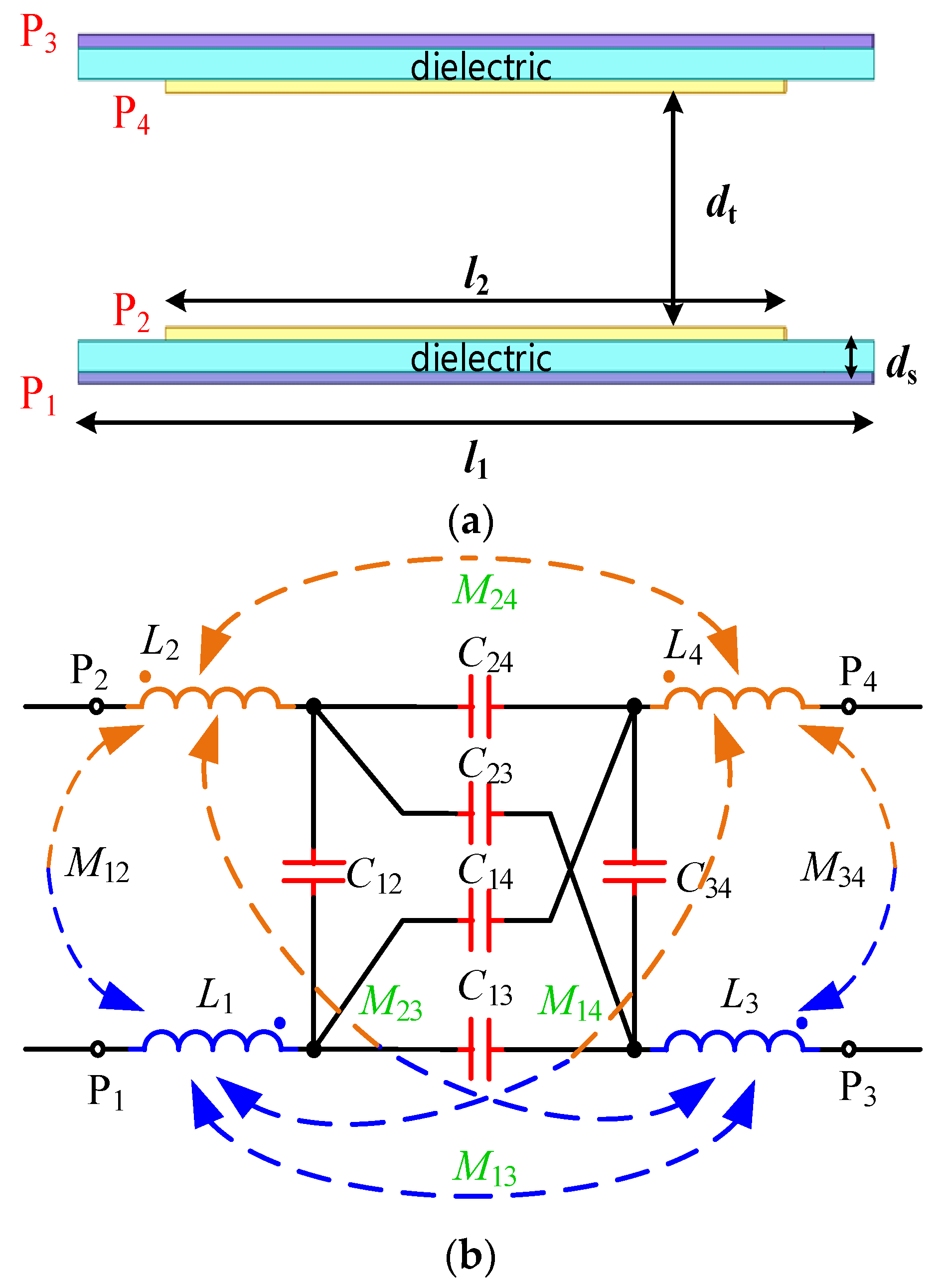

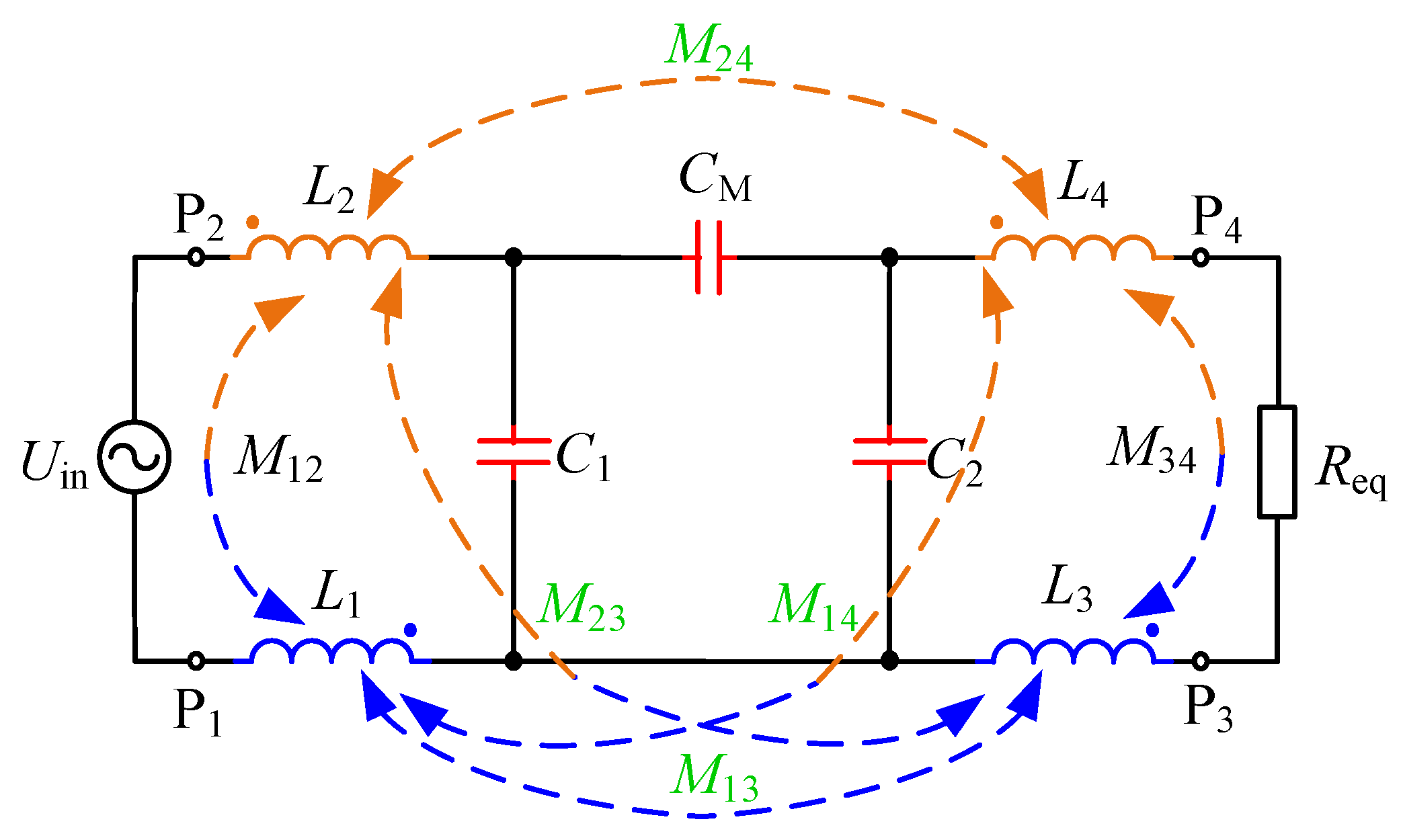

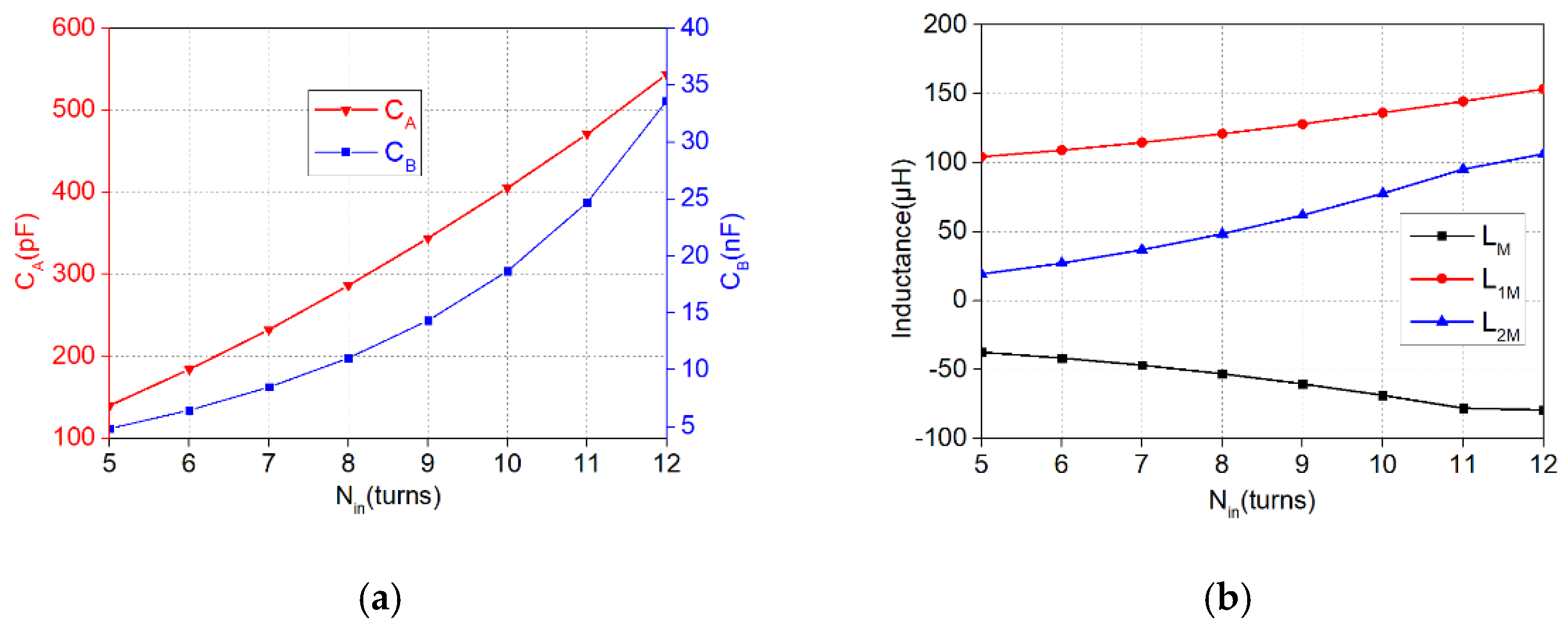

| Parameter | Nout | sout | wout | sin | win | ds | dt |
|---|---|---|---|---|---|---|---|
| Value | 15 | 5 mm | 10 mm | 5 mm | 10 mm | 10 mm | 60 mm |
| Parameter | Nout | sout | wout | sin | win | ds | dt |
|---|---|---|---|---|---|---|---|
| value | 15 | 5 mm | 10 mm | 5 mm | 10 mm | 10 mm | 60 mm |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| f | 1.056 MHz | L2 | 19.517 μH |
| Udc(Uin) | 78.54 V (100 V) | L3 | 64.407 μH |
| RL(Req) | 49.348 Ω (40 Ω) | L4 | 19.432 μH |
| C12 | 324.98 pF | M12 | 22.731 μH |
| C13 | 30.376 pF | M13 | 28.369 μH |
| C14 | 3.0998 pF | M14 | 12.367 μH |
| C23 | 3.1395 pF | M23 | 12.366 μH |
| C24 | 13.198 pF | M24 | 7.2848 μH |
| C34 | 324.14 pF | M34 | 22.722 μH |
| L1 | 64.622 μH |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, X.; Mao, M. A Copper Foil Electromagnetic Coupler and Its Wireless Power Transfer System without Compensation. World Electr. Veh. J. 2021, 12, 191. https://doi.org/10.3390/wevj12040191
Wu X, Mao M. A Copper Foil Electromagnetic Coupler and Its Wireless Power Transfer System without Compensation. World Electric Vehicle Journal. 2021; 12(4):191. https://doi.org/10.3390/wevj12040191
Chicago/Turabian StyleWu, Xueying, and Mingxuan Mao. 2021. "A Copper Foil Electromagnetic Coupler and Its Wireless Power Transfer System without Compensation" World Electric Vehicle Journal 12, no. 4: 191. https://doi.org/10.3390/wevj12040191
APA StyleWu, X., & Mao, M. (2021). A Copper Foil Electromagnetic Coupler and Its Wireless Power Transfer System without Compensation. World Electric Vehicle Journal, 12(4), 191. https://doi.org/10.3390/wevj12040191






