Static Eccentricity Fault Analysis of Surface-Mounted Permanent Magnet Electromotor with Skewed Slots Based on 2D FEA
Abstract
:1. Introduction
2. Geometric Structure of Air-Gap Eccentricity
3. Equivalent Algorithm
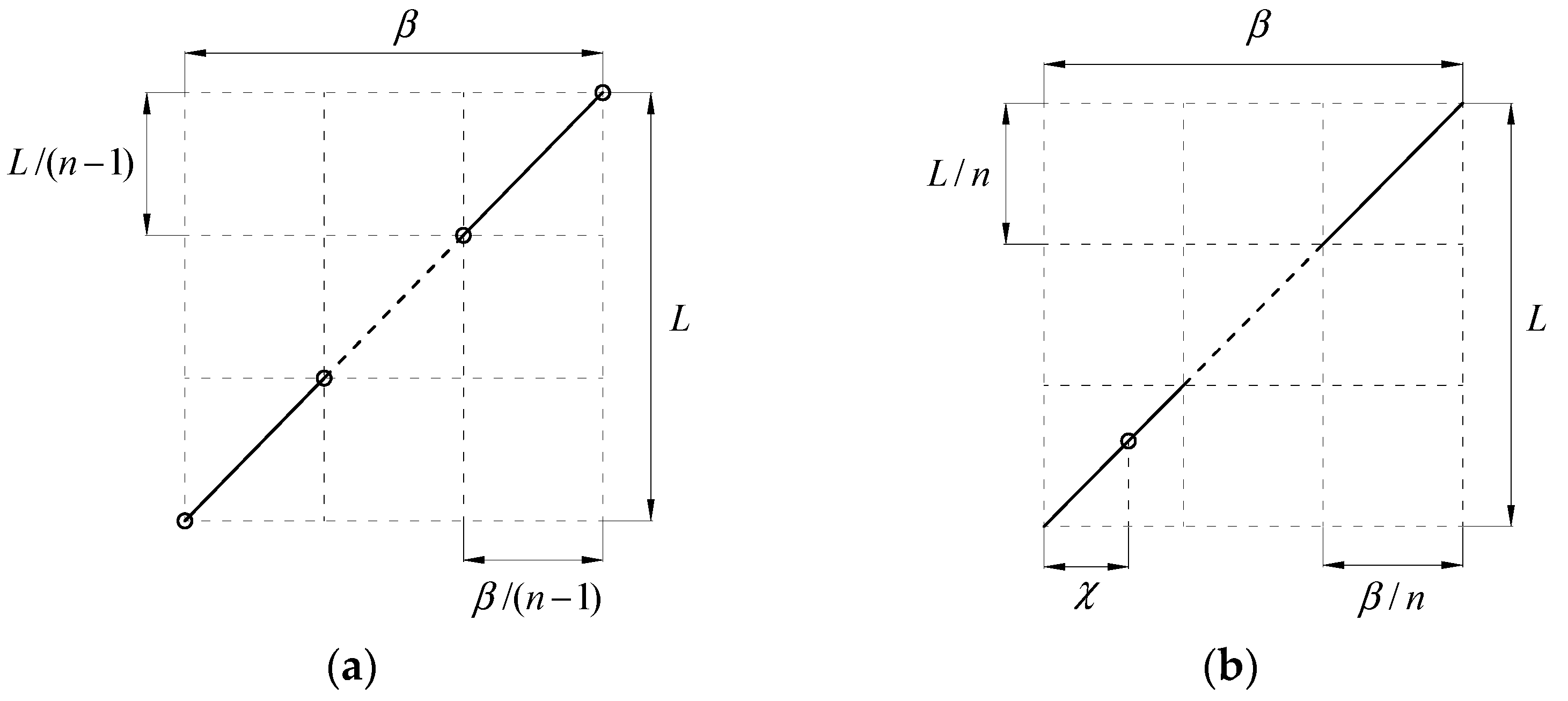
3.1. Principle
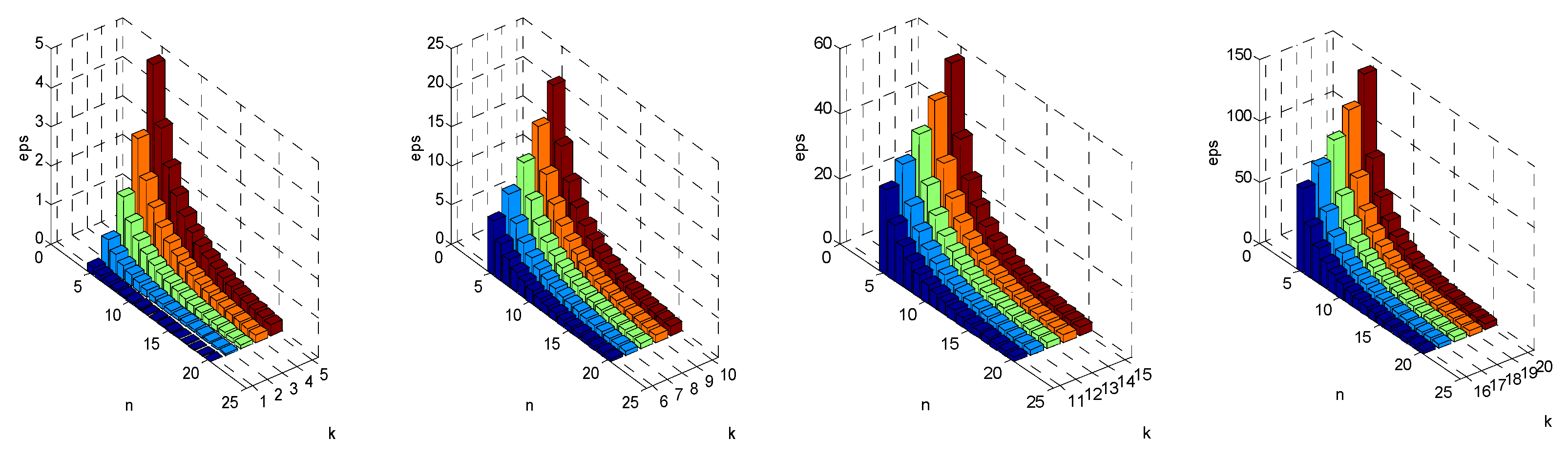
3.2. Calculation Accuracy
4. Calculation and Analysis of Prototype
4.1. Selection of Segment Number
4.2. Two-Dimensional Finite Element Model of Prototype
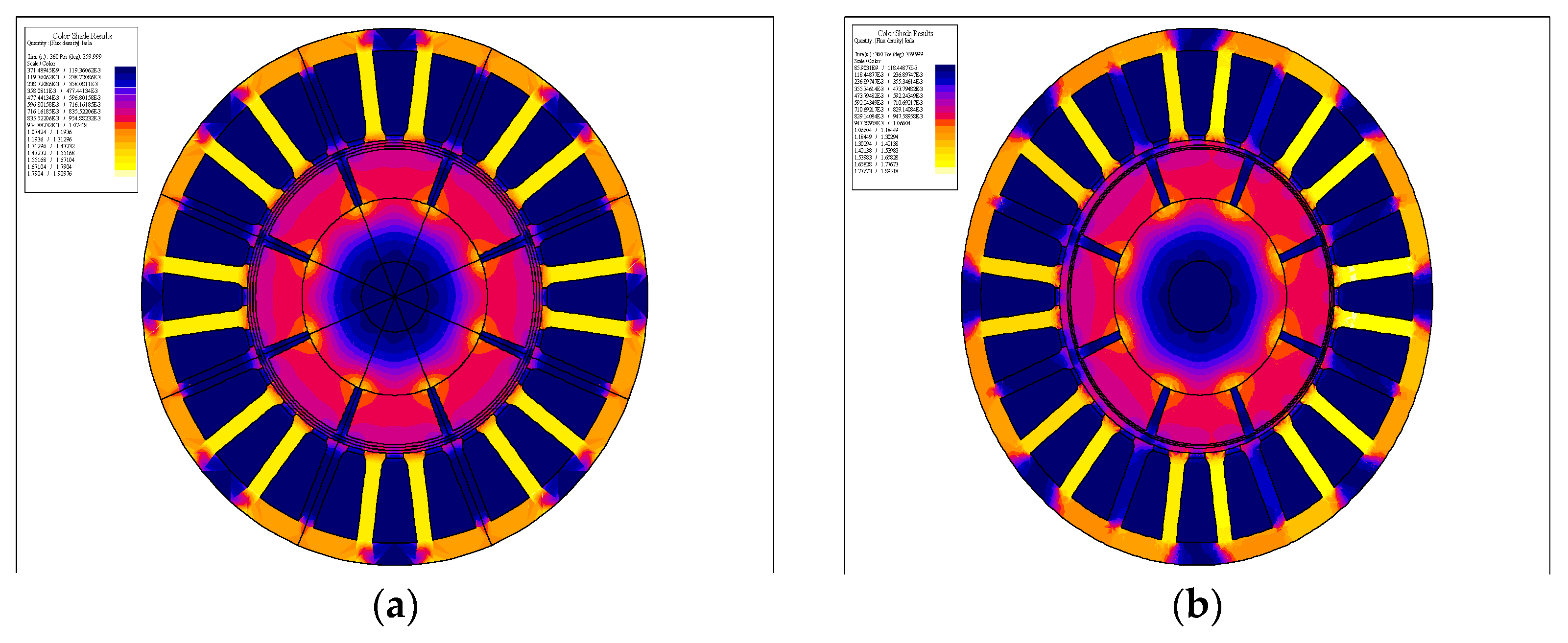
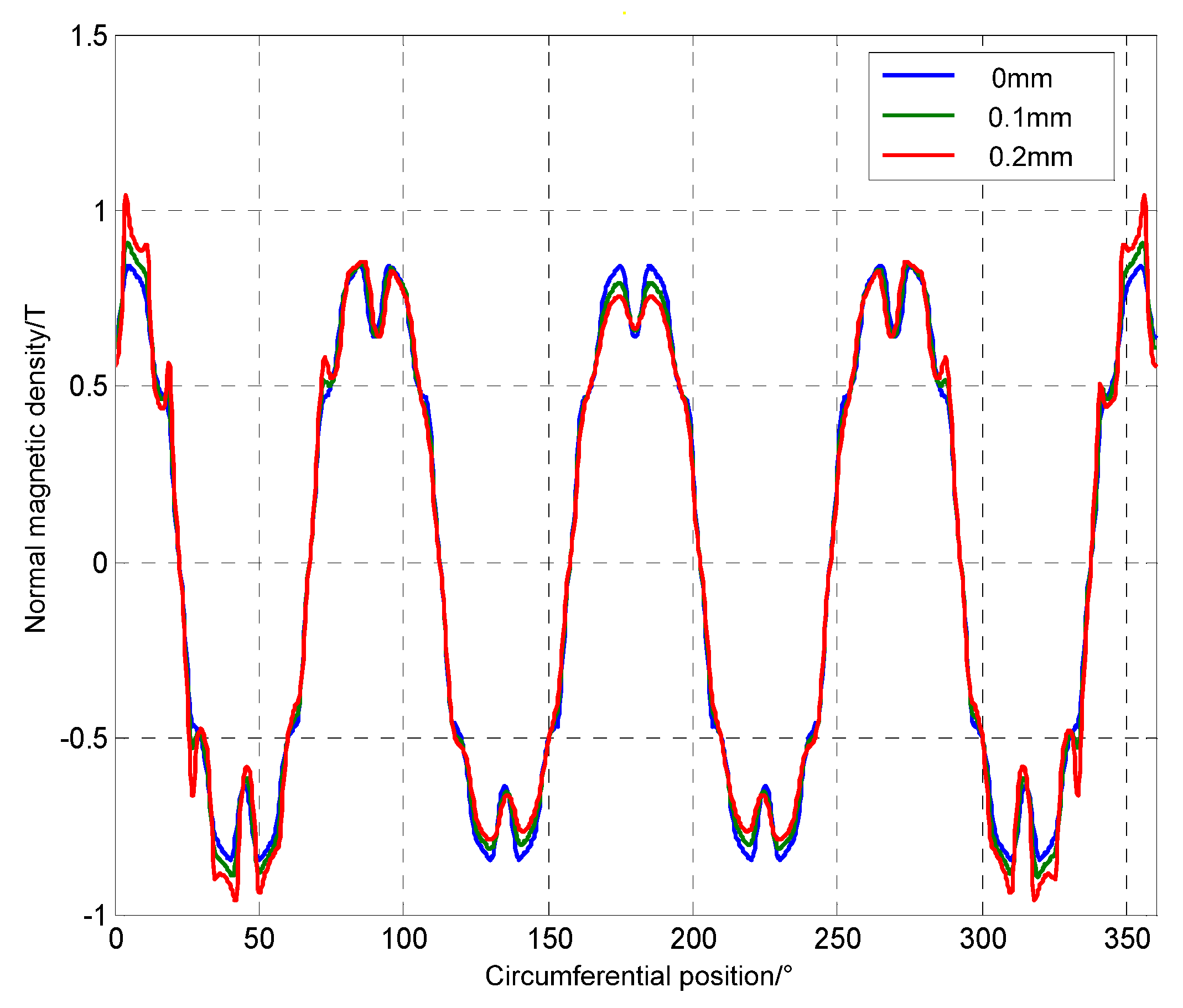
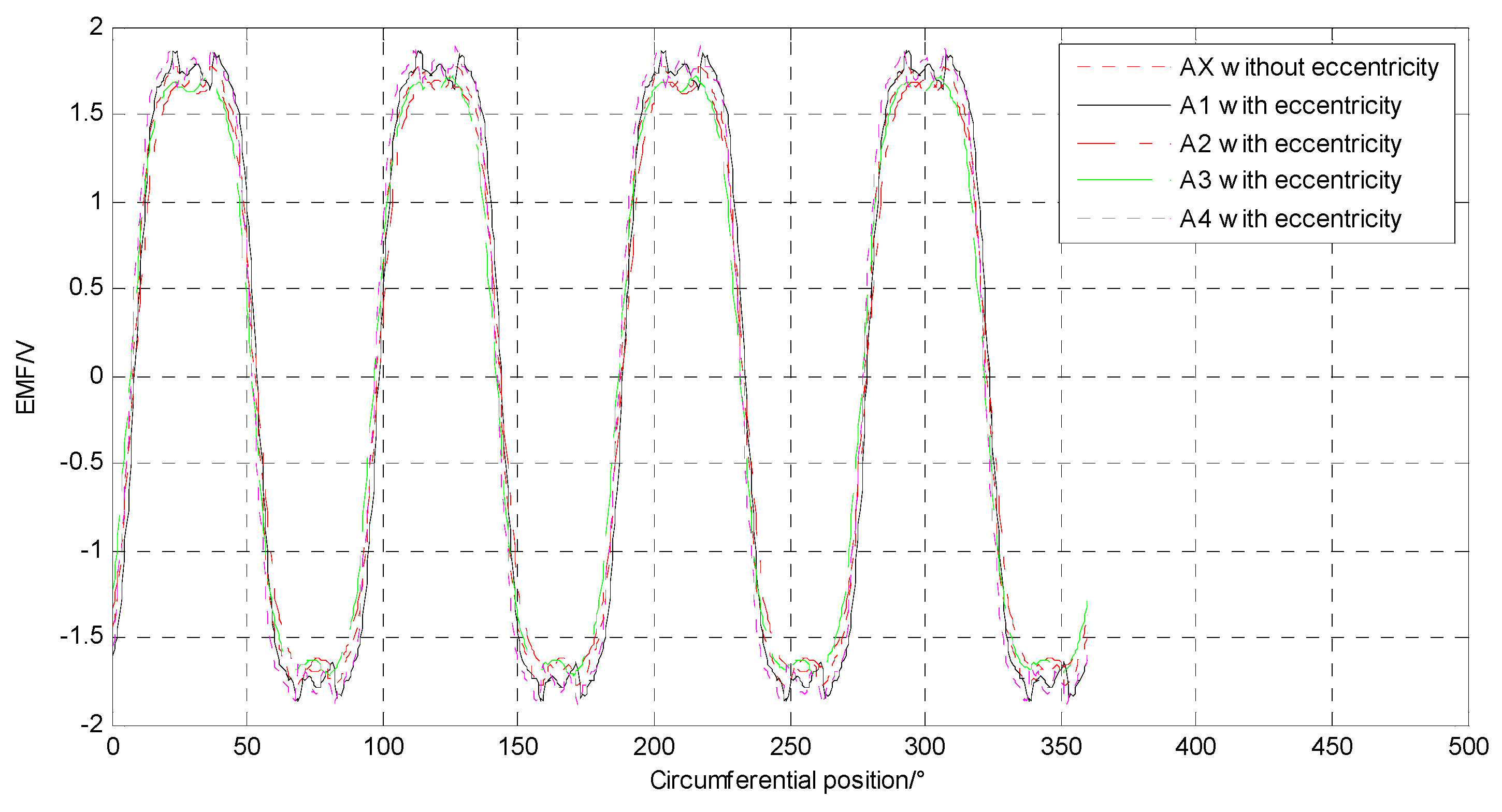
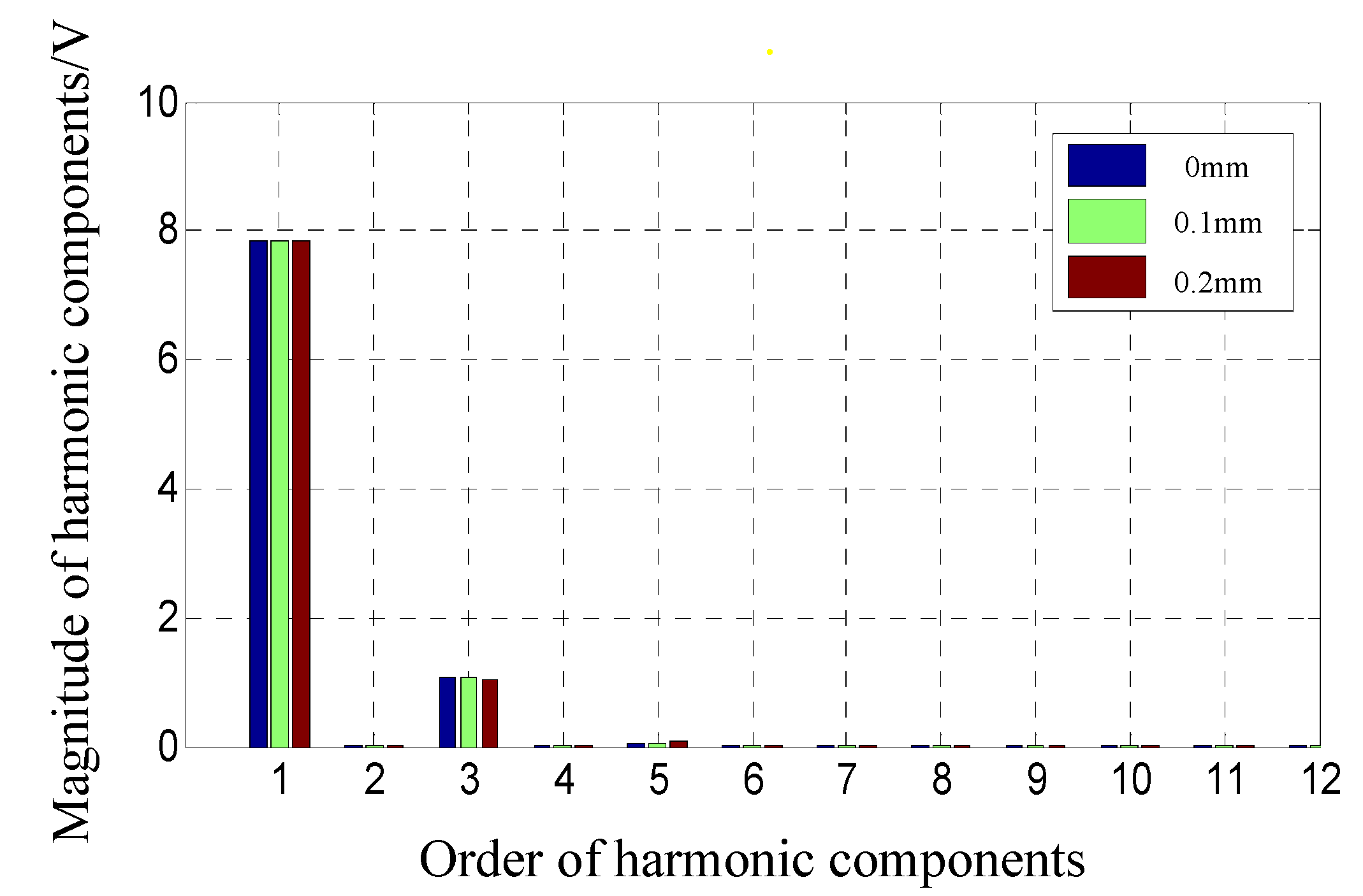
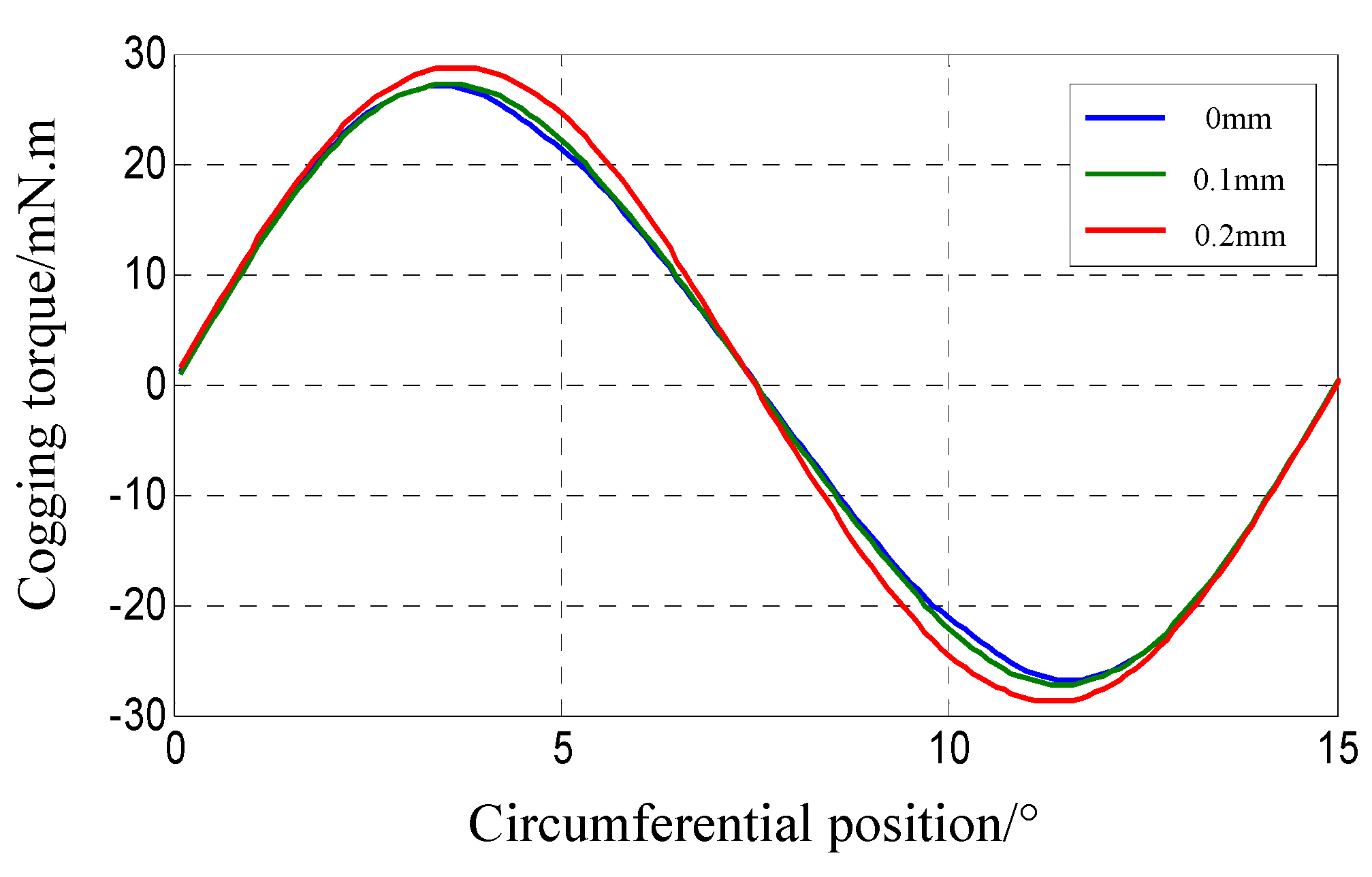
4.3. Calculation and Analysis
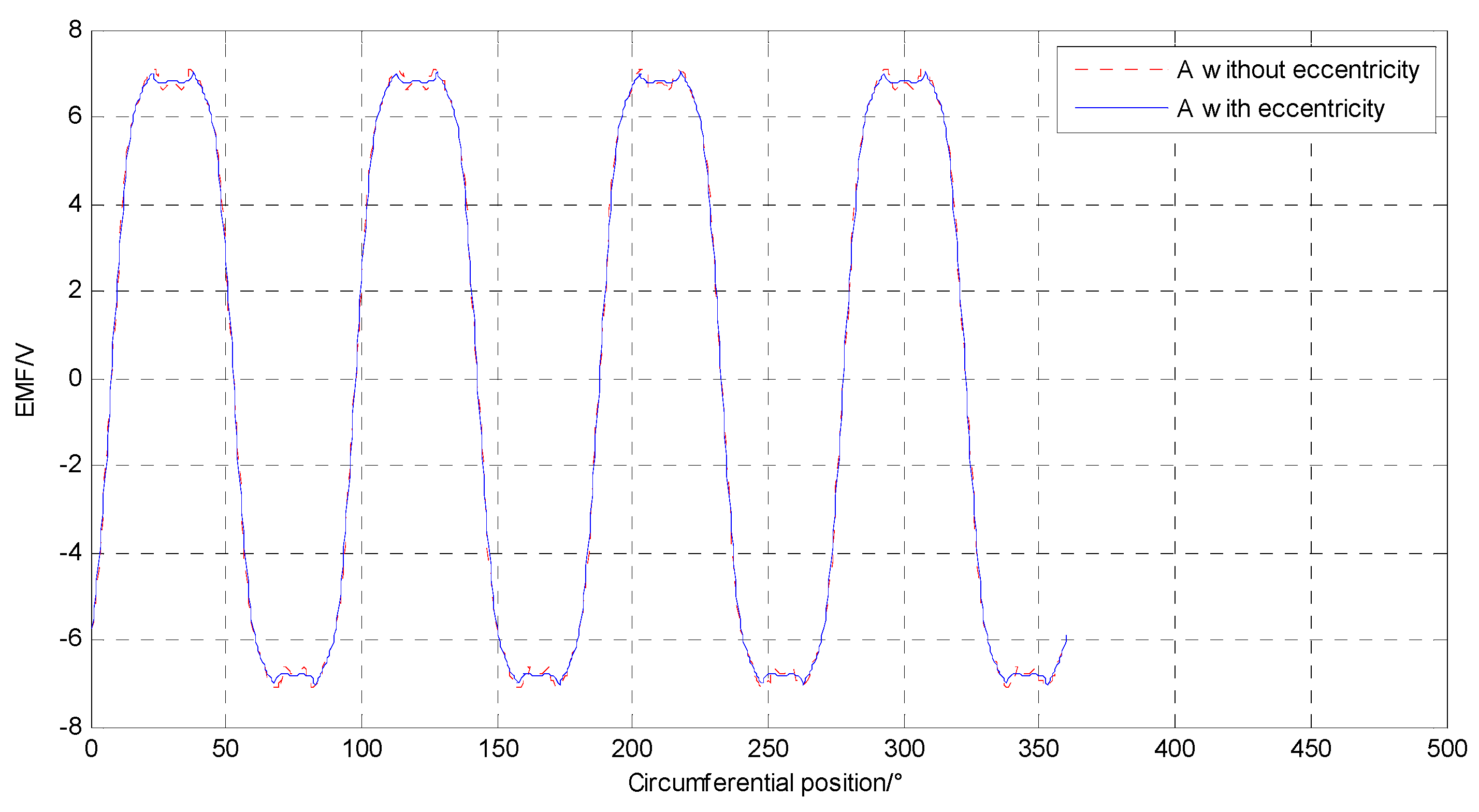
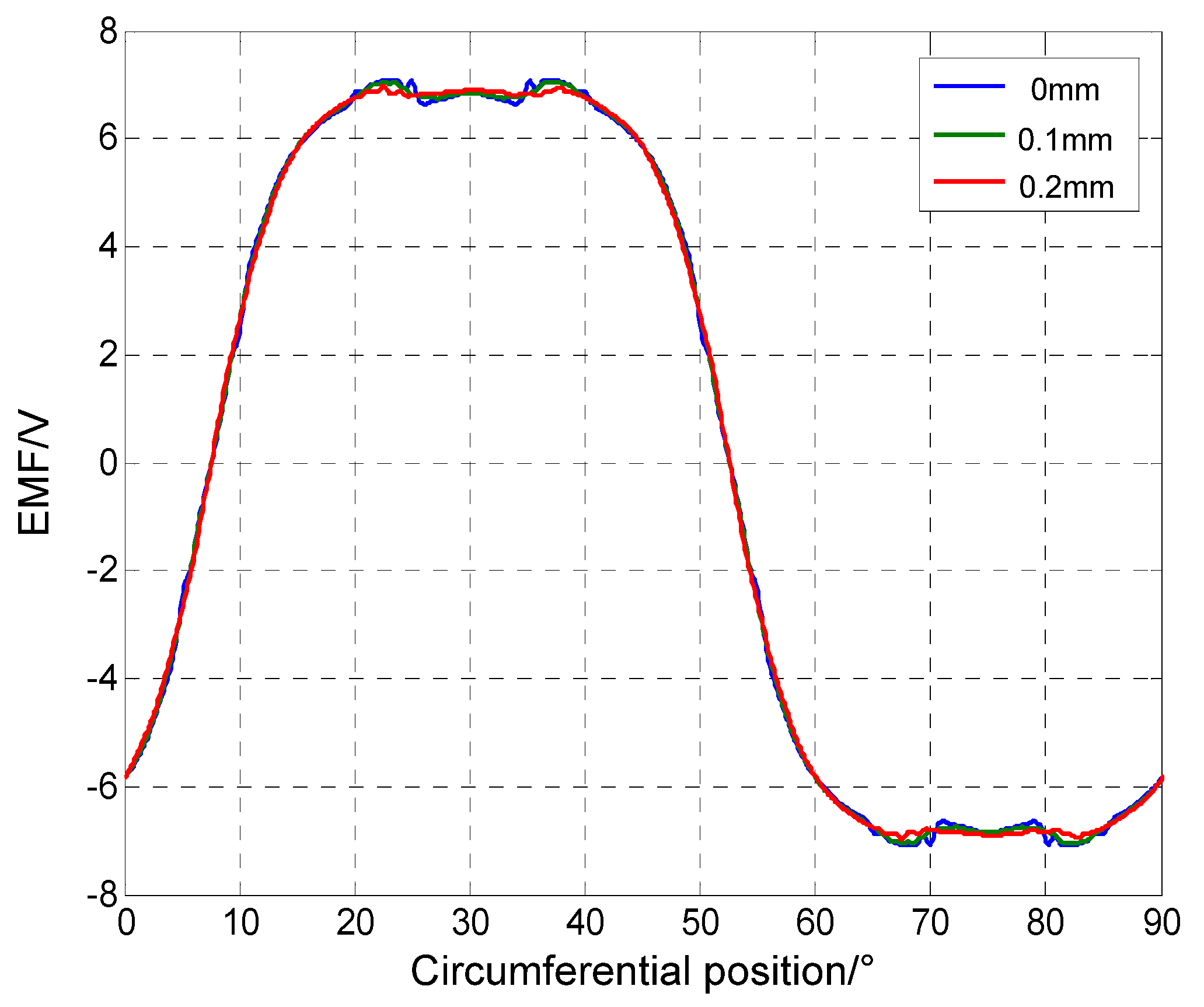
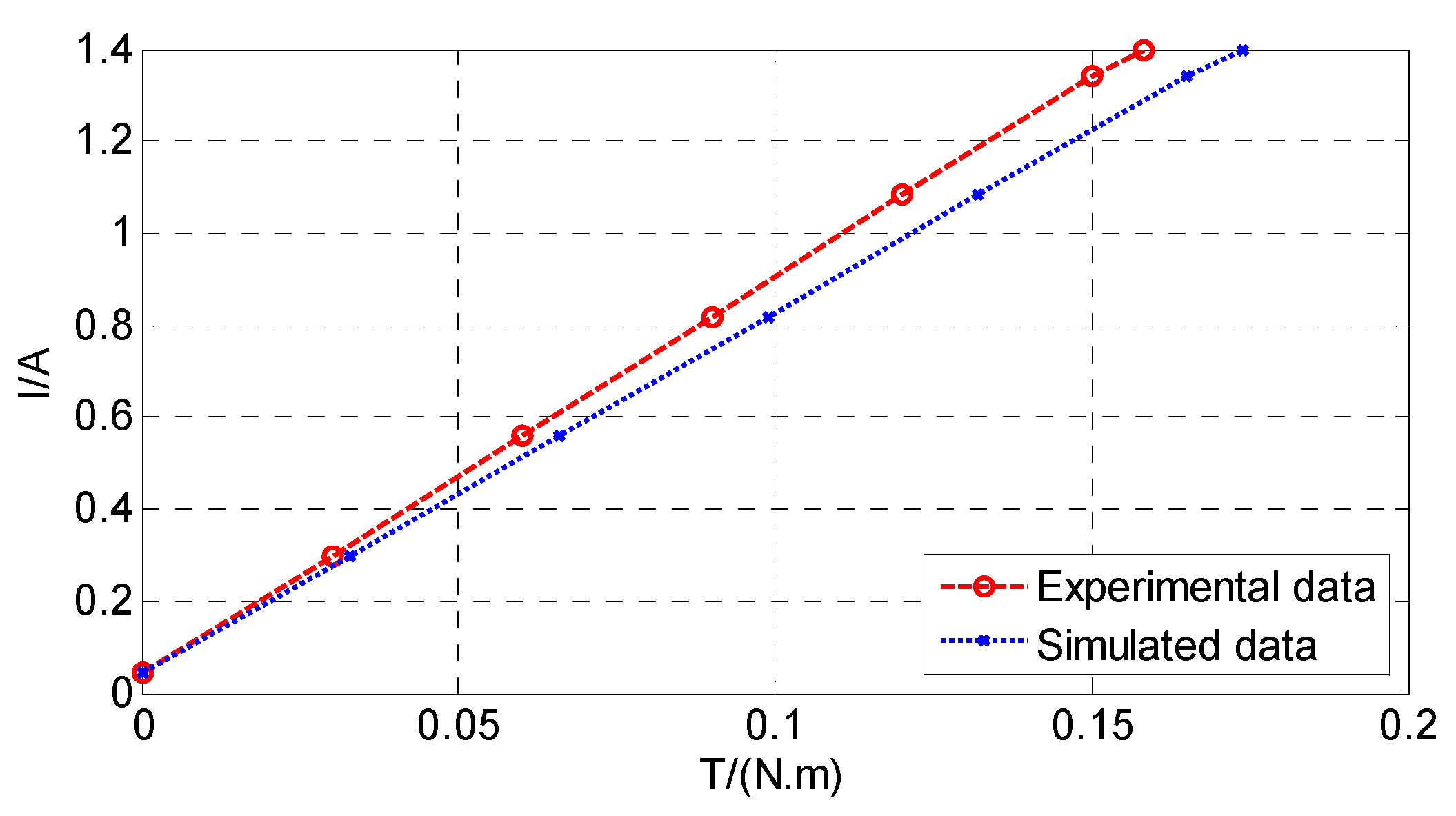
5. Experiment
6. Conclusions
Funding
Conflicts of Interest
References
- Zhang, X.; Zhang, W.; Liang, X.; Lu, P. Performance analysis and comparison for two topologies of flux-switching permanent magnet machine. China Electrotech. Soc. Trans. Electr. Mach. Syst. 2020, 4, 190–197. [Google Scholar] [CrossRef]
- Zhu, G.; Liu, X.; Li, L.; Chen, H.; Zhu, J. Coupled electromagnetic-thermal-fluidic analysis of permanent magnet synchronous machines with a modified model. China Electrotech. Soc. Trans. Electr. Mach. Syst. 2019, 3, 204–209. [Google Scholar] [CrossRef]
- Li, W.; Cheng, M. Investigation of influence of winding structure on reliability of permanent magnet machines. China Electrotech. Soc. Trans. Electr. Mach. Syst. 2020, 4, 87–95. [Google Scholar] [CrossRef]
- Sun, T.; Liu, X.; Zou, Y.; Huang, C.; Liang, J. Design and optimization of a mechanical variable-leakage-flux interior permanent magnet machine with auxiliary rotatable magnetic poles. China Electrotech. Soc. Trans. Electr. Mach. Syst. 2021, 5, 21–29. [Google Scholar] [CrossRef]
- Tong, W.; Dai, S.; Li, S.; Li, J.; Tang, R. Modeling and Analysis of Axial Flux Permanent Magnet Machines with Coexistence of Rotor Radial Deviation and Angular Eccentricity. IEEE Trans. Energy Convers. 2020, 35, 2181–2190. [Google Scholar] [CrossRef]
- Wang, W.; Zheng, P.; Wang, M.; Liu, Y.; Fu, Z.; Sui, Y. Demagnetization and Permanent-Magnet Minimization Analyses of Less-Rare-Earth Interior Permanent-Magnet Synchronous Machines Used for Electric Vehicles. IEEE Trans. Magn. 2018, 54, 1–5. [Google Scholar] [CrossRef]
- Ciceo, S.; Chauvicourt, F.; Martis, J.G.C. PMASynRM system-level NVH model with eccentricity. In Proceedings of the 2020 IEEE Transportation Electrification Conference & Expo (ITEC), Chicago, IL, USA, 23–26 June 2020; pp. 82–86. [Google Scholar] [CrossRef]
- Park, Y.; Fernandez, D.; Bin Lee, S.; Hyun, D.; Jeong, M.; Kommuri, S.K.; Cho, C.; Reigosa, D.D.; Briz, F. Online Detection of Rotor Eccentricity and Demagnetization Faults in PMSMs Based on Hall-Effect Field Sensor Measurements. IEEE Trans. Ind. Appl. 2019, 55, 2499–2509. [Google Scholar] [CrossRef]
- Ojaghi, M.; Mohammadi, M. Unified Modeling Technique for Axially Uniform and Nonuniform Eccentricity Faults in Three-Phase Squirrel Cage Induction Motors. IEEE Trans. Ind. Electron. 2017, 65, 5292–5301. [Google Scholar] [CrossRef]
- Echeverria, J.J.R.; Da Silva, P.V.V.; Bortoni, E.D.C. Analysis of Orbital Eccentricity and UMP in Large Salient Pole Synchronous Machines. IEEE Trans. Ind. Appl. 2019, 55, 4715–4722. [Google Scholar] [CrossRef]
- Lee, H.-K.; Shin, K.-H.; Bang, T.-K.; Nah, J.-H.; Choi, J.-Y. Experimental Verification and Analytical Study of Influence of Rotor Eccentricity on Electromagnetic Characteristics of Permanent Magnet Machine. IEEE Trans. Appl. Supercond. 2020, 30, 1–5. [Google Scholar] [CrossRef]
- Kim, H.; Posa, A.; Nerg, J.; Heikkinen, J.; Sopanen, J.T. Analysis of Electromagnetic Excitations in an Integrated Centrifugal Pump and Permanent Magnet Synchronous Motor. IEEE Trans. Energy Convers. 2019, 34, 1759–1768. [Google Scholar] [CrossRef]
- Lasjerdi, H.; Nasiri-Gheidari, Z.; Tootoonchian, F. Online Static/Dynamic Eccentricity Fault Diagnosis in Inverter-Driven Electrical Machines Using Resolver Signals. IEEE Trans. Energy Convers. 2020, 35, 1973–1980. [Google Scholar] [CrossRef]
- Aggarwal, A.; Allafi, I.M.; Strangas, E.G.; Agapiou, J.S. Off-Line Detection of Static Eccentricity of PMSM Robust to Machine Operating Temperature and Rotor Position Misalignment Using Incremental Inductance Approach. IEEE Trans. Transp. Electrif. 2021, 7, 161–169. [Google Scholar] [CrossRef]
- Shin, J.; Park, Y.; Bin Lee, S. Flux-Based Detection and Classification of Induction Motor Eccentricity, Rotor Cage, and Load Defects. IEEE Trans. Ind. Appl. 2021, 57, 2471–2480. [Google Scholar] [CrossRef]
- Wang, S.; Hong, J.; Sun, Y.; Cao, H. Effect Comparison of Zigzag Skew PM Pole and Straight Skew Slot for Vibration Mitigation of PM Brush DC Motors. IEEE Trans. Ind. Electron. 2019, 67, 4752–4761. [Google Scholar] [CrossRef]
- Shi, Z.; Sun, X.; Cai, Y.; Yang, Z.; Lei, G.; Guo, Y.; Zhu, J. Torque Analysis and Dynamic Performance Improvement of a PMSM for EVs by Skew Angle Optimization. IEEE Trans. Appl. Supercond. 2019, 29, 1–5. [Google Scholar] [CrossRef]
- Ueda, Y.; Takahashi, H. Transverse-Flux Motor Design with Skewed and Unequally Distributed Armature Cores for Reducing Cogging Torque. IEEE Trans. Magn. 2017, 53, 1–5. [Google Scholar] [CrossRef]
- Jo, I.-H.; Lee, H.-W.; Jeong, G.; Ji, W.-Y.; Park, C.-B. A Study on the Reduction of Cogging Torque for the Skew of a Magnetic Geared Synchronous Motor. IEEE Trans. Magn. 2019, 55, 1–5. [Google Scholar] [CrossRef]
- Wu, X.; Zhu, Z.Q.; Wu, Z.; Liu, T.; Li, Y. Analysis and Suppression of Rotor Eccentricity Effects on Fundamental Model Based Sensorless Control of Permanent Magnet Synchronous Machine. IEEE Trans. Ind. Appl. 2020, 56, 4896–4905. [Google Scholar] [CrossRef]
- Tong, W.; Li, S.; Pan, X.; Wu, S.; Tang, R. Analytical Model for Cogging Torque Calculation in Surface-Mounted Permanent Magnet Motors With Rotor Eccentricity and Magnet Defects. IEEE Trans. Energy Convers. 2020, 35, 2191–2200. [Google Scholar] [CrossRef]
- Hu, Y.; Wei, H.; Chen, H.; Sun, W.; Zhao, S.; Li, L. Vibration Study of Permanent Magnet Synchronous Motor Base on Static Eccentricity Model. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Ito, F.; Takeuchi, K.; Kotsugai, T.; Matsushita, M. A Study on Asymmetry of Electromagnetic Force Modes of Permanent Magnet Synchronous Motors With Rotor Eccentricity. IEEE Trans. Magn. 2021, 57, 1–6. [Google Scholar] [CrossRef]
- Abdi, S.; Abdi, E.; Toshani, H.; McMahon, R. Vibration Analysis of Brushless Doubly Fed Machines in the Presence of Rotor Eccentricity. IEEE Trans. Energy Convers. 2020, 35, 1. [Google Scholar] [CrossRef]
- De Gersem, H.; D’Angelo, L.A.M. Modeling Skew by Single- and Multi-Slice 2-D Machine Models. IEEE Trans. Magn. 2021, 57, 1–4. [Google Scholar] [CrossRef]
- Hong, C.; Huang, W.; Hu, Z. Performance Calculation of a Dual Stator Solid Rotor Axial Flux Induction Motor Using the Multi-Slice and Multi-Layer Method. IEEE Trans. Magn. 2018, 55, 1–9. [Google Scholar] [CrossRef]
- Zhang, D.; Liu, T.; He, C.; Wu, T. A New 2-D Multi-Slice Time-Stepping Finite Element Method and Its Application in Analyzing the Transient Characteristics of Induction Motors under Symmetrical Sag Conditions. IEEE Access 2018, 6, 47036–47046. [Google Scholar] [CrossRef]
- Liu, T.; Jiang, M.; Zhang, D.; Zhao, H.; Shuang, F. Effect of Symmetrical Voltage Sag on Induction Motor Considering Phase-Angle Factors Based on a New 2-D Multi-Slice Time-Stepping Finite Element Method. IEEE Access 2020, 8, 75946–75956. [Google Scholar] [CrossRef]


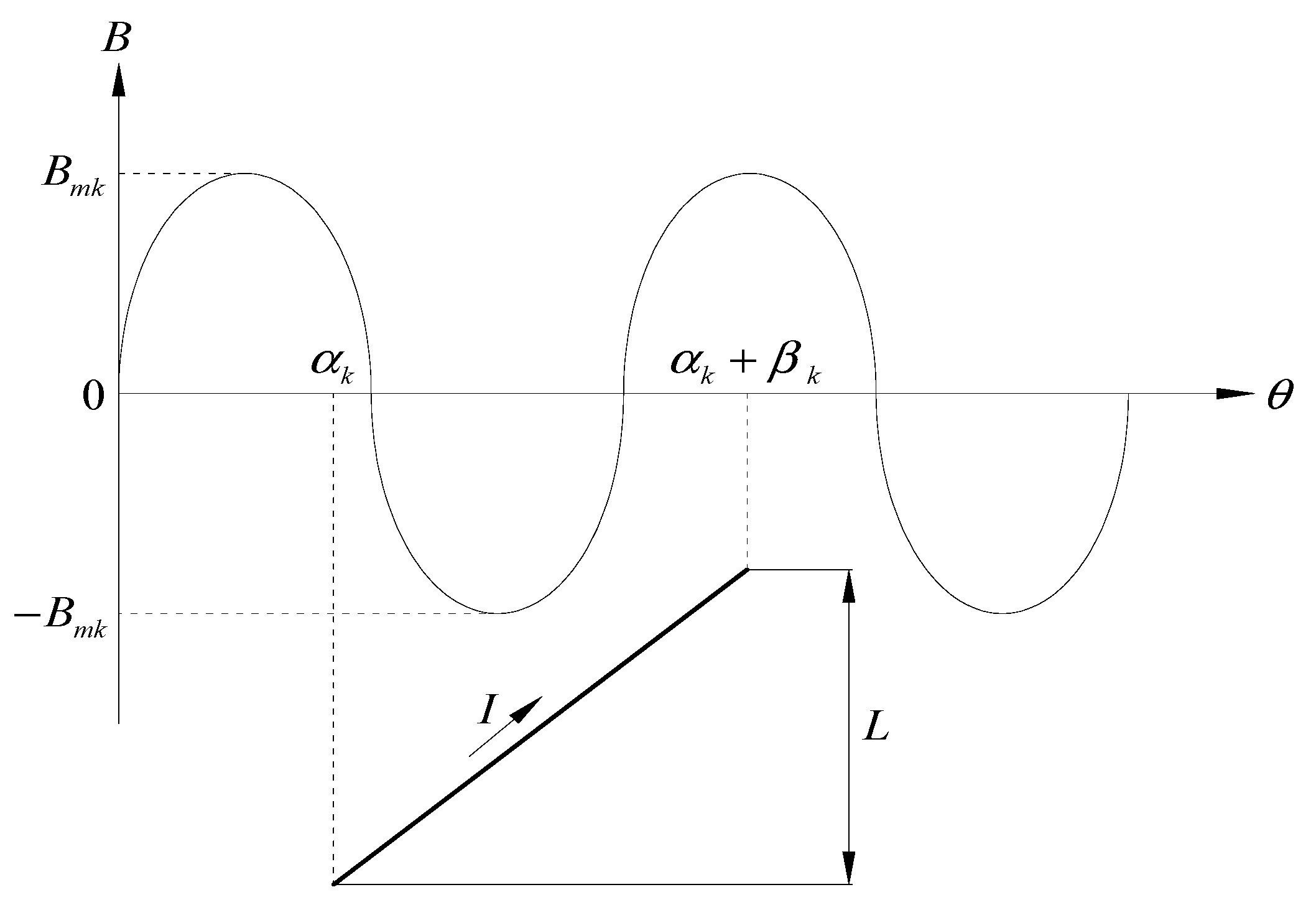







Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L. Static Eccentricity Fault Analysis of Surface-Mounted Permanent Magnet Electromotor with Skewed Slots Based on 2D FEA. World Electr. Veh. J. 2021, 12, 176. https://doi.org/10.3390/wevj12040176
Wang L. Static Eccentricity Fault Analysis of Surface-Mounted Permanent Magnet Electromotor with Skewed Slots Based on 2D FEA. World Electric Vehicle Journal. 2021; 12(4):176. https://doi.org/10.3390/wevj12040176
Chicago/Turabian StyleWang, Li. 2021. "Static Eccentricity Fault Analysis of Surface-Mounted Permanent Magnet Electromotor with Skewed Slots Based on 2D FEA" World Electric Vehicle Journal 12, no. 4: 176. https://doi.org/10.3390/wevj12040176
APA StyleWang, L. (2021). Static Eccentricity Fault Analysis of Surface-Mounted Permanent Magnet Electromotor with Skewed Slots Based on 2D FEA. World Electric Vehicle Journal, 12(4), 176. https://doi.org/10.3390/wevj12040176




