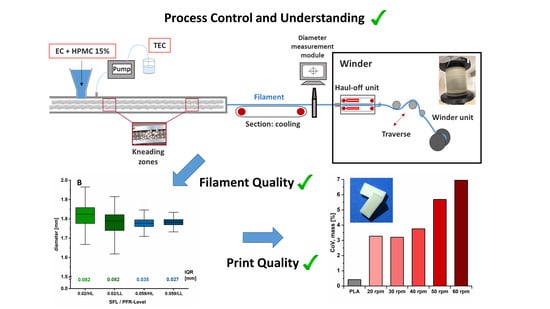
Hot-Melt Extrusion Process Fluctuations and Their Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
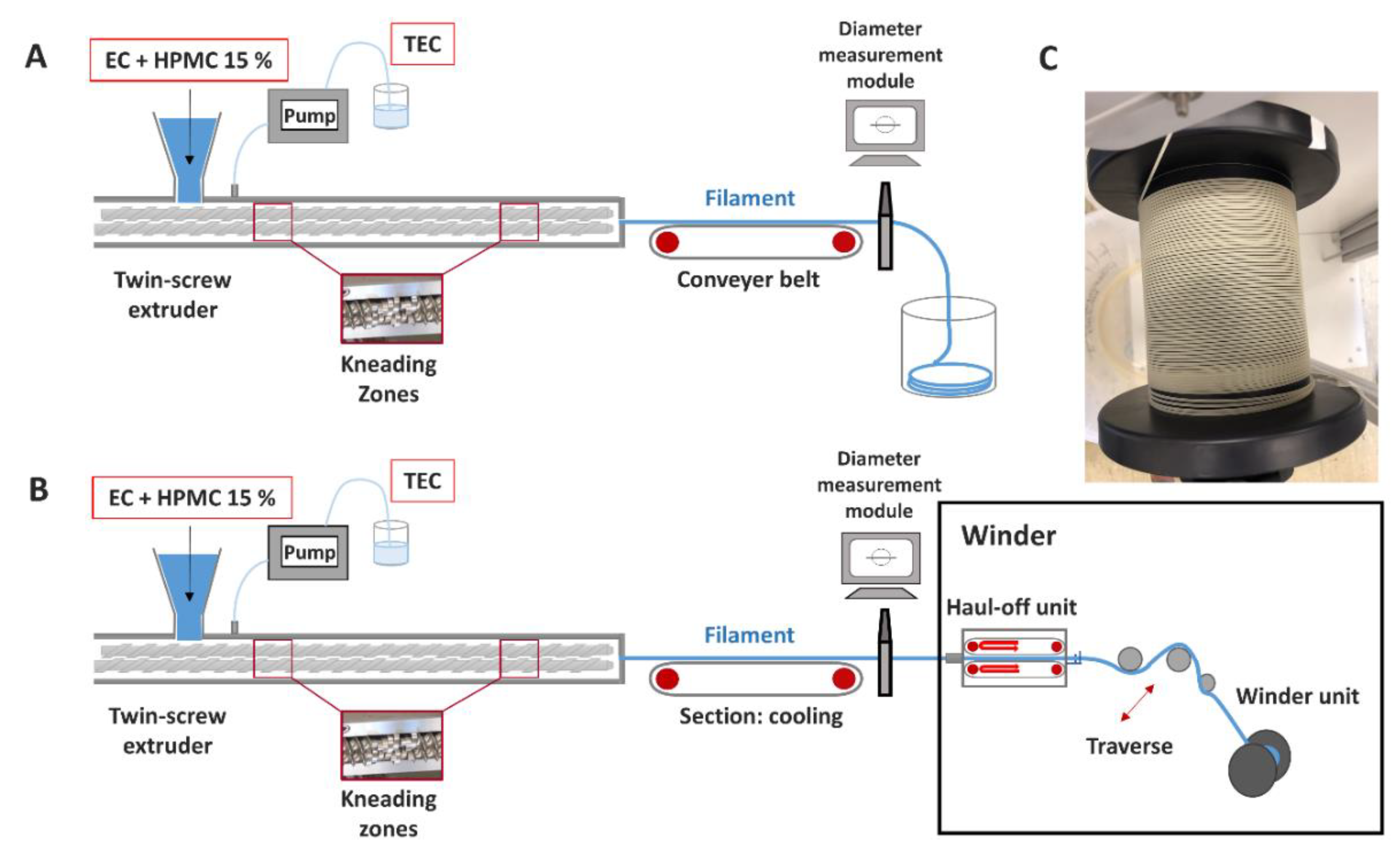
2.2.1. Production of Filaments via HME
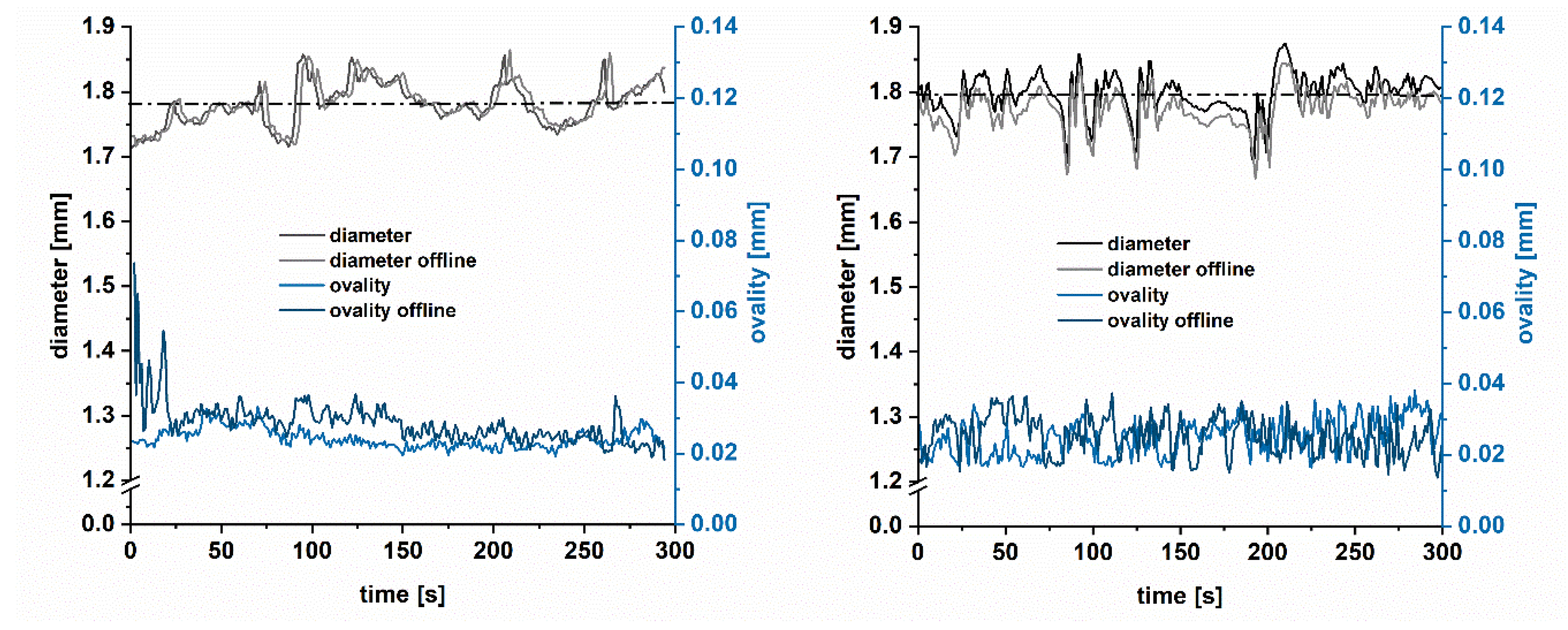
2.2.2. Inline Diameter and Ovality Determination of Filaments
2.2.3. Offline Diameter Determination of Filaments
2.2.4. D-Printing of Commercial and In-House Produced Filaments and Determination of Uniformity of Mass
2.2.5. Mechanical Properties
2.2.6. Determination of Specific Feed Load
2.2.7. Statistical Analysis
3. Results and Discussion
3.1. HME Process Setup
3.2. Process Analysis
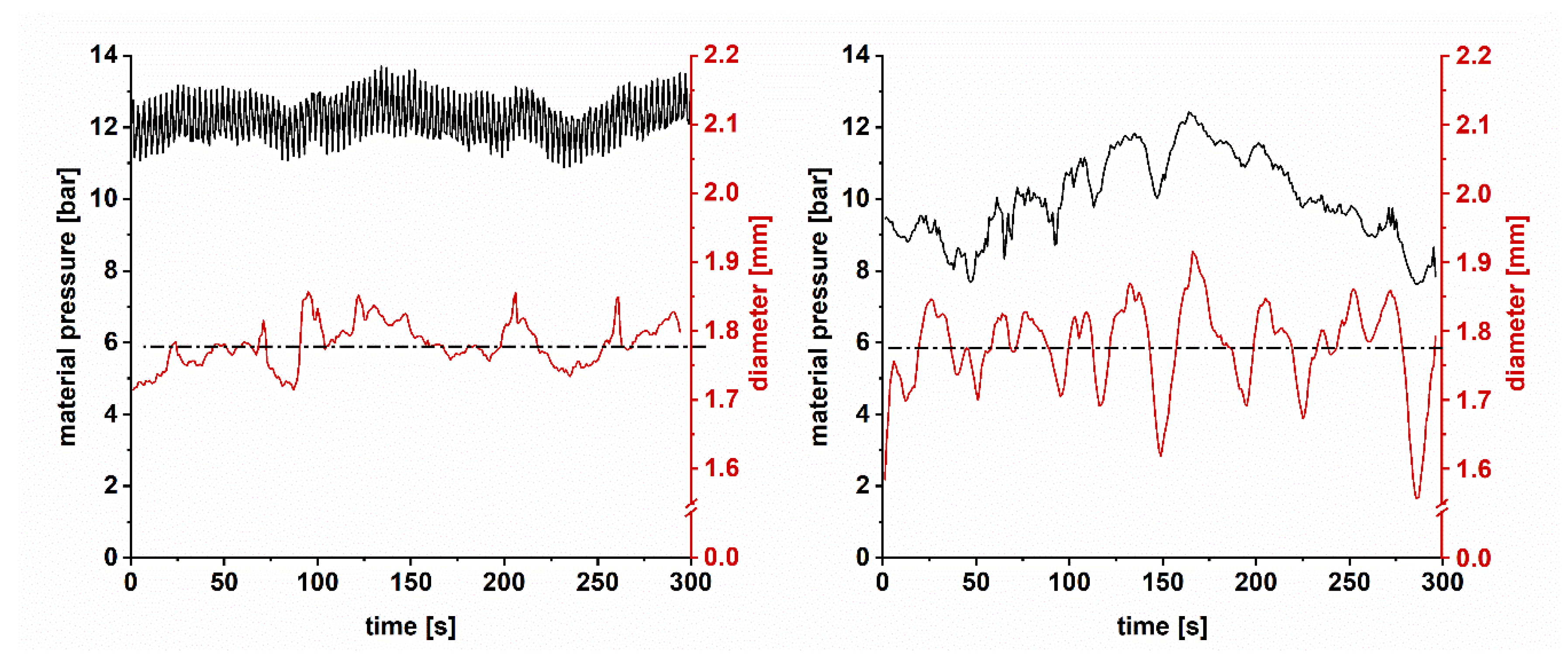
3.2.1. Correlation of Material Pressure and Diameter Variations
3.2.2. Influence of Powder Feed Rate
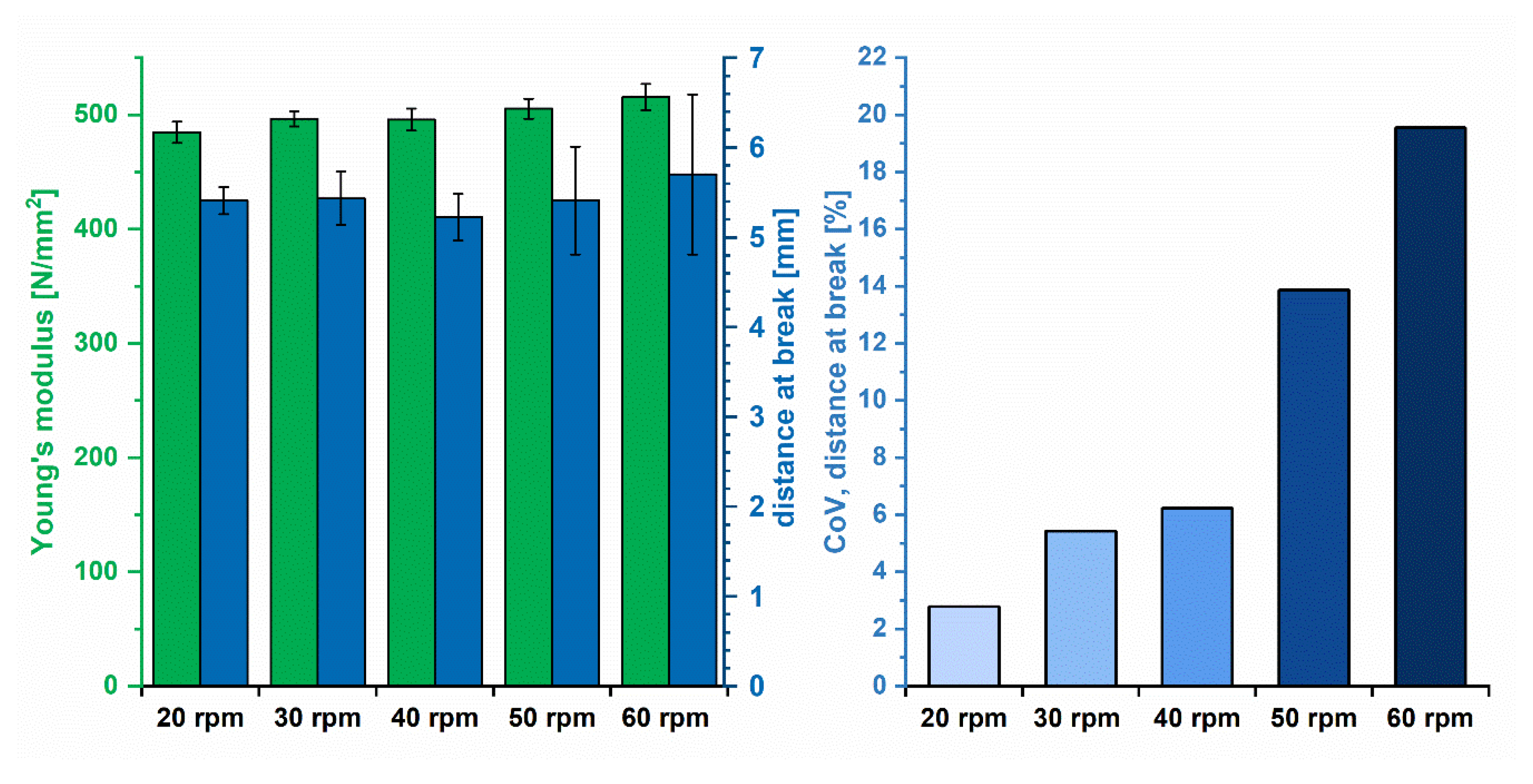
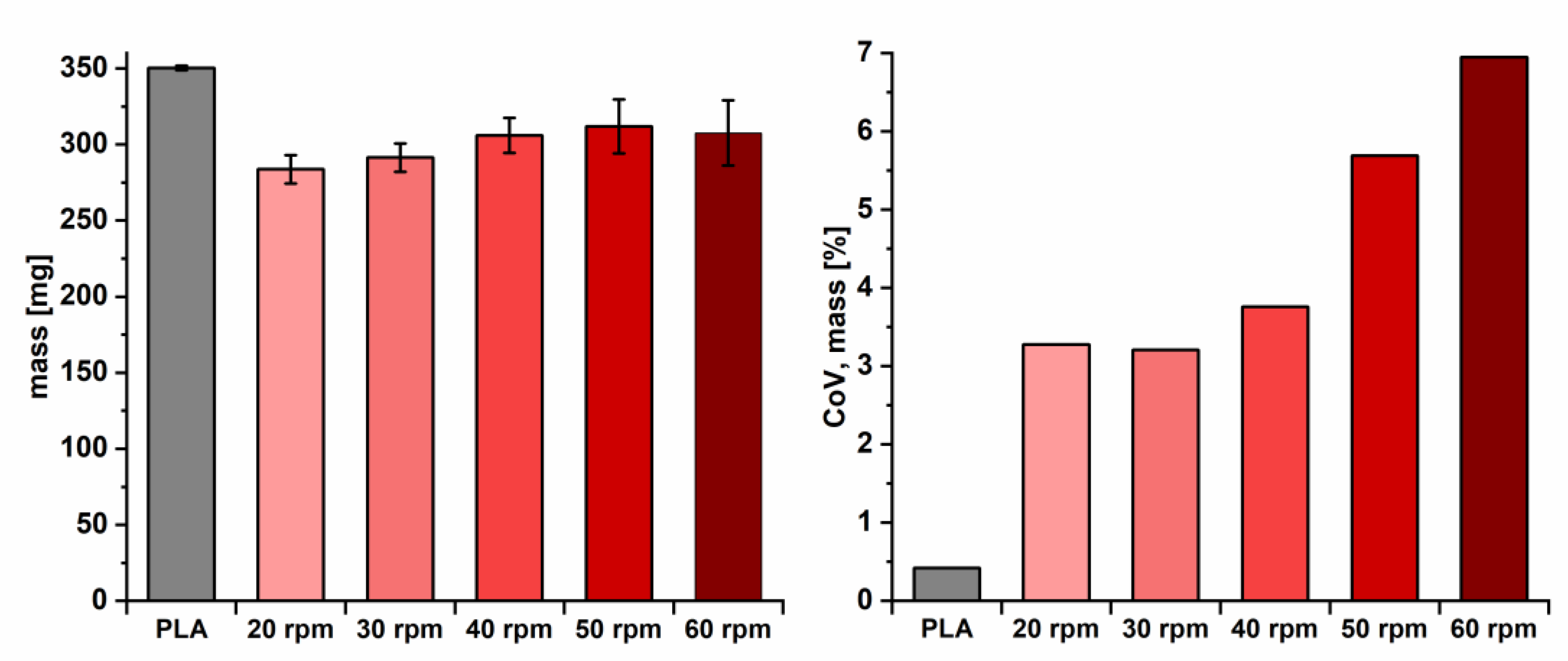
3.2.3. Influence of Screw Speed
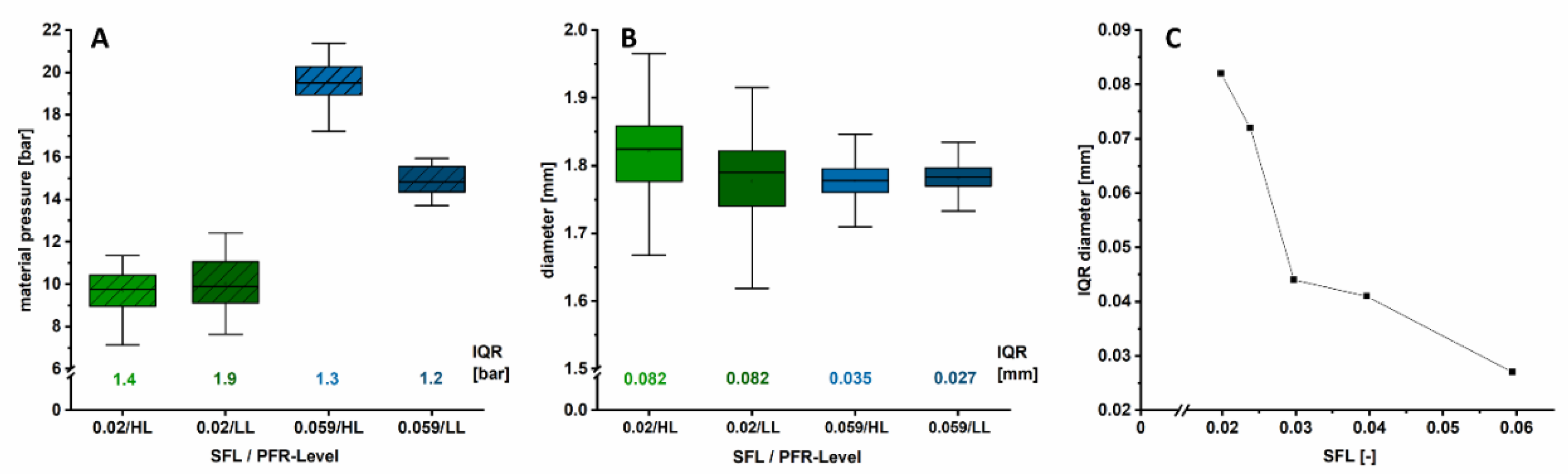
3.2.4. Influence of SFL on Diameter Homogeneity
3.2.5. Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Alhnan, M.A.; Okwuosa, T.C.; Sadia, M.; Wan, K.-W.; Ahmed, W.; Arafat, B. Emergence of 3D Printed Dosage Forms: Opportunities and Challenges. Pharm. Res. 2016, 33, 1817–1832. [Google Scholar] [CrossRef]
- El Aita, I.; Ponsar, H.; Quodbach, J. A Critical Review on 3D-printed Dosage Forms. Curr. Pharm. Des. 2019, 24, 4957–4978. [Google Scholar] [CrossRef]
- Kempin, W.; Franz, C.; Koster, L.-C.; Schneider, F.; Bogdahn, M.; Weitschies, W.; Seidlitz, A. Assessment of different polymers and drug loads for fused deposition modeling of drug loaded implants. Eur. J. Pharm. Biopharm. 2017, 115, 84–93. [Google Scholar] [CrossRef]
- Korte, C.; Quodbach, J. Formulation development and process analysis of drug-loaded filaments manufactured via hot-melt extrusion for 3D-printing of medicines. Pharm. Dev. Technol. 2018, 23, 1117–1127. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Feng, X.; Patil, H.; Tiwari, R.; Repka, M.A. Coupling 3D printing with hot-melt extrusion to produce controlled-release tablets. Int. J. Pharm. 2017, 519, 186–197. [Google Scholar] [CrossRef] [PubMed]
- Alice, M.; Parietti, F.; Maroni, A.; Foppoli, A.; Andrea, G.; Zema, L. Hot-melt extruded filaments based on pharmaceutical grade polymers for 3D printing by fused deposition modeling. Int. J. Pharm. 2016, 509, 255–263. [Google Scholar] [CrossRef]
- Nasereddin, J.; Wellner, N.; Alhijjaj, M.; Belton, P.; Qi, S. Development of a Simple Mechanical Screening Method for Predicting the Feedability of a Pharmaceutical FDM 3D Printing Filament. Pharm. Res. 2018, 35, 151. [Google Scholar] [CrossRef] [PubMed]
- Fuenmayor, E.; Forde, M.; Healy, A.V.; Devine, D.M.; Lyons, J.G.; McConville, C.; Major, I. Material Considerations for Fused-Filament Fabrication of Solid Dosage Forms. Pharmaceutics 2018, 10, 44. [Google Scholar] [CrossRef]
- Prasad, E.; Islam, M.; Goodwin, D.J.; Megarry, A.J.; Halbert, G.W.; Florence, A.J.; Robertson, J. Development of a hot-melt extrusion (HME) process to produce drug loaded Affinisol™ 15LV filaments for fused filament fabrication (FFF) 3D printing. Addit. Manuf. 2019, 29, 100776. [Google Scholar] [CrossRef]
- Muwaffak, Z.; Goyanes, A.; Clark, V.; Basit, A.W.; Hilton, S.T.; Gaisford, S.; Hassan, Z.M. Patient-specific 3D scanned and 3D printed antimicrobial polycaprolactone wound dressings. Int. J. Pharm. 2017, 527, 161–170. [Google Scholar] [CrossRef]
- Maroni, A.; Alice, M.; Parietti, F.; Foppoli, A.; Zema, L.; Gazzaniga, A. 3D printed multi-compartment capsular devices for two-pulse oral drug delivery. J. Control. Release 2017, 268, 10–18. [Google Scholar] [CrossRef] [PubMed]
- Beck, R.C.R.; Chaves, P.; Goyanes, A.; Vukosavljevic, B.; Buanz, A.; Windbergs, M.; Basit, A.; Gaisford, S. 3D printed tablets loaded with polymeric nanocapsules: An innovative approach to produce customized drug delivery systems. Int. J. Pharm. 2017, 528, 268–279. [Google Scholar] [CrossRef]
- Holländer, J.; Genina, N.; Jukarainen, H.; Khajeheian, M.; Rosling, A.; Mäkilä, E.M.; Sandler, N. Three-Dimensional Printed PCL-Based Implantable Prototypes of Medical Devices for Controlled Drug Delivery. J. Pharm. Sci. 2016, 105, 2665–2676. [Google Scholar] [CrossRef] [PubMed]
- Korte, C.; Quodbach, J. 3D-Printed Network Structures as Controlled-Release Drug Delivery Systems: Dose Adjustment, API Release Analysis and Prediction. AAPS PharmSciTech 2018, 19, 3333–3342. [Google Scholar] [CrossRef] [PubMed]
- Kempin, W.; Domsta, V.; Grathoff, G.; Brecht, I.; Semmling, B.; Tillmann, S.; Weitschies, W.; Seidlitz, A. Immediate Release 3D-Printed Tablets Produced Via Fused Deposition Modeling of a Thermo-Sensitive Drug. Pharm. Res. 2018, 35, 124. [Google Scholar] [CrossRef]
- Zhang, J.; Yang, W.; Vo, A.Q.; Feng, X.; Ye, X.; Kim, D.W.; Repka, M.A. Hydroxypropyl methylcellulose-based controlled release dosage by melt extrusion and 3D printing: Structure and drug release correlation. Carbohydr. Polym. 2017, 177, 49–57. [Google Scholar] [CrossRef]
- Dumpa, N.R.; Bandari, S.; Repka, M.A. Novel Gastroretentive Floating Pulsatile Drug Delivery System Produced via Hot-Melt Extrusion and Fused Deposition Modeling 3D Printing. Pharmaceutics 2020, 12, 52. [Google Scholar] [CrossRef]
- Verstraete, G.; Samaro, A.; Grymonpré, W.; Vanhoorne, V.; Van Snick, B.; Boone, M.; Hellemans, T.; Van Hoorebeke, L.; Remon, J.P.; Vervaet, C. 3D printing of high drug loaded dosage forms using thermoplastic polyurethanes. Int. J. Pharm. 2018, 536, 318–325. [Google Scholar] [CrossRef]
- Feuerbach, T.; Callau-Mendoza, S.; Thommes, M. Development of filaments for fused deposition modeling 3D printing with medical grade poly(lactic-co-glycolic acid) copolymers. Pharm. Dev. Technol. 2018, 24, 487–493. [Google Scholar] [CrossRef]
- Genina, N.; Holländer, J.; Jukarainen, H.; Mäkilä, E.M.; Salonen, J.; Sandler, N. Ethylene vinyl acetate (EVA) as a new drug carrier for 3D printed medical drug delivery devices. Eur. J. Pharm. Sci. 2016, 90, 53–63. [Google Scholar] [CrossRef]
- Kohlgrüber, K.; Wiedmann, W. Co-Rotating Twin-Screw Extruders-Fundamentals, Technology, and Applications; Carl Hanser Publisher: Munich, Germany, 2008. [Google Scholar]
- Melocchi, A. 3D printing by fused deposition modeling (FDM) of a swellable/erodible capsular device for oral pulsatile release of drugs. J. Drug Deliv. Sci. Technol. 2015, 30, 360. [Google Scholar] [CrossRef]
- Korte, C. 3D-Drug-Printing: Extrusion of Printable Drug-Loaded Filaments and Development of Novel Solid Dosage Forms. Ph.D. Thesis, Heinrich-Heine University, Duesseldorf, Germany, 2018. [Google Scholar]
- Meier, R.; Moll, K.-P.; Krumme, M.; Kleinebudde, P. Impact of fill-level in twin-screw granulation on critical quality attributes of granules and tablets. Eur. J. Pharm. Biopharm. 2017, 115, 102–112. [Google Scholar] [CrossRef] [PubMed]
- Aho, J.; Bøtker, J.P.; Genina, N.; Edinger, M.; Arnfast, L.; Rantanen, J. Roadmap to 3D-Printed Oral Pharmaceutical Dosage Forms: Feedstock Filament Properties and Characterization for Fused Deposition Modeling. J. Pharm. Sci. 2019, 108, 26–35. [Google Scholar] [CrossRef] [PubMed]
- Repka, M.A.; Langley, N.; DiNunzio, J. Melt Extrusion-Material, Technology and Drug Product Design; Springer: New York, NY, USA, 2013; p. 13. [Google Scholar]
- Forrest, A.R. Antialiasing in Practice. In Fundamental Algorithms for Computer Graphics; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 1985; pp. 113–134. [Google Scholar]
- D’Antona, G.; Ferrero, A. Digital Signal Processing for Measurement Systems: Theory and Applications; Springer: New York, NY, USA, 2006; pp. 115–126. [Google Scholar]
- Sadia, M.; Sośnicka, A.; Arafat, B.; Isreb, A.; Ahmed, W.; Kelarakis, A.; Alhnan, M.A. Adaptation of pharmaceutical excipients to FDM 3D printing for the fabrication of patient-tailored immediate release tablets. Int. J. Pharm. 2016, 513, 659–668. [Google Scholar] [CrossRef]
- Alhijjaj, M.; Nasereddin, J.; Belton, P.S.; Qi, S. Impact of Processing Parameters on the Quality of Pharmaceutical Solid Dosage Forms Produced by Fused Deposition Modeling (FDM). Pharmaceutics 2019, 11, 633. [Google Scholar] [CrossRef] [PubMed]



| Trial | Batch Name | PFR [g/min] | LFR [g/min] | Screw Speed [1/min] | SFL [–] | Haul-off Speed [m/min] |
|---|---|---|---|---|---|---|
| Winder implementation | T1 | 5 | 0.56 | 40 | 0.03 | 2.0 |
| T2 | 10 | 1.11 | 40 | 0.059 | 4.1 | |
| Influence of PFR | T1 | 5 | 0.56 | 40 | 0.03 | 2.0 |
| T3 | 7.5 | 0.83 | 40 | 0.045 | 3.0 | |
| T4 | 10 | 1.11 | 40 | 0.059 | 4.0 | |
| Influence of screw speed | T5 | 5 | 0.56 | 20 | 0.059 | 2.0 |
| T6 | 5 | 0.56 | 30 | 0.04 | 2.0 | |
| T1 | 5 | 0.56 | 40 | 0.03 | 2.0 | |
| T7 | 5 | 0.56 | 50 | 0.024 | 2.0 | |
| T8 | 5 | 0.56 | 60 | 0.02 | 2.0 | |
| Influence of SFL | T5 | 5 | 0.56 | 20 | 0.059 | 2.0 |
| T8 | 5 | 0.56 | 60 | 0.02 | 2.0 | |
| T4 | 10 | 1.11 | 40 | 0.059 | 4.0 | |
| T9 | 10 | 1.11 | 120 | 0.02 | 4.0 |
| Diameter | T1 | T2 | T3 | T4 | T5 | T6 | T7 | T8 | T9 | PLA |
|---|---|---|---|---|---|---|---|---|---|---|
| Mean [mm] | 1.781 | 1.798 | 1.779 | 1.778 | 1.782 | 1.789 | 1.776 | 1.777 | 1.821 | 1.756 |
| s [mm] | 0.032 | 0.031 | 0.035 | 0.033 | 0.019 | 0.032 | 0.054 | 0.064 | 0.062 | 0.004 |
| CoV [%] | 1.80 | 1.72 | 1.98 | 1.83 | 1.07 | 1.76 | 3.06 | 3.63 | 3.38 | 0.21 |
| Sample | Mass of Printed Dosage Form [mg] (Mean ± s, n = 20), | Ph.Eur. 2.9.5 | Mechanical Properties | ||
|---|---|---|---|---|---|
| n > 5% | n > 10% | YM [N/mm2] Mean ± CI (α = 0.05), n = 6 | Distance at Break [mm], Mean ± CI, (α = 0.05), n = 6 | ||
| PLA | 350 ± 1.5 | - | - | ND | ND |
| T5, 20 rpm | 283.8 ± 9.3 | 1 | - | 484.7 ± 9.4 | 5.408 ± 0.151 |
| T6, 30 rpm | 291.4 ± 9.2 | 1 | - | 496.5 ± 6.5 | 5.433 ± 0.295 |
| T1, 40 rpm | 306.1 ± 11.5 | 3 | 1 | 496.0 ± 9.7 | 5.225 ± 0.261 |
| T7, 50 rpm | 311.9 ± 17.7 | 7 | 1 | 505.5 ± 8.7 | 5.408 ± 0.600 |
| T8, 60 rpm | 307.5 ± 21.4 | 8 | 3 | 515.5 ± 11.4 | 5.700 ± 0.892 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ponsar, H.; Wiedey, R.; Quodbach, J. Hot-Melt Extrusion Process Fluctuations and Their Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms. Pharmaceutics 2020, 12, 511. https://doi.org/10.3390/pharmaceutics12060511
Ponsar H, Wiedey R, Quodbach J. Hot-Melt Extrusion Process Fluctuations and Their Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms. Pharmaceutics. 2020; 12(6):511. https://doi.org/10.3390/pharmaceutics12060511
Chicago/Turabian StylePonsar, Hanna, Raphael Wiedey, and Julian Quodbach. 2020. "Hot-Melt Extrusion Process Fluctuations and Their Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms" Pharmaceutics 12, no. 6: 511. https://doi.org/10.3390/pharmaceutics12060511
APA StylePonsar, H., Wiedey, R., & Quodbach, J. (2020). Hot-Melt Extrusion Process Fluctuations and Their Impact on Critical Quality Attributes of Filaments and 3D-Printed Dosage Forms. Pharmaceutics, 12(6), 511. https://doi.org/10.3390/pharmaceutics12060511