Influence of Acetylated Annealed Starch on the Release of β-Escin from the Anionic and Non-Ionic Hydrophilic Gels
Abstract
1. Introduction
2. Materials and Methods
2.1. Reagents
2.2. Hydrogel Preparation
2.3. Starch Preparation
2.4. Kinetics Study
3. Results
3.1. Kinetics
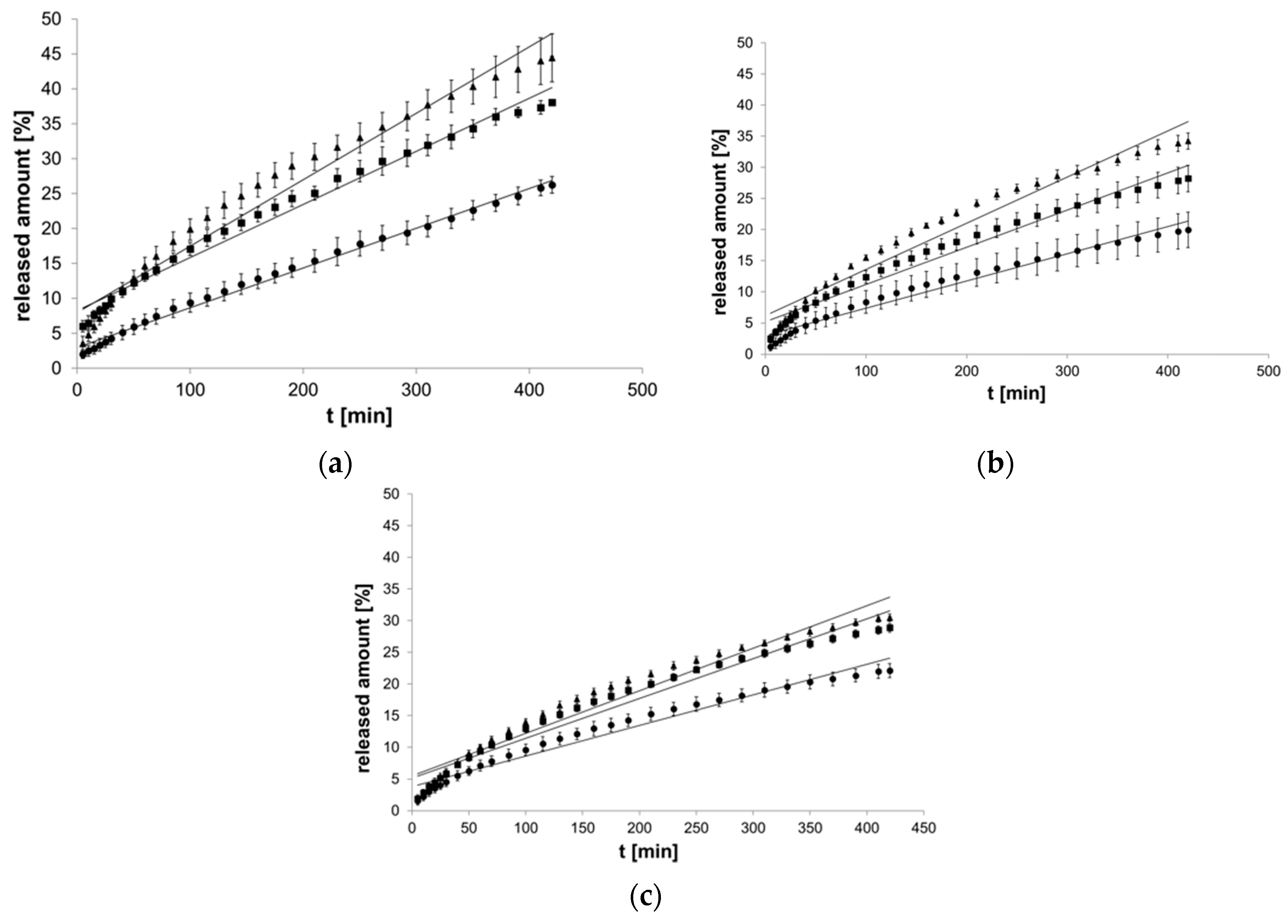
3.1.1. Zero-Order Kinetics Model
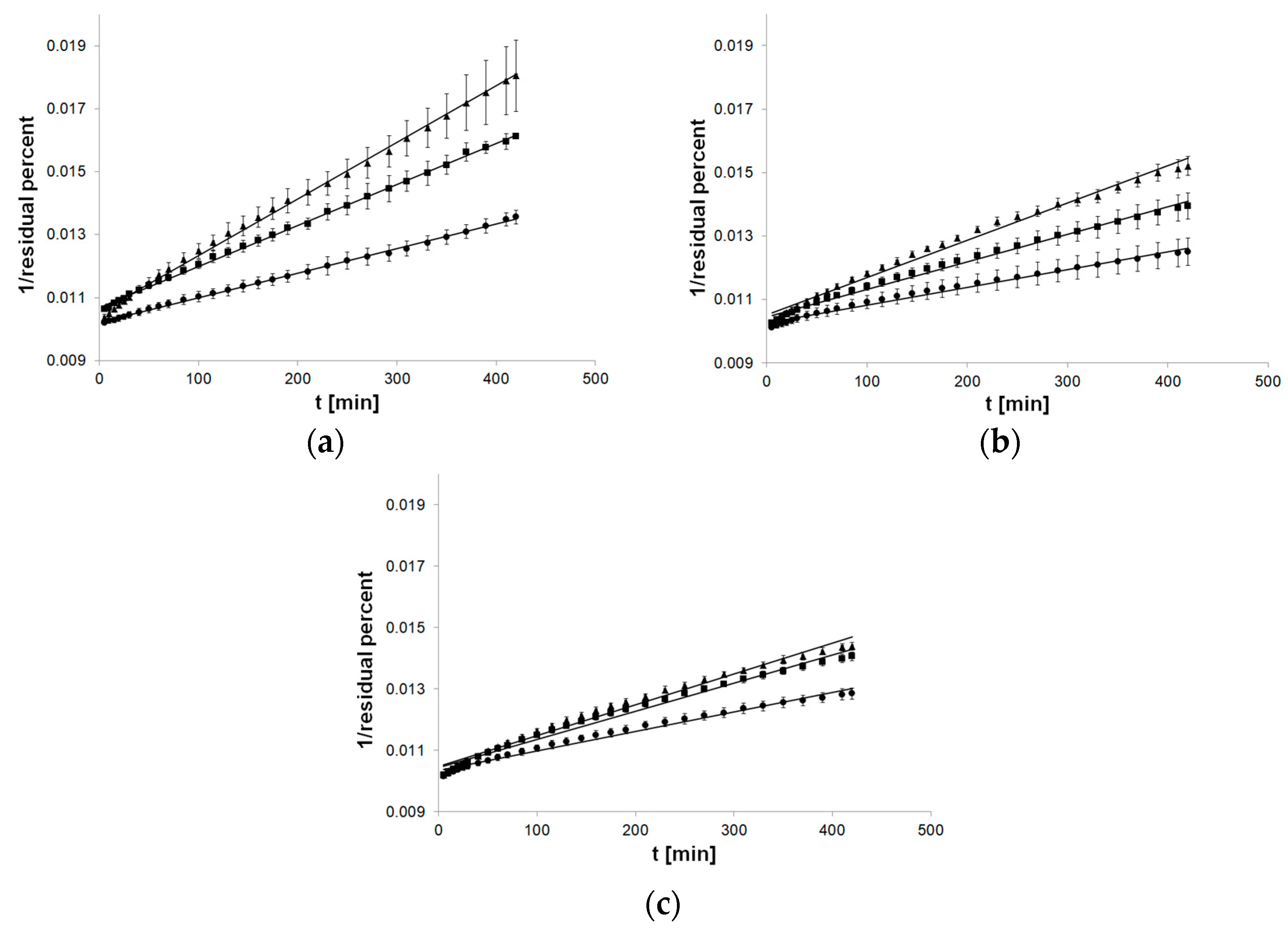
3.1.2. First-Order Kinetics Model
3.1.3. Second-Order Kinetics Model
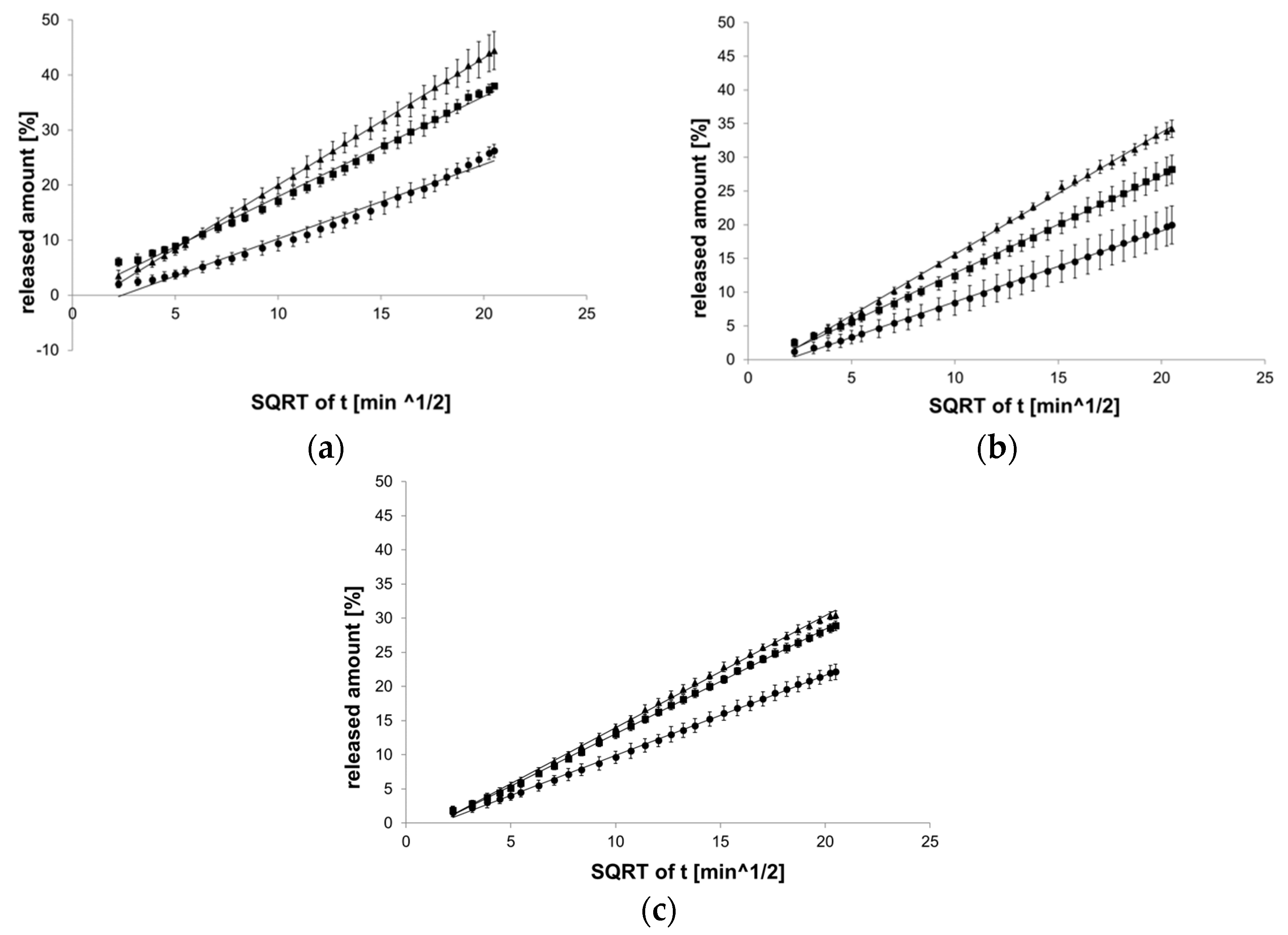
3.1.4. The Higuchi Model
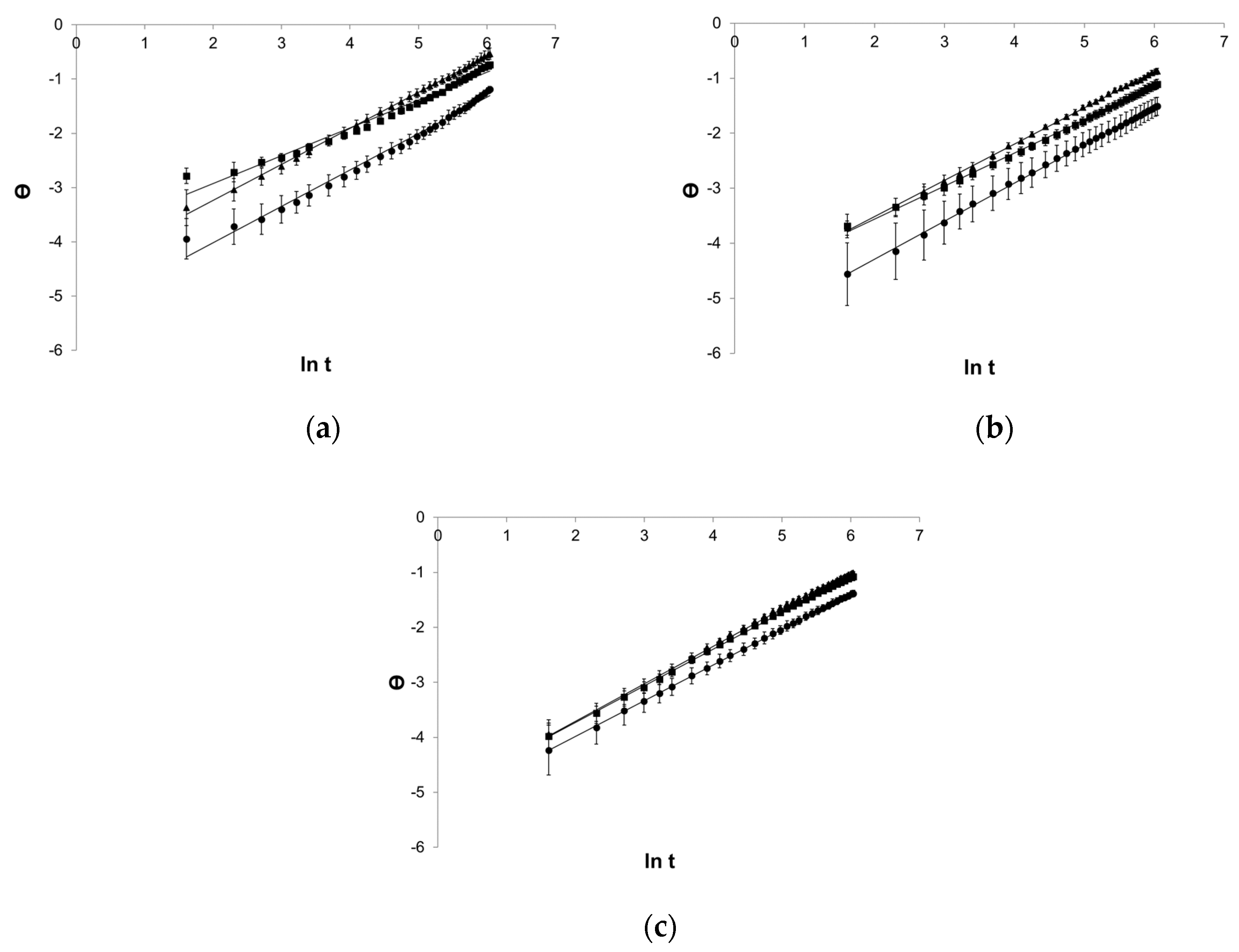
3.1.5. The Weibull Model
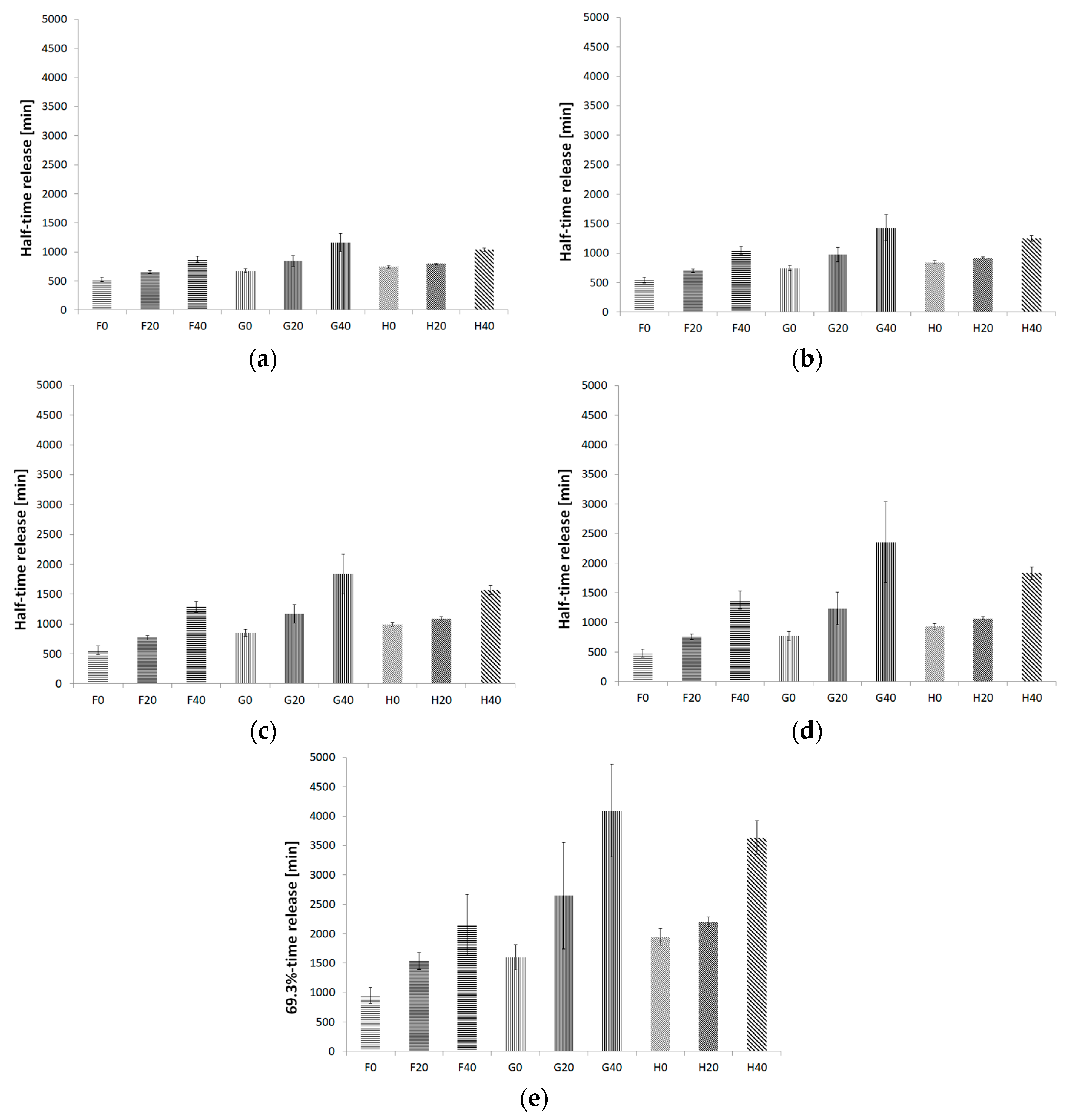
3.1.6. Kinetics Models Parameters
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Morrison, W.R.; Karkalas, J.S. Methods in Plant Biochemistry; Elsevier B.V., Ed.; Academic Press: New York, NY, USA, 1990; Volume 2, pp. 323–352. [Google Scholar]
- Gross, R.A.; Kalra, B. Biodegradable polymers for the environment. Science 2002, 297, 803–807. [Google Scholar] [CrossRef] [PubMed]
- Kapelko-Żeberska, M.; Zięba, T.; Singh, A.V. Physically and Chemically Modified Starches in Food and Non-Food Industries. In Surface Modification of Biopolymers, 1st ed.; Thakur, V.K., Singha, A.S., Eds.; John Wiley and Sons: Hoboken, NJ, USA, 2015; pp. 173–193. [Google Scholar]
- Rocha, T.S.; Felizardo, S.G.; Jane, J.; Franco, C.M.L. Effect of annealing on the semicrystalline structure of normal and waxy corn starches. Food Hydrocoll. 2012, 29, 93–99. [Google Scholar] [CrossRef]
- Piecyk, M.; Konarzewska, M.; Sitakiewicz, I. Effect of hydrothermal modification of annealing type on some selected properties of starch pea (Pisum sativum). Zywnosc Nauka Technologia Jakosc 2009, 16, 58–71. [Google Scholar]
- Tester, R.F.; Karkalas, J.; Qi, X. Starch structure and digestibility Enzyme-Substrate relationship. World Poult. Sci. J. 2004, 60, 186–195. [Google Scholar] [CrossRef]
- Chen, J.-F. Granular Cold-Water-Soluble Starch: Preparation, Characterization, and Its Use on Controlled Release of Atrazine. Ph.D. Thesis, Iowa State University, Ames, IA, USA, 1993. [Google Scholar]
- Sirtori, C.R. Aescin: Pharmacology, pharmacokinetics and therapeutic profile. Pharmacol. Res. 2001, 44, 183–193. [Google Scholar] [CrossRef] [PubMed]
- Kobryń, J.; Sowa, S.; Gasztych, M.; Dryś, A.; Musiał, W. Influence of Hydrophilic Polymers on the β Factor in Weibull Equation Applied to the Release Kinetics of a Biologically Active Complex of Aesculus hippocastanum. Int. J. Polym. Sci. 2017, 2017, 3486384. [Google Scholar] [CrossRef]
- Lisik, A.; Wójcik-Pastuszka, D.; Twarda, M.; Berkowski, R.; Musiał, W. Effect of standard and reversible arrangements of Ph.Eur./USP extraction cells during dissolution tests of calcium dobesilate in hydrogel formulation. Curr. Issues Pharm. Med. Sci. 2015, 28, 136–141. [Google Scholar] [CrossRef]
- Wójcik-Pastuszka, D.; Lisik, A.; Twarda, M.; Berkowski, R.; Musiał, W. The influence of hydrophylic polymers on the release rate of calcium dobesilate in hydrogel formulation assessed in vitro using porcine ear skin. Curr. Issues Pharm. Med. Sci. 2015, 28, 225–230. [Google Scholar] [CrossRef]
- Reddy, D.N. Design, development and characterization of clopidogrel bisulfate transdermal drug delivery system. Asian J. Pharm. Clin. Res. 2015, 8, 2. [Google Scholar]
- Hamishehkar, H.; Khoshbakht, M.; Jouyban, A.; Ghanbarzadeh, S. The Relationship between Solubility and Transdermal Absorption of Tadalafil. Adv. Pharm. Bull. 2015, 5, 411–417. [Google Scholar] [CrossRef][Green Version]
- Sanjivkumar, B.; Rajkumar, D.; Mallikarjun, P.; Karankumar, B.; Sreenivasa Rao, K. Development and method validation of Aesculus hippocastanum extract. Int. Res. J. Pharm. 2012, 3, 324–328. [Google Scholar]
- Dash, S.; Murthy, P.; Nath, L.; Chowdhury, P. Kinetic modeling on drug release from controlled drug delivery systems. Acta Pol. Pharm. 2010, 67, 217–223. [Google Scholar] [PubMed]
- Siepmann, J.; Siepmann, F. Modeling of diffusion controlled drug delivery. J. Control. Release 2012, 161, 351–362. [Google Scholar] [CrossRef] [PubMed]
- Costa, P.; Sousa Lobo, J.M. Modeling and comparison of dissolution profiles. Eur. J. Pharm. Sci. 2001, 13, 123–133. [Google Scholar] [CrossRef]
- Ramteke, K.H.; Dighe, P.A.; Kharat, A.R.; Patil, S.V. Mathematical Models of Drug Dissolution: A Review. Sch. Acad. J. Pharm. 2014, 3, 388–396. [Google Scholar]
- Lee, S.L.; Raw, A.S.; Yu, L. Dissolution Testing, in Biopharmaceutics Applications in Drug Development; Springer: New York, NY, USA, 2008; Volume 3, pp. 49–50. [Google Scholar]
- Silva, N.M.E.N. Modelling and Simulation in Bioequivalence. Ph.D. Thesis, University of Lisbon, Lisbon, Portugal, 2012; pp. 58, 74. [Google Scholar]
- Nasatto, P.L.; Pignon, F.; Silveira, J.L.M.; Duarte, M.E.R.; Noseda, M.D.; Rinaudo, M. Methylcellulose, a Cellulose Derivative with Original Physical Properties and Extended Applications. Polymers 2015, 7, 777–803. [Google Scholar] [CrossRef]
- Sarkar, N. Structural interpretation of the interfacial properties of aqueous solutions of methylcellulose and hydroxypropyl methylcellulose. Polymer 1984, 25, 481–486. [Google Scholar] [CrossRef]
- Milanović, M.; Krstonošić, V.; Dokic, L.; Hadnađev, M. Insight into the Interaction Between Carbopol® 940 and Ionic/Nonionic Surfactant. J. Surfactants Deterg. 2015, 18, 505–516. [Google Scholar] [CrossRef]
- Navarro, R.E.; Aguilera-Márquez, D.; Virués, C.; Inoue. Hydrogen bonding between carboxylic acids and amide-based macrocycles in their host–guest complexes. Supramol. Chem. 2008, 20, 737–742. [Google Scholar] [CrossRef]
- Zięba, T.; Gryszkin, A.; Kapelko, M. Selected properties of acetylated adipate of retrograded starch. Carbohydr. Polym. 2014, 99, 687–691. [Google Scholar] [CrossRef]
- El Halal, S.L.; Colussi, R.; Pinto, V.Z.; Bartz, J.; Radunz, M.; Carreño, N.L.; Dias, A.R.; Zavareze, E.R. Structure, morphology and functionality of acetylated and oxidised barley starches. Food Chem. 2015, 168, 247–256. [Google Scholar] [CrossRef] [PubMed]
- Palafox, M.A.; Núñez, M.G. Theoretical quantum chemical study of benzoic acid: Geometrical parameters and vibrational wavenumbers. Int. J. Quantum Chem. 2002, 89, 1–24. [Google Scholar] [CrossRef]
- Brady, J.E.; Dürig, T.; Lee, P.I.; Li, J.-X. Polymer Properties and Characterization. In Developing Solid Oral Dosage Forms; Elsevier: Amsterdam, The Netherlands, 2017; pp. 181–223. [Google Scholar]
- Gandolfi, L.; Galleguillos, R. Part 4.2.2. Rheology Modifiers and Consumer Perception. In Harry’s Cosmeticology, 9th ed.; Rosen, M.R., Ed.; Chemical Publishing: New York, NY, USA, 2015; Volume 2, pp. 768–806. [Google Scholar]
- Muzzarelli, R.A.A. Genipin-crosslinked chitosan hydrogels as biomedical and pharmaceutical aids. Carbohydr. Polym. 2009, 77, 1–9. [Google Scholar] [CrossRef]
- Berger, J.; Reist, M.; Mayer, J.M.; Felt, O.; Peppas, N.A.; Gurny, R. Structure and interactions in covalently and ionically crosslinked chitosan hydrogels for biomedical applications. Eur. J. Pharm. Biopharm. 2004, 57, 19–34. [Google Scholar] [CrossRef]
- Mahdavinia, G.R.; Pourjavadi, A.; Hosseinzadeh, H. Modified chitosan 4. Superabsorbent hydrogels from poly (acrylic acid-co-acrylamide) grafted chitosan with salt- and pH-responsiveness properties. Eur. Polym. J. 2004, 40, 1399–1407. [Google Scholar] [CrossRef]
- Papadopoulou, V.; Kosmidis, K.; Vlachou, M.; Macheras, P. On the use of the Weibull function for the discernment of drug release mechanisms. Int. J. Pharm. 2006, 309, 44–50. [Google Scholar] [CrossRef]
- Ritger, P.L.; Peppas, N.A. A simple equation for description of solute release II. Fickian and anomalous release from swellable devices. J. Control. Release 1987, 5, 37–42. [Google Scholar] [CrossRef]
- Siepmann, J.; Peppas, N.A. Modeling of drug release from delivery systems based on hydroxypropyl methylcellulose (HPMC). Adv. Drug Deliv. Rev. 2001, 48, 139–157. [Google Scholar] [CrossRef]
- Peppas, N.; Mikos, A.G. Preparation methods and structure of hydrogels. In Hydrogels in Med and Pharm; CRC Press Inc.: Boca Raton, FL, USA, 1986; pp. 1–26. [Google Scholar]
- Hopkinson, I.; Jones, R.A.L.; Black, S.; Lane, D.M.; McDonald, P.J. Fickian and Case II diffusion of water into amylose: A stray field NMR study. Carbohydr. Polym. 1997, 34, 39–47. [Google Scholar] [CrossRef]
- Gümüşderelioğlu, M.; Kesgin, D. Release kinetics of bovine serum albumin from pH-sensitive poly (vinyl ether) based hydrogels. Int. J. Pharm. 2005, 288, 273–279. [Google Scholar] [CrossRef]
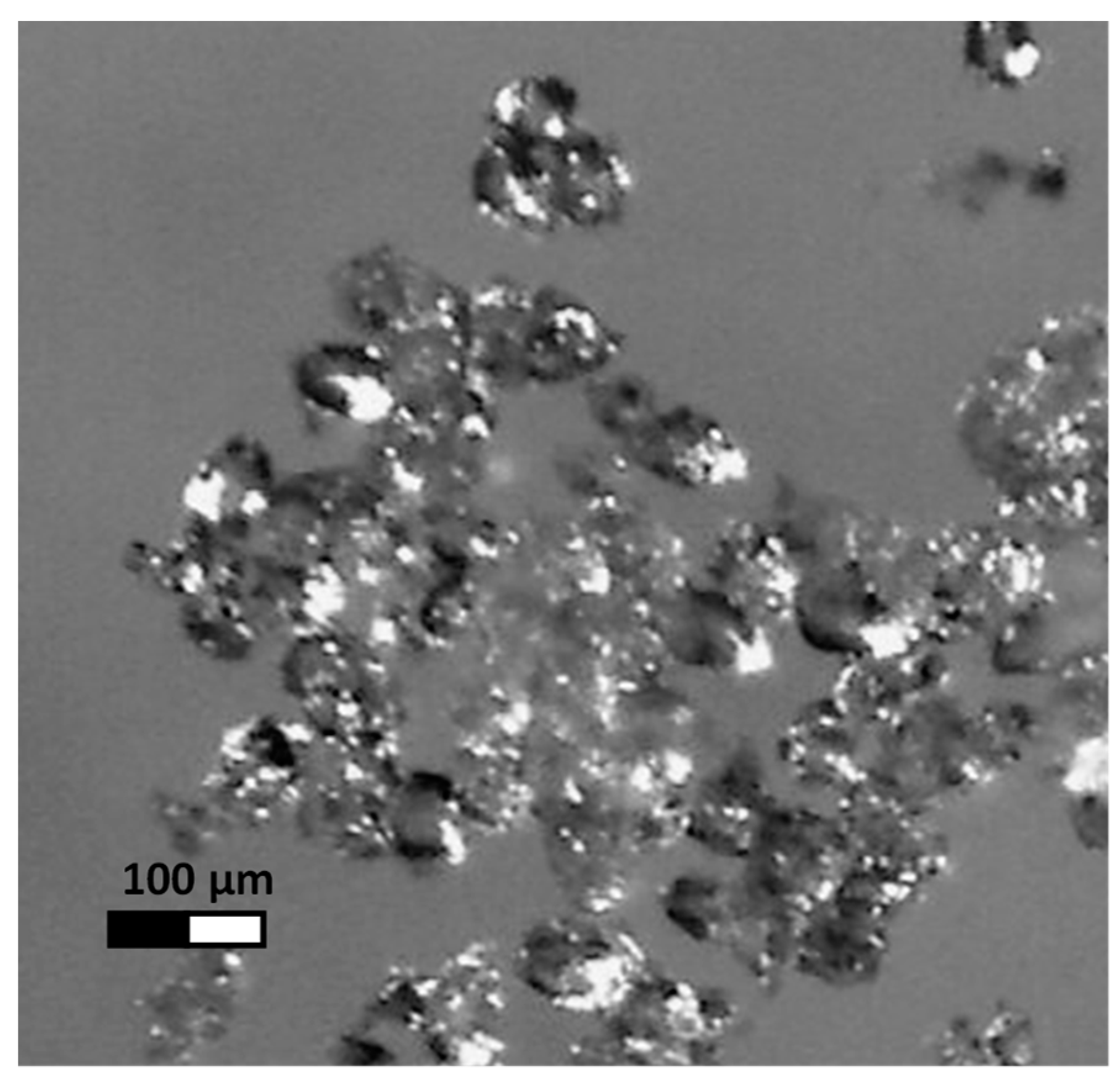




| Formulation | Composition (g) | |||||
|---|---|---|---|---|---|---|
| EH | MC | PA1 | PC-11 | MSA | H2O | |
| F0 * | 7.08 | 1.2 | - | - | - | 51.72 |
| F20 | 7.08 | 1.2 | - | - | 12.0 | 39.72 |
| F40 | 7.08 | 1.2 | - | - | 24.0 | 27.72 |
| G0 * | 7.08 | - | 0.9 | - | - | 52.02 |
| G20 | 7.08 | - | 0.9 | - | 12.0 | 40.02 |
| G40 | 7.08 | - | 0.9 | - | 24.0 | 28.02 |
| H0 * | 7.08 | - | - | 0.9 | - | 52.02 |
| H20 | 7.08 | - | - | 0.9 | 12.0 | 40.02 |
| H40 | 7.08 | - | - | 0.9 | 24.0 | 28.02 |
| Models Applied | General Equation | Parameters | |
|---|---|---|---|
| Zero-order | |||
| First-order | |||
| Second-order | |||
| Higuchi | |||
| Weibull | |||
| P: | Mathematical Model Dependent Methods | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0-Order | 1st-Order | 2nd-Order | Higuchi | Weibull | BF | |||||||
| K(0) (%·min−1) | SD | K(I) (min−1) | SD | K(II) (%−1·min−1) | SD | K(H) (%·min−1/2) | SD | β (–) | SD | |||
| F: | F0 (MC formulation) | |||||||||||
| C: | 9.0 × 10−2 | 6.59 × 10−3 | −1.29 × 10−3 | 1.23 × 10−4 | 1.80 × 10−5 | 2.26 × 10−6 | 2.31 | 1.56 × 10−1 | 6.76 × 10−1 | 3.01 × 10−2 | H | |
| r2 | 0.9638 | - | 0.9849 | - | 0.9950 | - | 0.9980 | - | 0.9981 | - | W | |
| F: | F20 (MC formulation) | |||||||||||
| C: | 7.59 × 10−2 | 2.45 × 10−3 | −9.89 × 10−4 | 3.98 × 10−5 | 1.30 × 10−5 | 6.40 × 10−7 | 1.82 | 5.85 × 10−2 | 5.98 × 10−1 | 2.61 × 10−2 | II | |
| r2 | 0.9798 | - | 0.9907 | - | 0.9954 | - | 0.9920 | - | 0.9889 | - | H | |
| F: | F40 (MC formulation) | |||||||||||
| C: | 5.70 × 10−2 | 3.33 × 10−3 | −6.6 × 10−4 | 4.30 × 10−5 | 7.81 × 10−6 | 5.67 × 10−7 | 1.35 | 7.88 × 10−2 | 7.69 × 10−1 | 9.23 × 10−2 | II | |
| r2 | 0.9899 | - | 0.9938 | - | 0.9948 | - | 0.987 | - | 0.9947 | - | W | |
| F: | G0 (PA1 formulation) | |||||||||||
| K: | 7.42 × 10−2 | 3.89 × 10−3 | −9.30 × 10−4 | 5.38 × 10−5 | 1.18 × 10−5 | 7.58 × 10−7 | 1.81 | 8.94 × 10−2 | 6.47 × 10−1 | 3.75 × 10−2 | W | |
| r2 | 0.9571 | - | 0.9749 | - | 0.9878 | - | 0.9900 | - | 0.9984 | - | H | |
| F: | G20 (PA1 formulation) | |||||||||||
| R: | 5.9710−2 | 6.52 × 10−3 | −7.17 × 10−4 | 8.65 × 10−5 | 8.65 × 10−6 | 1.16 × 10−6 | 1.44 | 1.58 × 10−1 | 6.26 × 10−1 | 5.78 × 10−2 | W | |
| r2 | 0.9708 | - | 0.9827 | - | 0.9913 | - | 0.9900 | - | 0.9991 | - | II | |
| F: | G40 (PA1 formulation) | |||||||||||
| C: | 4.37 × 10−2 | 5.37 × 10-3− | −4.93 × 10−4 | 6.94 × 10−5 | 5.59 × 10−6 | 8.90 × 10−7 | 1.05 | 1.33 × 10−1 | 6.69 × 10−1 | 6.60 × 10−2 | W | |
| r2 | 0.9730 | - | 0.9813 | - | 0.9880 | - | 0.9940 | - | 0.9993 | - | H | |
| F: | H0 (PC-11 formulation) | |||||||||||
| C: | 6.72 × 10−2 | 1.73 × 10−3 | −8.19 × 10−4 | 2.27 × 10−5 | 1.00 × 10−5 | 3.11 × 10−7 | 1.64 | 4.26 × 10−2 | 6.43 × 10−1 | 2.46 × 10−2 | W | |
| r2 | 0.9522 | - | 0.9694 | - | 0.9829 | - | 0.9930 | - | 0.9979 | - | H | |
| F: | H20 (PC-11 formulation) | |||||||||||
| C: | 6.29 × 10−2 | 8.43 × 10−4 | −7.57 × 10−4 | 1.37 × 10−5 | 9.15 × 10−6 | 2.21 × 10−7 | 1.53 | 2.16 × 10−2 | 6.41 × 10−1 | 2.07 × 10−2 | W | |
| r2 | 0.9594 | - | 0.9743 | - | 0.9859 | - | 0.9920 | - | 0.9992 | - | H | |
| F: | H40 (PC-11 formulation) | |||||||||||
| C: | 4.83 × 10−2 | 1.31 × 10−3 | −5.54 × 10−4 | 1.98 × 10−5 | 6.38 × 10−6 | 2.87 × 10−7 | 1.17 | 3.23 × 10−2 | 6.37 × 10−1 | 3.82 × 10−2 | W | |
| r2 | 0.9665 | - | 0.9765 | - | 0.9847 | - | 0.9930 | - | 0.9990 | - | H | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kobryń, J.; Zięba, T.; Sowa, S.K.; Musiał, W. Influence of Acetylated Annealed Starch on the Release of β-Escin from the Anionic and Non-Ionic Hydrophilic Gels. Pharmaceutics 2020, 12, 84. https://doi.org/10.3390/pharmaceutics12010084
Kobryń J, Zięba T, Sowa SK, Musiał W. Influence of Acetylated Annealed Starch on the Release of β-Escin from the Anionic and Non-Ionic Hydrophilic Gels. Pharmaceutics. 2020; 12(1):84. https://doi.org/10.3390/pharmaceutics12010084
Chicago/Turabian StyleKobryń, Justyna, Tomasz Zięba, Sandra Karolina Sowa, and Witold Musiał. 2020. "Influence of Acetylated Annealed Starch on the Release of β-Escin from the Anionic and Non-Ionic Hydrophilic Gels" Pharmaceutics 12, no. 1: 84. https://doi.org/10.3390/pharmaceutics12010084
APA StyleKobryń, J., Zięba, T., Sowa, S. K., & Musiał, W. (2020). Influence of Acetylated Annealed Starch on the Release of β-Escin from the Anionic and Non-Ionic Hydrophilic Gels. Pharmaceutics, 12(1), 84. https://doi.org/10.3390/pharmaceutics12010084






