Surface Crack Occurrence and Resistance During Moisture Content Changes in MF-Resin-Impregnated Paper-Decorated Blockboard
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
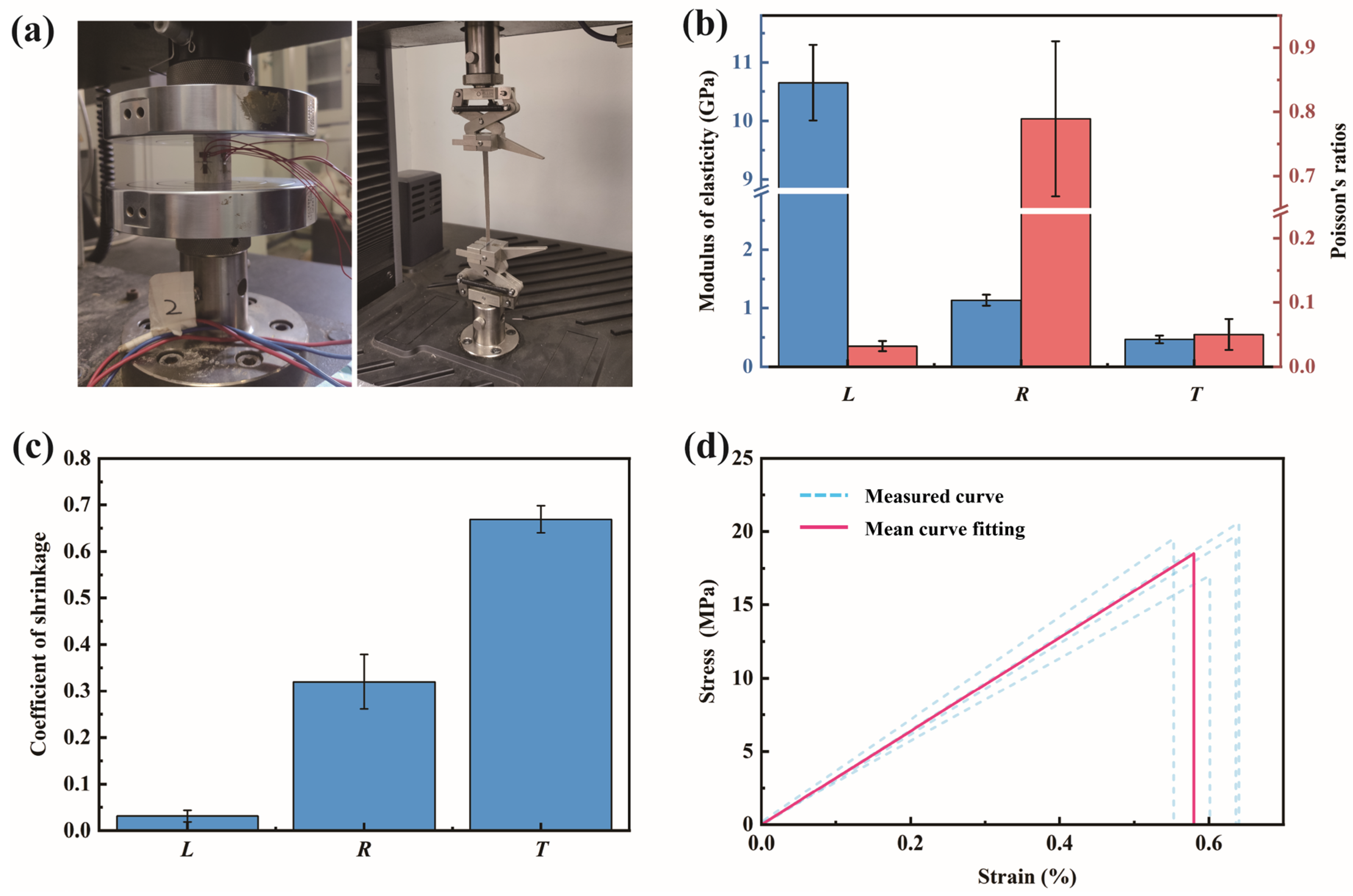
2.2.1. Coefficient of Shrinkage and Elasticity
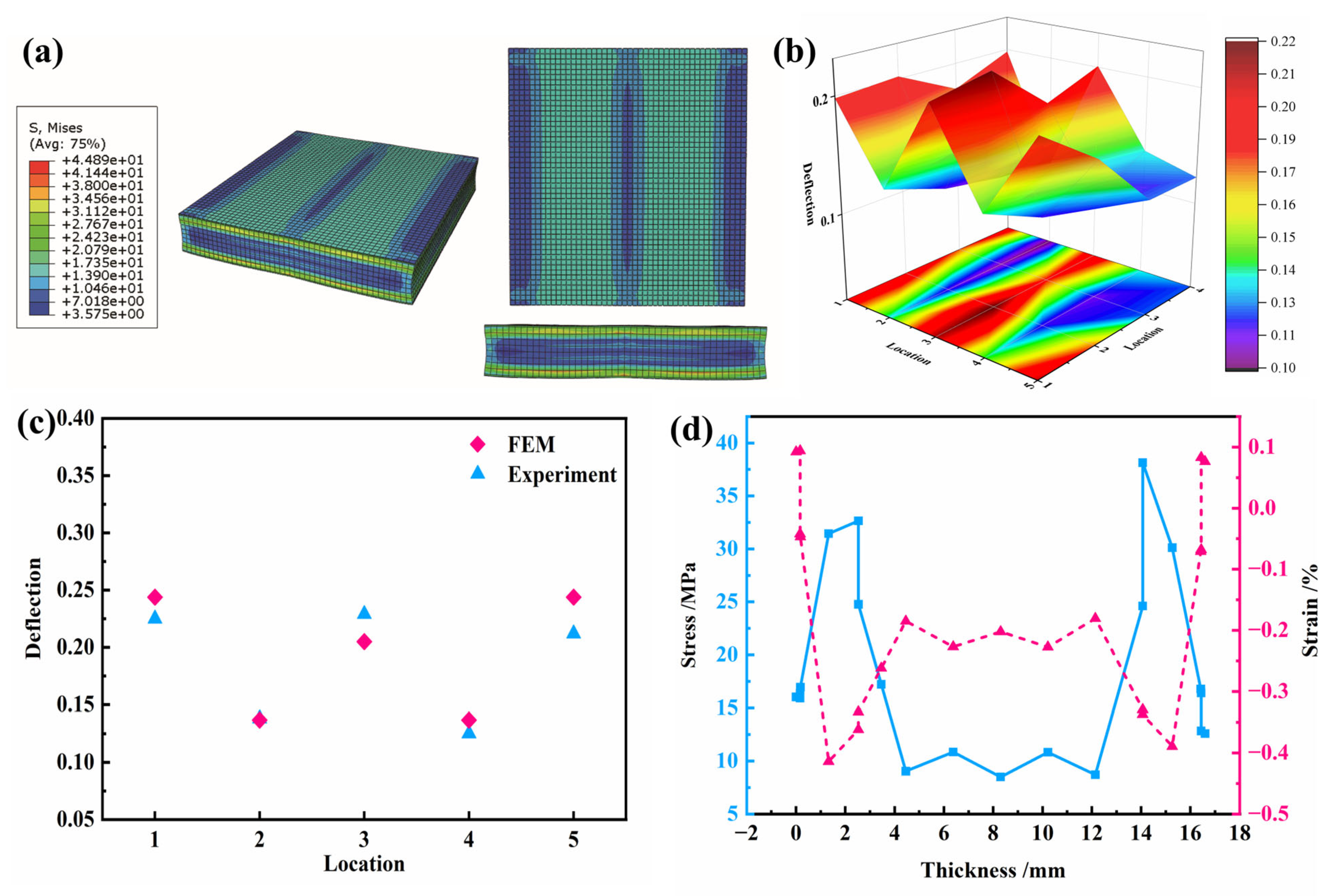
2.2.2. Deformity of Decorated Blockboards
2.2.3. Finite Element Model
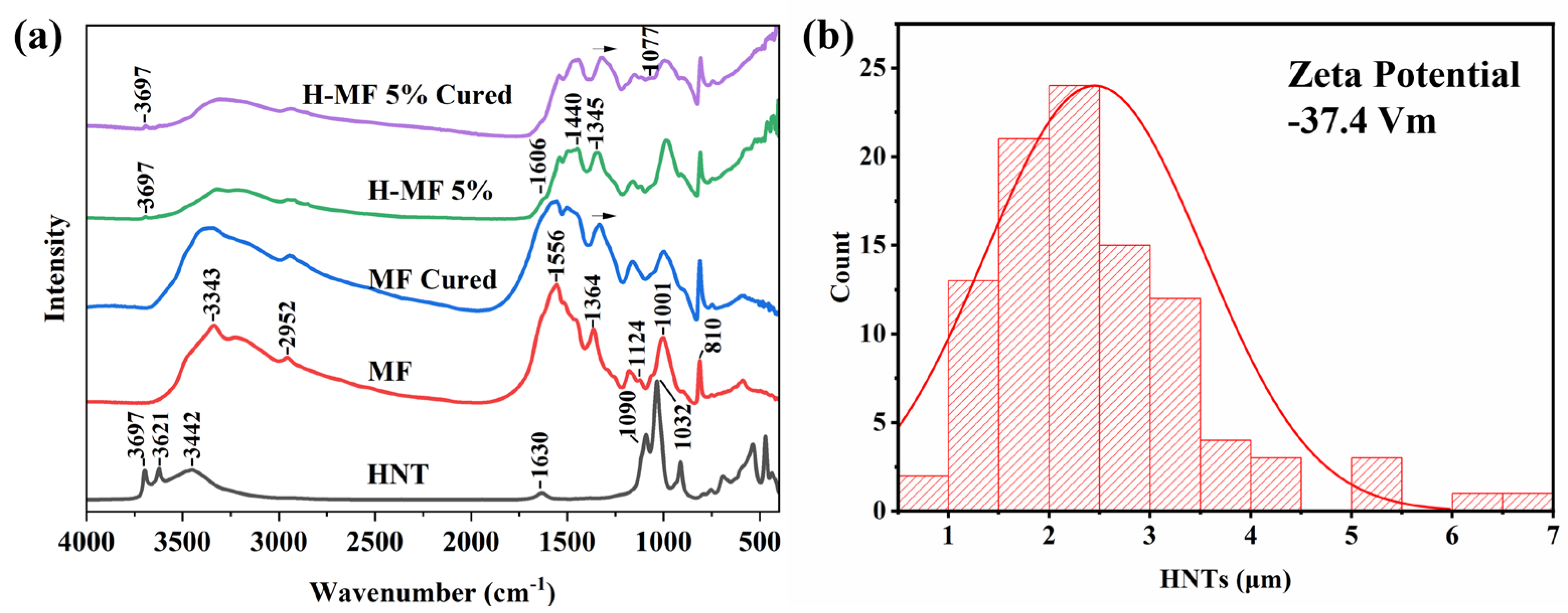
2.2.4. Preparation of the MF Resin with HNTs
2.2.5. Characterization of H-MF Resin
2.2.6. Cracking Resistance Test of the H-MF-Resin-Decorated Blockboard
3. Results and Discussion
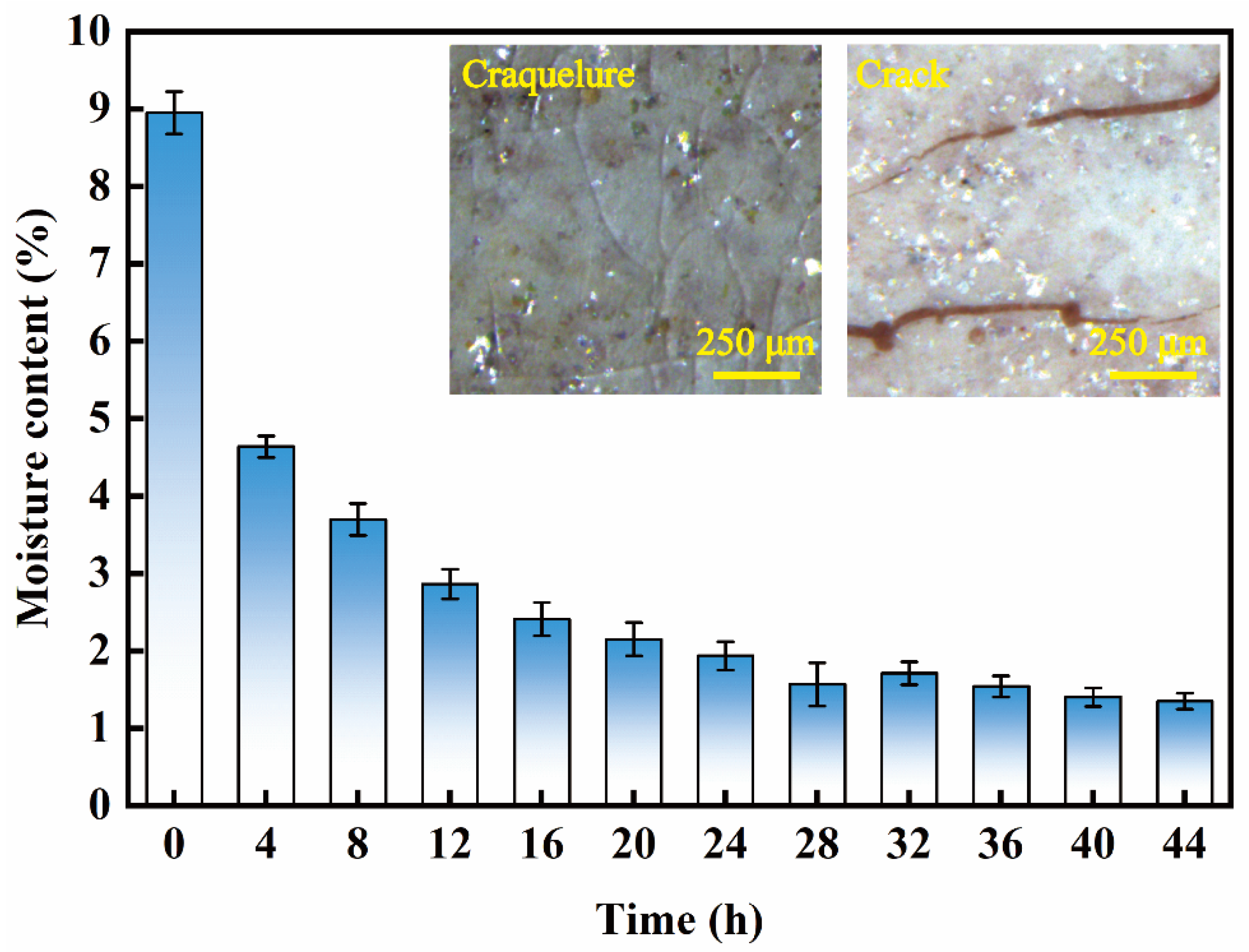
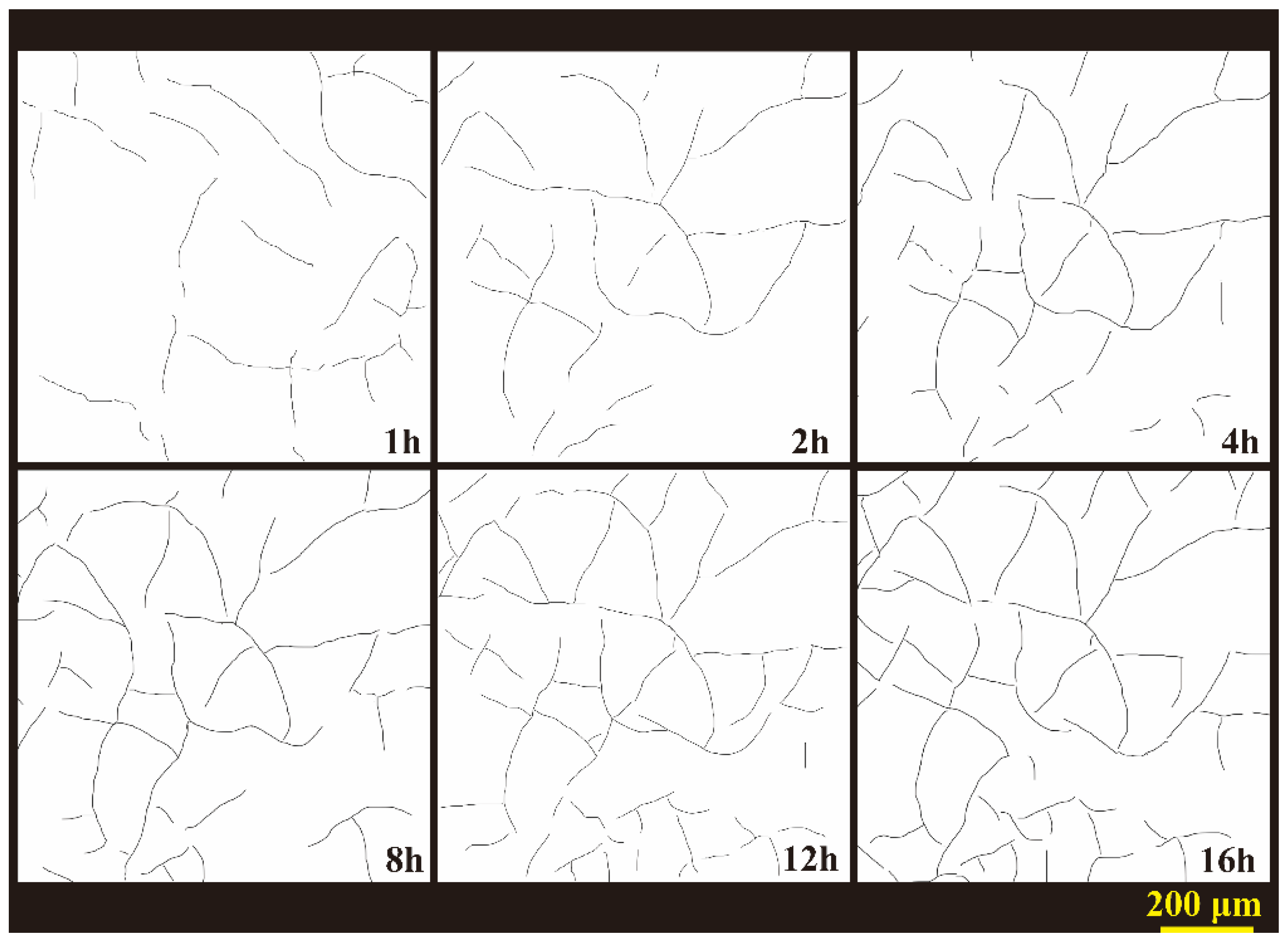
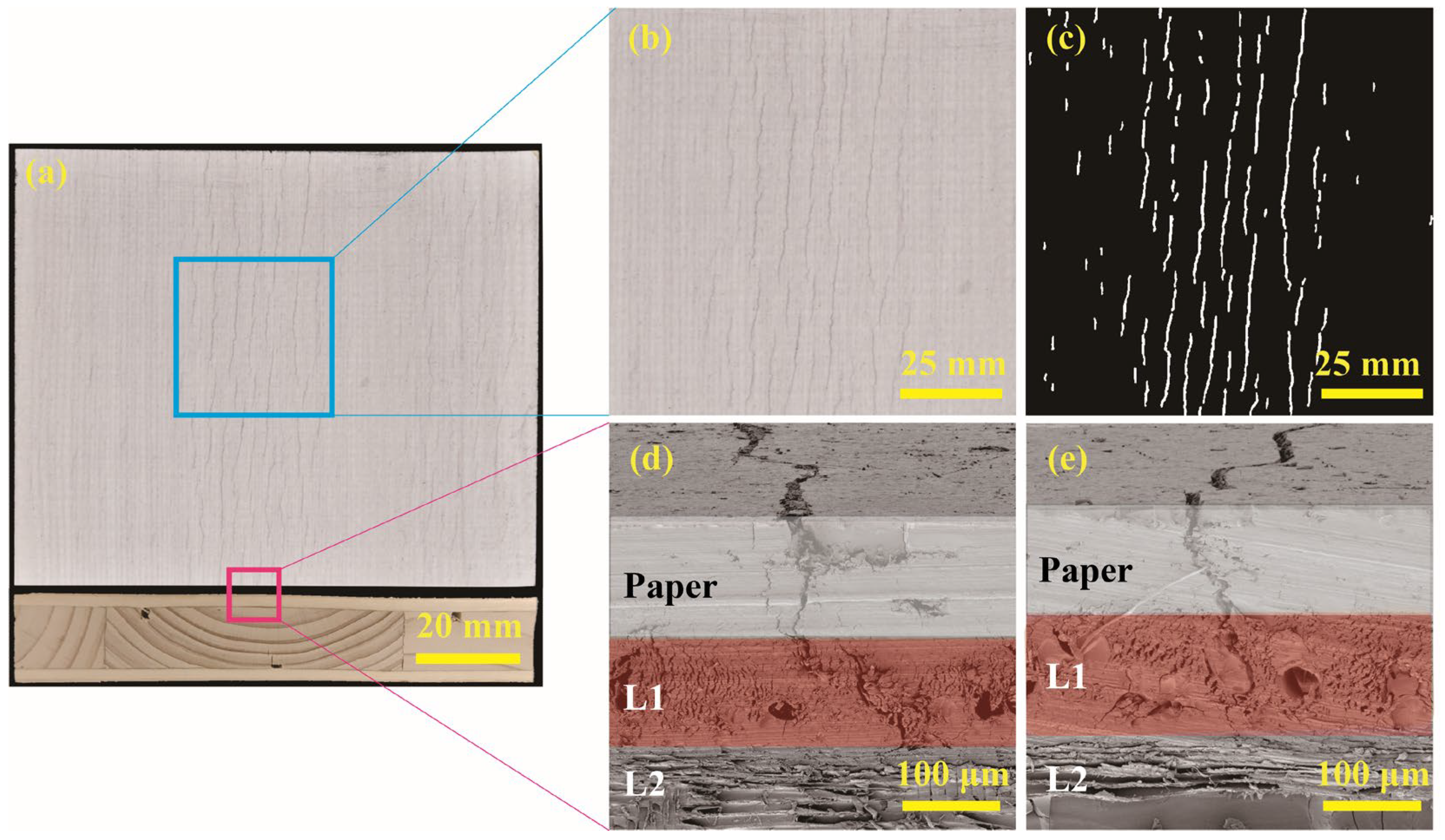
3.1. Cracking Characteristics of the Decorated Blockboard
3.2. Mechanical Properties of the Components
3.3. Finite Element Analysis
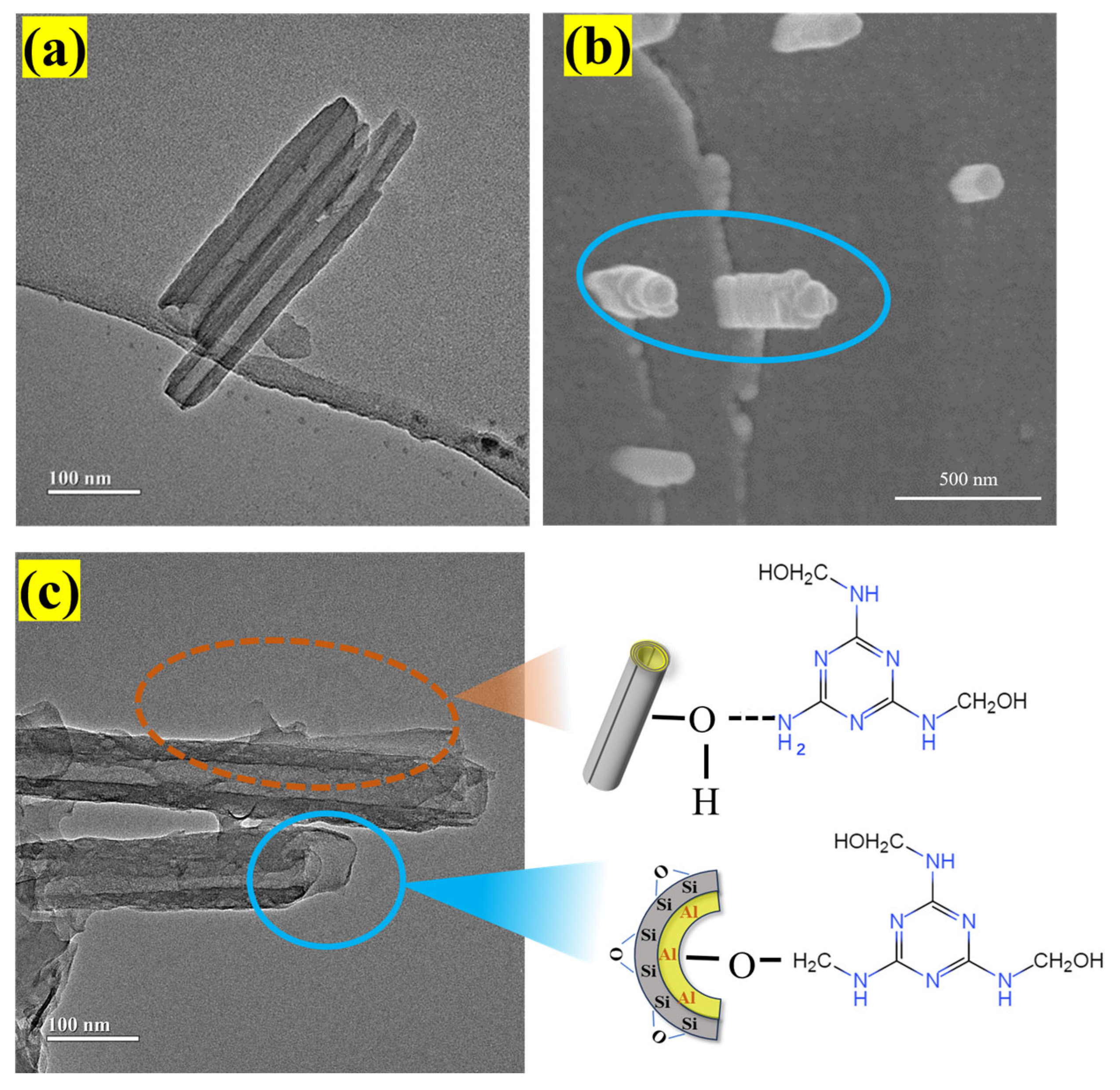
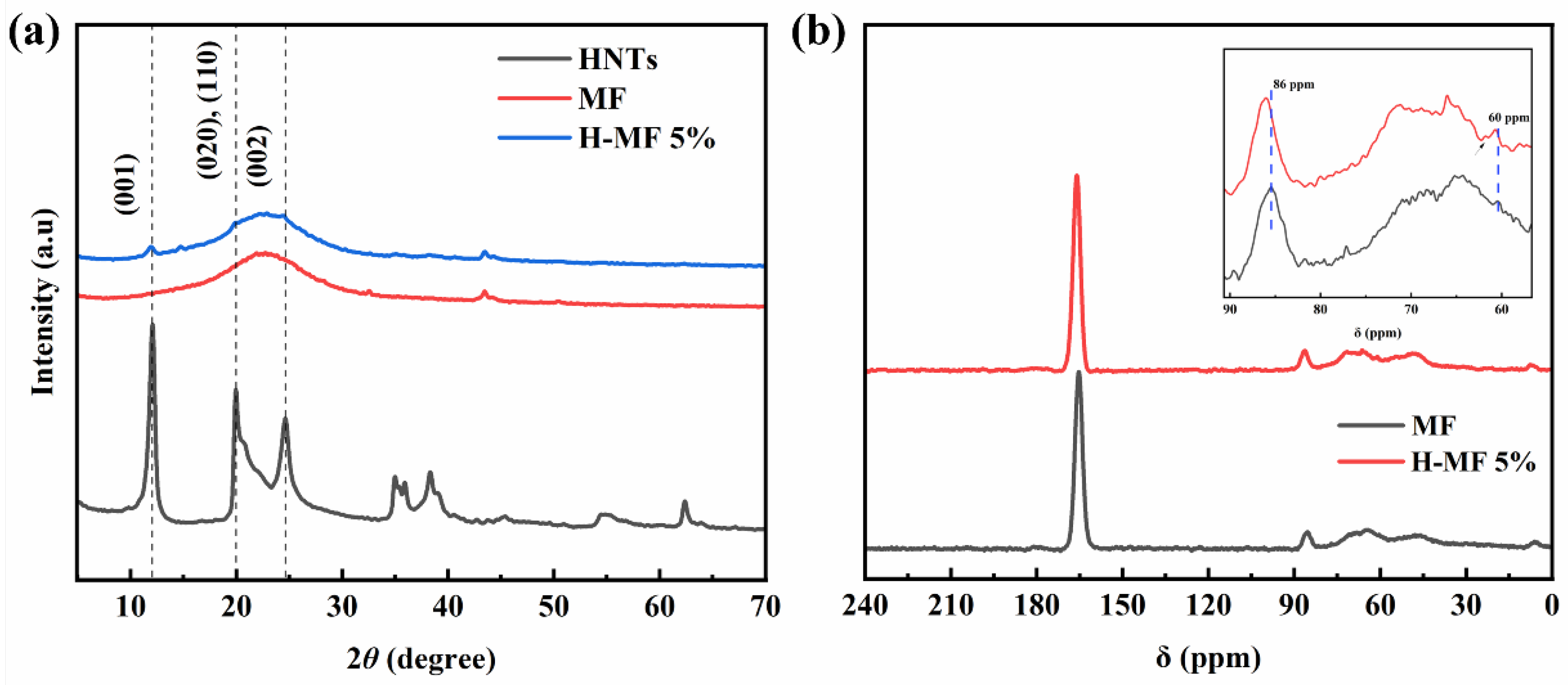
3.4. The Structure and Morphology of the H-MF Resin
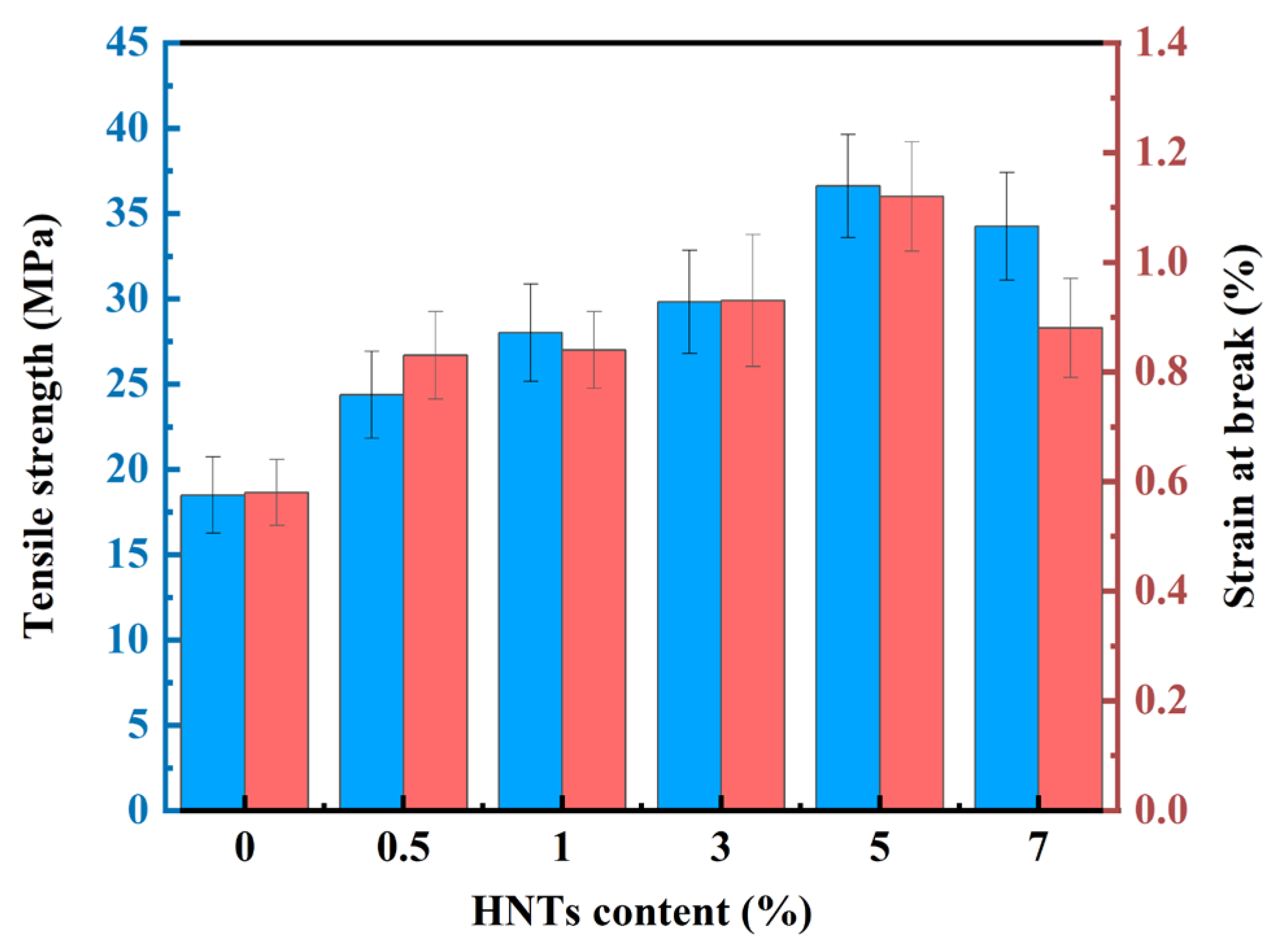
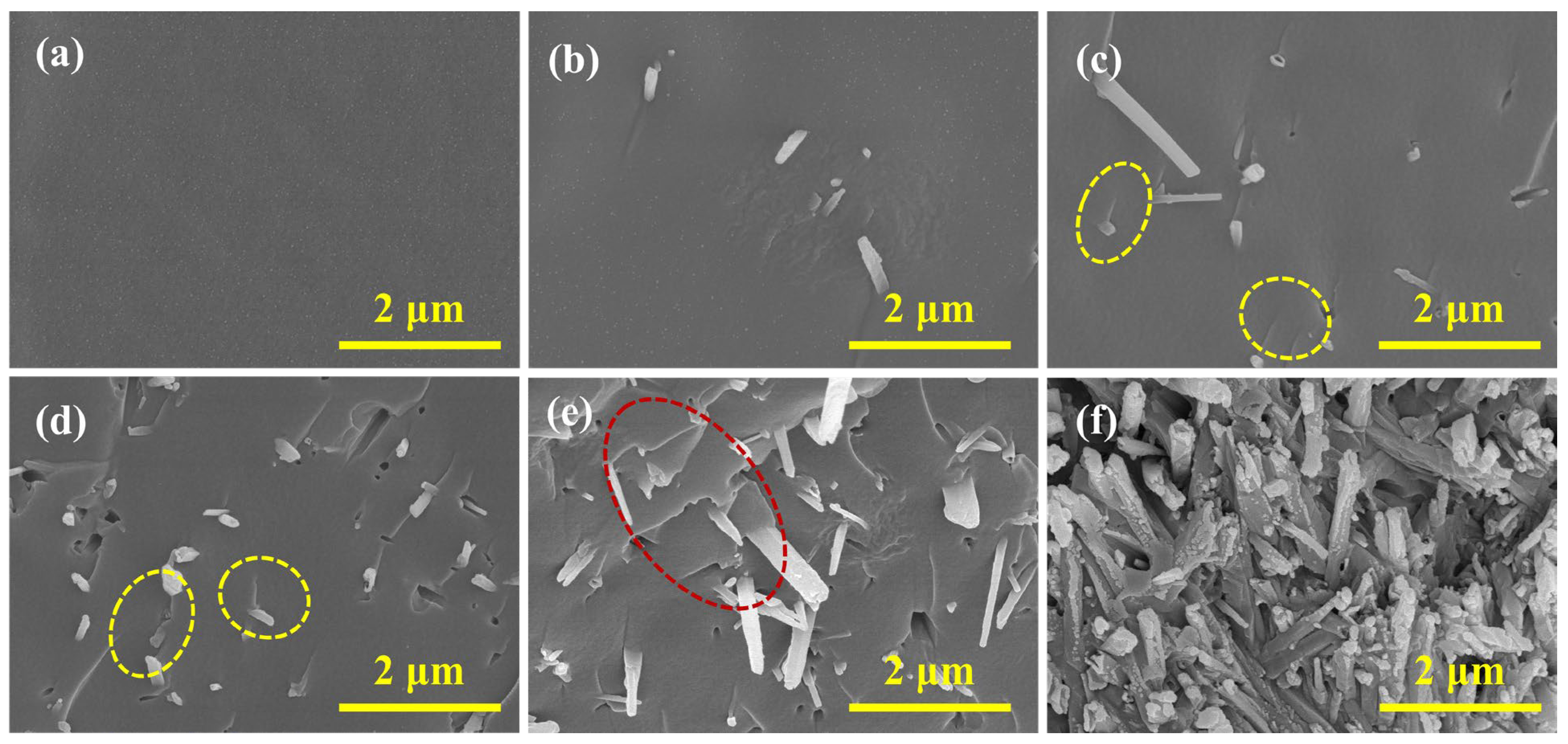
3.5. The Mechanical Property of H-MF-Impregnated Paper
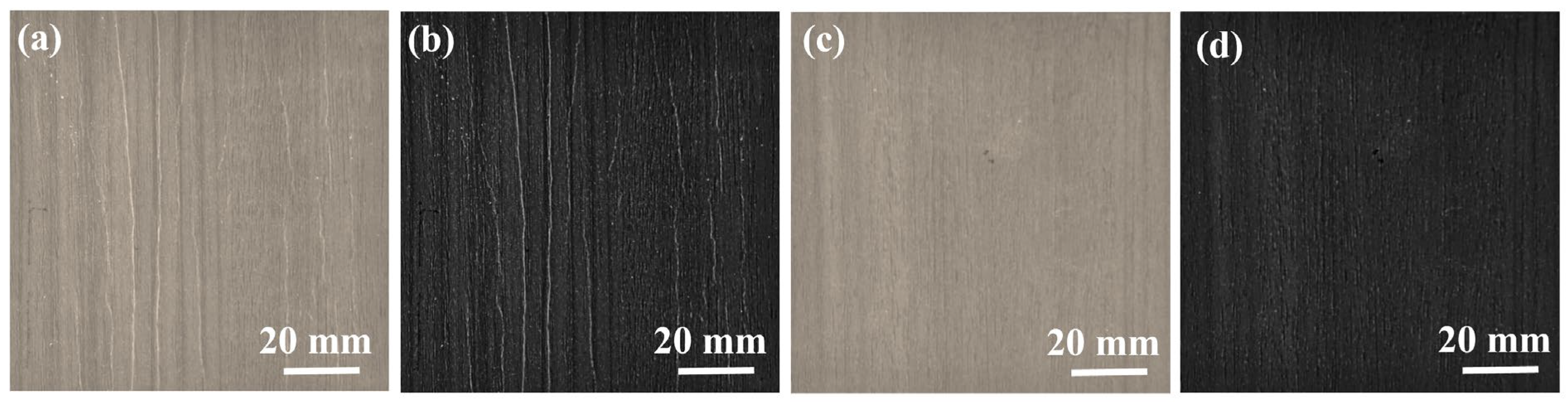
3.6. Cracking Resistance of the Decorated Blockboard
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Erikson, R.G.; Gorman, T.M.; Green, D.W.; Graham, D. Mechanical grading of lumber sawn from small-diameter lodge-pole pine, ponderosa pine, and grand fir trees from northern Idaho. For. Prod. J. 2000, 50, 59–65. [Google Scholar]
- Wiedenbeck, J.; Scholl, M.; Blankenhorn, P.; Ray, C. Lumber Volume and Value Recovery from Small-Diameter Black Cherry, Sugar Maple, and Red Oak Logs. BioResources 2016, 12, 853–870. [Google Scholar] [CrossRef][Green Version]
- Zeleniuc, O.; Coșereanu, C. Effect of Variable Conditions of Exposure on the Physical and Mechanical Properties of Blockboards. Appl. Sci. 2022, 12, 609. [Google Scholar] [CrossRef]
- Grenestedt, J.L.; Bekisli, B. Analyses and preliminary tests of a balsa sandwich core with improved shear properties. Int. J. Mech. Sci. 2003, 45, 1327–1346. [Google Scholar] [CrossRef]
- Laufenberg, T.; Ayrilmis, N.; White, R. Fire and bending properties of blockboard with fire retardant treated veneers. Holz als Roh- Werkst. 2006, 64, 137–143. [Google Scholar] [CrossRef]
- Fu, Z.; Weng, X.; Gao, Y.; Zhou, Y. Full-field tracking and analysis of shrinkage strain during moisture content loss in wood. Holzforschung 2021, 75, 436–443. [Google Scholar] [CrossRef]
- Gao, Y.; Fu, Z.; Zhou, Y.; Gao, X.; Zhou, F.; Cao, H. Moisture-Related Shrinkage Behavior of Wood at Macroscale and Cellular Level. Polymers 2022, 14, 5045. [Google Scholar] [CrossRef]
- Zhou, H.; Han, X.; Huang, L.; Wang, W.; Wang, S. Research Progress on Cracking Mechanism, Evaluation and Reinforcement of Ancient Building Timber Member. Chin. J. Wood Sci. Technol. 2024, 38, 13–22. [Google Scholar] [CrossRef]
- Han, Y.; Park, Y.; Park, J.-H.; Yang, S.-Y.; Eom, C.-D.; Yeo, H. The shrinkage properties of red pine wood assessed by image analysis and near-infrared spectroscopy. Dry. Technol. 2016, 34, 1613–1620. [Google Scholar] [CrossRef]
- Nkene Mezui, E.; Pambou Nziengui, C.F.; Moutou Pitti, R.; Ikogou, S.; Ekomy Ango, S.; Talla, P.K. Strain and cracks investigations on tropical green wood slices under natural drying: Experimental and numerical approaches. Eur. J. Wood Wood Prod. 2023, 81, 187–207. [Google Scholar] [CrossRef]
- Fortino, S.; Hradil, P.; Genoese, A.; Genoese, A.; Pousette, A. Numerical hygro-thermal analysis of coated wooden bridge members exposed to Northern European climates. Constr. Build. Mater. 2019, 208, 492–505. [Google Scholar] [CrossRef]
- Autengruber, M.; Lukacevic, M.; Fussl, J. Finite-element-based moisture transport model for wood including free water above the fiber saturation point. Int. J. Heat Mass Transf. 2020, 161, 120228. [Google Scholar] [CrossRef]
- Afshari, Z.; Malek, S. Moisture transport in laminated wood and bamboo composites bonded with thin adhesive layers—A numerical study. Constr. Build. Mater. 2022, 340, 127597. [Google Scholar] [CrossRef]
- Franke, B.; Franke, S.; Schiere, M.; Müller, A. Moisture content and moisture-induced stresses of large glulam members: Laboratory tests, in-situ measurements and modelling. Wood Mater. Sci. Eng. 2019, 14, 243–252. [Google Scholar] [CrossRef]
- Rindler, A.; Vay, O.; Hansmann, C.; Müller, U. Dimensional stability of multi-layered wood-based panels: A review. Wood Sci. Technol. 2017, 51, 969–996. [Google Scholar] [CrossRef]
- Yun, F.; Wei, Q.; Meihong, L.; Jinrong, H.; Limin, P.; Zongli, H. Research Review on Toughening Modification of Melamine Formaldehyde Resin. Chin. J. Wood Sci. Technol. 2022, 36, 17–21. [Google Scholar] [CrossRef]
- Zhai, R.; Zhang, B.; Wan, Y.; Li, C.; Wang, J.; Liu, J. Chitosan–halloysite hybrid-nanotubes: Horseradish peroxidase immobilization and applications in phenol removal. Chem. Eng. J. 2013, 214, 304–309. [Google Scholar] [CrossRef]
- Demirel, A.; Yilmaz, E.; Turk, S.; Caliskan, F. Preparation and investigation of porous chitosan/carbon nanotube biocomposite coating by space holder method. Diam. Relat. Mater. 2023, 138, 110217. [Google Scholar] [CrossRef]
- Tang, Y.; Deng, S.; Ye, L.; Yang, C.; Yuan, Q.; Zhang, J.; Zhao, C. Effects of unfolded and intercalated halloysites on mechanical properties of halloysite–epoxy nanocomposites. Compos. Part A 2011, 42, 345–354. [Google Scholar] [CrossRef]
- Xiao, K.; Zhang, Y.; Gong, Y.; Zhang, Y. Preparation of zinc oxide/halloysite compound and their reinforcement for styrene butadiene rubber composite. Appl. Clay Sci. 2023, 243, 107078. [Google Scholar] [CrossRef]
- Liu, M.; Jia, Z.; Jia, D.; Zhou, C. Recent advance in research on halloysite nanotubes-polymer nanocomposite. Prog. Polym. Sci. 2014, 39, 1498–1525. [Google Scholar] [CrossRef]
- Hashm, H.; Alam, S.; Gordon, S.; Kraft, J.; Yar Saleh, M.; Lvov, Y.; Matthews, J.; Radadia, A.; Weiss, L.; Amritphale, S.; et al. Halloysite clay nanotube composites as coating materials with enhanced properties. Constr. Build. Mater. 2023, 392, 131961. [Google Scholar] [CrossRef]
- Lapcík, L.; Sepetcioglu, H.; Murtaja, Y.; Lapcíková, B.; Vasina, M.; Ovsík, M.; Stanek, M.; Gautam, S. Study of mechanical properties of epoxy/graphene and epoxy/halloysite nanocomposites. Nanotechnol. Rev. 2023, 12, 20220520. [Google Scholar] [CrossRef]
- ASTM D3500-14; Standard Test Methods for Structural Panels in Tension. ASTM: West Conshohocken, PA, USA, 2014.
- EN 789:2004; Timber Structures—Test Methods—Determination of Mechanical Properties of Wood Based Panels. Standards Policy and Strategy Committee: Brussels, Belgium, 2004.
- Wang, T.; Wang, Y.; Crocetti, R.; Wålinder, M. In-plane mechanical properties of birch plywood. Constr. Build. Mater. 2022, 340, 127852. [Google Scholar] [CrossRef]
- GB/T 1943; Method for Determination of the Modulus of Elasticity in Compression Perpendicular to Grain of Wood. China National Standardization Administration: Beijing, China, 2009.
- Liu, J.Y.; Ross, R.J. Relationship Between Radial Compressive Modulus of Elasticity and Shear Modulus of Wood. Wood Fiber Sci. 2005, 37, 201–206. [Google Scholar]
- GB/T 17657; Test Methods of Evaluating the Properties of Wood-Based Panels and Surface Decorated Wood-Based Panels. China Academy of Forestry Sciences: Beijing, China, 2013.
- Chen, K.; Qiu, H.; Sun, M.; Lam, F. Experimental and numerical study of moisture distribution and shrinkage crack propagation in cross section of timber members. Constr. Build. Mater. 2019, 221, 219–231. [Google Scholar] [CrossRef]
- He, J.; Qu, W.; Feng, Y.; Jiang, J.; Luo, J.; Wu, Y.; Peng, L. Multiple synergistic antibacterial melamine-impregnated paper based on nano Ag-doped ZIF-8. Chem. Eng. J. 2024, 493, 152453. [Google Scholar] [CrossRef]
- Merline, D.J.; Vukusic, S.; Abdala, A.A. Melamine formaldehyde: Curing studies and reaction mechanism. Polym. J. 2013, 45, 413–419. [Google Scholar] [CrossRef]
- Weiss, S.; Urdl, K.; Mayer, H.A.; Zikulnig-Rusch, E.M.; Kandelbauer, A. IR spectroscopy: Suitable method for determination of curing degree and crosslinking type in melamine–formaldehyde resins. J. Appl. Polym. Sci. 2019, 136, 17691. [Google Scholar] [CrossRef]
- Kandelbauer, A.; Wuzella, G.; Mahendran, A.; Taudes, I.; Widsten, P. Model-free kinetic analysis of melamine-formaldehyde resin cure. Chem. Eng. J. 2009, 152, 556–565. [Google Scholar] [CrossRef]
- Kohlmayr, M.; Stultschnik, J.; Teischinger, A.; Kandelbauer, A. Drying and curing behaviour of melamine formaldehyde resin impregnated papers. J. Appl. Polym. Sci. 2014, 131, 39860. [Google Scholar] [CrossRef]
- Gao, Y.; Liu, S.; Wang, Q.; Wang, G. Preparation of melamine–formaldehyde resin grafted by (3-aminopropyl) triethoxysilane for high-performance hydrophobic materials. J. Appl. Polym. Sci. 2020, 137, 48664. [Google Scholar] [CrossRef]
- Barber, N.F.; Meylan, B.A. The Anisotropic Shrinkage of Wood. A Theoretical Model. Holzforschung 1964, 18, 146–156. [Google Scholar] [CrossRef]
- Fu, Z.; Zhao, J.; Yang, Y.; Cai, Y. Variation of Drying Strains between Tangential and Radial Directions in Asian White Birch. Forests 2016, 7, 59. [Google Scholar] [CrossRef]
- Yoshihara, H.; Maruta, M. Mode II fracture mechanics properties of solid wood measured by the tensile- and compressive-loading shear fracture tests. Eng. Fract. Mech. 2019, 213, 72–88. [Google Scholar] [CrossRef]
- Romanowicz, M. Numerical assessment of the apparent fracture process zone length in wood under mode I condition using cohesive elements. Theor. Appl. Fract. Mech. 2022, 118, 103229. [Google Scholar] [CrossRef]
- Barrett, J.D.; Foschi, R.O. Mode II stress-intensity factors for cracked wood beams. Eng. Fract. Mech. 1977, 9, 371–378. [Google Scholar] [CrossRef]
- Feng, Z.-R.; Wang, B.-B.; Dong, H.; Zhao, S.-P.; Wu, Y.-P.; Qiao, Q.; Ren, X.-M. Dielectrics and possible ferroelectricity in diol/glycerol covalently grafted kaolinites. Dalton T 2023, 52, 1089–1095. [Google Scholar] [CrossRef]
- Zhao, Q.; Cheng, X.; Kang, J.; Kong, L.; Zhao, X.; He, X.; Li, J. Polyvinyl alcohol flame retardant film based on halloysite nanotubes, chitosan and phytic acid with strong mechanical and anti-ultraviolet properties. Int. J. Biol. Macromol. 2023, 246, 125682. [Google Scholar] [CrossRef]
- Panangama, L.A.; Pizzi, A. A13C-NMR analysis method for MUF and MF resin strength and formaldehyde emission. J. Appl. Polym. Sci. 1996, 59, 2055–2068. [Google Scholar] [CrossRef]
- Park, B.D.; Jeong, H.W. Cure kinetics of melamine–formaldehyde resin/clay/cellulose nanocomposites. J. Ind. Eng. Chem. 2010, 16, 375–379. [Google Scholar] [CrossRef]
- Feng, Y.; Qu, W.; Wu, Y.; Zhang, J.; Peng, L. Crack-resistant melamine/formaldehyde-impregnated paper-decorated panels using blocked isocyanates as a temperature-responsive crosslinker. Prog. Org. Coat. 2022, 173, 107211. [Google Scholar] [CrossRef]
- Altgen, M.; Awais, M.; Altgen, D.; Klüppel, A.; Mäkelä, M.; Rautkari, L. Distribution and curing reactions of melamine formaldehyde resin in cells of impregnation-modified wood. Sci. Rep. 2020, 10, 3366. [Google Scholar] [CrossRef] [PubMed]
- Mohebali, A.; Abdouss, M.; Afshar Taromi, F. Fabrication of biocompatible antibacterial nanowafers based on HNT/PVA nanocomposites loaded with minocycline for burn wound dressing. Mater. Sci. Eng. C 2020, 110, 110685. [Google Scholar] [CrossRef]
- Paz Tal, O.; Mizrahi, A.; Lerner, N.; Gelfer, S.; Columbus, I.; Mizrahi, D. Preparation and characterization of Melamine-Formaldehyde (MF)-bound cyclen derivatives for efficient removal of uranium [U(VI)] ions from wastewater. J. Water Process Eng. 2022, 45, 102448. [Google Scholar] [CrossRef]
- Chen, L.; Jia, Z.; Guo, X.; Zhong, B.; Chen, Y.; Luo, Y.; Jia, D. Functionalized HNTs nanocluster vulcanized natural rubber with high filler-rubber interaction. Chem. Eng. J. 2018, 336, 748–756. [Google Scholar] [CrossRef]
- Zhang, W.; Dai, M.; Liang, X.; Wang, X.; Wei, W.; Zhou, Z. Interfacial Enhancement by CNTs Grafting towards High-Performance Mechanical Properties of Carbon Fiber-Reinforced Epoxy Composites. Materials 2023, 16, 3825. [Google Scholar] [CrossRef]
- Ravichandran, G.; Rathnakar, G.; Santhosh, N.; Chennakeshava, R.; Ahmad, H.M. Enhancement of mechanical properties of epoxy/halloysite nanotube (HNT) nanocomposites. SN Appl. Sci. 2019, 1, 296. [Google Scholar] [CrossRef]

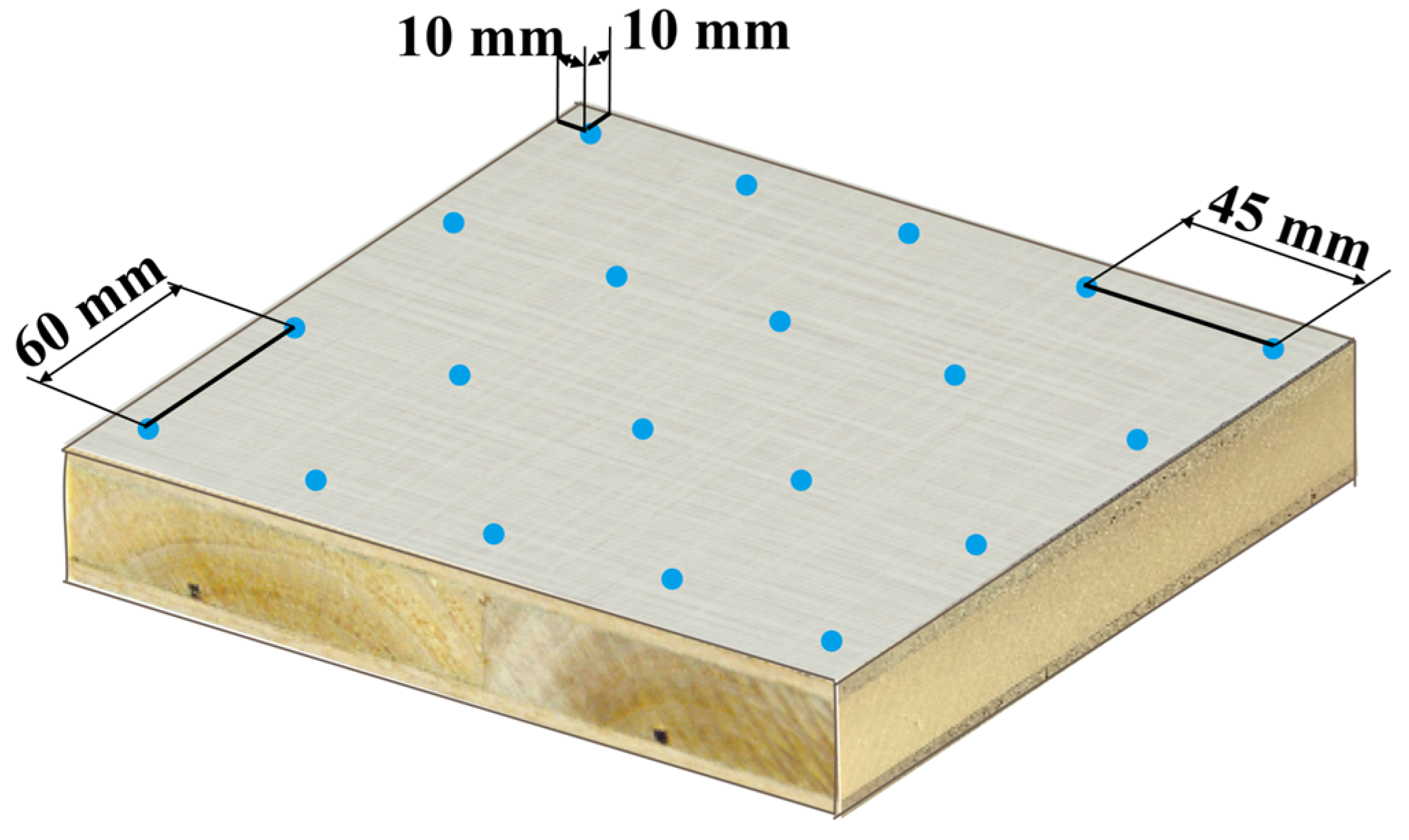
| Material | Thickness/mm | Angle/° | |
|---|---|---|---|
| L1 | Poplar (Populus × euramericana cv. ‘74/76’) | 0.40 | 90 |
| L2 | Poplar (Populus × euramericana cv. ‘74/76’) | 2.00 | 0 |
| L3 | Chinese fir (Cunninghamia lanceolata (Lamb.)) | 11.68 | 90 |
| Total | - | 16.48 | - |
| Humidity Field | Temperature Field |
| Moisture content: u | Temperature: T |
| Moisture diffusion coefficient: D | Thermal conductivity: λ |
| Equilibrium moisture content: We | External temperature: Te |
| Elastic Modulus (MPa) | Shear Modulus (MPa) | Poisson’s Ratio | Coefficient of Shrinkage | Density (g/cm3) |
|---|---|---|---|---|
| EL = 10,653 ± 649 | GTL = 799 | νTL = 0.032 ± 0.008 | 0.031 ± 0.013 | 0.45 ± 0.02 |
| ER = 1134 ± 93 | GRT = 639 | νRT = 0.789 ± 0.121 | 0.320 ± 0.059 | |
| ET = 465 ± 66 | GRL = 191 | νRL = 0.050 ± 0.024 | 0.669 ± 0.029 |
| Elastic Modulus (MPa) | Shear Modulus (MPa) | Poisson’s Ratio | Coefficient of Shrinkage | Density (g/cm3) |
|---|---|---|---|---|
| EL = 12,888 | GTL = 773 | νTL = 0.020 | 0.019 | 0.35 |
| ER = 1048 | GRT = 232 | νRT = 0.430 | 0.139 | |
| ET = 594 | GRL = 967 | νRL = 0.029 | 0.255 |
| MF | H-MF 0.5% | H-MF 1% | H-MF 3% | H-MF 5% | H-MF 7% | |
|---|---|---|---|---|---|---|
| Stress (MPa) | 18.50 ± 2.24 | 24.36 ± 2.54 | 28.00 ± 2.85 | 29.82 ± 3.03 | 36.60 ± 3.03 | 34.24 ± 3.15 |
| Strain (%) | 0.58 ± 0.06 | 0.83 ± 0.08 | 0.84 ± 0.07 | 0.93 ± 0.10 | 1.12 ± 0.10 | 0.88 ± 0.09 |
| Elasticity modulus (GPa) | 3.18 ± 0.06 | 2.93 ± 0.03 | 3.33 ± 0.06 | 3.21 ± 0.02 | 3.27 ± 0.02 | 3.89 ± 0.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Y.; Qu, W.; Wu, G.; Wu, Y.; He, J.; Shen, Y.; Cao, J.; Peng, L. Surface Crack Occurrence and Resistance During Moisture Content Changes in MF-Resin-Impregnated Paper-Decorated Blockboard. Forests 2025, 16, 411. https://doi.org/10.3390/f16030411
Feng Y, Qu W, Wu G, Wu Y, He J, Shen Y, Cao J, Peng L. Surface Crack Occurrence and Resistance During Moisture Content Changes in MF-Resin-Impregnated Paper-Decorated Blockboard. Forests. 2025; 16(3):411. https://doi.org/10.3390/f16030411
Chicago/Turabian StyleFeng, Yun, Wei Qu, Guofang Wu, Yuzhang Wu, Jinrong He, Yinlan Shen, Jinzhen Cao, and Limin Peng. 2025. "Surface Crack Occurrence and Resistance During Moisture Content Changes in MF-Resin-Impregnated Paper-Decorated Blockboard" Forests 16, no. 3: 411. https://doi.org/10.3390/f16030411
APA StyleFeng, Y., Qu, W., Wu, G., Wu, Y., He, J., Shen, Y., Cao, J., & Peng, L. (2025). Surface Crack Occurrence and Resistance During Moisture Content Changes in MF-Resin-Impregnated Paper-Decorated Blockboard. Forests, 16(3), 411. https://doi.org/10.3390/f16030411







