Growth Rate, Tree Rings, and Wood Anatomy of a Tropical Cloud Forest Tree Invader
Abstract
1. Introduction
2. Materials and Methods
2.1. Tree Rings
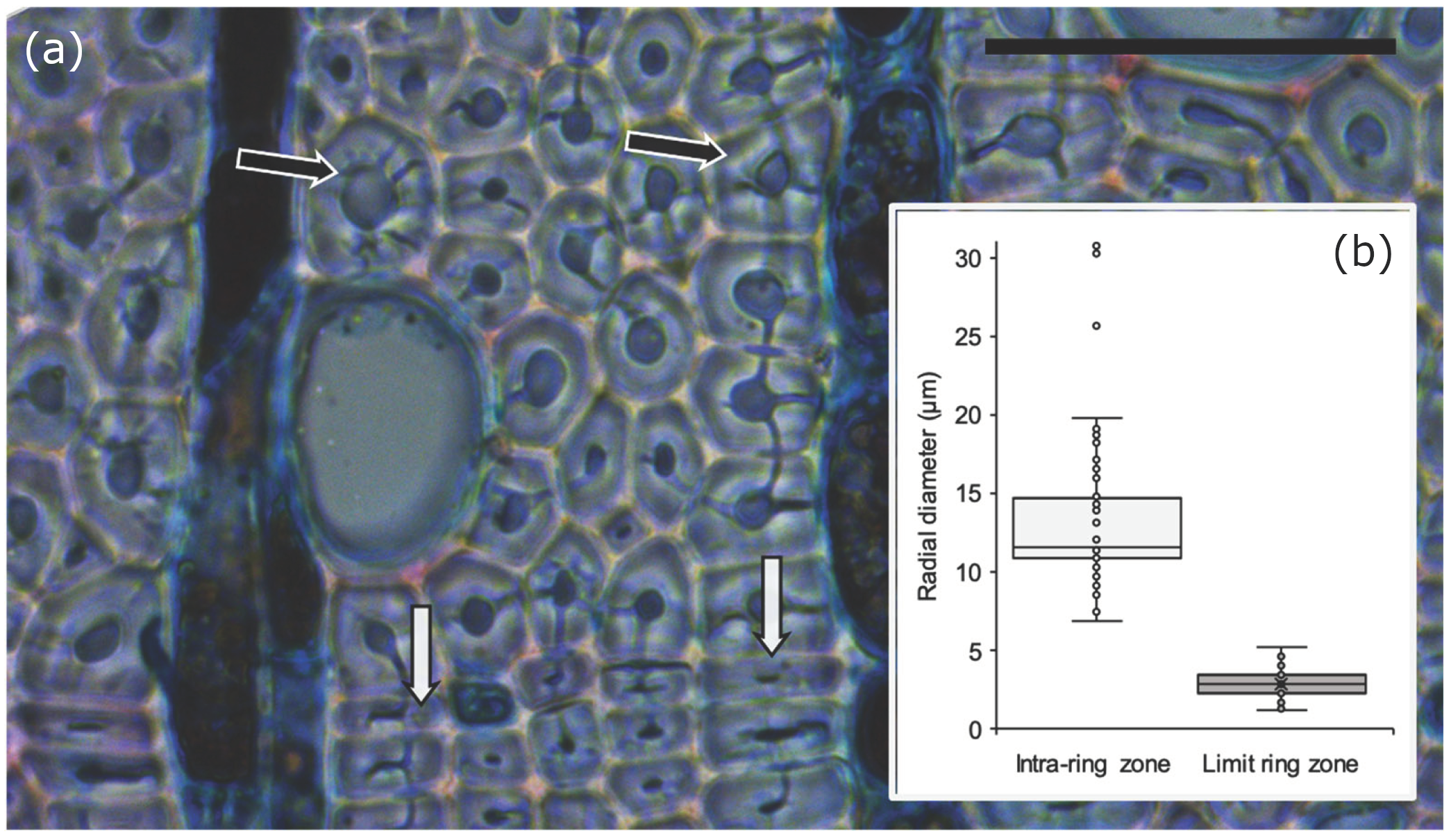
2.1.1. Anatomical Boundaries of the Tree Rings
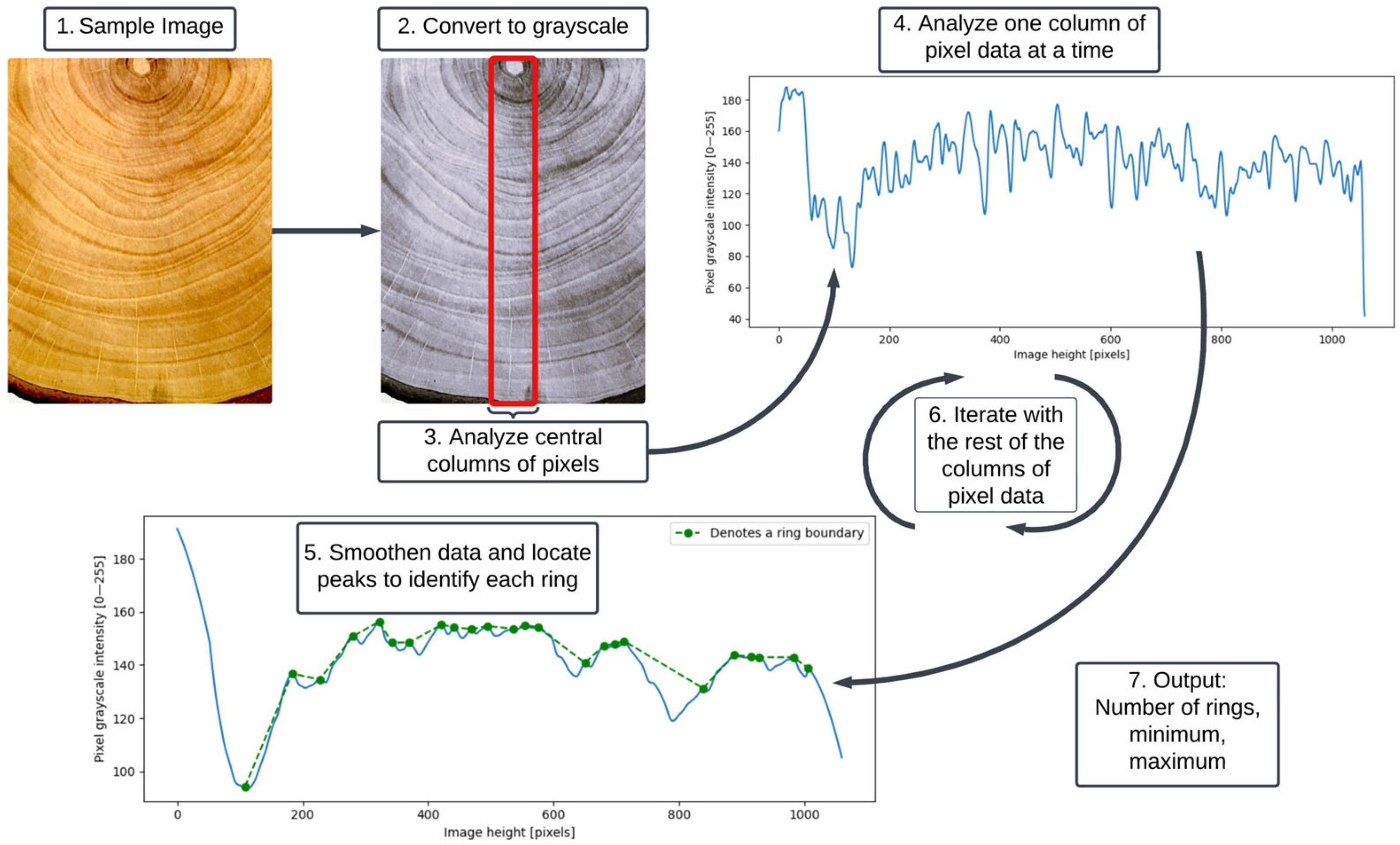
2.1.2. Counting Tree Rings
2.2. Wood Density
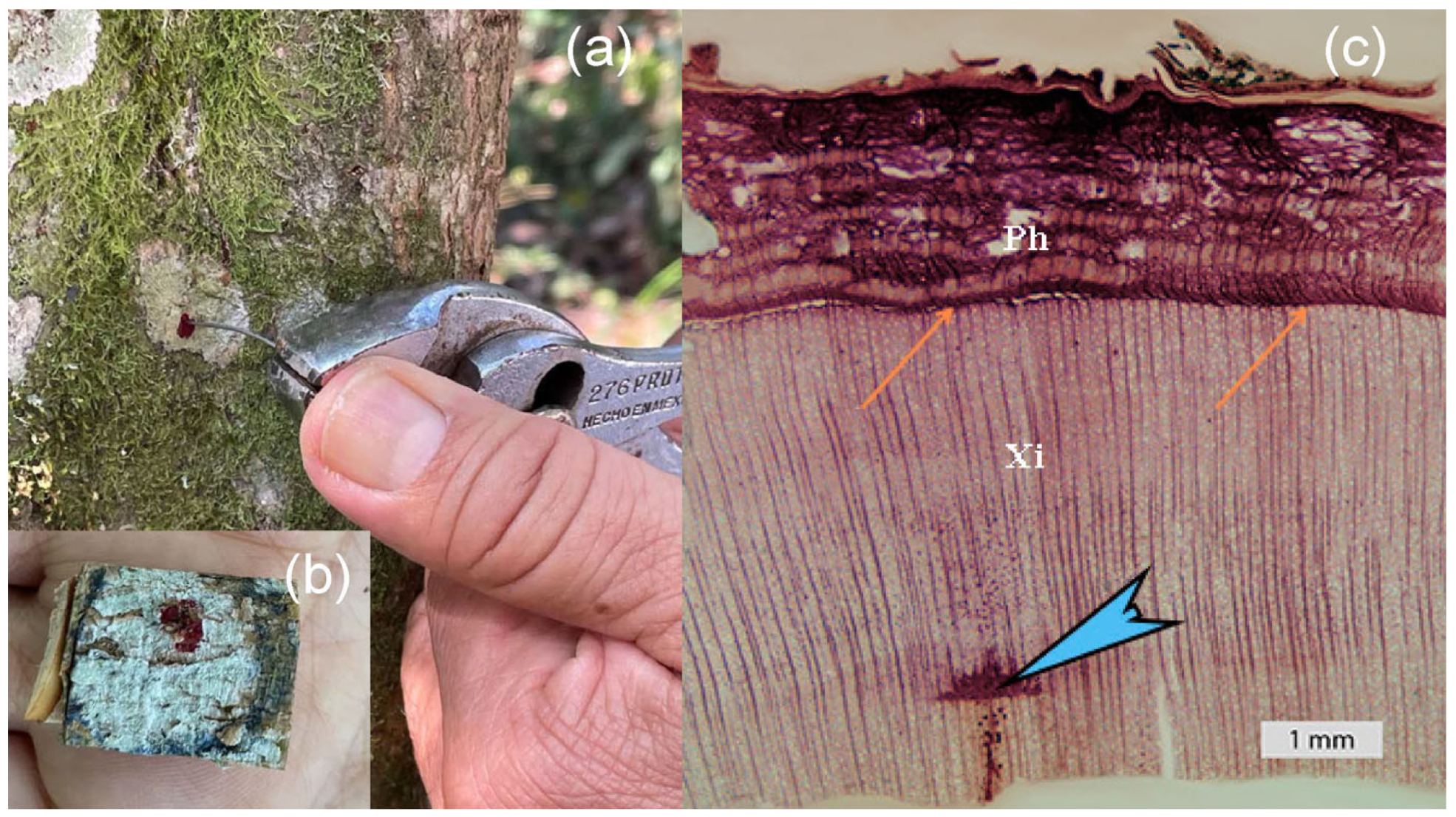
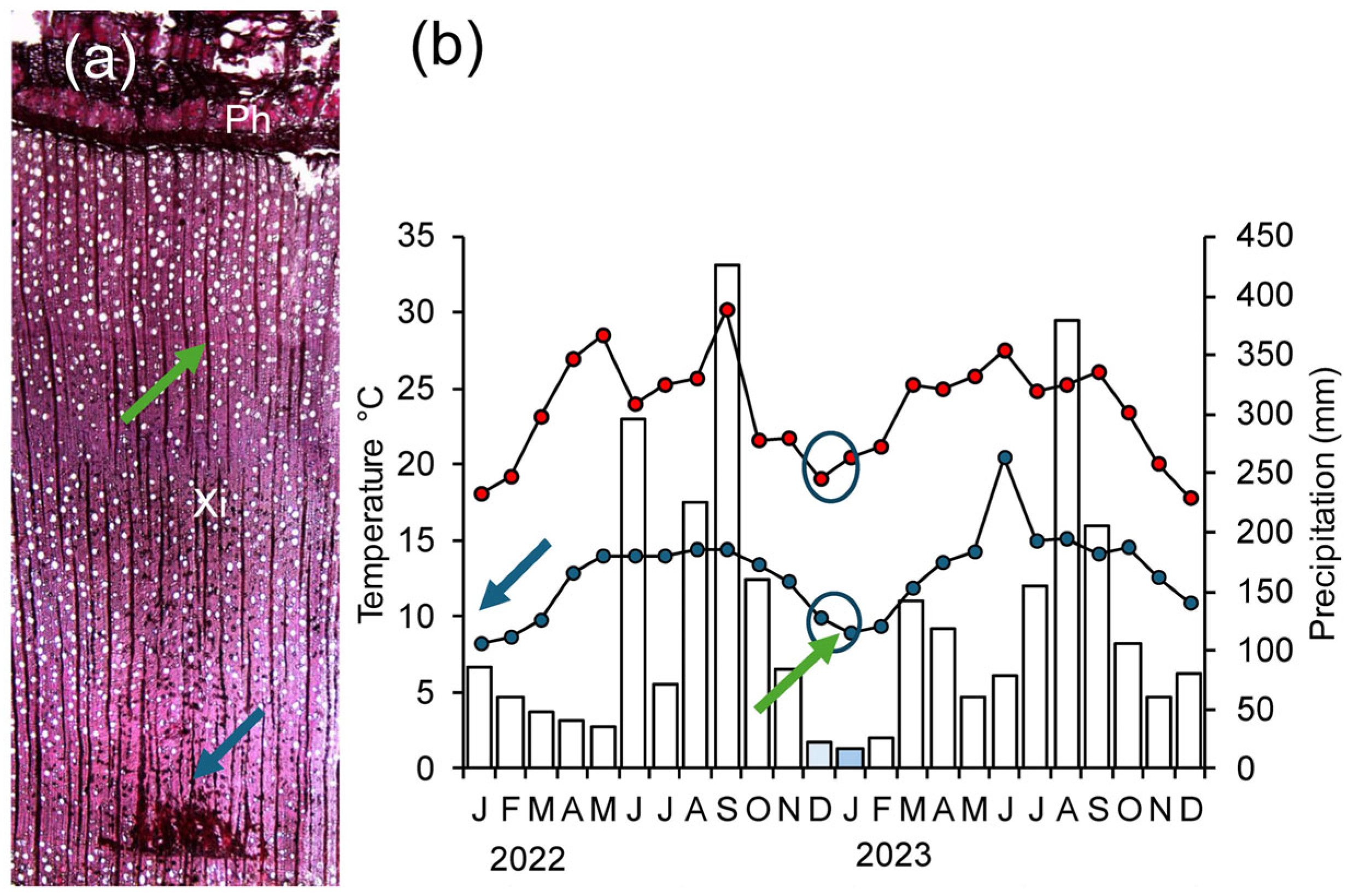
2.3. Pinning Method
2.4. Tree Growth Rate
2.5. Statistical Analysis
3. Results
3.1. Wood Density
3.2. Tree Rings
3.3. Pinning Method
3.4. Growth Rate
4. Discussion
4.1. Wood Density
4.2. Pinning Method
4.3. Tree Rings and Age
4.4. Tree Growth
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Delavaux, C.S.; Crowther, T.W.; Zohner, M.C.; Robmann, N.M.; Lauber, T.; Van den Hoogen, J.; Kuebbing, S.; Liang, J.; De-Miguel, S.; Nabuurs, G.J.; et al. Native diversity buffers against severity of non-native tree invasions. Nature 2023, 621, 773–781. [Google Scholar] [CrossRef] [PubMed]
- Martin, P.H.; Canham, C.D.; Marks, P.L. Why forests appear resistant to exotic plant invasions: Intentional introductions, stand dynamics, and the role of shade tolerance. Front. Ecol. Environ. 2009, 7, 142–149. [Google Scholar] [CrossRef]
- Heberling, J.M.; Fridley, J.D. Invaders do not require high resource levels to maintain physiological advantages in a temperate deciduous forest. Ecology 2016, 97, 874–884. [Google Scholar] [CrossRef] [PubMed]
- Fridley, J.D. Fast but steady: An integrated leaf-stem-root trait syndrome for woody forest invaders. Ecol. Lett. 2022, 25, 900–912. [Google Scholar] [CrossRef]
- Fridley, J.D.; Bellingham, P.J.; Closset-Kopp, D.; Daehler, C.C.; Dechoum, M.S.; Martin, P.H.; Murphy, H.T.; Rojas-Sandoval, J.; Tng, D. A general hypothesis of forest invasions by woody plants based on whole-plant carbon economics. J. Ecol. 2023, 111, 4–22. [Google Scholar] [CrossRef]
- Valladares, F.; Niinemets, U. Shade Tolerance, a Key Plant Feature of Complex Nature and Consequences. Annu. Rev. Ecol. Evol. Syst. 2008, 39, 237–257. [Google Scholar] [CrossRef]
- Grotkopp, E.; Rejmánek, M. High seedling relative growth rate and species leaf area traits of invasive species: Phyllogenetically independent contrasts of woody angiosperms. Am. J. Bot. 2007, 94, 526–532. [Google Scholar] [CrossRef]
- Kuroda, K.; Kiyono, Y. Seasonal rhythms of xylem growth measured by the wounding method and with a band-dendrometer: An instance of Chamaecyparis obtuse. IAWA J. 1997, 18, 291–299. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Yang, B.; Qin, C.; Shi, F. Research progress in monitoring and simulating stem radius growth: An overview. Sci. Cold. Arid. Reg. 2012, 4, 175–183. [Google Scholar] [CrossRef]
- Subah, S.; Derminder, S.; Sanjeev, C. An interactive computer vision system for tree ring analysis. Curr. Sci. 2017, 112, 1262–1265. [Google Scholar] [CrossRef]
- Seyoum, Y.; Fetene, M.; Strobl, S.; Beck, E. Foliage dynamics, leaf traits, and growth of coexisting evergreen and deciduous trees in a tropical montane forest in Ethiopia. Trees 2012, 26, 1495–1512. [Google Scholar] [CrossRef]
- Gibert, A.; Gray, E.F.; Westoby, M.; Wright, I.J.; Falster, D.S. On the link between functional traits and growth rate: Meta-analysis shows effects change with plant size, as predicted. J. Ecol. 2016, 104, 1488–1503. [Google Scholar] [CrossRef]
- Muller-Landau, H.C. Interspecific and inter-site variation in wood specific gravity of tropical trees. Biotropica 2004, 36, 20–32. [Google Scholar] [CrossRef]
- Chave, J.; Coomes, D.; Jansen, S.; Lewis, S.L.; Swenson, N.G.; Zanne, A.E. Towards a worldwide wood economics spectrum. Ecol. Lett. 2009, 12, 351–366. [Google Scholar] [CrossRef]
- Matos, B.; Borges Silva, L.; Camarinho, R.; Rodrigues, A.S.; Rego, R.; Câmara, M.; Silva, L. Linking Dendrometry and Dendrochronology in the Dominant Azorean Tree Laurus azorica (Seub.) Franco. Forests 2019, 10, 538. [Google Scholar] [CrossRef]
- Williams-Linera, G.; Berry, Z.C.; Diaz-Toribio, M.H.; Espejel-Ontiveros, X. Drought responses of an exotic tree (Eriobotrya japonica) in a tropical cloud forest suggest the potential to become an invasive species. New For. 2022, 53, 571–585. [Google Scholar] [CrossRef]
- Williams-Linera, G.; Díaz-Toribio, M.H. Resprouting and foliar functional traits driving the invasiveness of Eriobotrya japonica in a secondary cloud forest. Trees For. People 2023, 14, 100455. [Google Scholar] [CrossRef]
- Huang, J. Characterization of the complete chloroplast genome of Eriobotrya japonica in China and phylogenetic relationships. Mitochondrial DNA B 2019, 4, 1367–1369. [Google Scholar] [CrossRef]
- Rojas-Sandoval, J.; Tremblay, R.L.; Acevedo-Rodríguez, P.; Díaz-Soltero, H. Invasive plant species in the West Indies: Geographical, ecological, and floristic insights. Ecol. Evol. 2017, 7, 4522–4533. [Google Scholar] [CrossRef]
- Lisi, C.S.; Fo, M.T.; Botosso, P.C.; Roig, F.A.; Maria, V.R.; Ferreira-Fedele, L.; Voigt, A.R. Tree-ring formation, radial increment periodicity, and phenology of tree species from a seasonal semi-deciduous forest in southeast Brazil. IAWA J. 2008, 29, 189–207. [Google Scholar] [CrossRef]
- Tarelkin, Y.; Delvaux, C.; De Ridder, M.; El Berkani, T.; De Cannière, C.; Beeckman, H. Growth-ring distinctness and boundary anatomy variability in tropical trees. IAWA J. 2016, 37, 275–294. [Google Scholar] [CrossRef]
- Ferrero, M.E.; Villalba, R.; Rivera, S.M. An assessment of growth ring identification in subtropical forests from northwestern Argentina. Dendrochronologia 2014, 32, 113–119. [Google Scholar] [CrossRef]
- Seo, J.W.; Eckstein, D.; Schmitt, U. The pinning method: From pinning to data preparation. Dendrochronologia 2007, 25, 79–86. [Google Scholar] [CrossRef]
- Vizcaino-Bravo, Q.; Williams-Linera, G.; Asbjornsen, H. Biodiversity and carbon storage are correlated across land use intensification in a tropical montane forest watershed, Veracruz, Mexico. Basic. Appl. Ecol. 2020, 44, 24–34. [Google Scholar] [CrossRef]
- Zanne, A.E.; Lopez-Gonzalez, G.; Coomes, D.A.; Ilic, J.; Jansen, S.; Lewis, S.L.; Miller, R.B.; Swenson, N.G.; Wiemann, M.C.; Chave, J. Data from: Towards a Worldwide Wood Economics Spectrum—Global Wood Density Database; Dryad: Davis, CA, USA, 2009. [Google Scholar] [CrossRef]
- Larjavaara, M.; Muller-Landau, H.C. Rethinking the value of high wood density. Funct. Ecol. 2010, 24, 701–705. [Google Scholar] [CrossRef]
- Rodríguez-Ramírez, E.C.; Ferrero, M.E.; Acevedo-Vega, I.; Crispin-DelaCruz, D.B.; Ticse-Otarola, G.; Requena-Rojas, E.J. Plastic adjustments in xylem vessel traits to drought events in three Cedrela species from Peruvian tropical Andean forests. Sci. Rep. 2022, 12, 21112. [Google Scholar] [CrossRef]
- Pumijumnong, N.; Muangsong, C.; Buajan, S.; Songtrirat, P.; Chatwatthana, R.; Chareonwong, U. Factors affecting cambial growth periodicity and wood formation in tropical forest trees: A review. Forests 2023, 14, 1025. [Google Scholar] [CrossRef]
- Lin, Q.; He, T.; Sun, Y.; He, X.; Qiu, J. A computer-aided method for identifying the presence of softwood growth ring boundaries. PLoS ONE 2020, 15, e0235727. [Google Scholar] [CrossRef]
- Heitz, P. A simple program to measure and analyse tree rings using Excel, R and SigmaScan. Dendrochronologia 2011, 29, 245–250. [Google Scholar] [CrossRef]
- Mäkinen, H.; Seo, J.-W.; Nöjd, P.; Schmitt, U.; Jalkanen, R. Seasonal dynamics of wood formation: A comparison between pinning, microcoring and dendrometer measurements. Eur. J. Forest Res. 2008, 127, 235–245. [Google Scholar] [CrossRef]
- Wyckoff, P.H.; Clark, J.S. Tree growth prediction using size and exposed crown area. Can. J. For. Res. 2005, 35, 13–20. [Google Scholar] [CrossRef]
- Ouédraogo, D.Y.; Mortier, F.; Gourlet-Fleury, S.; Freycon, V.; Picard, N. Slow-growing species cope best with drought: Evidence from long-term measurements in a tropical semi-deciduous moist forest of Central Africa. J. Ecol. 2013, 101, 1459–1470. [Google Scholar] [CrossRef]
- Borges-Silva, L.; Teixeira, A.; Alves, M.; Elias, R.B.; Silva, L. Tree age determination in the widespread woody plant invader Pittosporum undulatum. For. Ecol. Manag. 2017, 400, 457–467. [Google Scholar] [CrossRef]


| Seasons | |||
|---|---|---|---|
| Relatively Dry–Cold | Dry–Warm | Rainy–Warm | |
| Months | November–March | April–May | June–October |
| Precipitation (mm) | 75 ± 13 | 63 ± 19 | 229 ± 46 |
| Maximum temperature (°C) | 20.9 ± 0.6 | 26.6 ± 0.8 | 26.1 ± 0.7 |
| Minimum temperature (°C) | 10.9 ± 0.6 | 13.7 ± 0.3 | 15.2 ± 0.8 |
| Annual Growth (cm yr−1) | RGR (cm cm−1 yr−1) | |
|---|---|---|
| A. Diameter tape | 0.156 ± 0.056 a | 0.0198 ± 0.0070 a |
| B. Dendrometer | 0.179 ± 0.059 a | 0.0250 ± 0.0073 a |
| C. Pinning method | 0.153 ± 0.048 a |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Williams-Linera, G.; Díaz-Toribio, M.H.; Angeles, G. Growth Rate, Tree Rings, and Wood Anatomy of a Tropical Cloud Forest Tree Invader. Forests 2025, 16, 258. https://doi.org/10.3390/f16020258
Williams-Linera G, Díaz-Toribio MH, Angeles G. Growth Rate, Tree Rings, and Wood Anatomy of a Tropical Cloud Forest Tree Invader. Forests. 2025; 16(2):258. https://doi.org/10.3390/f16020258
Chicago/Turabian StyleWilliams-Linera, Guadalupe, Milton H. Díaz-Toribio, and Guillermo Angeles. 2025. "Growth Rate, Tree Rings, and Wood Anatomy of a Tropical Cloud Forest Tree Invader" Forests 16, no. 2: 258. https://doi.org/10.3390/f16020258
APA StyleWilliams-Linera, G., Díaz-Toribio, M. H., & Angeles, G. (2025). Growth Rate, Tree Rings, and Wood Anatomy of a Tropical Cloud Forest Tree Invader. Forests, 16(2), 258. https://doi.org/10.3390/f16020258








