Quasistatic and Dynamic Nanoindentation Measurements of Pinus radiata D. Don S2 and CCML Cell Wall Layers
Abstract
1. Introduction
2. Materials and Methods
2.1. Sample Origin and Preparation
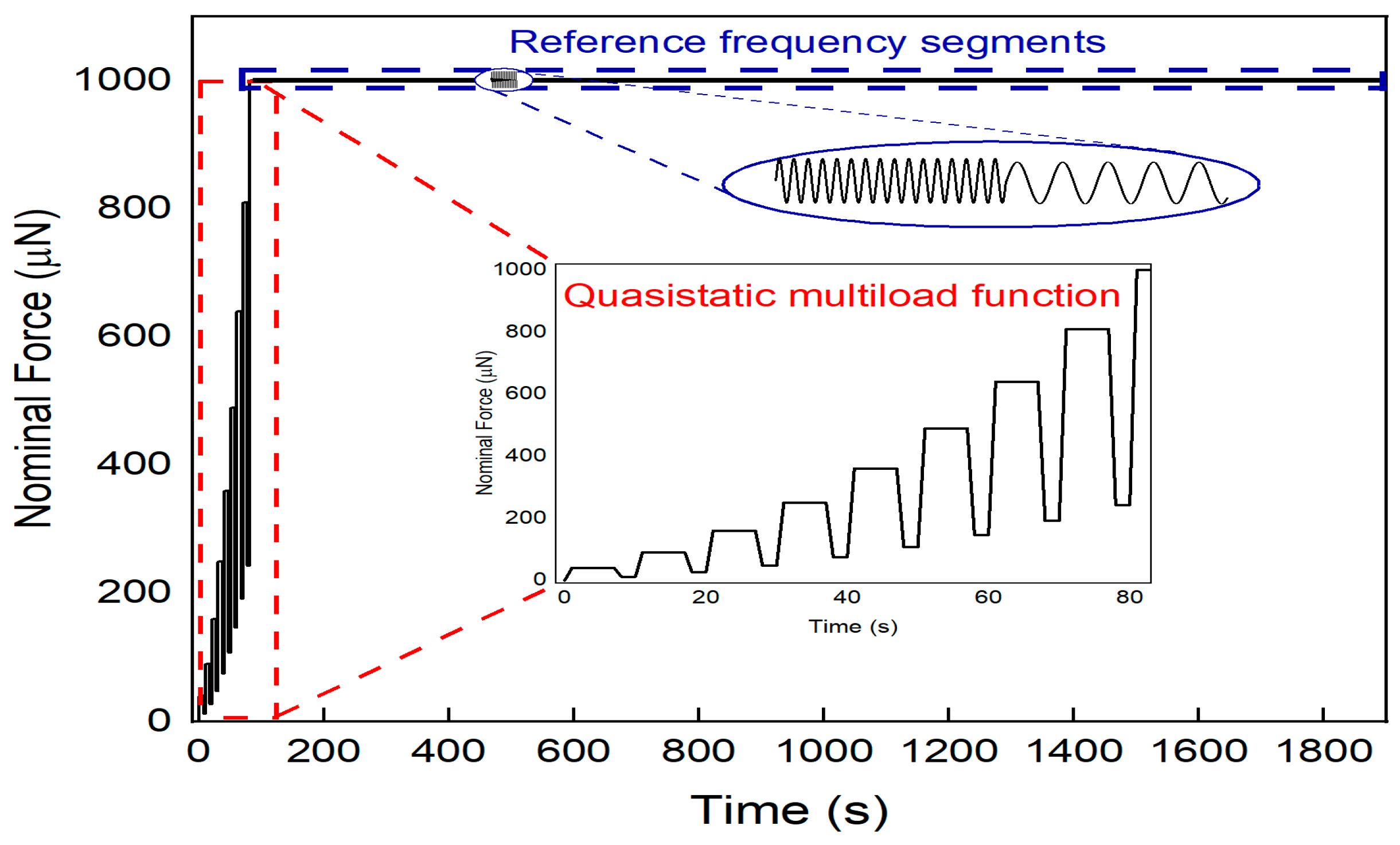
2.2. Nanoindentation
3. Results and Discussion
3.1. Quasistatic Nanoindentations
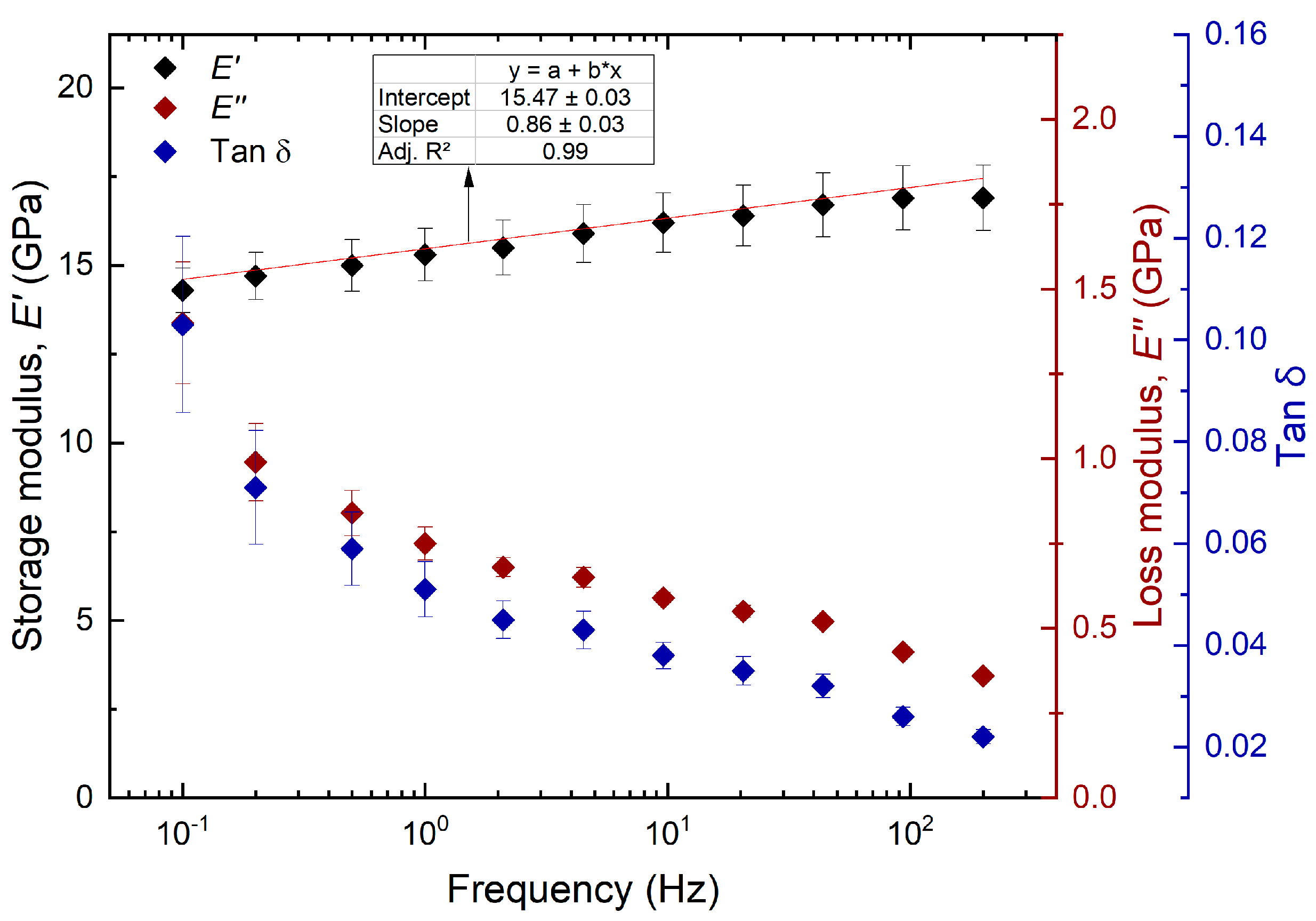
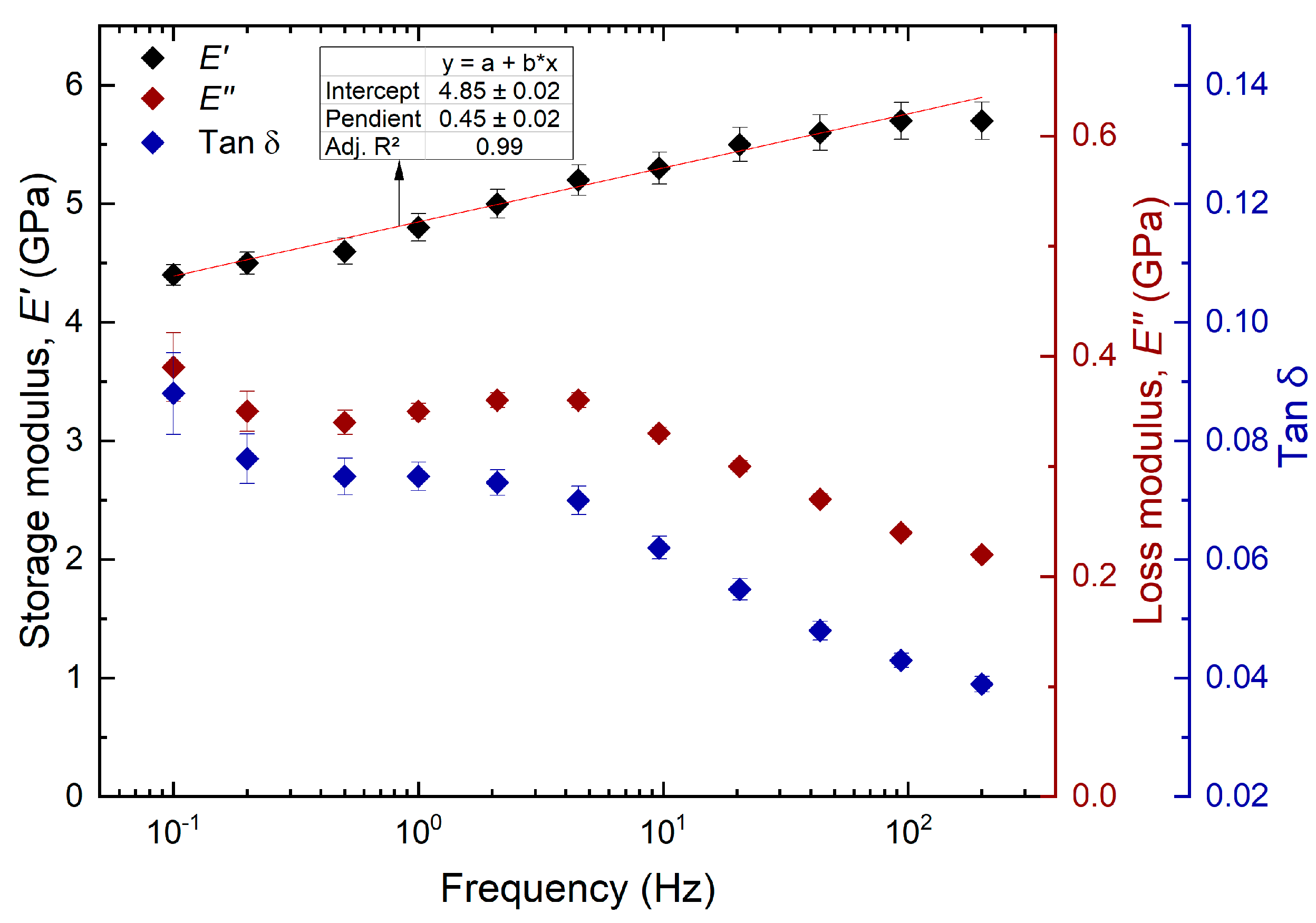
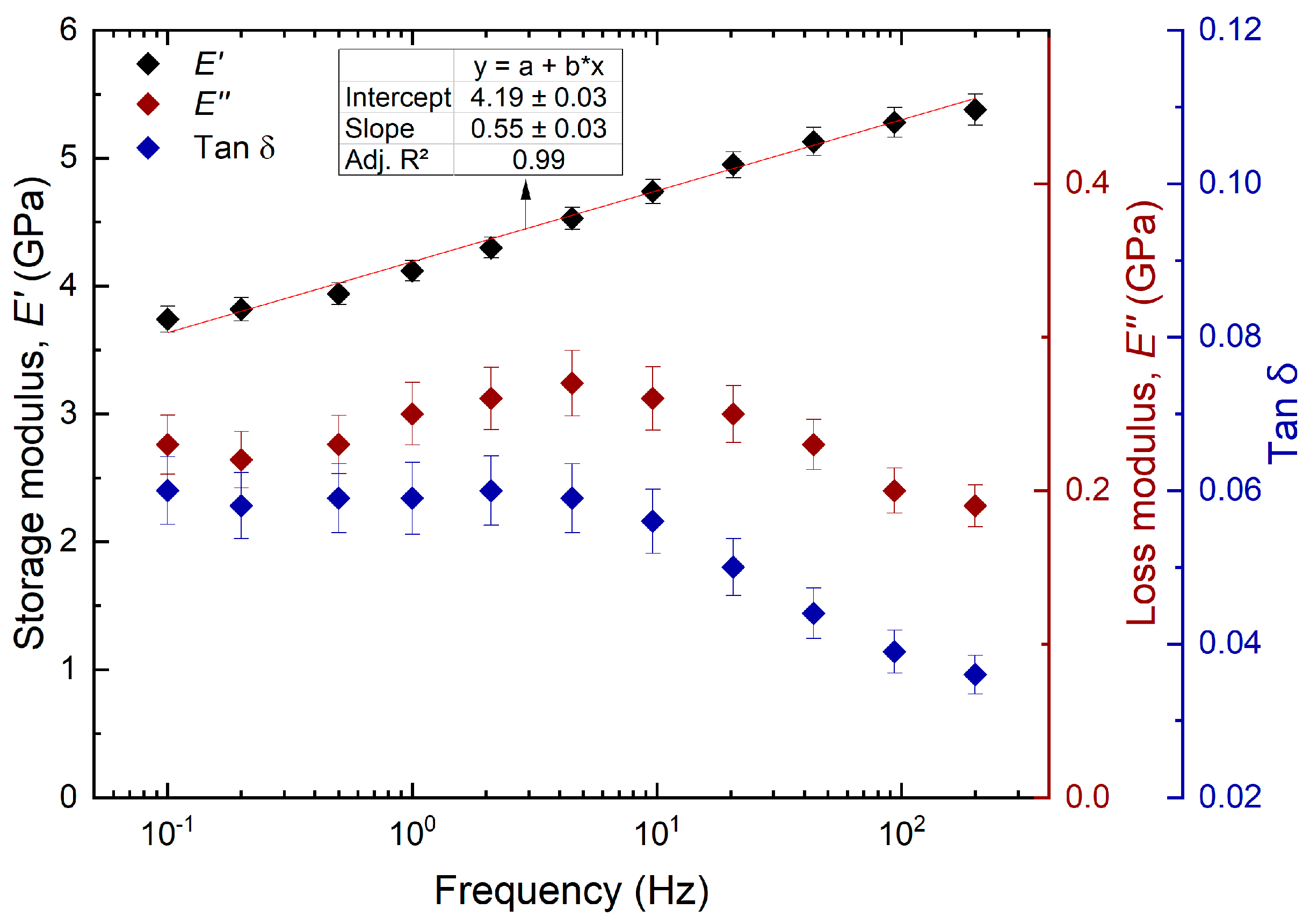
3.2. NanoDMA
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bergander, A.; Salmén, L. Cell Wall Properties and Their Effects on the Mechanical Properties of Fibers. J. Mater. Sci. 2002, 37, 151–156. [Google Scholar] [CrossRef]
- Winandy, J.E.; Rowell, R.M. Chemistry of Wood Strength; CRC Press: Boca Raton, FL, USA, 2012; ISBN 9781439853818. [Google Scholar]
- Kaboorani, A.; Blanchet, P.; Laghdir, A. A Rapid Method to Assess Viscoelastic and Mechanosorptive Creep in Wood. Wood Fiber Sci. 2013, 45, 370–382. [Google Scholar]
- Fathi, H.; Kazemirad, S.; Nasir, V. A Nondestructive Guided Wave Propagation Method for the Characterization of Moisture-Dependent Viscoelastic Properties of Wood Materials. Mater. Struct. Constr. 2020, 53, 147. [Google Scholar] [CrossRef]
- Sharma, M.; Brennan, M.; Chauhan, S.S.; Entwistle, K.M.; Altaner, C.M.; Harris, P.J. Wood Quality Assessment of Pinus radiata (Radiata pine) Saplings by Dynamic Mechanical Analysis. Wood Sci. Technol. 2015, 49, 1239–1250. [Google Scholar] [CrossRef]
- Pearson, H.; Gabbitas, B.; Ormarsson, S. Tensile Behaviour of Radiata Pine with Different Moisture Contents at Elevated Temperatures. Holzforschung 2012, 66, 659–665. [Google Scholar] [CrossRef]
- Fortino, S.; Metsäjoki, J.; Ronkainen, H.; Bjurhager, I.; Heinemann, S.; Salminen, L.I. Scratch Resistance of PEG-Impregnated Green Wood: A Method for Evaluation of Swollen Wood Properties. Wood Sci. Technol. 2020, 54, 715–735. [Google Scholar] [CrossRef]
- Soares, L.S.Z.R.; Fraga, I.F.; Paula, L.D.S.E.; Arroyo, F.N.; Ruthes, H.C.; Aquino, V.B.D.M.; Molina, J.C.; Panzera, T.H.; Branco, L.A.M.N.; Christoforo, A.L.; et al. Influence of Moisture Content on Physical and Mechanical Properties of Cedrelinga Catenaeformis Wood. BioResources 2021, 16, 6758–6765. [Google Scholar] [CrossRef]
- Olsson, A.-M.; Salmén, L. The Effect of Lignin Composition on the Viscoelastic Properties of Wood. Nord. Pulp Pap. Res. J. 1997, 12, 140–144. [Google Scholar] [CrossRef]
- Hu, W.; Chen, B.; Zhang, T. Experimental and Numerical Studies on Mechanical Behaviors of Beech Wood under Compressive and Tensile States. Wood Res. 2021, 66, 27–38. [Google Scholar] [CrossRef]
- Nowak, T.; Patalas, F.; Karolak, A. Estimating Mechanical Properties of Wood in Existing Structures—Selected Aspects. Materials 2021, 14, 1941. [Google Scholar] [CrossRef]
- Eder, M.; Arnould, O.; Dunlop, J.W.C.; Hornatowska, J.; Salmén, L. Experimental Micromechanical Characterisation of Wood Cell Walls. Wood Sci. Technol. 2013, 47, 163–182. [Google Scholar] [CrossRef]
- Gindl, W.; Gupta, H.S.; Schöberl, T.; Lichtenegger, H.C.; Fratzl, P. Mechanical Properties of Spruce Wood Cell Walls by Nanoindentation. Appl. Phys. A Mater. Sci. Process. 2004, 79, 2069–2073. [Google Scholar] [CrossRef]
- Gacitua, W.; Bahr, D.; Wolcott, M. Damage of the Cell Wall during Extrusion and Injection Molding of Wood Plastic Composites. Compos. Part A Appl. Sci. Manuf. 2010, 41, 1454–1460. [Google Scholar] [CrossRef]
- Wagner, L.; Bader, T.K.; De Borst, K. Nanoindentation of Wood Cell Walls: Effects of Sample Preparation and Indentation Protocol. J. Mater. Sci. 2014, 49, 94–102. [Google Scholar] [CrossRef]
- Li, J.; Yu, Y.; Feng, C.; Wang, H. Mechanical Characterization of Pinus massoniana Cell Walls Infected by Blue-Stain Fungi Using in Situ Nanoindentation. J. For. Res. 2020, 31, 661–665. [Google Scholar] [CrossRef]
- Vincent, M.; Tong, Q.; Terziev, N.; Daniel, G.; Bustos, C.; Escobar, W.G.; Duchesne, I. A Comparison of Nanoindentation Cell Wall Hardness and Brinell Wood Hardness in Jack Pine (Pinus banksiana Lamb.). Wood Sci. Technol. 2014, 48, 7–22. [Google Scholar] [CrossRef]
- Jakes, J.E.; Stone, D.S. Best Practices for Quasistatic Berkovich Nanoindentation of Wood Cell Walls. Forests 2021, 12, 1696. [Google Scholar] [CrossRef]
- Oliver, W.; Pharr, G. An Improved Technique for Determining Hardness and Elastic Modulus Using Load and Displacement Sensing Indentation Experiments. J. Mater. Res. 1992, 7, 1564–1583. [Google Scholar] [CrossRef]
- Qin, L.; Lin, L.; Fu, F.; Fan, M. Micromechanical Properties of Wood Cell Wall and Interface Compound Middle Lamella Using Quasi-Static Nanoindentation and Dynamic Modulus Mapping. J. Mater. Sci. 2018, 53, 549–558. [Google Scholar] [CrossRef]
- Prošek, Z.; Topič, J.; Tesárek, P.; Indrová, K.; Nežerka, V.; Králik, V.; Klapálek, P. Micromechanical Properties of Spruce Tissues Using Static Nanoindentation and Modulus Mapping. Appl. Mech. Mater. 2015, 732, 115–118. [Google Scholar] [CrossRef]
- Xing, D.; Wang, X.; Wang, S. Temperature-Dependent Creep Behavior and Quasi-Static Mechanical Properties of Heat-Treatedwood. Forests 2021, 12, 968. [Google Scholar] [CrossRef]
- Muñoz, F.; Valenzuela, P.; Gacitúa, W. Eucalyptus Nitens: Nanomechanical Properties of Bark and Wood Fibers. Appl. Phys. A Mater. Sci. Process. 2012, 108, 1007–1014. [Google Scholar] [CrossRef]
- Schuh, C.A. Nanoindentation Studies of Materials. Mater. Today 2006, 9, 32–40. [Google Scholar] [CrossRef]
- Hayes, S.A.; Goruppa, A.A.; Jones, F.R. Dynamic Nanoindentation as a Tool for the Examination of Polymeric Materials. J. Mater. Res. 2004, 19, 3298–3306. [Google Scholar] [CrossRef]
- Jakes, J.E.; Lakes, R.S.; Stone, D.S. Broadband Nanoindentation of Glassy Polymers: Part I. Viscoelasticity. J. Mater. Res. 2012, 27, 463–474. [Google Scholar] [CrossRef]
- Jakes, J.E.; Lakes, R.S.; Stone, D.S. Broadband Nanoindentation of Glassy Polymers: Part II. Viscoplasticity. J. Mater. Res. 2012, 27, 475–484. [Google Scholar] [CrossRef]
- Nayar, V.T.; Weiland, J.D.; Nelson, C.S.; Hodge, A.M. Elastic and Viscoelastic Characterization of Agar. J. Mech. Behav. Biomed. Mater. 2012, 7, 60–68. [Google Scholar] [CrossRef]
- Placet, V.; Passard, J.; Perré, P. Viscoelastic Properties of Green Wood across the Grain Measured by Harmonic Tests in the Range 0–95 °C: Hardwood vs. Softwood and Normal Wood vs. Reaction Wood. Holzforschung 2007, 61, 548–557. [Google Scholar] [CrossRef]
- Łukomski, M.; Bridarolli, A.; Fujisawa, N. Nanoindentation of Historic and Artists’ Paints. Appl. Sci. 2022, 12, 1018. [Google Scholar] [CrossRef]
- Chakravartula, A.; Komvopoulos, K. Viscoelastic Properties of Polymer Surfaces Investigated by Nanoscale Dynamic Mechanical Analysis. Appl. Phys. Lett. 2006, 88, 7–22. [Google Scholar] [CrossRef]
- Venugopal, G.; Veetil, J.C.; Raghavan, N.; Singh, V.; Kumar, A.; Mukkannan, A. Nano-Dynamic Mechanical and Thermal Responses of Single-Walled Carbon Nanotubes Reinforced Polymer Nanocomposite Thinfilms. J. Alloys Compd. 2016, 688, 454–459. [Google Scholar] [CrossRef]
- Liu, K.; Ostadhassan, M.; Bubach, B.; Dietrich, R.; Rasouli, V. Nano-Dynamic Mechanical Analysis (Nano-DMA) of Creep Behavior of Shales: Bakken Case Study. J. Mater. Sci. 2018, 53, 4417–4432. [Google Scholar] [CrossRef]
- Mallikarjunachari, G.; Ghosh, P. Pile-up Response of Polymer Thin Films to Static and Dynamic Loading. Thin Solid Films 2019, 677, 1–12. [Google Scholar] [CrossRef]
- Panchal, V.; Dobryden, I.; Hangen, U.D.; Simatos, D.; Spalek, L.J.; Jacobs, I.E.; Schweicher, G.; Claesson, P.M.; Venkateshvaran, D. Mechanical Properties of Organic Electronic Polymers on the Nanoscale. Adv. Electron. Mater. 2022, 8, 2101019. [Google Scholar] [CrossRef]
- Zhang, T.; Bai, S.L.; Zhang, Y.F.; Thibaut, B. Viscoelastic Properties of Wood Materials Characterized by Nanoindentation Experiments. Wood Sci. Technol. 2012, 46, 1003–1016. [Google Scholar] [CrossRef][Green Version]
- Wang, X.; Zhao, L.; Xu, B.; Li, Y.; Wang, S.; Deng, Y. Effects of Accelerated Aging Treatment on the Microstructure and Mechanics of Wood-Resin Interphase. Holzforschung 2017, 72, 235–241. [Google Scholar] [CrossRef]
- Prošek, Z.; Králík, V.; Topic, J.; Nežerka, V.; Indrová, K.; Tesárek, P. A Description of the Microstructure and the Micromechanical Properties of Spruce Wood. Acta Polytech. 2015, 55, 39–49. [Google Scholar] [CrossRef]
- Prošek, Z.; Topič, J.; Tesárek, P.; Nežerka, V.; Králik, V. Modulus Mapping and Its Use to Determine the Effect Process of Drying on the Cells of Spruce. Key Eng. Mater. 2016, 714, 25–28. [Google Scholar] [CrossRef]
- Wang, X.; Chen, X.; Xie, X.; Yuan, Z.; Cai, S.; Li, Y. Effect of Phenol Formaldehyde Resin Penetration on the Quasi-Static and Dynamic Mechanics Ofwood Cellwalls Using Nanoindentation. Nanomaterials 2019, 9, 1409. [Google Scholar] [CrossRef]
- Li, Y.; Yin, L.; Huang, C.; Meng, Y.; Fu, F.; Wang, S.; Wu, Q. Quasi-Static and Dynamic Nanoindentation to Determine the Influence of Thermal Treatment on the Mechanical Properties of Bamboo Cell Walls. Holzforschung 2015, 69, 909–914. [Google Scholar] [CrossRef]
- Lakes, R. Viscoelastic Materials; Cambridge University Press: New York, NY, USA, 2009. [Google Scholar]
- Jakes, J.E. Mechanism for Diffusion through Secondary Cell Walls in Lignocellulosic Biomass. J. Phys. Chem. B 2019, 123, 4333–4339. [Google Scholar] [CrossRef]
- CONAF Plantaciones Forestales. Available online: https://www.conaf.cl/nuestros-bosques/plantaciones-forestales/ (accessed on 18 April 2023).
- ASTM D2395:14; Standard Test Methods for Density and Specific Gravity (Relative Density) of Wood and Wood-Based Materials. American Society for Testing Materials: West Conshohocken, PA, USA, 2014; Volume 93, pp. 1–13. [CrossRef]
- Sedighi-Gilani, M.; Sunderland, H.; Navi, P. Within-Fiber Nonuniformities of Microfibril Angle. Wood Fiber Sci. 2006, 38, 132–138. [Google Scholar]
- Meng, Y.; Wang, S.; Cai, Z.; Young, T.M.; Du, G.; Li, Y. A Novel Sample Preparation Method to Avoid Influence of Embedding Medium during Nano-Indentation. Appl. Phys. A Mater. Sci. Process. 2012, 110, 361–369. [Google Scholar] [CrossRef]
- Jakes, J.E.; Frihart, C.R.; Beecher, J.F.; Moon, R.J.; Stone, D.S. Experimental Method to Account for Structural Compliance in Nanoindentation Measurements. J. Mater. Res. 2008, 23, 1113–1127. [Google Scholar] [CrossRef]
- Jakes, J.E. Improved Methods for Nanoindentation Berkovich Probe Calibrations Using Fused Silica. J. Mater. Sci. 2018, 53, 4814–4827. [Google Scholar] [CrossRef]
- Stone, D.S.; Yoder, K.B.; Sproul, W.D. Hardness and Elastic Modulus of TiN Based on Continuous Indentation Technique and New Correlation. J. Vac. Sci. Technol. A Vac. Surf. Film. 1991, 9, 2543–2547. [Google Scholar] [CrossRef]
- Asif, S.A.S.; Pethica, J.B. Nano-Scale Viscoelastic Properties of Polymer Materials. Mater. Res. Soc. Symp. Proc. 1997, 505, 103–108. [Google Scholar] [CrossRef]
- Syed Asif, S.A.; Pethica, J.B. Nanoindentation Creep of Single-Crystal Tungsten and Gallium Arsenide. Philos. Mag. A 1997, 76, 1105–1118. [Google Scholar] [CrossRef]
- Jakes, J.E.; Frihart, C.R.; Beecher, J.F.; Moon, R.J.; Resto, P.J.; Melgarejo, Z.H.; Suárez, O.M.; Baumgart, H.; Elmustafa, A.A.; Stone, D.S. Nanoindentation near the Edge. J. Mater. Res. 2009, 24, 1016–1031. [Google Scholar] [CrossRef]
- Baradit, E.; Fuentealba, C.; Yáñez, M. Elastic Constants of Chilean Pinus radiata Using Ultrasonic. Maderas Cienc. y Tecnol. 2021, 23, 1–10. [Google Scholar] [CrossRef]
- Herbert, E.G.; Oliver, W.C.; Pharr, G.M. Nanoindentation and the Dynamic Characterization of Viscoelastic Solids. J. Phys. D Appl. Phys. 2008, 41, 074021. [Google Scholar] [CrossRef]
- Jäger, A.; Bader, T.; Hofstetter, K.; Eberhardsteiner, J. The Relation between Indentation Modulus, Microfibril Angle, and Elastic Properties of Wood Cell Walls. Compos. Part A Appl. Sci. Manuf. 2011, 42, 677–685. [Google Scholar] [CrossRef]
- Tze, W.T.Y.; Wang, S.; Rials, T.G.; Pharr, G.M.; Kelley, S.S. Nanoindentation of Wood Cell Walls: Continuous Stiffness and Hardness Measurements. Compos. Part A Appl. Sci. Manuf. 2007, 38, 945–953. [Google Scholar] [CrossRef]
- Liu, M.; Lyu, S.; Peng, L.; Fan, Z.; Cai, L.; Huang, Z.; Lyu, J. Study on Properties of Radiata Pine Wood Treated with Furfuryl Alcohol as Fretboard Materials for String Instruments. Eur. J. Wood Wood Prod. 2022, 80, 1185–1200. [Google Scholar] [CrossRef]
- Erazo, O.; Moreno, P.; Valenzuela, P.; Gacitúa, W. Propriedades Nanomecânicas de Pinus radiata D. Don Para Uso Em Produtos de Alto Valor/Nanomechanical Properties of Pinus radiata D. Don for Use in High Value Products. Braz. J. Dev. 2021, 7, 109892–109905. [Google Scholar] [CrossRef]
- Moon, R.J.; Jakes, J.E.; Beecher, J.F.; Frihart, C.R.; Stone, D.S. Relating Nanoindentation to Macroindentation of Wood. In Advanced Biomass Science and Technology for Bio-Based Products; Research, Station; Hse, C., Jiang, Z., Kuo, M.L., Eds.; Chinese Academy of Forestry: Beijing, China; USDA Forest Service: Washington, DC, USA; Southern Research Station: Washington, DC, USA, 2009; pp. 145–160. [Google Scholar]
- Wimmer, R.; Lucas, B. Comparing Mechanical Properties of Secondary Cell Wall and Cell Corner Middle Lamella in Spruce Wood. IAWA J. 1997, 18, 77–88. [Google Scholar] [CrossRef]
- Donaldson, L.A. S3 Lignin Concentration in Radiata Pine Tracheids. Wood Sci. Technol. 1987, 21, 227–234. [Google Scholar] [CrossRef]
- Gindl, W.; Gupta, H.S.; Grünwald, C. Lignification of Spruce Tracheid Secondary Cell Walls Related to Longitudinal Hardness and Modulus of Elasticity Using Nano-Indentation. Can. J. Bot. 2002, 80, 1029–1033. [Google Scholar] [CrossRef]
- Jäger, A.; Hofstetter, K.; Buksnowitz, C.; Gindl-Altmutter, W.; Konnerth, J. Identification of Stiffness Tensor Components of Wood Cell Walls by Means of Nanoindentation. Compos. Part A Appl. Sci. Manuf. 2011, 42, 2101–2109. [Google Scholar] [CrossRef]
- Jiang, J.; Lu, J.; Yan, H. Dynamic Viscoelastic Properties of Wood Treated by Three Drying Methods Measured at High-Temperature Range. Wood Fiber Sci. 2008, 40, 72–79. [Google Scholar]
- Jiang, J.; Lu, J. Impact of Temperature on the Linear Viscoelastic Region of Wood. Can. J. For. Res. 2009, 39, 2092–2099. [Google Scholar] [CrossRef]
- Menard, K.P. Dynamic Mechanical Analysis: A Pratical Introduction; CRC Press: Boca Raton, FL, USA, 1999; ISBN 0849386888. [Google Scholar]
- Hay, J.; Herbert, E. Measuring the Complex Modulus of Polymers by Instrumented Indentation Testing. Exp. Tech. 2013, 37, 55–61. [Google Scholar] [CrossRef]



| Elastic Modulus (GPa) | Storage Modulus (GPa) | |
|---|---|---|
| S2-transverse plane | 15.7 (1.9) | 14.3 (0.62) |
| CCML-transverse plane | 4.6 (0.2) | 4.4 (0.08) |
| S2 longitudinal-tangential plane | 3.9 (0.2) | 3.7 (0.10) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Erazo, O.; Jakes, J.E.; Plaza, N.Z.; Vergara-Figueroa, J.; Valenzuela, P.; Gacitúa, W. Quasistatic and Dynamic Nanoindentation Measurements of Pinus radiata D. Don S2 and CCML Cell Wall Layers. Forests 2023, 14, 1900. https://doi.org/10.3390/f14091900
Erazo O, Jakes JE, Plaza NZ, Vergara-Figueroa J, Valenzuela P, Gacitúa W. Quasistatic and Dynamic Nanoindentation Measurements of Pinus radiata D. Don S2 and CCML Cell Wall Layers. Forests. 2023; 14(9):1900. https://doi.org/10.3390/f14091900
Chicago/Turabian StyleErazo, Oswaldo, Joseph E. Jakes, Nayomi Z. Plaza, Judith Vergara-Figueroa, Paulina Valenzuela, and William Gacitúa. 2023. "Quasistatic and Dynamic Nanoindentation Measurements of Pinus radiata D. Don S2 and CCML Cell Wall Layers" Forests 14, no. 9: 1900. https://doi.org/10.3390/f14091900
APA StyleErazo, O., Jakes, J. E., Plaza, N. Z., Vergara-Figueroa, J., Valenzuela, P., & Gacitúa, W. (2023). Quasistatic and Dynamic Nanoindentation Measurements of Pinus radiata D. Don S2 and CCML Cell Wall Layers. Forests, 14(9), 1900. https://doi.org/10.3390/f14091900








