Influence of the Tree Decay Duration on Mechanical Stability of Norway Spruce Wood (Picea abies (L.) Karst.)
Abstract
1. Introduction
2. Materials and Methods
2.1. Annual Increment
- S—mean width of annual increment [mm],
- N—number of annual rings in the length of the measuring segment [no],
- l—the length of the measuring segment [mm],
2.2. Mechanical Properties of Spruce Wood
- MOR—resistance to bending [MPa],
- a—the distance between the place of loading force and the closest support [m],
- Fmax—breaking load [N],
- W—indicator of cross-section resistance [m3]; (for a rectangle W = bh2/6, where:
- b—width of cross-section of the sample, h—height of cross-section of the sample),
- MOE—modulus of elasticity [MPa],
- l1—distance between pressure and support [mm],
- l2—spacing of supports [mm],
- Pi—load of the range [N],
- P1—initial load [N],
- fi—bending arrow at the load [mm],
- f1—bending arrow forced by initial load [mm],
- b—width of the sample [mm],
- h—height of the sample [mm].
- MOR—static bending resistance [MPa]
- MOE—modulus of elasticity [MPa]
- Y0—sample collected from living trees
- Y1—sample collected from trees 1 year after decay
- Y2—sample collected from trees 2 years after decay
- Y3—sample collected from trees 3 years after decay
- Y4—sample collected from trees 4 years after decay
- Y5—sample collected from trees 5 years after decay
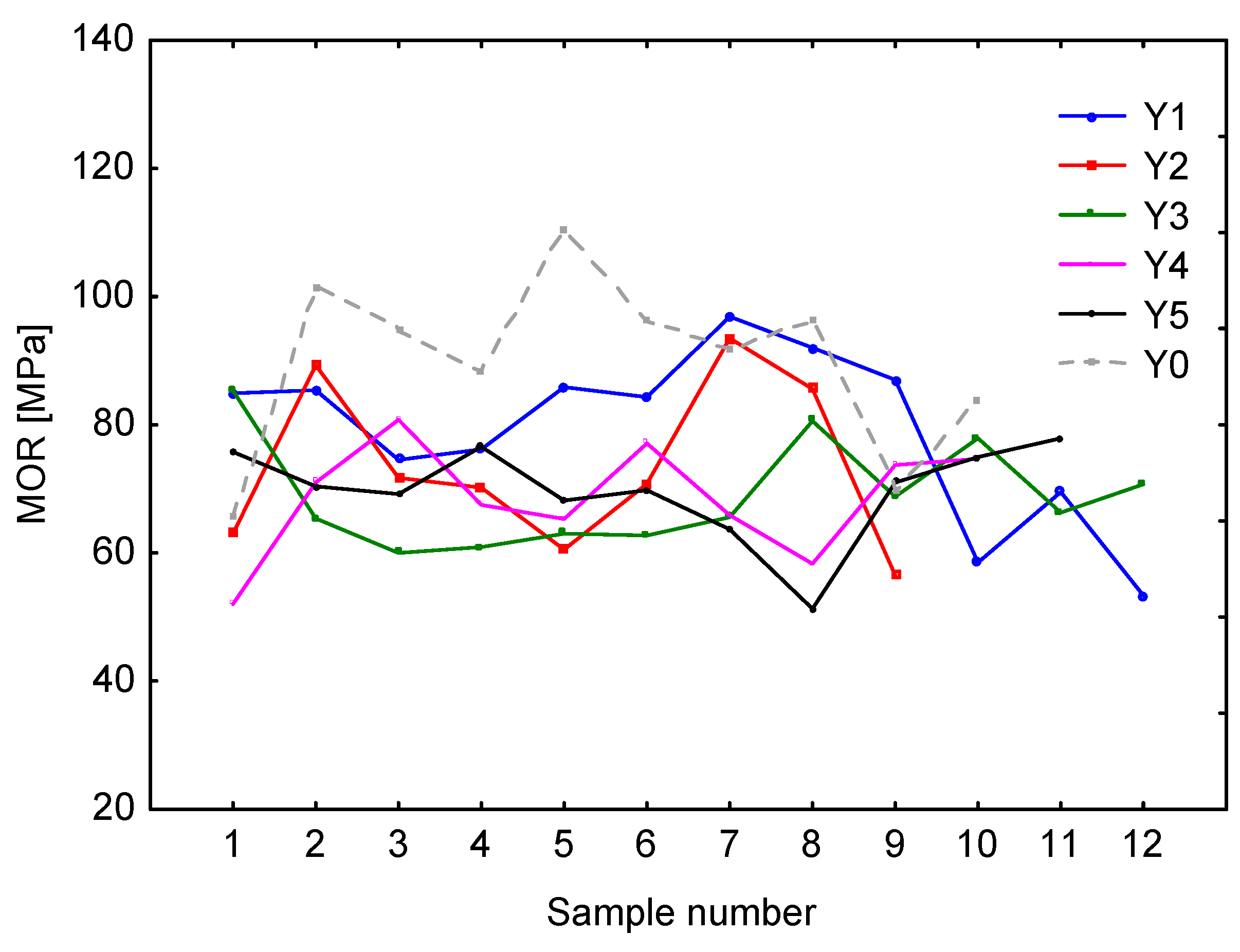
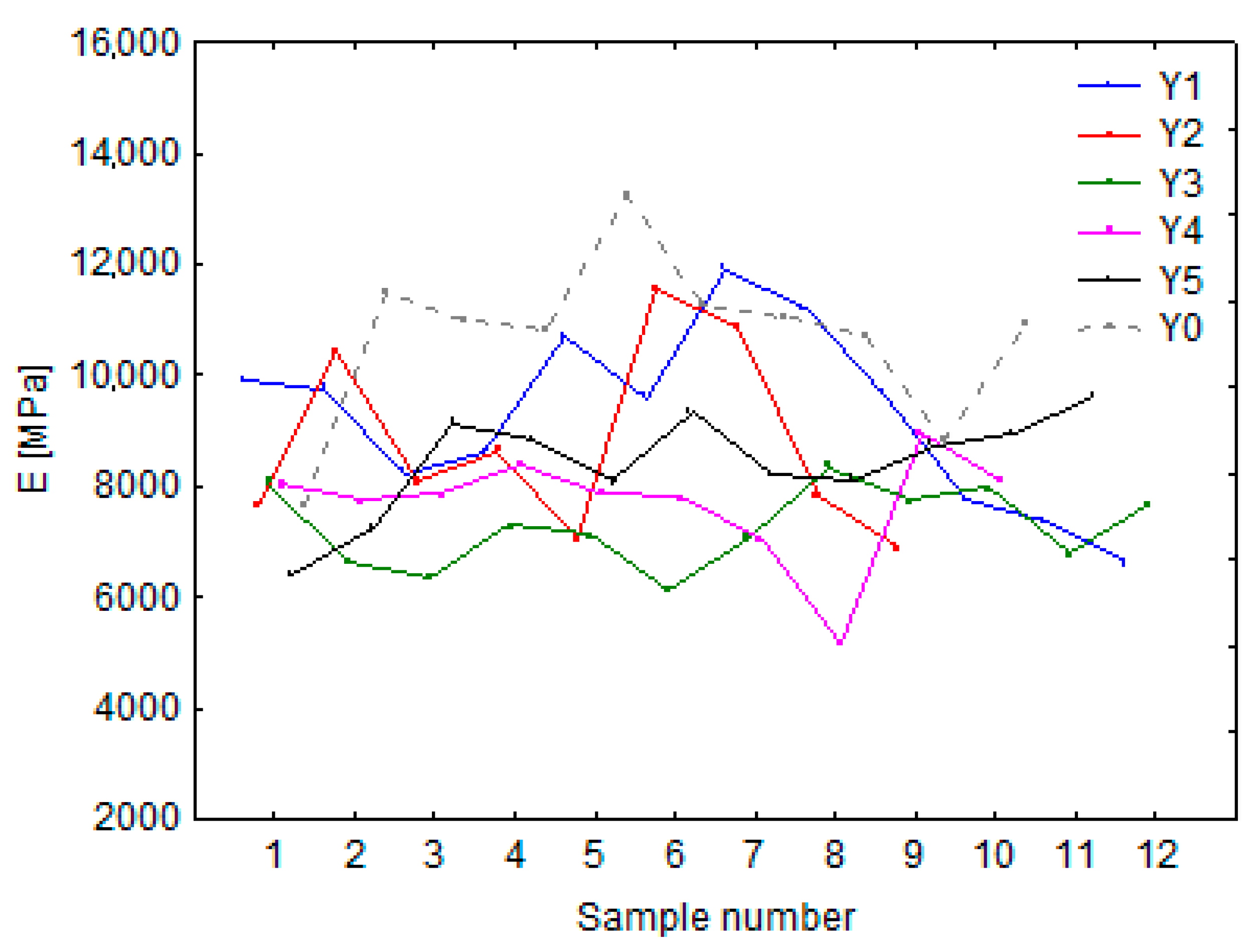
3. Results
4. Discussion
5. Conclusions
- In forests which play a social function, in nature reserves and parks, spruces can be considered potentially hazardous after the 3rd year since their decay and the areas with such trees should be excluded from recreational purposes (touristic purposes).
- In industry tree stands, the areas where dead trees have been decaying for 3 years and more should be excluded from use. Furthermore, in the 1st and 2nd year after the decay, spruce wood can be to some extent used in industry. However, it cannot be used as construction wood, but as fuel wood or left for further decay until total decomposition (dead tree).
Author Contributions
Funding
Conflicts of Interest
References
- Hari, P.; Kulmala, L. (Eds.) Boreal forest and climate change. In Advances in Global Change Research; Springer: Berlin, Germany, 2008; Volume 34, pp. 1–582. [Google Scholar]
- Lac, S.; McHenry, M.P. Climate Change and Forest Ecosystems; Nova Science Publishers: Hauppauge, NY, USA, 2014; ISBN 9781631177491. [Google Scholar]
- Diez Casero, J.J. Forest diseases and global change: Globalisation, climate change and legal issues. Patol. For. y cambio Glob. Glob. Cambio Clim. y Cuest. 2015, 39, 249–258. [Google Scholar]
- Hepting, G.H. Climate and forest diseases. Ann. Rev. Phytopathol. 1963, 1, 31–50. [Google Scholar] [CrossRef]
- Frankel, S.; Juzwik, J.; Koch, F. Forest Tree Diseases and Climate Change. Available online: http://www.fs.fed.us/ccrc/topics/forest-disease/ (accessed on 22 May 2020).
- Svoboda, M.; Fraver, S.; Janda, P.; Bače, R.; Zenáhlíková, J. Natural development and regeneration of a Central European montane spruce forest. For. Ecol. Manag. 2010. [Google Scholar] [CrossRef]
- Brůna, J.; Wild, J.; Svoboda, M.; Heurich, M.; Müllerová, J. Impacts and underlying factors of landscape-scale, historical disturbance of mountain forest identified using archival documents. For. Ecol. Manag. 2013. [Google Scholar] [CrossRef]
- Intergovernmental Panel on Climate Change (IPCC). IPCC Climate Change and Land: Summary for Policymakers; Intergovernmental Panel on Climate Change (IPCC): Geneva, Switzerland, 2019. [Google Scholar]
- Kellomäki, S.; Strandman, H.; Heinonen, T.; Asikainen, A.; Venäläinen, A.; Peltola, H. Temporal and spatial change in diameter growth of boreal scots pine, Norway spruce, and birch under recent-generation (CMIP5) global climate model projections for the 21st century. Forests 2018, 9, 118. [Google Scholar] [CrossRef]
- Briceño-Elizondo, E.; Garcia-Gonzalo, J.; Peltola, H.; Matala, J.; Kellomäki, S. Sensitivity of growth of Scots pine, Norway spruce and silver birch to climate change and forest management in boreal conditions. For. Ecol. Manag. 2006. [Google Scholar] [CrossRef]
- Hartl-Meier, C.; Zang, C.; Ditmar, C.; Esper, J.; Göttlein, A.; Rothe, A. Vulnerability of Norway spruceto climate change in mountain forests of the European Alps. Clim Res. 2014. [Google Scholar] [CrossRef]
- Jyske, T.; Mäkinen, H.; Saranpää, P. Wood density within Norway spruce stems. Silva Fenn. 2008. [Google Scholar] [CrossRef]
- Wimmer, R.; Grabner, M. A comparison of tree-ring features in Picea abies as correlated with climate. IAWA J. 2000. [Google Scholar] [CrossRef]
- Bunyard, P. The death of the trees. Ecologist 1986, 16, 4–14. [Google Scholar]
- Kohlmaier, G.H.; Siré, E.O.; Bröhl, H.; Kilian, W.; Fischbach, U.; Plöchl, M.; Müller, T.; Yunsheng, J. Dramatic development in the dying of German spruce-fir forests: In search of possible cause-effect relationships. Ecol. Modell. 1984. [Google Scholar] [CrossRef]
- Hinrichsen, D. The forest decline enigma: What underlies extensive dieback on two continents? Bioscience 1987, 37, 542–546. [Google Scholar] [CrossRef]
- Šrámek, V.; Vejpustková, M.; Novotný, R.; Hellebrandová, K. Yellowing of Norway spruce stands in the Silesian Beskids–damage extent and dynamics. J. For. Sci. 2008, 54, 55–63. [Google Scholar]
- Karanikola, P.; Tampakis, S.; Rantzoudi, E. Evaluation of the problems created by trees and bushes to the urban environment. J. Environ. Prot. Ecol. 2008, 8, 698–709. [Google Scholar]
- Egan, A.; Alerich, C. “Danger rees” in Central Appalachian Forests of the United States. J. Safety Res. 1998. [Google Scholar] [CrossRef]
- James, K.R.; Haritos, N.; Ades, P.K. Mechanical stability of trees under dynamic loads. Am. J. Bot. 2006. [Google Scholar] [CrossRef]
- Honkaniemi, J.; Lehtonen, M.; Väisänen, H.; Peltola, H. Effects of wood decay by heterobasidion annosum on the vulnerability of Norway spruce stands to wind damage: A mechanistic modelling approach. Can. J. For. Res. 2017. [Google Scholar] [CrossRef]
- McLean, J.P. Wood properties of four genotypes of Sitka spruce. Ph.D. Thesis, Department of Analytical and Environmental Chemistry, University of Glasgow, Glasgow, UK, 2008. [Google Scholar]
- Treacy, M.; Dhubháin, A.N.; Evertsen, J. The influence of microfibril angle on modulus of elasticity and modulus of rupture in four provenances of Irish grown Sitka spruce (Picea sitchensis (Bong.) Carr). J. Inst. Wood Sci. 2000, 15, 211–220. [Google Scholar]
- McLean, J.P.; Evans, R.; Moore, J.R. Predicting the longitudinal modulus of elasticity of Sitka spruce from cellulose orientation and abundance. Holzforschung 2010. [Google Scholar] [CrossRef]
- Verkasalo, E.; Leban, J.M. MOE and MOR in static bending of small clear specimens of Scots pine, Norway spruce and European fir from Finland and France and their prediction for the comparison of wood quality. Pap. ja Puu/Paper Timber 2002, 84, 332–341. [Google Scholar]
- Bacher, M.; Krzosek, S. Modulus of elasticity tension/bending ratio of polish grown pine (Pinus sylvestris L.) and spruce (Picea abies Karst.) timber. Ann. Warsaw Univ. Life Sci.—SGGW. For. Wood Technol. 2013, 82, 31–38. [Google Scholar]
- Lavers, G.M. The Strength Properties of Timber; Building Research Establishment Report; HMSO: London, UK, 1983. [Google Scholar]
- Bruchwald, A.; Dmytrenko, E. Zastosowanie modeli ryzyka uszkodzenia drzewostanu przez wiatr do oceny zagrożenia lasów nadleśnictwa. Sylwan 2011, 155, 459–471. [Google Scholar]
- Gardiner, B.; Byrne, K.; Hale, S.; Kamimura, K.; Mitchell, S.J.; Peltola, H.; Ruel, J.C. A review of mechanistic modelling of wind damage risk to forests. Forestry 2008, 81, 447–463. [Google Scholar] [CrossRef]
- Hale, S.A.; Gardiner, B.; Peace, A.; Nicoll, B.; Taylor, P.; Pizzirani, S. Comparison and validation of three versions of a forest wind risk model. Environ. Model. Softw. 2015. [Google Scholar] [CrossRef]
- Heinonen, T.; Pukkala, T.; Ikonen, V.P.; Peltola, H.; Venäläinen, A.; Dupont, S. Integrating the risk of wind damage into forest planning. For. Ecol. Manag. 2009. [Google Scholar] [CrossRef]
- Zeng, H.; Pukkala, T.; Peltola, H. The use of heuristic optimization in risk management of wind damage in forest planning. For. Ecol. Manag. 2007. [Google Scholar] [CrossRef]
- Locatelli, T.; Gardiner, B.; Tarantola, S.; Nicoll, B.; Bonnefond, J.M.; Garrigou, D.; Kamimura, K.; Patenaude, G. Modelling wind risk to Eucalyptus globulus (Labill.) stands. For. Ecol. Manag. 2016. [Google Scholar] [CrossRef]
- Lekes, V.; Dandul, I. Using airflow modelling and spatial analysis for defining wind damage risk classification (WINDARC). For. Ecol. Manag. 2000, 15, 331–344. [Google Scholar] [CrossRef]
- Talkkari, A.; Peltola, H.; Kellomäki, S.; Strandman, H. Integration of component models from the tree, stand and regional levels to assess the risk of wind damage at forest margins. For. Ecol. Manag. 2000, 15, 303–313. [Google Scholar] [CrossRef]
- Schütz, J.P.; Götz, M.; Schmid, W.; Mandallaz, D. Vulnerability of spruce (Picea abies) and beech (Fagus sylvatica) forest stands to storms and consequences for silviculture. Eur. J. For. Res. 2006. [Google Scholar] [CrossRef]
- Hale, S.A.; Gardiner, B.A.; Wellpott, A.; Nicoll, B.C.; Achim, A. Wind loading of trees: Influence of tree size and 522 competition. Eur. J. For. Res. 2012, 131, 203–217. [Google Scholar] [CrossRef]
- Gardiner, B.A.; Quine, C.P. Management of forests to reduce the risk of abiotic damage—A review with particular reference to the effects of strong winds. For. Ecol. Manag. 2000, 135, 261–277. [Google Scholar] [CrossRef]
- Blennow, K.; Andersson, M.; Sallnäs, O.; Olofsson, E. Climate change and the probability of wind damage in two Swedish forests. For. Ecol. Manag. 2010. [Google Scholar] [CrossRef]
- Peltola, H.M. Mechanical stability of trees under static loads. Am. J. Bot. 2006, 2006. 93, 11–1501. [Google Scholar] [CrossRef]
- Ancelin, P.; Courbaud, B.; Fourcaud, T. Development of an individual tree-based mechanical model to predict 483 wind damage within forest stands. For. Ecol. Manag. 2004, 203, 101–121. [Google Scholar] [CrossRef]
- Green, D.; Winandy, J.; Kretschmann, D. Mechanical Properties of Wood; U.S. Forest Service: Washington, DC, USA, 1999. [Google Scholar]
- Loehle, C. Biomechanical constraints on tree architecture. Trees Struct. Funct. 2016. [Google Scholar] [CrossRef]
- Achim, A.; Ruel, J.C.; Gardiner, B.A.; Laflamme, G.; Meunier, S. Modelling the vulnerability of balsam fir forests to wind damage. For. Ecol. Manag. 2005. [Google Scholar] [CrossRef]
- Kretschmann, D.E. Mechanical Properties of Wood. Wood Handbook as an Engineering Materials; U.S. Forest Service: Washington, DC, USA, 2010. [Google Scholar]
- Xu, P.; Donaldson, L.; Walker, J.; Evans, R.; Downes, G. Effects of density and microfibril orientation on the vertical variation of low-stiffness wood in radiata pine butt logs. Holzforschung 2004. [Google Scholar] [CrossRef]
- Kärenlampi, P.P.; Riekkinen, M. Maturity and growth rate effects on Scots pine basic density. Wood Sci. Technol. 2004. [Google Scholar] [CrossRef]
- Roszyk, E.; Moliński, W.; Fabisiak, E. Radial variation of mechanical properties of pine wood (Pinus sylvestris L.) determined upon tensile stress. Wood Res. 2013, 58, 329–342. [Google Scholar]
- Aleinikovas, M. Effect of the mean diameter increment on the pine wood mechanical-physical properties in Lithuania. Balt. For. 2007, 13, 103–107. [Google Scholar]
- Mellerowicz, E.J.; Sundberg, B. Wood cell walls: Biosynthesis, developmental dynamics and their implications for wood properties. Curr. Opin. Plant Biol. 2008, 11, 293–300. [Google Scholar] [CrossRef] [PubMed]
- Gibson, L.J. The hierarchical structure and mechanics of plant materials. J. R. Soc. Interface 2012, 9, 2749–2766. [Google Scholar] [CrossRef] [PubMed]


| Group | Tree Number | Height [m] | DBH [cm] | Average Age of Sample Trees | Forest Site Type | GPS (WGS-84) | Mean Precipitation [mm/year] |
|---|---|---|---|---|---|---|---|
| Y0 | 1 | 31.50 | 42.5 | 95 * | Vaccinio myrtilli-Piceetum | 52°75′39.570″N, 23°75′23.920″E | 593 * |
| 2 | 30.60 | 41.5 | |||||
| 3 | 29.50 | 39.0 | |||||
| Y1 | 1 | 32.00 | 43.0 | ||||
| 2 | 31.30 | 42.0 | |||||
| 3 | 30.20 | 40.5 | |||||
| Y2 | 1 | 31.60 | 41.5 | ||||
| 2 | 30.50 | 40.0 | |||||
| 3 | 29.80 | 38.5 | |||||
| Y3 | 1 | 32.20 | 43.5 | ||||
| 2 | 30.90 | 41.0 | |||||
| 3 | 29.80 | 38.5 | |||||
| Y4 | 1 | 31.50 | 42.5 | ||||
| 2 | 30.50 | 41.0 | |||||
| 3 | 30.10 | 39.5 | |||||
| Y5 | 1 | 31.90 | 43.5 | ||||
| 2 | 31.20 | 42.0 | |||||
| 3 | 30.10 | 40.5 |
| Variable | Year | Mean | SD | SE | Q25 | Median | Q75 |
|---|---|---|---|---|---|---|---|
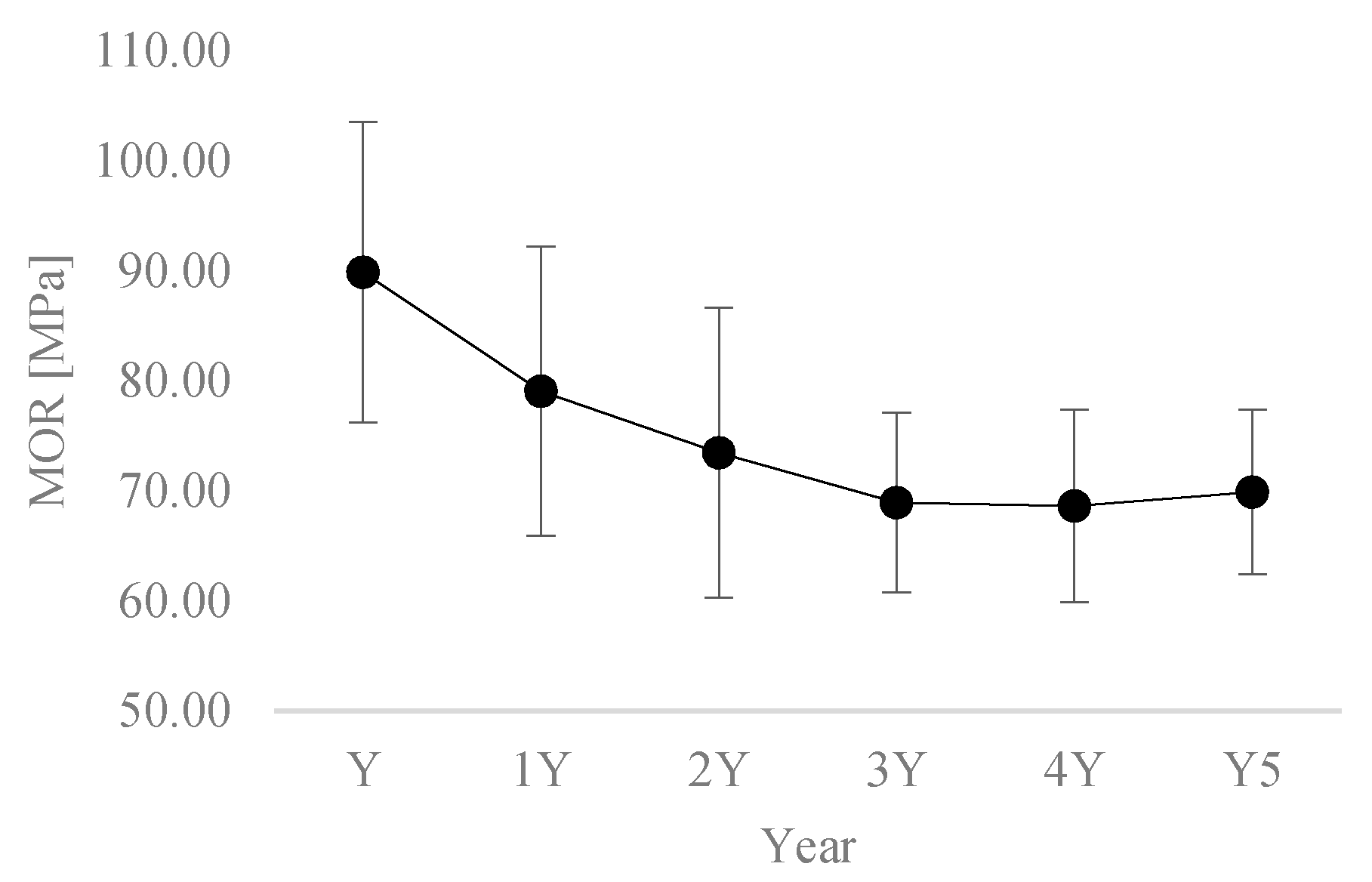
| MOR [Mpa] | Y0 | 89.85 * | 13.65 | 4.32 | 83.90 | 93.25 | 96.30 |
| Y1 | 79.04 | 13.14 | 3.79 | 72.00 | 84.60 | 86.45 | |
| Y2 | 73.46 | 13.16 | 4.39 | 63.00 | 70.80 | 85.60 | |
| Y3 | 68.93 * | 8.18 | 2.36 | 62.85 | 65.95 | 74.30 | |
| Y4 | 68.63 * | 8.76 | 2.77 | 65.30 | 69.25 | 74.70 | |
| Y5 | 69.90 * | 7.48 | 2.25 | 68.20 | 70.40 | 75.80 | |
| Mean | 74.85 | 12.91 | 1.61 | 65.70 | 71.35 | 85.10 | |
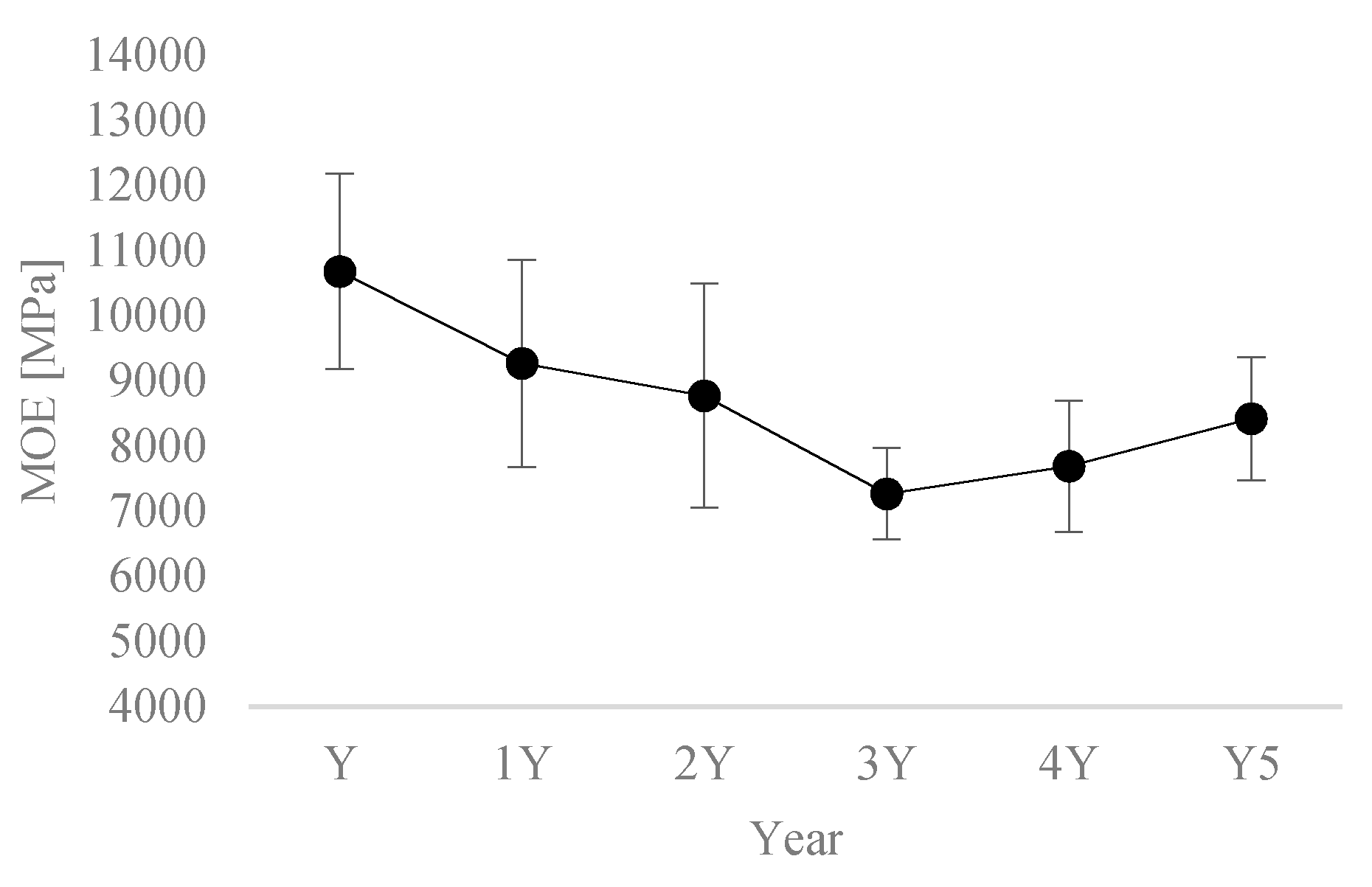
| MOE [Mpa] | Y0 | 10,679 * | 1501 | 475 | 10,686 | 10,941 | 11,269 |
| Y1 | 9269 | 1594 | 460 | 8002 | 9609 | 10,286 | |
| Y2 | 8772 | 1723 | 574 | 7669 | 8078 | 10,395 | |
| Y3 | 7269 * | 704 | 203 | 6711 | 7203 | 7854 | |
| Y4 | 7689 * | 1004 | 317 | 7741 | 7861 | 8068 | |
| Y5 | 8419 * | 942 | 284 | 8087 | 8735 | 9132 | |
| Mean | 8652 | 1662 | 208 | 7675 | 8140 | 9701 |
| MOR | |||||||
|---|---|---|---|---|---|---|---|
| Y1 | Y2 | Y3 | Y4 | Y5 | Y0 | ||
| MOE | Y1 | 0.942974 | |||||
| Y2 | 0.961817 | ||||||
| Y3 | 0.625188 | ||||||
| Y4 | 0.408902 | ||||||
| Y5 | 0.144510 | ||||||
| Y0 | 0.950618 | ||||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jelonek, T.; Klimek, K.; Kopaczyk, J.; Wieruszewski, M.; Arasimowicz-Jelonek, M.; Tomczak, A.; Grzywiński, W. Influence of the Tree Decay Duration on Mechanical Stability of Norway Spruce Wood (Picea abies (L.) Karst.). Forests 2020, 11, 980. https://doi.org/10.3390/f11090980
Jelonek T, Klimek K, Kopaczyk J, Wieruszewski M, Arasimowicz-Jelonek M, Tomczak A, Grzywiński W. Influence of the Tree Decay Duration on Mechanical Stability of Norway Spruce Wood (Picea abies (L.) Karst.). Forests. 2020; 11(9):980. https://doi.org/10.3390/f11090980
Chicago/Turabian StyleJelonek, Tomasz, Katarzyna Klimek, Joanna Kopaczyk, Marek Wieruszewski, Magdalena Arasimowicz-Jelonek, Arkadiusz Tomczak, and Witold Grzywiński. 2020. "Influence of the Tree Decay Duration on Mechanical Stability of Norway Spruce Wood (Picea abies (L.) Karst.)" Forests 11, no. 9: 980. https://doi.org/10.3390/f11090980
APA StyleJelonek, T., Klimek, K., Kopaczyk, J., Wieruszewski, M., Arasimowicz-Jelonek, M., Tomczak, A., & Grzywiński, W. (2020). Influence of the Tree Decay Duration on Mechanical Stability of Norway Spruce Wood (Picea abies (L.) Karst.). Forests, 11(9), 980. https://doi.org/10.3390/f11090980








