Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-Ray Densitometry and Ultrasonic Wave Velocity Measurement †
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Materials
2.2. Wood Ring Density and Width Measurement
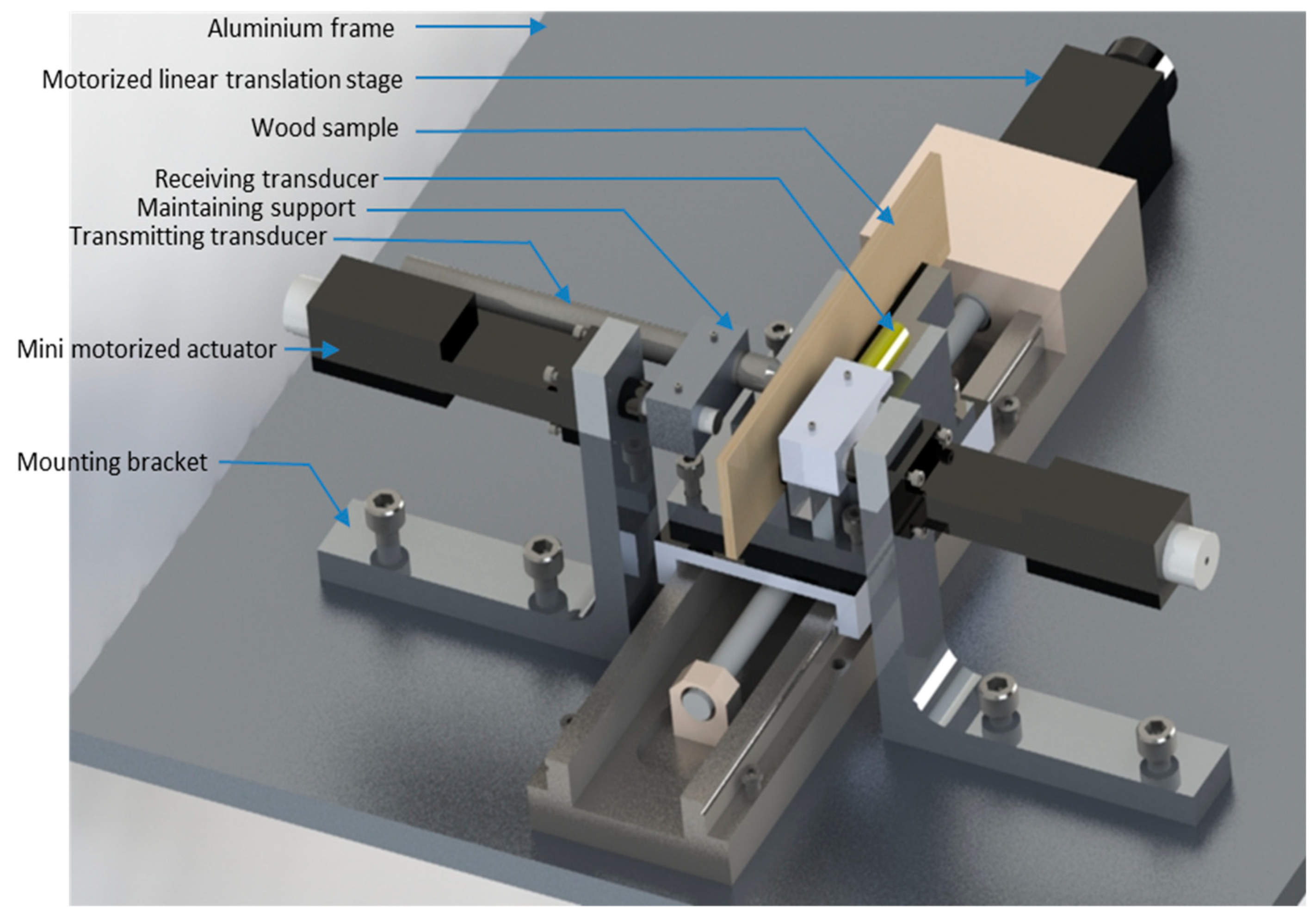
2.3. Wood Ultrasonic Wave Velocity Measurement
2.4. Modelling Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles
2.5. Statistical Analysis
3. Results and Discussion
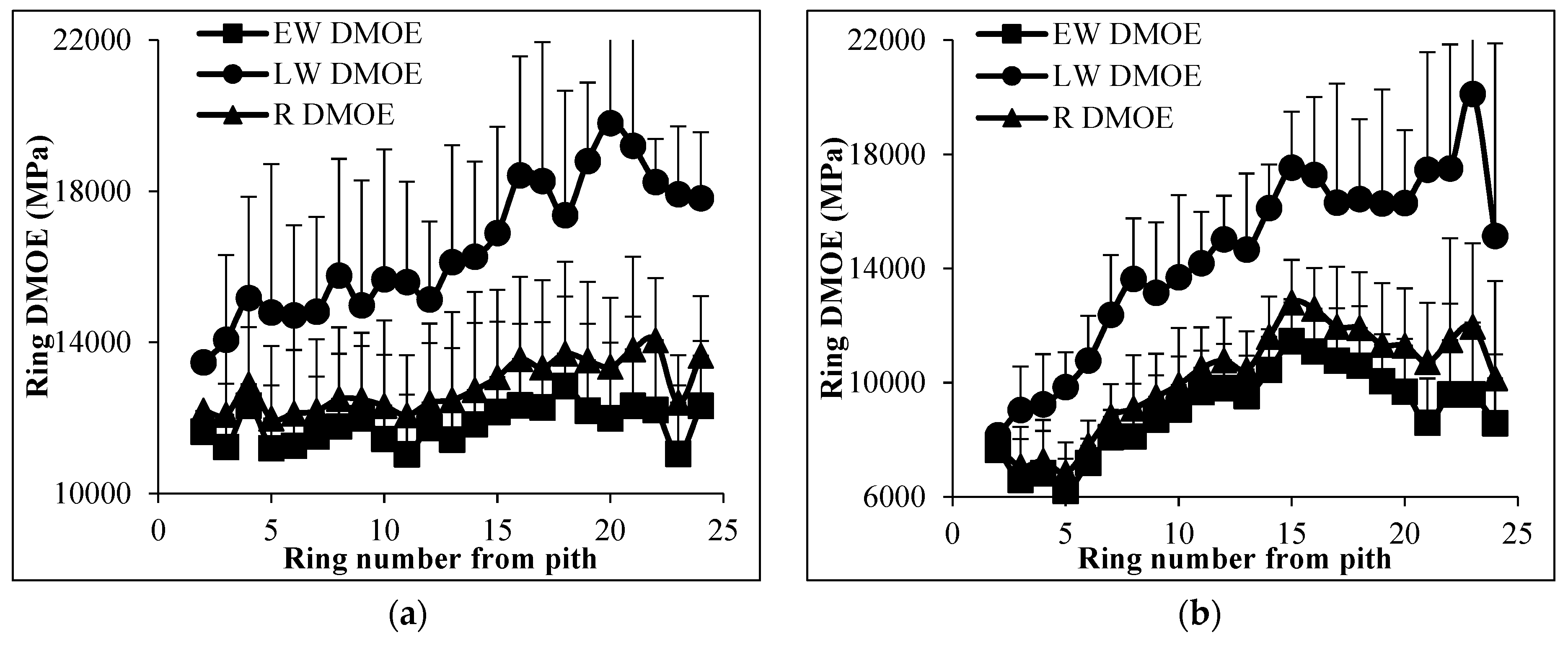
3.1. Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles
3.2. The Earlywood–Latewood Transition
3.3. Radial Variation in Ring Wood Density and Ring Dynamic Modulus of Elasticity
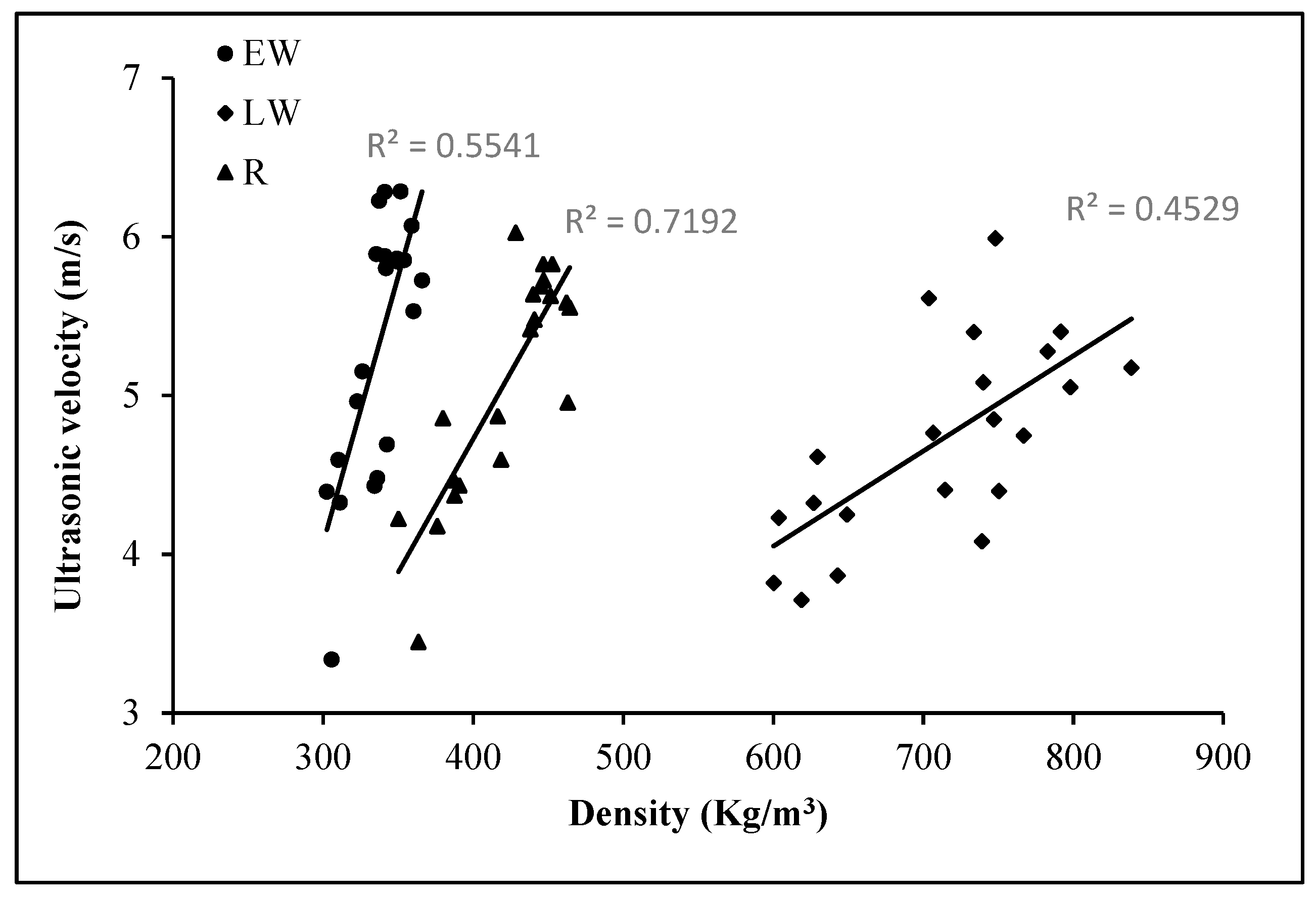
3.4. Relationships between Growth, Density, and Elastic Properties
4. Practical Implications
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Mitchell, H. A Concept of Intrinsic Wood Quality and Nondestructive Methods for Determining Quality in Standing Timber; Forest Service, U.S. Report No 2233; Forest Products Laboratory: Madison, WI, USA, 1961.
- Koubaa, A.; Tony Zhang, S.Y.; Makni, S. Defining the transition from earlywood to latewood in black spruce based on intra-ring wood density profiles from X-ray densitometry. Ann. For. Sci. 2002, 59, 511–518. [Google Scholar] [CrossRef]
- Panshin, A.J.; De Zeeuw, C. Textbook of Wood Technology; McGraw-Hill Book Co: New York, NY, USA, 1980; p. 772. [Google Scholar]
- Rozenberg, P.; Franc, A.; Bastien, C.; Cahalan, C. Improving models of wood density by including genetic effects: A case study in Douglas-Fir. Ann. For. Sci. 2001, 58, 385–394. [Google Scholar] [CrossRef]
- Zhang, S.Y.; Nepveu, G.; Owoundi, R.E. Intratree and intertree variation in selected wood quality characteristics of European oak (Quercus petraea and Quercus robur). Can. J. For. Res. 1994, 24, 1818–1823. [Google Scholar] [CrossRef]
- Bouslimi, B.; Koubaa, A.; Bergeron, Y. Anatomical properties in Thuja occidentalis: Variation and relationship to biological processes. IAWA J. 2014, 35, 363–384. [Google Scholar] [CrossRef]
- Zobel, B.J.; Van Buijtenen, J.P. Wood Variation: Its Causes and Control; Springer: Berlin, Germany, 1989; p. 363. [Google Scholar]
- Pernestal, K.; Jonsson, B.; Larsson, B. A simple model for density of annual rings. Wood Sci. Technol. 1995, 29, 441–449. [Google Scholar] [CrossRef]
- Mork, E. Die qualität des fichtenholzes unterbesonderer rücksichtnahme auf schleif-und papierholz. Papier-Fabrikant 1928, 26, 741–747. [Google Scholar]
- Denne, M.P. Definition of latewood according to Mork (1928). IAWA Bull. 1988, 10, 59–62. [Google Scholar] [CrossRef]
- Evans, R.; Gartside, G.; Downes, G. Present and prospective use of Silviscan for wood microstructure analysis. In Proceedings of the 49th Appita Annual General Conference proceedings 1995, Hobart, Australia, 2–7 April 1995; pp. 91–96. [Google Scholar]
- Barbour, R.J.; Bergqvist, G.; Amundson, C.; Larsson, B.; Johnson, J.A. New methods for evaluating intra-ring X-ray densitometry data: Maximum derivative methods as compared to Mork's index. In Proceedings of the CTIA/IUFRO International Wood Quality Workshop, Quebec, QC, Canada, 18–22 August 1997; p. 61. [Google Scholar]
- Ivkovic, M.; Rosenberg, P. A method for describing and modelling of within-ring wood density distribution in clones of three coniferous species. Ann. For. Sci. 2004, 61, 759–769. [Google Scholar] [CrossRef]
- Bucur, V.; Archer, R.R. Elastic constants for wood by an ultrasonic method. Wood Sci. Technol. 1984, 18, 255–265. [Google Scholar] [CrossRef]
- Hassan, K.T.; Horacek, P.; Tippner, J. Evaluation of stiffness and strength of Scots Pine wood using resonance frequency and ultrasonic techniques. Dynamic test of wood. BioResources 2013, 8, 1634–1645. [Google Scholar] [CrossRef]
- Najafi, S.K.; Bucur, V.; Ebrahimi, G. Elastic constants of particleboard with ultrasonic technique. Mater. Lett. 2005, 59, 2039–2042. [Google Scholar] [CrossRef]
- Brashaw, B.K.; Bucur, V.; Divos, F.; Gonçalves, R.; Lu, J.; Meder, R.; Pellerin, R.F.; Potter, S.; Ross, R.J.; Wang, X.; et al. Nondestructive testing and evaluation of wood: A Worldwide Research Update. For. Prod. J. 2009, 59, 7–13. [Google Scholar]
- Chiu, C.M.; Lin, C.H.; Yang, T.H. Application of nondestructive methods to evaluate mechanical Properties of 32-Year Old Taiwan Incense Cedar (Calocedrus formosana) wood. BioResources 2013, 8, 688–700. [Google Scholar] [CrossRef][Green Version]
- Proto, A.R.; Macri, G.; Bernardini, V.; Russo, D.; Zimbalatti, G. Acoustic evaluation of wood quality with a non destructive method in standing trees: A first survey in Italy. IForest 2017, 10, 700–706. [Google Scholar] [CrossRef]
- Yang, J.L.; Fortin, Y. Evaluating strength properties of Pinus radiata from ultrasonic measurements on increment cores. Holzforschung 2001, 55, 606–610. [Google Scholar] [CrossRef]
- Horáček, P.; Tippner, J. Nondestructive evaluation of static bending properties of Scots Pine wood using stress wave technique. Wood Res. 2012, 57, 359–366. [Google Scholar]
- Park, Y.I.D.; Koubaa, A.; Brais, S.; Mazerolle, M.J. Effects of cambial age and stem height on wood density and growth of jack pine grown in boreal stands. Wood Fib. Sci. 2009, 41, 346–358. [Google Scholar]
- Ourais, M. Variations intra-arbres de la largeur du cerne, de la masse volumique du bois et des propriétés morphologiques des trachéides de l’épinette noire (Picea Mariana (MILL.) B.S.P) avant et après traitements sylvicoles. Master’s Thesis, Université du Québec en Abitibi-Témiscamingue, Rouyn-Noranda, QC, Canada, 2012. [Google Scholar]
- Grabner, M.; Wimmer, R.; Gierlinger, N.; Evans, R.; Downes, G. Heartwood extractives in larch and effects on X-ray densitometry. Can. J. Res. 2005, 35, 2781–2786. [Google Scholar] [CrossRef]
- Hernández, R.; Koubaa, A.; Beaudoin, M.; Fortin, Y. Selected mechanical properties of fast-growing poplar hybrid clones. Wood Fib. Sci. 1998, 30, 138–147. [Google Scholar]
- Koubaa, A.; Isabel, N.; Zhang, S.Y.; Beaulieu, J.; Bousquet, J. Transition from juvenile to mature wood in black spruce (Picea Mariana (Mill.) B.S.P.). Wood Fib. Sci. 2005, 37, 445–455. [Google Scholar]
- Koubaa, A.; Zhang, S.Y.; Isabel, N.; Beaulieu, J.; Bousquet, J. Phenotypic correlations between juvenile-mature wood density and growth in black spruce. Wood Fib. Sci. 2000, 32, 61–71. [Google Scholar]
- Jeong, G.Y.; Zink-Sharp, A.; Hindman, D.P. Tensile properties of earlywood and latewood from lobolly pine (Pinus taeda) using digital image correlation. Wood Fib. Sci. 2009, 41, 51–63. [Google Scholar]
- Roszyk, E.; Molinski, W.; Kaminski, M. Tensile properties along the grains of earlywood and latewood of scots pine (Pinus Sylvestris L.) in dry and wet state. BioResources 2016, 11, 3027–3037. [Google Scholar] [CrossRef][Green Version]
- Cramer, S.; Kretschmann, D.; Lakes, R.; Schmidt, T. Earlywood and latewood elastic properties in loblolly pine. Holzforschung 2005, 59, 531–538. [Google Scholar] [CrossRef]
- Moliński, W.; Roszyk, E.; Puszyński, J. Variation in mechanical properties within individual annual ring of the resonance spruce wood [Picea abies (L.) Karst]. Dev. Ind. 2014, 65, 215–223. [Google Scholar]
- Mott, L.; Groom, L.; Shaler, S. Mechanical properties of individual southern pine fibers. Part II. Comparison of earlywood and latewood fibers with respect to tree height and juvenility. Wood Fib. Sci. 2002, 34, 221–237. [Google Scholar]
- Vehniäinen, A. Single Fiber Properties—A Key to the Characteristic Defibration Patterns from Wood to Paper Fibers. Ph.D. Thesis, Helsinki University of Technology, Helsinki, Finland, 2008. [Google Scholar]
- Jeong, G.Y.; Hindman, D.P. Modeling differently oriented loblly pine strands incorporating variating intraring properties using a stochastic finite element method. Wood Fib. Sci. 2010, 42, 51–61. [Google Scholar]
- Ross, R.J. Nondestructive Evaluation of Wood, 2nd ed.; Forest Products Laboratory, Forest Service, WI: U.S, General Technical Report FPL-GTR-238; Department of Agriculture: Madison, WI, USA, 2015.
- Sales, A.; Candian, M.; De Salles Cardin, V. Evaluation of the mechanical properties of Brazilian lumber (Goupia glabra) by nondestructive techniques. Constr. Build. Mater. 2011, 25, 1450–1454. [Google Scholar] [CrossRef]
- Evans, R.; Ilic, J. Rapid prediction of wood stiffness from microfibril angle and density. For. Prod. J. 2001, 51, 53–57. [Google Scholar]
- Lasserre, J.; Mason, E.G.; Watt, M.S.; Moore, J.R. Influence of initial planting spacing and genotype on microfibril angle, wood density, fiber properties and modulus of elasticity in Pinus radiata D. Don corewood. For. Ecol. Manag. 2009, 258, 1924–1931. [Google Scholar] [CrossRef]
- Russo, D.; Marziliano, P.A.; Macri, G.; Proto, A.R.; Zimbalatti, G.; Lambardi, F. Does Thinning Intensity Affect Wood Quality? An Analysis of Calabrian Pine in Southern Italy Using a Non-Destructive Acoustic Method. Forests 2019, 10, 303. [Google Scholar] [CrossRef]
- Wang, X.; Ross, R.J.; McClellan, M.; Barbour, R.J.; Erickson, J.R.; Forsman, J.W.; McGinnis, G.D. Nondestructive evaluation of standing trees with a stress wave method. Wood Fib. Sci. 2001, 33, 522–533. [Google Scholar]







| Ring from Pith | ||||
|---|---|---|---|---|
| 5 | 10 | 15 | 20 | |
| Wood density profiles | ||||
| Black spruce | ||||
| Average profiles | 0.96 (0.02) | 0.97 (0.02) | 0.96 (0.02) | 0.97(0.02) |
| Range | 0.88–0.99 | 0.91–0.99 | 0.91–0.99 | 0.92–1.00 |
| Jack pine | ||||
| Average profiles | 0.96 (0.02) | 0.95 (0.02) | 0.97 (0.02) | 0.98 (0.01) |
| Range | 0.92–0.98 | 0.90–1.00 | 0.89–0.99 | 0.96–0.99 |
| Dynamic modulus of elasticity profiles | ||||
| Black spruce | ||||
| Average profiles | 0.92 (0.03) | 0.94 (0.04) | 0.95 (0.03) | 0.95 (0.02) |
| Range | 0.82–0.99 | 0.88–0.99 | 0.86–0.99 | 0.91–0.99 |
| Jack pine | ||||
| Average profiles | 0.89 (0.04) | 0.93 (0.02) | 0.93 (0.03) | 0.94 (0.02) |
| Range | 0.80–0.97 | 0.88–0.98 | 0.82–0.99 | 0.91–0.99 |
| Black Spruce | Jack Pine | |||||
|---|---|---|---|---|---|---|
| Ring Number from the Pith | ||||||
| 5 | 10 | 20 | 5 | 10 | 20 | |
| Earlywood width | ||||||
| Av (mm) | 1.32 (0.37) | 1.26 (0.35) | 1.02 (0.20) | 2.65 (0.27) | 2.05 (0.31) | 1.23 (0.32) |
| Ra (mm) | 0.69–1.95 | 0.81–1.88 | 0.72–1.37 | 2.05–3.17 | 1.40–2.74 | 0.87–2.09 |
| Latewood width | ||||||
| Av (mm) | 0.39 (0.12) | 0.30 (0.08) | 0.23 (0.06) | 0.58 (0.06) | 0.50 (0.06) | 0.38 (0.10) |
| Ra (mm) | 0.22–0.61 | 0.15–0.48 | 0.16–0.31 | 0.47–0.67 | 0.44–0.58 | 0.25–0.63 |
| Earlywood density | ||||||
| Av (kg/m3) | 415 (27) | 403 (14) | 385 (17) | 316 (16) | 340 (13) | 331 (22) |
| Ra (kg/m3) | 376–491 | 383–432 | 344–418 | 296–348 | 318–367 | 295–359 |
| Latewood density | ||||||
| Av (kg/m3) | 637 (47) | 673 (20) | 692 (36) | 612 (58) | 734 (89) | 726 (62) |
| Ra (kg/m3) | 578–729 | 568–796 | 591–746 | 502–769 | 605–856 | 581–798 |
| Density at the earlywood latewood transition | ||||||
| Av (kg/m3) | 580 (33) | 596 (20) | 600 (29) | 520 (49) | 612 (49) | 614 (48) |
| Ra (kg/m3) | 536–653 | 541–655 | 547–649 | 433–623 | 548–672 | 482–674 |
| Earlywood dynamic modulus of elasticity | ||||||
| Av (GPa) | 11.2 (2.5) | 11.1 (1.6) | 11.8 (2.6) | 6.4 (0.8) | 9.9 (1.2) | 10.7 (1.9) |
| Ra (GPa) | 8.3–17.6 | 6.5–13.7 | 8.2–15.3 | 5.2–8.1 | 7.9–1.2 | 8.0–1.4 |
| Latewood dynamic modulus of elasticity | ||||||
| Av (GPa) | 14.8 (2.4) | 15.2 (2.5) | 17.9 (2.4) | 10.6 (1.6) | 14.6 (1.0) | 16.3 (3.6) |
| Ra (GPa) | 11.9–21.7 | 10.3–18.8 | 14.0–22.1 | 8.1–12.1 | 12.9–16.9 | 11.9–21.0 |
| Dynamic modulus of elasticity at the earlywood latewood transition | ||||||
| Av (GPa) | 13.4 (2.1) | 13.6 (1.9) | 15.4 (1.9) | 9.8 (1.4) | 12.9 (1.2) | 14.9 (1.9) |
| Ra (GPa) | 10.4–17.7 | 9.3–15.8 | 13.1–18.4 | 6.4–12.2 | 11.2–15.9 | 11.2–17.1 |
| RW | EWW | LWW | RD | EWD | LWD | RDMOE | EWDMOE | LWDMOE | |
|---|---|---|---|---|---|---|---|---|---|
| RW | 0.98 *** | 0.81 *** | −0.21 * | 0.00 ns | −0.36 * | −0.37 * | −0.29 * | −0.49 ** | |
| EWW | 0.99 *** | 0.73 *** | −0.25 * | −0.03 ns | −0.32 * | −0.38 * | −0.30 * | −0.49 ** | |
| LWW | 0.82 *** | 0.77 *** | −0.02 ns | 0.08 ns | −0.49 ** | −0.31 * | −0.25 * | −0.50 ** | |
| RD | −0.69 ** | −0.72 *** | −0.42 ** | 0.91 *** | 0.37 * | 0.45 ** | 0.44 ** | 0.33 * | |
| EWD | −0.58 ** | −0.58 ** | −0.39 * | 0.85 *** | 0.11 ns | 0.40 ** | 0.46 ** | 0.18 ns | |
| LWD | −0.50 ** | −0.49 ** | −0.52 ** | 0.75 *** | 0.48 ** | 0.18 ns | 0.09 ns | 0.49 ** | |
| RDMOE | −0.65 ** | −0.66 *** | −0.48 ** | 0.79 *** | 0.65 ** | 0.66 *** | 0.98 *** | 0.85 *** | |
| EWDMOE | −0.60 ** | −0.61 ** | −0.44 ** | 0.73 *** | 0.65 ** | 0.57 ** | 0.98 *** | 0.75 *** | |
| LWDMOE | −0.61 ** | −0.60 ** | −0.55 ** | 0.74 ** | 0.54 ** | 0.80 *** | 0.89 *** | 0.78 *** |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kharrat, W.; Koubaa, A.; Khlif, M.; Bradai, C. Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-Ray Densitometry and Ultrasonic Wave Velocity Measurement. Forests 2019, 10, 569. https://doi.org/10.3390/f10070569
Kharrat W, Koubaa A, Khlif M, Bradai C. Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-Ray Densitometry and Ultrasonic Wave Velocity Measurement. Forests. 2019; 10(7):569. https://doi.org/10.3390/f10070569
Chicago/Turabian StyleKharrat, Wassim, Ahmed Koubaa, Mohamed Khlif, and Chedly Bradai. 2019. "Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-Ray Densitometry and Ultrasonic Wave Velocity Measurement" Forests 10, no. 7: 569. https://doi.org/10.3390/f10070569
APA StyleKharrat, W., Koubaa, A., Khlif, M., & Bradai, C. (2019). Intra-Ring Wood Density and Dynamic Modulus of Elasticity Profiles for Black Spruce and Jack Pine from X-Ray Densitometry and Ultrasonic Wave Velocity Measurement. Forests, 10(7), 569. https://doi.org/10.3390/f10070569






