Review of Recent Advances in the Application of the Wavelet Transform to Diagnose Cracked Rotors
Abstract
:1. Introduction
2. The Wavelet Transform
2.1. Continuous Wavelet Transform
2.2. Discrete Wavelet Transform
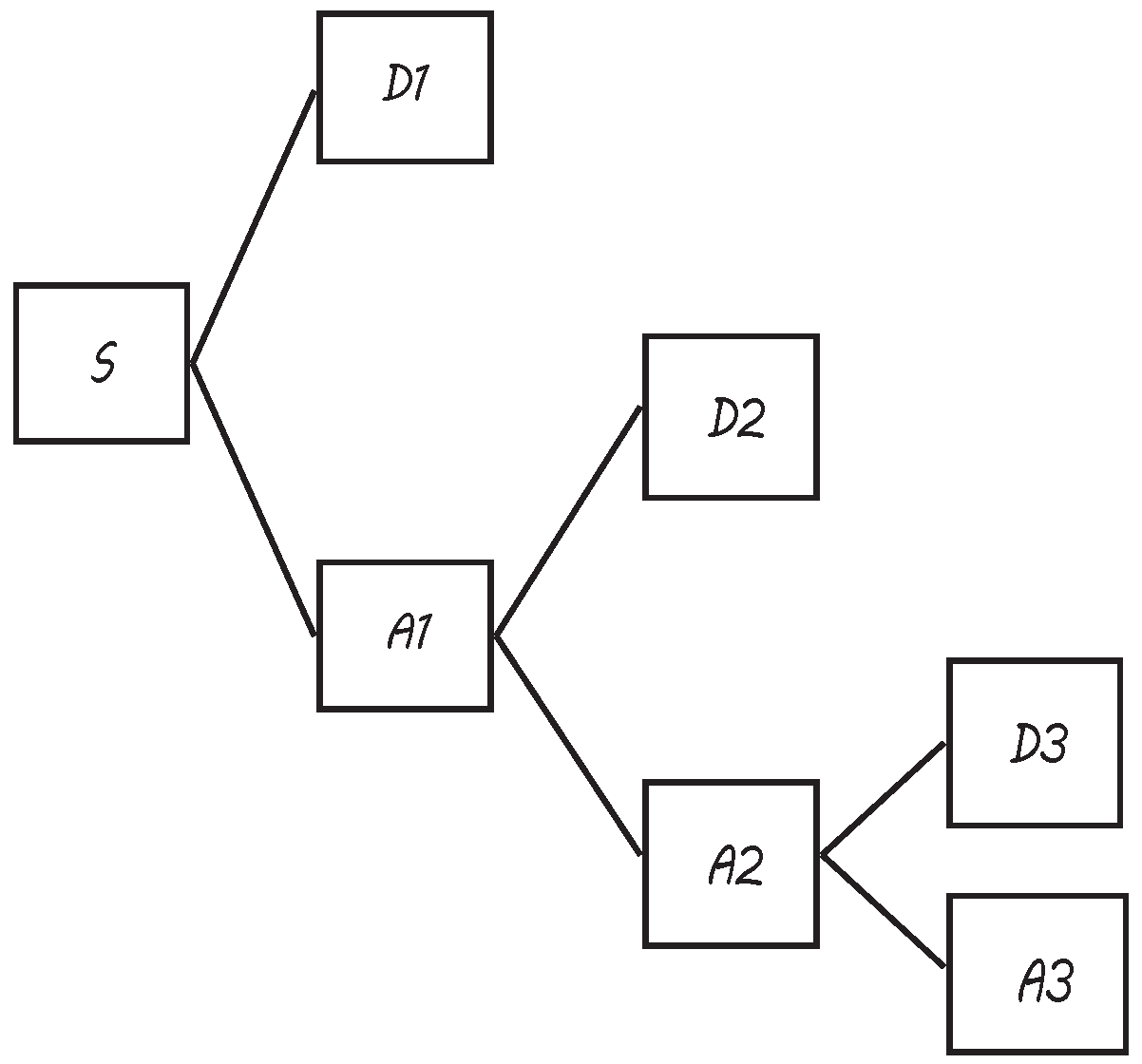
2.2.1. Multiresolution Analysis
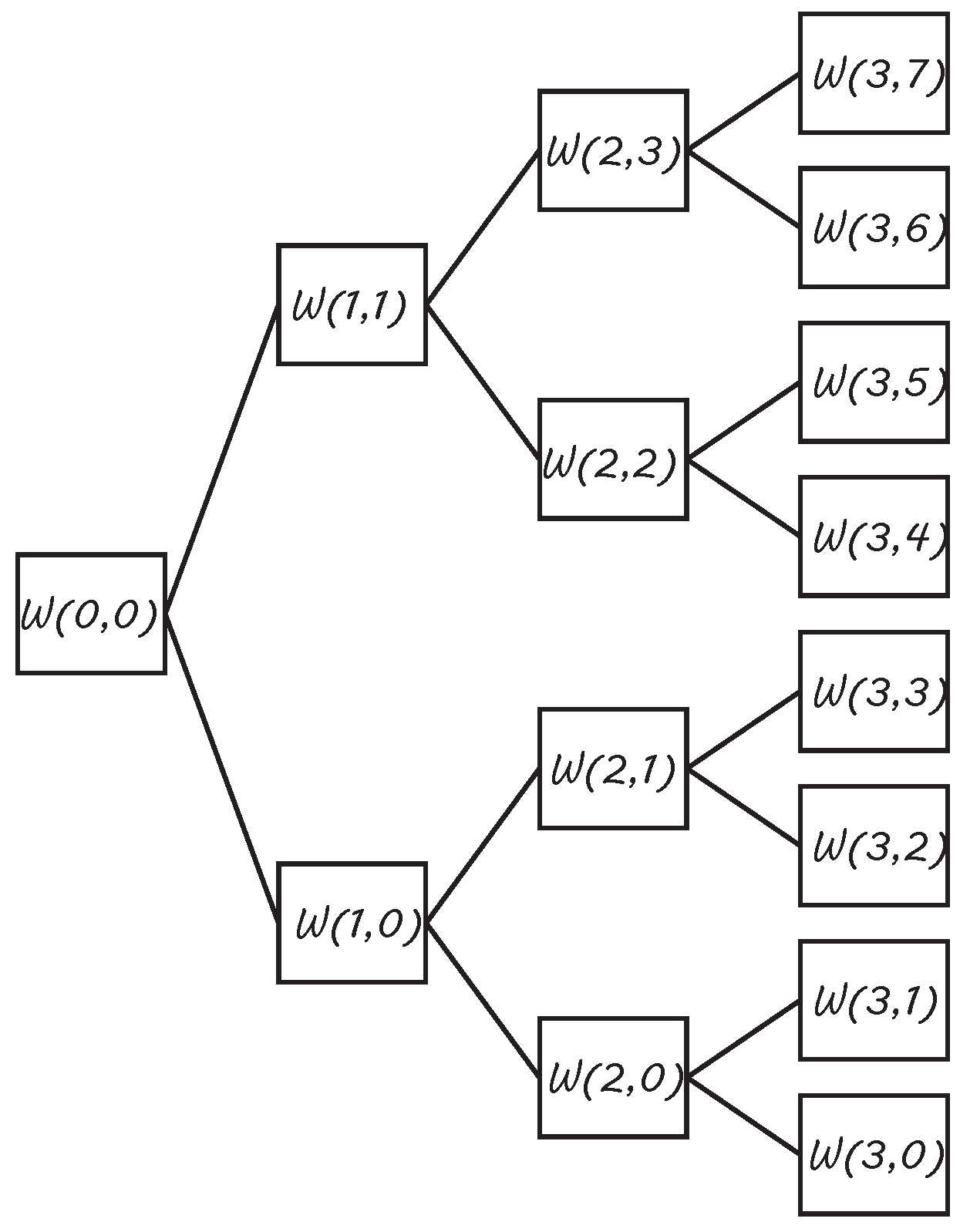
2.2.2. Wavelet Packets Transform
3. Wavelet Transform Parameters Selection
4. Results Presentation
5. Prospects
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations/Nomenclature
| CWT: Continuous Wavelet transform |
| FEA: Finite Elements Approach |
| WFEM: Wavelet Finite Element Methods |
| GA: Genetic algorithms |
| FT: Fourier transform |
| HT: Hilbert transform |
| WT: Wavelet transform |
| AE: Acoustic Emission |
| STFT: Short Time Fourier transform |
| CWT: Continuous Wavelet transform |
| XWT: Cross Wavelet Transform |
| ANN: Artificial Neural Network |
| DWT: Discrete Wavelet Transform |
| MRA: Multiresolution analysis |
| WPT: Wavelet Packets transform |
| SVM: Support Vector Machines |
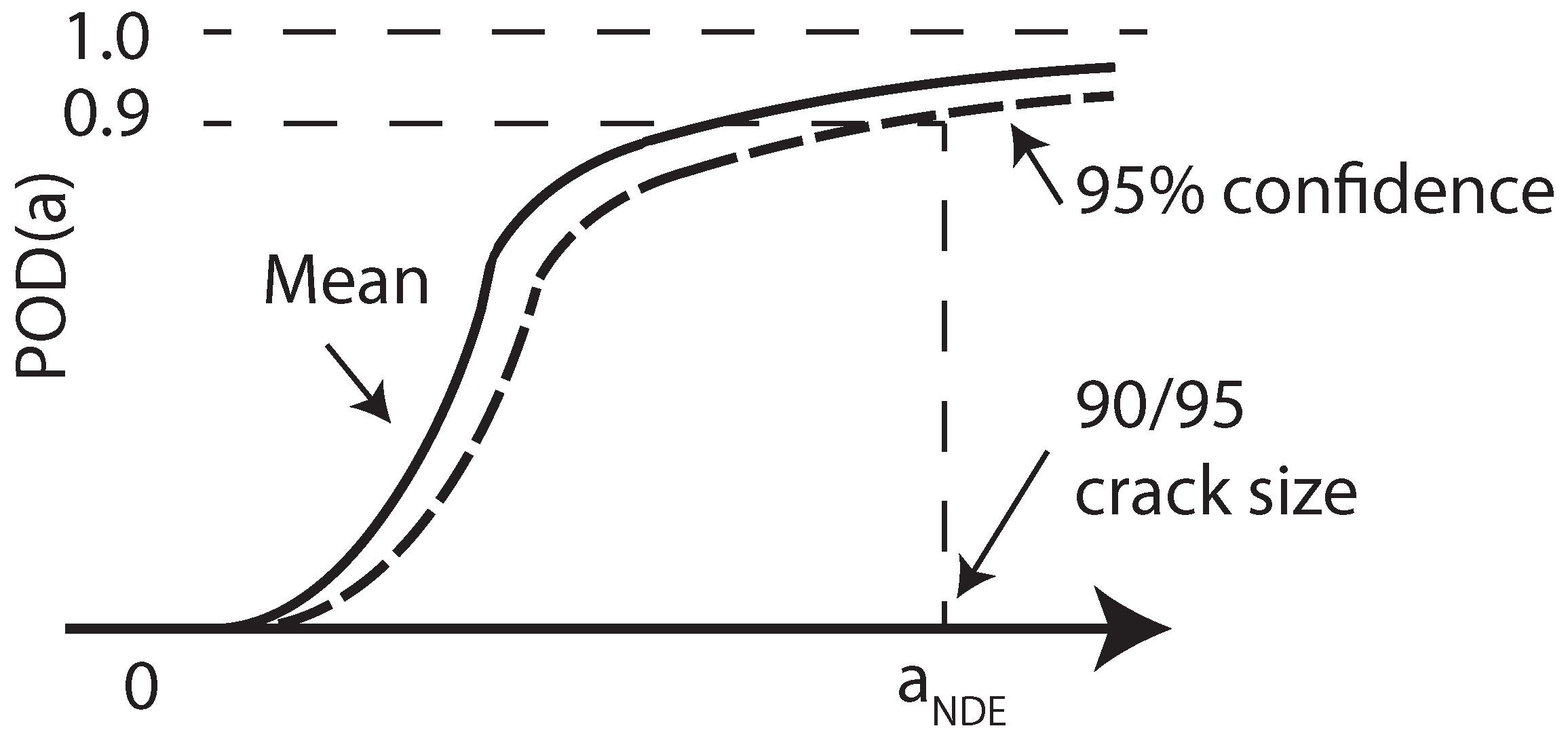
| NDE: Non Destructive Evaluation |
| POD: Probability of detection |
| t: Time |
| N: Number of samples for a time domain signal |
| c: Scale parameter in CWT |
| b: Shift parameter in CWT |
| s: Scale range evaluated in CWT |
| ψ: Wavelet function |
| w: Weighting function |
| x: Time domain signal |
| A: Approximation information |
| D: Detail information |
| k: Decomposition level |
| j: Position of a packet within decomposition level |
| : WPT coefficients |
| f: Frequency |
| : Central frequency of the wavelet function |
| : Sampling frequency |
| : Frequency resolution of a packet in WPT |
| a: Crack size |
| : Crack size that reaches certain POD at a given confidence level |
References
- Bachschmid, N.; Penacci, P. Crack effects in rotordynamics. Mech. Syst. Signal Process. 2008, 22, 761–762. [Google Scholar] [CrossRef]
- Sekhar, A.S. Multiple cracks effects and identification. Mech. Syst. Signal Process. 2008, 22, 845–878. [Google Scholar] [CrossRef]
- Papadopoulos, C.A. The strain energy release approach for modeling cracks in rotors: A state of the art review. Mech. Syst. Signal Process. 2008, 22, 763–789. [Google Scholar] [CrossRef]
- Sekhar, A.S. Identification of unbalance and crack acting simultaneously in a rotor system: Modal expansion versus reduced basis dynamic expansion. J. Vib. Control 2005, 11, 1125–1145. [Google Scholar] [CrossRef]
- Pennacchi, P.; Bachschmid, N.; Vania, A. A model-based identification method of transverse cracks in rotating shafts suitable for industrial machines. Mech. Syst. Signal Process. 2006, 20, 2112–2147. [Google Scholar] [CrossRef] [Green Version]
- Kulesza, Z.; Sawicki, J.T. Rigid finite element model of a cracked rotor. J. Sound Vib. 2012, 331, 4145–4169. [Google Scholar] [CrossRef]
- Sekhar, A.S.; Prabhu, B.S. Crack detection and vibration characteristics of cracked shafts. J. Sound Vib. 1992, 157, 375–381. [Google Scholar] [CrossRef]
- Gomez-Mancilla, J.; Sinou, J.; Nosov, V.R. The influence of crack-imbalance orientation and orbital evolution for an extended cracked Jeffcott rotor. C. R. Mecanique 2004, 332, 995–962. [Google Scholar] [CrossRef]
- Bachschmid, N.; Penacci, P.; Tanzi, E. Some remarks on breathing mechanism, on non-linear effects and on slant and helicoidal cracks. Mech. Syst. Signal Process. 2008, 22, 879–904. [Google Scholar] [CrossRef]
- Al-Shudeifat, A.; Butcher, E.A. New breathing functions for the transverse breathing crack of the cracked rotor system: Approach for critical and subcritical hermonic analysis. J. Sound Vib. 2011, 330, 526–544. [Google Scholar] [CrossRef]
- Gasch, R. Dynamic behaviour of the Laval rotor with a transverse crack. Mech. Syst.Signal Process. 2008, 22, 790–804. [Google Scholar] [CrossRef]
- Ishida, Y. Cracked rotors: Industrial machine case histories and nonlinear effects shown by simple Jeffcott rotor. Mech. Syst. Signal Process. 2008, 22, 805–817. [Google Scholar] [CrossRef]
- Kumar, C.; Rastogi, V. A Brief Review on Dynamics of a Cracked Rotor. Int. J. Rotat. Mach. 2009, 2009, 1–6. [Google Scholar] [CrossRef]
- Xiang, J.; Zhong, Y.; Chen, X.; He, Z. Crack detection in a shaft by combination of wavelet-based elements and genetic algorithm. Int. J. Solids Struct. 2008, 45, 4782–4795. [Google Scholar] [CrossRef]
- Zhao, B. The Application of Wavelet Finite Element Method on the Crack Recognition of the Gate Rotor Shaft of the Single Screw Compressor. Adv. Sci. Lett. 2011, 4, 3721–3726. [Google Scholar] [CrossRef]
- Li, B.; Dong, H. Quantitative Identification of Multiple Cracks in a Rotor Utilizing Wavelet Finite Element Method. CMES-Comput. Model. Eng. Sci. 2012, 84, 205–228. [Google Scholar]
- Sekhar, A.S. On-line rotor fault identification by combined model and signal based approach. Noise Vib. Worldw. 2004, 35, 16–30. [Google Scholar] [CrossRef]
- Feldman, M.; Seibold, S. Damage diagnosis of rotors: Application of Hubert transform and multihypothesis testing. J. Vib. Control 1999, 5, 421–442. [Google Scholar] [CrossRef]
- Sabnavis, G.; Kirk, R.G.; Kasarda, M.; Quinn, D. Cracked shaft detection and diagnostics: A literature review. Shock Vib. Dig. 2004, 36, 287–296. [Google Scholar] [CrossRef]
- Babu, T.R.; Srikanth, S.; Sekhar, A.S. Hilbert-Huang transform for detection and monitoring of crack in a transient rotor. Mech. Syst. Signal Process. 2008, 22, 905–914. [Google Scholar] [CrossRef]
- Jiao, W.; Yang, S.; Chang, Y.; Yan, G.; Hu, J. Detecting a Cracked Rotor with HHT-based Time-Frequency Representation. In Proceedings of the IEEE International Conference on Automation and Logistics, Qingdao, China, 1–3 September 2008; pp. 790–793.
- Mallat, S. A Wavelet Tour of Signal Processing; Academic Press: Burlington, VT, USA, 2009. [Google Scholar]
- Gu, D.; Kim, J.; Kelimu, T.; Huh, S.; Choi, B. Evaluation of the use of envelope analysis and DWT on AE signals generated from degrading shafts. Mater. Sci. Eng. 2012, 177, 1683–1690. [Google Scholar] [CrossRef]
- Elforjani, M.; Mba, D. Detecting Natural Crack Initiation and Growth in Slow Speed Shafts with the Acoustic Emission Technology. Eng. Fail. Anal. 2009, 16, 2121–2129. [Google Scholar] [CrossRef]
- Peng, Z.K.; Chu, F.L. Application of the wavelet Transform in machine condition monitoring and fault diagnostics: A review with bibliography. Mech. Syst. Signal Process. 2004, 18, 199–221. [Google Scholar] [CrossRef]
- Mallat, S. A Theory for Multiresolution Signal Decomposition: The Wavelet Representation. IEEE Trans. Pattern Anal. Mach. Intell. 1989, 11, 674–693. [Google Scholar] [CrossRef]
- Vignolo, L.; Milone, D.H.; Rufiner, H.L. Genetic wavelet packets for speech recognition. Expert Syst. Appl. 2011, 40, 2350–2359. [Google Scholar] [CrossRef]
- Avci, E.; Akpolat, Z.H. Speech recognition using a wavelet packet adaptive network based fuzzy inference system. Expert Syst. Appl. 2006, 31, 495–503. [Google Scholar] [CrossRef]
- Mercorelli, P. A denoising procedure using wavelet packets for instantaneous detection of pantograph oscillations. Mech. Syst. Signal Process. 2013, 35, 137–149. [Google Scholar] [CrossRef]
- Premanode, B.; Vongprasert, J.; Toumazou, C. Noise Reduction for Nonlinear Nonstationary Time Series Data using Averaging Intrinsic Mode Function. Algorithms 2013, 6, 407–429. [Google Scholar] [CrossRef]
- Tinati, M.A.; Mozaffary, B. A Wavelet Packets Approach to Elecrocardiograph Baseline Drift Cancellation. Int. J. Biomed. Imaging 2006, 2006, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Camps, A.; Tarongí, J.M. RFI Mitigation in Microwave Radiometry Using Wavelets. Algorithms 2009, 2, 1248–1262. [Google Scholar] [CrossRef]
- Gómez, M.J.; Castejón, C.; García-Prada, J.C. Crack detection in rotating shafts based on the 3x energy. Analytical and experimental analysis. Mech. Mach. Theory 2016, 96, 94–106. [Google Scholar] [CrossRef]
- Lou, X.; Loparo, K.A. Bearing fault diagnosis based on wavelet transform and fuzzy inference. Mech. Syst. Signal Process. 2004, 18, 1077–1095. [Google Scholar] [CrossRef]
- Castejón, C.; Lara, O.; García-Prada, J.C. Automated diagnosis of rolling bearings using MRA and neural networks. Mech. Syst. Signal Process. 2010, 24, 289–299. [Google Scholar] [CrossRef]
- Gómez, M.J.; Castejón, C.; García-Prada, J.C. Incipient Fault Detection in Bearings Through the use of WPT Energy and Neural Networks. In Advances in Condition Monitoring of Machinery in Non-Stationary Operations; Springer: Berlin, Germany, 2014; pp. 63–72. [Google Scholar]
- Wang, W.J.; McFaden, P.D. Application of wavelets to gearbox vibration signals for fault detection. J. Sound Vib. 1996, 192, 927–939. [Google Scholar] [CrossRef]
- Rafiee, J.; Rafiee, M.A.; Tse, P.W. Application of mother wavelet functions for automatic gear and bearing fault diagnosis. Expert Syst. Appl. 2010, 37, 4568–4579. [Google Scholar] [CrossRef]
- Vernekar, K.; Kumar, H.; Gandharan, K.V. Gear Fault Detection Using Vibration Analysis and Continuous Wavelet Transform. Proced. Mater. Sci. 2014, 5, 1846–1852. [Google Scholar] [CrossRef]
- Douka, E.; Loutridis, S.; Trochidis, A. Crack Identification in Beams Using Wavelet Analysis. Int. J. Solids Struct. 2003, 40, 3557–3569. [Google Scholar] [CrossRef]
- Nagaraju, C.; Narayana Rao, K.; Mallikarjuna Rao, K. Application of 3D wavelet transforms for crack detection in rotor systems. Sadhana 2009, 34, 407–419. [Google Scholar] [CrossRef] [Green Version]
- Babu, T.R.; Sekhar, A.S. Shaft Crack Identification Using Artificial Neural Networks and Wavelet Transform Data of a Transient Rotor. Adv. Vib. Eng. 2010, 9, 207–214. [Google Scholar]
- Huang, Y. Advances in Artificial Neural Networks—Methodological Development and Application. Algorithms 2009, 2, 979–1007. [Google Scholar] [CrossRef]
- Sinou, J.J. An Experimental Investigation of Condition Monitoring for Notched Rotors Through Transient Signals and Wavelet Transform. Exp. Mech. 2009, 5, 683–695. [Google Scholar] [CrossRef]
- Srinivas, H.K.; Srinivasan, K.S.; Umesh, K.N. Role of an Artificial Neural Network and a Wavelet Transform for Condition Monitoring of the Combined Faults of Unbalance and Cracked Rotors. Int. J. Acoust. Vib. 2010, 15, 121–127. [Google Scholar]
- Hee, L.M.; Leong, M.S.; Hui, K.H. Analysis of Residual Wavelet Scalogram for Machinery Fault Diagnosis. Mater. Ind. Manuf. Eng. Res. Adv. 2014, 845, 113–117. [Google Scholar] [CrossRef]
- Smith, J. Mathematics of the Discrete Fourier Transform (DFT); W3K: Charleston, SC, USA, 2007. [Google Scholar]
- Jensen, A.; la Cour-Harbo, A. Ripples in Mathematics; Springer: Berlin, Germany, 2000. [Google Scholar]
- Sawicki, J.T.; Sen, A.K.; Litak, G. Multiresolution Wavelet Analysis of the Dynamics of a Cracked Rotor. Int. J. Rotat. Mach. 2009, 2009, 1–8. [Google Scholar] [CrossRef]
- Castejón, C.; García-Prada, J.C.; Gómez, M.J.; Meneses, J.T. Automatic Detection of Cracked Rotors combining Multiresolution Analysis and Artificial Neural Networks. J. Vib. Control 2014. [Google Scholar] [CrossRef]
- Eftekharnejad, B.K.; Addali, A.; Mba, D. Shaft crack diagnostics in a gearbox. Appl. Acoust. 2012, 73, 723–733. [Google Scholar] [CrossRef]
- Liu, B.; Ling, S.F. Machinery diagnostic based on wavelet packets. J. Sound Vib. 1997, 3, 5–17. [Google Scholar]
- Bin, G.F.; Gao, J.J.; Li, X.J.; Dhillon, B.S. Early fault diagnosis of rotating machinery based on wavelet packets-Empirical Mode decomposition feature extraction and neural network. Mech. Syst. Signal Process. 2012, 27, 696–711. [Google Scholar] [CrossRef]
- Hu, Q.; He, Z.; Zhang, Z. Fault diagnosis of rotating machinery based on improved wavelet package transform and SVMs ensemble. Mech. Syst. Signal Process. 2007, 21, 688–705. [Google Scholar] [CrossRef]
- Shen, C.; Wang, D.; Kong, F.; Tse, P.W. Fault diagnosis of rotating machinery based on the statistical parameters of wavelet packet paving and a generic support vector regressive classifier. Meas. J. Int. Meas. Confed. 2013, 46, 1551–1564. [Google Scholar] [CrossRef]
- Feng, Y.; Schlindwein, F.S.; Li, X.J. Normalized wavelet packets quantifiers for condition monitoring. Mech. Syst. Signal Process. 2009, 23, 712–723. [Google Scholar] [CrossRef]
- Gómez, M.J.; Castejón, C.; García-Prada, J.C. New stopping criteria for crack detection during fatigue tests of failway axles. Eng. Fail. Anal. 2015, 56, 530–537. [Google Scholar] [CrossRef]
- Castejón, C.; Gómez, M.J.; García-Prada, J.C.; Ordoñez, A.; Rubio, H. Automatic Detection of Cracked Rotors Combining Multiresolution Analysis and Artificial Neural Networks. Int. J. Acoust. Vib. 2015, 20, 95–100. [Google Scholar] [CrossRef]
- Al-Badour, F.; Sunar, M.; Cheded, L. Vibration analysis of rotating machinery using time-frequency analysis and wavelet techniques. Mech. Syst. Signal Process. 2011, 25, 2083–2101. [Google Scholar] [CrossRef]
- He, K.F.; Li, X.J.; Li, X.C. Research on Feature Extraction Experiment for Acoustic Emission Signal of Rotor Crack Fault. Mech. Eng. Green Manuf. 2010, 34–35, 1005–1009. [Google Scholar] [CrossRef]
- Tan, R.H.G.; Ramachandaramurthy, V.K. Power System Transient Analysis using Scale Selection Wavelet Transform. In Proceedings of the Tencon IEEE Region 10 Conference Proceedings, Singapore, 23–26 January 2009; Volume 1–4, pp. 2171–2176.
- Kim, J.; Cho, B.H. An innovative approach for characteristic analysis and state-of-health diagnosis for a Li-ion cell based on the discrete wavelet transform. J. Power Sour. 2014, 260, 115–130. [Google Scholar] [CrossRef]
- Dessauer, M.P.; Dua, S. Discriminative Features and Classification Methods for Accurate Classification. Evolut. Bio-inspir. Comput. Theory Appl. 2010. [Google Scholar] [CrossRef]
- Safara, F.; Doraisamy, S.; Azman, A. Multi-level basis selection of wavelet packet decomposition tree for heart sound classification. Comput. Biol. Med. 2013, 43, 1407–1414. [Google Scholar] [CrossRef] [PubMed]
- Rehman, M.; Touqir, I.; Batool, W. Selection of Optimal Wavelet Bases for Image Compression using SPIHT Algorithm. In Proceedings of the Seven International Conferente on Mahcine Vision, Milan, Italy, 19 November 2014; Volume 9445.
- Omari, T.; Bereksi-Reguig, F. An Automatic Wavelet Selection Scheme for Heart Sounds Denoising. Proc. IWBBIO Int. Work-Conf. Bioinform. Biomed. Eng. 2015, 13. [Google Scholar] [CrossRef]
- Kumar, A.; Sing, M. Optimal Selection of Wavelet Function and Decomposition Level for Removal ECG Signal Artifacts. J. Med. Imaging Health Inform. 2015, 5, 138–146. [Google Scholar] [CrossRef]
- Li, B.; Xia, H. Research on Feature Recognition of Nuclear Power Equipment Based on the Optimal Wavelet Basis. In Proceedings of the 21st International Conference on Nuclear Engineering, Chengdu, China, 29 July–2 August 2013; Volume 5.
- Gómez, M.J.; Castejón, C.; García-Prada, J.C. Experimental development and validation of a vibration-based technique for crack detection in a shaft. Proc. 9th IFTOMM Int. Conf. Rotor Dyn. 2015, 21, 373–383. [Google Scholar]
- Rummel, W.D. Nondestructive inspection reliability history, status and future path. In Proceedings of the 18th World Conference on Nondestructive, Durban, South Africa, 16–20 April 2010.
- Cantini, S.; Beretta, S. Structural Reliability Assessment of Railway Axles; Lucchini RS: Lovere, Italy, 2011. [Google Scholar]
- Bachschmid, N.; Pennacchi, P.; Tanzi, E. Cracked Rotors: A Survey on Static and Dynamic Behaviour Including Modelling and Diagnosis; Springer: Berlin, Germany, 2010. [Google Scholar]
- Gao, C.W.; Meeker, W.Q. A Statistical Method for Crack Detection from Vibrothermography Inspection Data. Qual. Technol. Quant. Manag. 2012, 9, 59–77. [Google Scholar]
- Gao, C.W.; Meeker, W.Q.; Mayton, D. Detecting cracks in aircracft engine fan blades using vibrothermography nondestructive evaluation. Reliab. Eng. Syst. Saf. 2014, 131, 229–235. [Google Scholar] [CrossRef]
- Gagar, D.; Foote, P.; Irving, P.E. Detecting and Monitoring of Fatigue Cracks in Metallic Structures Using Acoustic Emission: Routes to Quantification of Probability of Detection. Advanced Materials Research 2014, 891–892, 1268–1274. [Google Scholar] [CrossRef]


© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gómez, M.J.; Castejón, C.; García-Prada, J.C. Review of Recent Advances in the Application of the Wavelet Transform to Diagnose Cracked Rotors. Algorithms 2016, 9, 19. https://doi.org/10.3390/a9010019
Gómez MJ, Castejón C, García-Prada JC. Review of Recent Advances in the Application of the Wavelet Transform to Diagnose Cracked Rotors. Algorithms. 2016; 9(1):19. https://doi.org/10.3390/a9010019
Chicago/Turabian StyleGómez, María J., Cristina Castejón, and Juan C. García-Prada. 2016. "Review of Recent Advances in the Application of the Wavelet Transform to Diagnose Cracked Rotors" Algorithms 9, no. 1: 19. https://doi.org/10.3390/a9010019
APA StyleGómez, M. J., Castejón, C., & García-Prada, J. C. (2016). Review of Recent Advances in the Application of the Wavelet Transform to Diagnose Cracked Rotors. Algorithms, 9(1), 19. https://doi.org/10.3390/a9010019






