A Mathematical Model on the Resolution of Extrusion Bioprinting for the Development of New Bioinks
Abstract
:1. Introduction
2. Materials and Methods
2.1. Pluronic F127 Hydrogel Preparation
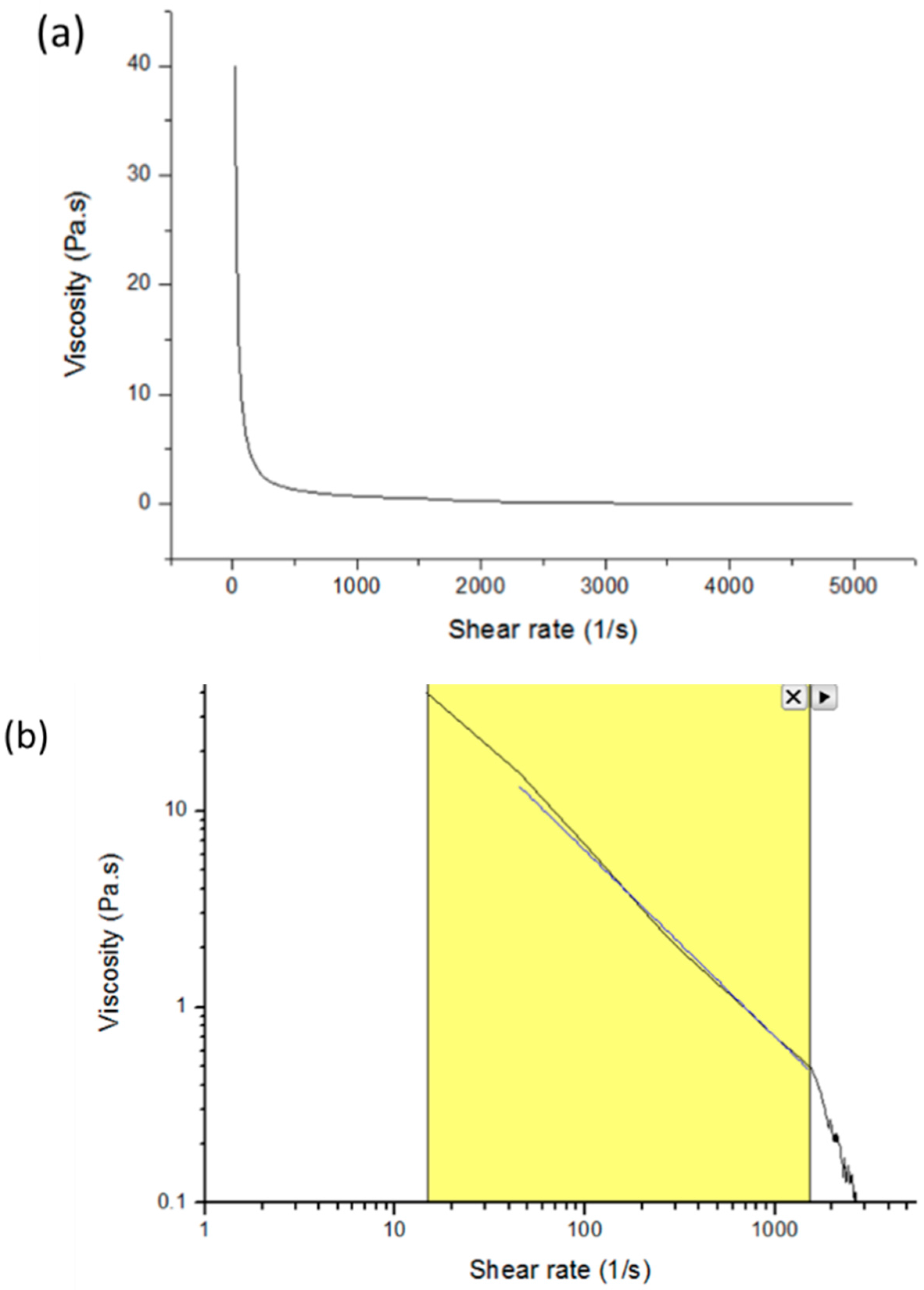
2.2. Rheological Characterization
2.3. Resolution Study Based on Three Different Factors
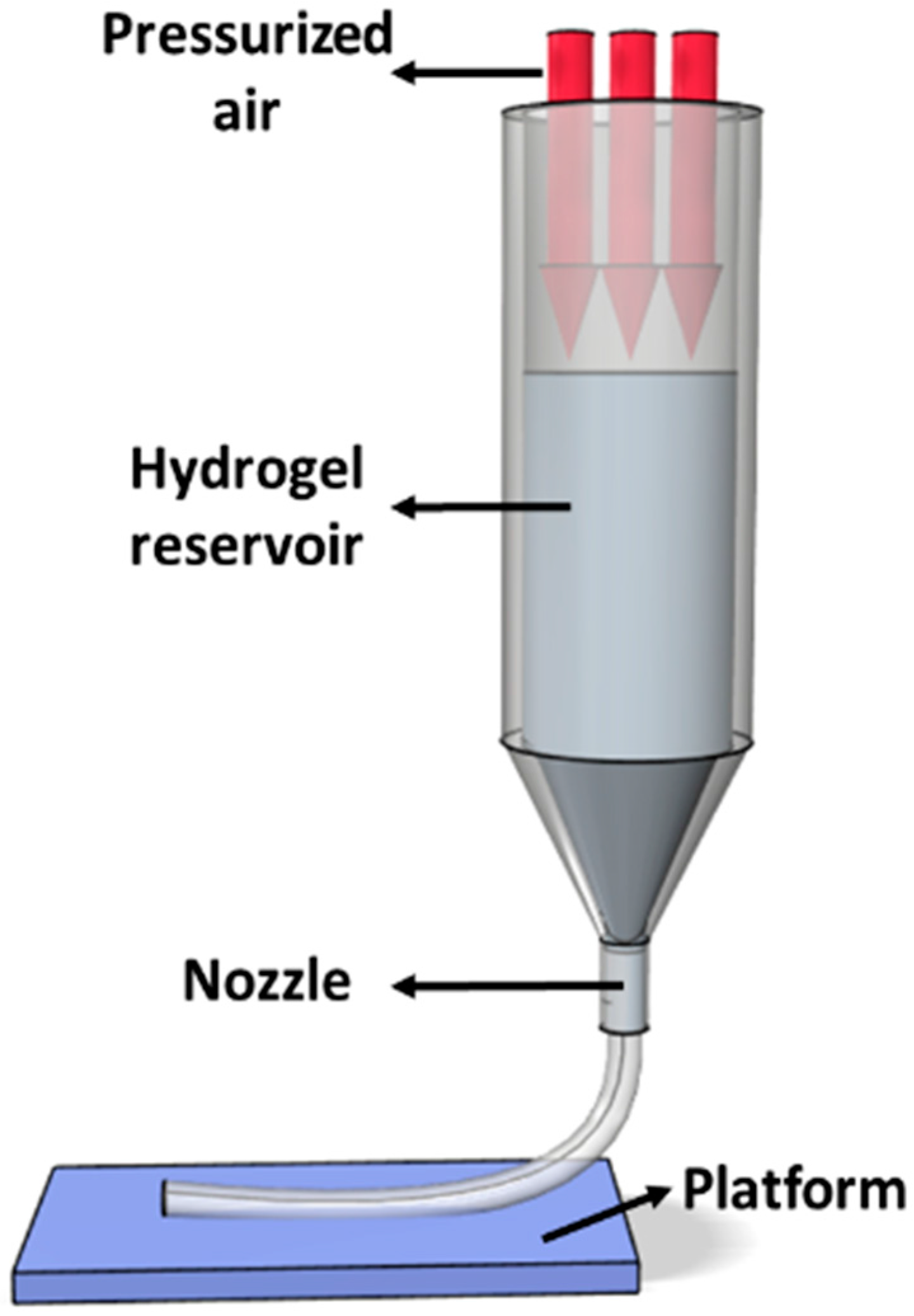
2.3.1. Pluronic F127 Hydrogel Printing
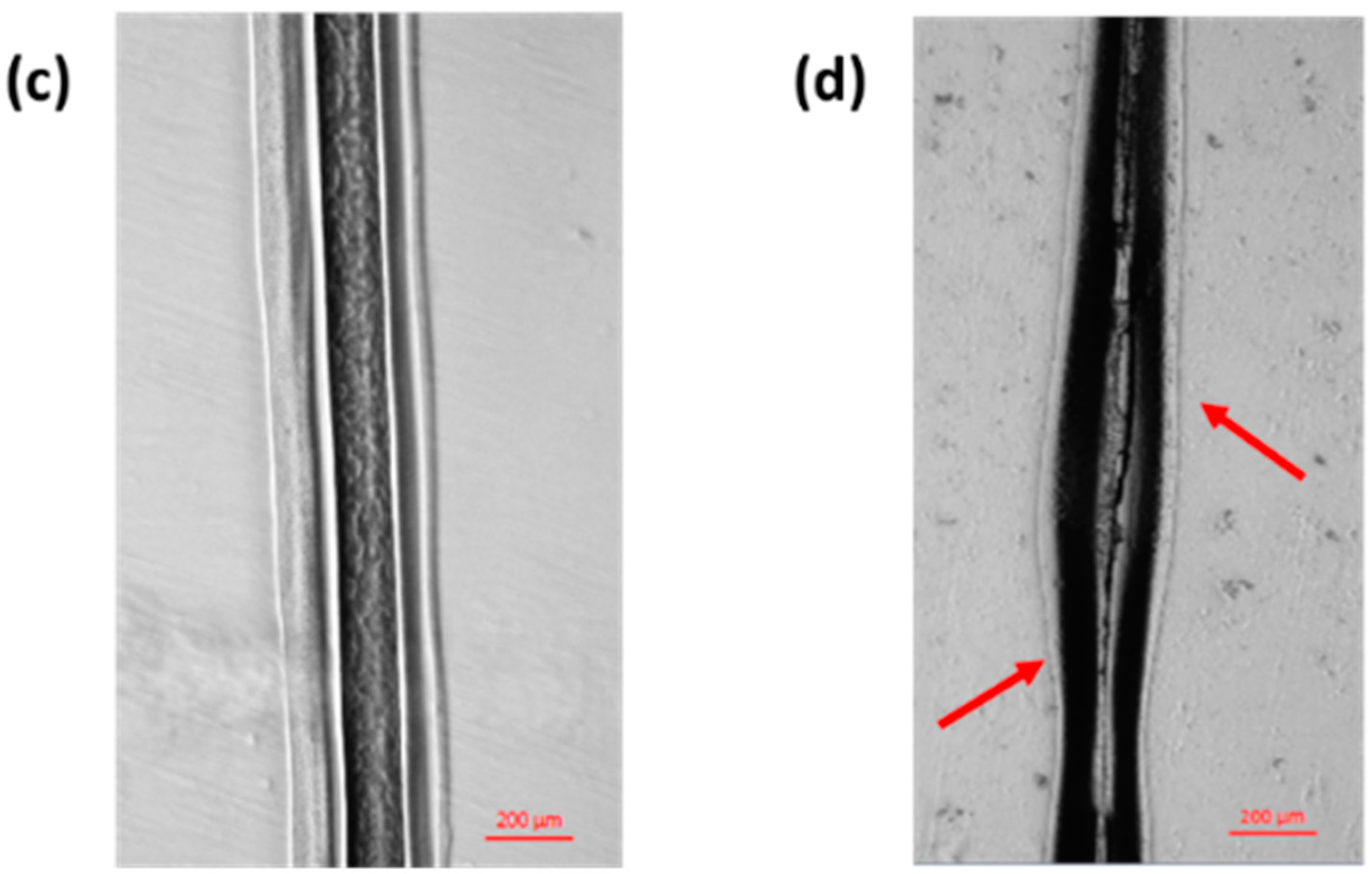
2.3.2. Pluronic F127 Hydrogel Characterization
2.4. Mathematic Modeling of Line Width, Gauge Pressure, and Stage Moving Speed
3. Results and Discussion
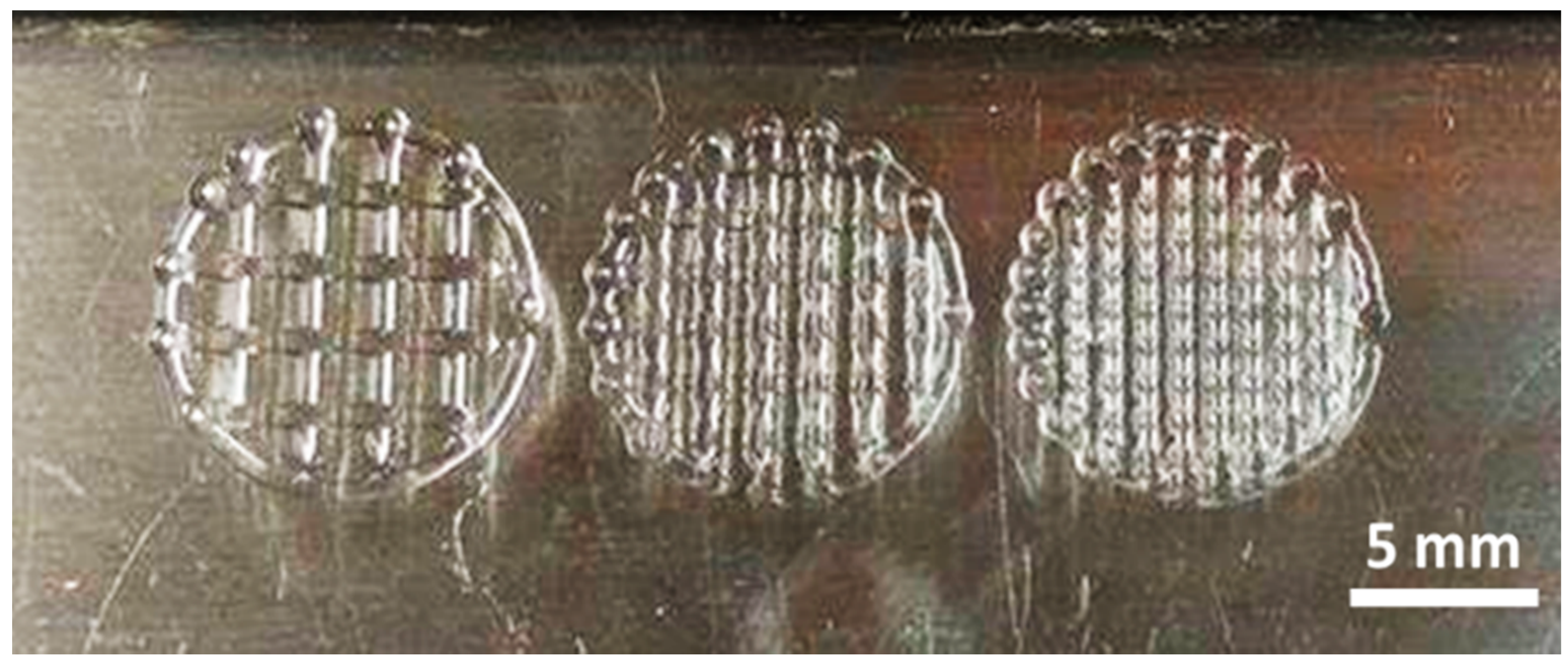
3.1. Resolution Study on a Pneumatic Extrusion-Based Bioprinter
3.2. Model Verification
4. Conclusions
Supplementary Materials
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ng, W.L.; Yeong, W.Y.; Naing, M.W. Polyelectrolyte gelatin-chitosan hydrogel optimized for 3D bioprinting in skin tissue engineering. Int. J. Bioprint. 2016, 2. [Google Scholar] [CrossRef]
- Cazon, A.; Aizpurua, J.; Paterson, A.; Bibb, R.; Campbell, R.I. Customised design and manufacture of protective face masks combining a practitioner-friendly modelling approach and low-cost devices for digitising and additive manufacturing. Virtual Phys. Prototyp. 2014, 9, 251–261. [Google Scholar] [CrossRef] [Green Version]
- Dean, D.; Mott, E.; Luo, X.; Busso, M.; Wang, M.O.; Vorwald, C.; Siblani, A.; Fisher, J.P. Multiple initiators and dyes for continuous digital light processing (CDLP) additive manufacture of resorbable bone tissue engineering scaffolds. Virtual Phys. Prototyp. 2014, 9, 3–9. [Google Scholar] [CrossRef]
- Wang, S.; Lee, J.M.; Yeong, W.Y. Smart hydrogels for 3D bioprinting. Int. J. Bioprint. 2015, 1. [Google Scholar] [CrossRef]
- Nakamura, M.; Mir, T.A.; Arai, K.; Ito, S.; Yoshida, T.; Iwanaga, S.; Kitano, H.; Obara, C.; Nikaido, T. Bioprinting with pre-cultured cellular constructs towards tissue engineering of hierarchical tissues. Int. J. Bioprint. 2015, 1, 39–48. [Google Scholar] [CrossRef]
- Chua, C.K.; Yeong, W.Y. Bioprinting: Principles and Applications; World Scientific: Singapore, Singapore, 2014. [Google Scholar]
- An, J.; Teoh, J.E.; Suntornnond, R.; Chua, C.K. Design and 3D printing of scaffolds and tissues. Engineering 2015, 1, 261–268. [Google Scholar] [CrossRef]
- Jungst, T.; Smolan, W.; Schacht, K.; Scheibel, T.; Groll, J. Strategies and molecular design criteria for 3D printable hydrogels. Chem. Rev. 2016, 116, 1496–1539. [Google Scholar] [CrossRef] [PubMed]
- Knowlton, S.; Onal, S.; Yu, C.H.; Zhao, J.J.; Tasoglu, S. Bioprinting for cancer research. Trends Biotechnol. 2015, 33, 504–513. [Google Scholar] [CrossRef] [PubMed]
- Murphy, S.V.; Atala, A. 3D bioprinting of tissues and organs. Nat. Biotechnol. 2014, 32, 779–785. [Google Scholar] [CrossRef] [PubMed]
- Müller, M.; Becher, J.; Schnabelrauch, M.; Zenobi-Wong, M. Printing thermoresponsive reverse molds for the creation of patterned two-component hydrogels for 3D cell culture. J. Vis. Exp. 2013, 77, 50632. [Google Scholar] [CrossRef] [PubMed]
- Ng, W.L.; Wang, S.; Yeong, W.Y.; Naing, M.W. Skin bioprinting: Impending reality or fantasy? Trends Biotechnol. 2016, 34, 689–699. [Google Scholar] [CrossRef] [PubMed]
- Kolesky, D.B.; Truby, R.L.; Gladman, A.; Busbee, T.A.; Homan, K.A.; Lewis, J.A. 3D bioprinting of vascularized, heterogeneous cell-laden tissue constructs. Adv. Mater. 2014, 26, 3124–3130. [Google Scholar] [CrossRef] [PubMed]
- Ozbolat, I.T.; Hospodiuk, M. Current advances and future perspectives in extrusion-based bioprinting. Biomaterials 2016, 76, 321–343. [Google Scholar] [CrossRef] [PubMed]
- Vaezi, M.; Yang, S. Extrusion-based additive manufacturing of peek for biomedical applications. Virtual Phys. Prototyp. 2015, 10, 123–135. [Google Scholar] [CrossRef]
- Chanthakulchan, A.; Koomsap, P.; Parkhi, A.A.; Supaphol, P. Environmental effects in fibre fabrication using electrospinning-based rapid prototyping. Virtual Phys. Prototyp. 2015, 10, 227–237. [Google Scholar] [CrossRef]
- Zhao, X.; He, J.; Xu, F.; Liu, Y.; Li, D. Electrohydrodynamic printing: A potential tool for high-resolution hydrogel/cell patterning. Virtual Phys. Prototyp. 2016, 11, 57–63. [Google Scholar] [CrossRef]
- Suntornnond, R.; An, J.; Yeong, W.Y.; Chua, C.K. Biodegradable polymeric films and membranes processing and forming for tissue engineering. Macromol. Mater. Eng. 2015, 300, 858–877. [Google Scholar] [CrossRef]
- Tan, E.Y.S.; Yeong, W.Y. Concentric bioprinting of alginate-based tubular constructs using multi-nozzle extrusion-based technique. Int. J. Bioprint. 2015, 1. [Google Scholar] [CrossRef]
- Julien, B.; Daniel, T.; Marie-Claude, H. Micro-extrusion of organic inks for direct-write assembly. J. Micromech. Microeng. 2008, 18, 115020. [Google Scholar]
- Cheng, J.; Lin, F.; Liu, H.; Yan, Y.; Wang, X.; Zhang, R.; Xiong, Z. Rheological properties of cell-hydrogel composites extruding through small-diameter tips. J. Manuf. Sci. Eng. 2008, 130, 021014. [Google Scholar] [CrossRef]
- Lee, J.M.; Yeong, W.Y. A preliminary model of time-pressure dispensing system for bioprinting based on printing and material parameters. Virtual Phys. Prototyp. 2015, 10, 3–8. [Google Scholar] [CrossRef]
- Abdel-Hamid, S.M.; Abdel-Hady, S.E.; El-Shamy, A.A.; El-Dessouky, H.F. Formulation of an antispasmodic drug as a topical local anesthetic. Int. J. Pharm. 2006, 326, 107–118. [Google Scholar] [CrossRef] [PubMed]
- Fried, J.R. Polymer Science and Technology; Pearson Education: Upper Saddle River, NJ, USA, 2014. [Google Scholar]
- Pati, F.; Jang, J.; Lee, J.W.; Cho, D.-W. Chapter 7—Extrusion bioprinting A2—Atala, Anthony. In Essentials of 3D Biofabrication and Translation; Yoo, J.J., Ed.; Academic Press: Boston, MA, USA, 2015; pp. 123–152. [Google Scholar]
- Matanović, M.R.; Kristl, J.; Grabnar, P.A. Thermoresponsive polymers: Insights into decisive hydrogel characteristics, mechanisms of gelation, and promising biomedical applications. Int. J. Pharm. 2014, 472, 262–275. [Google Scholar] [CrossRef] [PubMed]
- Chang, C.C.; Boland, E.D.; Williams, S.K.; Hoying, J.B. Direct-write bioprinting three-dimensional biohybrid systems for future regenerative therapies. J. Biomed. Mater. Res. Part B Appl. Biomater. 2011, 98, 160–170. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.H.; Moturi, V.; Lee, Y. Thixotropic property in pharmaceutical formulations. J. Control. Release 2009, 136, 88–98. [Google Scholar] [CrossRef] [PubMed]
- Iwami, K.; Noda, T.; Ishida, K.; Morishima, K.; Nakamura, M.; Umeda, N. Bio rapid prototyping by extruding/aspirating/refilling thermoreversible hydrogel. Biofabrication 2010, 2, 014108. [Google Scholar] [CrossRef] [PubMed]
- Feilden, E.; Blanca, E.G.-T.; Giuliani, F.; Saiz, E.; Vandeperre, L. Robocasting of structural ceramic parts with hydrogel inks. J. Eur. Ceram. Soc. 2016, 36, 2525–2533. [Google Scholar] [CrossRef]
- Chen, X.; Li, M.; Ke, H. Modeling of the flow rate in the dispensing-based process for fabricating tissue scaffolds. J. Manuf. Sci. Eng. 2008, 130, 021003. [Google Scholar] [CrossRef]
- Suntornnond, R.; An, J.; Tijore, A.; Leong, K.F.; Chua, C.K.; Tan, L.P. A solvent-free surface suspension melt technique for making biodegradable pcl membrane scaffolds for tissue engineering applications. Molecules 2016, 21, 386. [Google Scholar] [CrossRef] [PubMed]
- Song, S.J.; Choi, J.; Park, Y.D.; Lee, J.J.; Hong, S.Y.; Sun, K. A three-dimensional bioprinting system for use with a hydrogel-based biomaterial and printing parameter characterization. Artif. Organs 2010, 34, 1044–1048. [Google Scholar] [CrossRef] [PubMed]
- Bajpai, S.K.; Sharma, S. Investigation of swelling/degradation behaviour of alginate beads crosslinked with Ca2+ and Ba2+ ions. React. Funct. Polym. 2004, 59, 129–140. [Google Scholar] [CrossRef]
- Melchels, F.P.W.; Dhert, W.J.A.; Hutmacher, D.W.; Malda, J. Development and characterisation of a new bioink for additive tissue manufacturing. J. Mater. Chem. B 2014, 2, 2282–2289. [Google Scholar] [CrossRef]



| Pluronic Concentration | 24.5 wt % | 30 wt % |
|---|---|---|
| Power law index (n) | 0.0511 | 0.1656 |
| Apparent viscosity (Ƞ) | 1.04 * Pa.s | 1.46 Pa.s |
| Needle length (L) | 0.005 m | 0.005 m |
| Nozzle Size | Stage Moving Speed (m/s) | Gauge Pressure × 105 (Pa) | ||
| 1 | 2 | 3 | ||
| 21G (514 μm) | 0.01 | 566.17 ± 14 | 2668.05 ± 10 | 3215.85 ± 24 |
| 0.02 | 368.75 ± 7 b | 1771.66 ± 30 | 2237.90 ± 20 | |
| 0.03 | 325.48 ±2 b | 1512.28 ± 7 | 1852.55 ± 15 | |
| 25G (260 μm) | Stage Moving Speed (m/s) | Pressure × 105 (Pa) | ||
| 1 | 2 | 3 | ||
| 0.01 | N/A a | 325.06 ± 17 b | 1447.95 ± 1 | |
| 0.02 | N/A a | 286.72 ± 33 b | 527.68 ± 19 b | |
| 0.03 | N/A a | 220.72 ± 23 b | 406.20 ± 13 b | |
| 27G (210 μm) | Stage Moving Speed (m/s) | Pressure × 105 (Pa) | ||
| 1 | 2 | 3 | ||
| 0.01 | N/A a | 254.69 ± 9 b | 435.47 ± 7 b | |
| 0.02 | N/A a | N/A a | 385.46 ± 11 b | |
| 0.03 | N/A a | N/A a | 281.86 ± 18 b | |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suntornnond, R.; Tan, E.Y.S.; An, J.; Chua, C.K. A Mathematical Model on the Resolution of Extrusion Bioprinting for the Development of New Bioinks. Materials 2016, 9, 756. https://doi.org/10.3390/ma9090756
Suntornnond R, Tan EYS, An J, Chua CK. A Mathematical Model on the Resolution of Extrusion Bioprinting for the Development of New Bioinks. Materials. 2016; 9(9):756. https://doi.org/10.3390/ma9090756
Chicago/Turabian StyleSuntornnond, Ratima, Edgar Yong Sheng Tan, Jia An, and Chee Kai Chua. 2016. "A Mathematical Model on the Resolution of Extrusion Bioprinting for the Development of New Bioinks" Materials 9, no. 9: 756. https://doi.org/10.3390/ma9090756
APA StyleSuntornnond, R., Tan, E. Y. S., An, J., & Chua, C. K. (2016). A Mathematical Model on the Resolution of Extrusion Bioprinting for the Development of New Bioinks. Materials, 9(9), 756. https://doi.org/10.3390/ma9090756







