2.1. Fingerprinting of Minerals and Rocks with CF-HP 129Xe NMR
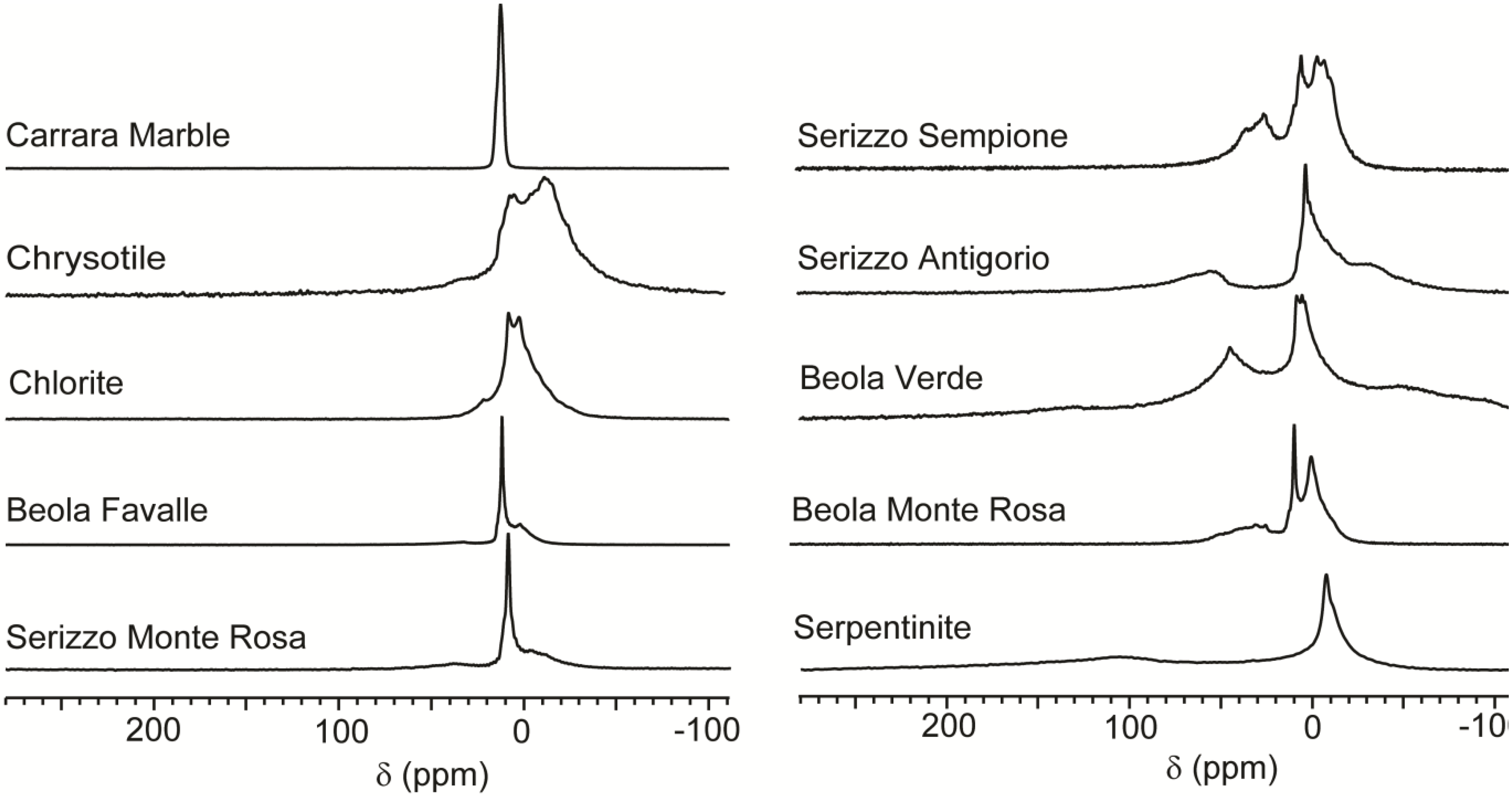
Figure 2 shows a selection of the CF-HP
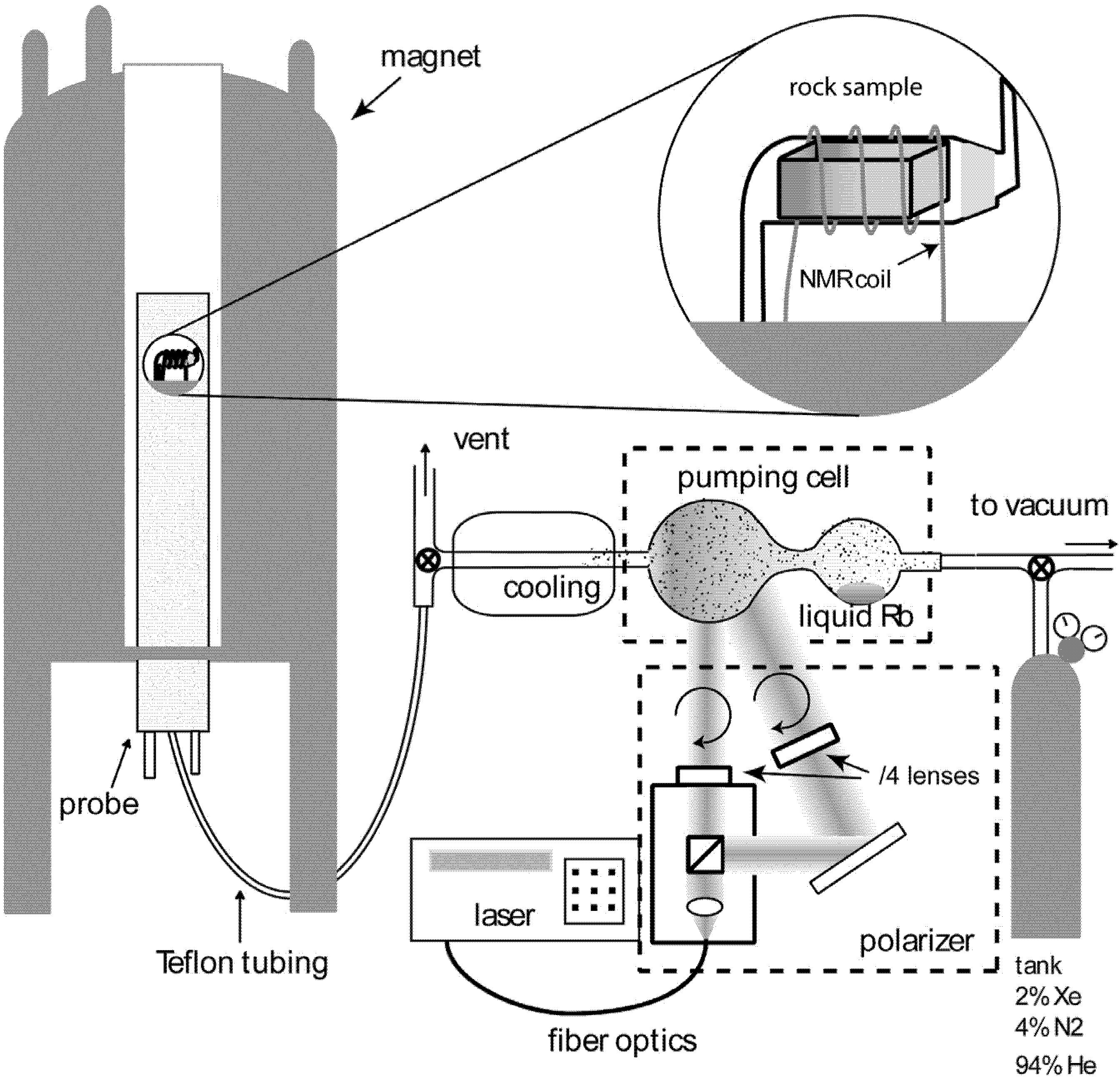
129Xe NMR spectra acquired of minerals and rocks. Samples were shaped as regular rectangular cuboids (50 × 6 × 6 mm, see experimental section for more details) in order to fit in the NMR detection coil. Fibrous chrysotile was loosely packed in the sample holder. Once prepared, the cuboids were equilibrated with the atmosphere at room temperature for several days.
Figure 2.
Continuous flow hyperpolarized xenon (CF-HP) 129Xe spectra of mineral and rock samples. Signal intensities were normalized arbitrarily to similar height for a more immediate comparison.
Figure 2.
Continuous flow hyperpolarized xenon (CF-HP) 129Xe spectra of mineral and rock samples. Signal intensities were normalized arbitrarily to similar height for a more immediate comparison.
The spectra displayed in
Figure 2 demonstrate the variety of interactions between stones and xenon gas. Since it has been reported that bulk diamagnetic susceptibility can affect the measured chemical shift of xenon [
32] and krypton [
33] in the case of macroscopic samples, for each cuboid, several CF-HP
129Xe NMR spectra were acquired changing the position and orientation of the cuboid in the sample holder. However, for the materials studied, no differences in the spectra were noticed. Also, spectra acquired from different slices of the same rock provided similar results. Thus each spectrum shown is representative of the material studied. A common feature of the spectra is a peak of varying line width around 0 ppm, but most samples also present a rich variety of signals. Due to the transient nature of the hyperpolarization, and to the low sensitivity of thermally polarized xenon, it is not possible to provide an internal standard in the form of sealed xenon volume. To define a chemical shift reference, a CF-HP
129Xe NMR spectrum was recorded during flow of hyperpolarized xenon in an empty sample holder, and the obtained signal of free xenon gas was set to 0 ppm. The signal of free xenon gas in the presence of rocks can be slightly (<10 ppm) shifted from the above-defined zero. This shift does not indicate a specific interaction, but rather the effect of the bulk material on the local magnetic field.
Some samples, exemplified by the Carrara Marble, display only a single peak around 0 ppm with 1 ppm line width or less. This is also the case of Rosso Verona marble (not shown in figure). Carrara Marble is a white veined metamorphic rock with a uniform saccaroid crystalline structure, with crystals ranging from 200 to 600 μm in size and an open porosity of around 2%. The components are mostly dolomite and quartz and other very compact rocks. In this case, the only detected signal is attributed to free xenon flowing around the sample without interaction. Moreover, interactions between the gas and a surface should cause a decrease in T
2 relaxation time and a concurrent line width increase that was not detected in systems with such low porosity. In fact, all other samples also display a signal around 0 ppm that is broader compared to the cases of Carrara Marble and Rosso Verona. Free xenon gas is always present, since the micro- and mesoporosity of rocks is not sufficient to confine all xenon atoms delivered by the gas flow during the NMR acquisition time, but macroporosity and bulk diamagnetic susceptibility effects [
32] produce broader resonances. Several samples present, in addition to free gas resonance, other signals at different chemical shifts whose interpretation needs a detailed analysis of the single stone.
The spectrum of chrysotile has several partially overlapping resonances in the region between 10 and −30 ppm. The line shape is difficult to fit as summation of Gaussian or Lorentzian functions, but at least one peak at −15 ppm is distinguishable from the peak at 0 ppm. Chrysotile is a mineral with a very large surface available for interaction with the gas, since it is formed by a bundle of fibrils (diameter < 1 μm), composed of microfibrils (diameter < 50 nm). Chrysotile is a phyllosilicate with the chemical formula Mg3(Si2O5)(OH)4. In real materials, a part of the Mg atoms is replaced by Fe or other atoms. This geometric and compositional complexity explains the presence of several overlapping resonances in the spectrum. As discussed in the introduction, negative chemical shifts for atomic xenon are rarely observed and they are associated with the presence of metal cations like Ag+ and Cu+ in close contact with the xenon atoms. Chrysotile is a natural material and it contains many metal cations at the ppm level and, among others, Cu+. Thus we suggest that copper cations, even though they are highly diluted in respect of the total bulk, are concentrated enough at the fibrils’ surface to provide a measurable effect. This effect is not limited to chrysotile, but also appears in several other samples that contain phyllosilicates, vide infra.
The spectrum of chlorite also has different resonances around zero, spanning a spectral region between 10 and −10 ppm. The upfield signal is so close to the gas peak that they partially overlap. Both chlorite and chrysotile also presents a downfield signal appearing as a small peak around 25 ppm. This shift is compatible with the presence of an accessible mesoporous system. In the case of chrysotile, this is possibly due to the empty interfibril spaces rather than adsorption by inherent porosity of the silicate, while in the case of chlorite the effect could be due to grain structure. In both phyllosilicates xenon does not give any large (> 100 ppm) downfield chemical shift signals as seen when the gas explores the interlamellar space of clays [
34]. Contrarily to synthetic clays that have been thoroughly emptied and whose galleries are made accessible, the interlamellar space of this sample is inaccessible.
The basic concepts developed in the study of minerals are useful in discussing the spectra of rocks such as different kinds of Beola or Serizzo samples, commercial names that indicate metamorphic gneisses of the Alpine region used as building stones for applications ranging from urban furniture to funeral art. For example, Beola Favalle and Serizzo Monte Rosa are rocks with different macroscopic appearance in terms of texture and color. Their mineralogical composition is instead similar, as indicated in
Table 1. They display very similar spectra, where the prevalent signal is the sharp peak attributed to the gas at −5 ppm, indicating a globally low surface interaction. They both display a partially overlapping peak around −15 ppm and a slightly more distant peak at 17 ppm (Beola Favalle) and 28 ppm (Serizzo Monte Rosa). Quartz, plagioclase and potassium-feldspar, the main components of those two rocks, are tectosilicates: very compact minerals without pores accessible to xenon. Upfield and downfield shifts are attributed to biotite or muscovite, phyllosilicates present in both samples.
Biotite is a dark, iron-rich 2:1 mica trioctahedral phyllosilicate with the idealized formula K(Mg,Fe
2+,Fe
3+)
3(AlSi
3O
10)(OH)
2. Biotite has a layered crystal structure and is found worldwide in granites and gneisses [
35]. Muscovite is instead a dioctahedral, with composition (KAl
2(AlSi
3O
10)(F,OH)
2). The occurrence is similar, and the two minerals are often found in the same rock, but muscovite has no iron content and is sometimes labeled “white mica” due to its color. The connection between mineral composition and spectra is demonstrated by
129Xe probes of the material at the microscopic level.
Other rocks, including Beola Verde, Beola Monte Rosa, Serizzo Sempione and Serizzo Antigorio display signals both upfield and downfield. The downfield peak is shifted more than 50 ppm and is then attributed to accessible micropore systems. Line width values in the range of tens of ppm are due to a specifically strong dephasing interaction, or simply to diffusion through different adsorption zones [
36,
37]. An interesting comparison can be made between Serizzo Antigorio and Serizzo Monte Rosa. Serizzo Antigorio has a broad peak at around 60 ppm, while the other sample has no spectral features in that region. The main compositional difference from the Serizzo Monte Rosa is that the biotite fraction is almost double (12% against 7%). A similar comparison could be drawn between Beola Favalle and Beola Monte Rosa. In the latter, the muscovite fraction is around 8%, against the 4% of the former. The resulting CF-HP
129Xe spectra suggest that once the percentage of phyllosilicates increases, they are not present as isolated spots but create a complex system of multiple sized pores, resulting in a residence time that is long in the NMR timescale and affects xenon resonance significantly. The spectrum of Beola Monte Rosa has several well-separated peaks that can be identified unambiguously with peak picking algorithms.
This material was then explored in greater detail to elucidate the nature of the shift, the connectivity between pores and the applicability of the models reported in the introduction.
Evaluation of xenon gas transport into the rock was performed by measuring the intensity of the peaks of Beola Monte Rosa as function of a buildup time, defined as the waiting time between a train of 90° saturation pulses, that destroys all the polarization inside the detection coil, and a reading pulse [
7,
38]. The signal intensity reached a plateau value in about 1000 ms uptake time without significant changes in the line shape, the value is to be compared to 200 ms for the empty sample holder. In order to understand this result, we should consider two limiting situations. Considering the Fickian diffusion in one dimension, like in mesopores, the mean square displacement of xenon can be evaluated by
where D is the diffusion coefficient. In uptake experiments, we can consider the xenon mean square displacement with a good approximation as the depth of the skin probed by the gas inside the sample. In the limit of macroporosity, xenon travels unhindered through the sample with a diffusion coefficient estimated by Mair in the range of 10
−6 m
2/s. In one second, the probed depth is then in the millimeter range. With the given sample shape (six by six section cuboid) the gas saturates a significant percentage of the sample volume prior to acquisition. In the presence of single-file diffusion, the mean square displacement of xenon can be evaluated by
, where
F(θ) is known as the single-file mobility and
θ is the fractional channel occupancy; in this case xenon atoms can be hindered further, to values of
F(θ) as small as 10
−12 m
2/s
1/2 as estimated by Cheng and Bowers [
16] for microporous systems with pores sized less than 0.6 nm. In this case, one second buildup times correspond to a probed depth in the micrometer range, reducing the effective fingerprinting region to a minimal skin. Using intermediate values, like 10
−9 m
2/s, typical of Xe diffusion in zeolites [
39], and not considering single file-diffusion, the probed depth is 30 µm. If this is the case, during the measurement we are probing 2% of the volume of the rocks.
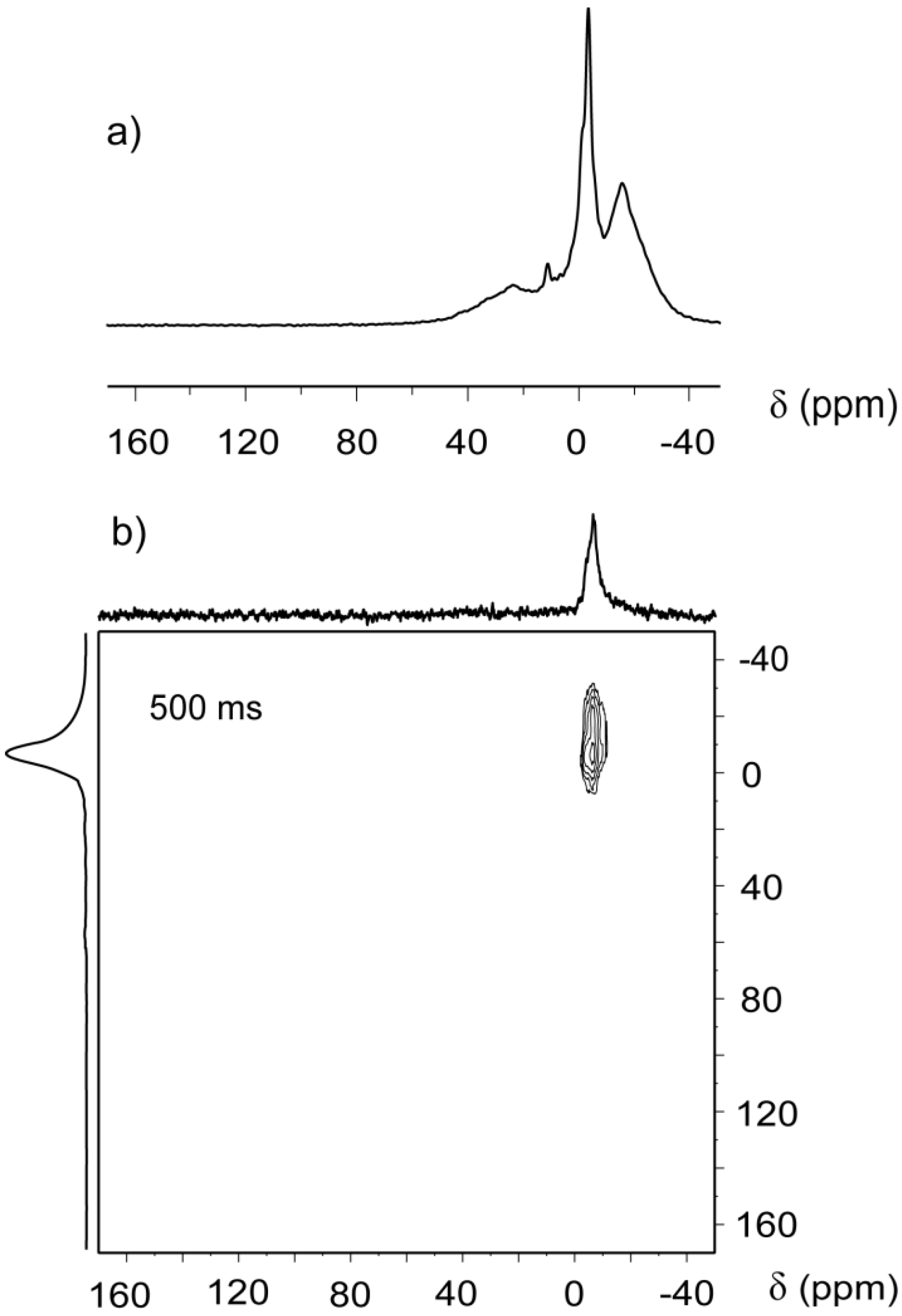
Two-dimensional CF-HP
129Xe (EXSY) exchange experiments [
9,
16] were also performed on the same Beola Rosa cuboid. The 2D spectrum with an exchange time of 500 ms, shown in
Figure 3b, contained only an “on diagonal” peak at −6 ppm without the rich spectral features present in the 1D spectrum replotted in
Figure 3a. The absence of peaks is due to the flow that continuously purges away the xenon atoms during the mixing period of the EXSY sequence. During the mixing time, xenon atoms do not remain within the pores that generate the −20, 11 and 23 ppm shifts, nor do they have any significant exchange with other pores. Instead, the single signal at −6 ppm is due to a system of macropores with low surface to volume ratio but capable to maintain enough xenon inside the coil for signal detection, at least 500 ms.
Rather than a system of interconnected pores with different sizes, the system is best represented as a combination of several different sized pore systems that are effectively independent and organized in shallow accessible regions close to the surface, in agreement with the buildup measurements.
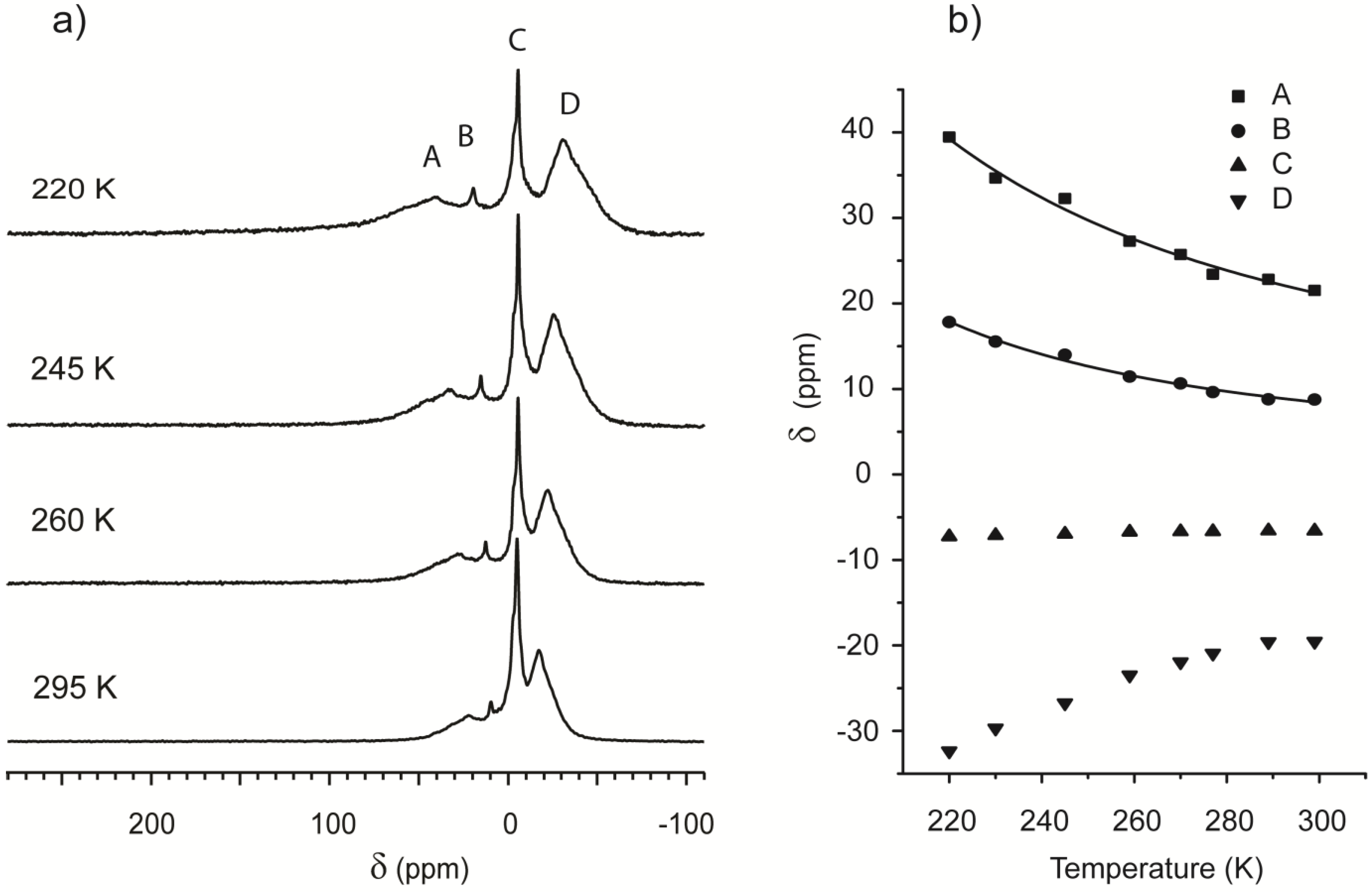
Further experiments were performed using a liquid nitrogen cooling system to cool both the sample and the incoming flow. CF-HP 129Xe NMR spectra were then acquired at intervals of 10 K from room temperature to 220 K.
Figure 4 contains a selection of the spectra obtained at different temperatures: Peaks remain well defined and separate in the probed range, indicating several non-interchanging adsorption regions. Peak picking was performed on all the spectra, and the values are plotted in
Figure 4b. In the framework of Equation (3), temperature dependence of the shift is a function of the adsorption parameters of each region.
Figure 3.
(a) CF-HP 129Xe spectrum of Beola Monte Rosa; (b) two-dimensional continuous flow hyperpolarized (2D EXSY) spectrum acquired with 500 ms exchange time and 1 s recycle delay. F1 and F2 projections are also depicted.
Figure 3.
(a) CF-HP 129Xe spectrum of Beola Monte Rosa; (b) two-dimensional continuous flow hyperpolarized (2D EXSY) spectrum acquired with 500 ms exchange time and 1 s recycle delay. F1 and F2 projections are also depicted.
Figure 4.
(a) 129Xe spectra of Beola Monte Rosa at different temperatures; (b) 129Xe NMR chemical shift for the main resonances present in Beola Monte Rosa spectrum as function of the temperature.
Figure 4.
(a) 129Xe spectra of Beola Monte Rosa at different temperatures; (b) 129Xe NMR chemical shift for the main resonances present in Beola Monte Rosa spectrum as function of the temperature.
The chemical shift of the peak indicated with the letter C is insensitive to temperature variation. Since it is located at −7 ppm and is the most intense, it is attributed to free gas. A common feature of the other chemical shifts is an apparent convergence toward free gas value at high temperature. Peak D, always being upfield, falls outside the strict definition of Equation (3). The data of the downfield Peaks A and B are instead plotted and fitted using the same equation, confirming that the origin of the chemical shift is consistent with the model presented in
Figure 1.
2.2. Transient Phenomena on Rock Surfaces as studied by CF-HP 129Xe NMR
The experiments described in the previous section were performed on cuboid samples, but the only limitation on sample form is the diameter of the NMR coil where the sample is positioned. Any stone material can be ground to powder and packed loosely in the sample holder. Sample powdering increases surface to volume ratio and should in principle enhance signals in regions associated with porous systems while reducing the relative intensity of the spectral region of free gas. In this way we could obtain more information on samples that are not sufficiently responsive in cuboid shape.
One such sample is serpentinite, a green rock that is made up mostly of antigorite and contains 4% magnetite Fe
3O
4. Magnetite, being ferromagnetic, generates a magnetic field that increases the relaxation of the hyperpolarized gas. The lamellar antigorite should favor this interaction by providing accessibility. The CF HP
129Xe spectrum shown in
Figure 2 presents a broad peak centered at δ = −9 ppm and an even broader spectral feature at around 105 ppm. The peak nearest to zero is typical of free gas interacting with a bulk material, as seen in several spectra in
Figure 2. Compared to the multiple signals of Beola Rosa, the spectrum of serpentinite is quite featureless, possibly due to the width of the signal associated to surface interaction (several tens of ppm). To enhance the signal, we ground the serpentinite cuboid to a fine dust using mechanical methods, and then acquired CF HP
129Xe NMR on the resulting powder.
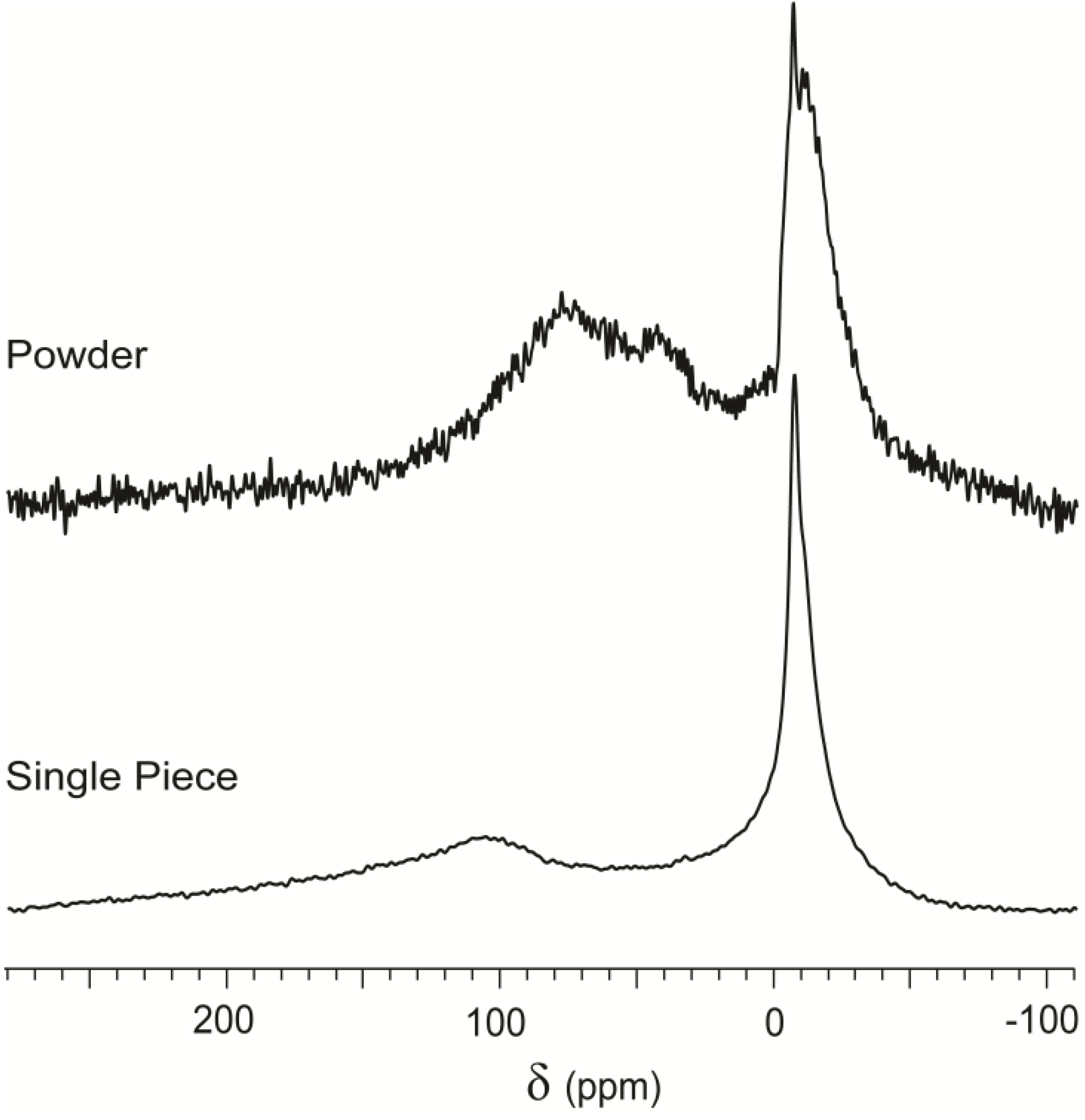
The spectrum of xenon interacting with a single cuboid of serpentinite is replotted in
Figure 5 for comparison with the same material in powder form. Relevant differences appeared in chemical shift, relative intensity of the peaks and total signal intensity. In the powder spectrum, the downfield spectral features (
δ > 20 ppm) are enhanced relative to the peak associated to free gas, as predicted for an increase of accessible surface. The chemical shift also changed, from a broad peak centered at more than 100 ppm to a complex signal constituted by at least two peaks around 65 and 40 ppm. Signals in
Figure 5 have been normalized to the same height for better readability, so the difference in signal intensity appears as significant noise in the spectrum of powdered material. Thus, increased accessible surface produced a decrease of the NMR signal by more than one order of magnitude.
To explain this counterintuitive behavior, we must consider that hyperpolarized Xe magnetization is far removed from the polarization due to the Boltzmann distribution of nuclear spins at thermal equilibrium in a static magnetic field. Thus the high polarization state is transient, and strongly depolarizing interactions with the material during the recycle delay reduce signal intensity. This is usually not an issue due to the amount of xenon flowing in the rf coil continuously, but magnetite is a powerful natural magnetic material, and powdering increases its accessibility. Changes in chemical shift also indicate a qualitative difference between the initial surface and the powder. Since the surface of the single piece was exposed to the atmosphere for several days before the experiment, and the powder was not, we can attribute the spectral differences to the interaction with atmospheric gases. For example, oxygen promotes the conversion of magnetite to hematite, with the reaction:
Figure 5.
CF-HP 129Xe NMR spectra of serpentinite powder and bulk material with the same gas flow and number of scans, normalized to the same maximum intensity of the highest peak.
Figure 5.
CF-HP 129Xe NMR spectra of serpentinite powder and bulk material with the same gas flow and number of scans, normalized to the same maximum intensity of the highest peak.
Rocks in the earth crust usually contain redox couples (like magnetite/hematite) whose equilibria are defined by mineral redox buffers, and are determined by local oxygen fugacity. When the rock surface is in contact with the atmosphere, the equilibrium shifts locally towards the most oxidized term.
This general concept is not limited to redox buffers. Paramagnetic centers embedded in the rock can be sensitive to atmospheric oxidation once exposed and can be changed into other, less active, species.
For a detailed study of sample evolution over time as function of weathering, we cut a new cuboid-shaped sample from the bulk of a Serizzo Monte Rosa rock. As indicated in
Table 1, this particular Serizzo contains more than 10% phyllosilicates (muscovite and biotite), thus providing a variety of local environments with zones of high density and zones of potentially accessible porosity with different chemical compositions. The sample was inserted into the sample holder immediately after the cut to minimize interaction with the atmosphere. The first CF HP
129Xe NMR spectrum was acquired as soon as possible after turning on the continuous flow. Other spectra were acquired under different conditions, and details are shown in
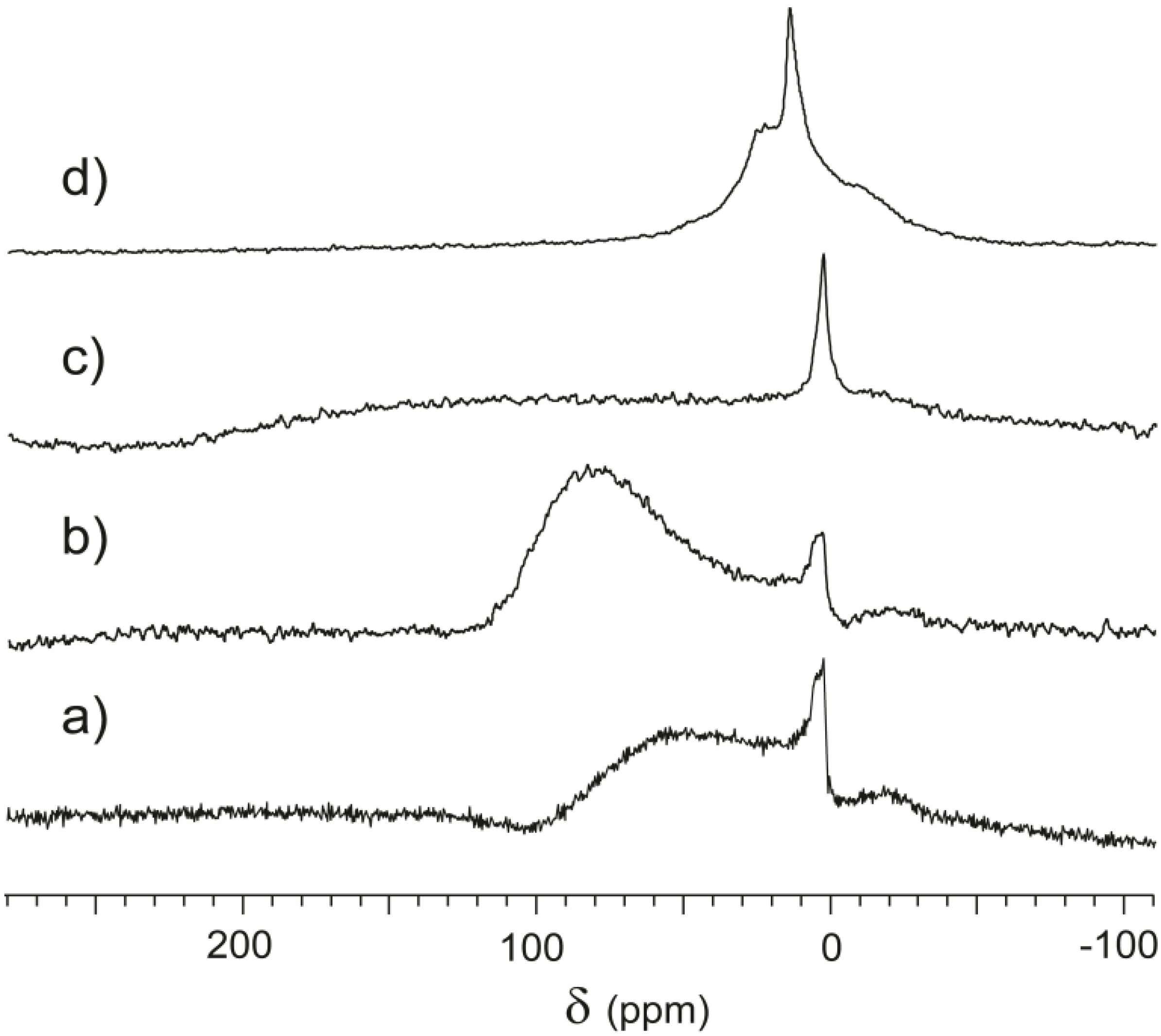
Figure 6.
In Spectrum 6a, it is possible to separate a peak around 0 ppm, attributed to the gas, and a very broad downfield spectral feature centered around 60 ppm and attributed to the rock surface. Total signal intensity is low, as demonstrated by high noise, similar to the case of powdered serpentinite represented in
Figure 5. Spectrum 6b, acquired after 30 minutes of continuous flow, also contains the same two features. The chemical shift of the broader peak increases to around 85 ppm and its intensity also increases. Actually, the integral of the downfield signal far surpasses the integral of the peak around 0 ppm, indicating significant xenon adsorption on the sample.
Figure 6.
CF-HP 129Xe NMR spectra acquired from a Serizzo Monte Rosa sample with different treatments: (a) freshly cut sample; (b) thirty minutes Xe/ N2/He continuous flow; (c) four hours contact with the atmosphere; (d) twenty-four hours contact with the atmosphere.
Figure 6.
CF-HP 129Xe NMR spectra acquired from a Serizzo Monte Rosa sample with different treatments: (a) freshly cut sample; (b) thirty minutes Xe/ N2/He continuous flow; (c) four hours contact with the atmosphere; (d) twenty-four hours contact with the atmosphere.
In between the acquisition of Spectrum 6a and 6b, the sample was immersed into the flow without contact with the atmosphere. The flow contained only xenon and its buffer gases (N
2, He) and in particular is free of oxygen and water, since it was in contact with extremely reactive Rb vapor during polarization. Rb vapors were in turn thoroughly removed by cooling the gas mixture outside the polarization cell. Such a mixture of inert gases cannot generate chemical modification on the surface. The variation in spectral response of the sample depends on physical sorption and desorption phenomena. The stone-cutting process could not be performed in vacuum, so even the freshly cut sample was in contact with the atmosphere for a few minutes during sample preparation. Thus, pores could be obstructed by atmospheric moisture or other molecules and become inaccessible to xenon atoms. The dry flow promoted desorption of those molecules, thus increasing the accessibility and explaining the differences in intensity between Spectrum 6a and 6b. To interpret the differences in chemical shift, we should mention the Strong Adsorption Site (SAS) model formulated by Fraissard [
40] during variable Xe pressure studies of zeolite materials. In some samples, sites of different adsorption strength are present. Strong adsorption implies a large contribution to chemical shift and, concurrently, a long residence time of Xe atoms on the site. In such cases, chemical shift can be very high at low xenon concentration, since the gas is preferentially adsorbed on the SAS. Further increase of xenon concentration actually decreases the chemical shift. Since the SAS are already saturated, excess gas is adsorbed on less interacting sites and rapid averaging between adsorption sites produces a single signal with reduced δ. In our experimental setup, the xenon concentration is kept constant, but there is an effective increase of available sites due to desorption of entrapped molecules like water and oxygen. In those conditions, xenon selectively explores the most interacting sites now available, explaining the increase of chemical shift from Spectrum 6a to 6b.
When the sample was put in contact with the atmosphere for several hours, we obtained spectra where the peak around 0 ppm is prevalent. After four hours of weathering, we also observed a very broad spectral feature spanning hundreds of ppm and difficult to separate from the baseline, plotted in
Figure 6c. The exposed material adsorbed atmospheric chemicals to a much greater extent than during sample preparation resulting in a wide array of local environments. Co-adsorption is known to increase the chemical shift [
41], and the diffusion of xenon through volumes with highly different interactions causes line broadening. Spectrum 6c then represents a transient state in the evolution of the rock surface, where very high heterogeneity does not produce specific signals.
Finally, after a long equilibration time in air, the sample reverts to being similar to those in
Figure 2. This is displayed in Spectrum 6d, where a free gas peak is surrounded by two shoulders, representative of the equilibrium porous structure and the effect of the paramagnetic centers that are not susceptible to atmospheric alteration.