Effect of Potential-Determining Ions on Rheological Properties of Calcite Paste

Abstract

The mechanical properties of calcite suspension are predominantly affected by different ions dissolved in solution. In this work, natural and synthetic calcites were employed to investigate the influence of potential-determining ions (PDIs) (Ca²⁺, Mg²⁺, OH⁻, CO₃²⁻ and SO₄²⁻) on the zeta potential and rheological behavior of calcite paste. Electric double layer (EDL) models were proposed to further interpret the ionic adsorption mode and zeta potential evolution. Experimental results show that moderate addition of the positive PDIs Ca²⁺ and Mg²⁺ significantly increases the positive charge of calcite and enhances paste flow. Calcite exhibits higher zeta potential in Ca(NO₃)₂ but lower viscosity in Mg(NO₃)₂, which is attributed to the different affinity of Ca²⁺ and Mg²⁺ for the calcite surface. As for the negative PDIs OH⁻, CO₃²⁻ and SO₄²⁻, they make the calcite negatively charged with the order of $\left|{\xi }_{N{a}_{2}C{O}_3}\right|>\left|{\xi }_{N{a}_{2}S{O}_4}\right|>\left|{\xi }_{NaOH}\right|$. The negatively charged calcite paste exhibits much higher viscosity, which is against the conventional DLVO (Derjaguin–Landau–Verwey–Overbeek) theory. Lattice site screening and specific attraction induced by negative PDIs may be the reason for the phenomenon. This work provides a comprehensive understanding on the correlation between ionic adsorption, surface charge and particle interactions. These theories are enlightening for calcite application in many areas such as paper manufacturing, wall coating and heritage conservation.

1. Introduction

Calcite, one of the most widely distributed minerals in the world, plays a significant role in many environmental and geochemical processes such as CO₂ sequestration and the storage of oil and gas [1,2,3,4]. Calcite minerals are also widely used in many fields, including paper and plastic filling [5,6], medicine production [7,8] and the construction industry [9,10,11,12,13]. Correlated to the application of calcite paste, its macroscopic behaviors such as fluidity and viscosity mainly depend on the interaction forces between calcite grains. According to the DLVO (Derjaguin–Landau–Verwey–Overbeek) theory [14,15], the interparticle force is dominated by the competing effect of electric double layer (EDL) repulsion and van der Waals attraction. The adsorption of different ions, mainly potential-determining ions (PDIs), can modify the surface charge of calcite, which in turn affects the EDL repulsion and the suspension flow.

Currently, it is widely accepted that Ca²⁺, Mg²⁺ and CO₃²⁻, i.e., the main composition of carbonates, are the PDIs for calcite [16,17,18,19,20,21,22,23,24]. Other investigations believe that H⁺, OH⁻ and SO₄²⁻ are also PDIs due to their good affinity to calcite surfaces [25]. As has been reported [26,27,28], the variation of pH can change the surface charge of calcite by protonation and deprotonation reactions. As for SO₄²⁻, decreasing zeta potential and polarity changes were observed with increasing SO₄²⁻ concentration [29,30,31]. The adsorption of PDIs can change the surface charge of calcite and hence the interparticle force and suspension flow. However, due to the complex interactions between calcite and different PDI species, the macroscopic behavior of calcite suspensions possibly disobeys the conventional DLVO model [3,8,32]. In recent studies, repulsive hydration force has been proposed as an explanation, and the short-distance repulsion was attributed to the compression and dehydration process of counterions adjacent to the calcite surface [33,34,35]. Other investigations reported short-distance attraction between cleaved calcite surfaces, and this was ascribed to ion–ion correlated force [36]. Due to the abnormal characteristics of calcite, the correlation between PDI adsorption, zeta potential and calcite suspension rheology remains unclear.

In order to reveal the effect of different ions on zeta potential of calcite and the interparticle forces in calcite suspension, in this work, two calcites from natural and synthetic sources were employed, and a series of model solutions containing different suggested PDIs or indifferent ions with varied concentration were prepared to test the zeta potential of natural and synthetic calcite. EDL models were also proposed to further interpret the ionic adsorption mode and zeta potential evolution. Meanwhile, a rheology test was conducted on natural calcite paste to interpret the dominant interactions in dense calcite suspension. The results exhibit a comprehensive understanding on the correlation between ionic adsorption, zeta potential and calcite paste rheology; they also present a vital insight for calcite application in many fields using surface charge control.

2. Materials and Methods

2.1. Materials and Samples

In this research, two calcites from natural and synthetic sources were used for experiments. The synthetic calcite was an analytical reagent of calcium carbonate (AR CaCO₃), and the natural calcite was a commercial product from Yangzhou, China, with an average particle size of 5.7 μm; the particle size distribution of natural calcite is shown in Figure 1. The chemical compositions of the two calcites are listed in

Table 1. The chemical composition of natural and synthetic calcites (wt.%).

	CaCO3	MgCO3	SiO2	Al2O3	Fe2O3	SO3	K2O	SrO	NiO	MnO	Cr2O3
Syntheticcalcite	>99	0.05	-	-	0.001	0.01	0.005	0.05	-	-	-
Natural calcite	91.05	7.19	1.21	0.30	0.11	0.05	0.03	0.02	0.02	0.01	0.01

. The composition of the synthetic calcite is provided by the manufacturer (Tianjin Fuchen Chemical Reagent Factory, Tianjin, China), whereas the composition of natural calcite was determined by X-ray Fluorescence Spectrometer.

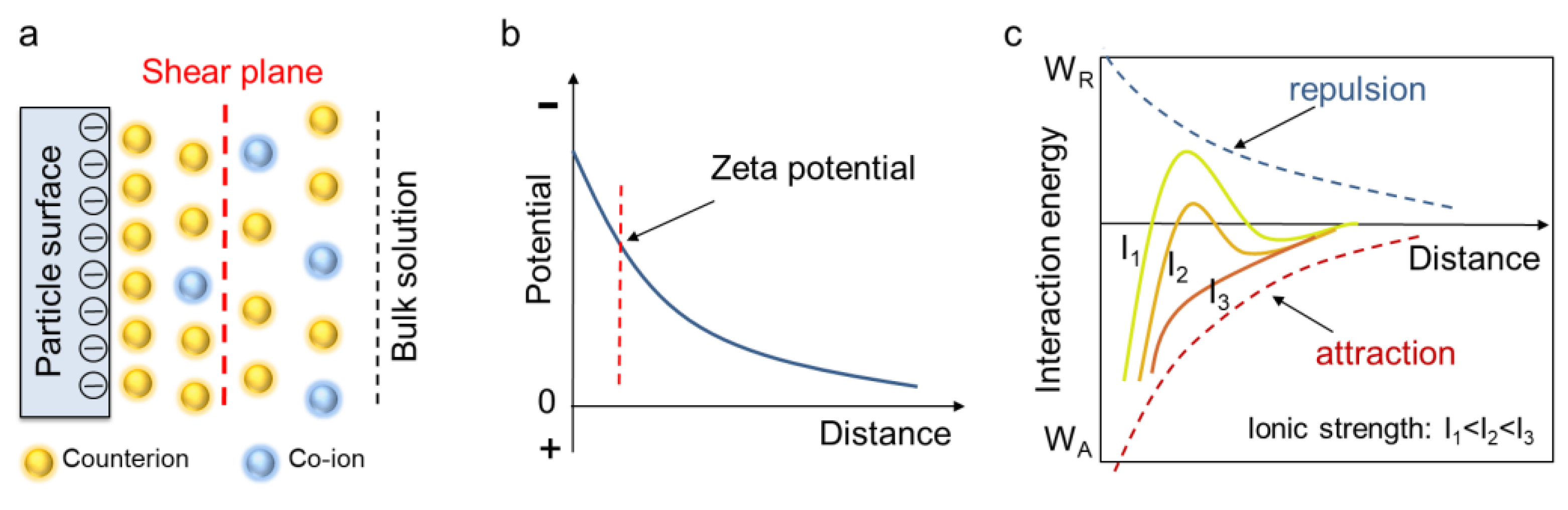
2.2. Zeta Potential Test and DLVO Theory

The surface of minerals usually carries a different charge in aqueous solution. Negatively charged surfaces, for instance, can adsorb cations (i.e., counterions) to balance the surface charge, which forms the electric double layer (EDL) (see Figure 2a). During the electrophoresis process, counterions adjacent to the mineral surface are firmly adsorbed and will move together with the particle, whereas the counterions at a greater distance from the mineral surface remain with the bulk solution. The sliding boundary between these counterion layers is called the shear plane, and the potential at the shear plane is the zeta potential, which can be determined by equipment (Figure 2b). Particles containing the same charge exhibit electric repulsion (or EDL repulsion) between each other, which helps to keep the suspension stable; on the other hand, there is also van der Waals attraction between particles, which leads to flocculation and accumulation. The competitive effect of electric repulsion and van der Waals attraction is described in the DLVO theory, which was proposed by Derjaguin, Landau, Verwey and Overbeek in 1948 [14,15]. The valance and concentration of ions (collectively named ionic strength) can change the zeta potential as well as the magnitude of the electric repulsion, thus altering the DLVO curve and the status of a mineral suspension. Zeta potential testing and DLVO theory provide an effective approach to investigate the rheological properties of different mineral suspensions, since the ionic adsorption can tune the particle interactions, thus enhancing or inhibiting suspension flow.

In order to reveal the effect of different ions (Na⁺, K⁺, Ca²⁺, Mg²⁺, OH⁻, CO₃²⁻ and SO₄²⁻) on the zeta potential and rheological behavior of calcite paste, a series of model solutions were prepared with the analytical reagents NaNO₃, KNO₃, Ca(NO₃)₂, Mg(NO₃)₂, NaOH, Na₂CO₃ and Na₂SO₄ in deionized water (DIW), and the concentration varied from 0.1 to 80 mM. In order to maintain the ionic strength at low concentrations, a background electrolyte of 10 mM NaNO₃ was introduced in all tested solutions. It should be noted that the NaOH and Na₂CO₃ increase the alkalinity of the solution, so the pH was recorded for further analysis. All the solutions were freshly made to avoid the influence of atmospheric CO₂.

Zeta potential tests were conducted with a NanoBrook Omni zeta potential analyzer (Brookhaven Instruments Corporation, Holtsville, NY, USA) based on a phase-analysis light-scattering method. The sample suspension was prepared by dispersing about 0.001 g calcite in 1.25 mL of model solution. In order to minimize the effect of particle settling, all samples were kept stationary for two minutes before measuring. Three replicated measurements with ten measuring cycles were conducted for each zeta potential test.

2.3. Rheology Test

The rheology test was conducted using a Brookfield R/S Plus Rheometer (Brookfield, Middleboro, MA, USA) with a coaxial cylinder of type CC40 at room temperature. The size of the coaxial cylinder is shown in Figure 3a. The calcite paste was prepared by mixing 200 g of natural calcite with 90 g model solution in a Hobart mixer, thus obtaining a dense calcite paste with a solid–liquid ratio of 0.45 by weight. Then, 68.5 mL of fresh mixed calcite paste was transferred into the cylinder and the rheological test was conducted immediately. The concentrations of model solutions for rheological experiments were in the gradient of 1 mM, 10 mM, 20 mM, 40 mM and 80 mM, with 10 mM background electrolyte NaNO₃ included. Compared with the concentration of model solutions, the ionic increase induced by calcite dissolution is much smaller (0.15 mM), which has negligible effects on the rheological behavior of calcite paste. The rheological curves were obtained by increasing the shear rate from 0 to 150 s⁻¹ in 90 s, and shear stress and viscosity were automatically recorded by the equipment.

3. Results and Analysis

3.1. Effect of Indifferent Ions

Figure 4a,b exhibit the zeta potential evolution of two calcites in NaNO₃ and KNO₃ solutions. The result shows that the synthetic calcite is positively charged in pure water (+14.5 mV), whereas the natural calcite is negative (−15.0 mV). With an increase in electrolyte concentration, the zeta potential of both calcites decreases, and the gradient seems higher in the NaNO₃ solution; typically, an isoelectric point (IEP) was observed for synthetic calcite when the NaNO₃ exceeded 40 mM. This distinction between Na⁺ and K⁺ is probably due to their different affinities to the carbonate surface, which is characterized by the “Covalency Index (CI)” and “Ionicity Index (II)” by Marchuk et al. [37,38,39]. The zeta potential result indicates that Na⁺, K⁺ and NO³⁻ are not PDIs for carbonates, and they change the zeta potential simply by tuning the EDL thickness (Figure 4c). The IEP observed for the synthetic calcite in the NaNO₃ solution is attributed to the overcompensation of counterions rather than specific adsorption.

The effect of the NaNO₃ concentration on the rheological behavior of natural calcite is shown in Figure 4d. Similar rheological curves were obtained in 10 mM NaNO₃ solution and deionized water (DIW). When NaNO₃ increased from 10 mM to 40 mM, the shear stress increased slightly from 142.7 Pa to 147.5 Pa at a shear rate of 150 s⁻¹. The rheology of this calcite paste indicates that the increasing indifferent ions have a very small effect on the interparticle force, which is in accordance with the zeta potential result.

3.2. Effect of Positive PDIs

The influence of the positive PDIs Ca²⁺ and Mg²⁺ on the zeta potential and rheological behavior of calcite paste was investigated with Ca(NO₃)₂ and Mg(NO₃)₂ solutions. Figure 5a,b show that both Ca²⁺ and Mg²⁺ can elevate the positive charge of calcites. For natural calcite, an isoelectric point (IEP) was observed at approximately 0.5 mM in both electrolytes, whereas the synthetic calcite was constantly positive over the entire concentration range. The maximum zeta potential was obtained at 10 mM or 20 mM, above which the zeta potential decreased again due to EDL compression. The zeta potential result confirmed that both Ca²⁺ and Mg²⁺ are PDIs for carbonates, and they are specifically adsorbed irrespective of the initial charge of different calcites. The configuration of the EDL was further illustrated in Figure 5c. According to previous reports [40,41,42], the specifically adsorbed Ca²⁺ and Mg²⁺ mainly distribute within the outer Helmholtz plane (OHP), which promotes the electric potential of the OHP and consequently the zeta potential at the shear plane. On the other hand, the negative counterions of NO₃⁻ are arranged in the diffusion layer by electrostatic attraction to ensure the neutrality of the EDL. The peak zeta potential indicates the completion of monolayer adsorption [18,20], and excessive PDIs will decrease the zeta potential by reducing the Debye length. It is also observed in Figure 5a,b that the synthetic calcite gains a much higher zeta potential in Ca²⁺ than in the condition of Mg²⁺; this may result from a closer shear plane to calcite surface distance, since the Ca²⁺ is more strongly bound due to a higher covalent index (Ca²⁺ = 0.33, Mg²⁺ = 0.27) [37,38,39]. It is noted that natural calcite is constantly more negative than synthetic calcite, which is in accordance with previous reports [21,42,43,44,45,46,47], and this is attributed to the presence of impurities (see Table 1) [17,48]. In natural conditions, impurities such as environmental solutes, minerals and organic matters may co-precipitate with calcite [49,50], which leads to a different initial charge on carbonates and consequently a different zeta potential evolution in model solutions.

The rheological behavior of natural calcite paste with Ca(NO₃)₂ solution is shown in Figure 5d. With the increase in Ca²⁺ concentration, the rheological curve descends significantly and reaches a minimum at 10 mM. On further increasing the concentration to 20 mM, the shear stress rises again due to EDL compression. The effect of Ca²⁺ on calcite paste rheology is basically in accordance with the zeta potential result (see Figure 5a). Obviously, moderate Ca²⁺ adsorption promotes the surface charge and enhances the EDL repulsion. In comparison, calcite reaches the minimum shear stress at a much higher concentration (40 mM) in Mg²⁺. Furthermore, the shear stress and viscosity are always smaller in Mg²⁺ than Ca²⁺ (Figure 5f), even though a higher zeta potential was obtained in Ca²⁺ at the same concentration. The difference between Ca²⁺ and Mg²⁺ is also probably attributed to their affinity to the calcite surface, which will be discussed in Section 4.2.

3.3. Effect of Negative PDIs

3.3.1. The Effect of OH⁻

Figure 6a shows that the zeta potential of synthetic calcite decreases monotonically with the increasing NaOH concentration, and the gradient is about −4.8 mV/decade. Particularly, an IEP was observed at 5–10 mM, which indicates the OH⁻ is also a PDI for calcite. The possible configuration of OH⁻ adsorption is further illustrated in Figure 6b. Different from Ca²⁺ and Mg²⁺ (also for CO₃²⁻), the OH⁻ ions mainly change the calcite charge in the hydrolysis layer (i.e., the hydrated >CaOH and >CO₃H sites, where “>” denotes chemical bond) by deprotonation [5,25,26,27,28]. As for natural calcite, the zeta potential firstly increases to a more negative state and then decreases again. The subsequent decrease may result from the enhanced adsorption of Na⁺ by impurities, which results in charge neutralization. A similar zeta potential trend was also reported in our previous investigation on quartz [38].

The rheological behavior of natural calcite with NaOH solutions was further investigated (Figure 6c). With the increase in OH⁻ concentration, a drastically increasing shear stress was observed on the rheological curves, even though the zeta potential varied within a very small range. Previous reports ascribed this phenomenon to ionic strength and electrostatic screening; however, in comparison to indifferent ions (see Figure 4 in Section 3.1), calcite paste exhibits much higher shear stress in OH⁻, even though ionic strengths are identical in the two electrolytes. The abnormal stiffening indicates a specific attraction between calcite particles which is beyond the DLVO correlated forces (EDL repulsion and van der Waals attraction), and this specific attraction leads to flocculation and hinders the paste flow.

3.3.2. The Effect of CO₃²⁻

The effect of CO₃²⁻ was investigated with model solutions of Na₂CO₃ reagent. The zeta potential result (Figure 7a) shows that both calcites become more negatively charged with the increasing Na₂CO₃ concentration, and a linear regression with a gradient of −8.2 mV/decade can be fitted to both curves within 0.5–20 mM. When the concentration exceeded 20 mM (or 40 mM for synthetic calcite), the zeta potential decreased again due to EDL collapse and enhanced adsorption of positive Na⁺ ions. The mechanism of CO₃²⁻ adsorption and zeta potential evolution was further illustrated in Figure 7b. Contrary to positive PDIs, the adsorption of CO₃²⁻ generates a negatively charged surface, and the positive Na⁺ counterions are arranged in the diffusion layer to balance the negative charge. It is noted that in Na₂CO₃ solution, the evolution of calcite charge is correlated to pH and the ionic species in solution. Firstly, possible carbonate species like H₂CO₃, HCO₃⁻ and CO₃²⁻ in Na₂CO₃ solution are highly dependent on pH (Figure 7c). With the increase in Na₂CO₃ concentration and consequently the pH (

Table 2. The pH of NaOH and Na₂CO₃ solution at different concentrations.

pH	1 mM	10 mM	20 mM	40 mM
NaOH	10.8	12.0	12.3	12.6
Na2CO3	10.3	11.1	11.3	11.4

), more monovalent HCO₃⁻ ions transform to divalent CO₃²⁻ and increase the negative charge. Secondly, the alkaline solution also leads to a deprotonation reaction on the calcite surface and enhances the negative charge [5,25,26,27,28].

The higher CO₃²⁻ concentration also leads to higher shear stress and viscosity (Figure 7d), which is similar to the effect seen in NaOH solution. However, the CO₃²⁻ seems less effective than OH⁻, because both shear stress and viscosity are lower in CO₃²⁻ than OH⁻ at 10 mM and 40 mM conditions, as shown in Figure 7e. The pH results in Table 2 indicate that the distinct rheological behavior of calcite in OH⁻ and CO₃²⁻ results from the different pH, the higher pH (i.e., OH⁻ concentration) creating the greater attractive force between calcite particles which hinders the suspension flow. From the rheology test, we also see a non-DLVO behavior in CO₃²⁻ since the calcite is highly negatively charged, which should generate repulsion and improve the paste flow. Herein, we proposed a specific attraction between calcite particles, and the mechanism is illustrated in Figure 7f. In the alkaline Na₂CO₃ solution, the intermediate negative Helmholtz layer, consisting of the negative PDI CO₃²⁻ and deprotonated sites >CaO⁻ can attract neighboring particles, which in turn inhibits the paste flow.

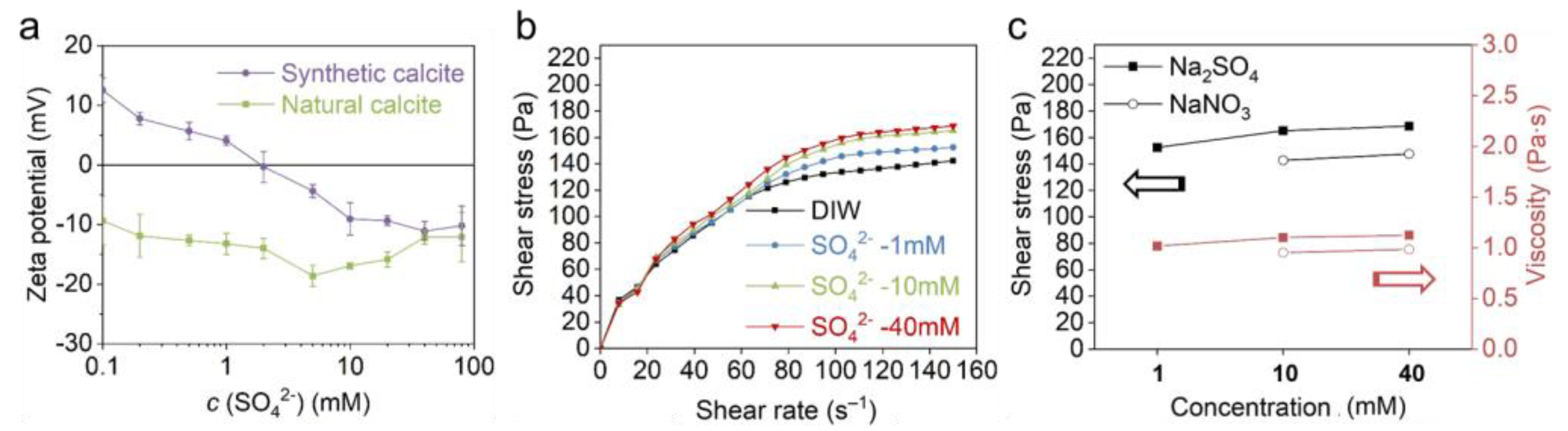
3.3.3. The Effect of SO₄²⁻

The effect of SO₄²⁻ was investigated with Na₂SO₄ solutions. Herein, the effect of pH can be ignored since the Na₂SO₄ solution is neutral. Figure 8a shows that the zeta potential of synthetic calcite constantly becomes more negative when Na₂SO₄ concentration increased to 40 mM, and the gradient is about −9.3 mV/decade. In addition, an IEP of 2 mM was also observed on the zeta potential curve. In contrast, the zeta potential of natural calcite is relatively moderate with an inflection point at 5 mM. The different zeta potential between natural and synthetic calcite is also attributed to the impurities, which leads to a different initial charge and consequently the zeta potential evolution. In addition, no IEP was observed on natural calcite since it is constantly negatively charged over the entire SO₄²⁻ range.

With respect to the calcite paste rheology (Figure 8b), the increasing SO₄²⁻ concentration also leads to higher shear stress. In comparison with OH⁻ and CO₃²⁻, the effect of SO₄²⁻ is less pronounced, but it is more effective than indifferent ions when the ionic strength is taken into consideration (Figure 8c). The stiffening of the calcite suspension indicates that, similar to CO₃²⁻, the SO₄²⁻ is also specifically adsorbed in the Helmholtz layer, thus leading to a specific attraction between calcite particles. Our suggestion is supported by Pourchet et al. [10]: it was detected by atomic force microscopy (AFM) measurement that a small amount of sulfate adsorption significantly increased the attractive interaction between calcite particles. The mechanism of SO₄²⁻ adsorption and particle attraction is referred to in Figure 7b,f.

4. Discussion

4.1. Zeta Potential and PDI Determination

In this work, the zeta potential of natural calcite is constantly more negative than synthetic calcite due to the presence of impurities, and similar results have also been reported by other researchers [17,48,49,50]. Given this, the identification of a PDI should not rely on the emergence of an IEP during the zeta potential test, because the IEP might not be encountered when the ionic polarity is the same as the initial charge of the calcite. For instance, the synthetic calcite exhibits no IEP throughout the entire Ca²⁺ and Mg²⁺ concentration range although they are both confirmed PDIs, and similarly, the natural calcite also has no IEP in OH⁻, CO₃²⁻ or SO₄²⁻ solutions. Herein, we proposed that the solubility of precipitates is feasible to define a PDI, or at least sequence the affinity of different ions to the calcite surface. For example, in this work, the solubility of anionic compounds follows the order of $S_{CaC{O}_3}<S_{CaS{O}_4}<S_{Ca{\left(OH\right)}_2}$, which is opposite to the maximum zeta potential of $\left|{\xi }_{C{O}_3^{2-}}\right|>\left|{\xi }_{S{O}_4^{2-}}\right|>\left|{\xi }_{O{H}^{-}}\right|$. Similarly, the solubility of $S_{CaC{O}_3}<S_{MgC{O}_3}$ is also opposite to the zeta potential of $\left|{\xi }_{C{a}^{2+}}\right|>\left|{\xi }_{M{g}^{2+}}\right|$. Our suggestion is supported by Fajans’ rule [51,52,53], which predicts that the ions that can form insoluble matter with the lattice ions of a matrix will be preferentially adsorbed. Furthermore, the rheology test in this research also indicates a feasible approach for PDI identification, since the calcite paste is insensitive to the concentration of indifferent ions.

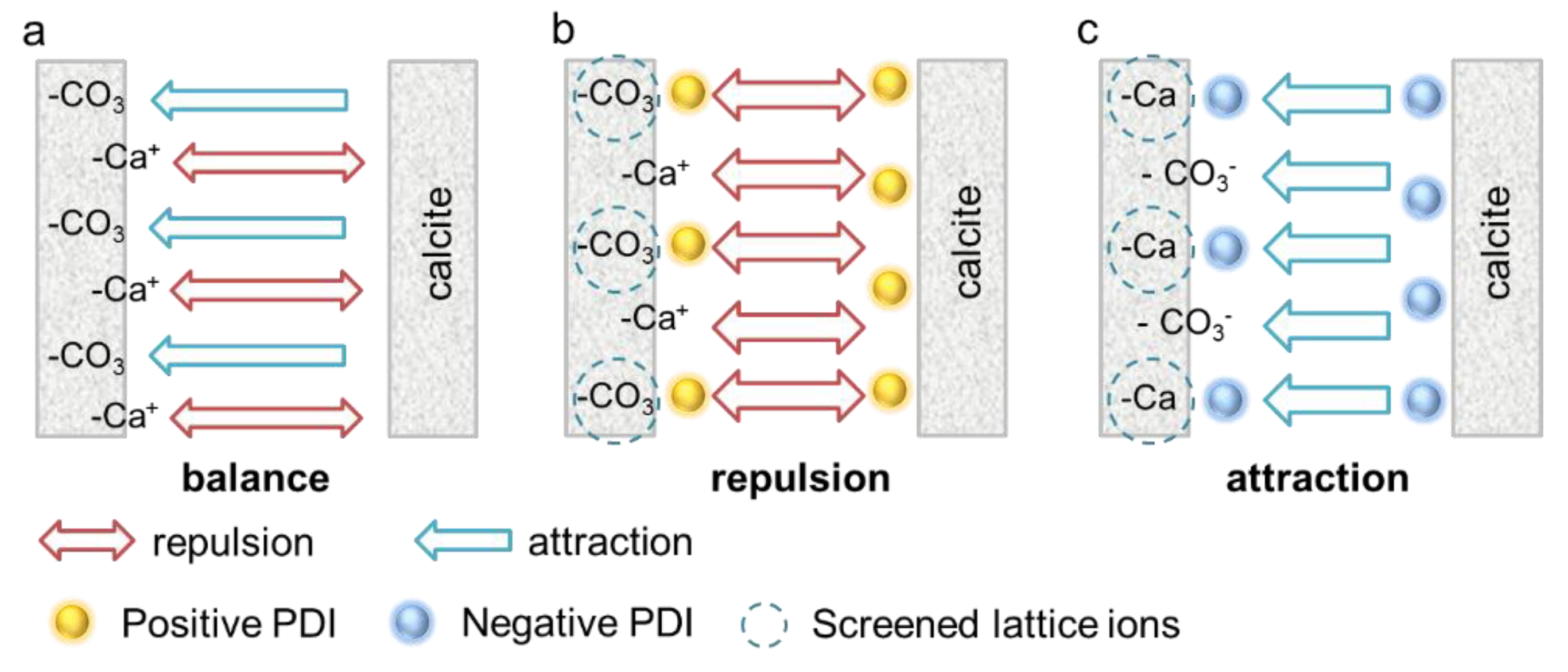
4.2. The Non-DLVO Behavior of Calcite Paste

In this work, non-DLVO behavior was observed in negative PDI conditions, where negatively charged calcite paste exhibits higher shear stress and viscosity. A similar result was also reported in other publications: Pourchet et al. [10] detected by AFM measurement an increasing attractive force between calcite particles when SO₄²⁻ was incorporated. Liberto et al. [8] also reported that NaOH completely screens the surface charge and leads to a more rigid calcite paste. In previous reports, the calcite stiffening was ascribed to ionic strength and electrostatic screening [8]. However, this cannot explain why the negative zeta potential generates attraction rather than repulsion. Moreover, it also cannot explain the distinct rheological behavior between OH⁻ and indifferent ions, i.e., both ions have a very small effect on zeta potential, but the OH⁻ significantly increased the viscosity of calcite paste whereas the indifferent ions have a negligible effect.

It is proposed here that the non-DLVO behavior in negative PDIs is dominated by a specific attraction beyond the regime of DLVO interaction. On a cleaved calcite surface, the positive >Ca²⁺ sites generate repulsion, whereas the negative >CO₃²⁻ sites induce specific attraction. The two forces are neutralized in DIW, as shown in Figure 9a. The incorporation of positive PDIs (Ca²⁺ and Mg²⁺) screens the negative >CO₃²⁻ sites and increases the repulsive force, thus enhancing the paste flow (see Figure 9b). On the contrary, the negative PDIs screen the positive >Ca²⁺ sites and promote the interparticle attraction (Figure 9c), which inhibits the suspension flow. Based on this model, it is expected that the effect of positive PDIs follows the DLVO model, i.e., the moderate adsorption of Ca²⁺ and Mg²⁺ enhances the EDL repulsion, and the negative PDIs act adversely even though the calcite is highly negatively charged. As for indifferent ions such as Na⁺, K⁺ and NO₃⁻, an increasing concentration will change the ionic strength and the Debye length, considering that the initial charge of calcite is weak, so they have negligible effect on calcite paste rheology, which also follows the DLVO model.

The secondary difference between Ca²⁺ and Mg²⁺ on calcite paste rheology is ascribed to different covalency indices and affinity to the calcite surface, where weakly attracted Mg²⁺ expands the Helmholtz layer and Debye length, and in turn enhances the EDL repulsion. As for negative PDIs, the OH⁻ binds more >Ca²⁺ sites, and the deprotonation process releases more >CO₃²⁻ sites, thus producing a stronger specific attraction and leading to a more rigid paste. In contrast, the weakly adsorbed SO₄²⁻ cannot entirely cover all >Ca²⁺ sites and produces the smallest specific attraction.

5. Conclusions

In this work, we systematically investigated the influence of positive PDIs (Ca²⁺ and Mg²⁺), negative PDIs (OH⁻, CO₃²⁻ and SO₄²⁻) and indifferent ions (Na⁺ and K⁺) on the zeta potential and rheological behavior of calcite paste. Zeta potential evolution and the ionic adsorption model were illustrated with different EDL models, and the rheological behavior of calcite paste was analyzed by interparticle force. Throughout this paper, the following conclusions can be drawn:

(1)

The adsorption of positive PDIs elevates the positive charge of calcite, whereas the negative PDIs make the calcite more negatively charged. Calcite exhibits higher zeta potential in Ca²⁺ than Mg²⁺, and in negative PDI solutions follows the order of $\left|{\xi }_{C{O}_3^{2-}}\right|>\left|{\xi }_{S{O}_4^{2-}}\right|>\left|{\xi }_{O{H}^{-}}\right|$. Indifferent ions such as Na⁺, K⁺ and NO₃²⁻ slightly change the zeta potential by tuning the ionic strength and Debye length.

(2)

The natural calcite is constantly more negative than synthetic calcite due to the existence of impurities, indicating that the detection of an IEP by zeta potential test is insufficient for PDI identification. Fajans’s rule, denoting the correlation between ionic adsorption and solubility of precipitates, provides a practical method for PDI identification. In addition, the rheology test also indicates a feasible approach, since the calcite paste rheology is insensitive to indifferent ions such as Na⁺, K⁺ and NO₃⁻.

(3)

The incorporation of positive PDIs significantly increases the positive charge of calcite and enhances the suspension flow, which basically follows the DLVO model. Ca²⁺ has a higher capability of improving calcite charge than Mg²⁺, whereas the Mg²⁺ is more capable in improving the suspension flow. The secondary differences may result from a different covalency index and affinity to the calcite surface, where Ca²⁺ is more strongly bound by calcite and the Mg²⁺ bond is relatively weak.

(4)

The increasing negative PDIs make the calcite negatively charged leading to a more viscous paste. Calcite paste exhibits non-DLVO behavior with negative PDI (OH⁻, CO₃²⁻ and SO₄²⁻) solutions, where negatively charged calcite paste exhibits much higher viscosity. Specific attraction and lattice site screening induced by negative PDIs may be the reason for this phenomenon. The deprotonation reaction by OH⁻ generates the highest specific attraction in calcite paste, thus inducing the greatest rigidity and viscosity, while the weakly attracted SO₄²⁻ induces a much smaller attraction. The interaction model, denoting an extended DLVO theory, provides a rational explanation for the non-DLVO behavior.

This study revealed how ionic admixtures affect the kinetics of calcite paste, and the results are inspiring for tuning the workability of carbonate suspensions via surface charge control. Relative mechanisms have broad applications in many industrial fields such as paper manufacturing, building materials and heritage conservation.