Fracture Analysis of Concrete Structures: Prediction Based on Boundary Effect Model
Abstract
1. Introduction
2. Linear Formulation for 3-P-B Geometry: Insights into Fracture Mechanics and Predictive Modeling
2.1. The Linear Boundary Effect Model (BEM): Core Concepts and Formulation
2.2. “Hall-Petch” Relation for Brittle Solids to Link dav with ft & KIC
2.3. Linear Function with Statistical Reliability
2.4. Comprehensive Results from the Literature in Statistical Reliability Analysis
3. Materials and Methods
4. Results and Discussion
4.1. Development and Evolution of Size Effect Models: From SEL to BEM
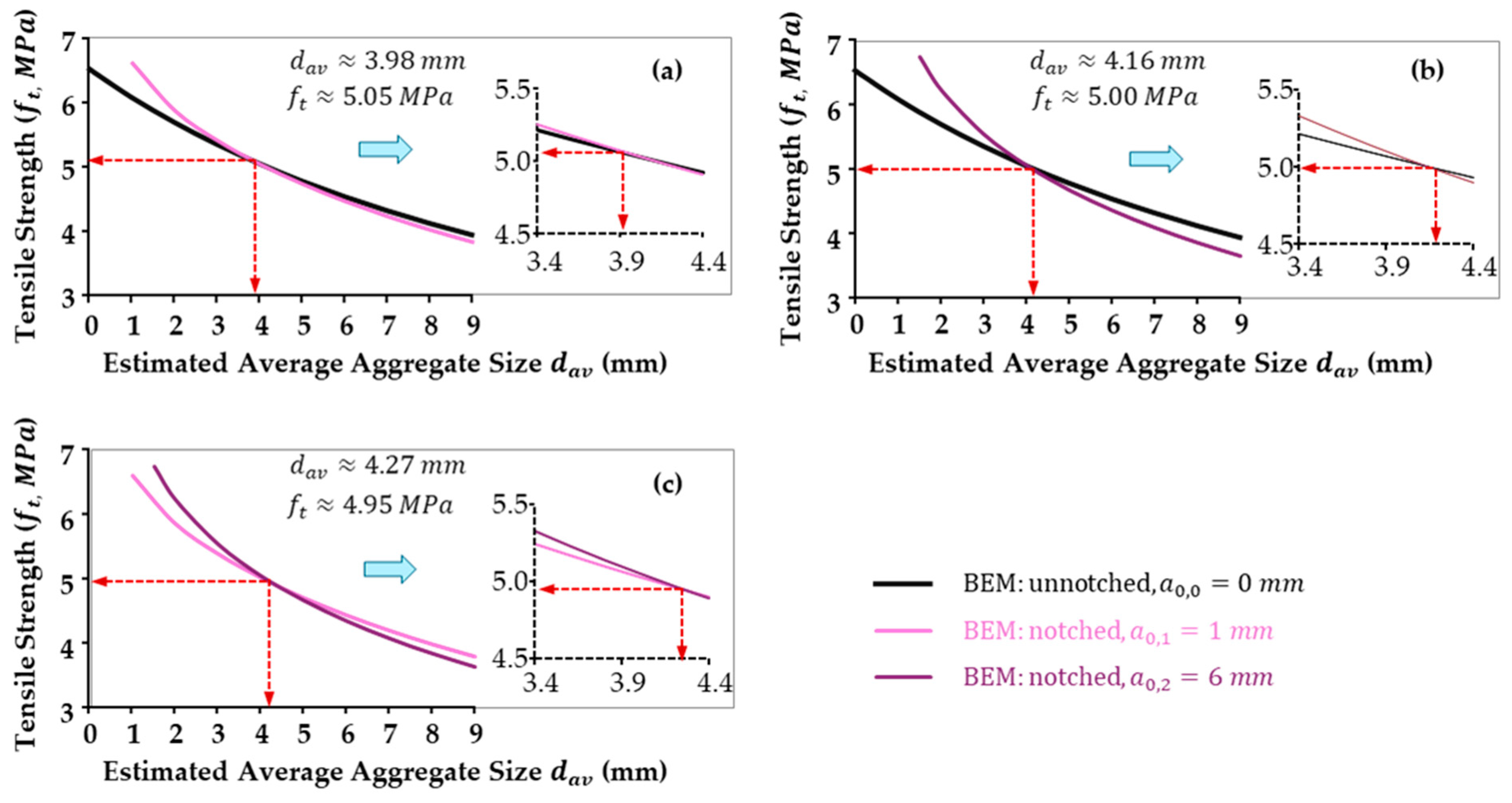
4.2. Aggregate Size Determination
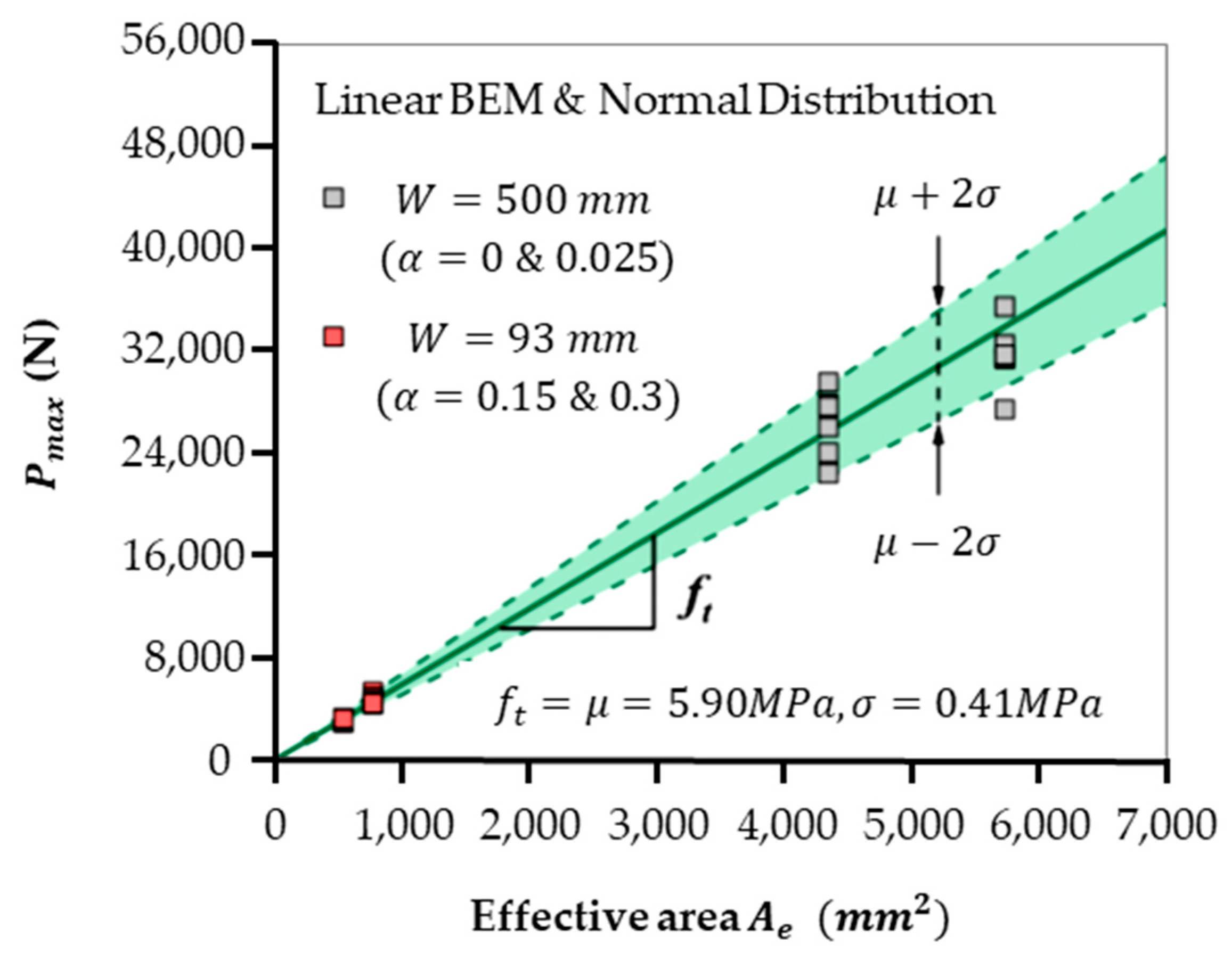
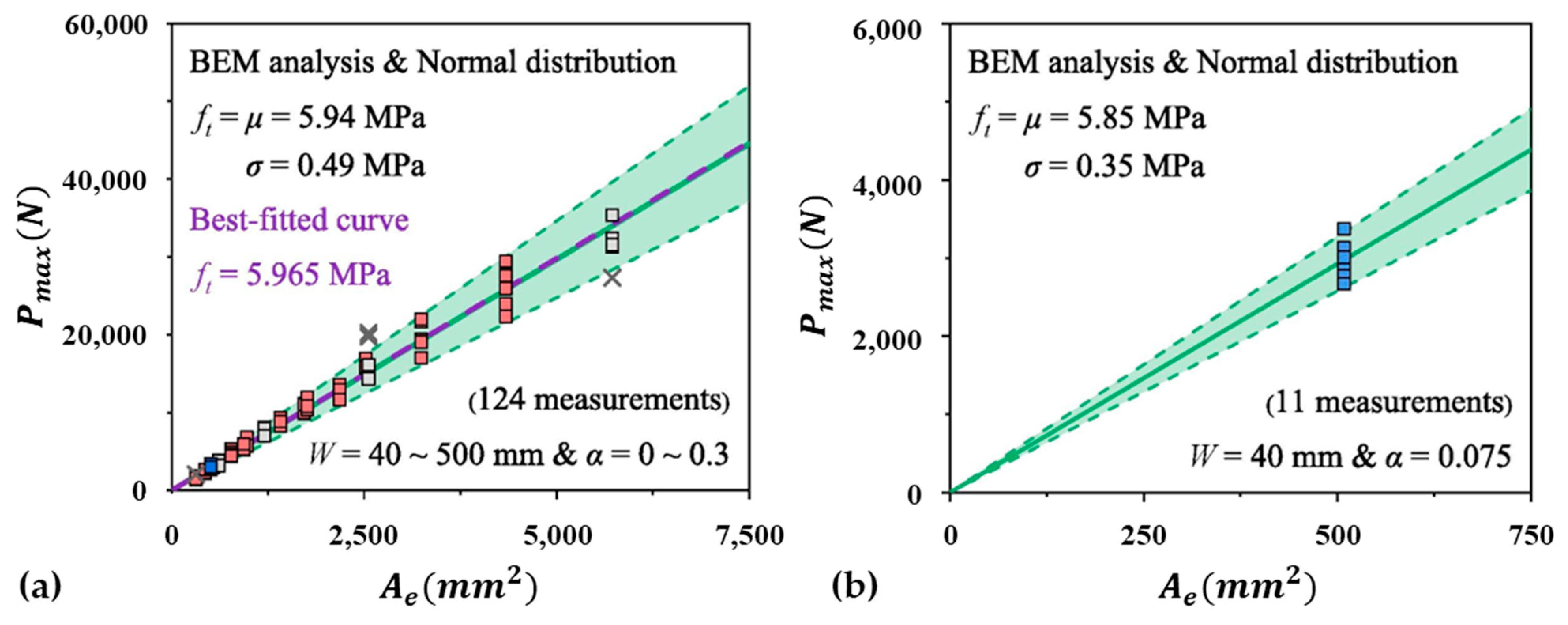
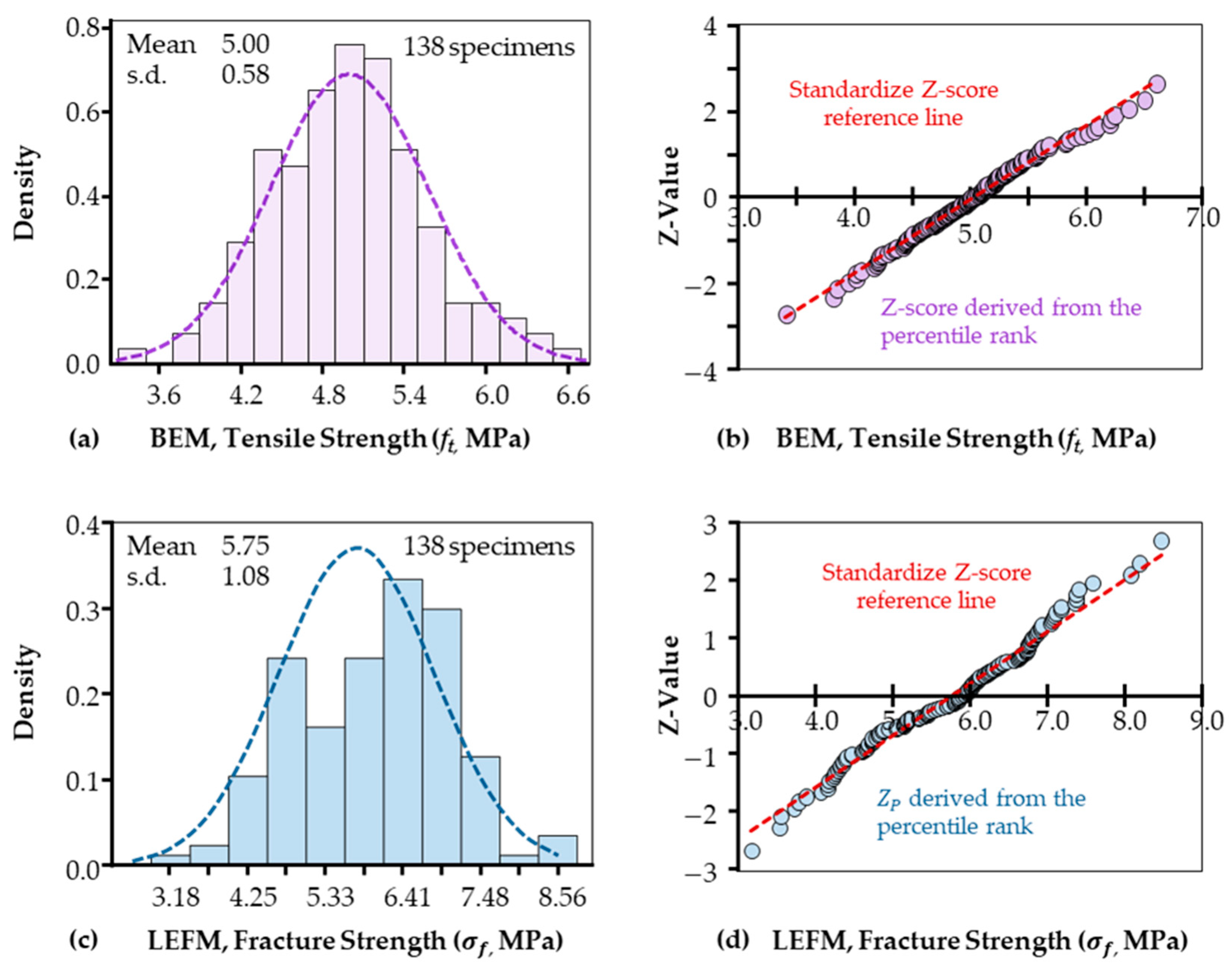
4.3. Reliability Analysis for BEM
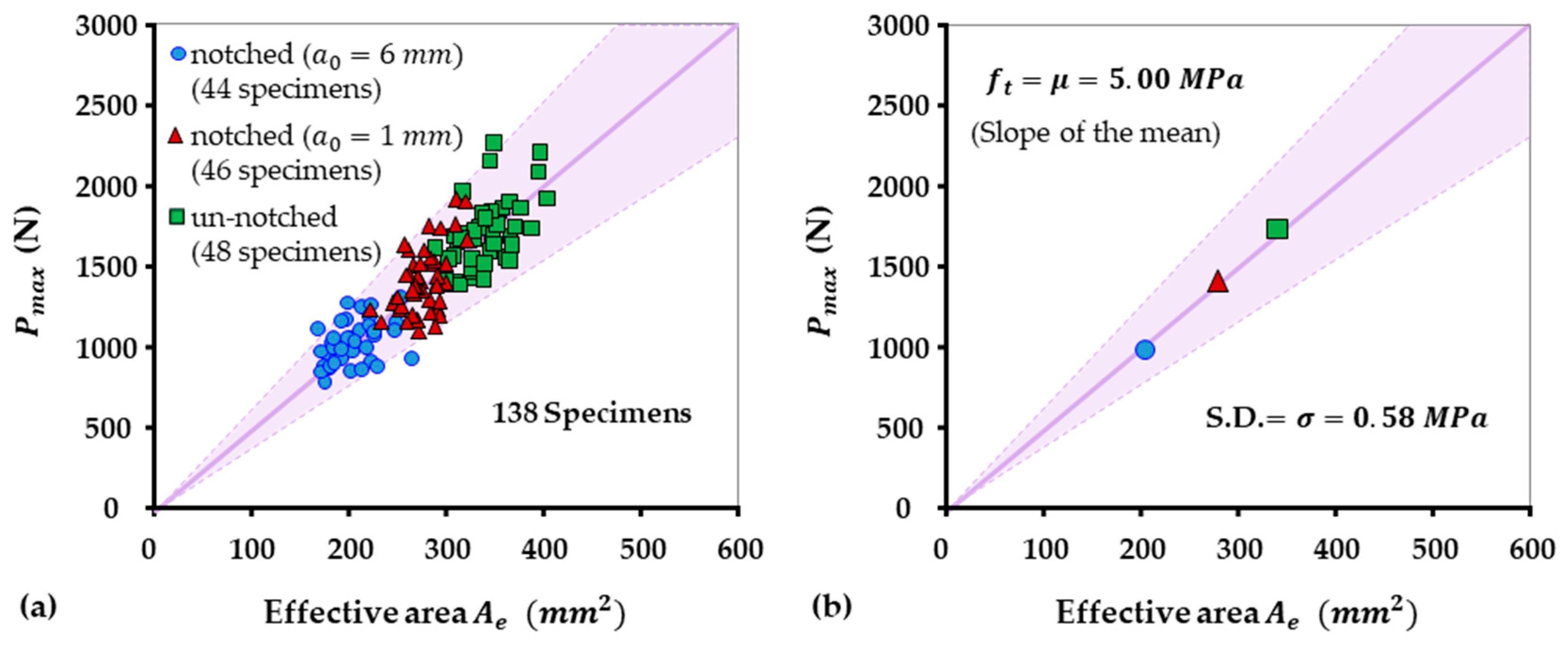
4.4. Analysis of Test Results Based on BEM for Pmax Prediction
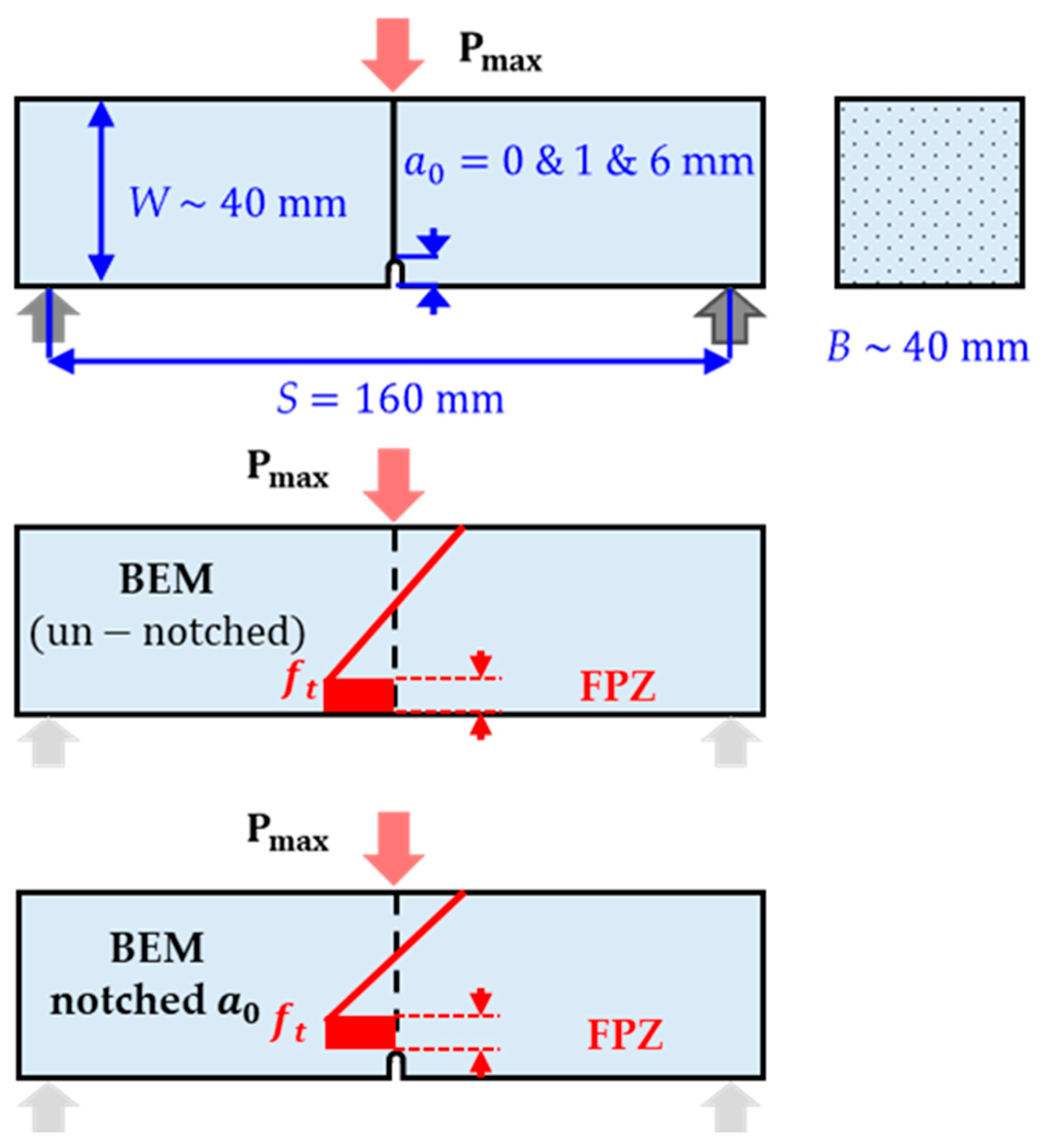
4.5. Notch Width Influence on KIC
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BEM | Boundary Effect Model |
| SEL | Size Effect Law |
| FPZ | Fracture Process Zone |
| LEFM | Linear Elastic Fracture Mechanics |
References
- Shah, S.P.; Swartz, S.E.; Ouyang, C. Fracture Mechanics of Concrete: Applications of Fracture Mechanics to Concrete, Rock and Other Quasi-Brittle Materials; John Wiley & Sons: Hoboken, NJ, USA, 1995. [Google Scholar]
- ASTM C33/03; Standard Specification for Concrete Aggregates. ASTM International: West Conshohocken, PA, USA, 2003.
- ASTM C1421-18; Standard Test Methods for Determination of Fracture Toughness of Advanced Ceramics at Ambient Temperature. ASTM International: West Conshohocken, PA, USA, 2010.
- Barenblatt, G. The formation of equilibrium cracks during brittle fracture. General ideas and hypotheses. Axially-symmetric cracks. J. Appl. Math. Mech. 1959, 23, 622–636. [Google Scholar] [CrossRef]
- Dugdale, D.S. Yielding of steel sheets containing slits. J. Mech. Phys. Solids 1960, 8, 100–104. [Google Scholar] [CrossRef]
- Hillerborg, A.; Modéer, M.; Petersson, P.-E. Analysis of crack formation and crack growth in concrete by means of fracture mechanics and finite elements. Cem. Concr. Res. 1976, 6, 773–781. [Google Scholar] [CrossRef]
- Xu, S.; Reinhardt, H. Determination of double-K criterion for crack propagation in quasi-brittle fracture, Part II: Analytical evaluating and practical measuring methods for three-point bending notched beams. Int. J. Fract. 1999, 98, 151–177. [Google Scholar] [CrossRef]
- Xu, F.; Wu, Z.; Zheng, J.; Zhao, Y.; Liu, K. Crack extension resistance curve of concrete considering variation of FPZ length. J. Mater. Civ. Eng. 2011, 23, 703–710. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Oh, B.H. Crack band theory for fracture of concrete. Mater. Struct. 1983, 16, 155–177. [Google Scholar] [CrossRef]
- Bažant, Z.P. Size effect in blunt fracture: Concrete, rock, metal. J. Eng. Mech. 1984, 110, 518–535. [Google Scholar] [CrossRef]
- Yu, Q.; Le, J.-L.; Hoover, C.G.; Bažant, Z.P. Problems with Hu-Duan boundary effect model and its comparison to size-shape effect law for quasi-brittle fracture. J. Eng. Mech. 2010, 136, 40–50. [Google Scholar] [CrossRef]
- Hoover, C.G.; Bažant, Z.P. Comparison of the Hu-Duan boundary effect model with the size-shape effect law for quasi-brittle fracture based on new comprehensive fracture tests. J. Eng. Mech. 2014, 140, 480–486. [Google Scholar] [CrossRef]
- Hu, X.; Guan, J.; Wang, Y.; Keating, A.; Yang, S. Comparison of boundary and size effect models based on new developments. Eng. Fract. Mech. 2017, 175, 146–167. [Google Scholar] [CrossRef]
- Hoover, C.G.; Bažant, Z.P.; Vorel, J.; Wendner, R.; Hubler, M.H. Comprehensive concrete fracture tests: Description and results. Eng. Fract. Mech. 2013, 114, 92–103. [Google Scholar] [CrossRef]
- Çağlar, Y.; Şener, S. Size effect tests of different notch depth specimens with support rotation measurements. Eng. Fract. Mech. 2016, 157, 43–55. [Google Scholar] [CrossRef]
- Chen, Y.; Hu, X. On interchangeability and selection of size effect and boundary effect experiments for characterization and prediction of quasi-brittle fracture of concrete. Theor. Appl. Fract. Mech. 2022, 122, 103629. [Google Scholar] [CrossRef]
- Han, X.; Chen, Y.; Hu, X.; Liu, W.; Li, Q.; Chen, S. Granite strength and toughness from small notched three-point-bend specimens of geometry dissimilarity. Eng. Fract. Mech. 2019, 216, 106–482. [Google Scholar] [CrossRef]
- Hu, X.; Li, Q.; Wu, Z.; Yang, S. Modelling fracture process zone width and length for quasi-brittle fracture of rock, concrete and ceramics. Eng. Fract. Mech. 2022, 259, 108158. [Google Scholar] [CrossRef]
- Hu, X.; Wittmann, F. Size effect on toughness induced by crack close to free surface. Eng. Fract. Mech. 2000, 65, 209–221. [Google Scholar] [CrossRef]
- Hall, E.O. The deformation and ageing of mild steel: III discussion of results. Proc. Phys. Soc. Sect. B 1951, 64, 747–753. [Google Scholar] [CrossRef]
- Petch, N.J. The cleavage strength of polycrystals. J. Iron Steel Inst. 1953, 174, 25–28. [Google Scholar]
- Hu, X.; Wu, Z. Prediction of crack initiation for notched concrete beam from FPZ at maximum load. Eng. Fract. Mech. 2024, 302, 110074. [Google Scholar] [CrossRef]
- Hu, X.; Mai, Y.-W. Re-interpretation of the Weibull strength distribution of polycrystalline ceramics—Characteristic strength and fracture toughness. J. Mech. Phys. Solids 2024, 196, 106021. [Google Scholar] [CrossRef]
- Hu, Y.; Chen, Y.; Yuan, B.; Hu, X. A simple closed-form model for fracture of CFRP panel with shallow surface scratch confirmed by comprehensive flexural/tensile tests. Mech. Mater. 2021, 155, 103725. [Google Scholar] [CrossRef]
- Hu, X. Additive effect of micro-damage zones from nano-scale crack tip and nano-grains for fracture toughness measurements of 3Y-TZP zirconia ceramics. J. Eur. Ceram. Soc. 2022, 42, 7174–7179. [Google Scholar] [CrossRef]
- Hu, Y.; Han, G.; Cheng, F.; Hu, X. Thickness effect on flexural strengths of laminar carbon fibre composites. Thin-Walled Struct. 2023, 186, 110690. [Google Scholar] [CrossRef]
- Hu, X. Size effect on tensile softening relation. Mater. Struct. 2010, 44, 129–138. [Google Scholar] [CrossRef]
- Hu, X.; Duan, K. Size effect and quasi-brittle fracture: The role of FPZ. Int. J. Fract. 2008, 154, 3–14. [Google Scholar] [CrossRef]
- Kardani, A.; Mehrafrooz, B.; Montazeri, A. MD-based computational design of new engineered Ni-based nanocatalysts: An in-depth study of the underlying mechanism. Eur. Phys. J. Plus 2018, 133, 88. [Google Scholar] [CrossRef]
- Kardani, A.; Montazeri, A.; Urbassek, H.M. Strain-rate-dependent plasticity of Ta-Cu nanocomposites for therapeutic implants. Sci. Rep. 2023, 13, 15788. [Google Scholar] [CrossRef]
- Shaikh, F.A.; Sohu, S. Implementation, advantages and management of ISO 9001 in the construction industry. Civ. Eng. J. 2020, 6, 1136–1142. [Google Scholar] [CrossRef]
- Obiora, C.O.; Okoye, M.N.; Belonwu, C.C.; Okeke, F.N. Evaluation of Strategic Measures for Effective Quality Control Management of Concrete Works in Anambra State, Nigeria. Br. J. Multidiscip. Adv. Stud. 2025, 6, 1–18. [Google Scholar] [CrossRef]
- Carpinteri, A. Scaling laws and renormalization groups for strength and toughness of disordered materials. Int. J. Solids Struct. 1994, 31, 291–302. [Google Scholar] [CrossRef]
- Du, X.; Jin, L. Size Effect in Concrete Materials and Structures; Springer Nature: Dordrecht, The Netherlands, 2021. [Google Scholar]
- Dubey, S.; Ray, S. Influence of heterogeneity on size effect models and fracture characteristics of plain concrete. Theor. Appl. Fract. Mech. 2023, 127, 103993. [Google Scholar] [CrossRef]
- Feng, D.-C.; Wu, J.-Y. Phase-field regularized cohesive zone model (CZM) and size effect of concrete. Eng. Fract. Mech. 2018, 197, 66–79. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, H.; Luo, S. Size effect model of nominal tensile strength with competing mechanisms between maximum defect and fracture process zone (CDF model) for quasi-brittle materials. Constr. Build. Mater. 2023, 399, 132538. [Google Scholar] [CrossRef]
- Raina, A. Size-dependent fracture characteristics of intermetallic alloys. Exp. Mech. 2022, 62, 863–877. [Google Scholar] [CrossRef]
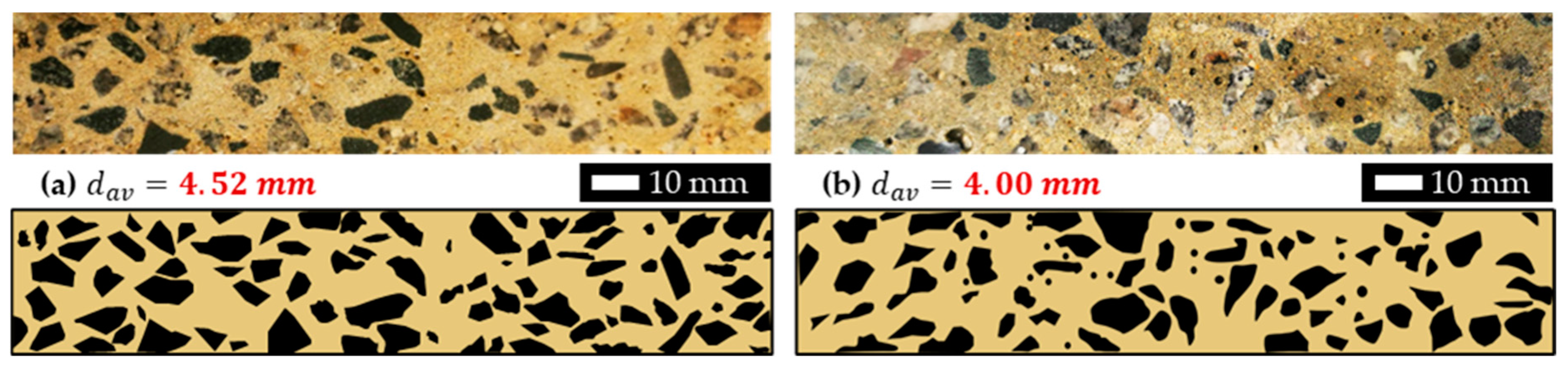

| (a) Sample 1 | |
| Aggregate Count | |
| % Area | |
| Original Sample Area | |
| Average Aggregate Size | |
| (b) Sample 2 | |
| Aggregate Count | |
| % Area | |
| Original Sample Area | |
| Average Aggregate Size | |
| Analysed by Image J |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, G.; Han, X.; Ji, Y.; Hu, X. Fracture Analysis of Concrete Structures: Prediction Based on Boundary Effect Model. Materials 2025, 18, 1877. https://doi.org/10.3390/ma18081877
Han G, Han X, Ji Y, Hu X. Fracture Analysis of Concrete Structures: Prediction Based on Boundary Effect Model. Materials. 2025; 18(8):1877. https://doi.org/10.3390/ma18081877
Chicago/Turabian StyleHan, Gang, Xiangyu Han, Yi Ji, and Xiaozhi Hu. 2025. "Fracture Analysis of Concrete Structures: Prediction Based on Boundary Effect Model" Materials 18, no. 8: 1877. https://doi.org/10.3390/ma18081877
APA StyleHan, G., Han, X., Ji, Y., & Hu, X. (2025). Fracture Analysis of Concrete Structures: Prediction Based on Boundary Effect Model. Materials, 18(8), 1877. https://doi.org/10.3390/ma18081877






