Study on the Approach to Obtaining Mechanical Properties Using Digital Image Correlation Technology
Abstract
1. Introduction
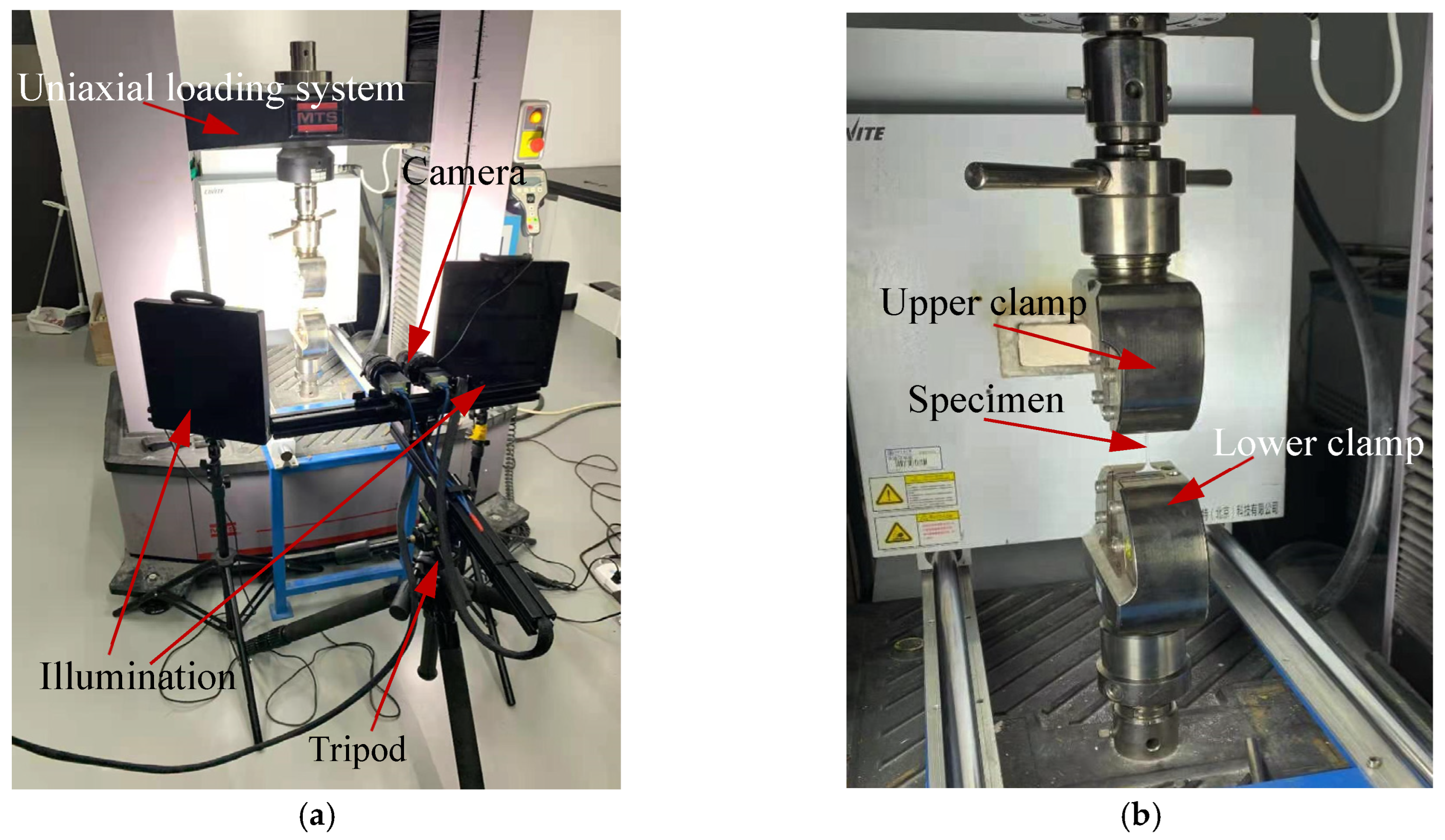
2. Experiment and Procedure
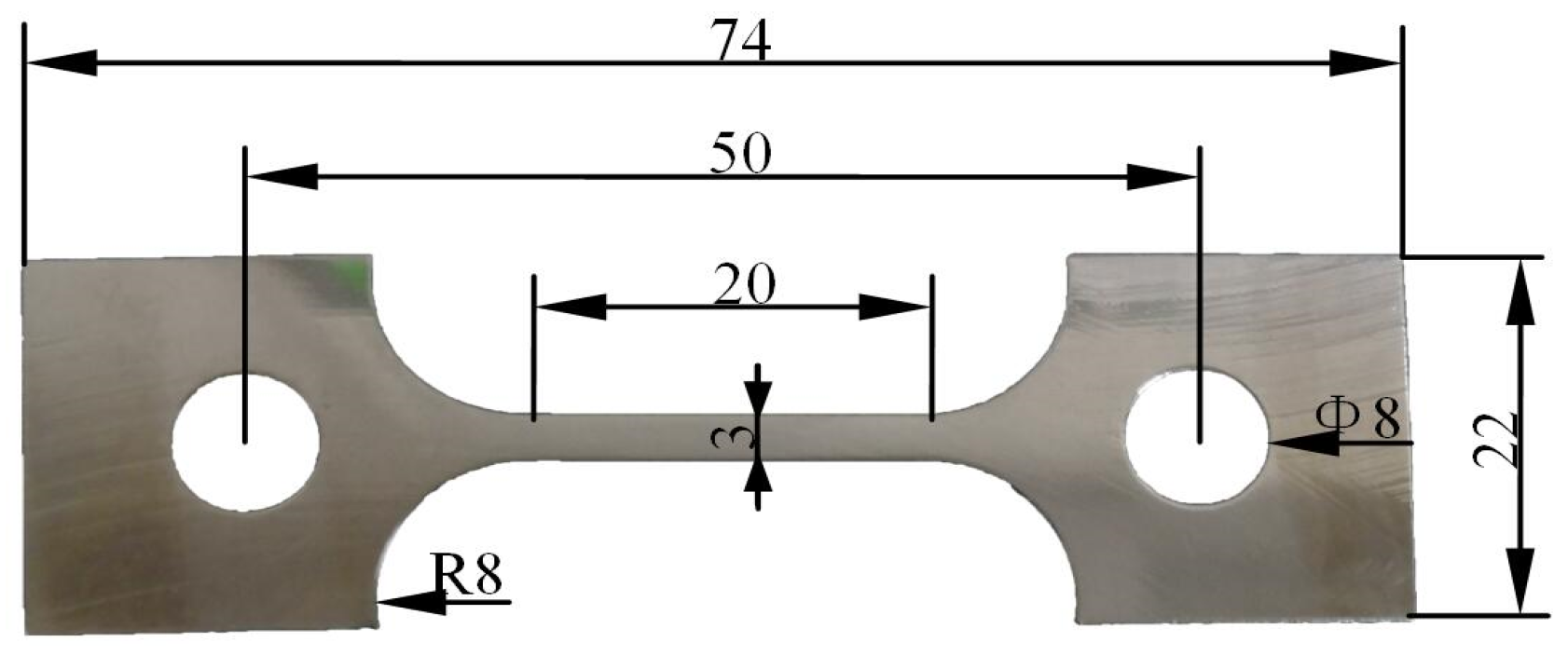
2.1. Specimen
2.2. Utilizing Strain Gauges to Acquire Strain Data During Material Stretching
- (1)
- Strain gauge patch
- (2)
- Connect strain gauge to deformeter
- (3)
- Experiment process
2.3. Utilizing DIC to Acquire Strain Data During Material Stretching
- (1)
- Speckle spraying
- (2)
- DIC device parameter adjustment
- (3)
- DIC software acquisition parameters and tensile speed settings.
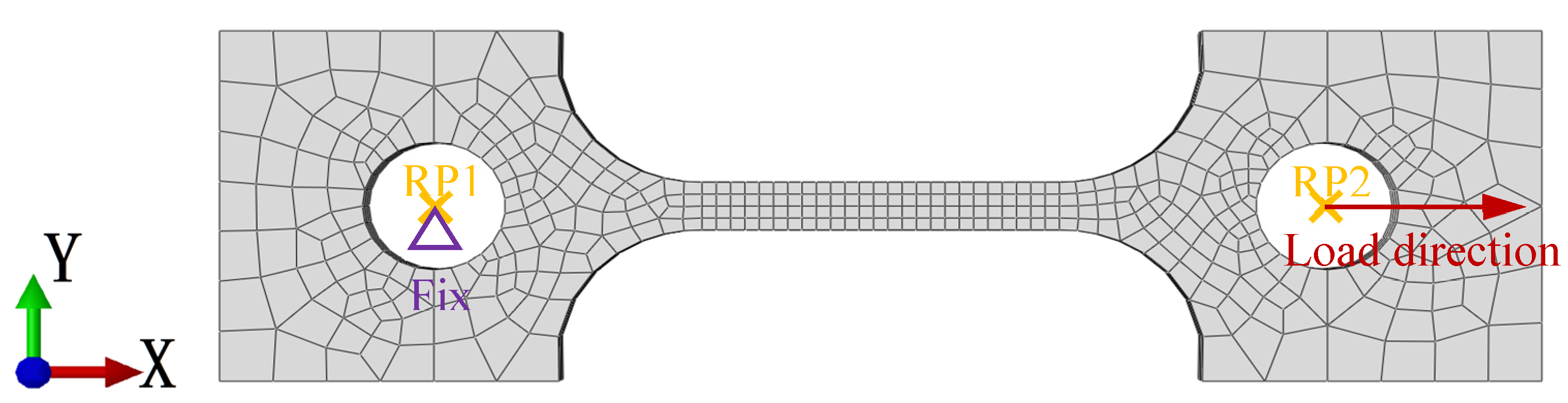
3. Numerical Modeling
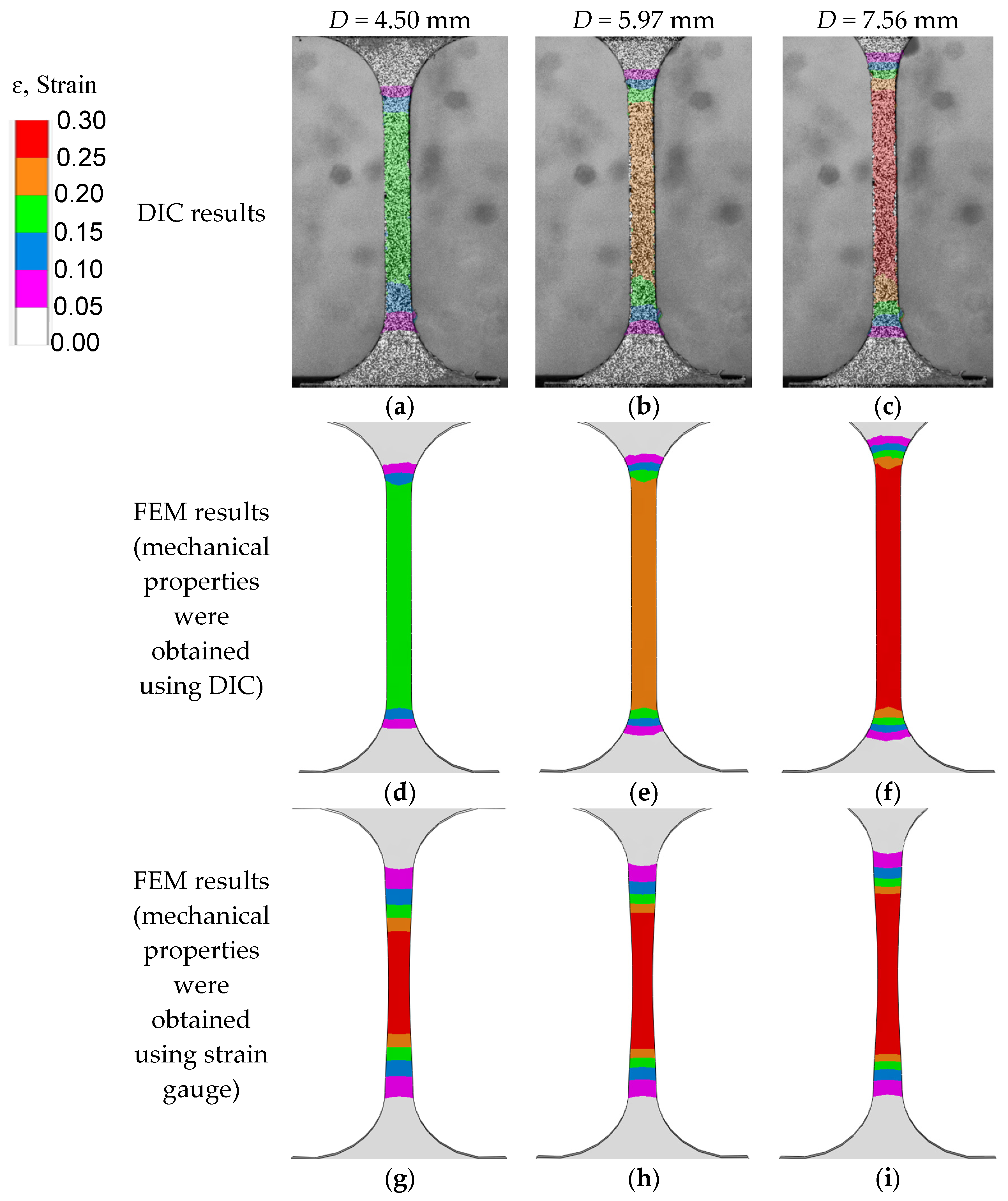
4. Results and Discussions
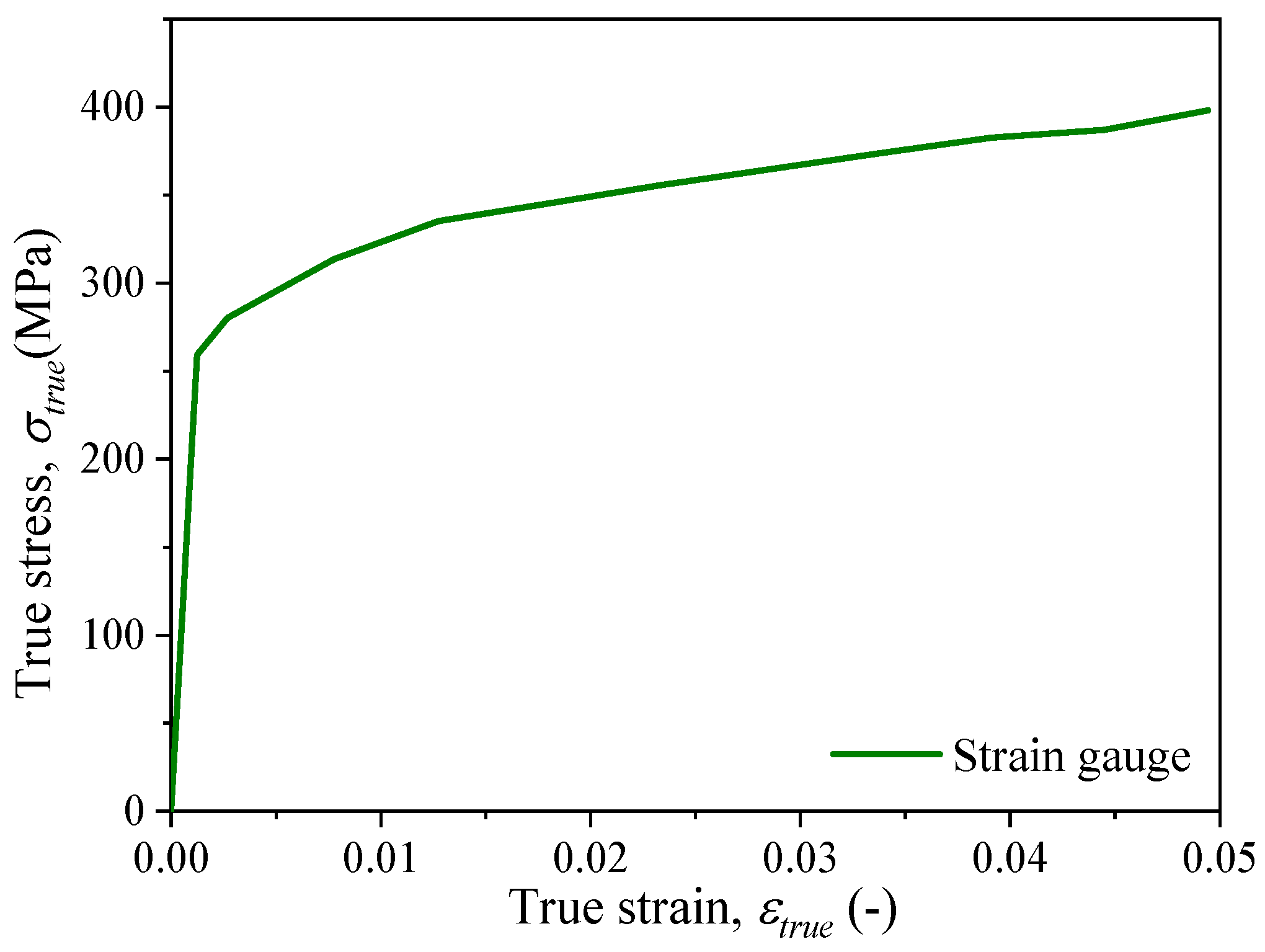
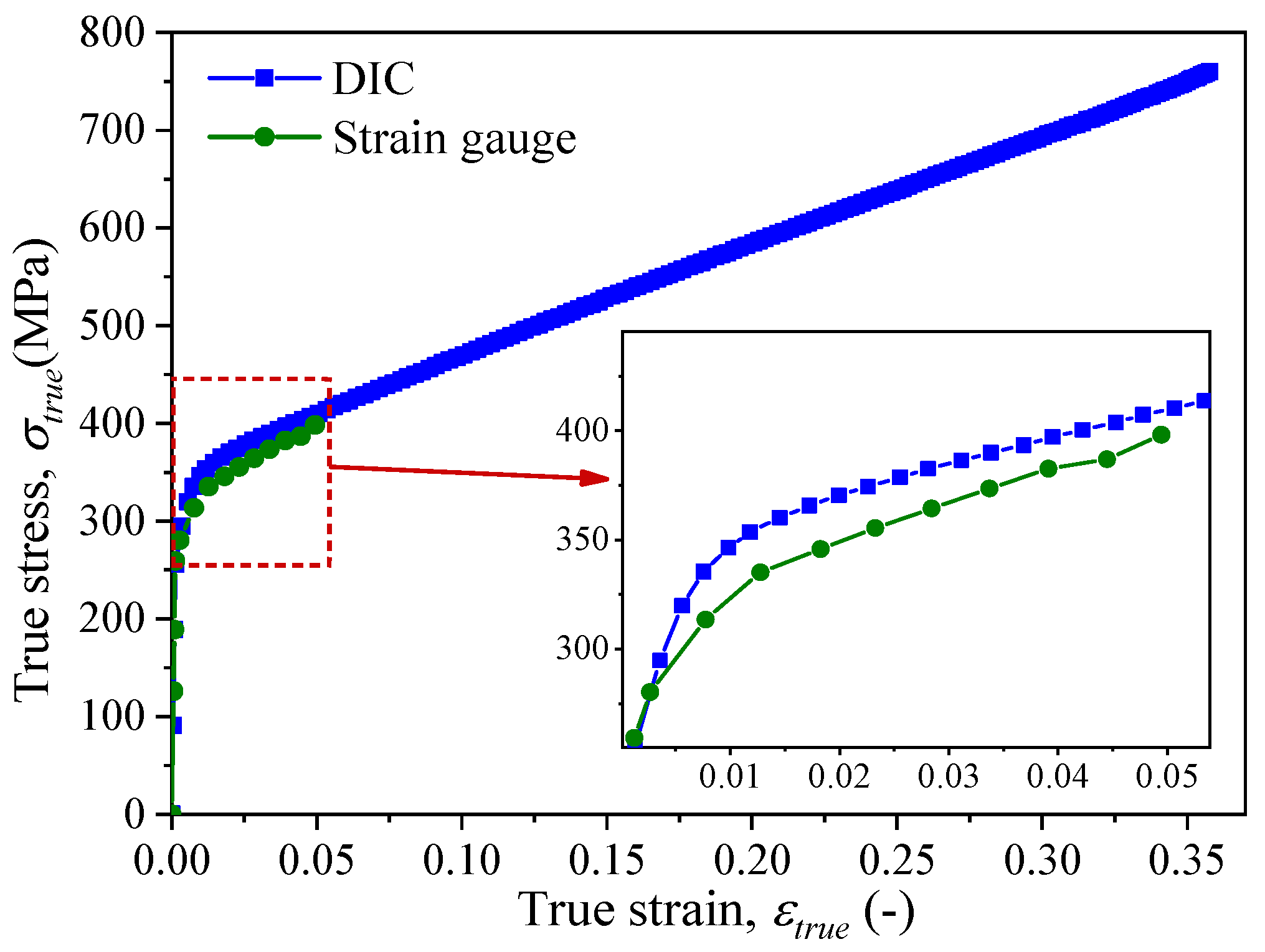
4.1. Strain Gauge Test Results
4.2. DIC Results Comparison
5. Conclusions
- (1)
- When the sample under study experiences significant deformation, the limited measurement range of the strain gauge can impose constraints on the quantity of strain data that can be collected. This, in turn, has a substantial impact on the acquisition of accurate mechanical property parameters. The restricted gauge range acts as a bottleneck, hindering the comprehensive characterization of the material’s mechanical behavior during large-scale deformation processes.
- (2)
- The utilization of the DIC technique for strain measurement offers a distinct advantage in enabling precise strain testing over a significantly broader range. This broader measurement scope allows for the collection of more comprehensive material mechanical parameters, which are crucial for conducting accurate elastoplastic finite element simulations. The DIC method provides a more holistic view of the material’s strain evolution, thereby enhancing the reliability and accuracy of the finite element models.
- (3)
- On the premise of accurately determining the mechanical property parameters of the material, the finite element simulation-derived strain distribution of 316L steel has been found to be in excellent agreement with the strain distribution obtained through DIC measurements. This congruence validates the effectiveness of the DIC method as an essential validation tool for finite element simulations. The DIC technique not only provides reliable experimental data but also serves as a benchmark for validating the numerical results of finite element models, thereby contributing to the overall improvement of the simulation accuracy and the understanding of the material’s mechanical behavior.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dong, Z.; Xie, X.; Jiang, W.; Niu, R.; Wan, Y.; Zhai, X.; Zhao, X. Fatigue failure mechanism of duplex stainless steel welded joints including role of heterogeneous cyclic hardening/softening: Experimental and modeling. Int. J. Fatigue 2024, 178, 108010. [Google Scholar] [CrossRef]
- Luo, P.; Li, D.; Zhang, C.; Ru, W.; Han, Z.; Ma, J. Experimental investigation on biaxial mechanical properties of laminated rock and floor heave mechanism of tunnel based on DIC. Eng. Fail. Anal. 2024, 156, 107848. [Google Scholar] [CrossRef]
- Wang, S.; Hou, C.; Wang, B.; Wu, G.; Fan, X.; Xue, H. Mechanical responses of L450 steel under biaxial loading in the presence of the stress discontinuity. Int. J. Press. Vessel. Pip. 2022, 198, 104662. [Google Scholar] [CrossRef]
- Zhang, X.W.; Wen, J.F.; Wang, T.; Zhang, X.-C.; Tu, S.-T. Ductile failure of flat plates containing two through-wall cracks: Experimental investigation and numerical modeling. Int. J. Press. Vessel. Pip. 2021, 191, 104377. [Google Scholar] [CrossRef]
- Obid, Š.; Starman, B.; Urevc, J.; Halilovič, M. Extraction of uniaxial stress–strain curve from bending test using DIC measurements. Eur. J. Mech. A/Solids 2024, 106, 105346. [Google Scholar] [CrossRef]
- Yang, R.; Li, Y.; Zeng, D.; Guo, P. Deep DIC: Deep learning-based digital image correlation for end-to-end displacement and strain measurement. J. Mater. Process. Technol. 2022, 302, 117474. [Google Scholar] [CrossRef]
- Zhang, S.; Liu, X.; Zhou, X.; Wang, X.; Qiu, L.; Gu, Z.; Xie, H. Spatial-temporal response and precursor characteristics of tensile failure on disc coal samples of different sizes combining AE, EMR and DIC techniques. Eng. Fract. Mech. 2024, 309, 110418. [Google Scholar] [CrossRef]
- Zhang, T.; Ma, X.; Yang, B.; Pei, X.; Ge, Z.; Jiang, W. An adaptive spherical indentation test integrating targeted testing scenarios, data acquisition, and model selection for uniaxial mechanical property predictions. Int. J. Press. Vessel. Pip. 2024, 212, 105365. [Google Scholar] [CrossRef]
- Venkatachalam, S.; Banjare, R.; Murthy, H.; Rao, B.C. Mechanical testing of micro-specimens of Al6061-T6 using DIC for strain measurement. Exp. Tech. 2019, 43, 125–135. [Google Scholar] [CrossRef]
- Quanjin, M.; Rejab, M.R.M.; Halim, Q.; Merzuki, M.; Darus, M. Experimental investigation of the tensile test using digital image correlation (DIC) method. Mater. Today Proc. 2020, 27, 757–763. [Google Scholar] [CrossRef]
- Nguyen, V.T.; Kwon, S.J.; Kwon, O.H.; Kim, Y.-S. Mechanical properties identification of sheet metals by 2D-digital image correlation method. Procedia Eng. 2017, 184, 381–389. [Google Scholar] [CrossRef]
- Valeri, G.; Koohbor, B.; Kidane, A.; Sutton, M.A. Determining the tensile response of materials at high temperature using DIC and the Virtual Fields Method. Opt. Lasers Eng. 2017, 91, 53–61. [Google Scholar] [CrossRef]
- Shen, X.; Li, M.; Dai, Y.; Zhou, X.; He, P. The effects of preparation temperature on the SiCf/SiC 3D4d woven composite. Ceram. Int. 2020, 46, 13088–13094. [Google Scholar] [CrossRef]
- Wang, C.; Wang, S.; Xie, L.; Liu, Y.; Deng, J.; He, W. Fatigue crack growth behavior of marine steel under variable amplitude loading-combining DIC technique and SEM observation. Int. J. Fatigue 2023, 170, 107508. [Google Scholar] [CrossRef]
- Chen, Y.; Gélébart, L.; Chateau, C.; Bornert, M.; King, A.; Aimedieu, P.; Sauder, C. 3D detection and quantitative characterization of cracks in a ceramic matrix composite tube using X-ray computed tomography. Exp. Mech. 2020, 60, 409–424. [Google Scholar] [CrossRef]
- Zhang, S.; Xue, H.; Wang, S.; Zhang, Y.B. A new method to determine cohesive parameters of elastic-plastic materials based on elastic component of J-integral. Eng. Fract. Mech. 2025, 315, 110793. [Google Scholar] [CrossRef]
- Zhu, J.G.; Xie, H.M.; Li, Y.J.; Hu, Z.X.; Luo, Q.; Gu, C.Z. Interfacial residual stress analysis of thermal spray coatings by miniature ring-core cutting combined with DIC method. Exp. Mech. 2014, 54, 127–136. [Google Scholar] [CrossRef]
- Zhang, H.; Li, X.; Gao, T.; Song, H.; Huang, G. Experimental study on deformation evolution and fracture behaviors of pure titanium at different stress triaxialities. Eng. Fract. Mech. 2021, 258, 108127. [Google Scholar] [CrossRef]
- Wang, D.Q.; Zhu, M.L.; Xuan, F.Z. Correlation of local strain with microstructures around fusion zone of a Cr-Ni-Mo-V steel welded joint. Mater. Sci. Eng. A 2017, 685, 205–212. [Google Scholar] [CrossRef]
- Gao, C.; Iwamoto, T.; Kusaka, T. A DIC-based Taylor impact test by measuring inertia force from acceleration distribution to obtain uniaxial stress–strain behavior of pure aluminum. Int. J. Impact Eng. 2024, 184, 104834. [Google Scholar] [CrossRef]
- Gehri, N.; Mata-Falcón, J.; Kaufmann, W. Automated crack detection and measurement based on digital image correlation. Constr. Build. Mater. 2020, 256, 119383. [Google Scholar] [CrossRef]
- Oka, J.M.; Vaidya, R.U.; Davis, J.T.; Webber, K.W.; Walzel, R.K. Nuclear material container drop testing using finite element analysis with verification using digital Image correlation. Nucl. Eng. Des. 2024, 421, 113057. [Google Scholar] [CrossRef]
- Novich, K.A.; Phero, T.L.; Cole, S.E.; Greseth, C.; Murtrey, M.; Estrada, D.; Jaques, B. Experimental Examination of Additively Manufactured Patterns on Structural Nuclear Materials for Digital Image Correlation Strain Measurements. Exp. Mech. 2024, 64, 1005–1020. [Google Scholar] [CrossRef]
- Wang, S.; Xue, H.; Wu, G.; Wang, Z.; Zhao, K.; Ni, C. Investigation of the Inhomogeneous Mechanical and Crack Driving Force of Low Alloy Steel SA508 and Its Welded 309L/308L Stainless Steel Cladding. Nucl. Sci. Eng. 2023, 197, 623–632. [Google Scholar] [CrossRef]
- GB/T 228.1-2010; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. China Quality and Standards Publishing & Media Company Limited: Beijing, China, 2011.
- GB/T 2039-1997; Metallic Materials—Creep and Stress-Rupture Test in Tension. China Quality and Standards Publishing & Media Company Limited: Beijing, China, 1997.
- Jordan, B.; Grolleau, V.; Mohr, D. Using surround DIC to extract true stress–strain curve from uniaxial tension experiments. Int. J. Solids Struct. 2023, 268, 112171. [Google Scholar] [CrossRef]

| Substrate Material | Wire Material | Length and Width (mm) | Resistance Ω | Sensitivity Coefficient | Nominal Voltage |
|---|---|---|---|---|---|
| acetal | constantan | 6.5 × 3.5 | 120.0 ± 0.3 | 2.06 ± 0.01 | ≤12 V |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Wang, B.; Mu, S.; Zhang, J.; Zhang, Y.; Gong, X. Study on the Approach to Obtaining Mechanical Properties Using Digital Image Correlation Technology. Materials 2025, 18, 1875. https://doi.org/10.3390/ma18081875
Wang S, Wang B, Mu S, Zhang J, Zhang Y, Gong X. Study on the Approach to Obtaining Mechanical Properties Using Digital Image Correlation Technology. Materials. 2025; 18(8):1875. https://doi.org/10.3390/ma18081875
Chicago/Turabian StyleWang, Shuai, Bin Wang, Shengyong Mu, Jianlong Zhang, Yubiao Zhang, and Xiaoyan Gong. 2025. "Study on the Approach to Obtaining Mechanical Properties Using Digital Image Correlation Technology" Materials 18, no. 8: 1875. https://doi.org/10.3390/ma18081875
APA StyleWang, S., Wang, B., Mu, S., Zhang, J., Zhang, Y., & Gong, X. (2025). Study on the Approach to Obtaining Mechanical Properties Using Digital Image Correlation Technology. Materials, 18(8), 1875. https://doi.org/10.3390/ma18081875








