Effect of Defects on Piezoelectric Properties of Sm-Doped K0.47Na0.53NbO3 Ceramics
Abstract
1. Introduction
2. Materials and Methods
2.1. The Preparation of KNSN Ceramics: Spark Plasma Sintering Method
2.2. Characterization and Methods
3. Results and Discussion
3.1. Phase Formation and Microscopic Morphology
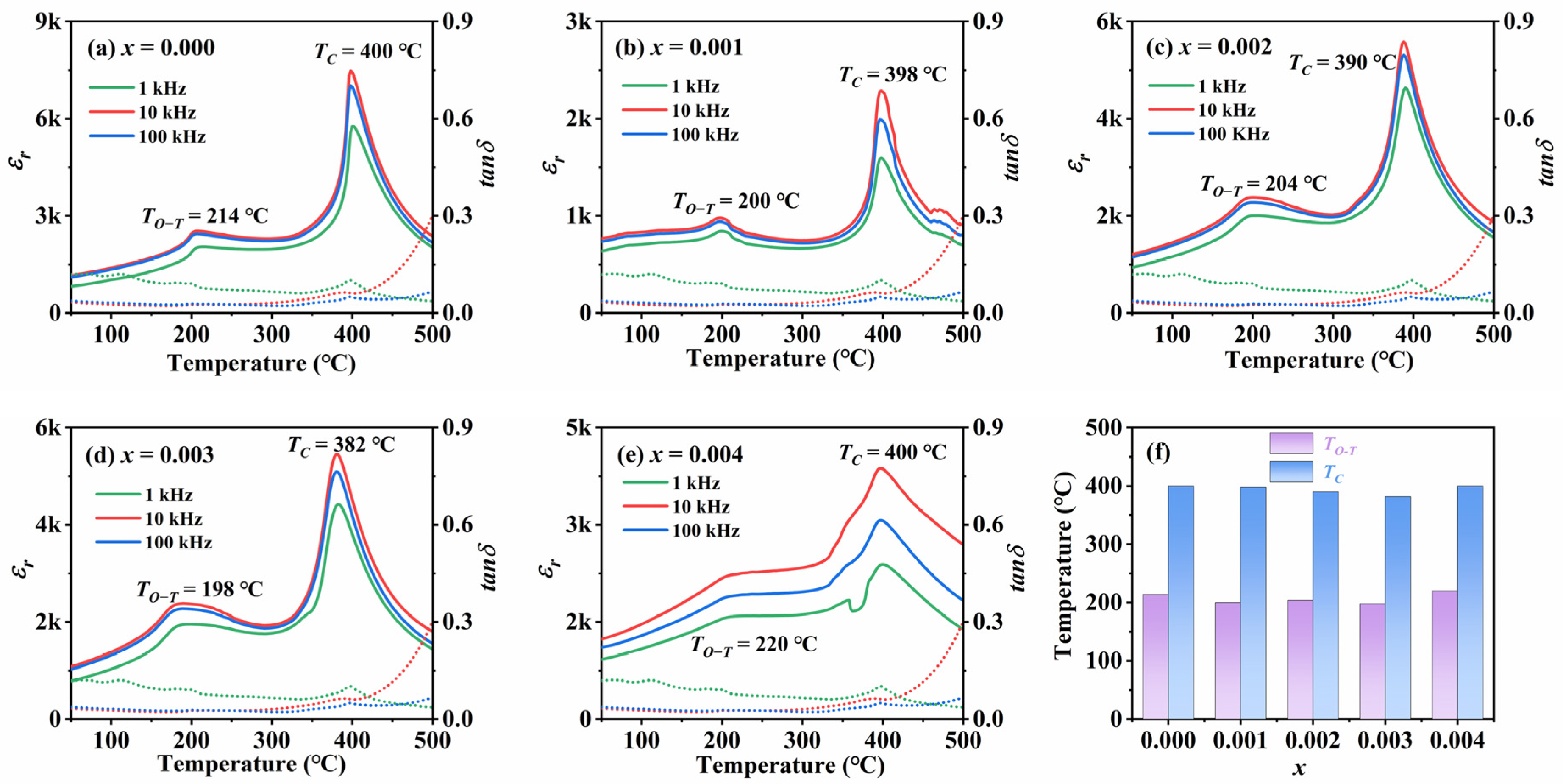
3.2. Dielectric and Relaxation Properties
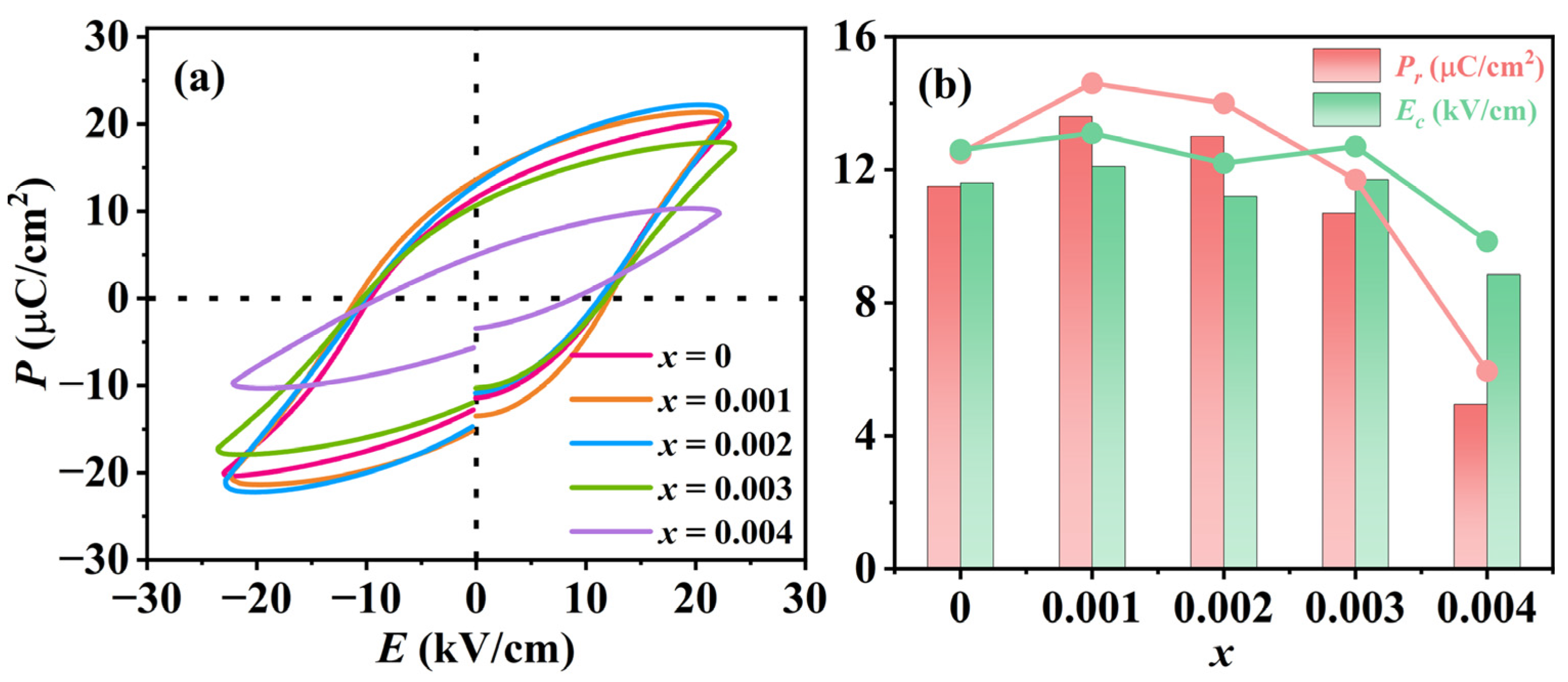
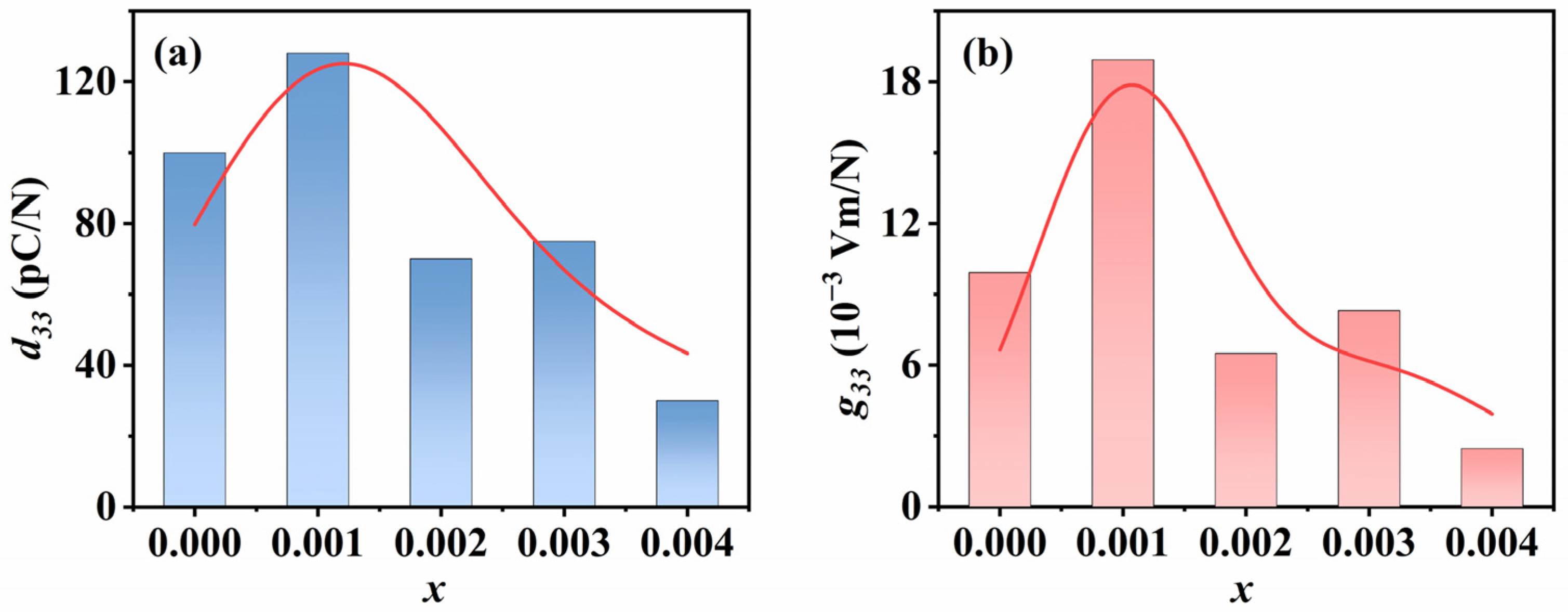
3.3. Ferroelectric and Piezoelectric Properties
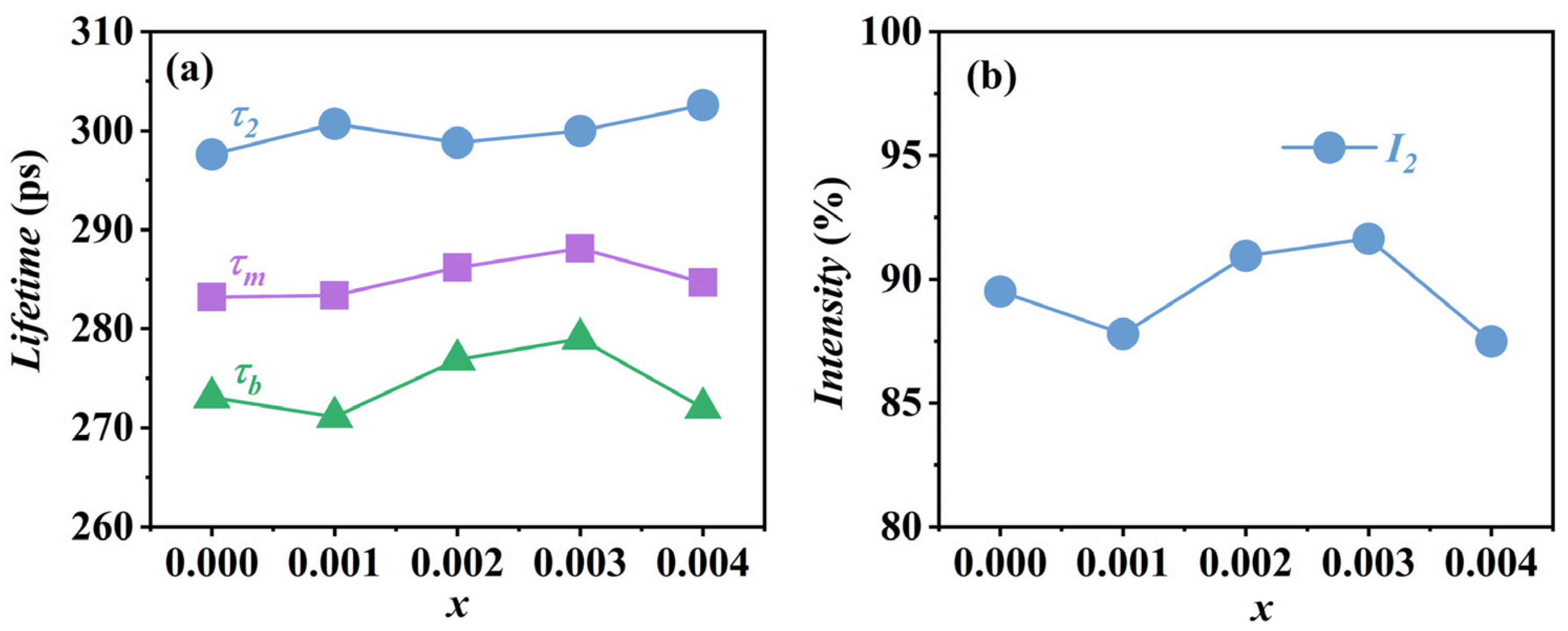
3.4. Positron Annihilation Lifetime Spectroscopy
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Liu, T.; Zheng, Z.; Li, Y.; Jia, P.; Wang, Y. Improved comprehensive properties induced by multi-phase coexistence in KNN ceramics. Mater. Chem. Phys. 2022, 290, 126640. [Google Scholar] [CrossRef]
- Wang, Z.; Ma, D.; Wang, Y.; Xie, Y.; Yu, Z.; Cheng, J.; Li, L.; Sun, L.; Dong, S.; Wang, H. Kirigami-origami-inspired lead-free piezoelectric ceramics. Adv. Sci. 2023, 10, 2207059. [Google Scholar] [CrossRef]
- Lee, G.-S.; Kim, J.-S.; Kim, S.-H.; Kwak, S.; Kim, B.; Kim, I.-S.; Nahm, S. Recent developments in (K,Na)NbO3-based lead-free piezoceramics. Micromachines 2024, 15, 325. [Google Scholar] [CrossRef]
- Pan, Q.; Pu, Y.; Wang, B.; Xie, H.; Zhang, L.; Zhang, J.; Hao, Y.; Yang, Y.; Qian, J. Substantial increase in resistance and suppression of resistance degradation in SrTiO3-based ceramics with colossal permittivity and low dielectric loss. Ceram. Int. 2025, 51, 187–195. [Google Scholar] [CrossRef]
- Ali, S.M.; Shankar, J.; Kumar, A.S.; Raju, P. Effect of sintering temperature on physical and dielectric properties of SrTiO3 ceramics. Mater. Today Proc. 2023, 92, 976–979. [Google Scholar] [CrossRef]
- Clabel, J.L.; Paula, K.T.; Pereira-da-Silva, M.A.; Vollet-Filho, J.D.; Marega, E.; Mendonça, C.R. Fabrication of micro patterns on BaTiO3:Er3+/Yb3+ perovskite films by femtosecond laser micromachining. Appl. Surf. Sci. 2023, 634, 157658. [Google Scholar] [CrossRef]
- Lv, X.; Zhu, J.; Xiao, D.; Zhang, X.; Wu, J. Emerging new phase boundary in potassium sodium-niobate based ceramics. Chem. Soc. Rev. 2020, 49, 671–707. [Google Scholar] [CrossRef]
- Trolier-McKinstry, S.; Zhang, S.; Bell, A.J.; Tan, X. High-performance piezoelectric crystals, ceramics, and films. Annu. Rev. Mater. Res. 2018, 48, 191–217. [Google Scholar] [CrossRef]
- Saito, Y.; Takao, H.; Tani, T.; Nonoyama, T.; Takatori, K.; Homma, T.; Nagaya, T.; Nakamura, M. Lead-free piezoceramics. Nature 2004, 432, 84–87. [Google Scholar] [CrossRef]
- Miwa, Y.; Hayashi, H.; Kawada, S.; Tanaka, N. The dielectric and piezoelectric properties of CaZrO3-modified (K,Na)NbO3 bulk and multilayer ceramics with different ZrO2 additions and MnO content. Jpn. J. Appl. Phys. 2023, 62, SM0801. [Google Scholar] [CrossRef]
- Li, H.; Hao, Y.; Lin, Z.; He, X.; Cai, J.; Gong, X.; Gu, Y.; Zhang, R.; Cheng, H.; Zhang, B. (K,Na)NbO3 lead-free piezoceramics prepared by microwave sintering and solvothermal powder synthesis. Solid State Commun. 2022, 353, 114871. [Google Scholar] [CrossRef]
- Wu, Y.; Cheng, Y.; Guan, S.; Wang, X.; Shi, W.; Xu, H.; Lang, R.; Xing, J.; Zhu, J.; Chen, Q. KNN-based lead-free piezoelectric ceramics with high Qm and enhanced d33 via a donor-acceptor codoping strategy. Inorg. Chem. 2023, 62, 15094–15103. [Google Scholar] [CrossRef]
- Vendrell, X.; García, J.; Cerdeiras, E.; Ochoa, D.; Rubio-Marcos, F.; Fernández, J.; Mestres, L. Effect of lanthanide doping on structural, microstructural and functional properties of K0.5Na0.5NbO3 lead-free piezoceramics. Ceram. Int. 2016, 42, 17530–17538. [Google Scholar] [CrossRef]
- Zhang, Z.; Shang, X.; Liu, X.; He, Y.; Zhang, Z.; Guo, J. Achieving excellent mechanical and electrical properties in transition metal oxides and rare earth oxide-doped KNN-based piezoceramics. J. Am. Ceram. Soc. 2024, 107, 5923–5938. [Google Scholar] [CrossRef]
- Hreščak, J.; Dražić, G.; Deluca, M.; Arčon, I.; Kodre, A.; Dapiaggi, M.; Rojac, T.; Malič, B.; Bencan, A. Donor doping of K0.5Na0.5NbO3 ceramics with strontium and its implications to grain size, phase composition and crystal structure. J. Eur. Ceram. Soc. 2017, 37, 2073–2082. [Google Scholar] [CrossRef]
- Morshed, T.; Haq, E.U.; Silien, C.; Tofail, S.A.M.; Zubair, M.A.; Islam, M.F. Piezo and pyroelectricity in spark plasma sintered potassium sodium niobate (KNN) ceramics. IEEE Trans. Dielectr. Electr. Insul. 2020, 27, 1428–1432. [Google Scholar] [CrossRef]
- Li, J.; Wang, K.; Zhang, B.; Zhang, L. Ferroelectric and piezoelectric properties of fine-grained Na0.5K0.5NbO3 lead-free piezoelectric ceramics prepared by spark plasma sintering. J. Am. Ceram. Soc. 2006, 89, 706–709. [Google Scholar] [CrossRef]
- Tuomisto, F.; Makkonen, I. Defect identification in semiconductors with positron annihilation: Experiment and theory. Rev. Mod. Phys. 2013, 85, 1583–1631. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, T.; Wang, L.; Liu, J.; Zhao, M.; Ye, B. Structural defects and non-ferroelectric piezoelectricity in an unpoled SrTiO3-Bi12TiO20 (ST-BT) composite ceramics. Scr. Mater. 2012, 67, 61–64. [Google Scholar] [CrossRef]
- Nagai, Y.; Tang, Z.; Hasegawa, M. Chemical analysis of precipitates in metallic alloys using coincidence Doppler broadening of positron annihilation radiation. Radiat. Phys. Chem. 2000, 58, 737–742. [Google Scholar] [CrossRef]
- Zhang, Q.; Xu, F.; Yang, R.; Lu, Y.; Li, P.; Shang, X.; Zhou, T.; He, Y. Suppressed tanδ and enhanced Qm in KCT and Ni2O3 co-modified [(K0.43Na0.57)0.94Li0.06] [(Nb0.94Sb0.06)0.95Ta0.05O3 lead-free piezoelectric ceramics. Ceram. Int. 2017, 43, 2537–2540. [Google Scholar] [CrossRef]
- Zhang, Y.; Shen, B.; Zhai, J.; Zeng, H. New insight on sintering progress of KNN-based lead-free ceramics. J. Am. Ceram. Soc. 2016, 99, 752–755. [Google Scholar] [CrossRef]
- Shi, W.; Feng, Y.; Lu, T.; Lu, Y.; Shen, J.; Xue, J.; Du, J.; Fu, P.; Hao, J.; Li, W. Photoluminescence and impedance properties of rare-earth doped (K0.5Na0.5)NbO3 lead-free ceramics. J. Mater. Sci. Mater. Electron. 2019, 30, 9–16. [Google Scholar] [CrossRef]
- Xiong, Y.; Wu, M.; Yang, W.; Chen, W.; Wang, L.; Zhou, Z. Structure, piezoelectric performance and liquid phase aid sintering of SiO2 doped (K0.5Na0.5)NbO3 lead-free ceramics. J. Mater. Sci. Mater. Electron. 2017, 28, 17363–17369. [Google Scholar] [CrossRef]
- Yu, F.; Chi, Y.; Wang, P.; Ma, B.; Wu, X.; Lin, C.; Zhao, C.; Gao, M.; Lin, T.; Zhang, Q. Highly responsive photochromic behavior with large coloration contrast in Ba/Sm co-doped (K0.5Na0.5)NbO3 transparent ceramics. Ceram. Int. 2022, 48, 18899–18908. [Google Scholar] [CrossRef]
- Xing, J.; Xie, S.; Wu, B.; Tan, Z.; Jiang, L.; Xie, L.; Cheng, Y.; Wu, J.; Xiao, D.; Zhu, J. Influence of different lanthanide ions on the structure and properties of potassium sodium niobate based ceramics. Scr. Mater. 2020, 177, 186–191. [Google Scholar] [CrossRef]
- Liu, H.; Veber, P.; Rödel, J.; Rytz, D.; Fabritchnyi, P.B.; Afanasov, M.I.; Patterson, E.A.; Frömling, T.; Maglione, M.; Koruza, J. High-performance piezoelectric (K,Na,Li)(Nb,Ta,Sb)O3 single crystals by oxygen annealing. Acta Mater. 2018, 148, 499–507. [Google Scholar] [CrossRef]
- Yang, D.; Ma, C.; Yang, Z.; Wei, L.; Chao, X.; Yang, Z.; Yang, J. Optical and electrical properties of pressureless sintered transparent (K0.37Na0.63)NbO3-based ceramics. Ceram. Int. 2016, 42, 4648–4657. [Google Scholar] [CrossRef]
- Zang, J.; Li, M.; Sinclair, D.C.; Jo, W.; Rödel, J. Impedance spectroscopy of (Bi1/2Na1/2)TiO3-BaTiO3 ceramics modified with (K0.5Na0.5)NbO3. J. Am. Ceram. Soc. 2014, 97, 1523–1529. [Google Scholar] [CrossRef]
- Jiang, M.; Li, X.; Liu, J.; Zhu, J.; Zhu, X.; Li, L.; Chen, Q.; Zhu, J.; Xiao, D. Structural and electrical properties of Cu-doped (K0.5Na0.5)NbO3-MgTiO3 lead-free ceramics. J. Alloys Compd. 2009, 479, L18–L21. [Google Scholar] [CrossRef]
- Liu, W.; Wang, H.; Hu, W.; Du, Y.; Cheng, C. Understanding the origin of the high piezoelectric performance of KNN-based ceramics from the perspective of lattice distortion. Ceram. Int. 2022, 48, 9731–9738. [Google Scholar] [CrossRef]
- Rubio-Marcos, F.; Fernandez, J.F.; Ochoa, D.A.; García, J.E.; Rojas-Hernandez, R.E.; Castro, M.; Ramajo, L. Understanding the piezoelectric properties in potassium-sodium niobate-based lead-free piezoceramics: Interrelationship between intrinsic and extrinsic factors. J. Eur. Ceram. Soc. 2017, 37, 3501–3509. [Google Scholar] [CrossRef]
- Klym, H.; Karbovnyk, I.; Luchechko, A.; Kostiv, Y.; Popov, A.I. Extended positron-trapping defects in the Eu3+-doped BaGa2O4 ceramics studied by positron annihilation lifetime method. Phys. Status Solidi B 2022, 259, 2100485. [Google Scholar] [CrossRef]
- Espinosa, A.P.; Ramajo, L.; Rubio-Marcos, F.; Macchi, C.; Somoza, A.; Castro, M. Influence of the BaTiO3 addition to K0.5Na0.5NbO3 lead-free ceramics on the vacancy-like defect structure and dielectric properties. J. Eur. Ceram. Soc. 2021, 41, 1288–1298. [Google Scholar] [CrossRef]
- Wang, T.; Zhu, T.; Wang, D.; Zhang, P.; Song, Y.; Ye, F.; Wang, Q.; Jin, S.; Yu, R.; Liu, F.; et al. Effect of vacancy behavior on precipitate formation in a reduced-activation V-Cr-Mn medium-entropy alloy. Materials 2022, 16, 153. [Google Scholar] [CrossRef]
- Yang, J.; Feng, S.-R.; Zhang, T.; Niu, X.-P.; Wang, R.; Li, M.; Yu, R.-S.; Cao, X.-Z.; Wang, B.-Y. Analysis of defects in B-vacancy compensated Sm-doped PZT(54/46) ceramics and their influences on piezoelectric properties. Acta Phys. Sin. 2024, 73, 077701. [Google Scholar] [CrossRef]
- Sheng, Y.; Huang, Y.; Chen, C.; Zhang, M.; Deng, N.; Ma, L. Effect of oriented defect-dipoles on the ferroelectric and piezoelectric properties of CuO-doped (K0.48Na0.52)0.96Li0.04Nb0.805Ta0.075Sb0.12O3 ceramics. Ceram. Int. 2018, 44, 10141–10146. [Google Scholar] [CrossRef]
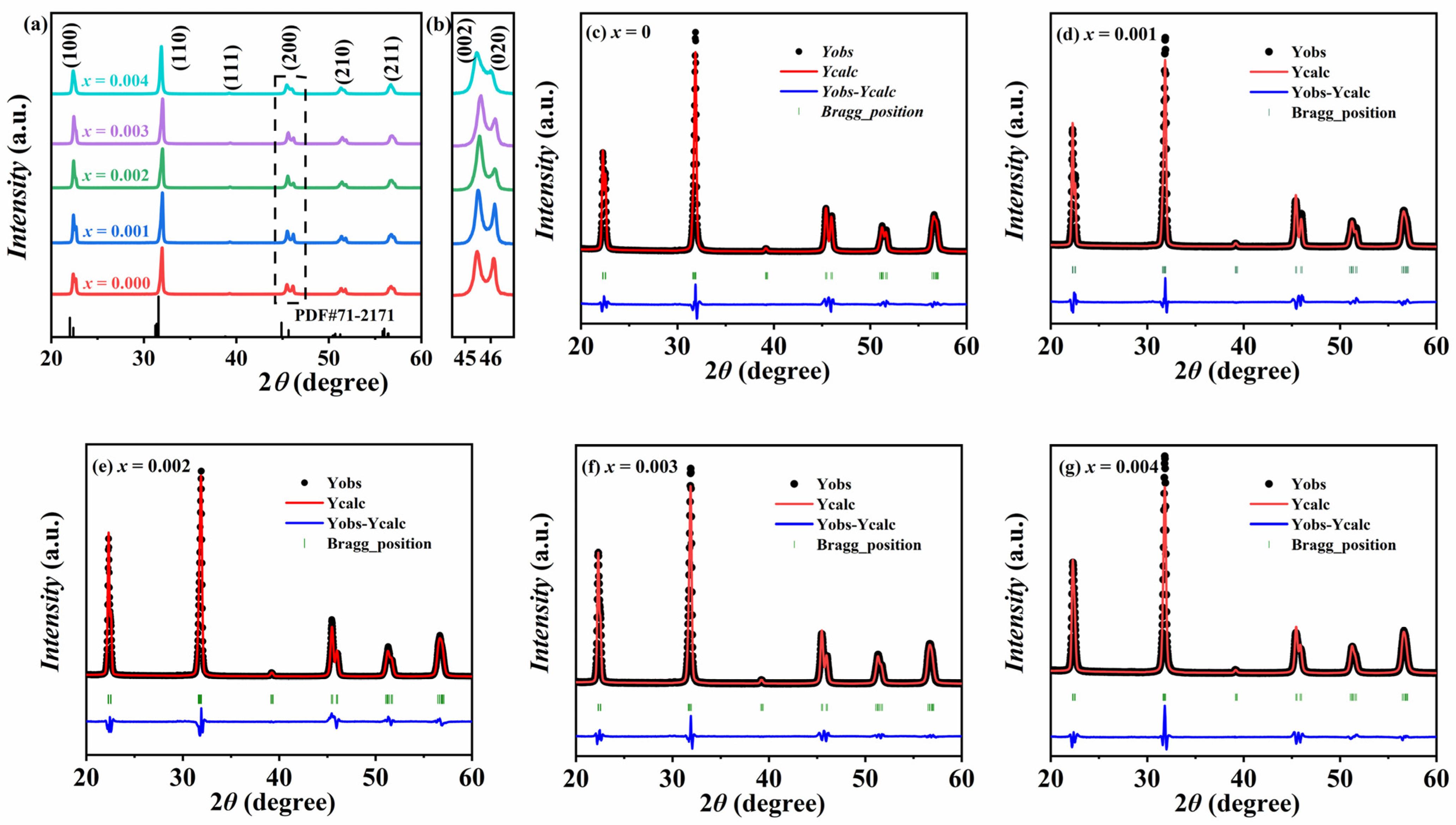
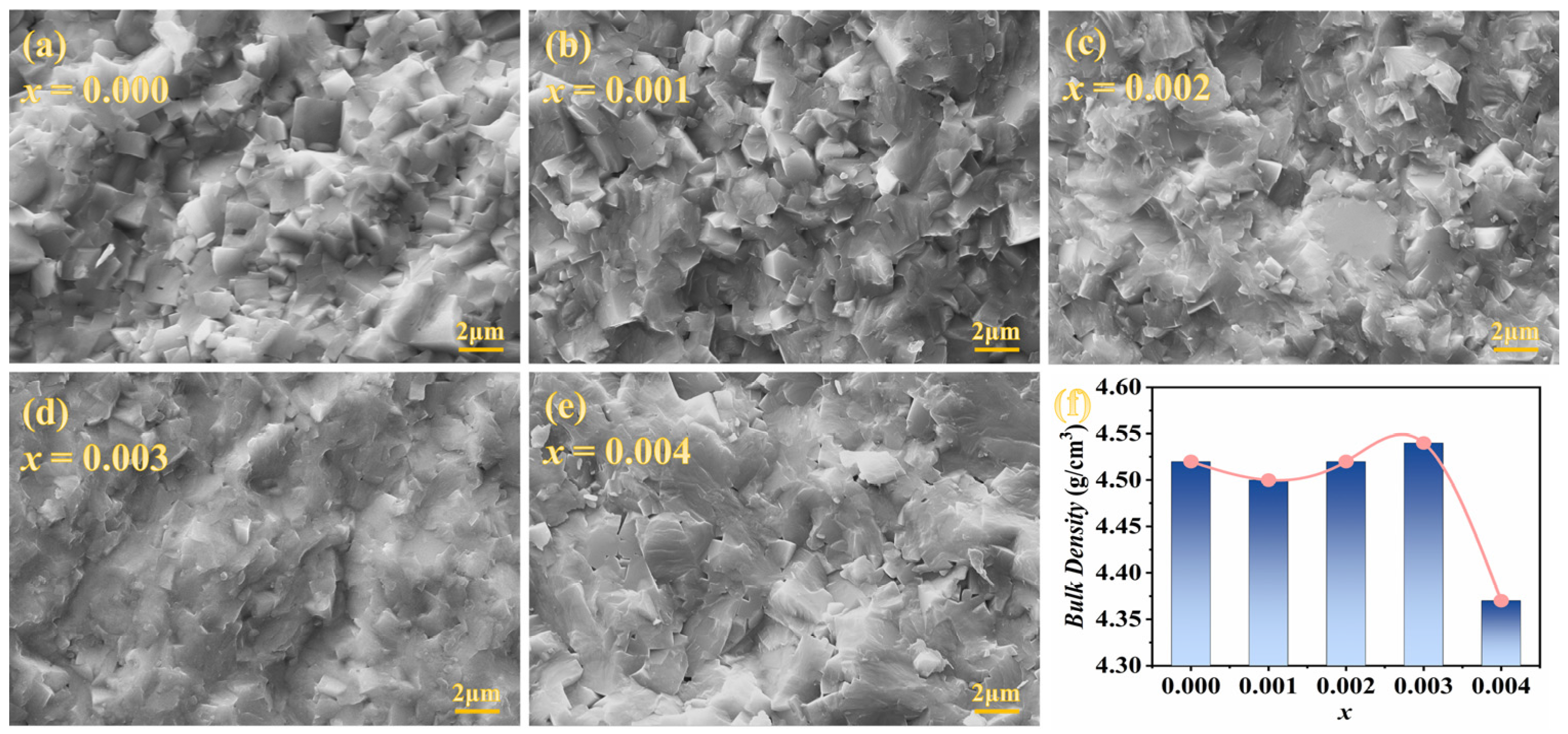
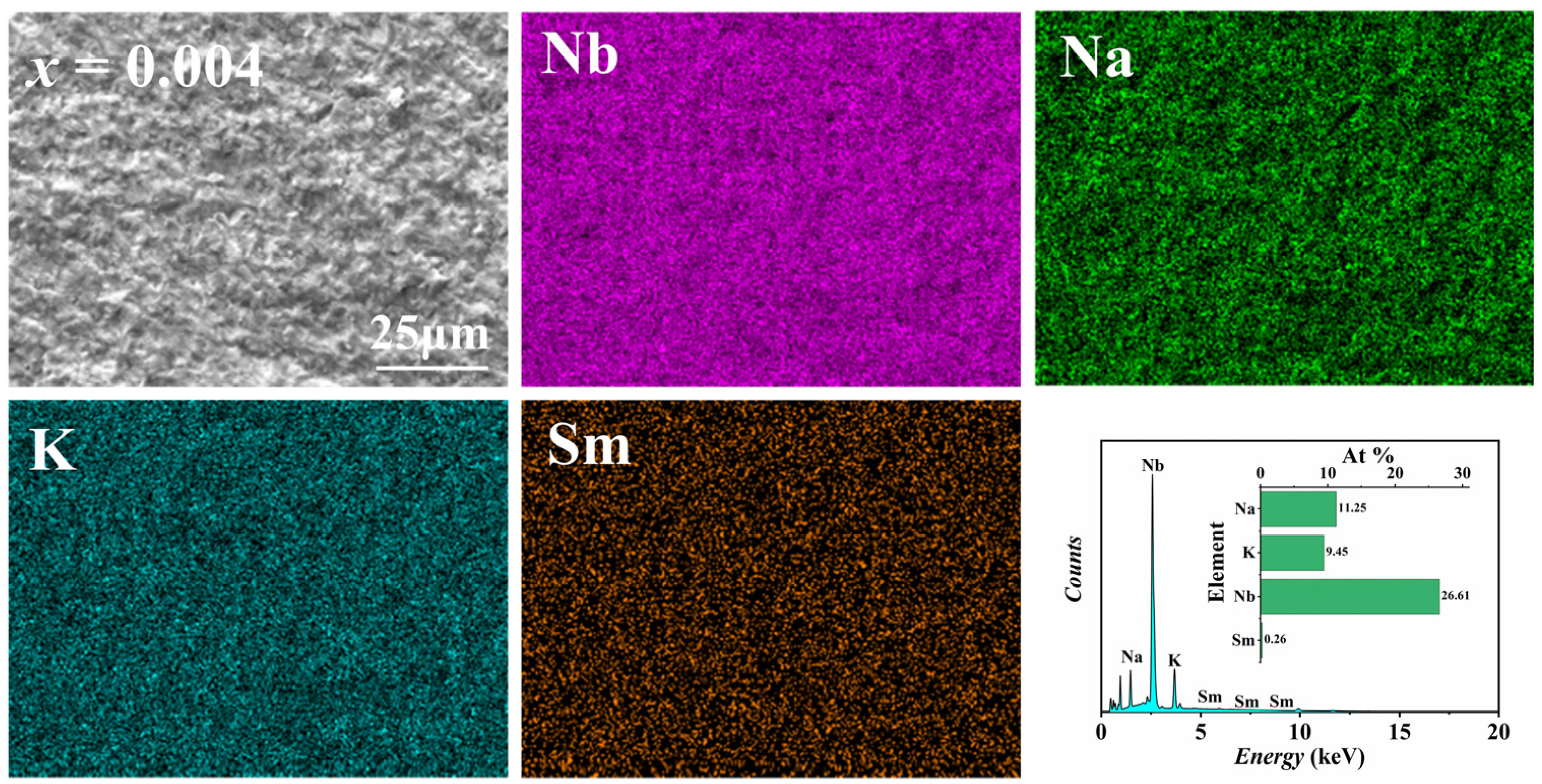

| x | 0 Sm | 0.001 Sm | 0.002 Sm | 0.003 Sm | 0.004 Sm |
|---|---|---|---|---|---|
| O-Phase (%) | 100 | 100 | 100 | 100 | 100 |
| a (Å) | 3.9477 | 3.9478 | 3.9469 | 3.9473 | 3.9543 |
| b (Å) | 5.6354 | 5.6332 | 5.6289 | 5.6258 | 5.6336 |
| c (Å) | 5.6616 | 5.6624 | 5.6543 | 5.6526 | 5.6538 |
| α = γ = β | 90° | 90° | 90° | 90° | 90° |
| Volume (Å3) | 125.956 | 125.926 | 125.692 | 125.526 | 125.949 |
| χ2 | 4.33 | 5.96 | 3.85 | 4.17 | 6.25 |
| Rwp | 8.52 | 9.13 | 9.66 | 10.1 | 8.78 |
| Element (at%) | K | Na | O | Nb | Sm | K/Na Atomic Ratio |
|---|---|---|---|---|---|---|
| 0 Sm | 8.40 | 10.84 | 61.87 | 18.92 | 0 | 0.77 |
| 0.001 Sm | 9.87 | 10.72 | 52.46 | 26.84 | 0.13 | 0.92 |
| 0.002 Sm | 7.61 | 8.42 | 51.19 | 21.67 | 0.14 | 0.90 |
| 0.003 Sm | 10.07 | 10.69 | 54.86 | 24.20 | 0.17 | 0.94 |
| 0.004 Sm | 9.45 | 11.25 | 52.43 | 26.61 | 0.26 | 0.84 |
| Samples | εr | tanδ | TO−T | TC |
|---|---|---|---|---|
| 0 Sm | 762 | 0.037 | 214 °C | 400 °C |
| 0.001 Sm | 604 | 0.032 | 200 °C | 398 °C |
| 0.002 Sm | 887 | 0.038 | 204 °C | 390 °C |
| 0.003 Sm | 717 | 0.041 | 198 °C | 382 °C |
| 0.004 Sm | 892 | 0.092 | 220 °C | 400 °C |
| Samples | τ1 (ps) | I1 (%) | τ2 (ps) | I2 (%) | τm (ps) | τb (ps) |
|---|---|---|---|---|---|---|
| 0 Sm | 160.3 | 10.49 | 297.6 | 89.51 | 283.2 | 273.1 |
| 0.001 Sm | 158.7 | 12.20 | 300.7 | 87.80 | 283.4 | 271.1 |
| 0.002 Sm | 159.4 | 9.05 | 298.8 | 90.95 | 286.2 | 276.9 |
| 0.003 Sm | 157.9 | 8.36 | 300.0 | 91.64 | 288.1 | 279.0 |
| 0.004 Sm | 159.3 | 12.51 | 302.6 | 87.49 | 284.7 | 272.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, P.; Wang, D.; Zhou, F.; Ren, Y.; Zhang, J.; Zang, G.; Wang, X.; Cao, X. Effect of Defects on Piezoelectric Properties of Sm-Doped K0.47Na0.53NbO3 Ceramics. Materials 2025, 18, 1760. https://doi.org/10.3390/ma18081760
Wu P, Wang D, Zhou F, Ren Y, Zhang J, Zang G, Wang X, Cao X. Effect of Defects on Piezoelectric Properties of Sm-Doped K0.47Na0.53NbO3 Ceramics. Materials. 2025; 18(8):1760. https://doi.org/10.3390/ma18081760
Chicago/Turabian StyleWu, Pengkun, Dandan Wang, Fengzi Zhou, Yongpeng Ren, Junhu Zhang, Guozhong Zang, Xiaofei Wang, and Xingzhong Cao. 2025. "Effect of Defects on Piezoelectric Properties of Sm-Doped K0.47Na0.53NbO3 Ceramics" Materials 18, no. 8: 1760. https://doi.org/10.3390/ma18081760
APA StyleWu, P., Wang, D., Zhou, F., Ren, Y., Zhang, J., Zang, G., Wang, X., & Cao, X. (2025). Effect of Defects on Piezoelectric Properties of Sm-Doped K0.47Na0.53NbO3 Ceramics. Materials, 18(8), 1760. https://doi.org/10.3390/ma18081760







