Fatigue Cracking Characteristics of Ultra-Large Particle Size Asphalt Mixture Under Temperature and Loading Using Digital Image Correlation Techniques
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.1.1. Raw Materials
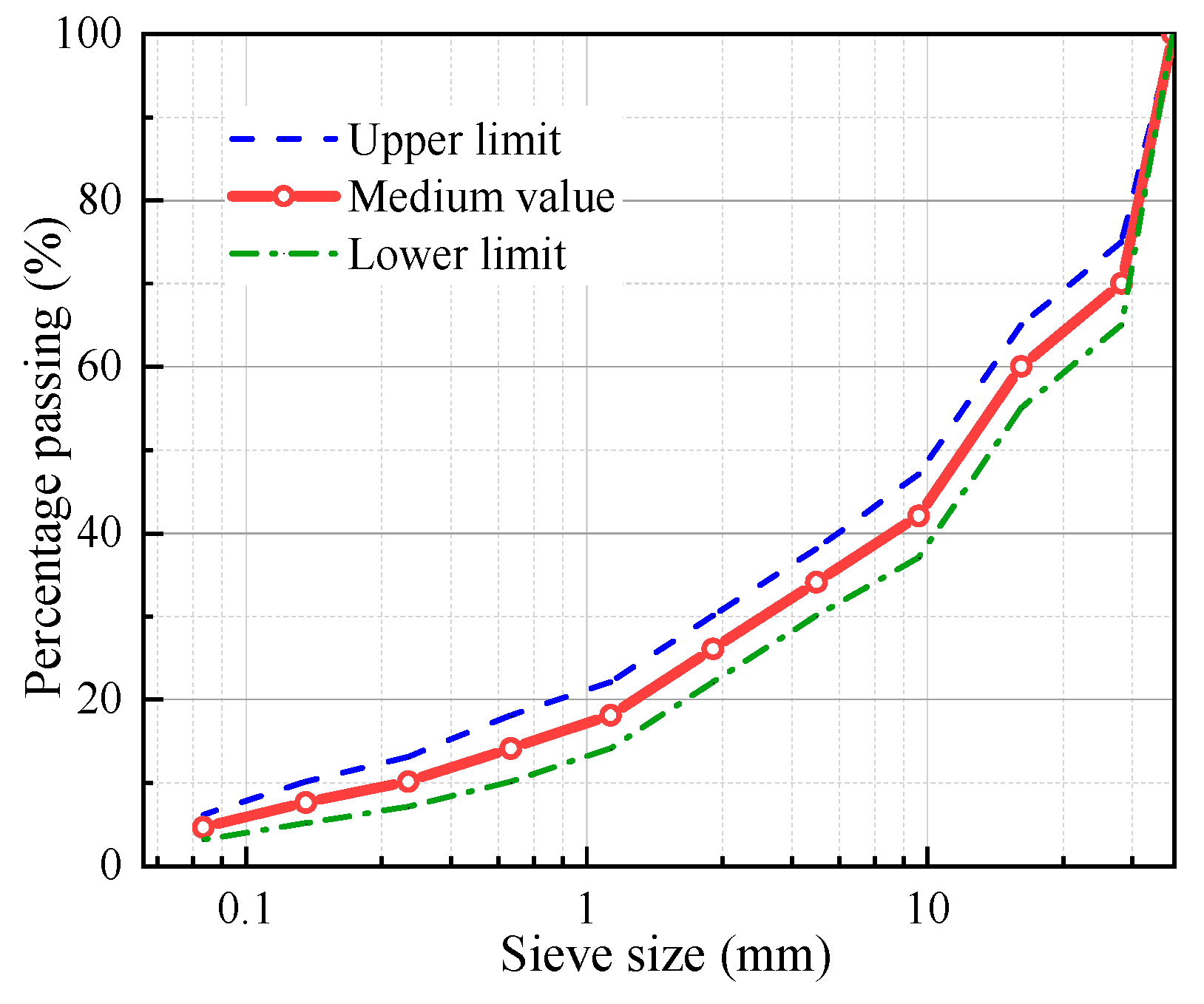
2.1.2. Gradation
2.1.3. Asphalt Mixture
2.2. Specimen Preparation
2.3. Test Methods
2.3.1. Splitting Fatigue Test
2.3.2. Digital Image Correlation
3. Results and Discussion
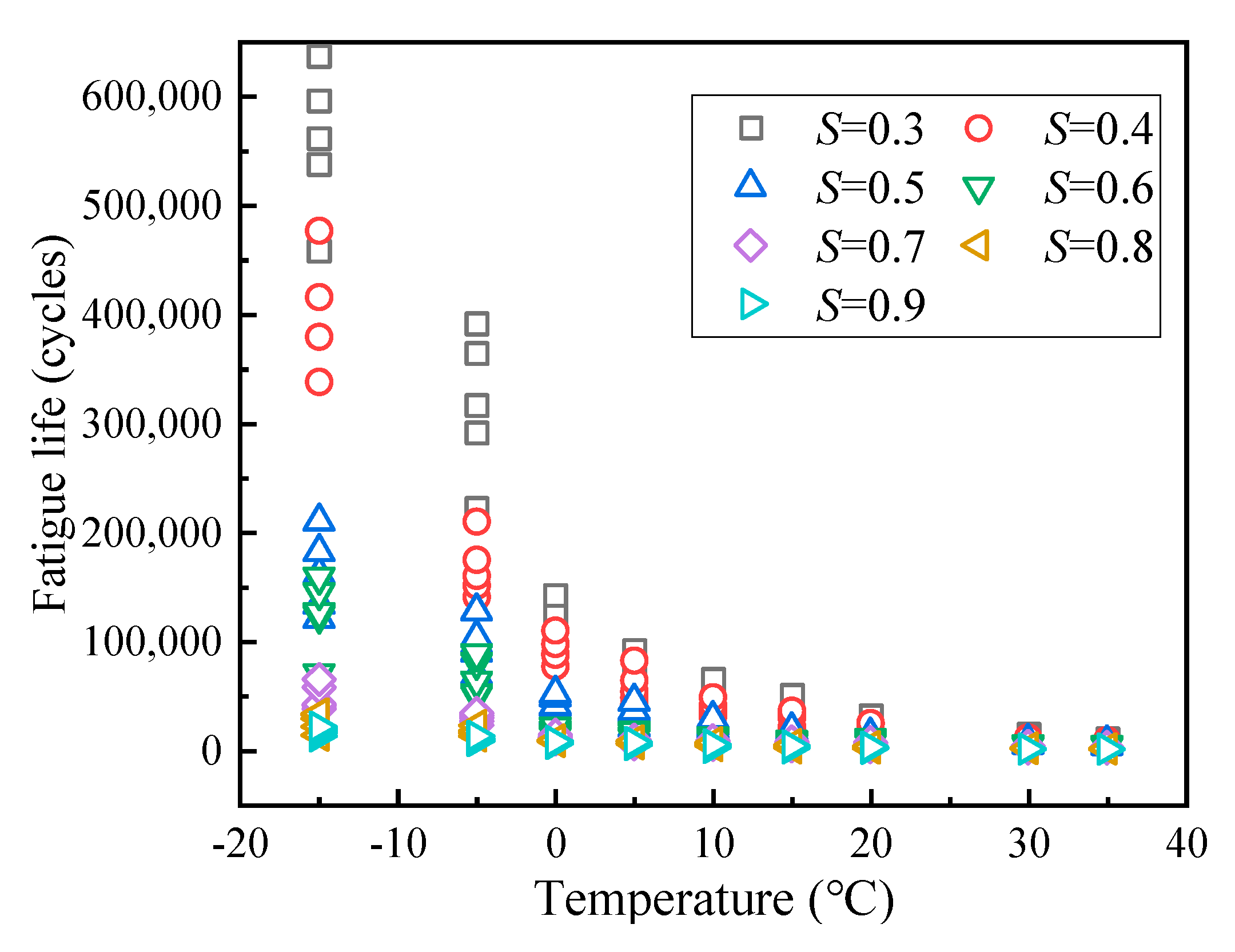
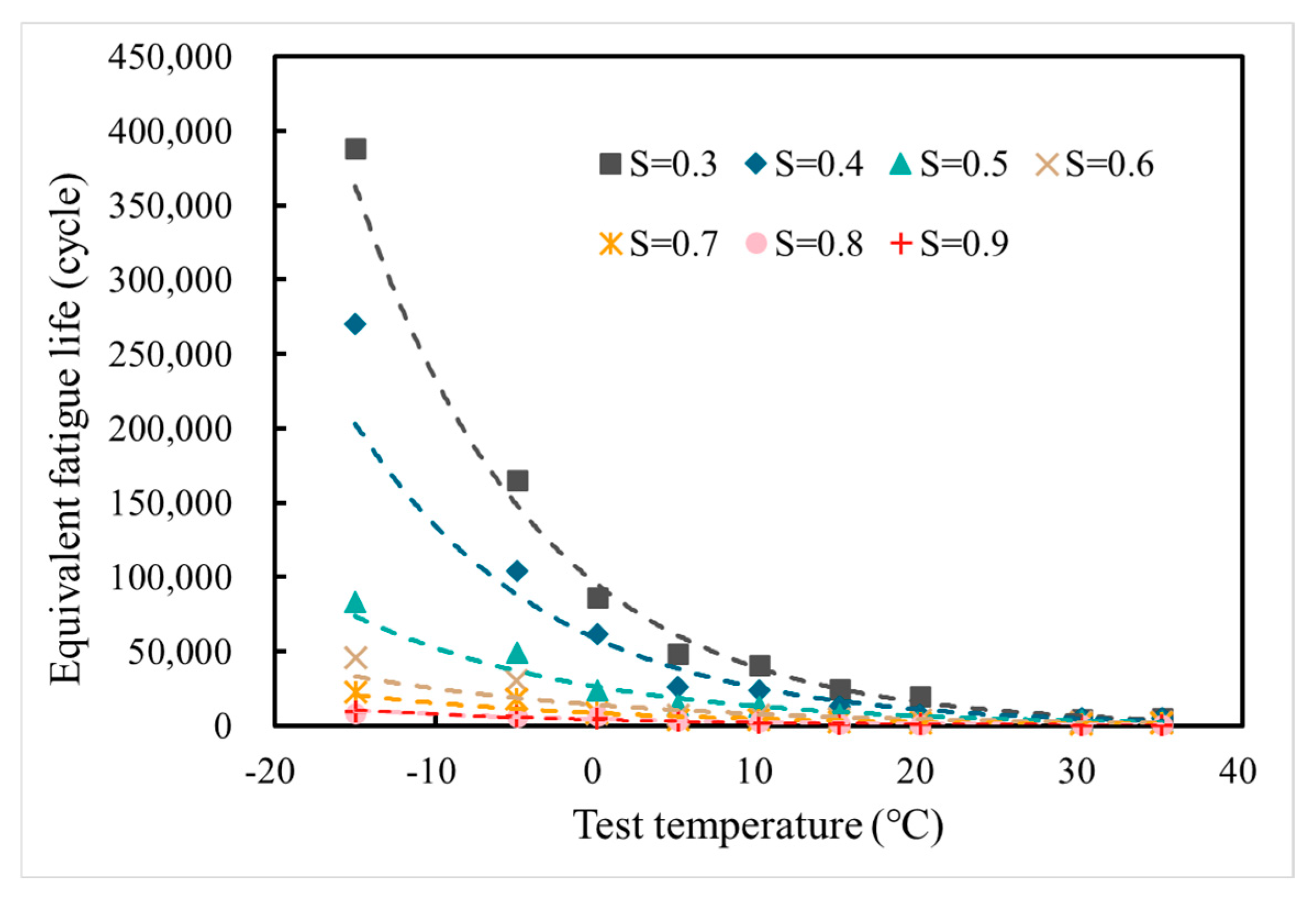
3.1. Equivalent Fatigue Life Calculation
3.2. Fatigue Cracking Displacement Evolution
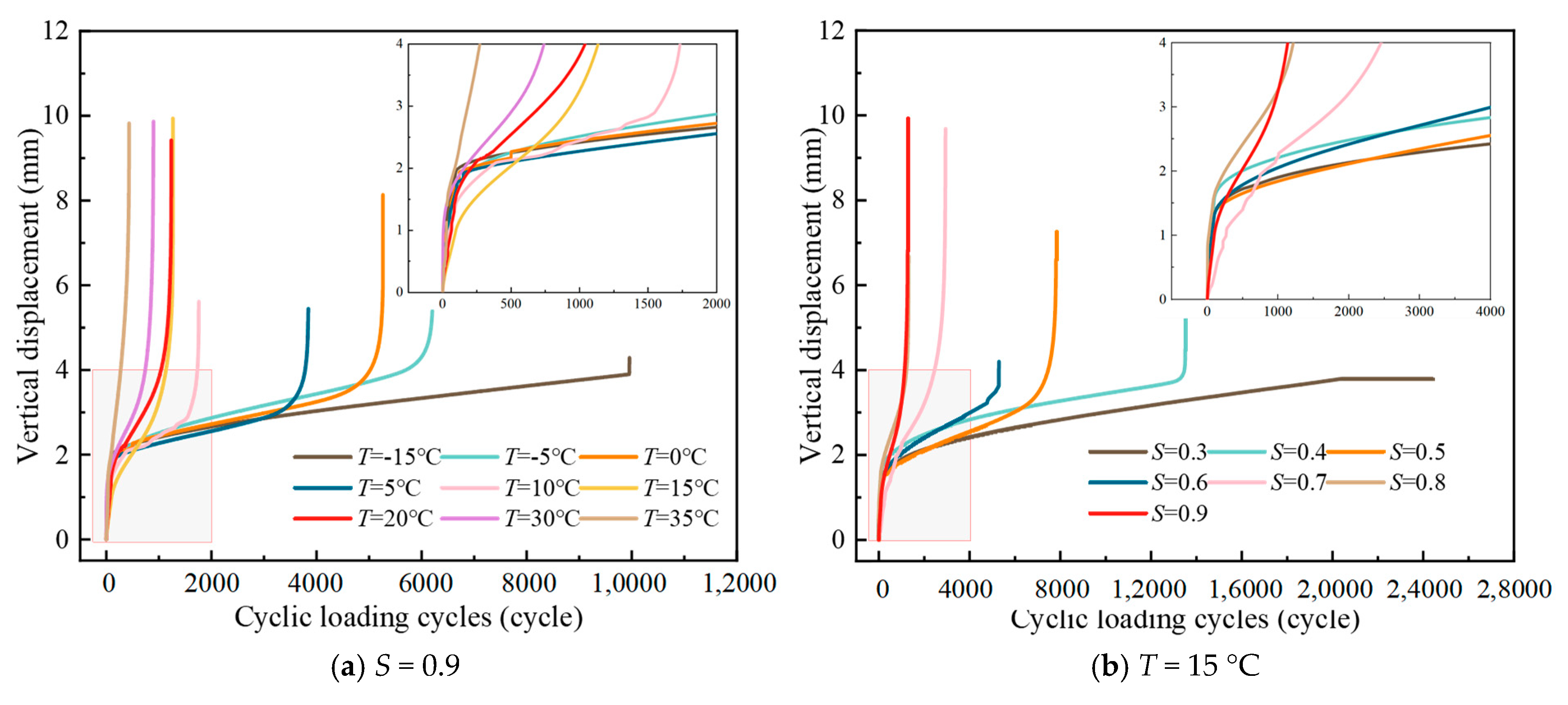
3.2.1. Vertical Displacement
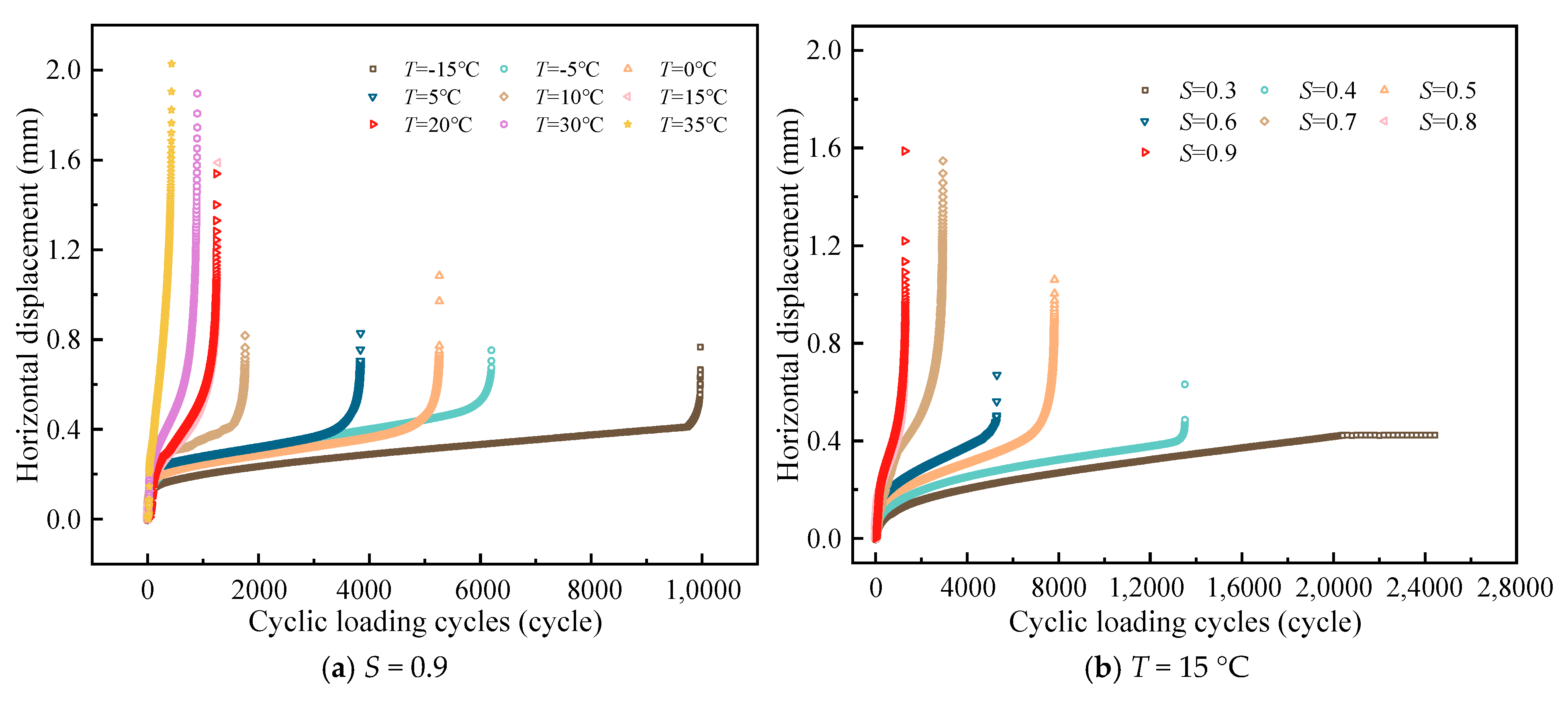
3.2.2. Horizontal Displacement
3.3. Crack Expansion Behavior Analysis
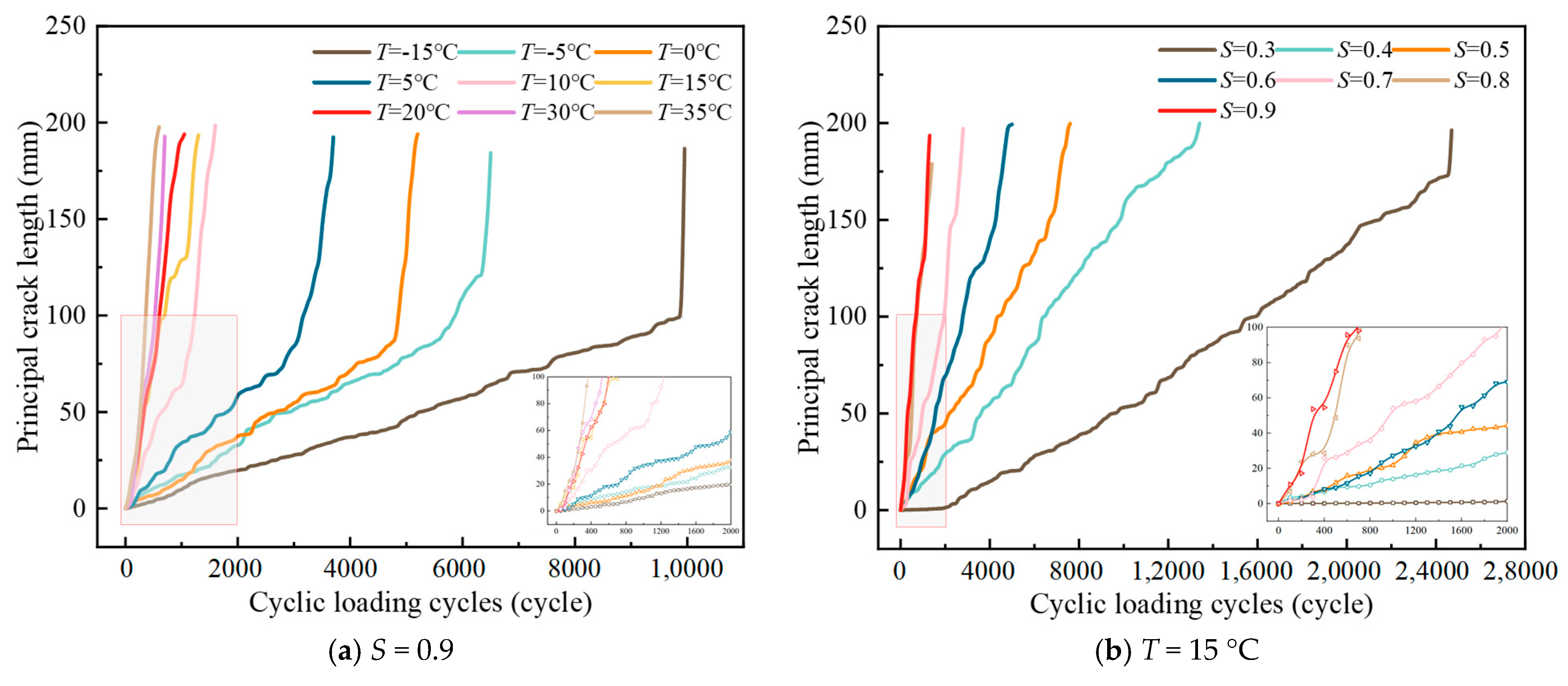
3.3.1. Main Crack Length
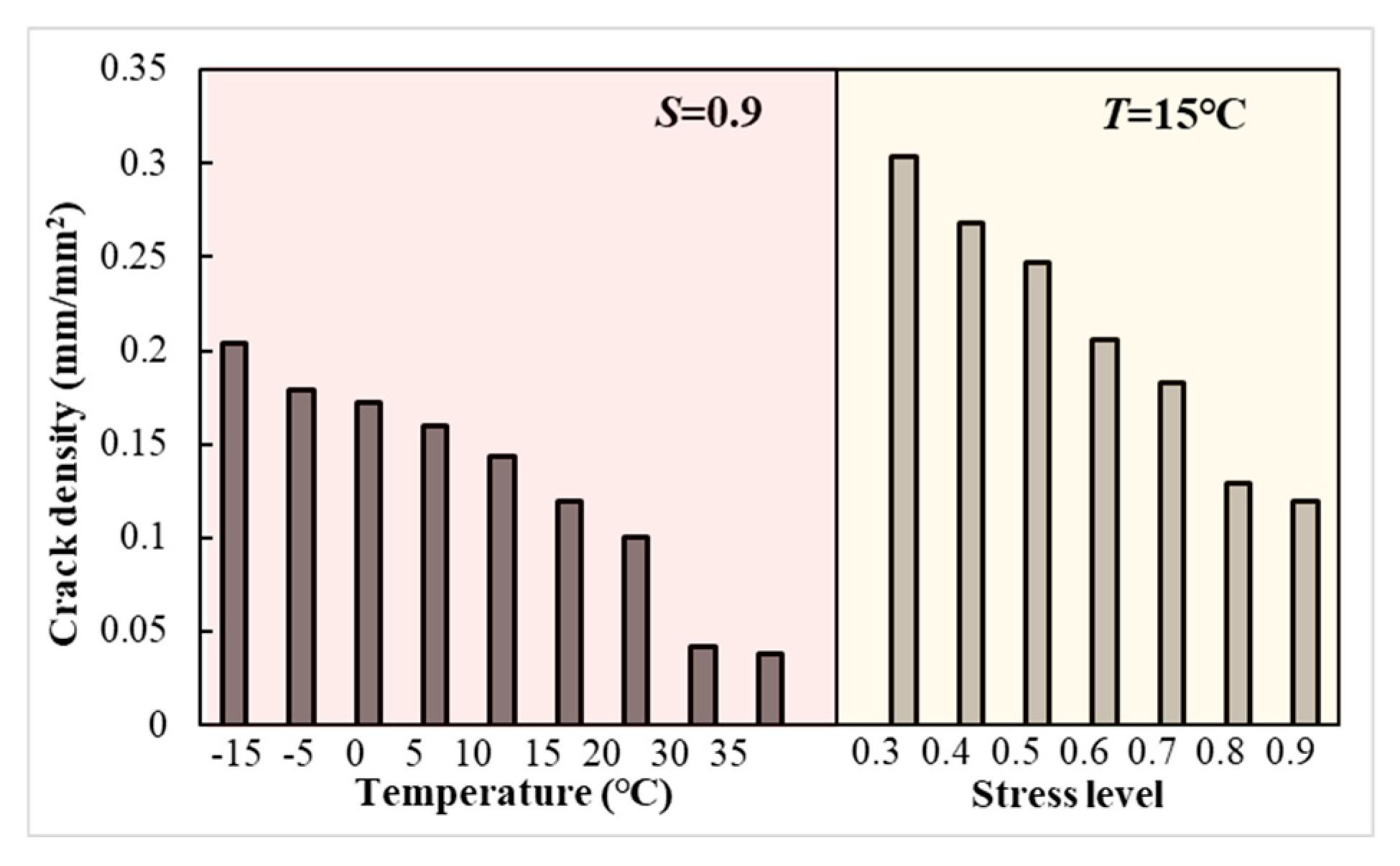
3.3.2. Crack Density
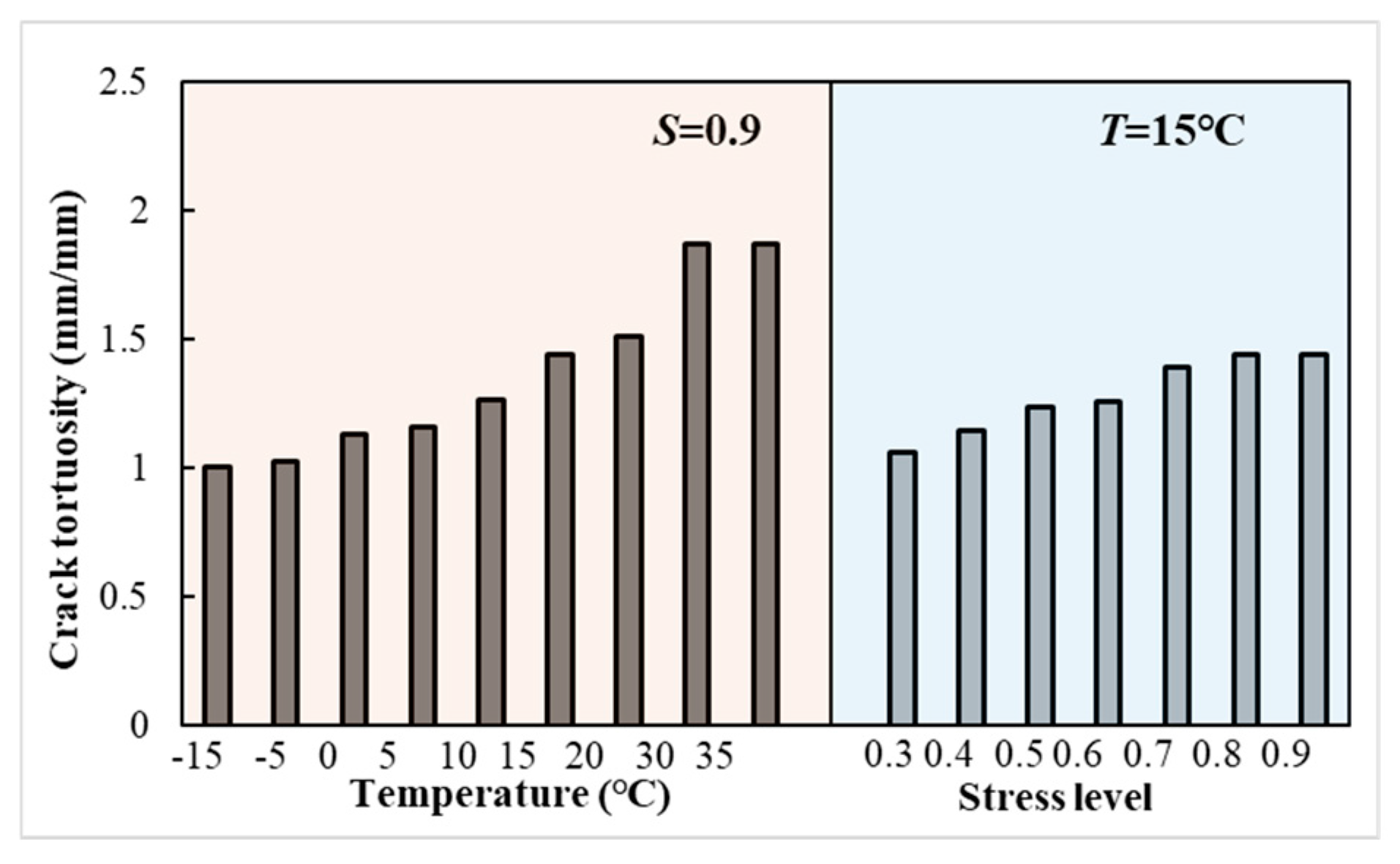
3.3.3. Crack Curvature
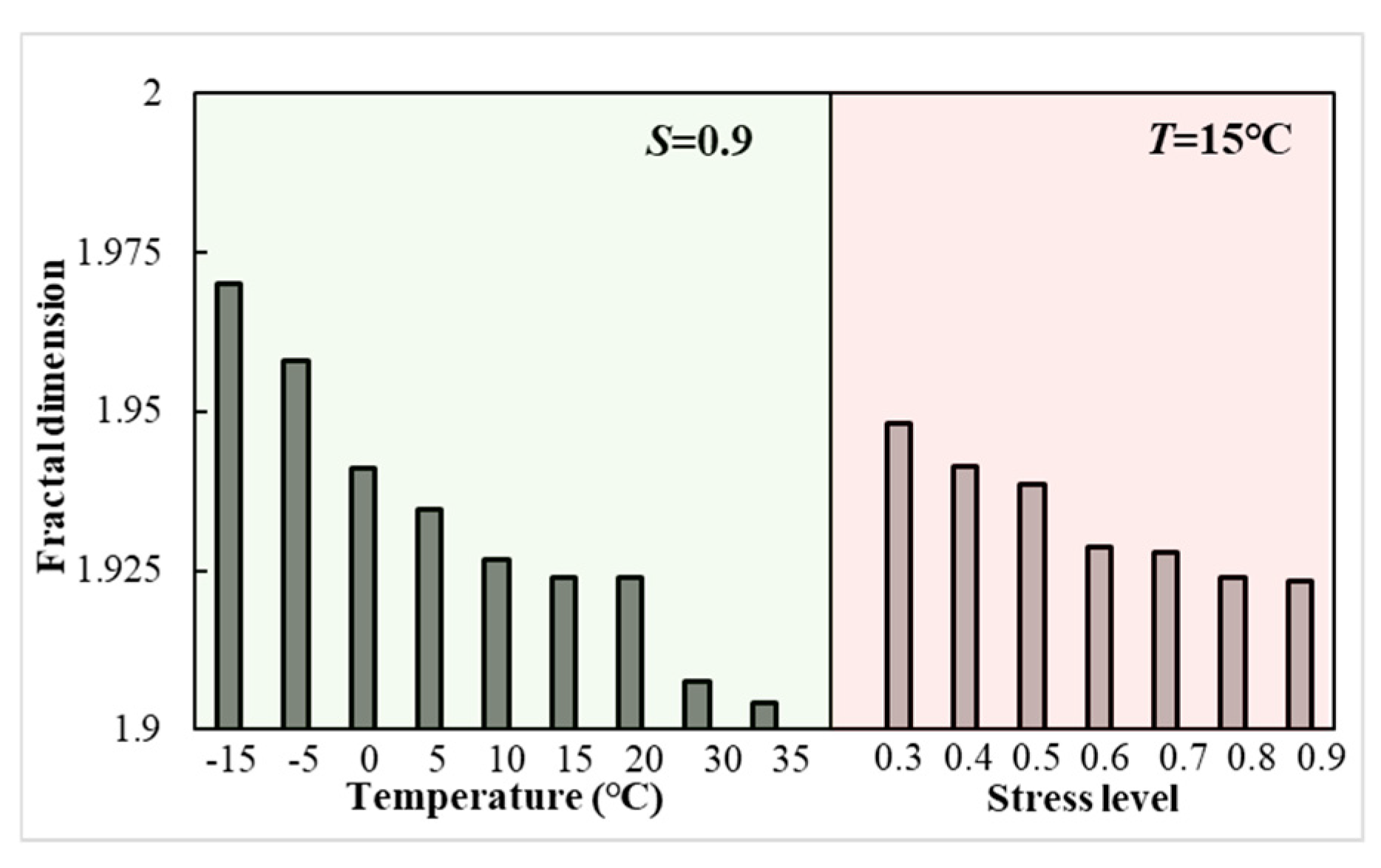
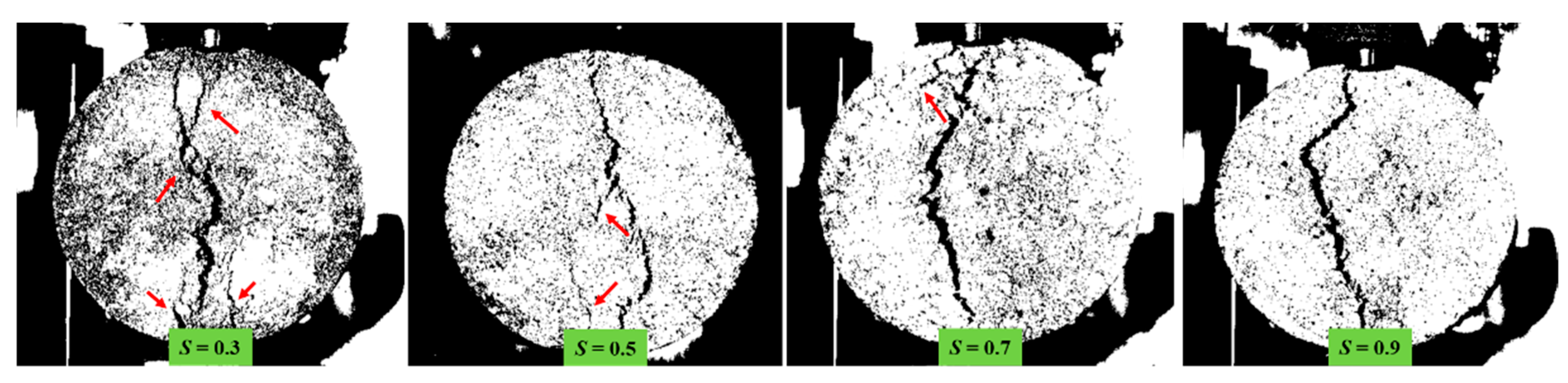
3.3.4. Fractal Dimension
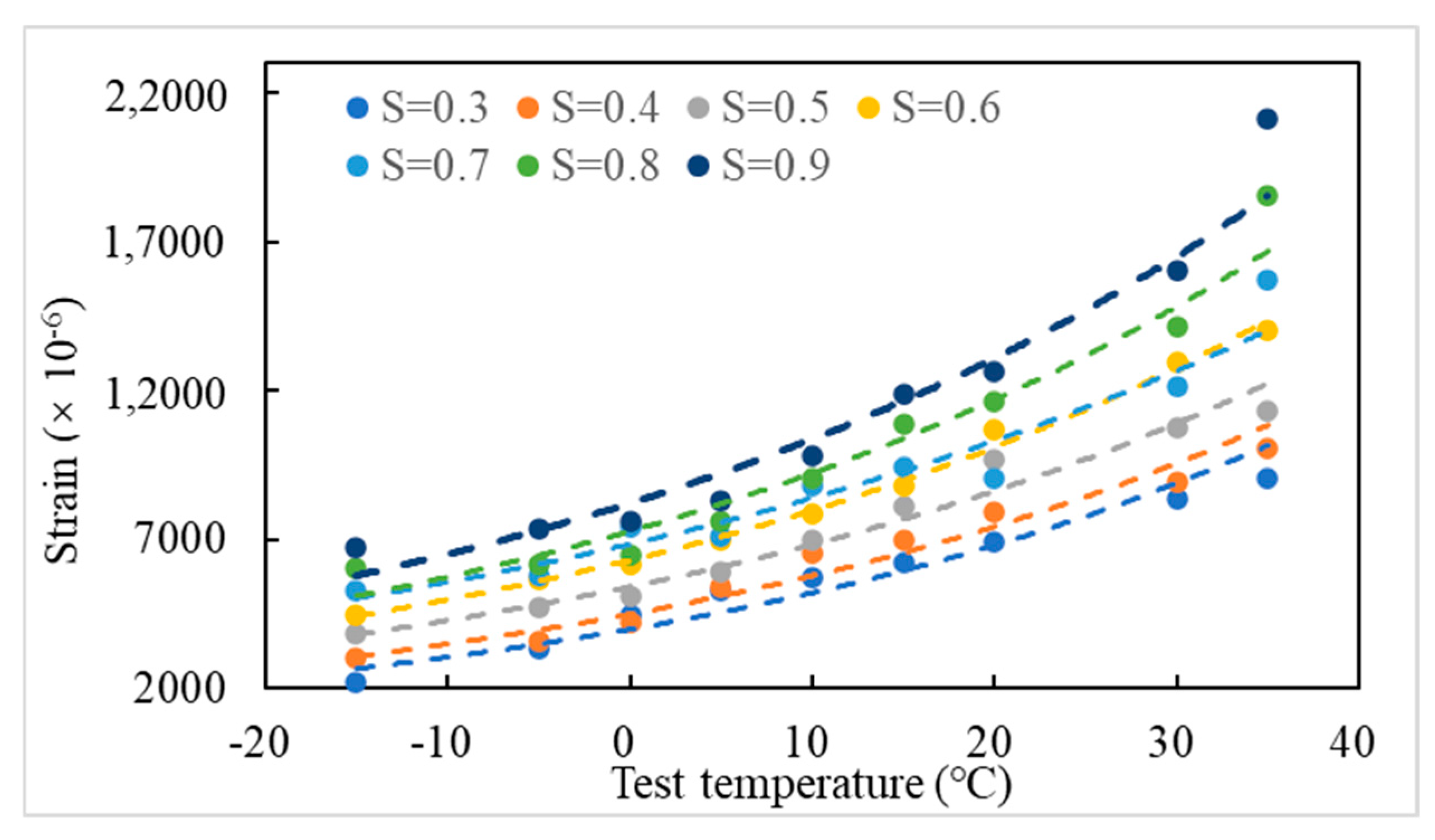
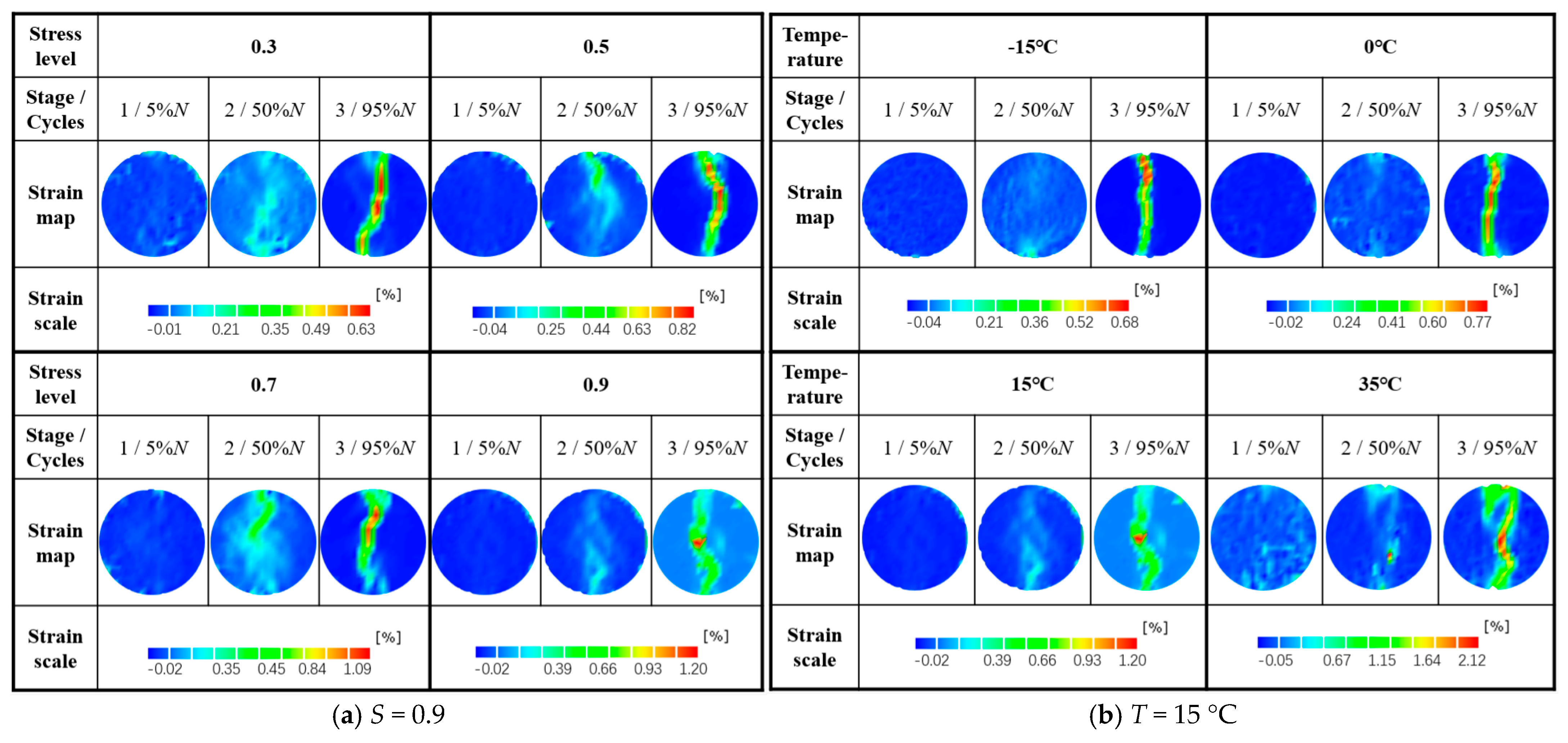
3.3.5. Strain
3.4. Fatigue Life Prediction Modeling
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qian, G.; Hu, C.; Yu, H.; Gong, X. Case study: Ten year field performance evaluation of flexible base asphalt pavement design in heavy load condition. J. Mater. Civ. Eng. 2020, 32, 04020187. [Google Scholar] [CrossRef]
- Ziari, H.; Khabiri, M.M. Interface condition influence on prediction of flexible pavement life. J. Civ. Eng. Manag. 2007, 13, 71–76. [Google Scholar] [CrossRef]
- Tian, T.; Jiang, Y.; Li, S.; Nie, C.; Yi, Y.; Zhang, Y.; Deng, C. Bending fatigue properties and prediction of asphalt mixtures with ultra-large aggregates. Constr. Build. Mater. 2024, 414, 134961. [Google Scholar] [CrossRef]
- Tian, T.; Jiang, Y.; Yi, Y.; Nie, C. The splitting fatigue properties of ultra-large particle size asphalt mixture under the coupling effect of temperature and load. Eng. Fract. Mech. 2025, 319, 110990. [Google Scholar] [CrossRef]
- Xing, C.; Tang, S.; Chang, Z.; Han, Z.; Li, H.; Zhu, B. A comprehensive review on the plant-mixed cold recycling technology of emulsified asphalt: Raw materials and factors affecting performances. Constr. Build. Mater. 2024, 439, 137344. [Google Scholar] [CrossRef]
- Wei, S.; Wang, Y.; Cheng, H.; Wang, D. Stress distributions in the textures of prefabricated pavement surface created with the assistance of 3D printing technology. Int. J. Pavement Eng. 2021, 24, 2005058. [Google Scholar] [CrossRef]
- Luo, X.; Gu, F.; Ling, M.; Lytton, R. Review of mechanistic-empirical modeling of top-down cracking in asphalt pavements. Constr. Build. Mater. 2018, 191, 1053–1070. [Google Scholar] [CrossRef]
- Fan, X.; Liu, C.; Lv, S.; Ge, D.; Liu, J.; Pan, Q. Unified fatigue characterization of asphalt mixture under multi-field coupling condition: Stress state, frequency, and temperature. Constr. Build. Mater. 2022, 353, 129027. [Google Scholar] [CrossRef]
- Li, X.; Sha, A.; Jiao, W.; Cao, Y.; Song, R. Strain response and creep behavior of asphalt mixture based on multi-damage fractional visco-elasto-plastic constitutive model. Constr. Build. Mater. 2025, 472, 140834. [Google Scholar] [CrossRef]
- Li, X.; Sha, A.; Song, R.; Jiao, W. Fractional viscoelastic constitutive modelling of real-time strain response for asphalt pavement composites subjected to simulating wheel loadings. Int. J. Pavement Eng. 2024, 25, 2380526. [Google Scholar] [CrossRef]
- Son, S.; Said, I.M.; Al-Qadi, I.L. Fracture degradation of asphalt concrete under repeated loading. J. Transp. Eng. B-Pave 2021, 147, 04021032. [Google Scholar] [CrossRef]
- Al-Khateeb, G.; Ghuzlan, K. The combined effect of loading frequency, temperature, and stress level on the fatigue life of asphalt paving mixtures using the IDT test configuration. Int. J. Fatigue 2014, 59, 254–261. [Google Scholar] [CrossRef]
- Ehsan, T.; Mohammad, Z.; Zahra, M.; Alireza, N.; Hossein, G.; Mohammad, W. Pre- and post-cracking behavior of asphalt mixtures under modes I and III at low and intermediate temperatures. Theor. Appl. Fract. Mec. 2023, 124, 103826. [Google Scholar] [CrossRef]
- Cheng, H.; Liu, J.; Sun, L.; Liu, L.; Zhang, Y. Fatigue behaviours of asphalt mixture at different temperatures in four-point bending and indirect tensile fatigue tests. Constr. Build. Mater. 2021, 273, 121675. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, H.; Yu, T.; Sun, J.; Shan, Z.; He, D. Meso-structural characteristics of porous asphalt mixture based on temperature-stress coupling and its influence on aggregate damage. Constr. Build. Mater. 2022, 342, 128064. [Google Scholar] [CrossRef]
- Luo, Y.; Wu, H.; Song, W.; Yin, J.; Zhan, Y.; Yu, J.; Wada, S. Thermal fatigue and cracking behaviors of asphalt mixtures under different temperature variations. Constr. Build. Mater. 2023, 369, 130623. [Google Scholar] [CrossRef]
- Margaritis, A.; Jacobs, G.; Pipintakos, G.; Blom, J.; Van den Bergh, W. Fatigue resistance of bituminous mixtures and mortars containing high reclaimed asphalt content. Materials 2020, 13, 5680. [Google Scholar] [CrossRef]
- Guo, Q.; Wang, H.; Gao, Y.; Jiao, Y.; Liu, F.; Dong, Z. Investigation of the low-temperature properties and cracking resistance of fiber-reinforced asphalt concrete using the DIC technique. Eng. Fract. Mech. 2020, 229, 106951. [Google Scholar] [CrossRef]
- Ren, J.; Li, M.; Zhao, Z.; Lu, H.; Tong, X.; Xu, Y.; Li, D. Effects of moisture contents, fracture modes, and temperatures on fracture toughness and crack distribution of asphalt concrete at low-temperature. Case Stud. Constr. Mater. 2024, 21, e03647. [Google Scholar] [CrossRef]
- Wang, Y.; Li, M.; Chen, M.; Zhao, Z.; Zhu, F.; Jiang, Y.; Ren, J. Synergistic effects of aggregate-void distribution on low-temperature fracture performance and crack propagation of asphalt concrete under mode I and mode II fracturing. Eng. Fract. Mech. 2024, 307, 110352. [Google Scholar] [CrossRef]
- Lytton, R.L.; Zhang, Y.; Gu, F.; Luo, X. Characteristics of damaged asphalt mixtures in tension and compression. Int. J. Pavement Eng. 2018, 19, 292–306. [Google Scholar] [CrossRef]
- Hill, B.; Buttlar, W. Evaluation of polymer modification in asphalt mixtures through digital image correlation and performance space diagrams. Constr. Build. Mater. 2016, 122, 667–673. [Google Scholar] [CrossRef]
- Jiang, J.; Ni, F.; Dong, Q.; Zhao, Y.; Xu, K. Fatigue damage model of stone matrix asphalt with polymer modified binder based on tensile strain evolution and residual strength degradation using digital image correlation methods. Measurement 2018, 123, 30–38. [Google Scholar] [CrossRef]
- Yuan, M. Study on Fatigue Failure Mechanism of Asphalt Mixture Based on Digital Speckle Correlation Method. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2013. (In Chinese). [Google Scholar]
- Han, Z.; Liu, Z.; Jiang, Y.; Wu, P.; Li, S.; Sun, G.; Zhang, L. Engineering properties and air void characteristics of cold recycled mixtures with different compaction methods. J. Build. Eng. 2023, 77, 107430. [Google Scholar] [CrossRef]
- Ministry of Transport of the People’s Republic of China. Test Code for Asphalt and Asphalt Mixtures in Highway Engineering [S]. JTG E20-2011; China Communications Press: Beijing, China, 2011. (In Chinese) [Google Scholar]
- Li, M.; Han, Z.; Cheng, H.; Yang, R.; Yuan, J.; Jin, T. Low-temperature performance im-provement strategies for high RAP content recycled asphalt mixtures: Focus on RAP gradation variability and mixing process. Fuel 2025, 387, 134362. [Google Scholar] [CrossRef]
- Tian, T.; Jiang, Y.; Yi, Y.; Fan, J.; Deng, C. Laboratory fatigue performance of the cement-stabilised loess fabricated using different compaction methods. Road Mater. Pavement Des. 2023, 24, 501–519. [Google Scholar] [CrossRef]
- Han, Z.; Jiang, D.; Liu, L.; Sun, L. Low-temperature performance improvement measures for emulsified asphalt cold re-cycled mixture: A comparative study. J. Mater. Civ. Eng. 2023, 35, 04023108. [Google Scholar] [CrossRef]
- De Freitas, E.F.; Pereira, P.; Picado, L.; Papagiannakis, A.T. Effect of construction quality, temperature, and rutting on initiation of top-down cracking. Transp. Res. Rec. 2005, 1929, 174–182. [Google Scholar] [CrossRef]
- Epps, J.; Monismith, C. Fatigue of Asphalt Concrete Mixtures—Summary of Existing Information. In Fatigue of Compacted Bituminous Aggregate Mixtures; Gallaway, B.M., Ed.; ASTM International: West Conshohocken, PA, USA, 1972. [Google Scholar]
- Pell, P. Fatigue characteristics of bitumen and bituminous mixes. In Proceedings of the International Conference on the Structural Design of Asphalt Pavements, Ann Arbor, MI, USA, 20–24 August 1962. [Google Scholar]
- Pell, P.; Taylor, I. Asphaltic road materials in fatigue. In Proceedings of the Association of Asphalt Paving Technologists Proceedings (AAPT), Los Angeles, CA, USA, 10–12 February 1969; pp. 577–593. [Google Scholar]
- Yu, J.; Li, Z.; Wang, S. A study of fatigue performance of asphalt mixes based on the uniform design method. In Proceedings of the 24th Southern African Conference, Pretoria, South Africa, 11–13 July 2005. [Google Scholar]
- Radeef, H.; Hassan, N.; Abidin, A. Effect of aging and moisture damage on the cracking resistance of rubberized asphalt mixture. Mater. Today Proc. 2021, 42, 2853–2858. [Google Scholar] [CrossRef]
- Al-Qadi, I.; Ozer, H.; Lambros, J. Testing Protocols to Ensure Mix Performance W/High RAP and RAS; Illinois Center for Transportation: Rantoul, IL, USA, 2015. [Google Scholar]
- Li, J.; Wang, Z.; Zhang, Z.; Chen, C.; Wu, C.; Jing, H.; Tang, B. Evaluation on performances restoration and warm-mix effect of rejuvenated SBS modified bitumen incorporating a compound rejuvenator. Mater. Struct. 2025, 58, 17. [Google Scholar] [CrossRef]
- Shi, K.; Ma, F.; Lou, B.; Barbieri, D.; Li, J.; Song, R.; Yuan, D. Comprehensive Evaluation of Sulfur Content and Curing Duration Effects on the Rheological Performance of Sulfur-Extended Bitumen. Fuel 2025, 393, 134979. [Google Scholar] [CrossRef]
- Jia, C. Composite Material Course; Higher Education Press: Beijing, China, 2010. (In Chinese) [Google Scholar]
- Tian, T.; Jiang, Y.; Yi, Y.; Zhang, Y.; Fan, J.; Zhang, H. Dynamic viscoelastic properties of ultra-large particle size asphalt mixture based on fractional derivative constitutive model. Constr. Build. Mater. 2024, 445, 137893. [Google Scholar] [CrossRef]
- Jameson, G.; Sharp, K.; Potter, D. New guidelines for the design of flexible pavements for Australian conditions. In Proceedings of the International Conference on Asphalt Pavements, Copenhagen, Denmark, 17–22 August 2002. [Google Scholar]







| Test Projects | Measured Values | |
|---|---|---|
| Penetration (25 °C, 100 g, 5 s) (0.1 mm) | 69 | |
| Ductility (5 cm/min, 15 °C) (cm) | >100 | |
| Ductility (5 cm/min, 10 °C) (cm) | 39 | |
| Softening point (°C) | 47.3 | |
| Density of asphalt (g/cm3, 15 °C) | 1.038 | |
| Penetration index (PI) | −0.315 | |
| Solubility in trichloroethylene (%) | 99.91 | |
| Paraffin content (%) | 1.6 | |
| Dynamic viscosity (Pa·s, 60 °C) | 210 | |
| Rolling Thin-Film Oven Test (RTFO) (163 °C, 5 h) | Mass loss (%) | −0.1 |
| Ductility (cm, 10 °C) | 7 | |
| Residual penetration ratio (%, 25 °C) | 63 | |
| Test Projects | Measured Values |
|---|---|
| Asphalt content (%) | 2.9 |
| Density (g/cm3) | 2.551 |
| Air voids (%) | 3.9 |
| Voids in the mineral aggregate (%) | 7.8 |
| Voids filled with asphalt (%) | 51.3 |
| Compressive strength (MPa, 20 °C) | 7.4 |
| Splitting strength (MPa, 20 °C) | 1.15 |
| Bending strength (MPa, 20 °C) | 3.02 |
| Test Parameter | Test Temperature (°C) | Load Control Mode | Loading Frequency | Stress Level | Waveform |
|---|---|---|---|---|---|
| Set value | 35, 30, 20, 15, 10, 5, 0, −5, −15 | Stress control | 10 Hz | 0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9 | Sinusoidal wave |
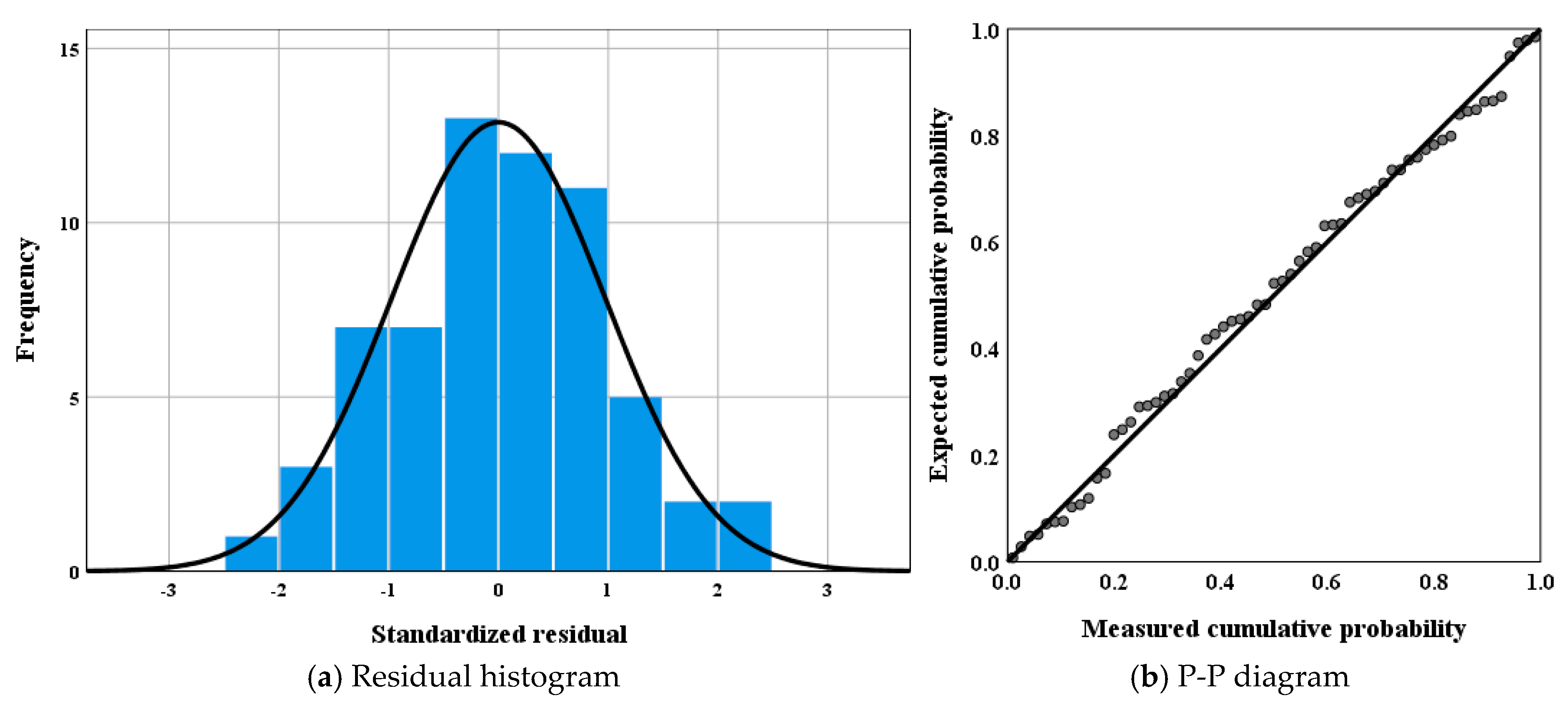
| Statistical Analysis Parameter | R | R2 | R2 After Adjustment | Errors in Standard Estimates | Durbin-Watson Value |
|---|---|---|---|---|---|
| Calculated value | 0.977 | 0.954 | 0.951 | 0.14328 | 1.310 |
| Index | Calculated Values of the Following Statistical Analysis Parameters | ||||
|---|---|---|---|---|---|
| Sum of Squares | Degree of Freedom | Mean Square | F Value | Significance | |
| Regression | 25.024 | 3 | 8.341 | 406.292 | 0.000 |
| Residual error | 1.211 | 59 | 0.021 | - | - |
| total | 26.235 | 62 | - | - | - |
| Argument | Statistical Analysis Coefficients of Different Dependent Variables | ||||||
|---|---|---|---|---|---|---|---|
| Unstandardized Coefficient | Standardization Coefficient Beta | t Value | Significance | Collinear Statistics | |||
| B | Standard Error | Tolerance | VIF | ||||
| constant | 9.741 | 1.670 | - | 5.832 | 0.000 | - | - |
| Lgε | −1.213 | 0.480 | −0.358 | −2.526 | 0.014 | 0.039 | 25.665 |
| T | −0.017 | 0.005 | −0.404 | −3.341 | 0.001 | 0.053 | 18.713 |
| S | −1.579 | 0.255 | −0.489 | −6.204 | 0.000 | 0.126 | 7.952 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, T.; Jiang, Y.; Yi, Y.; Nie, C.; Deng, C. Fatigue Cracking Characteristics of Ultra-Large Particle Size Asphalt Mixture Under Temperature and Loading Using Digital Image Correlation Techniques. Materials 2025, 18, 1475. https://doi.org/10.3390/ma18071475
Tian T, Jiang Y, Yi Y, Nie C, Deng C. Fatigue Cracking Characteristics of Ultra-Large Particle Size Asphalt Mixture Under Temperature and Loading Using Digital Image Correlation Techniques. Materials. 2025; 18(7):1475. https://doi.org/10.3390/ma18071475
Chicago/Turabian StyleTian, Tian, Yingjun Jiang, Yong Yi, Chenliang Nie, and Changqing Deng. 2025. "Fatigue Cracking Characteristics of Ultra-Large Particle Size Asphalt Mixture Under Temperature and Loading Using Digital Image Correlation Techniques" Materials 18, no. 7: 1475. https://doi.org/10.3390/ma18071475
APA StyleTian, T., Jiang, Y., Yi, Y., Nie, C., & Deng, C. (2025). Fatigue Cracking Characteristics of Ultra-Large Particle Size Asphalt Mixture Under Temperature and Loading Using Digital Image Correlation Techniques. Materials, 18(7), 1475. https://doi.org/10.3390/ma18071475






