Material Flow Control and Process Design in Constraint Ring Rolling of Thin-Walled Conical Cylinders with Three Ring Ribs
Abstract
1. Introduction
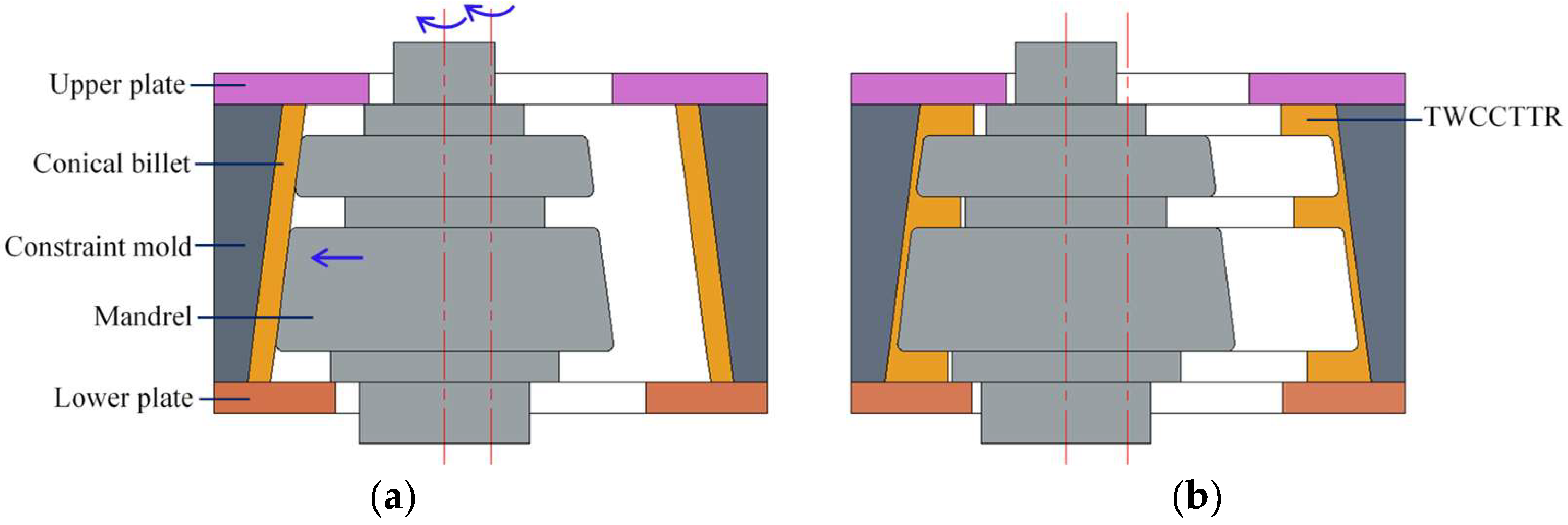
2. Analysis of Material Flow in Single-Pass CRR of TWCCTRR
2.1. Material and Geometric Parameters
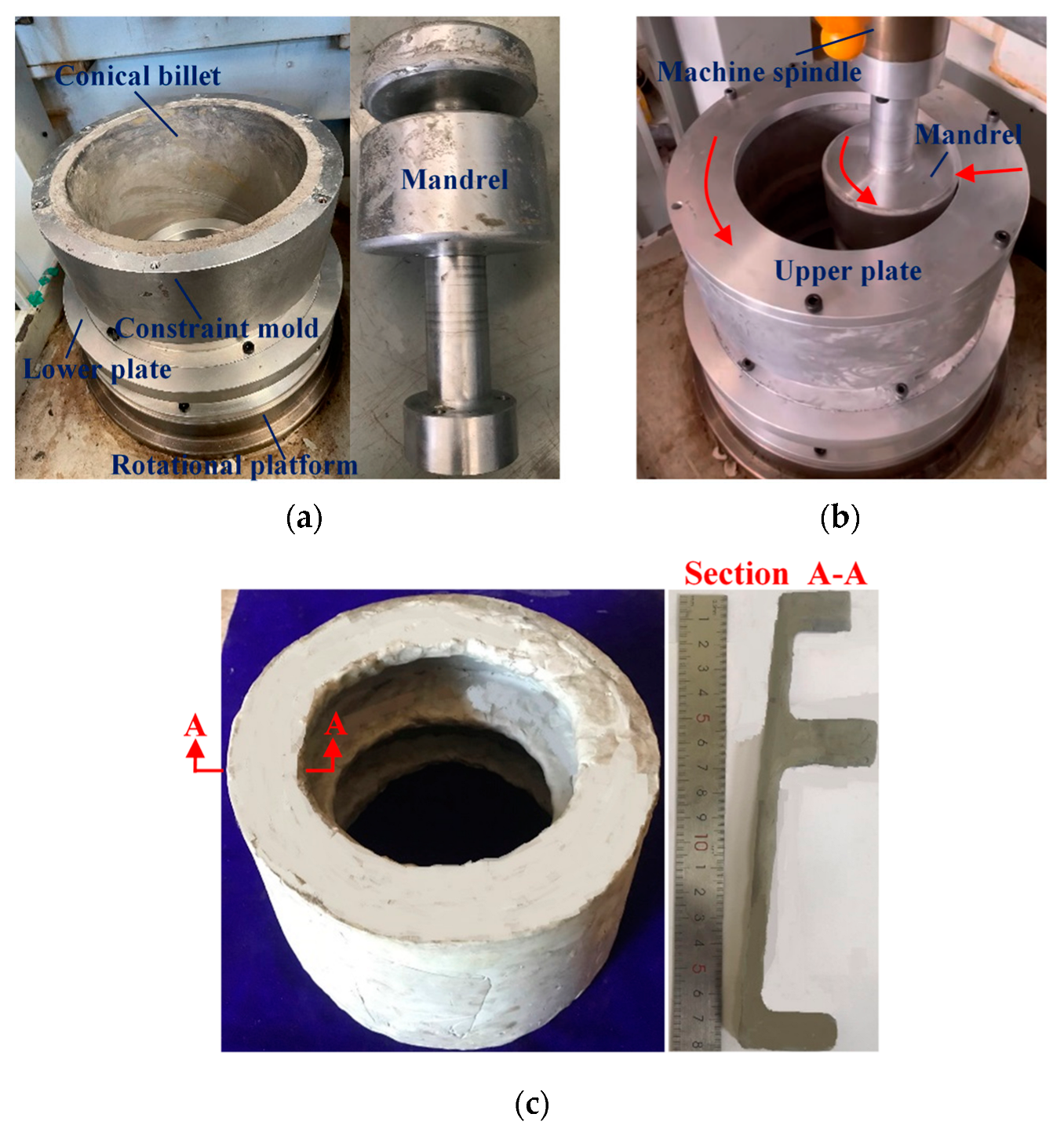
2.2. Physical Experiment
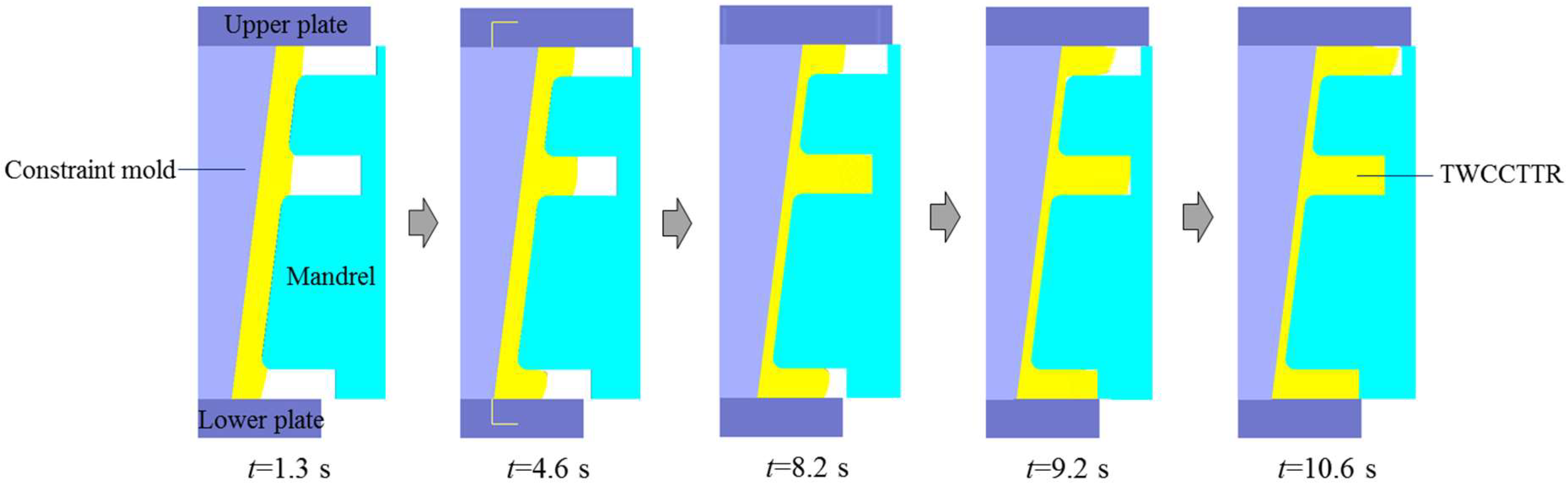
2.3. Numerical Modeling and Analysis
3. Process Design and Analysis for Double-Pass CRR of TWCCTRR
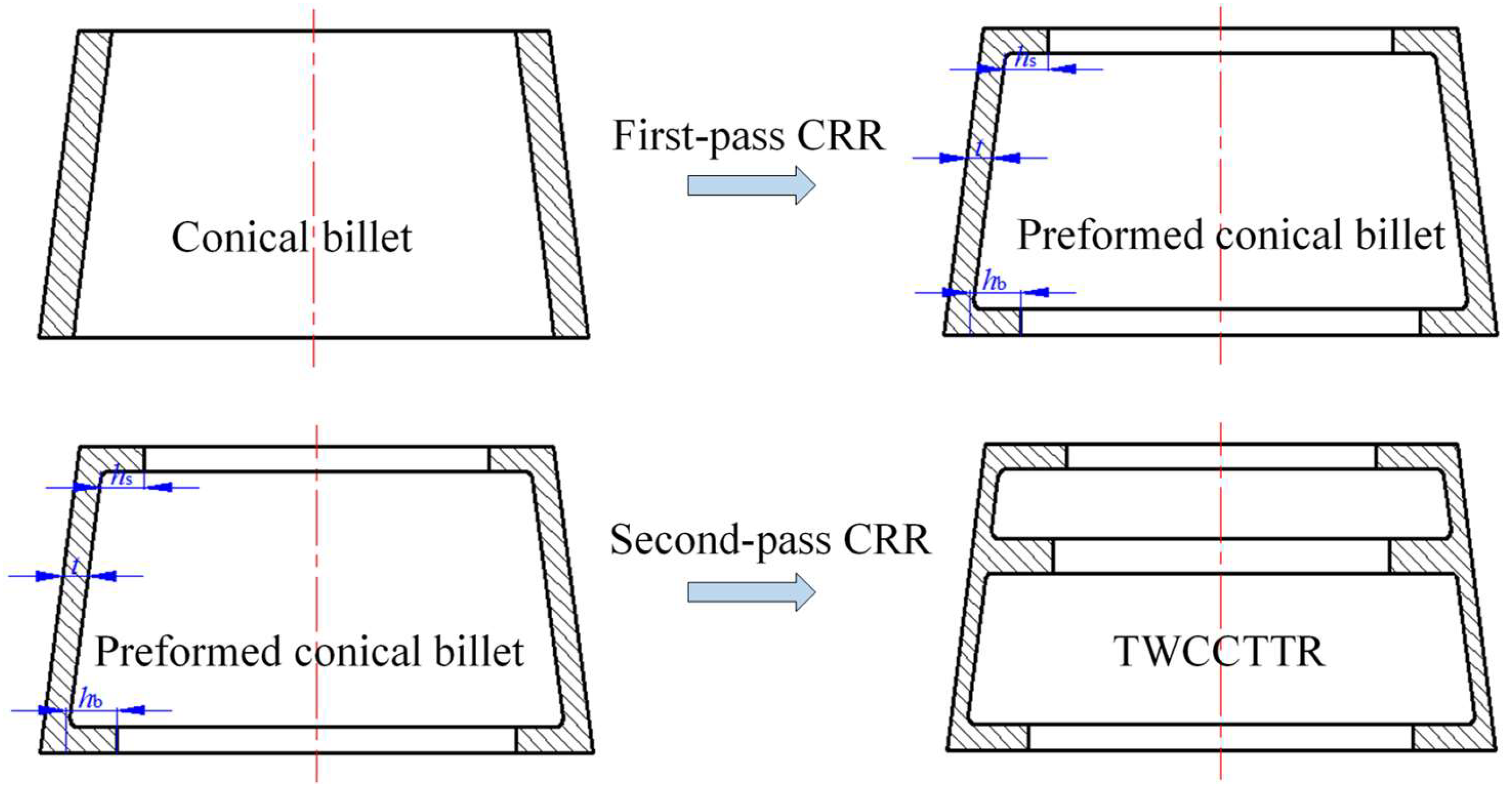
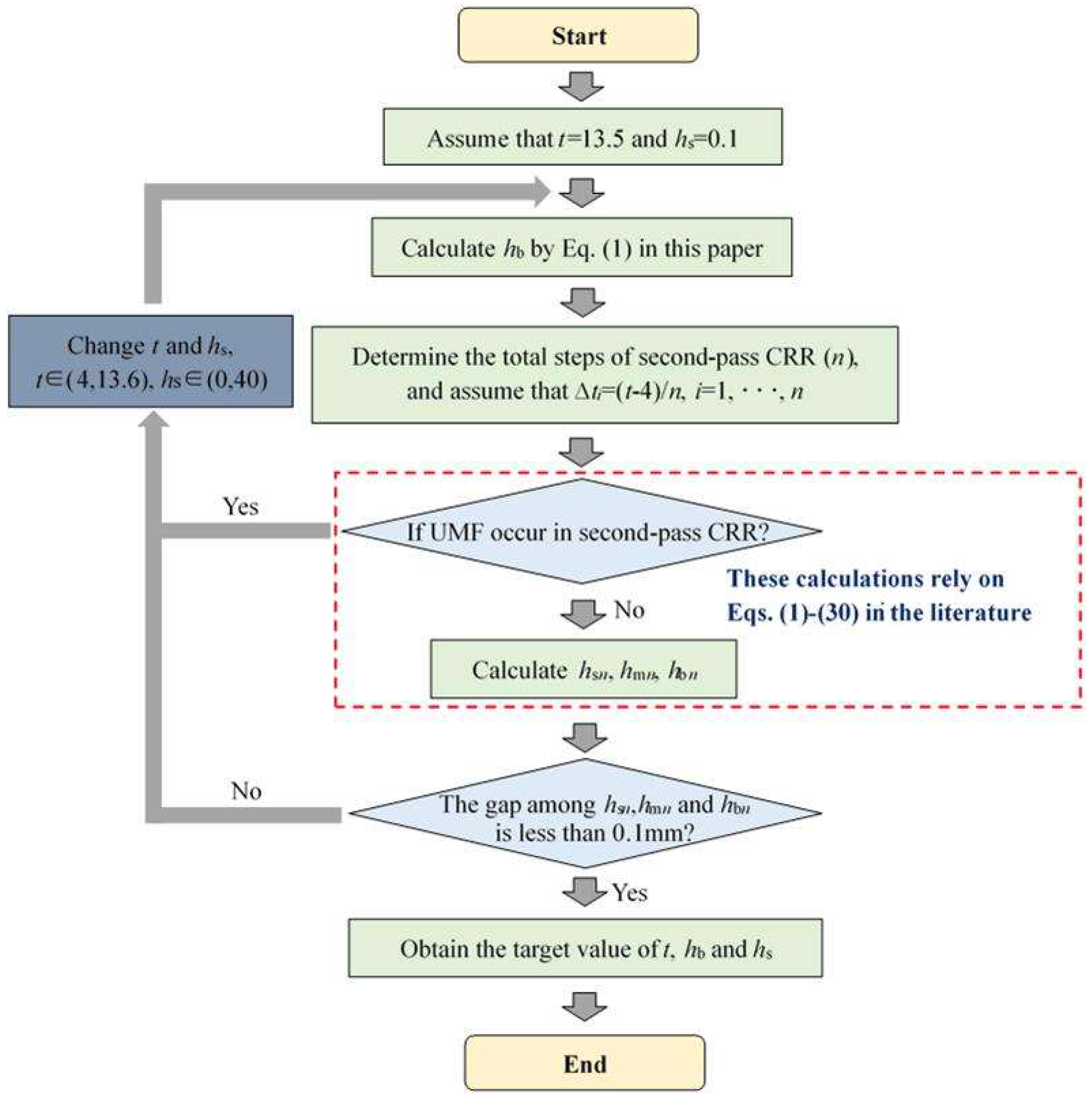
3.1. Design of Preformed Conical Billet
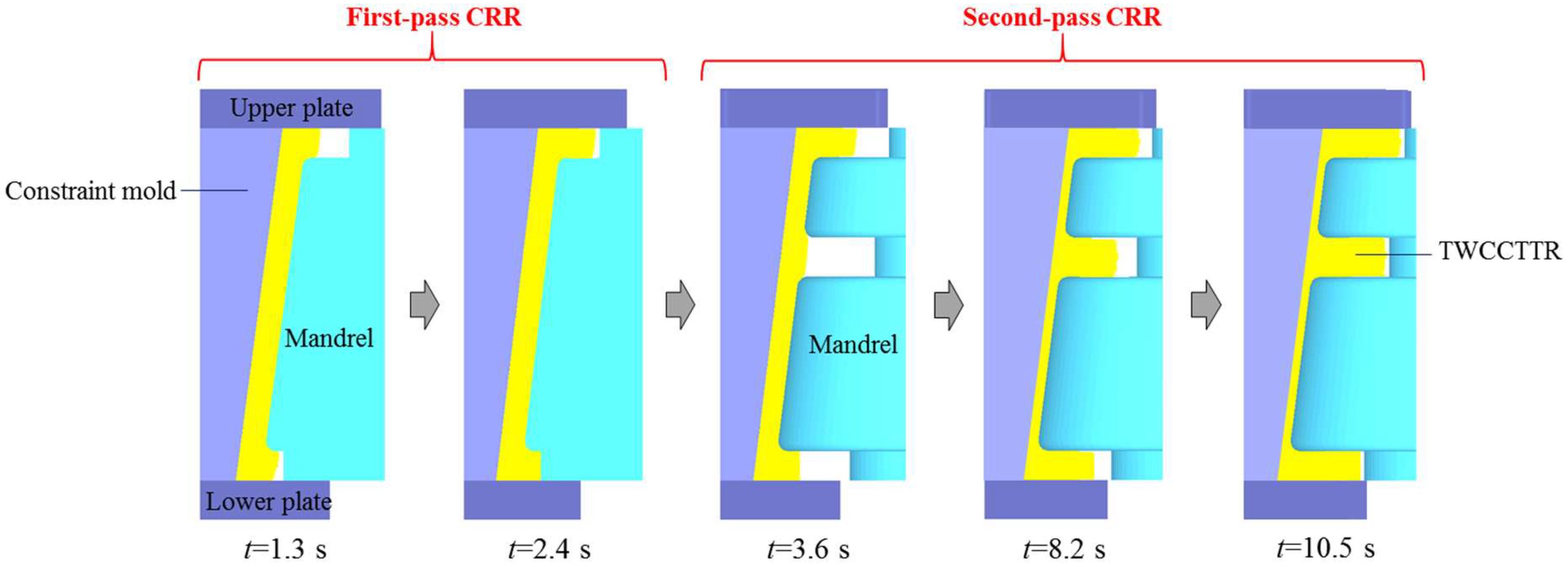
3.2. Analysis of Material Flow in Double-Pass CRR of TWCCTRR
4. Contrastive Analysis of Deformation Behaviors in Single-Pass CRR and Double-Pass CRR
5. Conclusions
- (1)
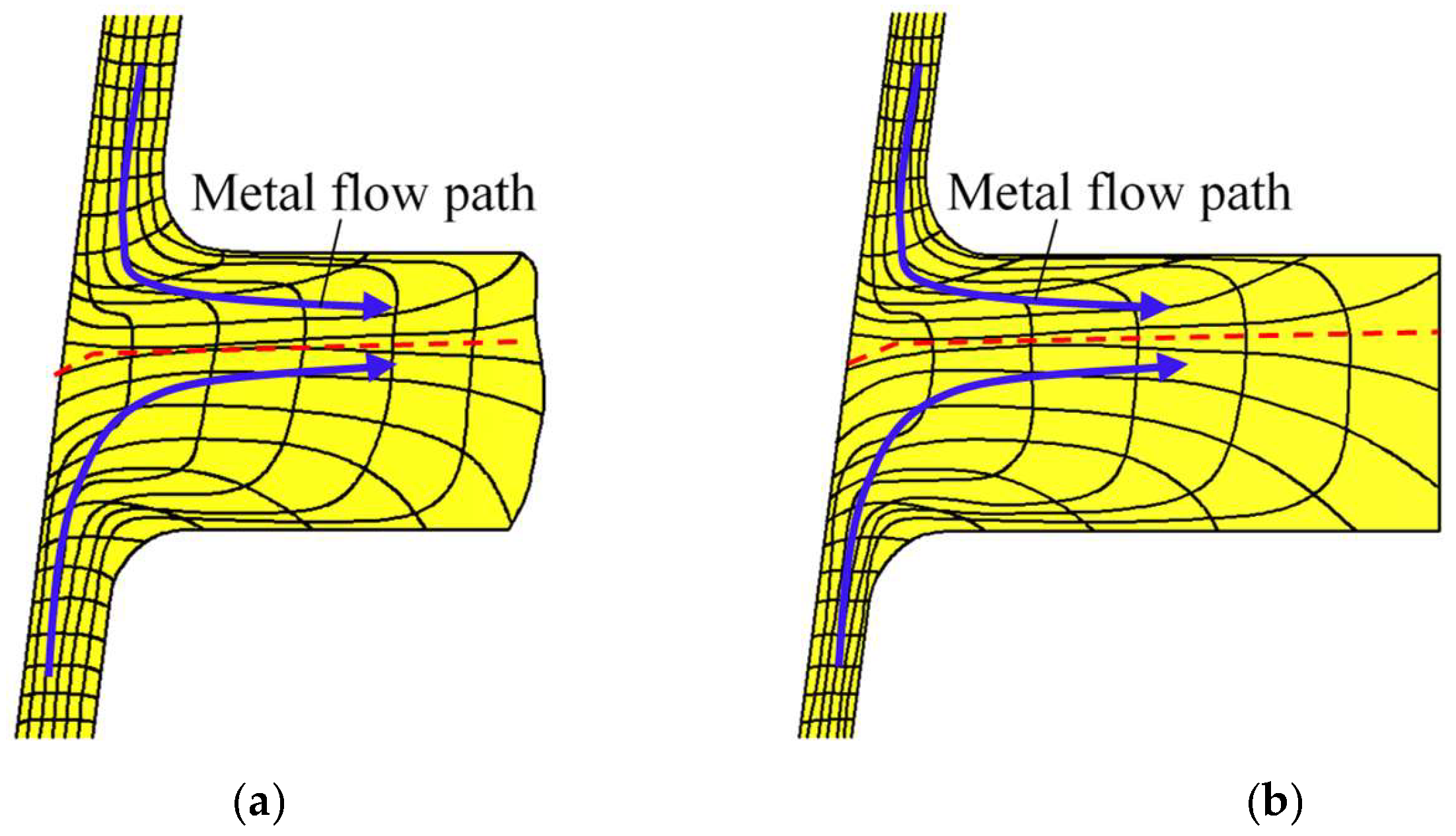
- During the single-pass CRR of TWCCTRR, the growth of the rib at the middle part is the fastest, while the growth of the rib at the small part is the slowest among the three ribs at the large, middle and small parts. Once the two ribs at the large and middle parts are completely filled, the material in the upper and lower loading areas of the skin flows together to the rib at the small part, resulting in the occurrence of UMF around the rib at the middle part.
- (2)
- The double-pass CRR process is proposed for forming TWCCTRR without UMF; that is, a conical billet is formed into the conical billet with two ring ribs by the first-pass CRR, and then the preformed conical billet with two ring ribs is formed into TWCCTRR by the second-pass CRR. The dimensions of the preformed conical billet with two ribs are critical to avoiding UMF in the second-pass CRR. Thus, a high-efficiency design method of preformed conical billet with two ribs is proposed, which ensures that the three ribs could be simultaneously and completely filled in the second-pass CRR to avoid UMF.
- (3)
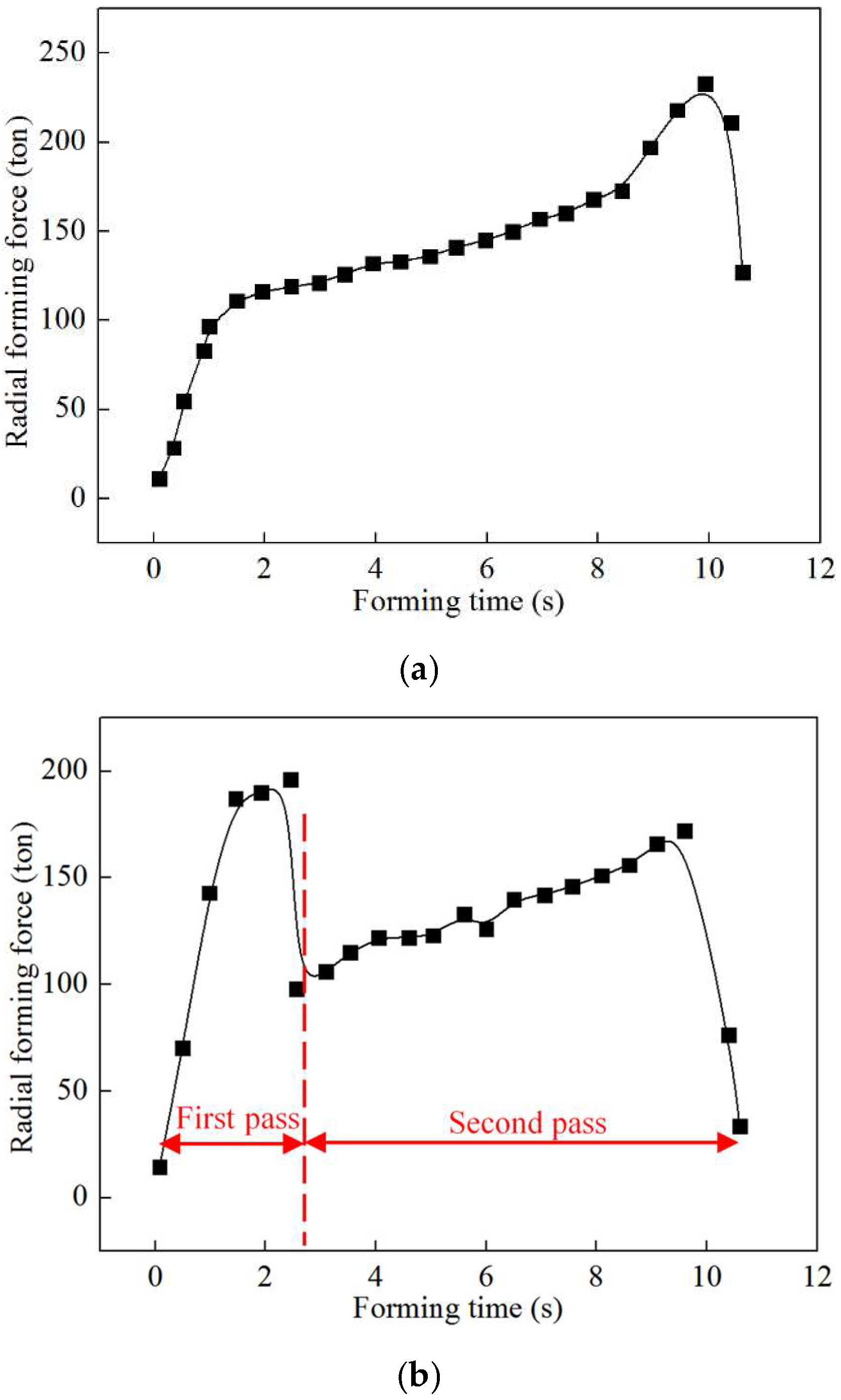
- In contrast to single-pass CRR, the deformation homogeneity of TWCCTRR is obviously improved, and meanwhile, the maximum radial forming force is reduced by approximately 15% in the double-pass CRR due to the variation in material flow mode.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gao, P.; Gong, Y.; Ren, Z.; Zhan, M. A new spinning-extrusion forming technology for the inner-ribbed component. Int. J. Mech. Sci. 2024, 279, 109494. [Google Scholar] [CrossRef]
- Park, J.; Hwang, H. Preform design for precision forging of an asymmetric rib-web type component. J. Mater. Prcoessing Technol. 2007, 187–188, 595–599. [Google Scholar] [CrossRef]
- Shan, D.; Xu, W.; Si, C.; Lu, Y. Research on local loading method for an aluminum-alloy hatch with cross ribs and thin webs. J. Mater. Prcoessing Technol. 2007, 187–188, 480–485. [Google Scholar] [CrossRef]
- Hua, L.; Tian, D.; Han, X.; Zhuang, W. Modelling and analysis of material flowing behaviors in constraint ring rolling of tapered ring with thin wall and three high ribs. Chin. J. Aeronaut. 2023, 36, 476–492. [Google Scholar] [CrossRef]
- Tian, D.; Han, X.; Hua, L.; Hu, X. An innovative constraining ring rolling process for manufacturing conical rings with thin sterna and high ribs. Chin. J. Aeronaut. 2022, 35, 324–329. [Google Scholar] [CrossRef]
- Feng, W.; Zhao, P. Buckling defect optimization of constrained ring rolling of thin-walled conical rings with inner high ribs combining response surface method with FEM. Metals 2024, 14, 378. [Google Scholar] [CrossRef]
- Tian, D.; Han, X.; Hua, L.; Wang, X.; Chen, F. Constraining ring rolling of thin-walled conical rings with transverse ribs. Int. J. Mech. Sci. 2022, 226, 107394. [Google Scholar] [CrossRef]
- Zheng, Y.; Liu, D.; Zhang, Z.; Yang, Y.; Ren, L. The flow line evolution of hot open ACDR process for titanium alloy discs. Arch. Civ. Mech. Eng. 2017, 17, 827–838. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, Z.; Xue, Y.; Zhang, X.; Wang, Q. Investigation on low hydrostatic stress extrusion technology for forming of large thin-walled components with high ribs. Int. J. Mach. Tools Manuf. 2024, 198, 104149. [Google Scholar] [CrossRef]
- Zhang, D.; Xu, H.; Xu, S.; Tong, F.; Chen, K.; Li, Z.; Zuo, J.; Shu, X. Material flow behavior and energy consumption model during the extrusion process of a 6063 aluminum alloy profile with complex cross-section. J. Mater. Res. Technol. 2024, 33, 9911–9925. [Google Scholar] [CrossRef]
- Yang, H.; Zhang, W.; Zhuang, X.; Zhao, Z. Experimental and modeling investigation on anisotropic plastic flow of metal plates under nonproportional loading conditions. Int. J. Solids Struct. 2025, 309, 113174. [Google Scholar] [CrossRef]
- Lin, P.; Guan, Y.; Kong, P.; Jiang, S.; Sun, D.; Guo, S.; Yan, B.; Feng, H.; Yang, L.; Zhang, Y. Transfer mechanisms of folding defect for thin-walled tube end flanges formed by flaring-upsetting hybrid process. J. Manuf. Process. 2024, 125, 321–336. [Google Scholar] [CrossRef]
- Meng, Y.; Yu, Z.; Zhao, Y. Folding defects mechanism of aluminum alloy thin-walled stiffened cylinders during flow forming. Thin-Walled Struct. 2024, 30, 112018. [Google Scholar] [CrossRef]
- Gao, P.; Yang, H.; Fan, X.; Lei, P.; Meng, M. Prediction of folding defect in transitional region during local loading forming of Titanium alloy large-scale rib-web component. Procedia Eng. 2014, 81, 528–533. [Google Scholar] [CrossRef]
- Gao, P.; Fei, M.; Fan, X.; Wang, S.; Li, Y.; Xing, L.; Wei, K.; Zhan, M.; Zhou, T.; Keyim, Z. Prediction of the folding defect in die forging: A versatile approach for three typical types of folding defects. J. Manuf. Process. 2019, 39, 181–191. [Google Scholar] [CrossRef]
- Gao, P.; Yang, H.; Fan, X.; Lei, P. Forming defects control in transitional region during isothermal local loading of Ti-alloy rib-web component. Int. J. Adv. Manuf. Technol. 2015, 76, 857–868. [Google Scholar] [CrossRef]
- Gao, P.; Li, X.; Yang, H.; Fan, X.; Lei, Z. Improving the process forming limit considering forming defects in the transitional region in local loading forming of Ti-alloy rib-web components. Chin. J. Aeronaut. 2017, 30, 1270–1280. [Google Scholar] [CrossRef]
- Xia, Q.; Long, J.; Xiao, G.; Yuan, S.; Qin, Y. Deformation mechanism of ZK61 magnesium alloy cylindrical parts with longitudinal inner ribs during hot backward flow forming. J. Mater. Process. Technol. 2021, 296, 117197. [Google Scholar] [CrossRef]
- Zhu, X.; Liu, D.; Yang, Y.; Hu, Y.; Liu, G.; Wang, Y. Effects of blank dimension on forming characteristics during conical-section ring rolling of Inco718 alloy. Int. J. Adv. Manuf. Technol. 2016, 84, 2707–2718. [Google Scholar] [CrossRef]
- Deng, J.; Mao, H. A blank optimization design method for three-roll cross rolling of complex-groove and small-hole ring. Int. J. Mech. Sci. 2015, 93, 218–228. [Google Scholar] [CrossRef]
- Zhuang, W.; Hua, L.; Han, X.; Zheng, F. Design and hot forging manufacturing of non-circular spur bevel gear. Int. J. Mech. Sci. 2017, 133, 129–146. [Google Scholar] [CrossRef]
- Zhou, W.; Lin, J.; Dean, T.; Wang, L. Analysis and modelling of a novel process for extruding curved metal alloy profiles. Int. J. Mech. Sci. 2018, 138–139, 524–536. [Google Scholar] [CrossRef]
- Zhou, W.; Lin, J.; Dean, T.; Wang, L. Feasibility stumolds of a novel extrusion process for curved profiles: Experimentation and modelling. Int. J. Mach. Tools Manuf. 2018, 126, 27–43. [Google Scholar] [CrossRef]
- Zhou, W.; Yu, J.; Lin, J.; Dean, T. Manufacturing a curved profile with fine grains and high strength by differential velocity sideways extrusion. Int. J. Mach. Tools Manuf. 2019, 140, 77–88. [Google Scholar] [CrossRef]
- Tian, D.; Han, X.; Hua, L.; Huang, B.; Yang, S. A novel process for axial closed extrusion of ring part with mesh-like ribs. Int. J. Mech. Sci. 2020, 165, 105186. [Google Scholar] [CrossRef]
- Zong, Y.; Chen, L.; Zhao, Z.; Shan, D. Flow lines, microstructure, and mechanical properties of flow control formed 4032 aluminum alloy. Mater. Manuf. Process. 2014, 29, 466–471. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Diameter of conical loading surface of mandrel at the large part | 180 mm |
| Axial height of the conical loading surface of mandrel | 150 mm |
| Width of cavity on the conical loading surface of mandrel | 20 mm |
| Distance from the cavity on the conical loading surface to the upper surface of the conical loading surface of mandrel | 41 mm |
| Conical angle of the conical loading surface of mandrel | 7.2° |
| Inner diameter of lower plate | 190 mm |
| Inner diameter of upper plate | 146 mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, D.; Han, X.; Lu, Z.; Zhuang, W.; Zhang, Z.; Deng, Z.; Hua, L. Material Flow Control and Process Design in Constraint Ring Rolling of Thin-Walled Conical Cylinders with Three Ring Ribs. Materials 2025, 18, 1262. https://doi.org/10.3390/ma18061262
Tian D, Han X, Lu Z, Zhuang W, Zhang Z, Deng Z, Hua L. Material Flow Control and Process Design in Constraint Ring Rolling of Thin-Walled Conical Cylinders with Three Ring Ribs. Materials. 2025; 18(6):1262. https://doi.org/10.3390/ma18061262
Chicago/Turabian StyleTian, Duanyang, Xinghui Han, Zhuwei Lu, Wuhao Zhuang, Zhaosen Zhang, Zushen Deng, and Lin Hua. 2025. "Material Flow Control and Process Design in Constraint Ring Rolling of Thin-Walled Conical Cylinders with Three Ring Ribs" Materials 18, no. 6: 1262. https://doi.org/10.3390/ma18061262
APA StyleTian, D., Han, X., Lu, Z., Zhuang, W., Zhang, Z., Deng, Z., & Hua, L. (2025). Material Flow Control and Process Design in Constraint Ring Rolling of Thin-Walled Conical Cylinders with Three Ring Ribs. Materials, 18(6), 1262. https://doi.org/10.3390/ma18061262







