Investigation of the Noise Emitted from Elevated Urban Rail Transit Paved with Various Resilient Tracks
Abstract
1. Introduction
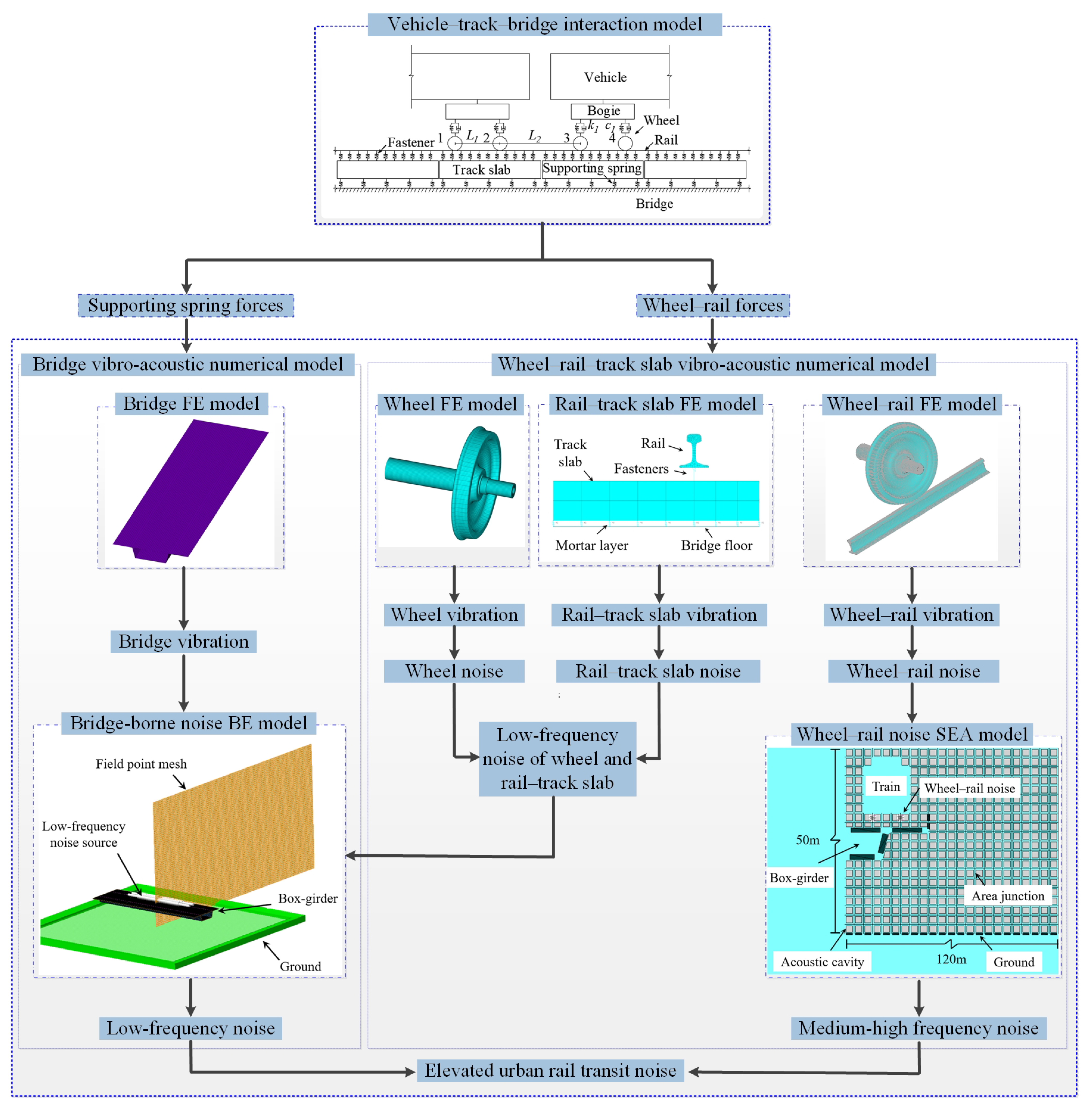
2. Methodology for the Noise Prediction of Elevated Urban Rail Transit
2.1. Vehicle–Track–Bridge Interaction Model
2.2. Prediction Model for the Structure-Borne Noise of the Box-Girder
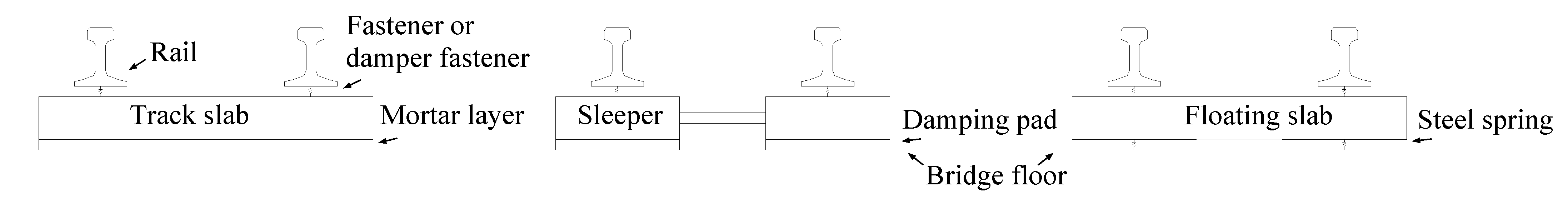
2.3. Prediction Model for the Low-Frequency Noise of the Wheel–Rail–Track Slab
2.4. Prediction Model for the Medium–High Frequency Wheel–Rail Noise
3. Validation of the Noise Prediction Model
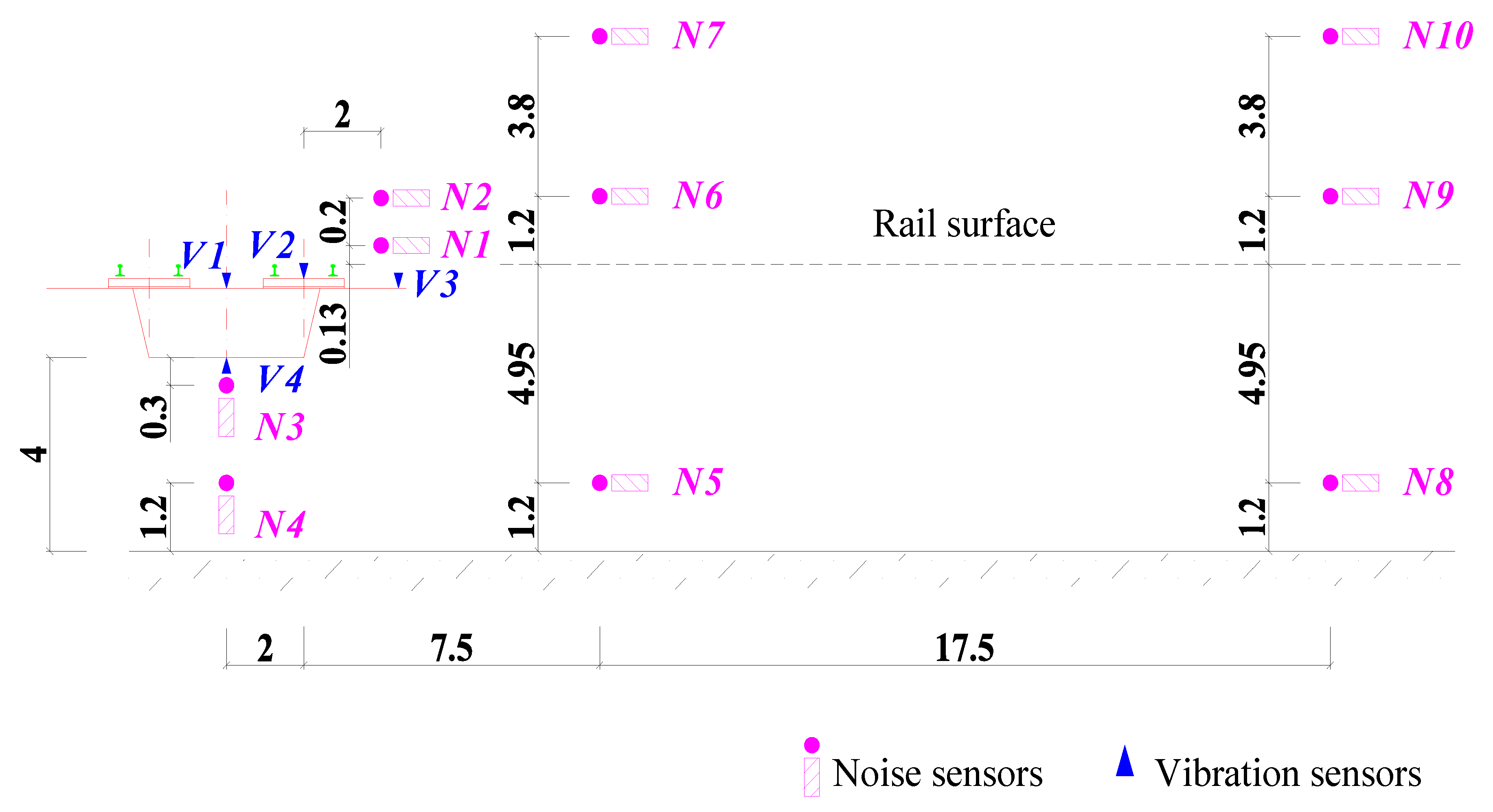
3.1. Measurement Overview
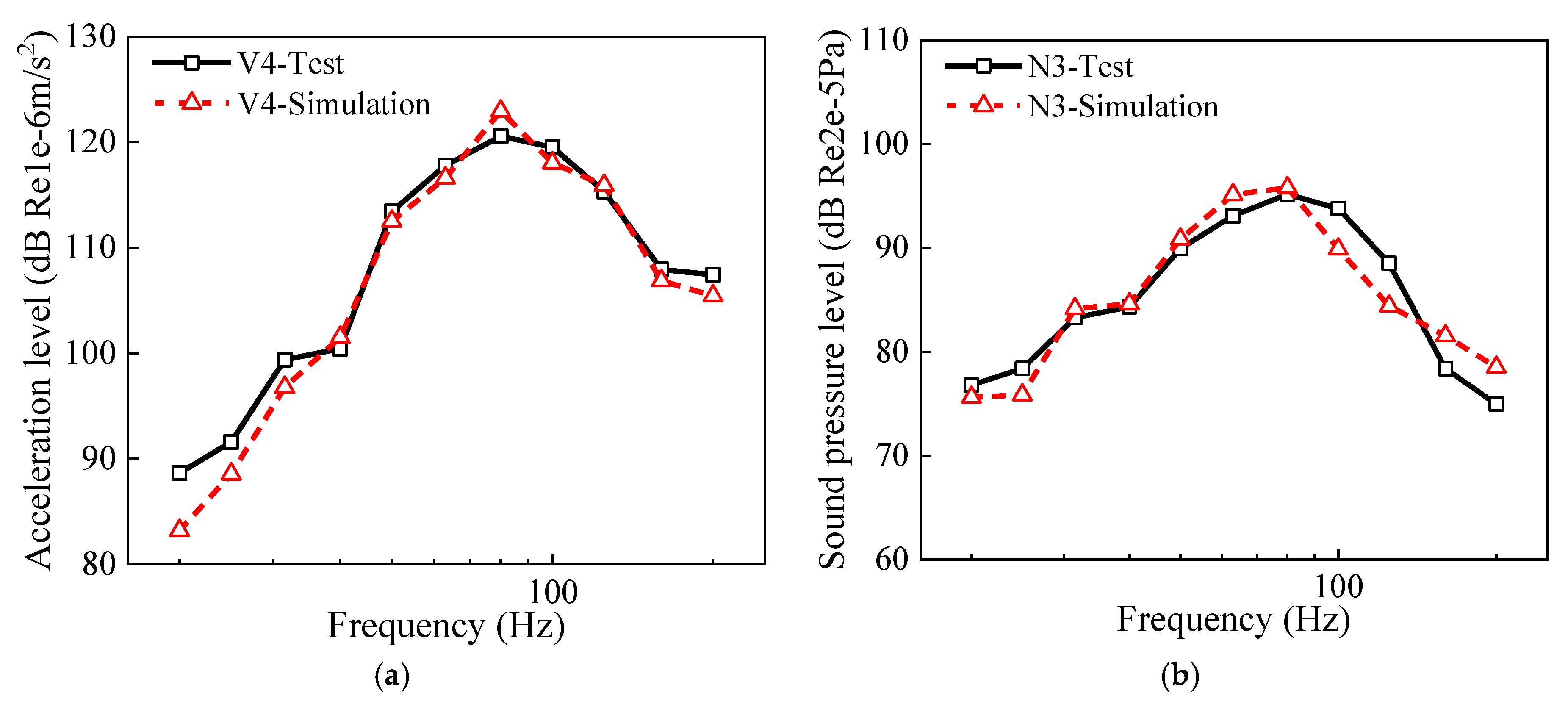
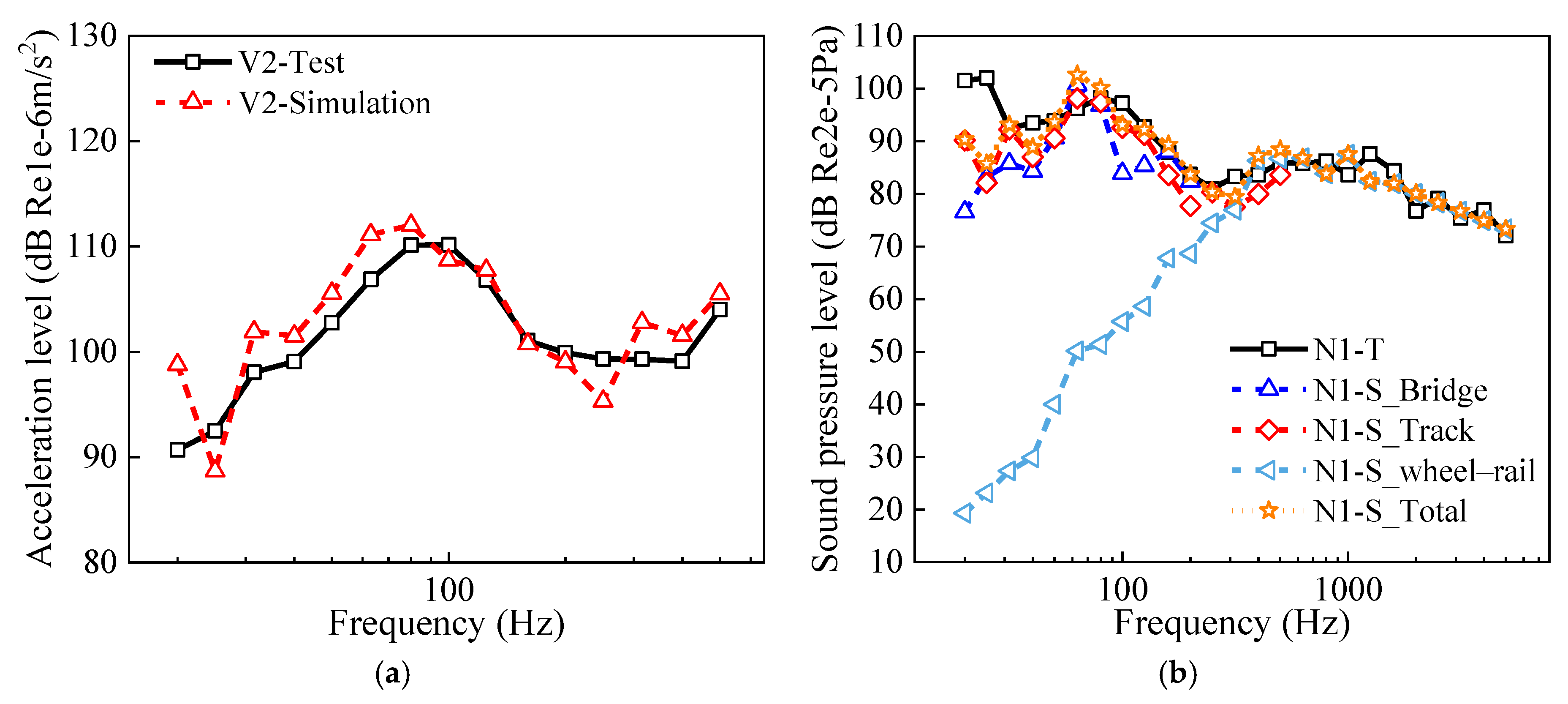
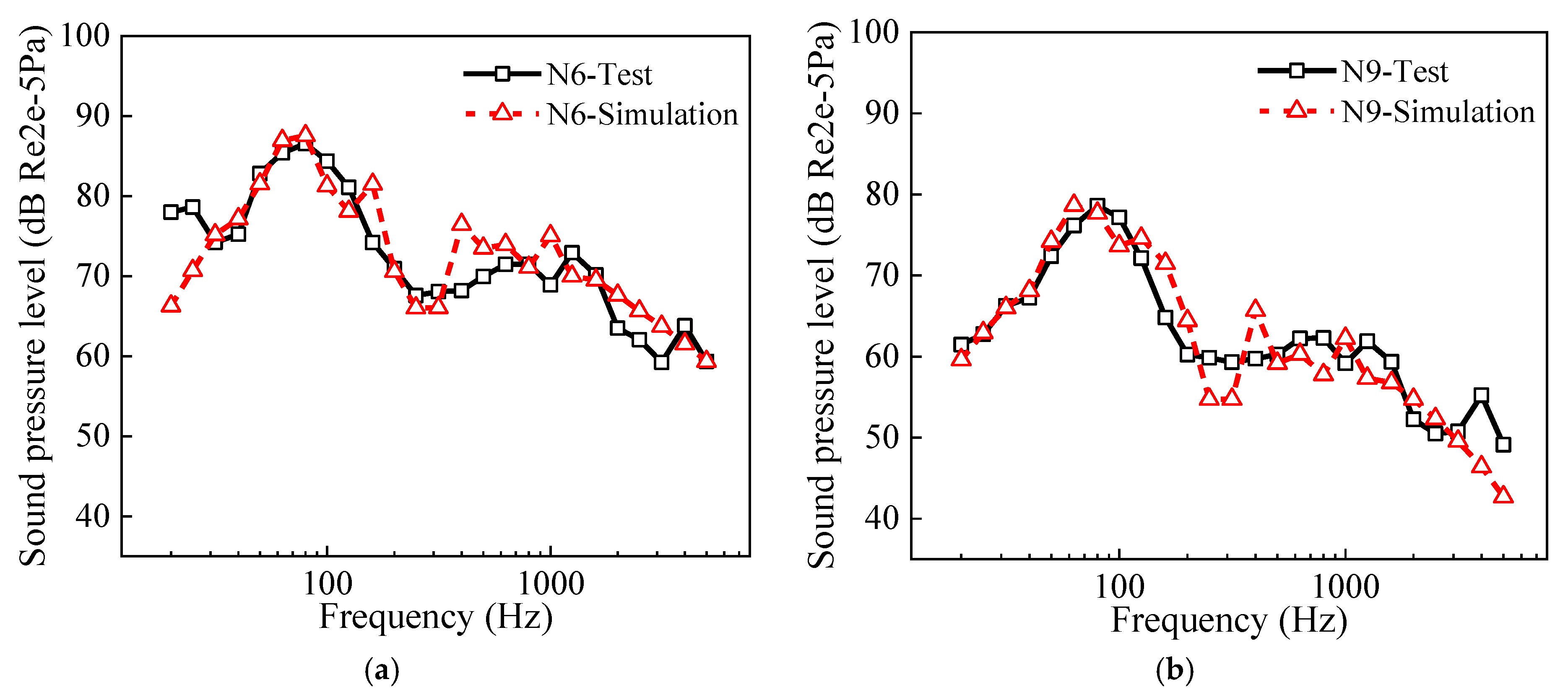
3.2. Verification of the Noise Prediction Model
4. Comparison of Noise Levels of a Viaduct with Various Track Forms
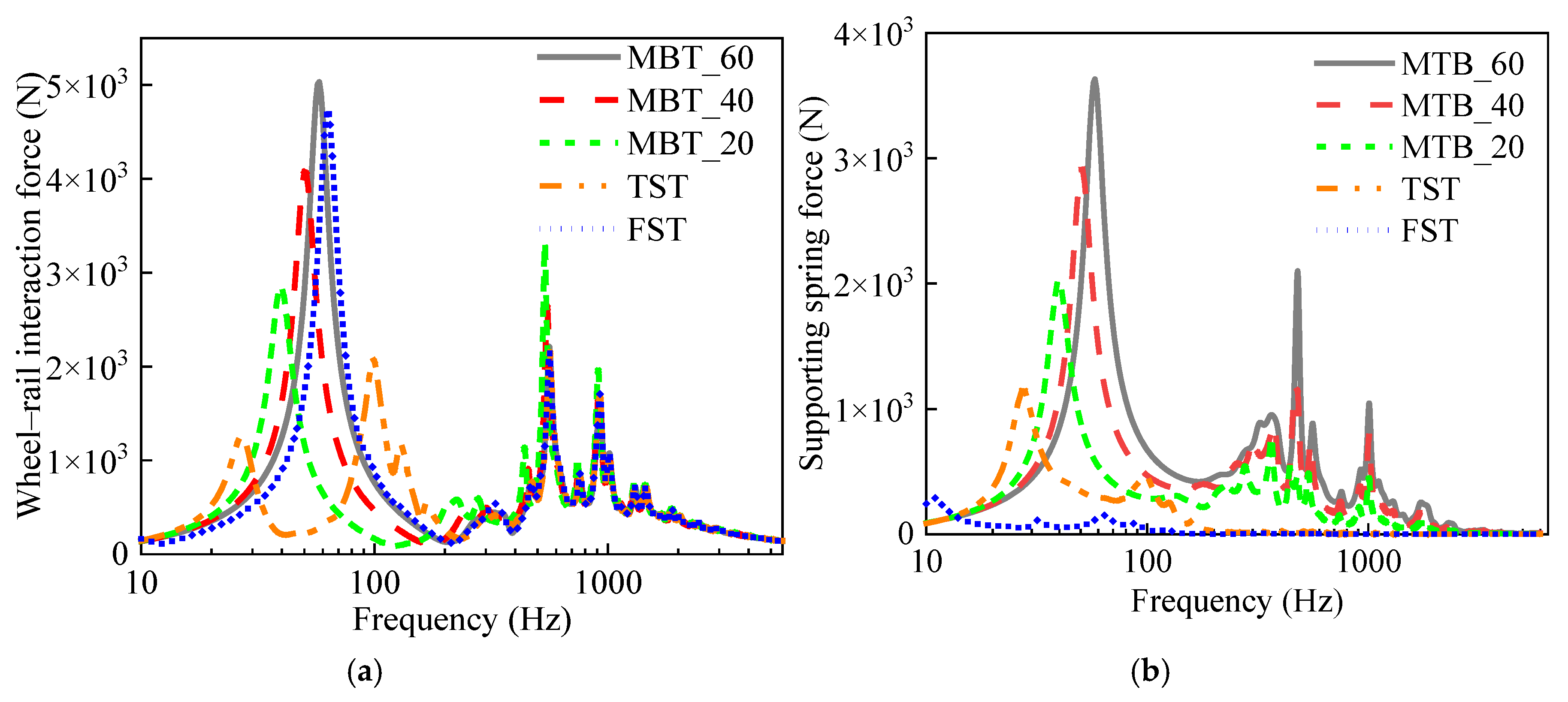
4.1. Variations in Forces
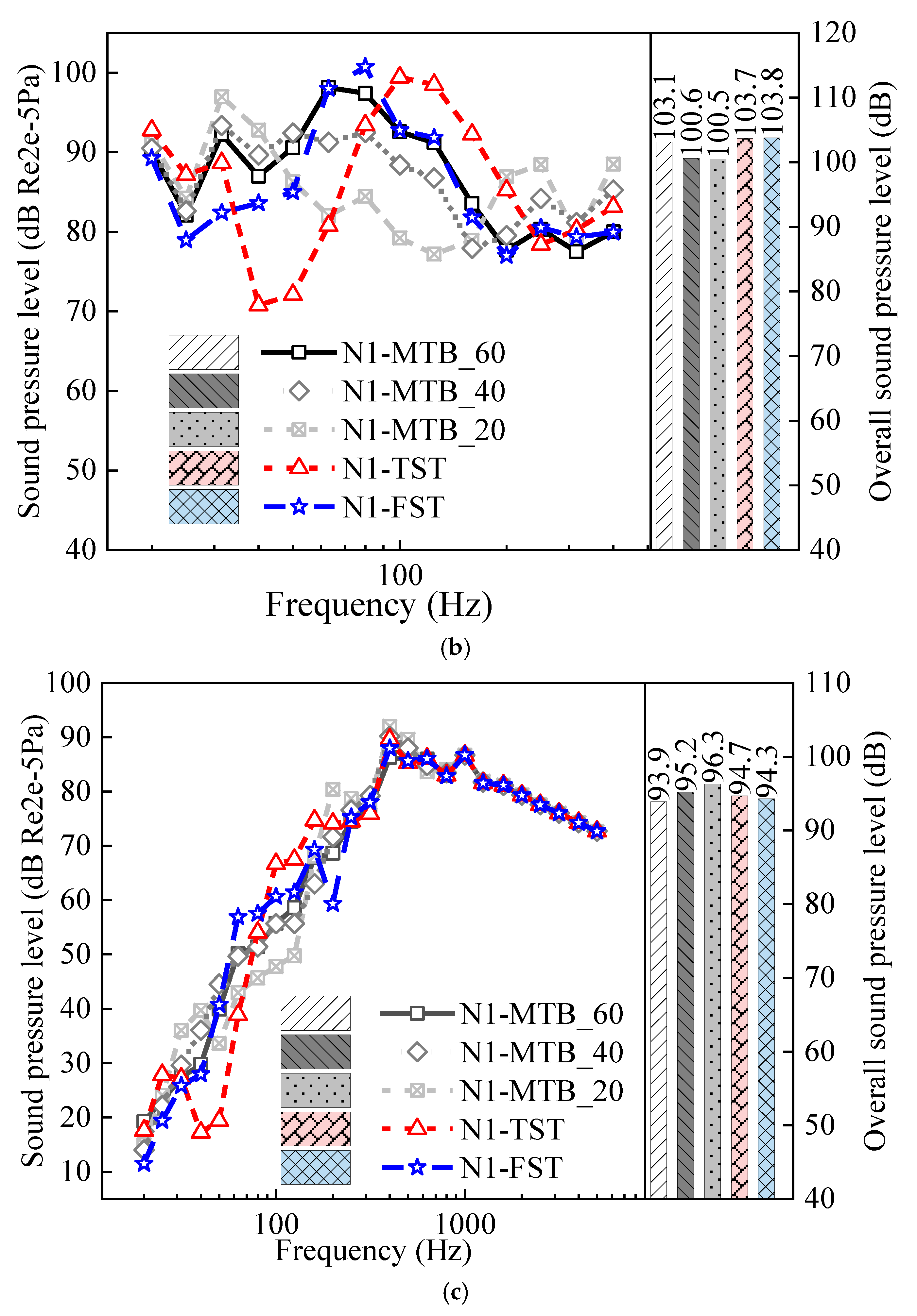
4.2. Variations in Sound Pressure Levels
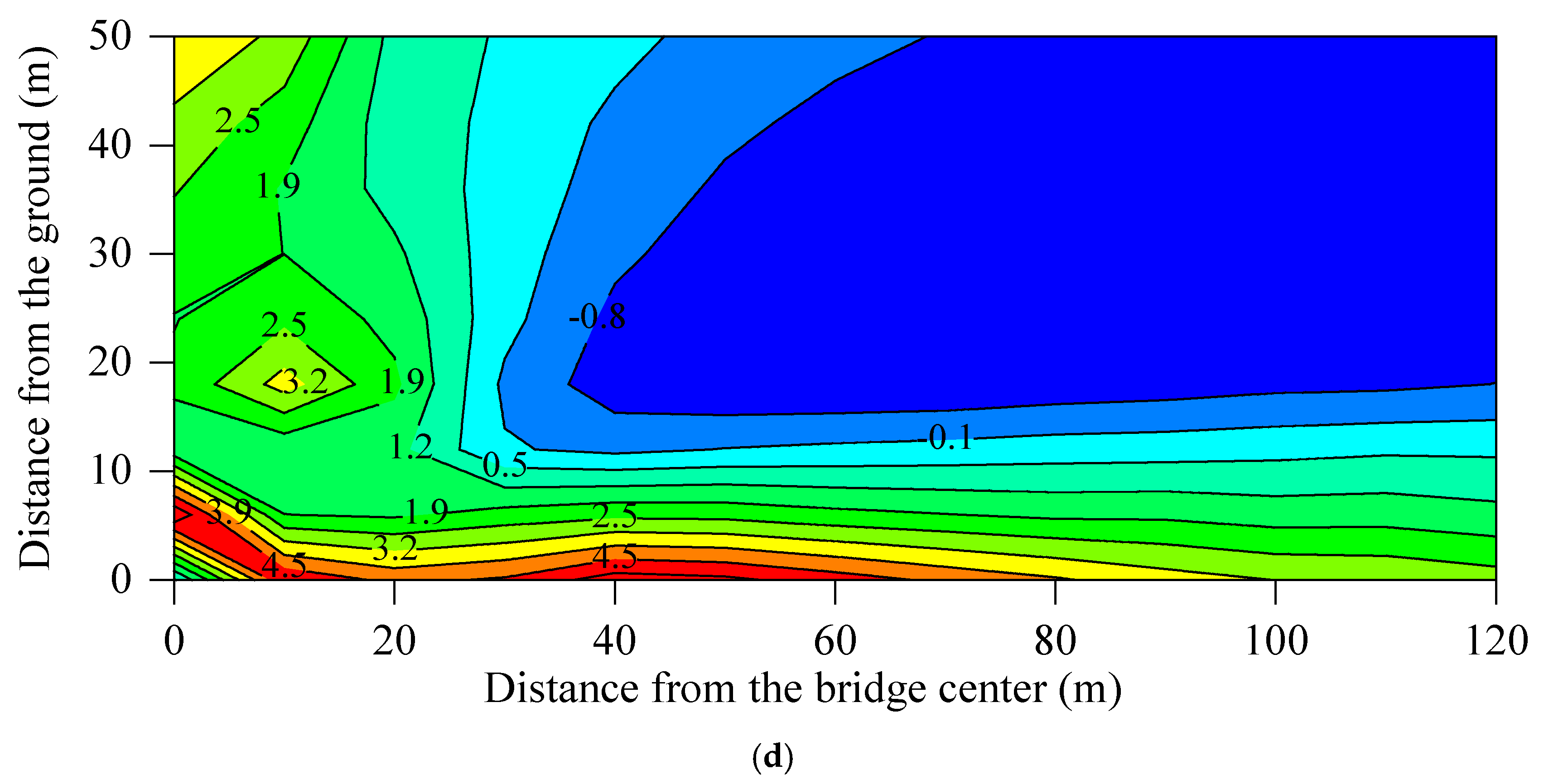
4.3. Variations in the Sound Pressure Levels of the Total Noise
5. Conclusions
- (1)
- The elastic components of the resilient track exert significant influence on the box-girder structure-borne noise. Variations in track configurations alter the amplitude and spectral characteristics of forces transmitted from the wheel–rail interface to the bridge, thereby modifying the distribution of structure-borne noise. The noise reduction effects on bridge structure-borne noise, ranked from largest to smallest, are FST, TST, MTB_20, and MTB_40. As the stiffness of the fastener decreases, the bridge structure-borne noise slightly increases in the frequency band below 50 Hz.
- (2)
- As an effective vibration reduction measure installed beneath the rail, the resilient fastener can mitigate noise radiated from the track slab by approximately 2.5 dB. In contrast, the radiated noise from the track slab of the TST and FST is approximately 0.6 dB higher than that from MTB_60 due to the increasing peak value of wheel–rail forces in the low-frequency range.
- (3)
- The alteration in track structure primarily influences wheel–rail noise below 1000 Hz, whereas it does not significantly change the wheel–rail noise produced above 1000 Hz. Specifically, the application of resilient fasteners results in an increase of 1.3 dB and 2.4 dB in wheel–rail noise. Additionally, the sound pressure levels of the wheel–rail noise for the TST and FST are 0.8 dB and 0.4 dB higher than that of MTB_60.
- (4)
- The structure-borne noise from the box-girder and the track slab are significant contributors to the total noise of viaducts with MTB_60. As the stiffness of the fasteners decreases, the overall sound pressure level of the track slab’s noise radiation increases. This suggests that the stiffness of the fasteners should be optimally matched with other components to achieve the maximum reduction in comprehensive noise.
- (5)
- The slab noise radiation from the TST and FST is higher than the structure-borne noise and wheel–rail noise. The total noise from viaducts paved with FSTs exceeds that of the MTB_60 in the upper far field. This suggests that installing sound barriers on the box-girder is necessary to mitigate radiated noise from the track slab. Noise reduction for elevated urban rail transit requires the integration and coordination of multiple measures to achieve maximum noise mitigation outcomes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, Q.M.; Gao, K.; Song, L.Z.; Liu, L.Y.; Luo, Y.K. Investigation on multiple traffic noise near an airport and their effect on nearby residents. Sci. Rep. 2024, 14, 29680. [Google Scholar] [CrossRef] [PubMed]
- Thompson, D.J. Wheel-rail noise generation Ⅰ: Introduction and interaction model. J. Sound Vib. 1993, 161, 387–400. [Google Scholar] [CrossRef]
- Song, L.Z.; Gao, K.; Liu, Q.M.; Zhang, L.T.; Feng, Q.S.; Guo, W.J. Acoustic performance of near-rail low-height noise barriers installed on suburban railway bridges. Environ. Sci. Pollut. R. 2022, 29, 62330–62346. [Google Scholar] [CrossRef] [PubMed]
- Saurenman, H.; Phillips, J. In-service tests of the effectiveness of vibration control measures on the BART rail transit system. J. Sound Vib. 2005, 293, 888–900. [Google Scholar] [CrossRef]
- Gao, X.G.; Yan, H.D.; Feng, Q.S.; Ma, Y.F.; Du, M.J. Performance research and effect evaluation of a high-grade damping fastener in subway line. Eng. Fail. Anal. 2025, 168, 109098. [Google Scholar] [CrossRef]
- Song, X.D.; Li, Q. Numerical and experimental study on noise reduction of concrete LRT bridges. Sci. Total Environ. 2018, 643, 208–224. [Google Scholar] [CrossRef]
- Sadeghi, J.; Seyedkazemi, M.; Khajehdezfuly, A. Nonlinear simulation of vertical behavior of railway fastening system. Eng. Struct. 2020, 209, 110340. [Google Scholar] [CrossRef]
- Li, X.Z.; Liang, L.; Wang, D.X. Vibration and noise characteristics of an elevated box girder paved with different track structures. J. Sound Vib. 2018, 425, 21–40. [Google Scholar] [CrossRef]
- Yan, B.; Pan, L.; Xu, L.; Deng, X.Y.; Liu, W.; Du, X.G. Dynamic performance analysis of floating slab track system considering flexible wheelset. Constr. Build. Mater. 2023, 393, 132074. [Google Scholar]
- Liang, L.; Li, X.Z.; Yin, J.; Wang, D.X.; Gao, W.; Guo, Z. Vibration characteristics of damping pad floating slab on the long-span steel truss cable-stayed bridge in urban rail transit. Eng. Struct. 2019, 191, 92–103. [Google Scholar] [CrossRef]
- Liang, L.; Li, X.Z.; Zheng, J.; Lei, K.N.; Gou, H.Y. Structure-borne noise from long-span steel truss cable-stayed bridge under damping pad floating slab: Experimental and numerical analysis. Appl. Acoust. 2020, 157, 106988. [Google Scholar] [CrossRef]
- Liu, X.Z.; Wang, Y.; Yin, Z.R.; Gao, T.C.; Luo, Q. In-situ measurement of subway train-induced vibration and noise of steel spring floating slab with MEMS-based sensing units. Meas. Sci. Technol. 2024, 35, 085112. [Google Scholar] [CrossRef]
- Zhao, Z.M.; Wei, K.; Ding, W.H.; Du, W.; Li, H.L. UM-SIMULINK Co-simulation for the vibration reduction optimization of a magnetorheological damping steel-spring floating slab track. Constr. Build. Mater. 2021, 307, 124923. [Google Scholar] [CrossRef]
- Huang, X.D.; Zeng, Z.P.; Wang, D.; Luo, X.W.; Li, P.; Wang, W.D. Experimental study on the vibration reduction characteristics of the floating slab track for 160 km/h urban rail transit. Structures 2023, 51, 1230–1244. [Google Scholar] [CrossRef]
- He, W.; Zou, C.; Pang, Y.T.; Wang, X.M. Environmental noise and vibration characteristics of rubber-spring floating slab track. Environ. Sci. Pollut. R. 2020, 28, 13671–13689. [Google Scholar] [CrossRef] [PubMed]
- Song, L.Z.; Gao, K.; Liu, Q.M.; Liu, L.Y.; Feng, Q.S. Study on the structure-borne noise of U-shaped girder bridges with fully-enclosed sound barriers. Appl. Acoust. 2023, 211, 109497. [Google Scholar] [CrossRef]
- Li, X.Z.; Hu, X.H.; Zheng, J. Statistical energy method for noise reduction performance of the vertical noise barrier alongside railway bridges. Appl. Acoust. 2020, 170, 107503. [Google Scholar] [CrossRef]
- Zhang, Y.F.; Li, L.; Lei, Z.Y.; Yu, L.B.; Bu, Z. Environmental noise beside an elevated box girder bridge for urban rail transit. J. Zhejiang Univ. Sci. A. 2021, 22, 53–69. [Google Scholar] [CrossRef]
- Thompson, D.J. Railway Noise and Vibration: Mechanisms, Modelling and Means of Control; Elsevier: Oxford, UK, 2009. [Google Scholar]
- Li, X.Z.; Zhang, X.; Zhang, Z.J.; Liu, Q.M.; Li, Y.D. Experimental research on noise emanating from concrete box-girder bridges on intercity railway lines. Proc. Inst. Mech. Eng. F J. Rail Rapid Transit 2015, 229, 125–135. [Google Scholar] [CrossRef]
- Zhang, X.; Li, X.Z.; Song, L.Z.; Su, B.; Li, Y.D. Vibrational and acoustical performance of concrete box-section bridges subjected to train wheel-rail excitation: Field test and numerical analysis. Noise Control Eng. J. 2016, 64, 217–229. [Google Scholar] [CrossRef]
- Song, L.Z.; Li, X.Z.; Hao, H.; Zhang, X. Medium- and high-frequency vibration characteristics of a box-girder by the waveguide finite element method. Int. J. Struct. Stab. Dyn. 2018, 18, 1850141. [Google Scholar] [CrossRef]
- Thompson, D.J. Wheel-rail noise generation, Part V: Inclusion of wheel rotation. J. Sound Vib. 1993, 161, 467–482. [Google Scholar] [CrossRef]
- Thompson, D.J.; Hemsworth, B.; Vincent, N. Experimental validation of the TWINS prediction program for rolling noise, Part Ⅰ: Description of the model and method. J. Sound Vib. 1996, 193, 123–135. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Squicciarini, G.; Thompson, D.J. Sound radiation of a railway rail inclose proximity to the ground. J. Sound Vib. 2016, 362, 111–124. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Thompson, D.J.; Squicciarini, G. Sound radiation from railway sleepers. J. Sound Vib. 2016, 369, 178–194. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Thompson, D.J.; Jeong, H.; Squicciarini, G. The effects of ballast on the sound radiation from railway track. J. Sound Vib. 2017, 399, 137–150. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Jeong, H.; Thompson, D.J.; Squicciarini, G. The noise radiated by ballasted and slab tracks. Appl. Acoust. 2019, 151, 193–205. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Thompson, D.J.; Ryue, J.; Jeong, H.; Squicciarini, G. The effect of rail shields on railway rolling noise. Int. J. Rail Transp. 2023, 11, 552–572. [Google Scholar] [CrossRef]
- Thompson, D.J.; Jones, C.J.C. A review of the modelling of wheel/rail noise generation. J. Sound Vib. 2000, 231, 519–536. [Google Scholar] [CrossRef]
- He, Y.P.; Zhou, Q.; Xu, F.; Sheng, X.Z.; He, Y.L.; Han, J. An investigation into the effect of rubber design parameters of a resilient wheel on wheel-rail noise. Appl. Acoust. 2023, 205, 109259. [Google Scholar] [CrossRef]
- Song, L.Z.; Zhang, J.; Liu, Q.M.; Zhang, L.T.; Wu, X.L. Characteristics of noise caused by trains passing on urban rail transit viaducts. Sustainability 2024, 17, 94. [Google Scholar] [CrossRef]
- Brebbia, A.C. The birth of the boundary element method from conception to application. Eng. Anal. Bound. Elem. 2017, 77, iii-x. [Google Scholar] [CrossRef]
- Zhang, X.A.; Zhai, W.M.; Chen, Z.W.; Yang, J.J. Characteristic and mechanism of structural acoustic radiation for box girder bridge in urban rail transit. Sci. Total Environ. 2018, 627, 1303–1314. [Google Scholar] [CrossRef]
- Lyon, R.H. Statistical Energy Analysis of Dynamical Systems: Theory and Applications; The MIT Press: Cambridge, MA, USA, 1975. [Google Scholar]
- Liu, Q.M.; Thompson, D.J.; Xu, P.P.; Feng, Q.S.; Li, X.Z. Investigation of train-induced vibration and noise from a steel-concrete composite railway bridge using a hybrid finite element-statistical energy analysis method. J. Sound Vib. 2020, 471, 115197. [Google Scholar] [CrossRef]
- Liu, Q.M.; Liu, L.Y.; Chen, H.P.; Zhou, Y.L.; Lei, X.Y. Prediction of vibration and noise from steel/composite bridges based on receptance and statistical energy analysis. Steel Compos. Struct. 2020, 37, 291–306. [Google Scholar]
- Harrison, M.F.; Thompson, D.J.; Jones, C.J.C. The calculation of noise from railway viaducts and bridges. Proc. Inst. Mech. Eng. F J. Rail Rapid Transit 2000, 214, 125–134. [Google Scholar] [CrossRef]
- Sheng, X.Z.; Cheng, G.; Thompson, D.J. Modelling wheel/rail rolling noise for a high-speed train running along an infinitely long periodic slab track. J. Acoust. Soc. Am. 2020, 148, 174–190. [Google Scholar] [CrossRef]
- Yang, X.W.; Shi, G.T. The effect of slab track on wheel/rail rolling noise in high speed railway. Intell. Autom. Soft Comput. 2014, 20, 575–585. [Google Scholar] [CrossRef]
- Zhou, Q.; He, Y.P.; Li, M.X.; Liu, Z.; He, Y.L.; Sheng, X.Z. A parametric study on the structural noise radiation characteristics of a steel spring floating slab track. Adv. Mech. Eng. 2022, 14, 16878132221119921. [Google Scholar] [CrossRef]
- ISO 3095: 2013; Acoustic–Railway Applications–Measurement of Noise Emitted by Railbound Vehicles. International Organization for Standardization: Geneva, Switzerland, 2013.
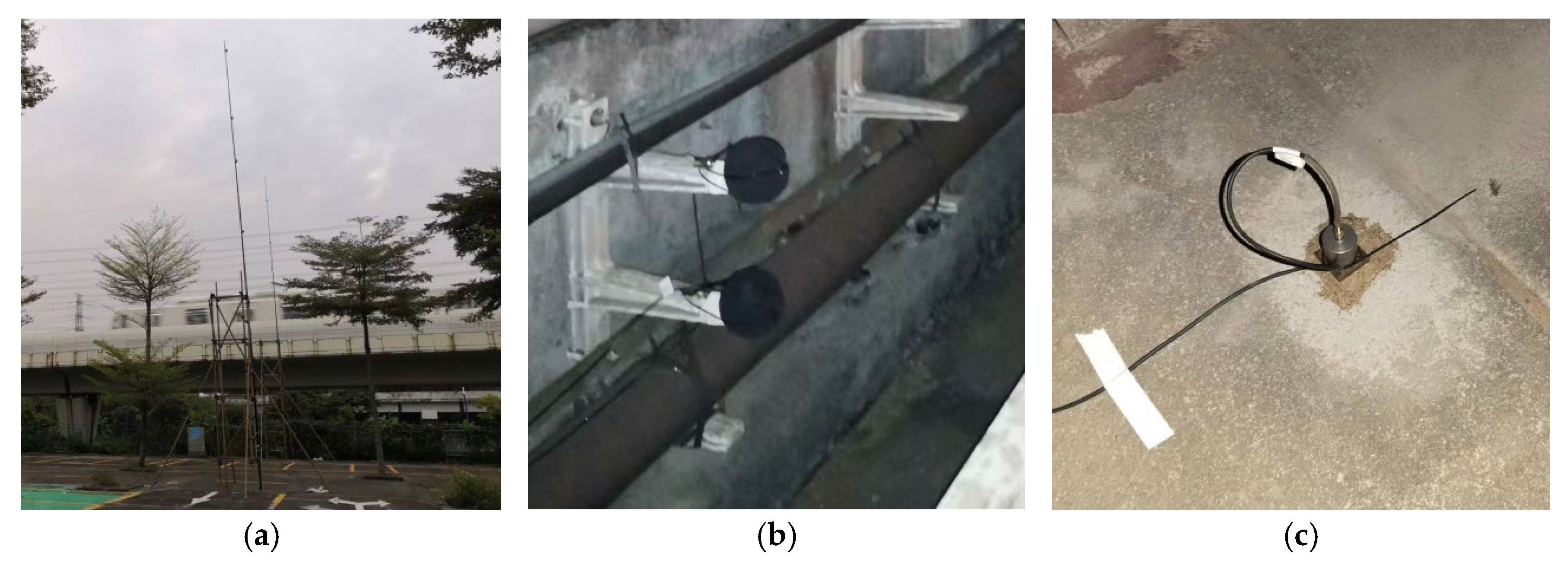



| Component | Parameter | Unit | Value | |
|---|---|---|---|---|
| Vehicle | Wheel | Young’s modulus | Pa | 2.06 × 1011 |
| Diameter | mm | 840 | ||
| Density | kg/m3 | 7850 | ||
| Poisson’s ratio | − | 0.3 | ||
| Mass of the wheelset | kg | 1744 | ||
| Unit length mass of the wheel tread | kg/m | 63.4 | ||
| Thickness of the wheel web | m | 0.025 | ||
| Wheelbase | mm | 2500 | ||
| Bogie | Length between the centers of the bogies | m | 15.7 | |
| Mass | kg | 2430 | ||
| Stiffness of the primary suspension | N/m | 1.25 × 106 | ||
| Damping of the primary suspension | N∙s/m | 10,000 | ||
| Track | Rail | Young’s modulus | Pa | 2.06 × 1011 |
| Section moment of inertia | m4 | 3.2 × 10−5 | ||
| Shear modulus | Pa | 7.7 × 1010 | ||
| Density | kg/m3 | 7850 | ||
| Cross area | mm2 | 7745 | ||
| Poisson’s ratio | − | 0.3 | ||
| Loss factor | − | 0.01 | ||
| Normal fastener | Stiffness | N/m | 6 × 107 | |
| Loss factor | − | 0.25 | ||
| Fastener spacing | m | 0.625 | ||
| Resilient fastener 1 | Stiffness | N/m | 2 × 107 | |
| Resilient fastener 2 | Stiffness | N/m | 4 × 107 | |
| Monolithic track bed | Young’s modulus | Pa | 3.45 × 1010 | |
| Density | kg/m3 | 2500 | ||
| Loss factor | − | 0.25 | ||
| Length, width, and height | m | 3.5 × 1.05 × 0.2 | ||
| Stiffness of the supporting spring | N/m | 6.89 × 1010 | ||
| Spacing of the supporting spring | m | 1.2 | ||
| Loss factor of the supporting spring | − | 0.25 | ||
| Trapezoidal sleeper track | Young’s modulus | Pa | 3.5 × 1010 | |
| Density | kg/m3 | 2500 | ||
| Loss factor | − | 0.02 | ||
| Length, width, and height | m | 7 × 0.48 × 0.2 | ||
| Stiffness of the supporting spring | N/m | 2 × 107 | ||
| Spacing of the supporting spring | m | 1.2 | ||
| Loss factor of the supporting spring | − | 0.25 | ||
| Floating slab track | Young’s modulus | Pa | 3.5 × 1010 | |
| Density | kg/m3 | 2500 | ||
| Loss factor | − | 0.02 | ||
| Length, width, and height | m | 3.5 × 2.1 × 0.3 | ||
| Stiffness of the supporting spring | N/m | 6 × 106 | ||
| Spacing of the supporting spring | m | 1.2 | ||
| Loss factor of the supporting spring | − | 0.25 | ||
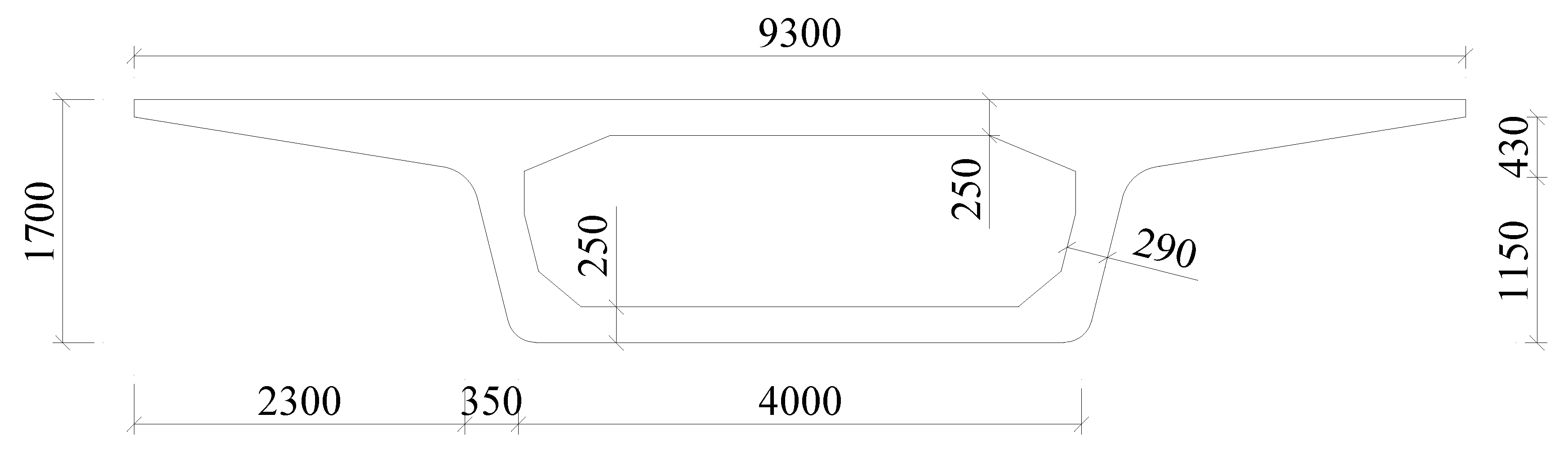
| Bridge | Main girder | Length | m | 30 |
| Height | m | 1.7 | ||
| Width of the deck | m | 9.3 | ||
| Width of the bottom | m | 4 | ||
| Young’s modulus | Pa | 3.45 × 1010 | ||
| Density | kg/m3 | 2500 | ||
| Loss factor | − | 0.02 | ||
| Poisson’s ratio | − | 0.2 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Gao, K.; Miao, Y.; Song, L.; Yue, S. Investigation of the Noise Emitted from Elevated Urban Rail Transit Paved with Various Resilient Tracks. Materials 2025, 18, 968. https://doi.org/10.3390/ma18050968
Liu Q, Gao K, Miao Y, Song L, Yue S. Investigation of the Noise Emitted from Elevated Urban Rail Transit Paved with Various Resilient Tracks. Materials. 2025; 18(5):968. https://doi.org/10.3390/ma18050968
Chicago/Turabian StyleLiu, Quanmin, Kui Gao, Yifei Miao, Lizhong Song, and Si Yue. 2025. "Investigation of the Noise Emitted from Elevated Urban Rail Transit Paved with Various Resilient Tracks" Materials 18, no. 5: 968. https://doi.org/10.3390/ma18050968
APA StyleLiu, Q., Gao, K., Miao, Y., Song, L., & Yue, S. (2025). Investigation of the Noise Emitted from Elevated Urban Rail Transit Paved with Various Resilient Tracks. Materials, 18(5), 968. https://doi.org/10.3390/ma18050968





