Ritz Solution of Vibration Analysis of Functionally Graded Porous Elliptic Shells and Panels Under Various Arbitrary Boundary Types
Abstract
1. Introduction
2. Theoretical Equations
2.1. Overview of the FGP Model
2.2. Modified Variational Formulation Model
2.3. Allowable Displacement Functions and Corresponding Motion Equations
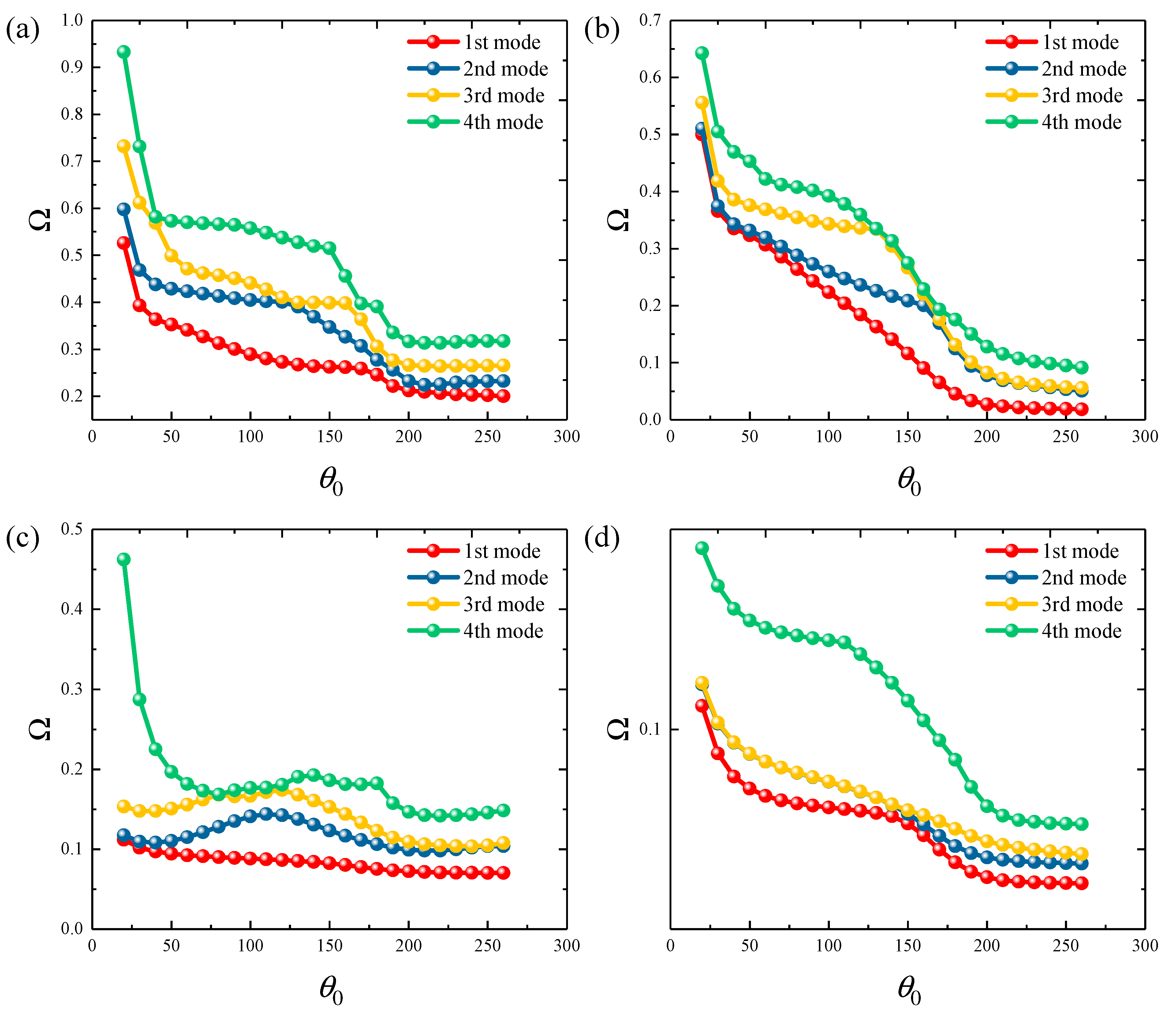
3. Computational Results and Analysis
3.1. Convergence and Validation
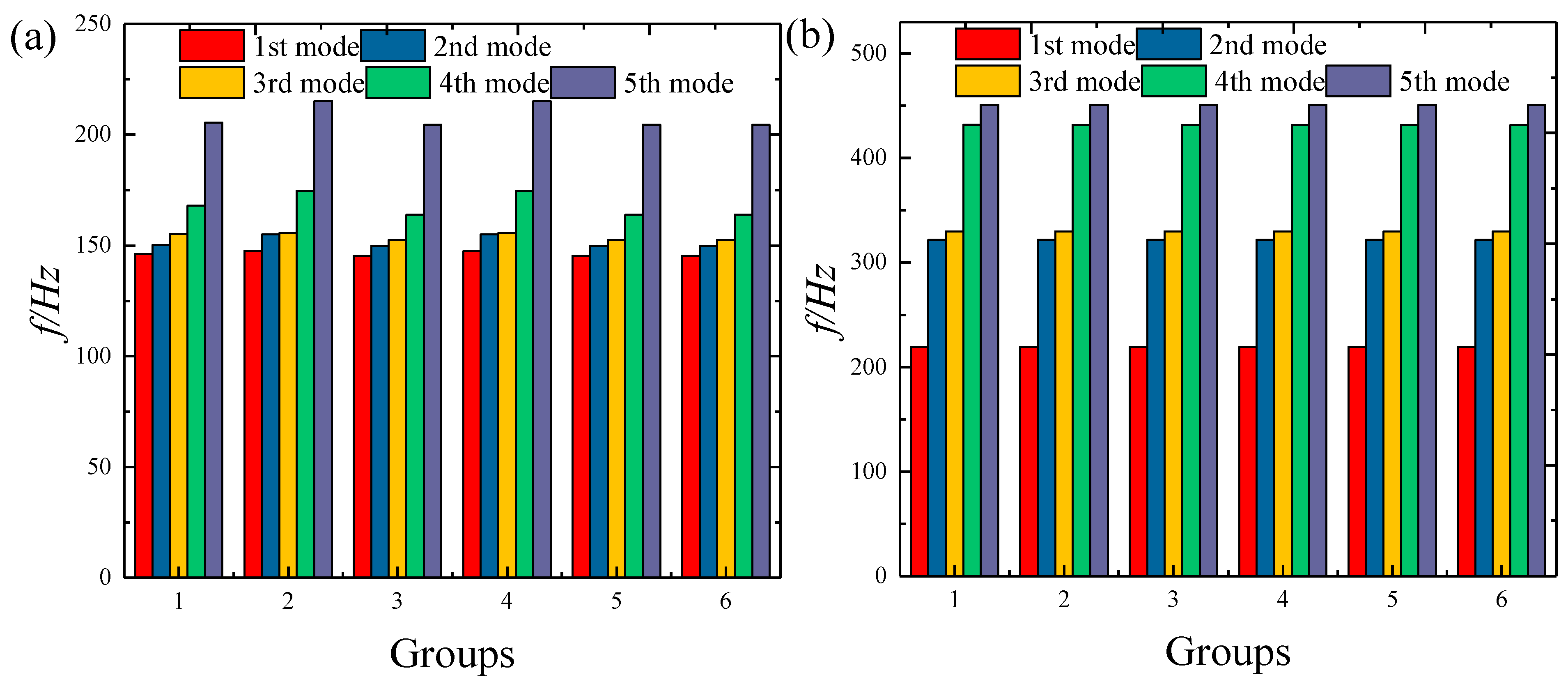
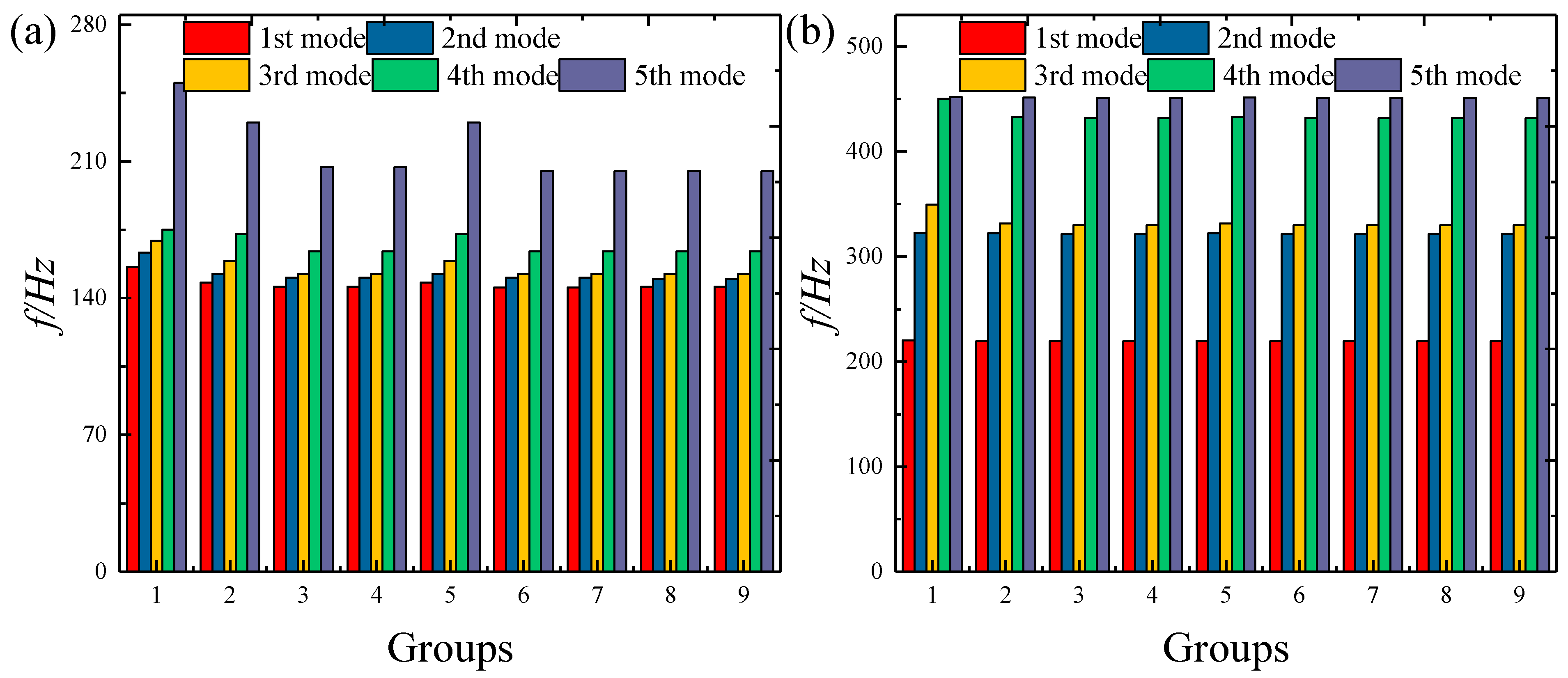
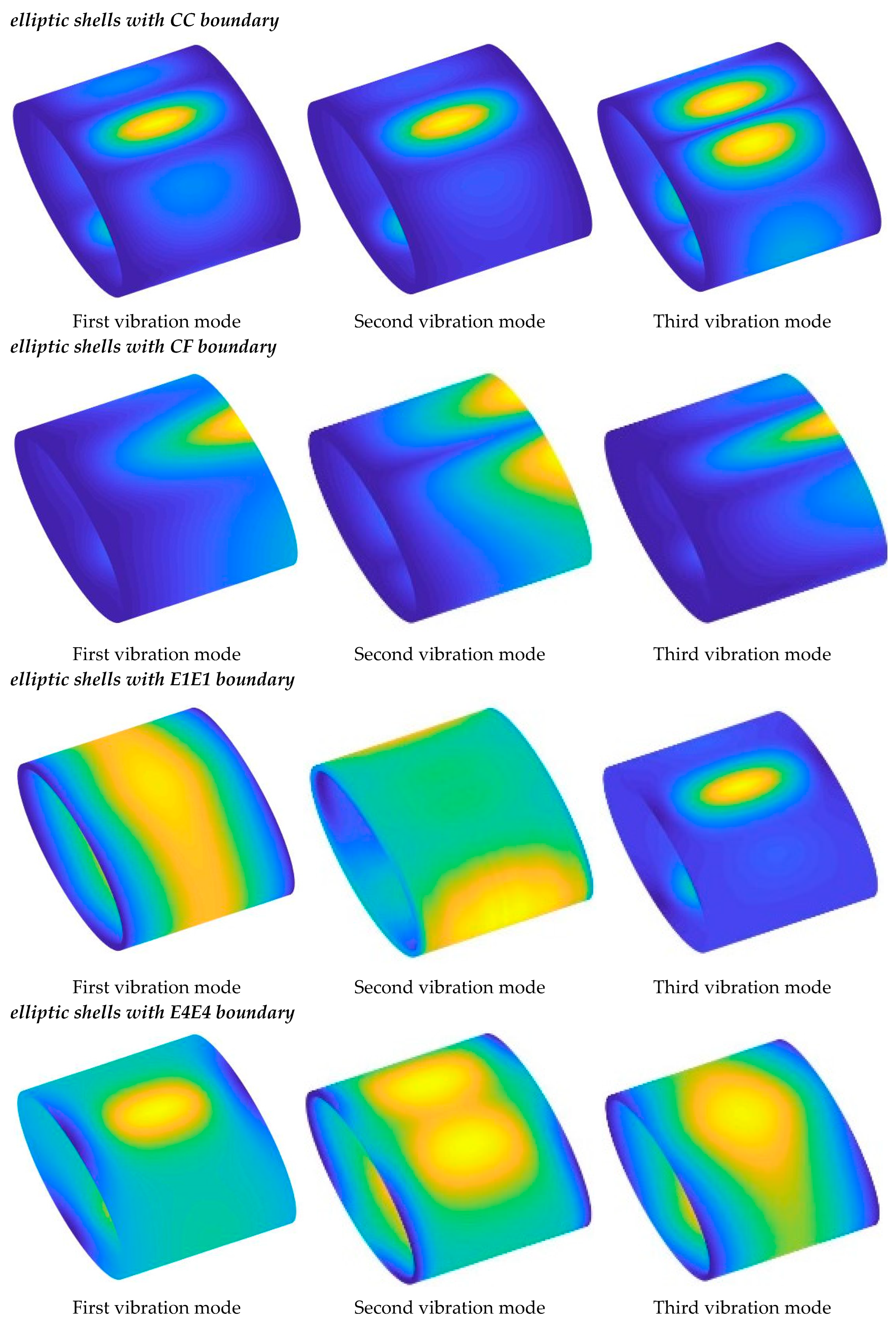
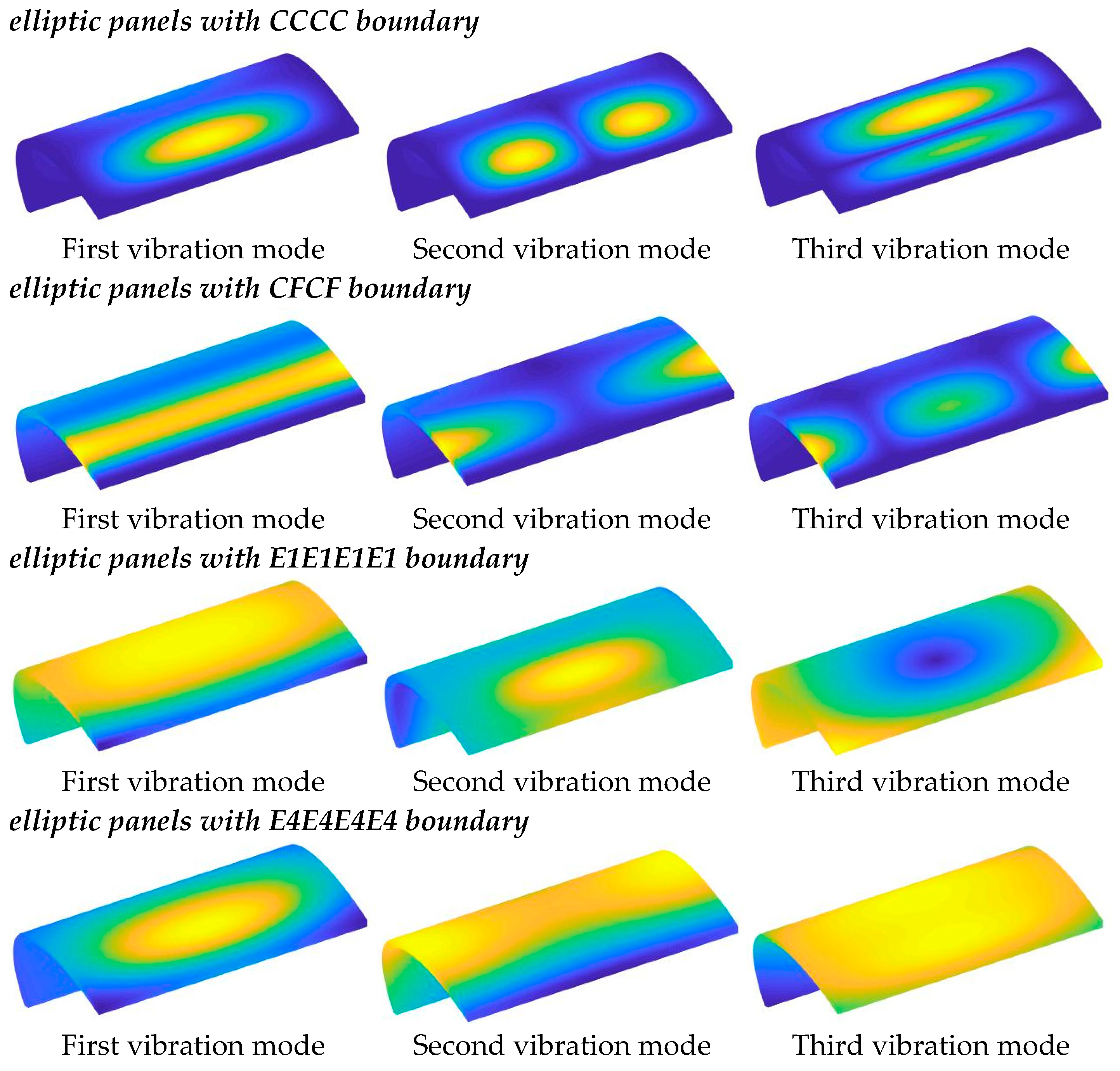
3.2. Numerical Analysis and Parameter Investigations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Melaibari, A.; Daikh, A.A.; Basha, M.; Wagih, A.; Othman, R.; Almitani, K.H.; Hamed, M.A.; Abdelrahman, A.; Eltaher, M.A. A Dynamic Analysis of Randomly Oriented Functionally Graded Carbon Nanotubes/Fiber-Reinforced Composite Laminated Shells with Different Geometries. Mathematics 2022, 10, 408. [Google Scholar] [CrossRef]
- Zhang, G.J.; Li, T.Y.; Zhu, X.; Yang, J.; Miao, Y.Y. Free and forced vibration characteristics of submerged finite elliptic cylindrical shell. Ocean Eng. 2017, 129, 92–106. [Google Scholar] [CrossRef]
- Wang, Y.; Arabnejad, S.; Tanzer, M.; Pasini, D. Hip implant design with three-dimensional porous architecture of optimized graded density. J. Mech. Des. 2018, 140, 111406. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Free and forced vibrations of shear deformable functionally graded porous beams. Int. J. Mech. Sci. 2016, 108–109, 14–22. [Google Scholar] [CrossRef]
- Filipich, C.P.; Piovan, M.T. The dynamics of thick curved beams constructed with functionally graded materials. Mech. Res. Commun. 2010, 37, 565–570. [Google Scholar] [CrossRef]
- Kitipornchai, S.; Chen, D.; Yang, J. Free vibration and elastic buckling of functionally graded porous beams reinforced by graphene platelets. Mater. Des. 2017, 116, 656–665. [Google Scholar] [CrossRef]
- Chen, D.; Yang, J.; Kitipornchai, S. Elastic buckling and static bending of shear deformable functionally graded porous beam. Compos. Struct. 2015, 133, 54–61. [Google Scholar] [CrossRef]
- Chen, D.; Kitipornchai, S.; Yang, J. Nonlinear free vibration of shear deformable sandwich beam with a functionally graded porous core. Thin-Walled Struct. 2016, 107, 39–48. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Zia, M. Large amplitude nonlinear vibration analysis of functionally graded Timoshenko beams with porosities. Acta Astronaut. 2015, 116, 117–125. [Google Scholar] [CrossRef]
- Mojahedin, A.; Jabbari, M.; Khorshidvand, A.R.; Eslami, M.R. Buckling analysis of functionally graded circular plates made of saturated porous materials based on higher order shear deformation theory. Thin-Walled Struct. 2016, 99, 83–90. [Google Scholar] [CrossRef]
- Rezaei, A.S.; Saidi, A.R.; Abrishamdari, M.; Mohammadi, M.H.P. Natural frequencies of functionally graded plates with porosities via a simple four variable plate theory: An analytical approach. Thin-Walled Struct. 2017, 120, 366–377. [Google Scholar] [CrossRef]
- Rezaei, A.; Saidi, A. Exact solution for free vibration of thick rectangular plates made of porous materials. Compos. Struct. 2015, 134, 1051–1060. [Google Scholar] [CrossRef]
- Rezaei, A.; Saidi, A. Buckling response of moderately thick fluid-infiltrated porous annular sector plates. Acta Mech. 2017, 228, 3929–3945. [Google Scholar] [CrossRef]
- Rezaei, A.; Saidi, A. An analytical study on the free vibration of moderately thick fluid-infiltrated porous annular sector plates. J. Vibrat. Control 2017, 24, 4130–4144. [Google Scholar] [CrossRef]
- Rezaei, A.S.; Saidi, A.R. On the effect of coupled solid-fluid deformation on natural frequencies of fluid saturated porous plates. Eur. J. Mech.-A/Solids 2017, 63, 99–109. [Google Scholar] [CrossRef]
- Zenkour, A.M. A quasi-3D refined theory for functionally graded single-layered and sandwich plates with porosities. Compos. Struct. 2018, 201, 38–48. [Google Scholar] [CrossRef]
- Guan, X.; Sok, K.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. A general vibration analysis of functionally graded porous structure elements of revolution with general elastic restraints. Compos. Struct. 2019, 209, 277–299. [Google Scholar] [CrossRef]
- Belica, T.; Magnucki, K. Stability of a porous-cellular cylindrical shell subjected to combined loads. J. Theor. Appl. Mech. 2013, 51, 927–936. [Google Scholar]
- Gao, K.; Gao, W.; Wu, B.; Wu, D.; Song, C. Nonlinear primary resonance of functionally graded porous cylindrical shells using the method of multiple scales. Thin-Walled Struct. 2018, 125, 281–293. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, D. Free vibration of functionally graded porous cylindrical shell using a sinusoidal shear deformation theory. Aerosp. Sci. Technol. 2017, 66, 83–91. [Google Scholar] [CrossRef]
- Zhao, J.; Xie, F.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. Vibration behavior of the functionally graded porous (FGP) doubly-curved panels and shells of revolution by using a semi-analytical method. Compos. Part B-Eng. 2019, 157, 219–238. [Google Scholar] [CrossRef]
- Zhao, J.; Xie, F.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. Dynamics analysis of functionally graded porous (FGP) circular, annular and sector plates with general elastic restraints. Compos. Part B-Eng. 2019, 159, 20–43. [Google Scholar] [CrossRef]
- Zhao, J.; Xie, F.; Wang, A.; Shuai, C.; Tang, J.; Wang, Q. A unified solution for the vibration analysis of functionally graded porous (FGP) shallow shells with general boundary conditions. Compos. Part B-Eng. 2019, 156, 406–424. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, Q.; Deng, X.; Choe, K.; Xie, F.; Shuai, C. A modified series solution for free vibration analyses of moderately thick functionally graded porous (FGP) deep curved and straight beams. Compos. Part B-Eng. 2019, 165, 155–166. [Google Scholar] [CrossRef]
- Zhao, J.; Choe, K.; Shuai, C.; Wang, A.; Wang, Q. Free vibration analysis of laminated composite elliptic cylinders with general boundary conditions. Compos. Part B-Eng. 2019, 158, 55–66. [Google Scholar] [CrossRef]
- Qu, Y.; Long, X.; Li, H.; Meng, G. A variational formulation for dynamic analysis of composite laminated beams based on a general higher-order shear deformation theory. Compos. Struct. 2013, 102, 175–192. [Google Scholar] [CrossRef]
- Qu, Y.; Long, X.; Yuan, G.; Meng, G. A unified formulation for vibration analysis of functionally graded shells of revolution with arbitrary boundary conditions. Compos. Part B-Eng. 2013, 50, 381–402. [Google Scholar] [CrossRef]




| κ | Elliptic Shells | Elliptic Panels | ||||||
|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 1 | 2 | 3 | 4 | |
| 104 | 0.0886 | 0.0887 | 0.0887 | 0.0887 | 0.1146 | 0.1146 | 0.2130 | 0.2131 |
| 106 | 0.0899 | 0.0920 | 0.0923 | 0.0943 | 0.1167 | 0.1188 | 0.2145 | 0.2170 |
| 108 | 0.1114 | 0.1612 | 0.1729 | 0.1839 | 0.1689 | 0.2271 | 0.2566 | 0.3300 |
| 1010 | 0.1680 | 0.1805 | 0.1853 | 0.2023 | 0.2615 | 0.3850 | 0.3880 | 0.5019 |
| 1012 | 0.1817 | 0.1868 | 0.1932 | 0.2089 | 0.2727 | 0.4003 | 0.4102 | 0.5370 |
| 1014 | 0.1819 | 0.1869 | 0.1933 | 0.2090 | 0.2729 | 0.4005 | 0.4105 | 0.5375 |
| 1016 | 0.1819 | 0.1870 | 0.1933 | 0.2090 | 0.2729 | 0.4005 | 0.4105 | 0.5375 |
| 1018 | 0.1819 | 0.1870 | 0.1933 | 0.2090 | 0.2729 | 0.4006 | 0.4105 | 0.5375 |
| 1020 | 0.1821 | 0.1871 | 0.1933 | 0.2094 | 0.2704 | 0.4011 | 0.4106 | 0.5347 |
| 1022 | 0.1525 | 0.1779 | 0.2646 | 0.2646 | 0.3255 | 0.4131 | 0.5307 | 0.6446 |
| 1024 | 0.4118 | 0.4118 | 0.4793 | 0.5368 | 0.9096 | 0.9096 | 1.0145 | 1.3892 |
| Value | Elliptic Shells | Elliptic Panels | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| ku | kv | kw | Kx | Kθ | ku | kv | kw | Kx | Kθ | |
| 104 | 0.1759 | 0.1712 | 0.1759 | 0.1728 | 0.1818 | 0.2700 | 0.2633 | 0.2689 | 0.2668 | 0.2729 |
| 106 | 0.1759 | 0.1712 | 0.1759 | 0.1729 | 0.1818 | 0.2700 | 0.2633 | 0.2689 | 0.2668 | 0.2729 |
| 108 | 0.1759 | 0.1714 | 0.1766 | 0.1782 | 0.1819 | 0.2700 | 0.2634 | 0.2692 | 0.2702 | 0.2729 |
| 1010 | 0.1780 | 0.1781 | 0.1813 | 0.1818 | 0.1819 | 0.2708 | 0.2682 | 0.2724 | 0.2728 | 0.2729 |
| 1012 | 0.1818 | 0.1818 | 0.1819 | 0.1819 | 0.1819 | 0.2728 | 0.2728 | 0.2729 | 0.2729 | 0.2729 |
| 1014 | 0.1819 | 0.1819 | 0.1819 | 0.1819 | 0.1819 | 0.2729 | 0.2729 | 0.2729 | 0.2729 | 0.2729 |
| 1016 | 0.1819 | 0.1819 | 0.1819 | 0.1819 | 0.1819 | 0.2729 | 0.2729 | 0.2729 | 0.2729 | 0.2729 |
| 1018 | 0.1819 | 0.1819 | 0.1819 | 0.1818 | 0.1819 | 0.2729 | 0.2729 | 0.2729 | 0.2729 | 0.2729 |
| 1020 | 0.1819 | 0.1819 | 0.1819 | 0.1502 | 0.1819 | 0.2729 | 0.2729 | 0.2729 | 0.2669 | 0.2729 |
| 1022 | 0.1819 | 0.1819 | 0.1819 | 0.1997 | 0.1764 | 0.2729 | 0.2729 | 0.2730 | 0.3605 | 0.2772 |
| 1024 | 0.1819 | 0.1821 | 0.1752 | 0.2641 | 0.1903 | 0.2729 | 0.2730 | 0.2727 | 0.4777 | 0.0883 |
| Mode No. | Type 1 | Type 2 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| e0 = 0.2 | e0 = 0.4 | e0 = 0.2 | e0 = 0.4 | |||||||||
| Ref. [20] | Ref. [17] | Present | Ref. [20] | Ref. [17] | Present | Ref. [20] | Ref. [17] | Present | Ref. [20] | Ref. [17] | Present | |
| 1 | 1.2155 | 1.2161 | 1.2161 | 1.1893 | 1.1900 | 1.1900 | 1.2037 | 1.2043 | 1.2043 | 1.1598 | 1.1606 | 1.1605 |
| 2 | 1.2118 | 1.2121 | 1.2121 | 1.1862 | 1.1866 | 1.1866 | 1.1997 | 1.1996 | 1.1995 | 1.1559 | 1.1565 | 1.1564 |
| 3 | 1.2064 | 1.2064 | 1.2063 | 1.1818 | 1.1818 | 1.1817 | 1.1938 | 1.1940 | 1.1939 | 1.1501 | 1.1507 | 1.1505 |
| 4 | 1.2006 | 1.2002 | 1.2000 | 1.1772 | 1.1768 | 1.1766 | 1.1872 | 1.1871 | 1.1869 | 1.1438 | 1.1441 | 1.1438 |
| 5 | 1.1958 | 1.1952 | 1.1956 | 1.1740 | 1.1733 | 1.1726 | 1.1815 | 1.1813 | 1.1806 | 1.1382 | 1.1384 | 1.1378 |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| C-C | SD-SD | C-F | C-S | E1-E1 | E2-E2 | E3-E3 | E4-E4 | ||
| a/b = 1 | 0 | 0.2926 | 0.2187 | 0.1158 | 0.2855 | 0.0577 | 0.2834 | 0.2848 | 0.0570 |
| 0.2 | 0.2848 | 0.2131 | 0.1140 | 0.2777 | 0.0597 | 0.2756 | 0.2773 | 0.0589 | |
| 0.4 | 0.2770 | 0.2075 | 0.1125 | 0.2699 | 0.0623 | 0.2677 | 0.2698 | 0.0613 | |
| 0.8 | 0.2657 | 0.2000 | 0.1128 | 0.2581 | 0.0715 | 0.2559 | 0.2590 | 0.0700 | |
| a/b = 2 | 0 | 0.1853 | 0.1525 | 0.0824 | 0.1762 | 0.0577 | 0.1755 | 0.1777 | 0.0553 |
| 0.2 | 0.1819 | 0.1500 | 0.0803 | 0.1728 | 0.0597 | 0.1720 | 0.1746 | 0.0570 | |
| 0.4 | 0.1787 | 0.1477 | 0.0782 | 0.1695 | 0.0623 | 0.1687 | 0.1719 | 0.0592 | |
| 0.8 | 0.1770 | 0.1473 | 0.0755 | 0.1671 | 0.0715 | 0.1662 | 0.1706 | 0.0669 | |
| a/b = 3 | 0 | 0.1279 | 0.1028 | 0.0612 | 0.1158 | 0.0577 | 0.1157 | 0.1199 | 0.0525 |
| 0.2 | 0.1256 | 0.1006 | 0.0596 | 0.1134 | 0.0597 | 0.1134 | 0.1180 | 0.0539 | |
| 0.4 | 0.1236 | 0.0984 | 0.0579 | 0.1111 | 0.0623 | 0.1112 | 0.1162 | 0.0556 | |
| 0.8 | 0.1227 | 0.0963 | 0.0554 | 0.1090 | 0.0715 | 0.1096 | 0.1158 | 0.0620 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| C-C | SD-SD | C-F | C-S | E1-E1 | E2-E2 | E3-E3 | E4-E4 | ||
| a/b = 1 | 0 | 0.2926 | 0.2187 | 0.1158 | 0.2855 | 0.0577 | 0.2834 | 0.2848 | 0.0570 |
| 0.2 | 0.2829 | 0.2115 | 0.1121 | 0.2753 | 0.0597 | 0.2740 | 0.2750 | 0.0589 | |
| 0.4 | 0.2723 | 0.2036 | 0.1077 | 0.2639 | 0.0622 | 0.2637 | 0.2643 | 0.0613 | |
| 0.8 | 0.2485 | 0.1854 | 0.0951 | 0.2376 | 0.0713 | 0.2414 | 0.2406 | 0.0697 | |
| a/b = 2 | 0 | 0.1853 | 0.1525 | 0.0824 | 0.1762 | 0.0577 | 0.1755 | 0.1777 | 0.0553 |
| 0.2 | 0.1794 | 0.1478 | 0.0797 | 0.1702 | 0.0597 | 0.1700 | 0.1723 | 0.0570 | |
| 0.4 | 0.1727 | 0.1423 | 0.0768 | 0.1634 | 0.0622 | 0.1638 | 0.1661 | 0.0591 | |
| 0.8 | 0.1544 | 0.1267 | 0.0700 | 0.1448 | 0.0714 | 0.1477 | 0.1495 | 0.0661 | |
| a/b = 3 | 0 | 0.1279 | 0.1028 | 0.0612 | 0.1158 | 0.0577 | 0.1157 | 0.1199 | 0.0525 |
| 0.2 | 0.1239 | 0.0996 | 0.0593 | 0.1121 | 0.0597 | 0.1124 | 0.1167 | 0.0538 | |
| 0.4 | 0.1193 | 0.0960 | 0.0571 | 0.1079 | 0.0623 | 0.1089 | 0.1131 | 0.0553 | |
| 0.8 | 0.1068 | 0.0868 | 0.0524 | 0.0972 | 0.0714 | 0.1000 | 0.1032 | 0.0598 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| C-E1 | C-E2 | C-E3 | C-E4 | SD-E1 | SD-E2 | SD-E3 | SD-E4 | ||
| a/b = 1 | 0 | 0.2598 | 0.2879 | 0.2886 | 0.1550 | 0.0407 | 0.2509 | 0.2515 | 0.0407 |
| 0.2 | 0.2534 | 0.2801 | 0.2810 | 0.1547 | 0.0422 | 0.2442 | 0.2450 | 0.0421 | |
| 0.4 | 0.2471 | 0.2723 | 0.2733 | 0.1548 | 0.0440 | 0.2375 | 0.2384 | 0.0440 | |
| 0.8 | 0.2390 | 0.2607 | 0.2623 | 0.1604 | 0.0505 | 0.2278 | 0.2291 | 0.0505 | |
| a/b = 2 | 0 | 0.1716 | 0.1800 | 0.1813 | 0.1120 | 0.0407 | 0.1644 | 0.1653 | 0.0407 |
| 0.2 | 0.1686 | 0.1766 | 0.1781 | 0.1115 | 0.0422 | 0.1614 | 0.1625 | 0.0421 | |
| 0.4 | 0.1659 | 0.1734 | 0.1752 | 0.1112 | 0.0440 | 0.1586 | 0.1599 | 0.0440 | |
| 0.8 | 0.1646 | 0.1711 | 0.1737 | 0.1142 | 0.0505 | 0.1570 | 0.1588 | 0.0505 | |
| a/b = 3 | 0 | 0.1191 | 0.1206 | 0.1237 | 0.0870 | 0.0407 | 0.1094 | 0.1108 | 0.0407 |
| 0.2 | 0.1172 | 0.1182 | 0.1216 | 0.0866 | 0.0422 | 0.1071 | 0.1087 | 0.0421 | |
| 0.4 | 0.1155 | 0.1161 | 0.1197 | 0.0865 | 0.0440 | 0.1050 | 0.1066 | 0.0440 | |
| 0.8 | 0.1155 | 0.1148 | 0.1191 | 0.0891 | 0.0505 | 0.1030 | 0.1051 | 0.0505 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| C-E1 | C-E2 | C-E3 | C-E4 | SD-E1 | SD-E2 | SD-E3 | SD-E4 | ||
| a/b = 1 | 0 | 0.2598 | 0.2879 | 0.2886 | 0.1550 | 0.0407 | 0.2509 | 0.2515 | 0.0407 |
| 0.2 | 0.2513 | 0.2784 | 0.2789 | 0.1530 | 0.0421 | 0.2426 | 0.2431 | 0.0421 | |
| 0.4 | 0.2418 | 0.2679 | 0.2682 | 0.1507 | 0.0439 | 0.2336 | 0.2338 | 0.0439 | |
| 0.8 | 0.2195 | 0.2449 | 0.2445 | 0.1456 | 0.0503 | 0.2133 | 0.2129 | 0.0503 | |
| a/b = 2 | 0 | 0.1716 | 0.1800 | 0.1813 | 0.1120 | 0.0407 | 0.1644 | 0.1653 | 0.0407 |
| 0.2 | 0.1662 | 0.1744 | 0.1757 | 0.1108 | 0.0421 | 0.1593 | 0.1602 | 0.0421 | |
| 0.4 | 0.1600 | 0.1680 | 0.1693 | 0.1096 | 0.0439 | 0.1534 | 0.1543 | 0.0439 | |
| 0.8 | 0.1428 | 0.1509 | 0.1519 | 0.1078 | 0.0503 | 0.1375 | 0.1382 | 0.0503 | |
| a/b = 3 | 0 | 0.1191 | 0.1206 | 0.1237 | 0.0870 | 0.0407 | 0.1094 | 0.1108 | 0.0407 |
| 0.2 | 0.1154 | 0.1171 | 0.1201 | 0.0861 | 0.0421 | 0.1061 | 0.1075 | 0.0421 | |
| 0.4 | 0.1112 | 0.1131 | 0.1161 | 0.0851 | 0.0439 | 0.1025 | 0.1038 | 0.0439 | |
| 0.8 | 0.0991 | 0.1030 | 0.1049 | 0.0827 | 0.0504 | 0.0933 | 0.0942 | 0.0504 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| CCCC | SDSDSDSD | CFCF | CSCS | E1E1E1E1 | E2E2E2E2 | E3E3E3E3 | E4E4E4E4 | ||
| a/b = 1 | 0 | 0.4641 | 0.2727 | 0.1324 | 0.3887 | 0.0898 | 0.3471 | 0.4195 | 0.0858 |
| 0.2 | 0.4574 | 0.2685 | 0.1300 | 0.3807 | 0.0929 | 0.3433 | 0.4139 | 0.0885 | |
| 0.4 | 0.4519 | 0.2594 | 0.1280 | 0.3732 | 0.0969 | 0.3406 | 0.4094 | 0.0920 | |
| 0.8 | 0.4541 | 0.2434 | 0.1273 | 0.3671 | 0.1112 | 0.3313 | 0.4126 | 0.1043 | |
| a/b = 2 | 0 | 0.2774 | 0.1669 | 0.1056 | 0.2455 | 0.0835 | 0.2155 | 0.2577 | 0.0773 |
| 0.2 | 0.2729 | 0.1649 | 0.1044 | 0.2406 | 0.0864 | 0.2125 | 0.2538 | 0.0796 | |
| 0.4 | 0.2689 | 0.1632 | 0.1035 | 0.2359 | 0.0901 | 0.2098 | 0.2504 | 0.0825 | |
| 0.8 | 0.2683 | 0.1651 | 0.1051 | 0.2322 | 0.1033 | 0.2094 | 0.2505 | 0.0930 | |
| a/b = 3 | 0 | 0.1704 | 0.1093 | 0.0902 | 0.1557 | 0.0781 | 0.1401 | 0.1580 | 0.0674 |
| 0.2 | 0.1678 | 0.1078 | 0.0895 | 0.1530 | 0.0808 | 0.1385 | 0.1559 | 0.0691 | |
| 0.4 | 0.1656 | 0.1065 | 0.0891 | 0.1507 | 0.0843 | 0.1372 | 0.1542 | 0.0713 | |
| 0.8 | 0.1659 | 0.1072 | 0.0916 | 0.1500 | 0.0966 | 0.1383 | 0.1551 | 0.0793 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| CCCC | SDSDSDSD | CFCF | CSCS | E1E1E1E1 | E2E2E2E2 | E3E3E3E3 | E4E4E4E4 | ||
| a/b = 1 | 0 | 0.4641 | 0.2727 | 0.1324 | 0.3887 | 0.0898 | 0.3471 | 0.4195 | 0.0858 |
| 0.2 | 0.4490 | 0.2641 | 0.1282 | 0.3777 | 0.0929 | 0.3374 | 0.4088 | 0.0884 | |
| 0.4 | 0.4313 | 0.2535 | 0.1234 | 0.3653 | 0.0968 | 0.3262 | 0.3964 | 0.0916 | |
| 0.8 | 0.3787 | 0.2185 | 0.1102 | 0.3347 | 0.1108 | 0.2947 | 0.3597 | 0.1024 | |
| a/b = 2 | 0 | 0.2774 | 0.1669 | 0.1056 | 0.2455 | 0.0835 | 0.2155 | 0.2577 | 0.0773 |
| 0.2 | 0.2686 | 0.1618 | 0.1024 | 0.2392 | 0.0863 | 0.2110 | 0.2512 | 0.0794 | |
| 0.4 | 0.2585 | 0.1557 | 0.0986 | 0.2322 | 0.0900 | 0.2063 | 0.2437 | 0.0820 | |
| 0.8 | 0.2294 | 0.1369 | 0.0865 | 0.2148 | 0.1030 | 0.1969 | 0.2220 | 0.0899 | |
| a/b = 3 | 0 | 0.1704 | 0.1093 | 0.0902 | 0.1557 | 0.0781 | 0.1401 | 0.1580 | 0.0674 |
| 0.2 | 0.1652 | 0.1060 | 0.0876 | 0.1517 | 0.0808 | 0.1377 | 0.1543 | 0.0688 | |
| 0.4 | 0.1590 | 0.1021 | 0.0843 | 0.1472 | 0.0842 | 0.1352 | 0.1498 | 0.0704 | |
| 0.8 | 0.1411 | 0.0905 | 0.0734 | 0.1349 | 0.0964 | 0.1284 | 0.1365 | 0.0744 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| CE1CE1 | CE2CE2 | CE3CE3 | CE4CE4 | SDE1SDE1 | SDE2SDE2 | SDE3SDE3 | SDE4SDE4 | ||
| a/b = 1 | 0 | 0.2843 | 0.3619 | 0.4248 | 0.1970 | 0.0689 | 0.3301 | 0.3985 | 0.0689 |
| 0.2 | 0.2770 | 0.3580 | 0.4190 | 0.1971 | 0.0714 | 0.3273 | 0.3935 | 0.0714 | |
| 0.4 | 0.2696 | 0.3551 | 0.4142 | 0.1981 | 0.0744 | 0.3258 | 0.3896 | 0.0744 | |
| 0.8 | 0.2594 | 0.3443 | 0.4169 | 0.2081 | 0.0854 | 0.3225 | 0.3936 | 0.0854 | |
| a/b = 2 | 0 | 0.2279 | 0.2476 | 0.2634 | 0.1569 | 0.0605 | 0.2280 | 0.2447 | 0.0605 |
| 0.2 | 0.2246 | 0.2430 | 0.2593 | 0.1567 | 0.0626 | 0.2241 | 0.2409 | 0.0626 | |
| 0.4 | 0.2216 | 0.2385 | 0.2556 | 0.1571 | 0.0653 | 0.2202 | 0.2375 | 0.0653 | |
| 0.8 | 0.2205 | 0.2342 | 0.2553 | 0.1634 | 0.0750 | 0.2164 | 0.2372 | 0.0750 | |
| a/b = 3 | 0 | 0.1518 | 0.1659 | 0.1649 | 0.1260 | 0.0529 | 0.1478 | 0.1462 | 0.0529 |
| 0.2 | 0.1500 | 0.1629 | 0.1625 | 0.1256 | 0.0548 | 0.1448 | 0.1438 | 0.0548 | |
| 0.4 | 0.1487 | 0.1602 | 0.1604 | 0.1256 | 0.0571 | 0.1419 | 0.1417 | 0.0571 | |
| 0.8 | 0.1507 | 0.1587 | 0.1610 | 0.1296 | 0.0655 | 0.1391 | 0.1411 | 0.0655 | |
| a/b | e0 | Boundary Types | |||||||
|---|---|---|---|---|---|---|---|---|---|
| CE1CE1 | CE2CE2 | CE3CE3 | CE4CE4 | SDE1SDE1 | SDE2SDE2 | SDE3SDE3 | SDE4SDE4 | ||
| a/b = 1 | 0 | 0.2843 | 0.3619 | 0.4248 | 0.1970 | 0.0689 | 0.3301 | 0.3985 | 0.0689 |
| 0.2 | 0.2756 | 0.3516 | 0.4141 | 0.1951 | 0.0713 | 0.3211 | 0.3888 | 0.0713 | |
| 0.4 | 0.2662 | 0.3396 | 0.4015 | 0.1932 | 0.0744 | 0.3105 | 0.3774 | 0.0744 | |
| 0.8 | 0.2472 | 0.3055 | 0.3645 | 0.1890 | 0.0851 | 0.2793 | 0.3423 | 0.0851 | |
| a/b = 2 | 0 | 0.2279 | 0.2476 | 0.2634 | 0.1569 | 0.0605 | 0.2280 | 0.2447 | 0.0605 |
| 0.2 | 0.2209 | 0.2408 | 0.2566 | 0.1551 | 0.0626 | 0.2220 | 0.2386 | 0.0626 | |
| 0.4 | 0.2126 | 0.2332 | 0.2486 | 0.1531 | 0.0653 | 0.2154 | 0.2314 | 0.0653 | |
| 0.8 | 0.1879 | 0.2146 | 0.2256 | 0.1473 | 0.0747 | 0.1990 | 0.2102 | 0.0747 | |
| a/b = 3 | 0 | 0.1518 | 0.1659 | 0.1649 | 0.1260 | 0.0529 | 0.1478 | 0.1462 | 0.0529 |
| 0.2 | 0.1472 | 0.1608 | 0.1604 | 0.1239 | 0.0548 | 0.1432 | 0.1424 | 0.0548 | |
| 0.4 | 0.1417 | 0.1550 | 0.1552 | 0.1213 | 0.0571 | 0.1381 | 0.1380 | 0.0571 | |
| 0.8 | 0.1247 | 0.1388 | 0.1397 | 0.1124 | 0.0654 | 0.1245 | 0.1252 | 0.0654 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, Q.; Liu, T.; Teng, Y.; Ma, B.; Li, X. Ritz Solution of Vibration Analysis of Functionally Graded Porous Elliptic Shells and Panels Under Various Arbitrary Boundary Types. Materials 2025, 18, 1101. https://doi.org/10.3390/ma18051101
Gong Q, Liu T, Teng Y, Ma B, Li X. Ritz Solution of Vibration Analysis of Functionally Graded Porous Elliptic Shells and Panels Under Various Arbitrary Boundary Types. Materials. 2025; 18(5):1101. https://doi.org/10.3390/ma18051101
Chicago/Turabian StyleGong, Qingtao, Tao Liu, Yao Teng, Binjie Ma, and Xin Li. 2025. "Ritz Solution of Vibration Analysis of Functionally Graded Porous Elliptic Shells and Panels Under Various Arbitrary Boundary Types" Materials 18, no. 5: 1101. https://doi.org/10.3390/ma18051101
APA StyleGong, Q., Liu, T., Teng, Y., Ma, B., & Li, X. (2025). Ritz Solution of Vibration Analysis of Functionally Graded Porous Elliptic Shells and Panels Under Various Arbitrary Boundary Types. Materials, 18(5), 1101. https://doi.org/10.3390/ma18051101







