Mechanical Characterization and Modeling of Glass Fiber-Reinforced Polyamide Built by Additive Manufacturing
Abstract
1. Introduction
2. Materials and Methods
2.1. Mechanical Behavior of a Lamina and Laminate
2.2. Mechanical Strength of a Lamina and Laminate
2.3. Manufacturing of Layered Composites by AM
3. Results
3.1. Experimental Plan
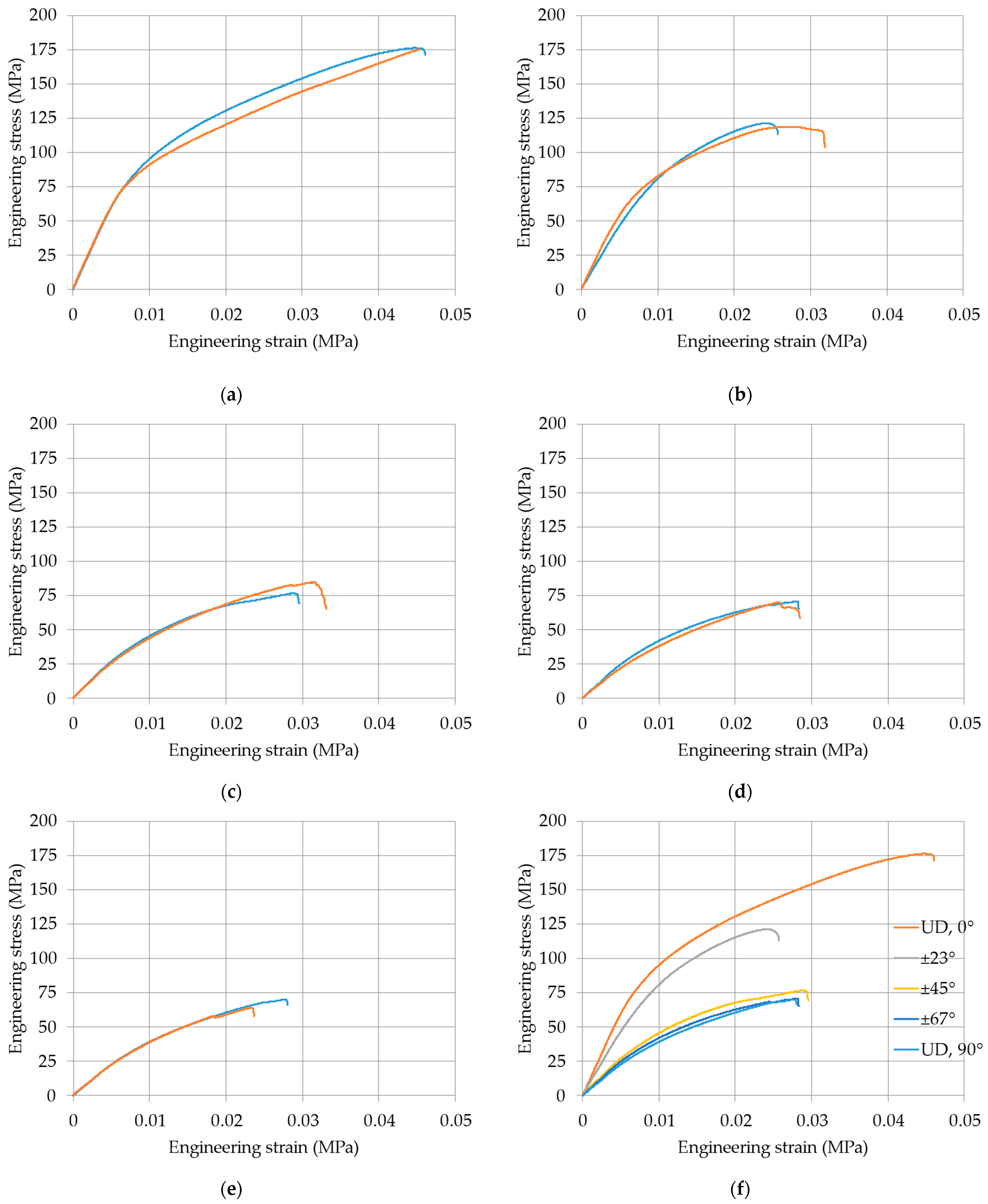
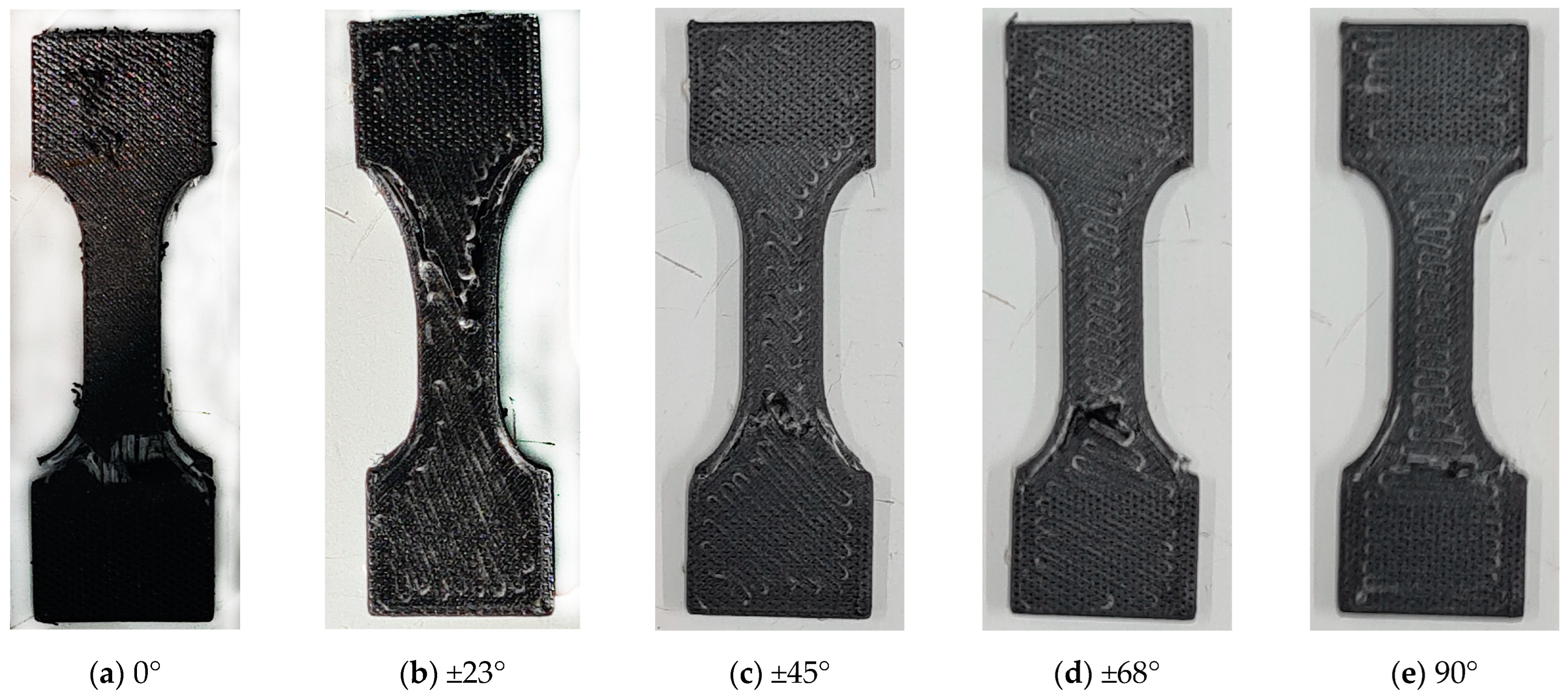
3.2. Experimental Results
3.3. Identification of the Model Parameters
3.3.1. Evaluation of the Elastic Properties
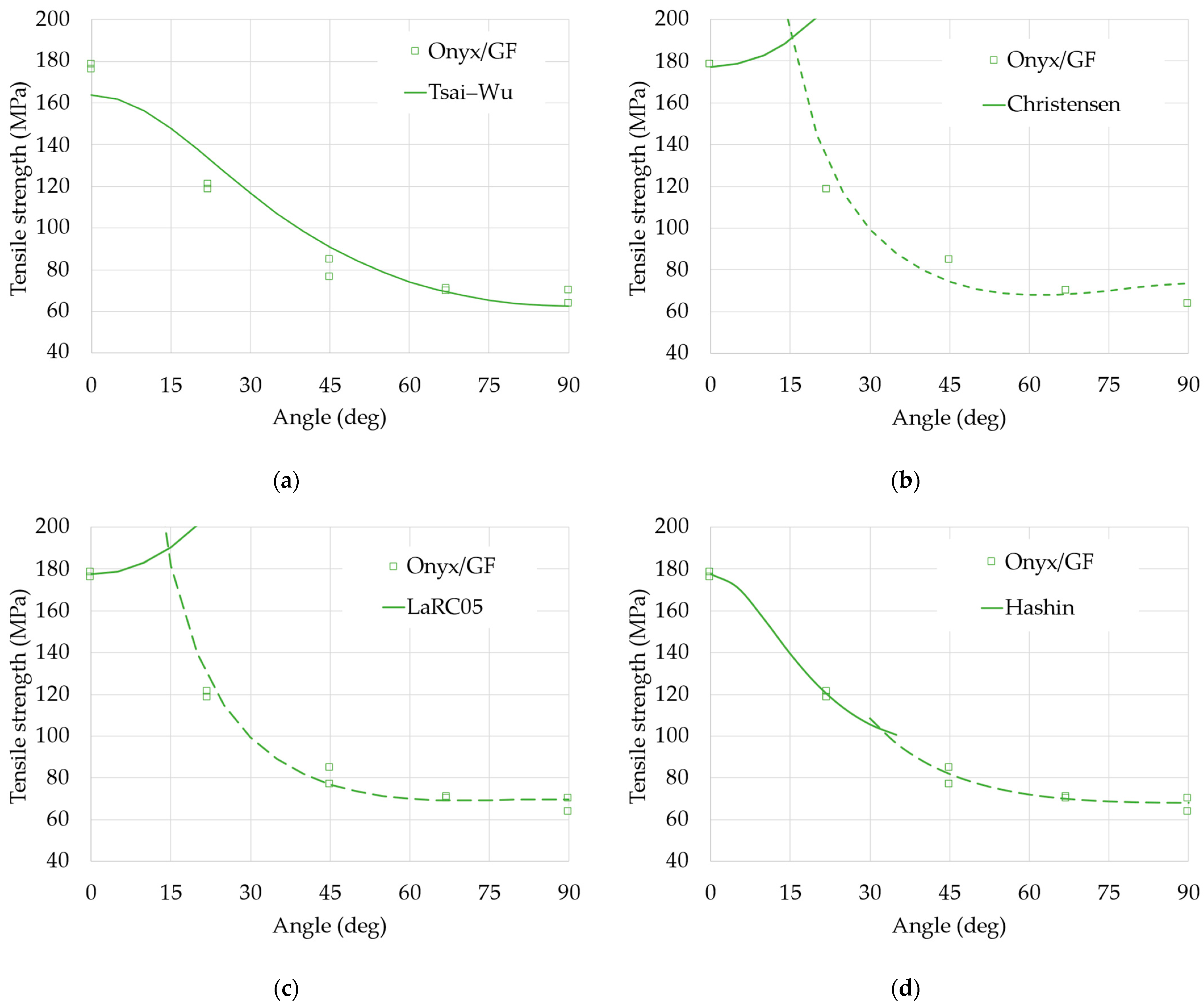
3.3.2. Analysis of the Strength and Identification of a Suitable Failure Model
- Tsai–Wu;
- Christensen;
- LaRC05;
- Hashin.
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pham, D.T.; Gault, R.S. A Comparison of Rapid Prototyping Technologies. Int. J. Mach. Tools Manuf. 1998, 38, 1257–1287. [Google Scholar] [CrossRef]
- Gao, W.; Zhang, Y.; Ramanujan, D.; Ramani, K.; Chen, Y.; Williams, C.B.; Wang, C.C.L.; Shin, Y.C.; Zhang, S.; Zavattieri, P.D. The Status, Challenges, and Future of Additive Manufacturing in Engineering. CAD Comput. Aided Des. 2015, 69, 65–89. [Google Scholar] [CrossRef]
- Bourell, D.L. Perspectives on Additive Manufacturing. Annu. Rev. Mater. Res. 2016, 46, 1–18. [Google Scholar] [CrossRef]
- ASTM F2792-12; Standard Terminology for Additive Manufacturing Technologies. ASTM International: West Conshohochen, PA, USA, 2012.
- Crump, S.S. Fast, Precise, Safe Prototypes with FDM. Am. Soc. Mech. Eng. Prod. Eng. Div. PED 1991, 50, 53–60. [Google Scholar]
- Fico, D.; Rizzo, D.; Casciaro, R.; Corcione, C.E. A Review of Polymer-Based Materials for Fused Filament Fabrication (FFF): Focus on Sustainability and Recycled Materials. Polymers 2022, 14, 465. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Jiang, M.; Zhou, Z.; Gou, J.; Hui, D. 3D Printing of Polymer Matrix Composites: A Review and Prospective. Compos. Part B Eng. 2017, 110, 442–458. [Google Scholar] [CrossRef]
- Daminabo, S.C.; Goel, S.; Grammatikos, S.A.; Nezhad, H.Y.; Thakur, V.K. Fused Deposition Modeling-Based Additive Manufacturing (3D Printing): Techniques for Polymer Material Systems. Mater. Today Chem. 2020, 16, 100248. [Google Scholar] [CrossRef]
- Christ, J.F.; Aliheidari, N.; Ameli, A.; Pötschke, P. 3D Printed Highly Elastic Strain Sensors of Multiwalled Carbon Nanotube/Thermoplastic Polyurethane Nanocomposites. Mater. Des. 2017, 131, 394–401. [Google Scholar] [CrossRef]
- Musenich, L.; Berardengo, M.; Avalle, M.; Haj-Ali, R.; Sharabi, M.; Libonati, F. Anisotropic Mechanical and Sensing Properties of Carbon Black-Polylactic Acid Nanocomposites Produced by Fused Filament Fabrication. Smart Mater. Struct. 2024, 33, 095010. [Google Scholar] [CrossRef]
- Ngo, T.D.; Kashani, A.; Imbalzano, G.; Nguyen, K.T.Q.; Hui, D. Additive Manufacturing (3D Printing): A Review of Materials, Methods, Applications and Challenges. Compos. Part B Eng. 2018, 143, 172–196. [Google Scholar] [CrossRef]
- Demircali, A.A.; Yilmaz, D.; Yilmaz, A.; Keskin, O.; Keshavarz, M.; Uvet, H. Enhancing Mechanical Properties and Surface Quality of FDM-Printed ABS: A Comprehensive Study on Cold Acetone Vapor Treatment. Int. J. Adv. Manuf. Technol. 2024, 130, 4027–4039. [Google Scholar] [CrossRef]
- Wong, J.; Altassan, A.; Rosen, D.W. Additive Manufacturing of Fiber-Reinforced Polymer Composites: A Technical Review and Status of Design Methodologies. Compos. Part B Eng. 2023, 255, 110603. [Google Scholar] [CrossRef]
- Yaragatti, N.; Patnaik, A. A Review on Additive Manufacturing of Polymers Composites. Mater. Today Proc. 2020, 44, 4150–4157. [Google Scholar] [CrossRef]
- Xian, G.; Zhou, P.; Li, C.; Dong, S.; Du, H.; Tian, J.; Guo, R.; Peng, Z.; Zhang, Z.; He, T. Mechanical Properties Evaluation of Glass Fiber Reinforced Thermoplastic Composite Plate under Combined Bending Loading and Water Immersion. Constr. Build. Mater. 2024, 440, 137470. [Google Scholar] [CrossRef]
- Jiang, D.; Smith, D.E. Anisotropic Mechanical Properties of Oriented Carbon Fiber Filled Polymer Composites Produced with Fused Filament Fabrication. Addit. Manuf. 2017, 18, 84–94. [Google Scholar] [CrossRef]
- Ke, L.; Li, Y.; Li, C.; Cheng, Z.; Ma, K.; Zeng, J. Bond Behavior of CFRP-Strengthened Steel Structures and Its Environmental Influence Factors: A Critical Review. Sustain. Struct. 2024, 4, 000038. [Google Scholar] [CrossRef]
- Dong, G.; Tang, Y.; Li, D.; Zhao, Y.F. Mechanical Properties of Continuous Kevlar Fiber Reinforced Composites Fabricated by Fused Deposition Modeling Process. Procedia Manuf. 2018, 26, 774–781. [Google Scholar] [CrossRef]
- Karimi, A.; Rahmatabadi, D.; Baghani, M. Various FDM Mechanisms Used in the Fabrication of Continuous-Fiber Reinforced Composites: A Review. Polymers 2024, 16, 831. [Google Scholar] [CrossRef]
- Vaithiyanathan, A.; Farhan, H.; Raja, D.E.; Singh, S.P.; Sonar, T. Microstructural Characteristics and Mechanical Properties of 3D Printed Kevlar Fibre Reinforced Onyx Composite. Mater. Test. 2024, 66, 1519–1527. [Google Scholar] [CrossRef]
- Gao, G.; Xu, F.; Xu, J.; Tang, G.; Liu, Z. A Survey of the Influence of Process Parameters on Mechanical Properties of Fused Deposition Modeling Parts. Micromachines 2022, 13, 553. [Google Scholar] [CrossRef] [PubMed]
- Lanzotti, A.; Grasso, M.; Staiano, G.; Martorelli, M. The Impact of Process Parameters on Mechanical Properties of Parts Fabricated in PLA with an Open-Source 3-D Printer. Rapid Prototyp. J. 2015, 21, 604–617. [Google Scholar] [CrossRef]
- Gonabadi, H.; Yadav, A.; Bull, S.J. The Effect of Processing Parameters on the Mechanical Characteristics of PLA Produced by a 3D FFF Printer. Int. J. Adv. Manuf. Technol. 2020, 111, 695–709. [Google Scholar] [CrossRef]
- Vǎlean, C.; Marşavina, L.; Mǎrghitaşl, M.; Linul, E.; Razavi, N.; Berto, F. Effect of Manufacturing Parameters on Tensile Properties of FDM Printed Specimens. Procedia Struct. Integr. 2020, 26, 313–320. [Google Scholar] [CrossRef]
- Ahn, S.H.; Montero, M.; Odell, D.; Roundy, S.; Wright, P.K. Anisotropic Material Properties of Fused Deposition Modeling ABS. Rapid Prototyp. J. 2002, 8, 248–257. [Google Scholar] [CrossRef]
- Wu, W.; Geng, P.; Li, G.; Zhao, D.; Zhang, H.; Zhao, J. Influence of Layer Thickness and Raster Angle on the Mechanical Properties of 3D-Printed PEEK and a Comparative Mechanical Study between PEEK and ABS. Materials 2015, 8, 5834–5846. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Olmi, G. Experimental Characterization and Analytical Modelling of the Mechanical Behaviour of Fused Deposition Processed Parts Made of ABS-M30. Comput. Mater. Sci. 2013, 79, 506–518. [Google Scholar] [CrossRef]
- Cantrell, J.T.; Rohde, S.; Damiani, D.; Gurnani, R.; DiSandro, L.; Anton, J.; Young, A.; Jerez, A.; Steinbach, D.; Kroese, C.; et al. Experimental Characterization of the Mechanical Properties of 3D-Printed ABS and Polycarbonate Parts. Rapid Prototyp. J. 2017, 23, 811–824. [Google Scholar] [CrossRef]
- Hameed, A.Z.; Aravind Raj, S.; Kandasamy, J.; Shahzad, M.A.; Baghdadi, M.A. 3D Printing Parameter Optimization Using Taguchi Approach to Examine Acrylonitrile Styrene Acrylate (ASA) Mechanical Properties. Polymers 2022, 14, 3256. [Google Scholar] [CrossRef] [PubMed]
- Sun, Q.; Rizvi, G.M.; Bellehumeur, C.T.; Gu, P. Effect of Processing Conditions on the Bonding Quality of FDM Polymer Filaments. Rapid Prototyp. J. 2008, 14, 72–80. [Google Scholar] [CrossRef]
- Syrlybayev, D.; Zharylkassyn, B.; Seisekulova, A.; Akhmetov, M.; Perveen, A.; Talamona, D. Optimisation of Strength Properties of FDM Printed Parts—A critical review. Polymers 2021, 13, 1587. [Google Scholar] [CrossRef]
- Somireddy, M.; Singh, C.V.; Czekanski, A. Analysis of the Material Behavior of 3D Printed Laminates Via FFF. Exp. Mech. 2019, 59, 871–881. [Google Scholar] [CrossRef]
- Alaimo, G.; Marconi, S.; Costato, L.; Auricchio, F. Influence of Meso-Structure and Chemical Composition on FDM 3D-Printed Parts. Compos. Part B Eng. 2017, 113, 371–380. [Google Scholar] [CrossRef]
- Rajpurohit, S.R.; Dave, H.K.; Rajurkar, K.P. Prediction of Tensile Strength of Fused Deposition Modeling (FDM) Printed PLA Using Classic Laminate Theory. Eng. Solid Mech. 2022, 10, 13–24. [Google Scholar] [CrossRef]
- Dai, S.; Deng, Z.C.; Yu, Y.J.; Zhang, K.; Wang, S.H.; Ye, J. Orthotropic Elastic Behaviors and Yield Strength of Fused Deposition Modeling Materials: Theory and Experiments. Polym. Test. 2020, 87, 106520. [Google Scholar] [CrossRef]
- YİLMAZ, C.; ALİ, H.Q.; YILDIZ, M. Klasik Laminasyon Teorisinin Üç Boyutlu Yazıcı Ile Eriyik Yığma Modelleme Yöntemi Kullanılarak Üretilmiş Plastiklere Uygulanması ve Tam Alanlı Yüzey Gerinim Haritalanması. Afyon Kocatepe Univ. J. Sci. Eng. 2022, 22, 342–352. [Google Scholar] [CrossRef]
- Avalle, M.; Monti, M.; Frascio, M. Modeling the Strength of Laminated Parts Made by Fused Filament Fabrication Additive Manufacturing. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023. [Google Scholar] [CrossRef]
- Du, H.; Xian, G.; Tian, J.; Ma, Z.; Li, C.; Xin, M.; Zhang, Y. Effect of Fiber Surface Treatment with Silane Coupling Agents and Carbon Nanotubes on Mechanical Properties of Carbon Fiber Reinforced Polyamide 6 Composites. Polym. Compos. 2024, 46, 1267–1283. [Google Scholar] [CrossRef]
- Jones, R.M. Mechanics of Composite Materials; Taylor and Francis: Philadelphia, PA, USA, 1984; ISBN 0938994247. [Google Scholar]
- Halpin, J.C. Primer on Composite Materials Analysis, Second Edition (Revised), 2nd ed.; Routledge: Lancaster, PA, USA, 2017; ISBN 9780203742235. [Google Scholar]
- Nettles, A.T. Basic Mechanics of Laminated Composite Plates; NASA Reference Publication 1351; NASA: Washington, DC, USA, 1994; p. 107.
- Casavola, C.; Cazzato, A.; Moramarco, V.; Pappalettere, C. Orthotropic Mechanical Properties of Fused Deposition Modelling Parts Described by Classical Laminate Theory. Mater. Des. 2016, 90, 453–458. [Google Scholar] [CrossRef]
- Nasirov, A.; Hasanov, S.; Fidan, I. Prediction of Mechanical Properties of Fused Deposition Modeling Made Parts Using Multiscale Modeling and Classical Laminate Theory. In Proceedings of the 2019 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 12–14 August 2019; pp. 1373–1382. [Google Scholar]
- Somireddy, M.; Czekanski, A.; Singh, C.V. Development of Constitutive Material Model of 3D Printed Structure via FDM. Mater. Today Commun. 2018, 15, 143–152. [Google Scholar] [CrossRef]
- Hill, R. Theory of Mechanical Properties of Fibre-Strengthened Materials—III. Self-Consistent Model. J. Mech. Phys. Solids 1965, 13, 189–198. [Google Scholar] [CrossRef]
- Tsai, S.W. Strength Theories of Filamentary Structures, in Fundamental Aspects of Fiber Reinforced Plastic Composites. In Proceedings of the Fundamental Aspects of Fiber Reinforced Plastic Composites; Schwartz, R.T., Schwartz, H.S., Eds.; Interscience Publishers: New York, NY, USA, 1968; p. 284. [Google Scholar]
- Tsai, S.W.; Wu, E.M. A General Theory of Strength for Anisotropic Materials. J. Compos. Mater. 1971, 5, 58–80. [Google Scholar] [CrossRef]
- ISO 527-1:2019; Plastics—Determination of Tensile Properties Part 1: General Principles. ISO: Geneva, Switzerland, 2019.
- ASTM D638-14; Standard Test Method for Tensile Properties of Plastics. ASTM International: West Conshohochen, PA, USA, 2014.
- Hill, R. A Theory of the Yielding and Plastic Flow of Anisotropic Metals. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 193, 281–297. [Google Scholar] [CrossRef]
- Azzi, V.D.; Tsai, S.W. Anisotropic Strength of Composites-Investigation Aimed at Developing a Theory Applicable to Laminated as Well as Unidirectional Composites, Employing Simple Material Properties Derived from Unidirectional Specimens Alone. Exp. Mech. 1965, 5, 283–288. [Google Scholar] [CrossRef]
- Hashin, Z.; Rotem, A. A Fatigue Failure Criterion for Fiber Reinforced Materials. J. Compos. Mater. 1973, 7, 448–464. [Google Scholar] [CrossRef]
- Hashin, Z. Failure Criteria for Unidirectional Fiber Composites. J. Appl. Mech. Trans. ASME 1980, 47, 329–334. [Google Scholar] [CrossRef]
- Christensen, R.M. Completion and Closure on Failure Criteria for Unidirectional Fiber Composite Materials. J. Appl. Mech. 2014, 81, 1–6. [Google Scholar] [CrossRef]
- Pinho, S.T.; Dávila, C.G.; Camanho, P.P.; Iannucci, L.; Robinson, P. Failure Models and Criteria for FRP Under In-Plane or Three-Dimensional Stress States Including Shear Non-Linearity; NASA/TM-2005-213530; NASA: Washington, DC, USA, 2005; p. 68.
- Pinho, S.T.; Darvizeh, R.; Robinson, P.; Schuecker, C.; Camanho, P.P. Material and Structural Response of Polymer-Matrix Fibre-Reinforced Composites. J. Compos. Mater. 2012, 46, 2313–2341. [Google Scholar] [CrossRef]
- ISO 527-2:2012; Plastics—Determination of Tensile Properties Part 2: Test Conditions for Moulding and Extrusion Plastics. ISO: Geneva, Switzerland, 2012.
- Vedrtnam, A.; Ghabezi, P.; Gunwant, D.; Jiang, Y.; Sam-Daliri, O.; Harrison, N.; Goggins, J.; Finnegan, W. Mechanical Performance of 3D-Printed Continuous Fibre Onyx Composites for Drone Applications: An Experimental and Numerical Analysis. Compos. Part C Open Access 2023, 12, 100418. [Google Scholar] [CrossRef]
- Ghebretinsae, F.; Mikkelsen, O.; Akessa, A.D. Strength Analysis of 3D Printed Carbon Fibre Reinforced Thermoplastic Using Experimental and Numerical Methods. IOP Conf. Ser. Mater. Sci. Eng. 2019, 700, 012024. [Google Scholar] [CrossRef]
- Yun, J.H.; Yoon, G.W.; Jeon, Y.J.; Kang, M.S. Evaluation of the Properties of 3D-Printed Onyx–Fiberglass Composites. Materials 2024, 17, 4140. [Google Scholar] [CrossRef]
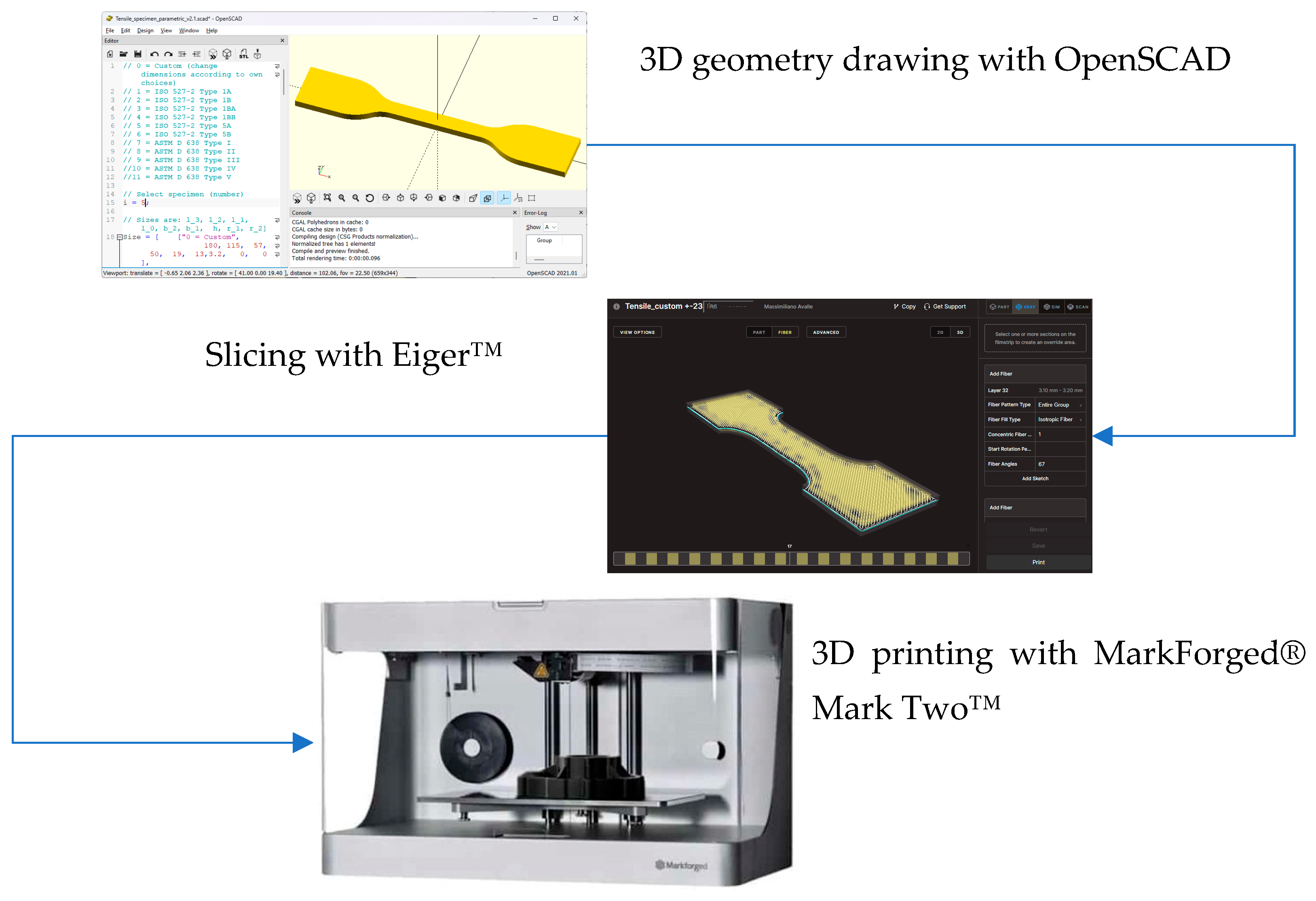



| Material | Onyx | Glass Fiber |
|---|---|---|
| Elastic modulus (GPa) | 2.4 | 21 |
| Yield strength (MPa) | 40 | - |
| Ultimate strength (MPa) | 37 | 590 |
| Deformation at failure (%) | 25 | 3.8 |
| Density | 1.2 | 1.5 |
| Angle | Lamination |
|---|---|
| 0° | 08 |
| 23° | [±23]4s |
| 45° | [±45]4s |
| 68° | [±68]4s |
| 90° | 908 |
| Angle | Modulus (MPa) | Strength (MPa) |
|---|---|---|
| 0° | 11842 ± 135 | 177 ± 2 |
| 22.5° | 8847 ± 63 | 120 ± 2 |
| 45° | 4978 ± 15 | 81 ± 6 |
| 67.5° | 4254 ± 53 | 71 ± 1 |
| 90° | 4249 ± 46 | 67 ± 4 |
| Property | Value |
|---|---|
| Elastic modulus along the fiber direction, Exx | 11.7 GPa |
| Transverse elastic modulus, Eyy | 4.3 GPa |
| Poisson’s ratio, νyx | 0.37 |
| Elastic tangential modulus, Gxy | 1.43 GPa8 |
| Property | Value |
|---|---|
| Tensile strength in the fiber direction, Xt | 178 MPa |
| Tensile strength in the transverse direction, Yt | 67.9 MPa |
| In-plane shear strength, S | 51.1 MPa |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Avalle, M.; Frascio, M. Mechanical Characterization and Modeling of Glass Fiber-Reinforced Polyamide Built by Additive Manufacturing. Materials 2025, 18, 745. https://doi.org/10.3390/ma18040745
Avalle M, Frascio M. Mechanical Characterization and Modeling of Glass Fiber-Reinforced Polyamide Built by Additive Manufacturing. Materials. 2025; 18(4):745. https://doi.org/10.3390/ma18040745
Chicago/Turabian StyleAvalle, Massimiliano, and Mattia Frascio. 2025. "Mechanical Characterization and Modeling of Glass Fiber-Reinforced Polyamide Built by Additive Manufacturing" Materials 18, no. 4: 745. https://doi.org/10.3390/ma18040745
APA StyleAvalle, M., & Frascio, M. (2025). Mechanical Characterization and Modeling of Glass Fiber-Reinforced Polyamide Built by Additive Manufacturing. Materials, 18(4), 745. https://doi.org/10.3390/ma18040745







