Dynamic Responses and Crack Propagation of Rock with Crossed Viscoelastic Joints Under Blasting Loads
Highlights
- Viscoelastic joints significantly attenuate stress wave propagation in rock masses.
- Joint thickness and angle critically influence rock damage patterns under blasting.
- Crack propagation at joint tips is driven by principal stress differences.
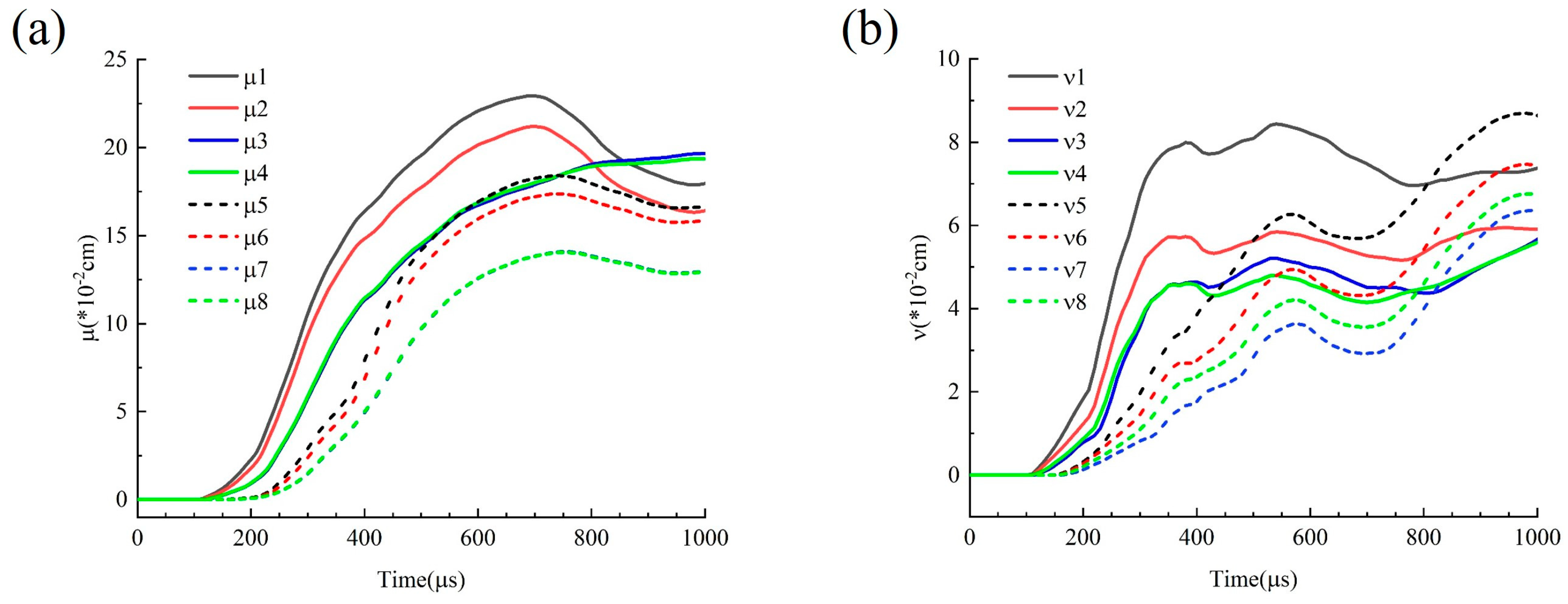
- Vibration velocity is notably weakened at the second joint interface.
Abstract
1. Introduction
2. Fundamental Principles of Blasting Simulation
2.1. RHT Model
2.2. Blasting Load Analysis
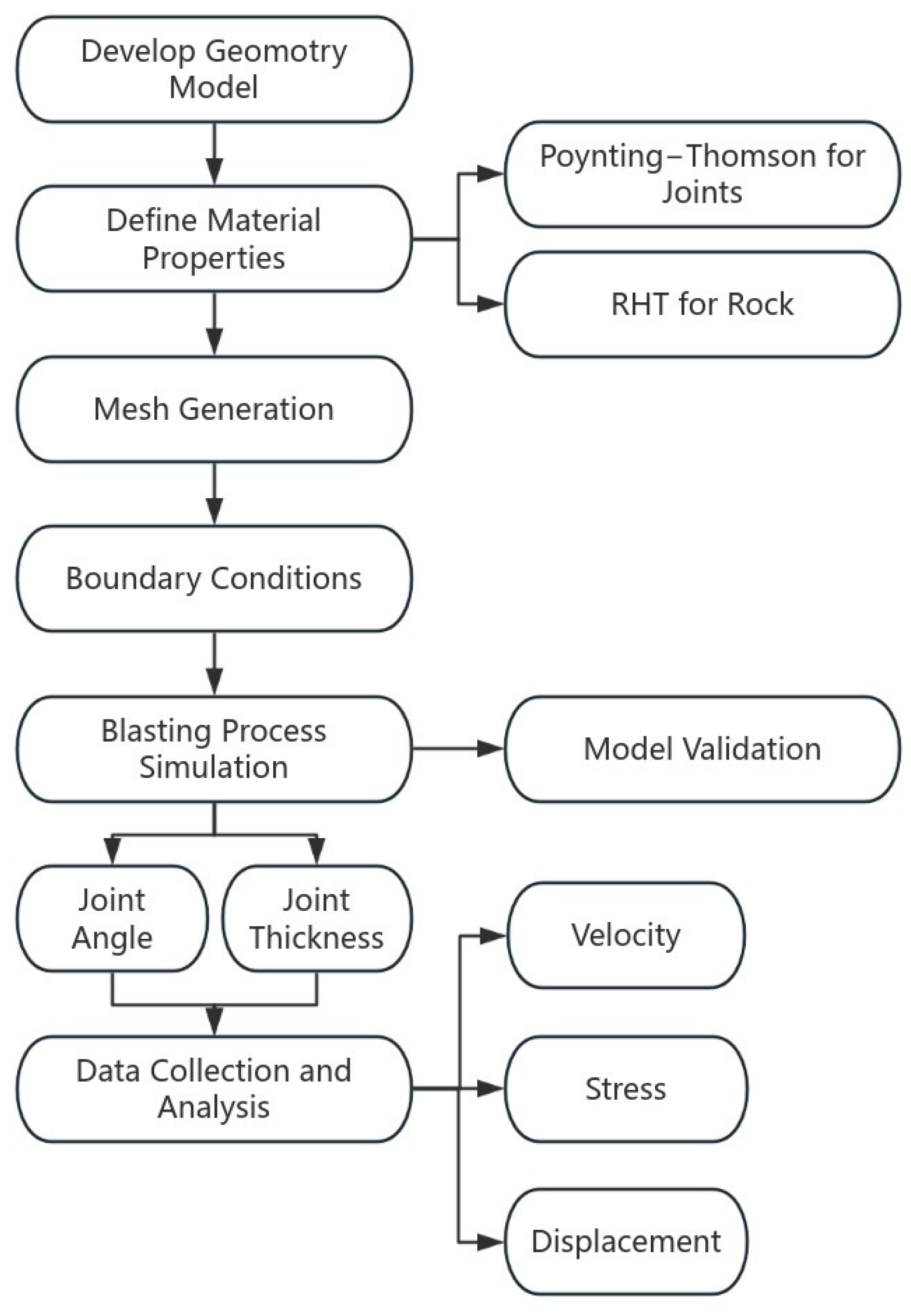
3. Establishment of Numerical Model
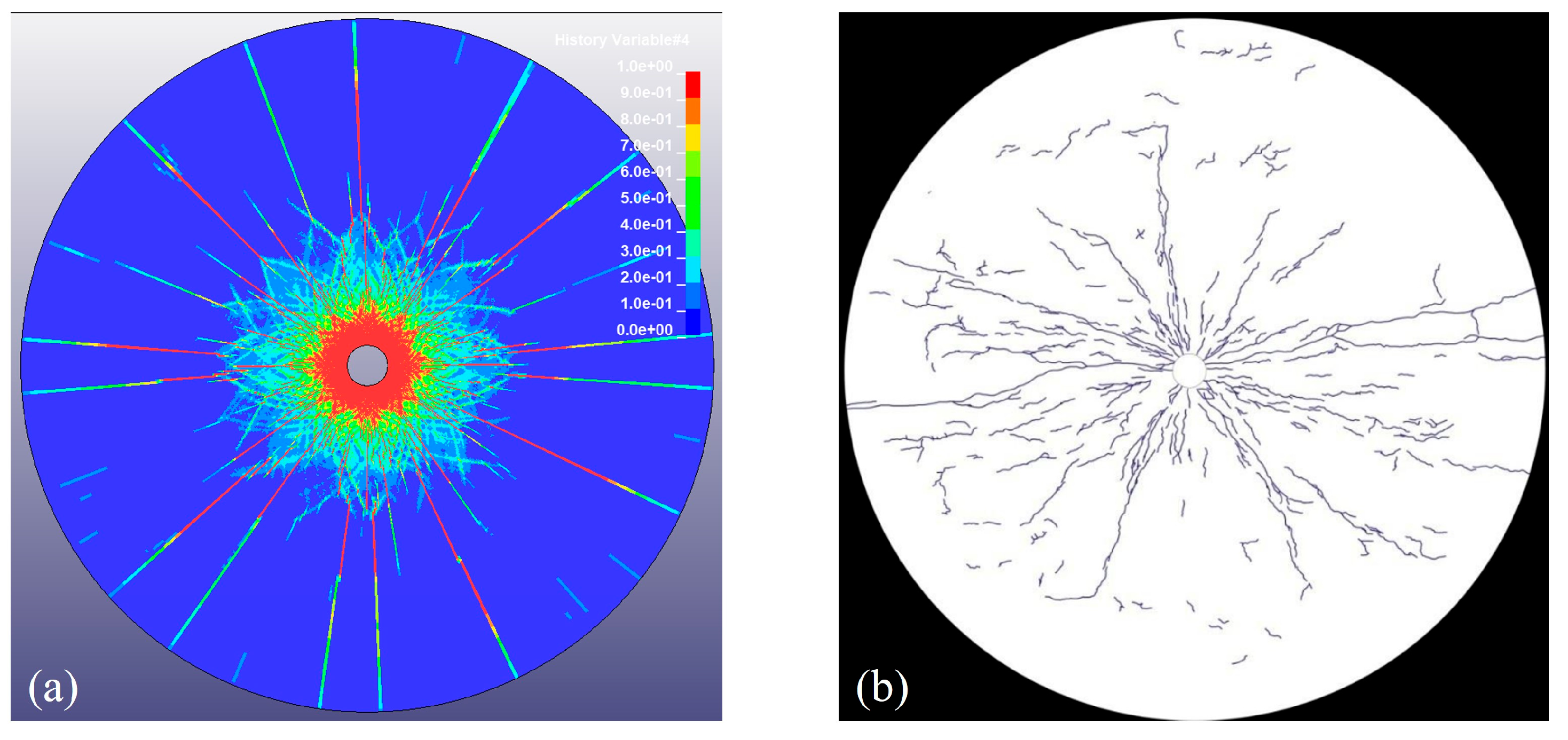
3.1. Model Parameters and Verification
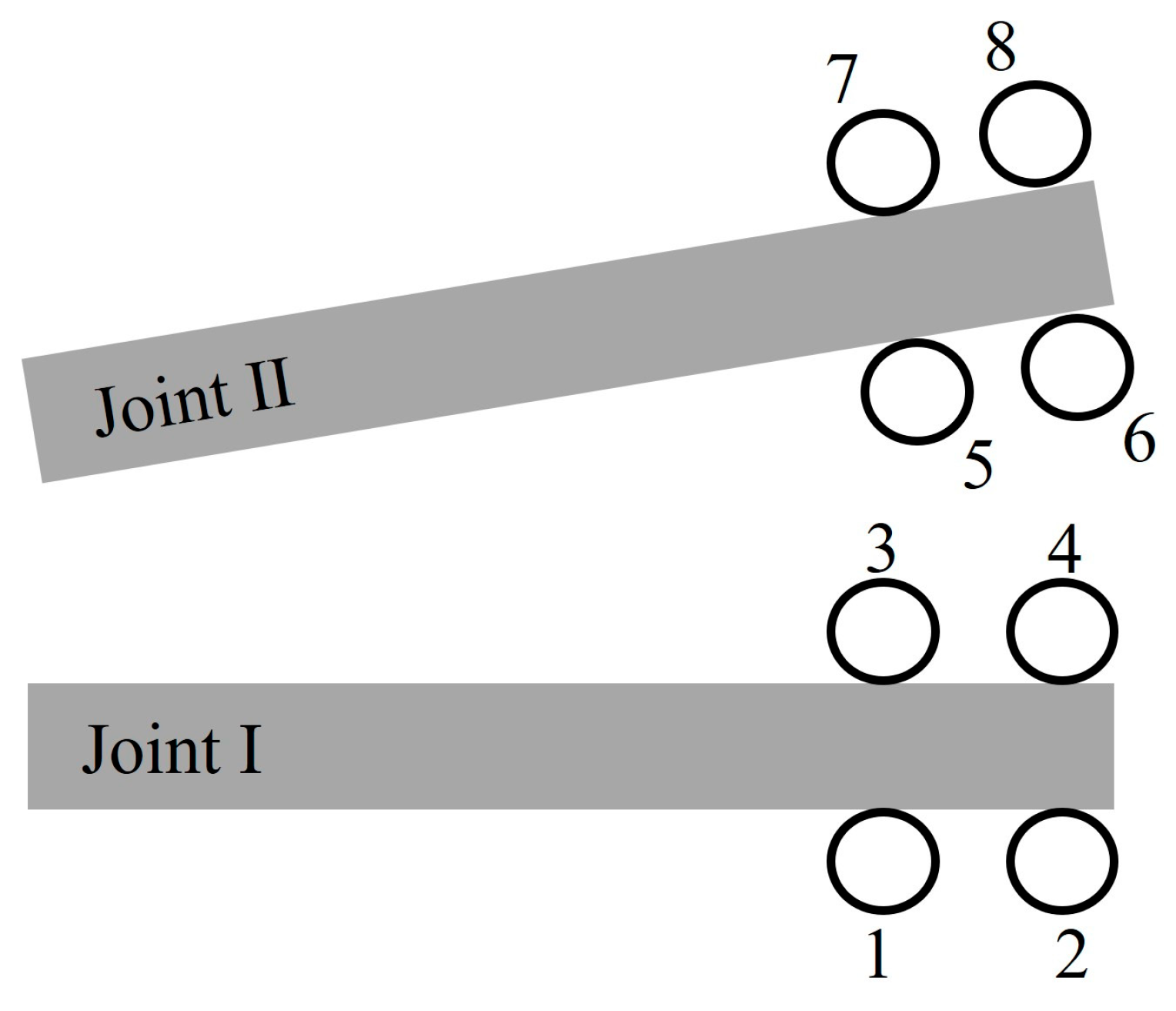
3.2. Geometric Model and Mesh Division
4. Numerical Results
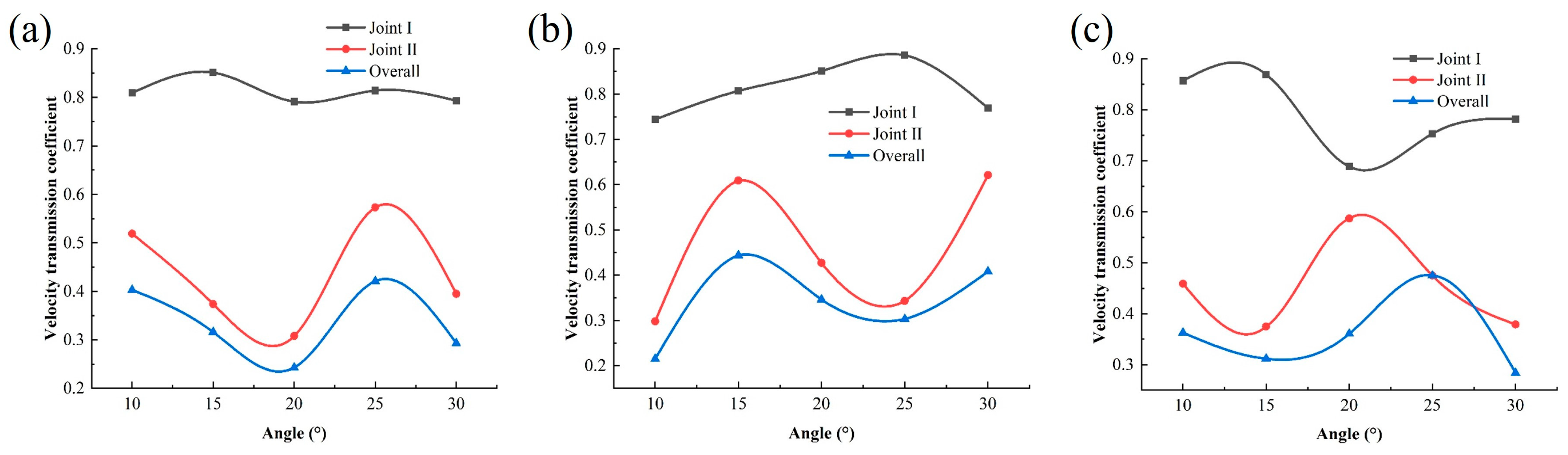
4.1. The Effect of Joint Angle θ on Stress Wave Propagation
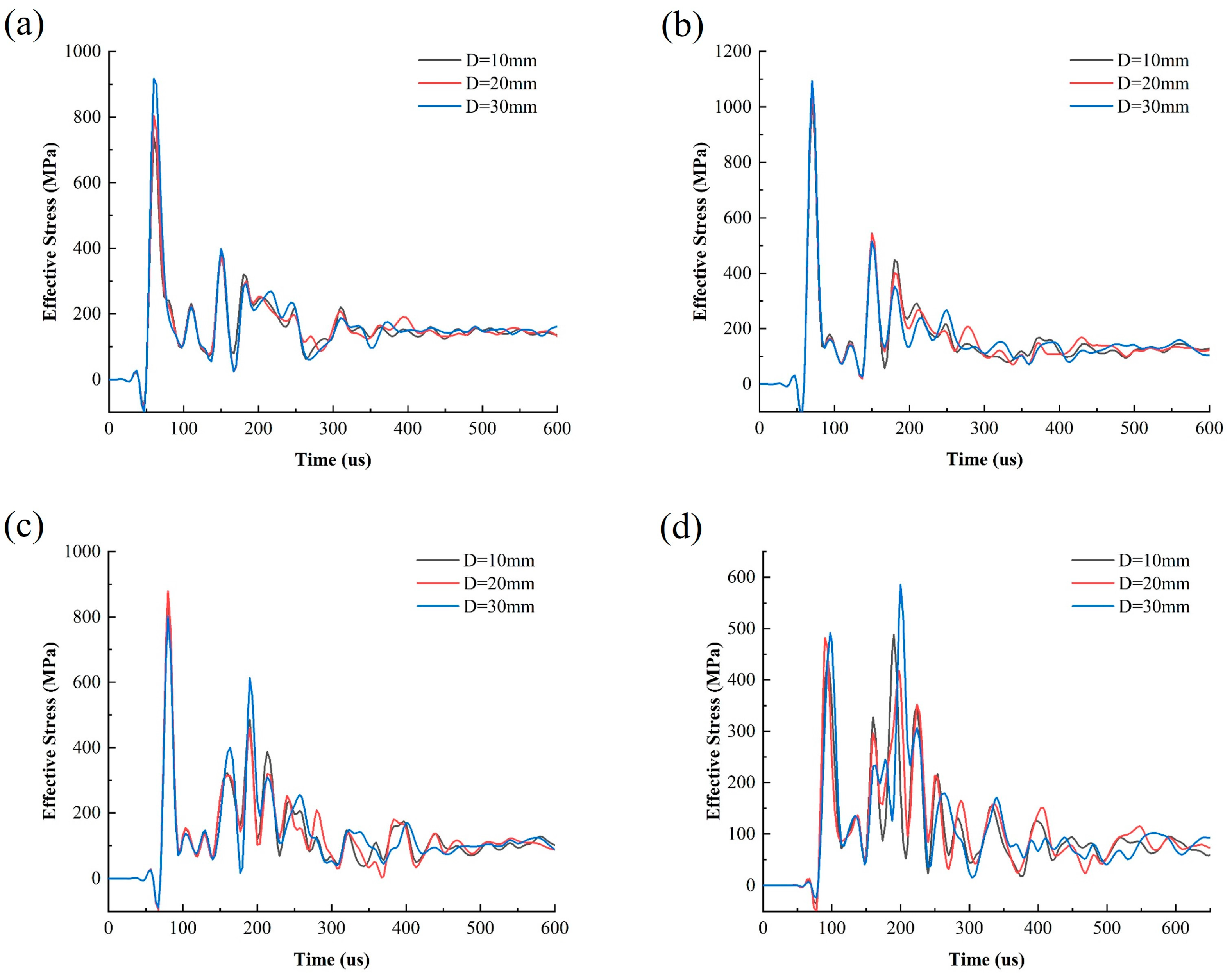
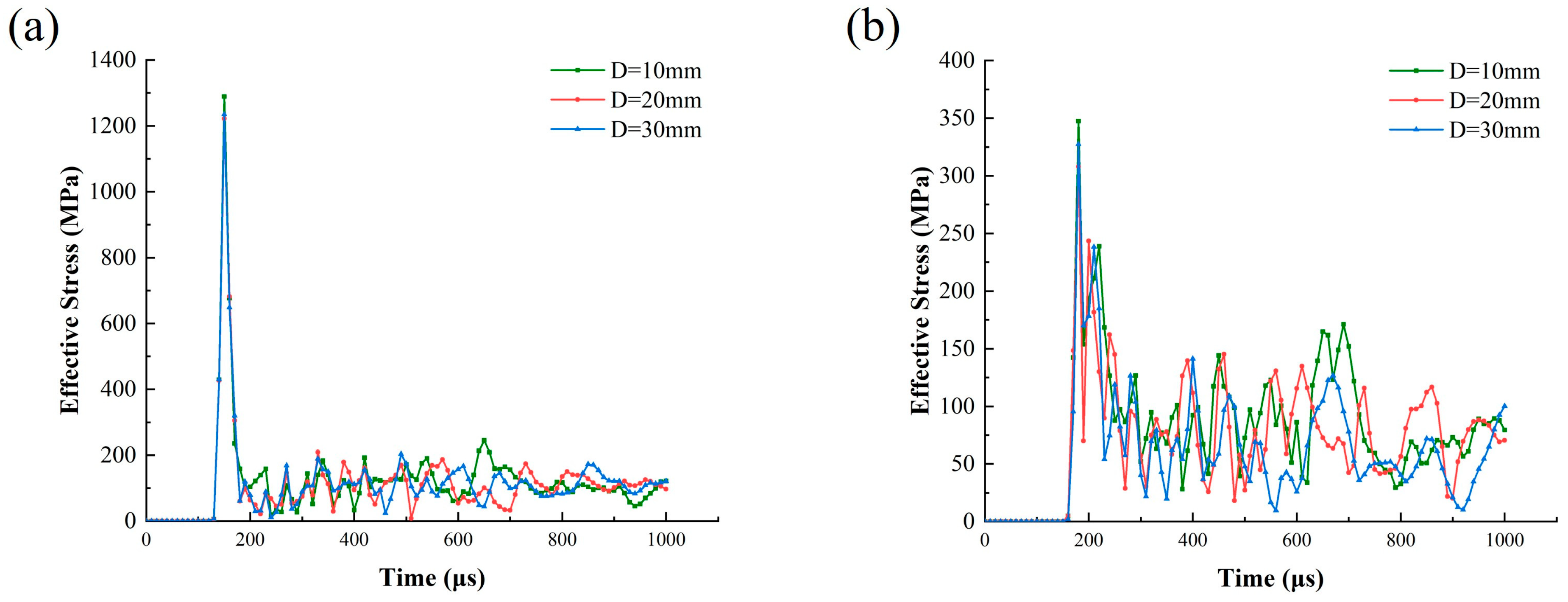
4.2. The Effect of Joint Thickness D on Stress Wave Propagation
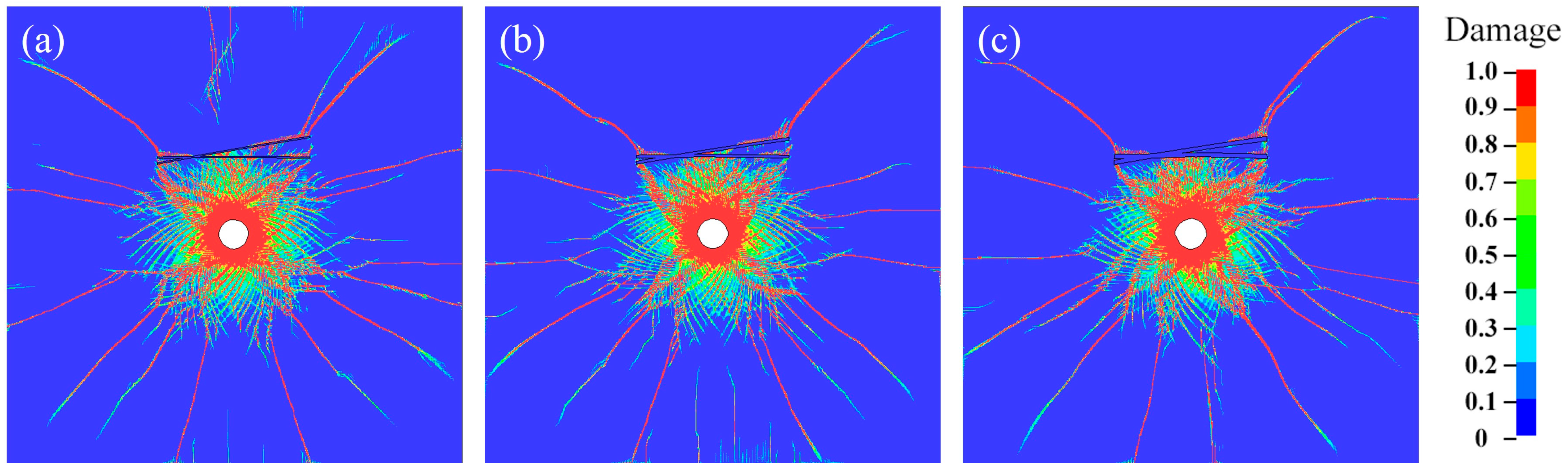
4.3. Extension of the Joints Under Blasting
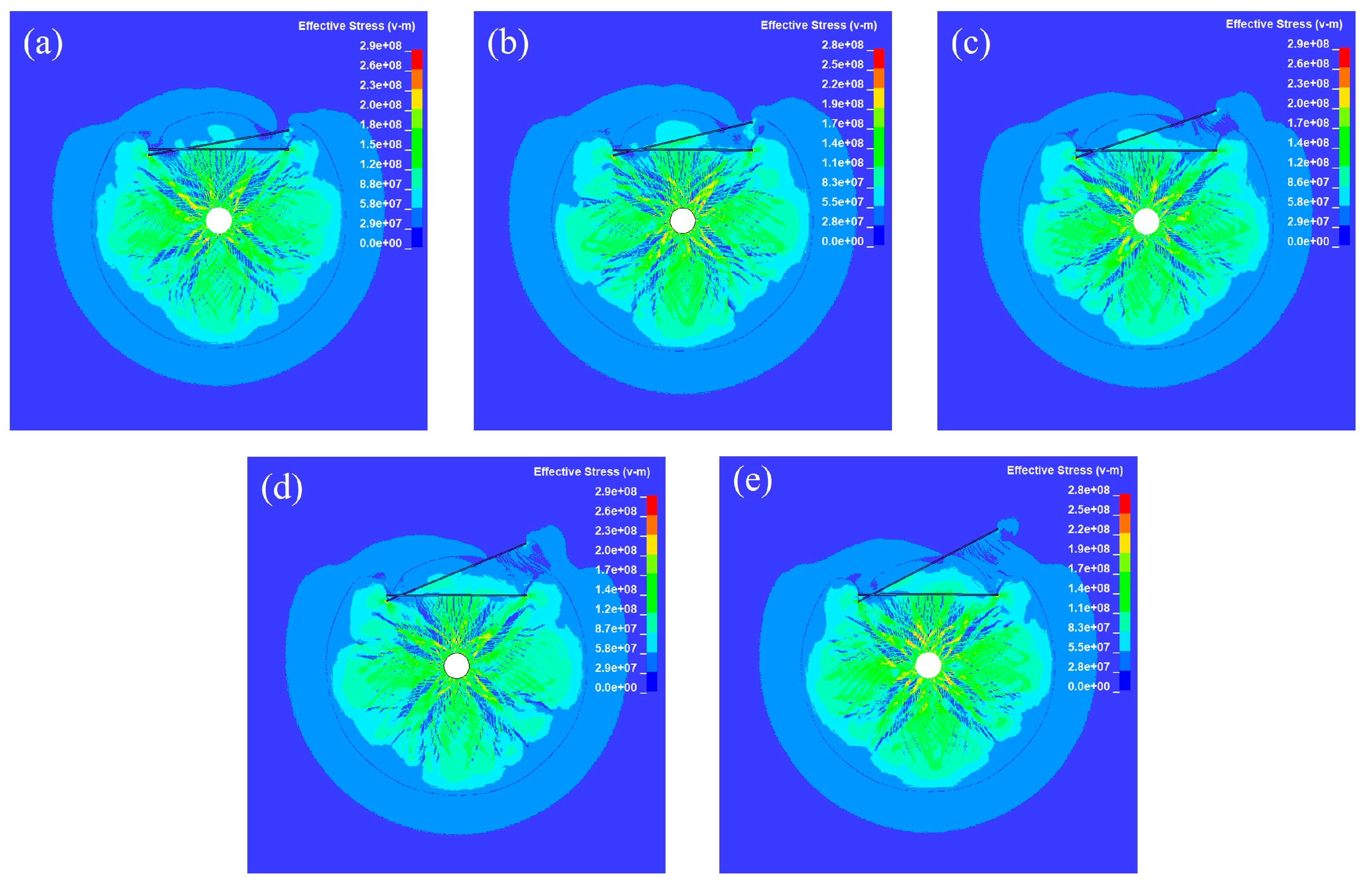
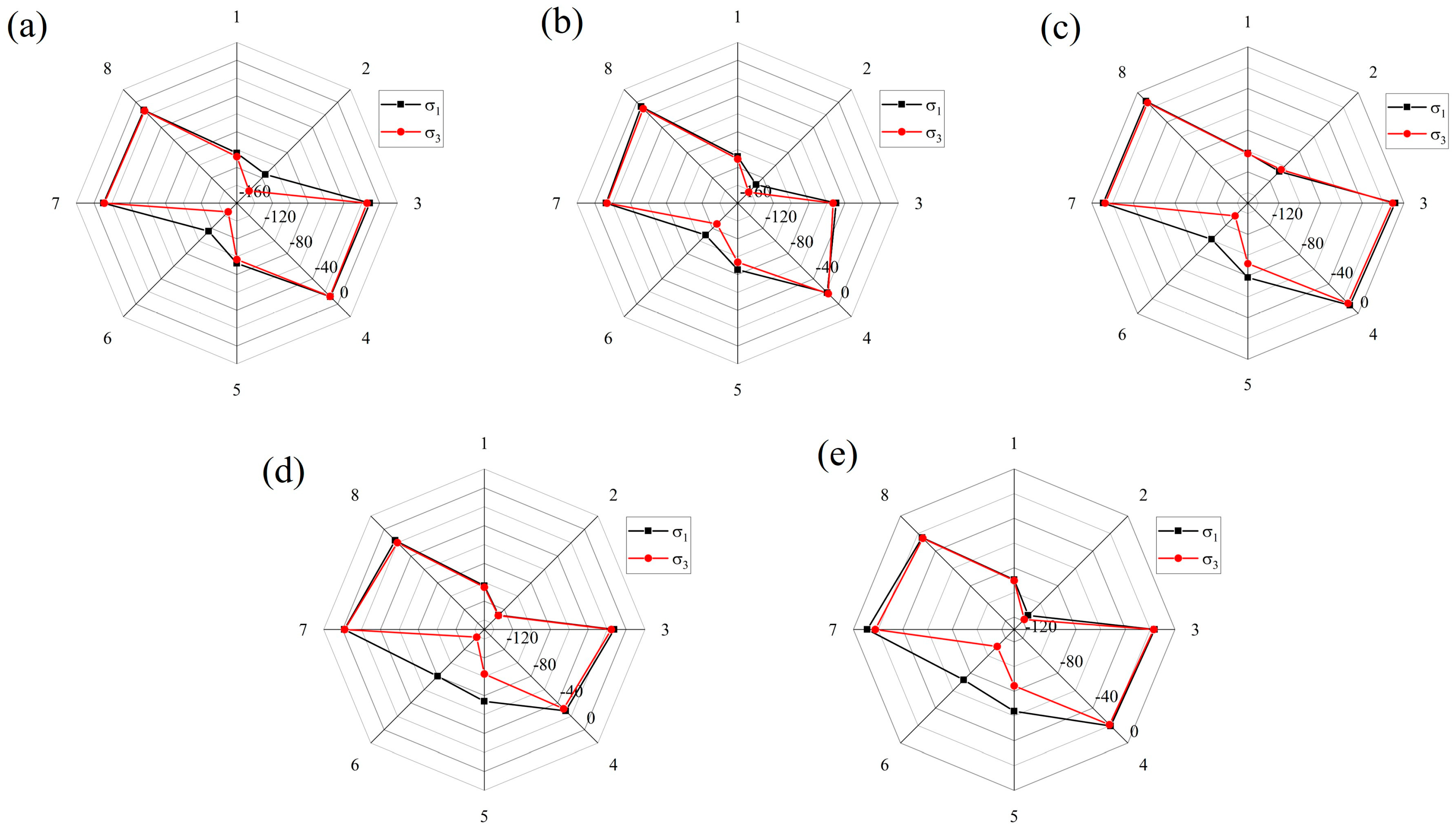
4.3.1. Distribution of Principal Stresses Around the Tip of the Joints
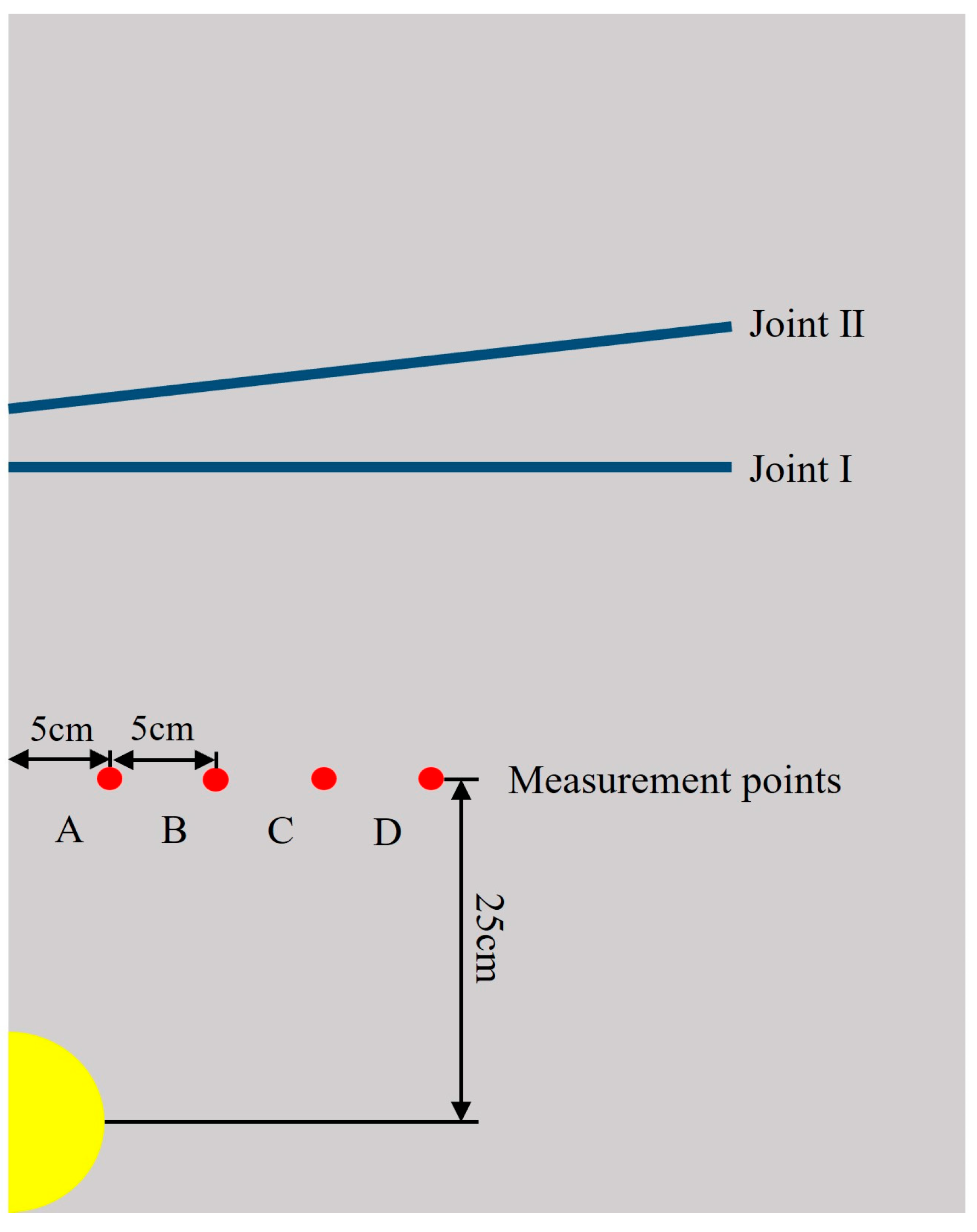
4.3.2. Displacements Around the Tip of Joints
5. Conclusions
- (1)
- The presence of intersecting viscoelastic joints in the rock mass tends to cause a stress concentration, with the intensity inversely proportional to the joint thickness D. At the same time, viscoelastic joints significantly attenuate and obstruct the propagation of blast stress waves. The extent of attenuation grows with the number of joints.
- (2)
- Under explosive loading, the initial cracks at the intersections of crossed joints in the rock mass are all wing cracks. The larger the joint filling thickness D, the better the blasting effect on the rock mass at the blast-facing side of Joint I, and the worse the effect on the back side. The extension of wing cracks at Joint II is primarily determined by θ. As θ increases, the cracks on the back side of the joint become fewer and thinner, and the rock failure mode shifts from tensile failure to shear-tensile failure. Owing to the reflected waves in front of Joint I, the dense cracks in the crush zone surrounding the borehole extend to the joint surface, creating longitudinal cracks in the joint and connecting with the transverse cracks, resulting in improved fragmentation in that area.
- (3)
- The larger θ and D, the more pronounced the effect of the joints on wave speed. When the joint is thin, stress waves are predominantly transmitted. When the joint is thick, reflection becomes the primary mode of wave interaction. Among them, Joint II primarily plays a role in reducing the stress wave velocity, accounting for an average of 68.9% of the overall weakening effect. The difference in principal stress on the blast-facing side of the joint increases with θ, showing increases of 7.5 MPa and 16.3 MPa, which is strongly correlated with the rock mass damage.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| TDRM | time-domain recursive method |
| SPH | smoothed particle hydrodynamics |
| FEM | finite element method |
| DEM | discrete element method |
| DSIF | dynamic stress intensity factor |
| DIC | digital image correlation |
| RHT | Riedel–Hiermaier–Thoma |
| JWL | Jones-Wilkins-Lee |
References
- Li, J.; Fan, L.; Li, Z. Progress of methods for wave propagation across rock masses. Chin. J. Appl. Mech. 2022, 39, 845–858. [Google Scholar] [CrossRef]
- Yi, C.P.; Johansson, D.; Greberg, J. Effects of in-situ stresses on the fracturing of rock by blasting. Comput. Geotech. 2018, 104, 321–330. [Google Scholar] [CrossRef]
- Zhao, J. Modelling of crack growth using a new fracture criteria based peridynamics. J. Appl. Comput. Mech. 2019, 5, 498–516. [Google Scholar] [CrossRef]
- Li, J.; Ma, G. Analysis of blast wave interaction with a rock joint. Rock Mech. Rock Eng. 2010, 43, 777–787. [Google Scholar] [CrossRef]
- Liu, T.; Li, X.; Zheng, Y.; Luo, Y.; Guo, Y.; Cheng, G.; Zhang, Z. Study on S-wave propagation through parallel rock joints under in situ stress. Wave Random Complex Media 2022, 32, 1174–1197. [Google Scholar] [CrossRef]
- Chai, S.B.; Li, J.C.; Zhang, Q.B.; Li, H.B.; Li, N.N. Stress wave propagation across a rock mass with two non-parallel joints. Rock Mech. Rock Eng. 2016, 49, 4023–4032. [Google Scholar] [CrossRef]
- Chai, S.; Tian, W.; Zhao, J. Study of the cylindrical wave propagation across a single rock joint with nonlinear normal deformation. Wave Random Complex Media 2021, 31, 1505–1522. [Google Scholar] [CrossRef]
- Li, J.; Ma, G.; Zhao, J. An equivalent viscoelastic model for rock mass with parallel joints. J. Geophys. Res. Solid Earth 2010, 115, B3. [Google Scholar] [CrossRef]
- Zhu, J.; Perino, A.; Zhao, G.F.; Barla, G.; Li, J.C.; Ma, G.W.; Zhao, J. Seismic response of a single and a set of filled joints of viscoelastic deformational behaviour. Geophys. J. Int. 2011, 186, 1315–1330. [Google Scholar] [CrossRef]
- Wang, S.M.; Wang, Z.L.; Jia, S.L.; Wang, H.R.; Wang, H.C. Analysis of propagation characteristics of stress waves in viscoelastic jointed rock mass. J. Harbin Inst. Tech. 2022, 54, 99–107. [Google Scholar] [CrossRef]
- Zhu, J.B.; Deng, X.F.; Zhao, X.; Zhao, J. A numerical study on wave transmission across multiple intersecting joint sets in rock masses with UDEC. Rock Mech. Rock Eng. 2013, 46, 1429–1442. [Google Scholar] [CrossRef]
- Liu, T.T.; Zeng, L.L.; Zhang, C.; Yang, T. Study on the influence of joint distribution on dynamic mechanical characteristics and failure mode of cross-jointed rock mass. Chin. J. Rock Mech. Eng. 2023, 43, 90–102. [Google Scholar]
- Chang, L.; Konietzky, H.; Frühwirt, T. Strength anisotropy of rock with crossing joints: Results of physical and numerical modeling with gypsum models. Rock Mech. Rock Eng. 2019, 52, 2293–2317. [Google Scholar] [CrossRef]
- Wu, X.; Zhang, L.; Sun, J.; Guo, Q.; Pan, J.; Gao, J. Strength characteristics and failure mechanism of granite with cross cracks at different angles based on DIC method. Adv. Mater. Sci. Eng. 2022, 2022, 9144673. [Google Scholar] [CrossRef]
- Zhou, W.H.; Hu, C.Z.; Bao, J.; Zheng, J.J.; Liang, R. Numerical study on crack propagation and stress wave propagation during blasting of jointed rock mass. Chin. J. Theor. Appl. Mech. 2022, 54, 2501–2512. [Google Scholar] [CrossRef]
- Ding, X.; Yang, Y.; Zhou, W.; An, W.; Li, J.; Ebelia, M. The law of blast stress wave propagation and fracture development in soft and hard composite rock. Sci. Rep. 2022, 12, 17120. [Google Scholar] [CrossRef]
- Zhu, J.B.; Zhao, G.F.; Zhao, X.B.; Zhao, J. Validation study of the distinct lattice spring model (DLSM) on P-wave propagation across multiple parallel joints. Comput. Geotech. 2011, 38, 298–304. [Google Scholar] [CrossRef]
- Jayasinghe, L.; Shang, J.; Zhao, Z.; Goh, A. Numerical investigation into the blasting-induced damage characteristics of rocks considering the role of in-situ stresses and discontinuity persistence. Comput. Geotech. 2019, 116, 103207. [Google Scholar] [CrossRef]
- Wang, Z.; Konietzky, H. Modelling of blast-induced fractures in jointed rock masses. Eng. Fract. Mech. 2009, 76, 1945–1955. [Google Scholar] [CrossRef]
- Shang, J.; West, L.J.; Hencher, S.R.; Zhao, Z. Geological discontinuity persistence: Implications and quantification. Eng. Geol. 2018, 241, 41–54. [Google Scholar] [CrossRef]
- Luo, G.; Qi, S.; Zheng, B. Rate effect on the direct shear behavior of granite rock bridges at low to subseismic shear rates. J. Geophys. Res. Solid Earth 2022, 127, e2022JB024348. [Google Scholar] [CrossRef]
- Elmo, D.; Donati, D.; Stead, D. Challenges in the characterisation of intact rock bridges in rock slopes. Eng. Geol. 2018, 245, 81–96. [Google Scholar] [CrossRef]
- Zou, C.; Wong, L.N.Y. Experimental studies on cracking processes and failure in marble under dynamic loading. Eng. Geol. 2014, 173, 19–31. [Google Scholar] [CrossRef]
- Zou, C.; Wong, L.N.Y.; Loo, J.J.; Gan, B.S. Different mechanical and cracking behaviors of single-flawed brittle gypsum specimens under dynamic and quasi-static loadings. Eng. Geol. 2016, 201, 71–84. [Google Scholar] [CrossRef]
- Jiang, X.; Xue, Y.; Kong, F.; Gong, H.; Fu, Y.; Zhang, W. Dynamic responses and damage mechanism of rock with discontinuity subjected to confining stresses and blasting loads. Int. J. Impact Eng. 2023, 172, 104404. [Google Scholar] [CrossRef]
- Yilmaz, O.; Unlu, T. Three-dimensional numerical rock damage analysis under blasting load. Tunn. Undergr. Space Tech. 2013, 38, 266–278. [Google Scholar] [CrossRef]
- Liu, L.W.; Li, H.B.; Li, X.F.; Wu, D.; Zhang, G.K. Underlying mechanisms of crack initiation for granitic rocks containing a single pre-existing flaw: Insights from digital image correlation (DIC) analysis. Rock Mech. Rock Eng. 2021, 54, 857–873. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, H.; Wang, J.; Tian, N. Finite element analyses of constitutive models performance in the simulation of blast-induced rock cracks. Comput. Geotech. 2021, 135, 104172. [Google Scholar] [CrossRef]
- Xie, L.; Lu, W.; Zhang, Q.; Jiang, Q.; Chen, M.; Zhao, J. Analysis of damage mechanisms and optimization of cut blasting design under high in-situ stresses. Tunn. Undergr. Space Technol. 2017, 66, 19–33. [Google Scholar] [CrossRef]
- Banadaki, M.M.D. Stress-Wave Induced Fracture in Rock Due to Explosive Action. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2010. [Google Scholar]
- Yang, R.; Chen, C.; Xu, P.; Ding, C.; Zhang, Z. Experimental investigation of obliquely incident blast wave effect on deflection of running cracks. J. Test. Eval. 2021, 49, 866–880. [Google Scholar] [CrossRef]
- Li, J.; Liu, T.; Li, H.; Liu, Y.; Liu, B.; Xia, X. Shear wave propagation across filled joints with the effect of interfacial shear strength. Rock Mech. Rock Eng. 2015, 48, 1547–1557. [Google Scholar] [CrossRef]



| ρ (kg·m−3) | D (m·s−1) | PCJ (GPa) | A (GPa) | B (GPa) | R1 | R2 | ω | E0 (GPa) |
|---|---|---|---|---|---|---|---|---|
| 1320 | 6690 | 16 | 586 | 21.6 | 5.81 | 1.77 | 0.282 | 7.38 |
| ρ (kg·m−3) | G (GPa) | (MPa) | (MPa) | (GPa) |
|---|---|---|---|---|
| 2700 | 24.17 | 119 | 40 | 6 |
| ρ (kg·m−3) | C4 | C5 | E0 (GPa) |
|---|---|---|---|
| 1.29 | 0.4 | 0.4 | 0.025 |
| ρ (kg·m−3) | E (GPa) | (GPa) | (GPa) | |
|---|---|---|---|---|
| 1160 | 0.585 | 0.591 | 0.126 | 7 × 10−4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Jiang, D.; Zhao, J.; Zhang, T.; Kuang, R. Dynamic Responses and Crack Propagation of Rock with Crossed Viscoelastic Joints Under Blasting Loads. Materials 2025, 18, 548. https://doi.org/10.3390/ma18030548
Li C, Jiang D, Zhao J, Zhang T, Kuang R. Dynamic Responses and Crack Propagation of Rock with Crossed Viscoelastic Joints Under Blasting Loads. Materials. 2025; 18(3):548. https://doi.org/10.3390/ma18030548
Chicago/Turabian StyleLi, Chengyang, Dongju Jiang, Jinhai Zhao, Tuo Zhang, and Renfei Kuang. 2025. "Dynamic Responses and Crack Propagation of Rock with Crossed Viscoelastic Joints Under Blasting Loads" Materials 18, no. 3: 548. https://doi.org/10.3390/ma18030548
APA StyleLi, C., Jiang, D., Zhao, J., Zhang, T., & Kuang, R. (2025). Dynamic Responses and Crack Propagation of Rock with Crossed Viscoelastic Joints Under Blasting Loads. Materials, 18(3), 548. https://doi.org/10.3390/ma18030548







