Wetting Transition from Wenzel to Cassie States: Thermodynamic Analysis
Abstract
1. Introduction
2. Methods
2.1. MD Simulations
2.2. CA Measurements
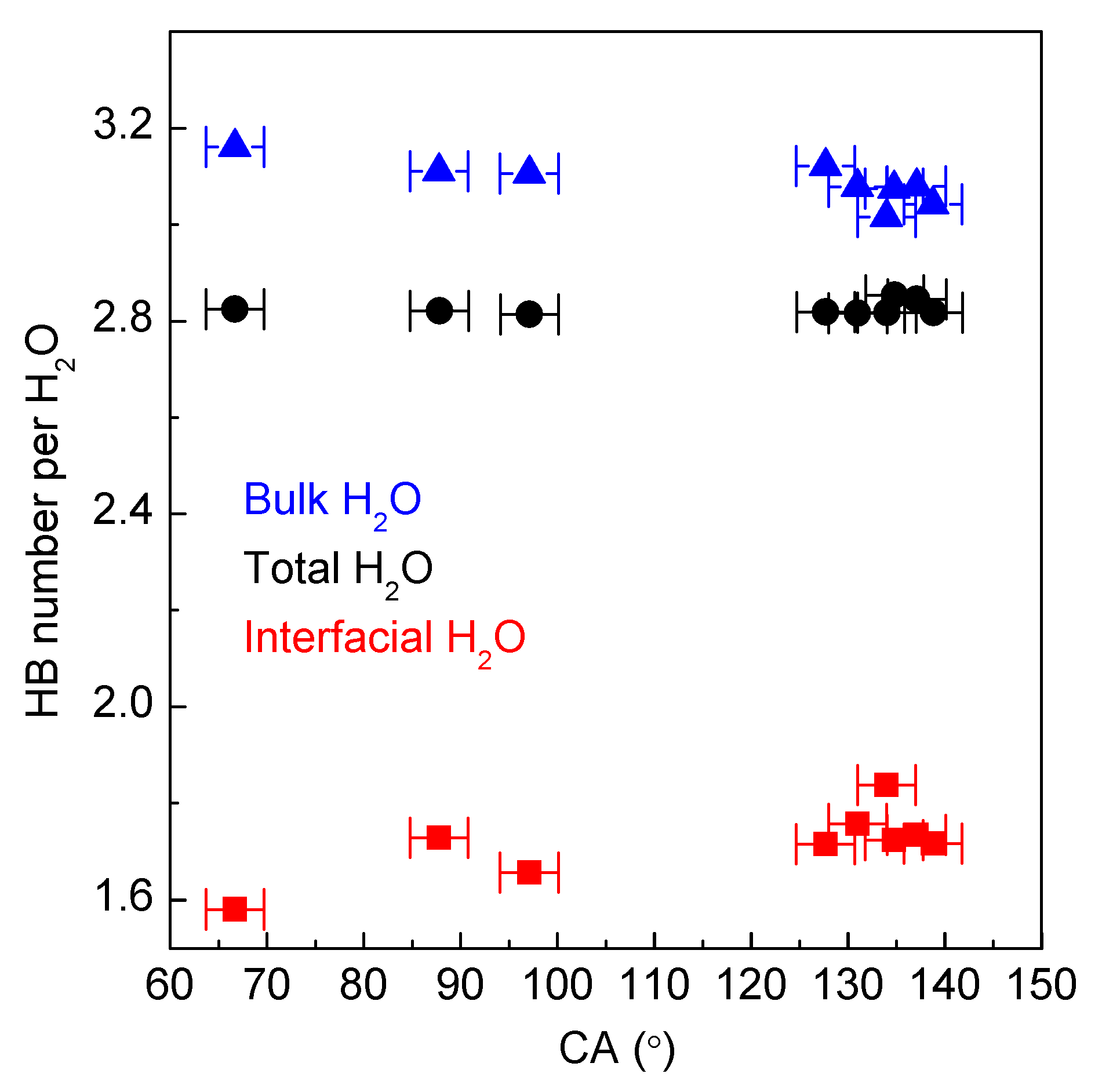
2.3. Hydrogen Bonding
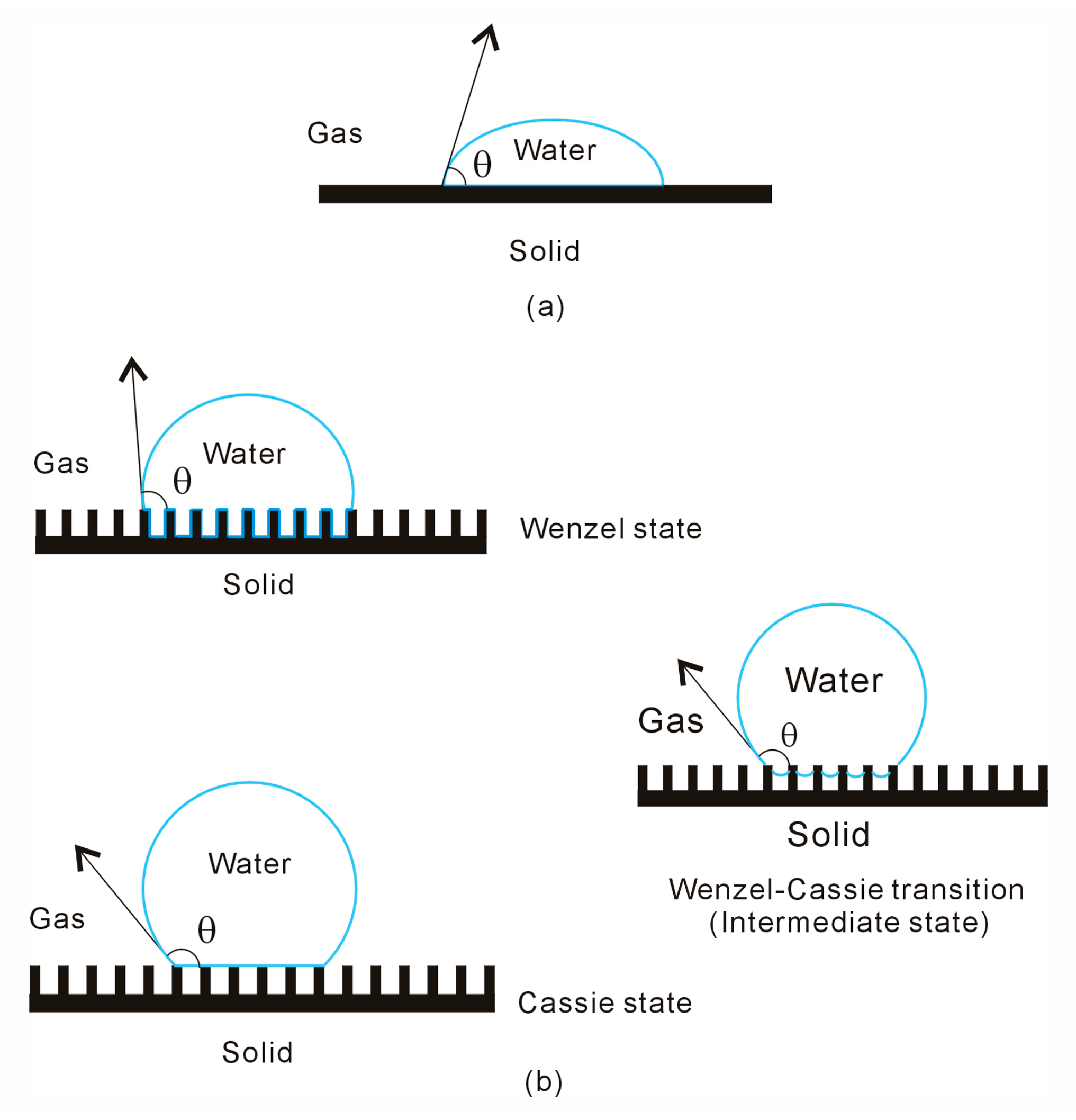
3. Results and Discussion
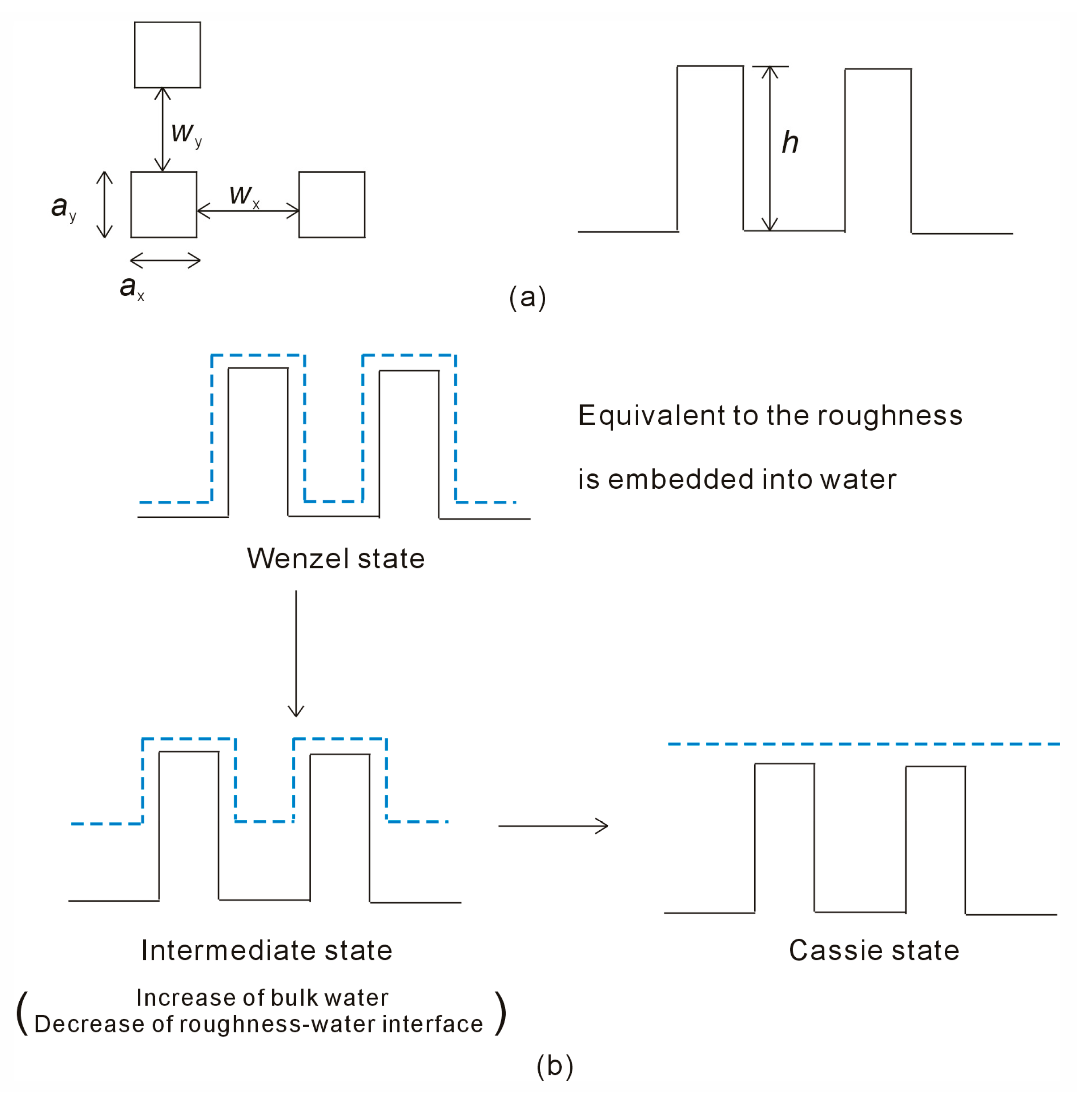
3.1. Thermodynamic Analysis of Wenzel–Cassie Transition
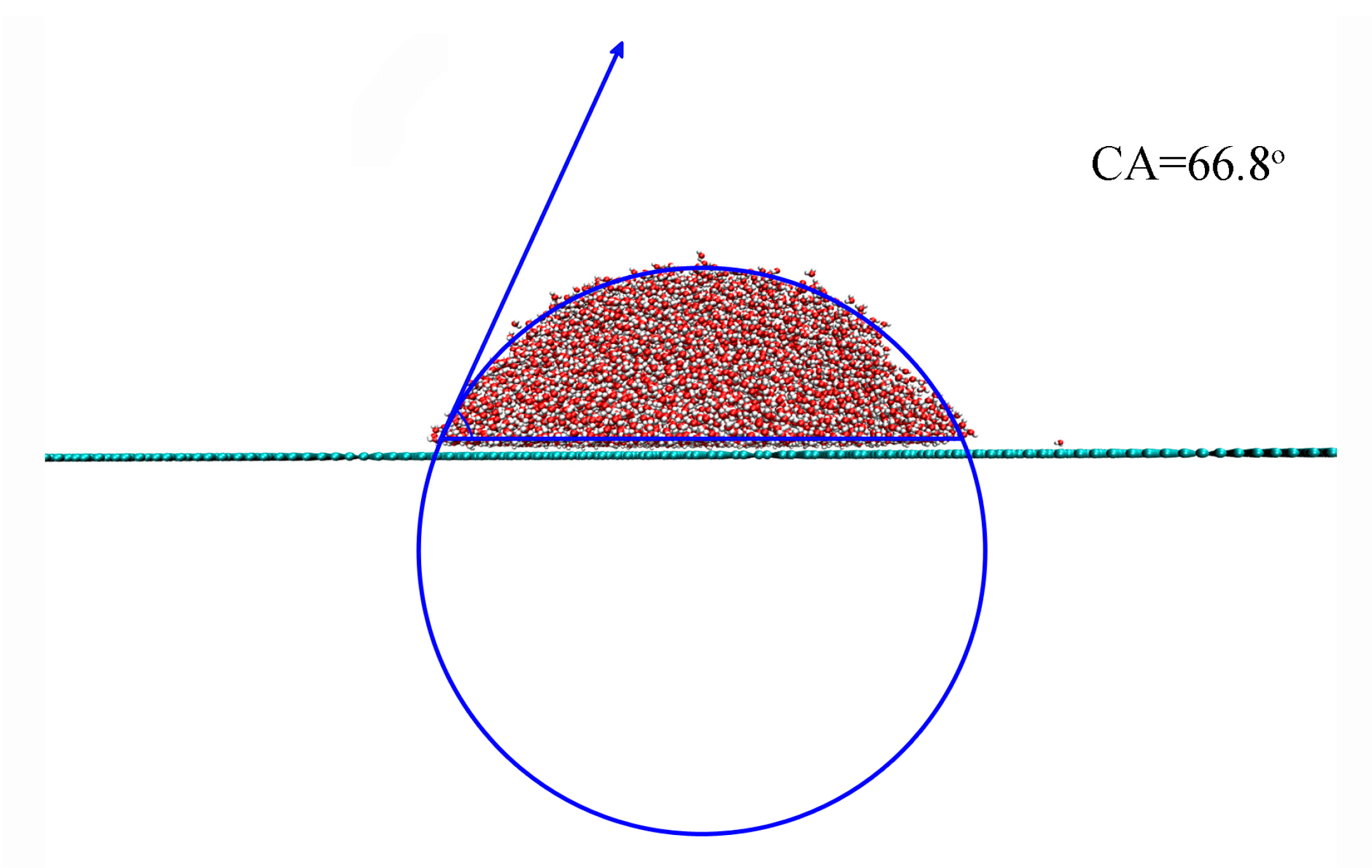
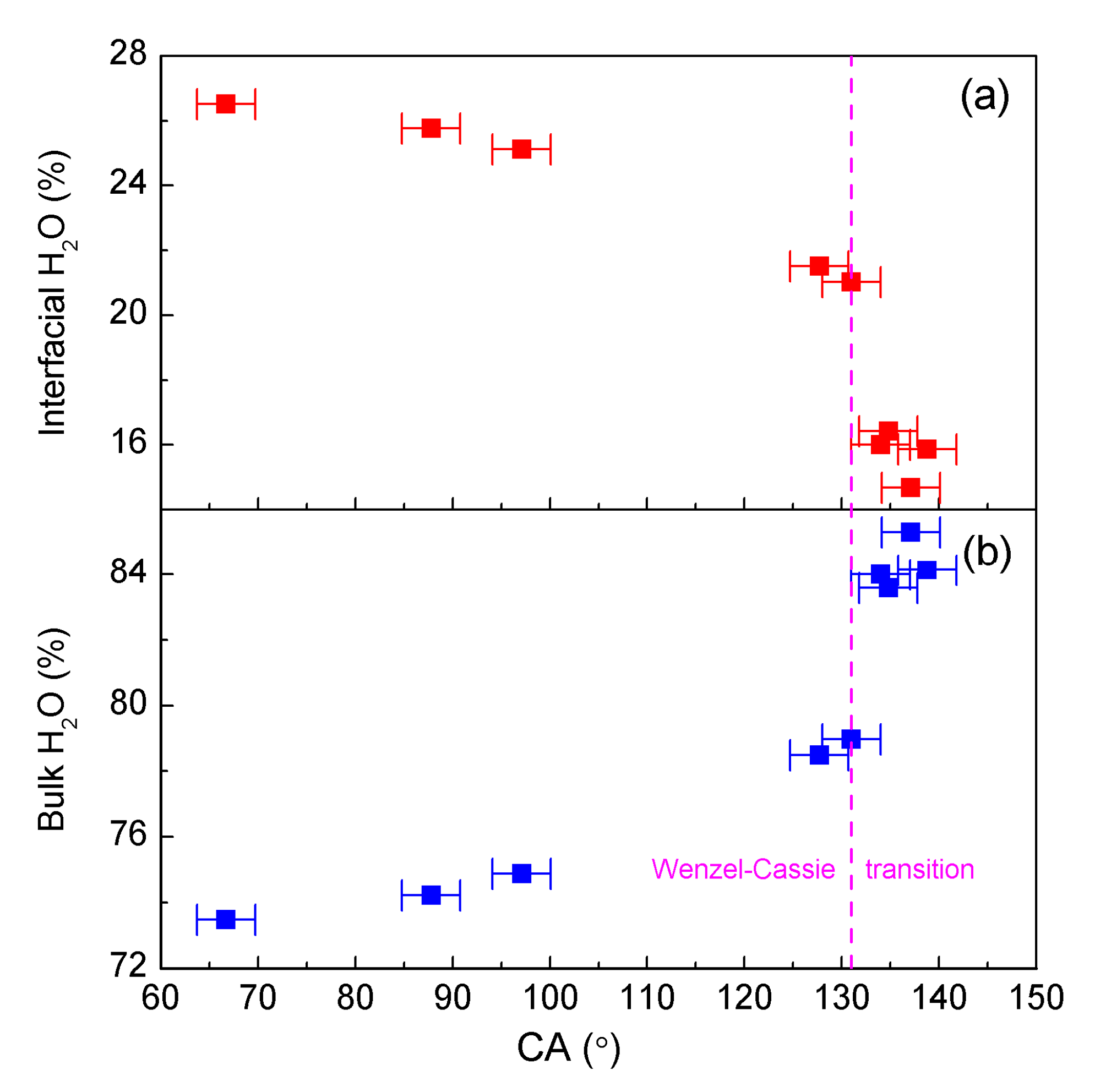
3.2. MD Simulations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, S.; Liu, K.; Yao, X.; Jiang, L. Bioinspired surfaces with superwettability: New insight on theory, design, and applications. Chem. Rev. 2015, 115, 8230–8293. [Google Scholar] [CrossRef] [PubMed]
- Su, B.; Tian, Y.; Jiang, L. Bioinspired interfaces with superwettability: From materials to chemistry. J. Am. Chem. Soc. 2016, 138, 1727–1748. [Google Scholar] [CrossRef] [PubMed]
- Luo, J.; Guo, Z. Recent advances in biomimetic superhydrophobic surfaces: Focusing on abrasion resistance, self-healing and anti-icing. Nanoscale 2024, 16, 16404–16419. [Google Scholar] [CrossRef]
- Cai, H.; Duan, C.; Fu, M.; Zhang, J.; Huang, H.; Hu, Y.; Shi, J.; Ye, D. Scalable fabrication of superhydrophobic coating with rough coral reef-like structures for efficient self-cleaning and oil-water separation: An experimental and molecular dynamics simulation study. Small 2023, 19, 2207118. [Google Scholar] [CrossRef]
- Khan, M.Z.; Militky, J.; Petru, M.; Tomková, B.; Ali, A.; Tören, E.; Perveen, S. Recent advances in superhydrophobic surfaces for practical applications: A review. Eur. Polym. J. 2022, 178, 111481. [Google Scholar] [CrossRef]
- Gateman, S.M.; Page, K.; Halimi, I.; Nascimento, A.R.C.; Savoie, S.; Schulz, R.; Moreau, C.; Parkin, I.P.; Mauzeroll, J. Corrosion of one-step superhydrophobic stainless-steel thermal spray coatings. ACS Appl. Mater. Interfaces 2020, 12, 1523–1532. [Google Scholar] [CrossRef]
- Nine, M.J.; Cole, M.A.; Johnson, L.; Tran, D.N.H.; Losic, D. Robust superhydrophobic graphene-based composite coatings with self-cleaning and corrosion barrier properties. ACS Appl. Mater. Interfaces 2015, 7, 28482–28493. [Google Scholar] [CrossRef]
- Lambley, H.; Graeber, G.; Vogt, R.; Gaugler, L.C.; Baumann, E.; Schutzius, T.M.; Poulikakos, D. Freezing-induced wetting transitions on superhydrophobic surfaces. Nat. Phys. 2023, 19, 649–655. [Google Scholar] [CrossRef]
- Hussain, S.; Muangnapoh, T.; Traipattanakul, B.; Lekmuenwai, M. Anti-icing property of superhydrophobic nanostructured brass via deposition of silica nanoparticles and nanolaser treatment. Nanomaterials 2023, 13, 1139. [Google Scholar] [CrossRef]
- Li, Y.; Jin, H.; Nie, S.; Zhang, P.; Gao, N. Dynamic behavior of water droplets and flashover characteristics on a superhydrophobic silicone rubber surface. Appl. Phys. Lett. 2017, 110, 201602. [Google Scholar] [CrossRef]
- Wang, Q.; Liu, S.; Liu, S.; Zuo, Z.; Gao, Y.; Wu, C.; Liang, X. Super-hydrophobic silicone rubber for outdoor electrical insulation. Nano Today 2024, 58, 102406. [Google Scholar] [CrossRef]
- Kota, A.K.; Kwon, G.; Choi, W.; Mabry, J.M.; Tuteja, A. Hygro-responsive membranes for effective oil–water separation. Nat. Commun. 2012, 3, 1025. [Google Scholar] [CrossRef]
- Gao, X.; Zhou, J.; Du, R.; Xie, Z.; Deng, S.; Liu, R.; Liu, Z.; Zhang, J. Robust superhydrophobic foam: A graphdiyne-based hierarchical architecture for oil/water separation. Adv. Mater. 2016, 28, 168–173. [Google Scholar] [CrossRef] [PubMed]
- Kang, L.; Wang, B.; Zeng, J.; Cheng, Z.; Li, J.; Xu, J.; Gao, W.; Chen, K. Degradable dual superlyophobic lignocellulosic fibers for high-efficiency oil/water separation. Green Chem. 2020, 22, 504–512. [Google Scholar] [CrossRef]
- Pang, Y.; Yu, Z.; Chen, H.; Xiang, Q.; Wang, Q.; Xie, C.; Liu, Y. Superhydrophobic polyurethane sponge based on sepiolite for efficient oil/water separation. J. Hazard. Mater. 2022, 434, 128833. [Google Scholar] [CrossRef]
- Young, T. An essay on the cohesion of fluids. Philos. Trans. R. Soc. Lond. 1805, 95, 65–87. [Google Scholar]
- Makkonen, L. Young’s equation revisited. J. Phys. Condens. Matter 2016, 28, 135001. [Google Scholar] [CrossRef]
- Whyman, G.; Bormashenko, E.; Stein, T. The rigorous derivation of Young, Cassie–Baxter and Wenzel equations and the analysis of the contact angle hysteresis phenomenon. Chem. Phys. Lett. 2008, 450, 355–359. [Google Scholar] [CrossRef]
- Makkonen, L. A thermodynamic model of contact angle hysteresis. J. Chem. Phys. 2017, 147, 064703. [Google Scholar] [CrossRef]
- Li, Y.; Liu, J.; Dong, J.; Du, Y.; Han, J.; Niu, Y. Theoretical analysis of contact angle and contact angle hysteresis of Wenzel drops on superhydrophobic surfaces. Nanomaterials 2024, 14, 1978. [Google Scholar] [CrossRef]
- Li, Y. An analytical approach for determining contact angle hysteresis on smooth, micropillared, and micropored homogeneous surfaces. J. Colloid Interface Sci. 2025, 679, 554–565. [Google Scholar] [CrossRef] [PubMed]
- Shafrin, E.G.; Zisman, W.A. Contact Angle, Wettability and Adhesion; Fowkes, F.M., Ed.; Advances in Chemistry Series; American Chemical Society: Washington, DC, USA, 1964; Volume 43, pp. 145–167. [Google Scholar]
- Barthlott, W.; Neinhuis, C. Purity of the sacred lotus, or escape from contamination in biological surfaces. Planta 1997, 202, 1–8. [Google Scholar] [CrossRef]
- Wenzel, R.N. Resistance of solid surfaces to wetting by water. Ind. Eng. Chem. 1936, 28, 988–994. [Google Scholar] [CrossRef]
- Wenzel, R.N. Surface roughness and contact angle. J. Phys. Colloid Chem. 1949, 53, 1466–1467. [Google Scholar] [CrossRef]
- Cassie, A.B.D.; Baxter, S. Wettability of porous surfaces. Trans. Faraday Soc. 1944, 40, 546–551. [Google Scholar] [CrossRef]
- Onda, T.; Shibuichi, S.; Satoh, N.; Tsujii, K. Super-water-repellent fractal surfaces. Langmuir 1996, 12, 2125–2127. [Google Scholar] [CrossRef]
- Shibuichi, S.; Onda, T.; Satoh, N.; Tsujii, K. Super water-repellent surfaces resulting from fractal structure. J. Phys. Chem. 1996, 100, 19512–19517. [Google Scholar] [CrossRef]
- Davis, E.; Liu, Y.; Jiang, L.; Lu, Y.; Ndao, S. Wetting characteristics of 3-dimensional nanostructured fractal surfaces. Appl. Surf. Sci. 2017, 392, 929–935. [Google Scholar] [CrossRef]
- Koishi, T.; Yasuoka, K.; Fujikawa, S.; Ebisuzaki, T.; Zeng, X.C. Coexistence and transition between Cassie and Wenzel state on pillared hydrophobic surface. Proc. Natl. Acad. Sci. USA 2009, 106, 8435–8440. [Google Scholar] [CrossRef]
- Lafuma, A.; Quéré, D. Superhydrophobic states. Nat. Mater. 2003, 2, 457–460. [Google Scholar] [CrossRef]
- Hernández-Acosta, M.A.; Martines-Arano, H.; Soto-Ruvalcaba, L.; Martínez-González, C.L.; Martínez-Gutiérrez, H.; Torres-Torres, C. Fractional thermal transport and twisted light induced by an optical two-wave mixing in single-wall carbon nanotubes. Int. J. Therm. Sci. 2020, 147, 106136. [Google Scholar] [CrossRef]
- Wang, X.; Fu, C.; Zhang, C.; Qiu, Z.; Wang, B. A comprehensive review of wetting transition mechanism on the surfaces of microstructures from theory and testing methods. Materials 2022, 15, 4747. [Google Scholar] [CrossRef]
- Lundgren, M.; Allan, N.L.; Cosgrove, T.; George, N. Molecular dynamics study of wetting of a pillar surface. Langmuir 2003, 19, 7127–7129. [Google Scholar] [CrossRef]
- Niu, D.; Tang, G.H. Static and dynamic behavior of water droplet on solid surfaces with pillar-type microstructures from molecular dynamics simulation. Int. J. Heat Mass Transfer 2014, 79, 647–654. [Google Scholar] [CrossRef]
- Zhong, X.; Xie, S.; Guo, Z. The challenge of superhydrophobicity: Environmentally facilitated Cassie–Wenzel transitions and structural design. Adv. Sci. 2024, 11, 2305961. [Google Scholar] [CrossRef]
- Li, H.; Yan, T. Importance of moderate size of pillars and dual-scale structures for stable superhydrophobic surfaces: A molecular dynamics simulation study. Comput. Mater. Sci. 2020, 175, 109613. [Google Scholar] [CrossRef]
- Ren, H.; Yang, F.; Li, C.; Deng, C. Controllable dewetting transition on graphene-based nanotextured surfaces. App. Surf. Sci. 2020, 520, 146374. [Google Scholar] [CrossRef]
- Liu, J.-L.; Feng, X.-Q.; Wang, G.; Yu, S.-W. Mechanisms of superhydrophobicity on hydrophilic substrates. J. Phys. Condens. Matter 2007, 19, 356002. [Google Scholar] [CrossRef]
- Lee, H.; Bin Yang, J.; Kim, D.R. Anti-frosting characteristics of superhydrophobic-hydrophilic wettability switchable surfaces. Int. J. Heat Mass Transfer 2024, 221, 125035. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, W.; Han, M.; Guan, L.; Han, L.; Hemraj, A.; Tam, K.C. Sustainable superhydrophobic surface with tunable nanoscale hydrophilicity for water harvesting applications. Angew. Chem. 2022, 134, e202115238. [Google Scholar] [CrossRef]
- Sun, Q. The Raman OH stretching bands of liquid water. Vib. Spectrosc. 2009, 51, 213–217. [Google Scholar] [CrossRef]
- Sun, Q. Local statistical interpretation for water structure. Chem. Phys. Lett. 2013, 568–569, 90–94. [Google Scholar] [CrossRef]
- Sun, Q.; Guo, Y. Vibrational sum frequency generation spectroscopy of the air/water interface. J. Mol. Liq. 2016, 213, 28–32. [Google Scholar] [CrossRef]
- Berendsen, H.J.C.; van der Spoel, D.; van Drunen, R. GROMACS: A message-passing parallel molecular dynamics implementation. Comput. Phys. Commun. 1995, 91, 43–56. [Google Scholar] [CrossRef]
- van der Spoel, D.; Lindahl, E.; Hess, B.; Groenhof, G.; Mark, A.E.; Berendsen, H.J.C. GROMACS: Fast, flexible and free. J. Comput. Chem. 2005, 26, 1701–1718. [Google Scholar] [CrossRef]
- Rasband, W.S. ImageJ Image Analysis Software; U.S. National Institutes of Health: Bethesda, MD, USA, 1997–2018. [Google Scholar]
- Buahom, P. Measuring the Contact Angle Using ImageJ with Contact Angle Plug-In. 2018. Available online: https://www.researchgate.net/publication/328733959_Measuring_the_Contact_Angle_using_ImageJ_with_Contact_Angle_plug-in (accessed on 15 August 2022).
- Kumar, R.; Schmidt, J.R.; Skinner, J.L. Hydrogen bonding definitions and dynamics in liquid water. J. Chem. Phys. 2007, 126, 204107. [Google Scholar] [CrossRef]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual molecular dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef]
- Stanley, H.E.; Teixeira, J. Interpretation of the unusual behavior of H2O and D2O at low temperatures: Tests of a percolation model. J. Chem. Phys. 1980, 73, 3404–3422. [Google Scholar] [CrossRef]
- Nilsson, A.; Pettersson, L.G.M. Perspective on the structure of liquid water. Chem. Phys. 2011, 389, 1–34. [Google Scholar] [CrossRef]
- Collins, K.D.; Neilson, G.W.; Enderby, J.E. Ions in water: Characterizing the forces that control chemical processes and biological structure. Biophys. Chem. 2007, 128, 95–104. [Google Scholar] [CrossRef]
- Cappa, C.D.; Smith, J.D.; Messer, B.M.; Cohen, R.C.; Saykally, R.J. Effects of cations on the hydrogen bond network of liquid water: New results from X-ray absorption spectroscopy of liquid microjets. J. Phys. Chem. B 2006, 110, 5301–5309. [Google Scholar] [CrossRef] [PubMed]
- Omta, A.W.; Kropman, M.F.; Woutersen, S.; Bakker, H.J. Negligible effect of ions on the hydrogen-bond structure in liquid water. Science 2003, 301, 347–349. [Google Scholar] [CrossRef] [PubMed]
- Moilanen, D.E.; Wong, D.; Rosenfeld, D.E.; Fenn, E.E.; Fayer, M.D. Ion–water hydrogen-bond switching observed with 2D IR vibrational echo chemical exchange spectroscopy. Proc. Natl. Acad. Sci. USA 2009, 106, 375–380. [Google Scholar] [CrossRef] [PubMed]
- Turton, D.A.; Hunger, J.; Hefter, G.; Buchner, R.; Wynne, K. Glasslike behavior in aqueous electrolyte solutions. J. Chem. Phys. 2008, 128, 161102. [Google Scholar] [CrossRef]
- Scatena, L.F.; Brown, M.G.; Richmond, G.L. Water at hydrophobic surfaces: Weak hydrogen bonding and strong orientation effects. Science 2001, 292, 908–912. [Google Scholar] [CrossRef]
- Jubb, A.M.; Hua, W.; Allen, H.C. Organization of water and atmospherically relevant ions and solutes: Vibrational sum frequency spectroscopy at the vapor/liquid and liquid/solid interfaces. Acc. Chem. Res. 2012, 45, 110–119. [Google Scholar] [CrossRef]
- Shen, Y.R.; Ostroverkhov, V. Sum-frequency vibrational spectroscopy on water interfaces: Polar orientation of water molecules at interfaces. Chem. Rev. 2006, 106, 1140–1154. [Google Scholar] [CrossRef]
- Richmond, G.L. Molecular bonding and interactions at aqueous surfaces as probed by vibrational sum frequency spectroscopy. Chem. Rev. 2002, 102, 2693–2724. [Google Scholar] [CrossRef]
- Tian, C.S.; Shen, Y.R. Sum-frequency vibrational spectroscopic studies of water/vapor interfaces. Chem. Phys. Lett. 2009, 470, 1–6. [Google Scholar] [CrossRef]
- Ji, N.; Ostroverkhov, V.; Tian, C.S.; Shen, Y.R. Characterization of vibrational resonances of water-vapor interfaces by phase-sensitive sum-frequency spectroscopy. Phys. Rev. Lett. 2008, 100, 096102. [Google Scholar] [CrossRef]
- Sun, Q. The physical origin of hydrophobic effects. Chem. Phys. Lett. 2017, 672, 21–25. [Google Scholar] [CrossRef]
- Sun, Q. The hydrophobic effects: Our current understanding. Molecules 2022, 27, 7009. [Google Scholar] [CrossRef] [PubMed]
- Lm, S.J.; Kim, D.; Kim, Y.; Jeong, S.; Pang, C.; Ryu, S.; Weon, B.M. Hydrophobicity evolution on rough surfaces. Langmuir 2020, 36, 689–696. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Jiang, L. Definition of superhydrophobic states. Adv. Mater. 2007, 19, 3423–3424. [Google Scholar] [CrossRef]
- Dorsey, N.E. Properties of Ordinary Water Substance; ACS Monograph No. 81; Reinhold Publishing Corp.: New York, NY, USA, 1940. [Google Scholar]
- Chen, F.; Smith, P.E. Simulated surface tensions of common water models. J. Chem. Phys. 2007, 126, 221101. [Google Scholar] [CrossRef]
- Vega, C.; de Miguel, E. Surface tension of the most popular models of water by using the test-area simulation method. J. Chem. Phys. 2007, 126, 154707. [Google Scholar] [CrossRef]
- Thompson, H.; Soper, A.K.; Ricci, M.A.; Bruni, F.; Skipper, N.T. The three-dimensional structure of water confined in nanoporous vycor glass. J. Phys. Chem. B 2007, 111, 5610–5620. [Google Scholar] [CrossRef]
- Giri, A.K.; Teixeira, F.; Cordeiro, M.N.D.S. Structure and kinetics of water in highly confined conditions: A molecular dynamics simulation study. J. Mol. Liq. 2018, 268, 625–636. [Google Scholar] [CrossRef]

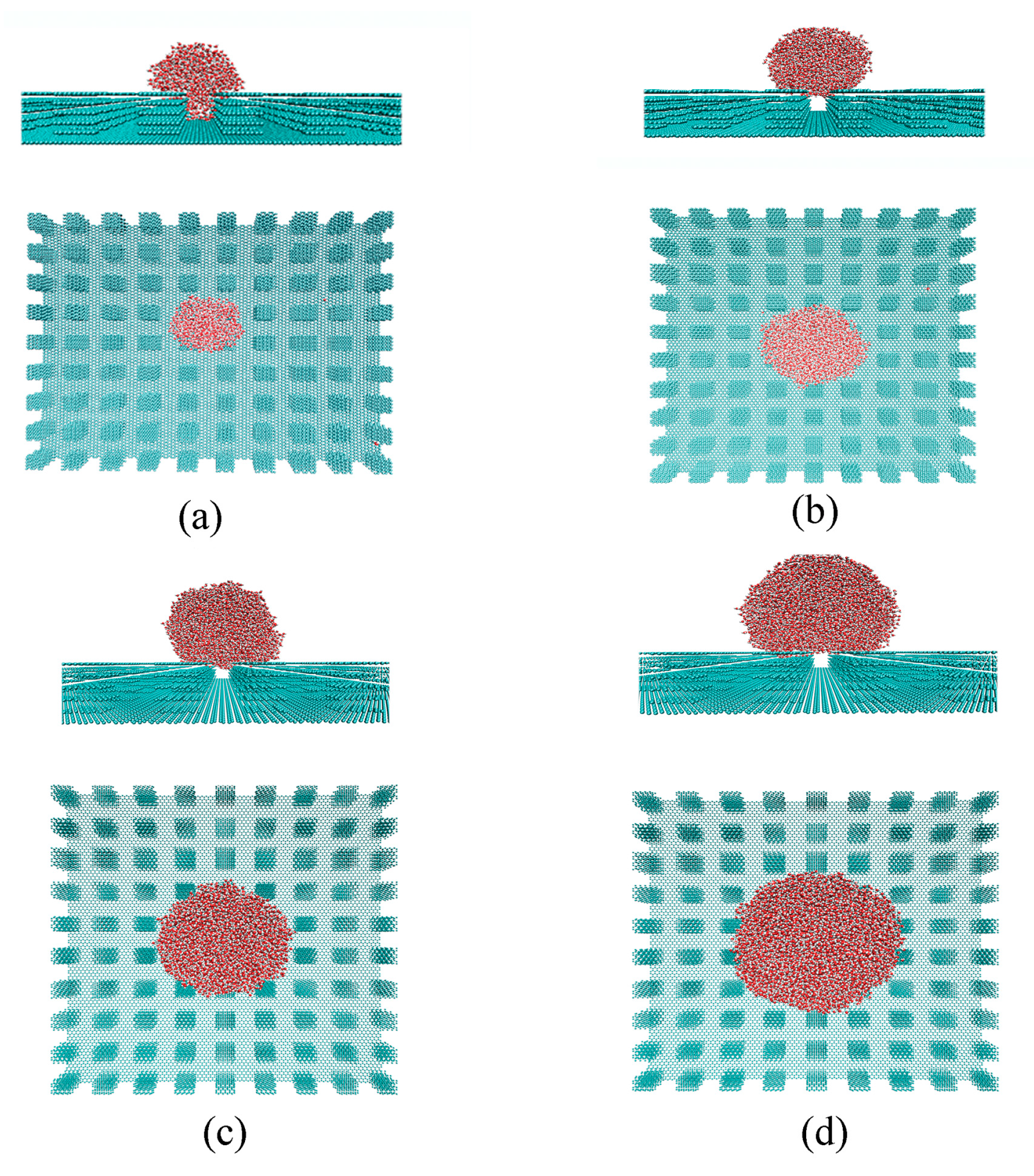
| System | ax (Å) | ay (Å) | wx (Å) | wy (Å) | h (Å) | Wettability | WRoughness | r-WRoughness | CA |
|---|---|---|---|---|---|---|---|---|---|
| a | 7.37 | 7.09 | 7.37 | 7.399 | 10.05 | Cassie | 0.9713 | 0.5696 | 132° |
| 7.37 | 7.09 | 7.37 | 7.399 | 6.7 | Wenzel | 1.1803 | 0.6663 | 118° | |
| b | 7.37 | 7.09 | 9.824 | 9.926 | 13.4 | Cassie | 0.9713 | 0.5696 | 135° |
| 7.37 | 7.09 | 9.824 | 9.926 | 10.05 | Wenzel | 1.1106 | 0.634 | 128° | |
| c | 7.37 | 7.09 | 12.28 | 12.05 | 13.4 | Cassie | 1.0906 | 0.6248 | 133° |
| 7.37 | 7.09 | 12.28 | 12.05 | 10.05 | Wenzel | 1.2696 | 0.7076 | 120° | |
| d | 9.824 | 8.508 | 12.28 | 12.05 | 16.75 | Cassie | 0.7632 | 0.4915 | 134° |
| 9.824 | 8.508 | 12.28 | 12.05 | 13.4 | Wenzel | 0.8444 | 0.5345 | 131° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Q.; Chen, Y.-N.; Liu, Y.-Z. Wetting Transition from Wenzel to Cassie States: Thermodynamic Analysis. Materials 2025, 18, 543. https://doi.org/10.3390/ma18030543
Sun Q, Chen Y-N, Liu Y-Z. Wetting Transition from Wenzel to Cassie States: Thermodynamic Analysis. Materials. 2025; 18(3):543. https://doi.org/10.3390/ma18030543
Chicago/Turabian StyleSun, Qiang, Yan-Nan Chen, and Yu-Zhen Liu. 2025. "Wetting Transition from Wenzel to Cassie States: Thermodynamic Analysis" Materials 18, no. 3: 543. https://doi.org/10.3390/ma18030543
APA StyleSun, Q., Chen, Y.-N., & Liu, Y.-Z. (2025). Wetting Transition from Wenzel to Cassie States: Thermodynamic Analysis. Materials, 18(3), 543. https://doi.org/10.3390/ma18030543





