Energetics of Intrinsic Point Defects in NpO2 from DFT + U Calculations
Abstract
1. Introduction
2. Computational Methodologies
3. Results and Discussion
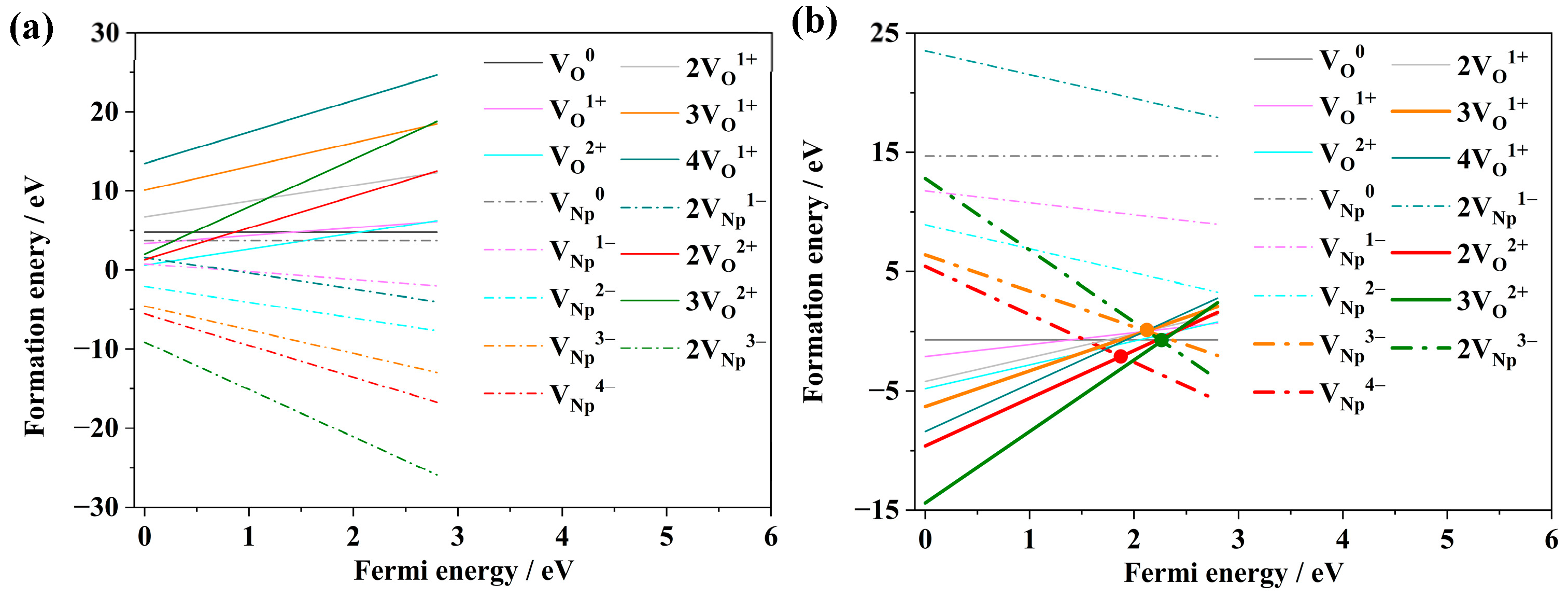
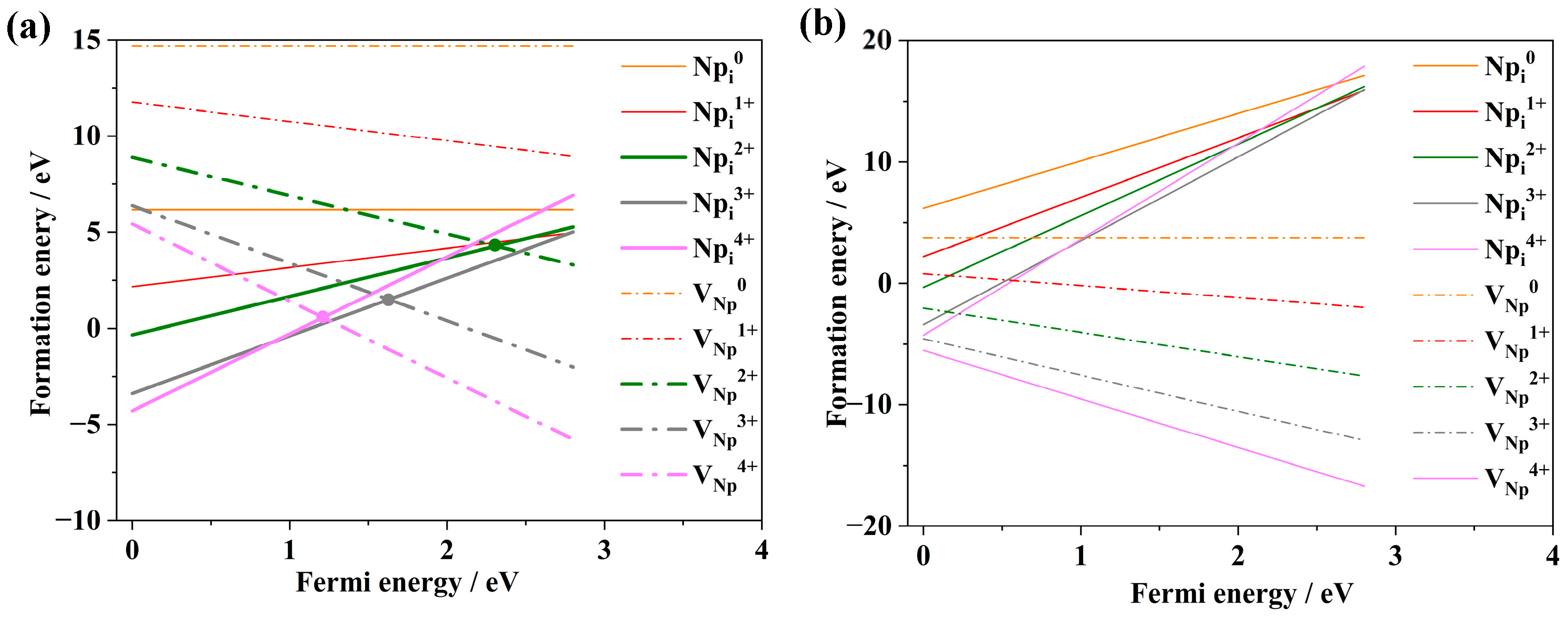
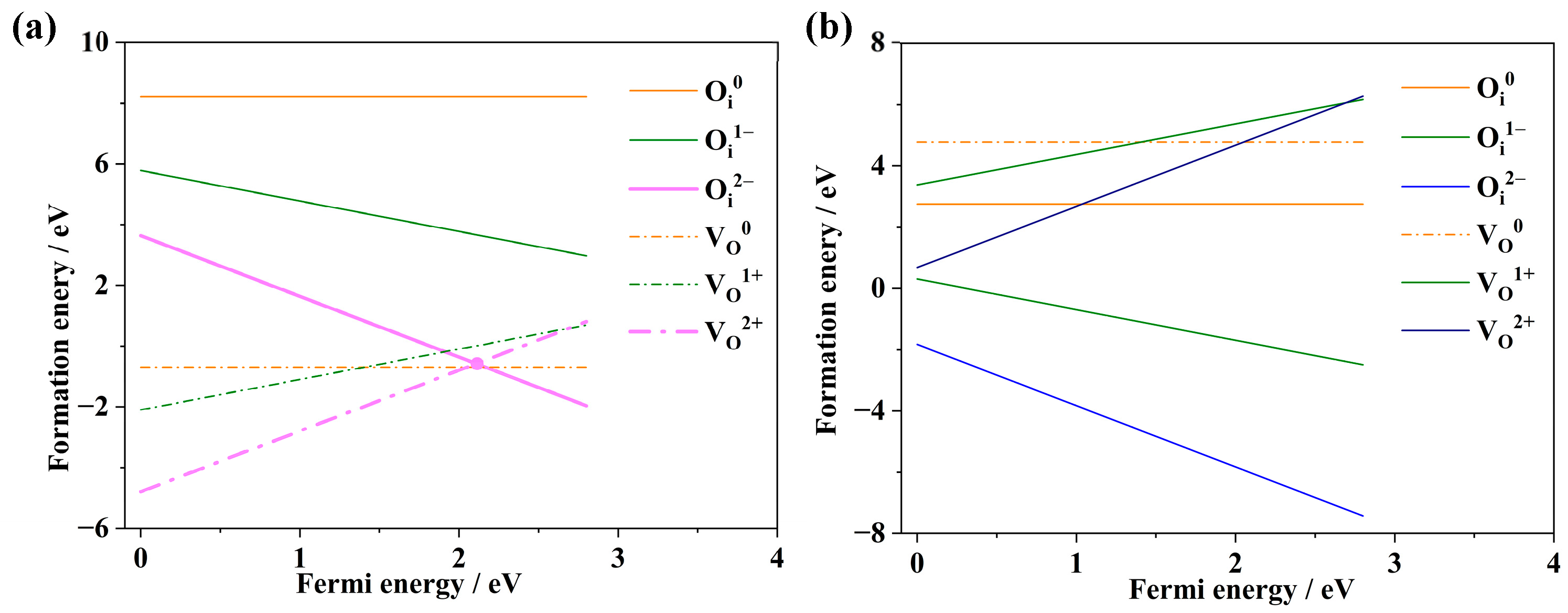
3.1. Intrinsic Point Defect
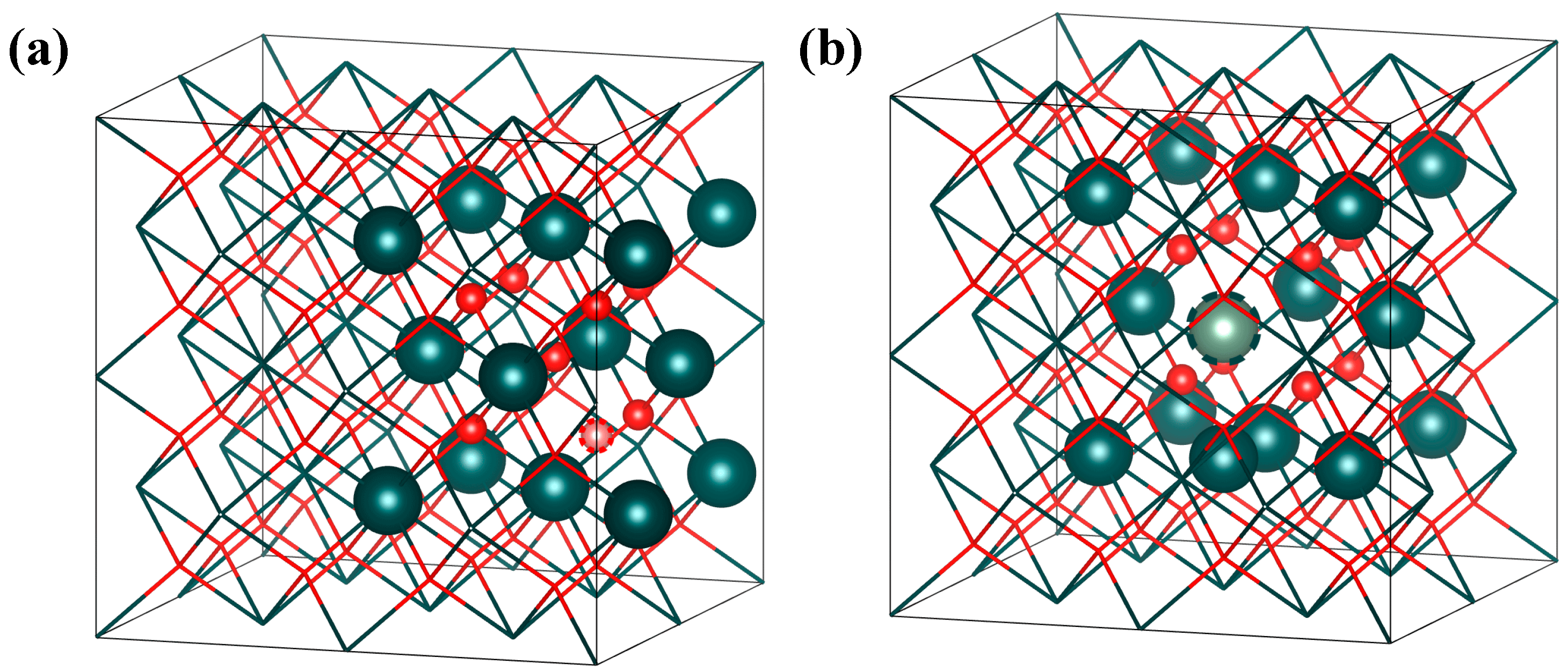
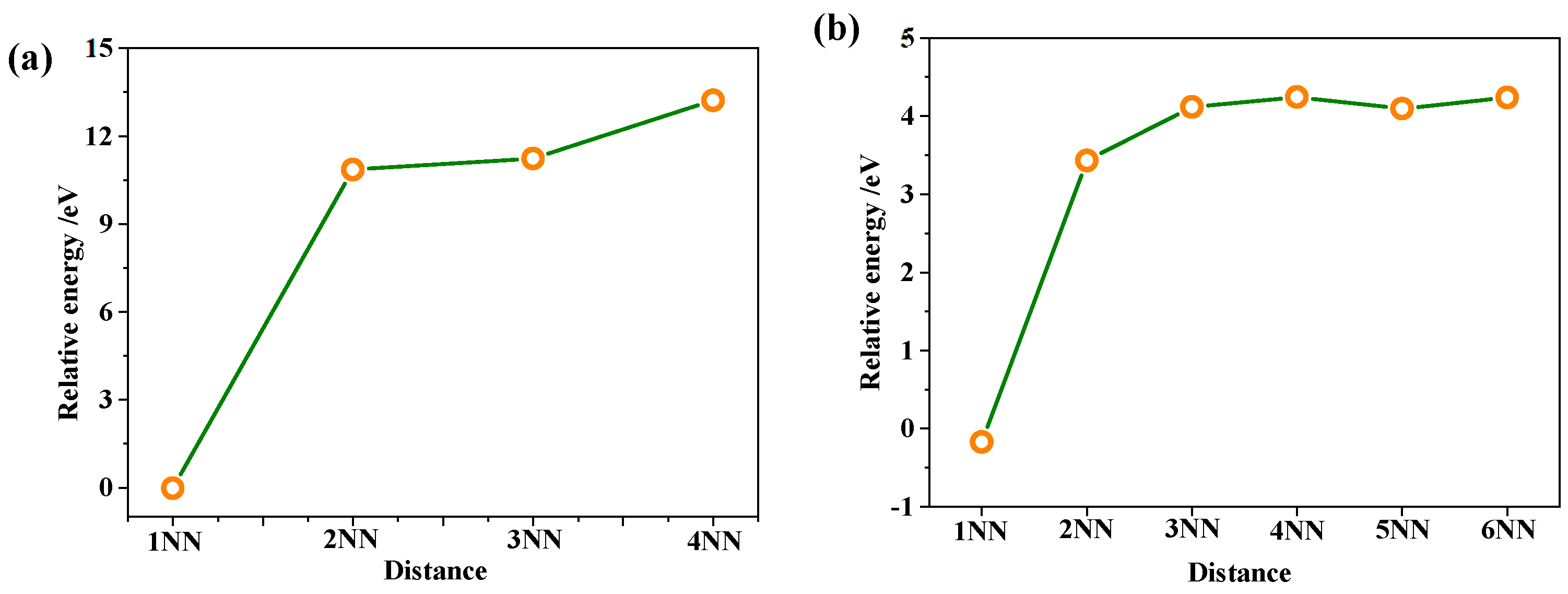
3.2. Schottky Defect
3.3. Frenkel Defect
3.4. Antisite Defects
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bo, T.; Lan, J.H.; Zhao, Y.L.; Zhang, Y.J.; He, C.H.; Chai, Z.F.; Shi, W.Q. Surface properties of NpO2 and water reacting with stoichiometric and reduced NpO2 (111), (110), and (100) surfaces from ab initio atomistic thermodynamics. Surf. Sci. 2016, 644, 153–164. [Google Scholar] [CrossRef]
- Günay, S.D. Actinide and lanthanide dioxide lattice dilatation mechanisms with defect ingrowths. J. Am. Ceram. Soc. 2023, 106, 3895–3910. [Google Scholar] [CrossRef]
- Petit, L.; Svane, A.; Szotek, Z.; Temmerman, W.M.; Stocks, G.M. electronic structure and ionicity of actinide oxides from first principles. Phys. Rev. B 2010, 81, 045108. [Google Scholar] [CrossRef]
- Tiwary, P.; van de Walle, A.; Jeon, B.; Grønbech-Jensen, N. Interatomic potentials for mixed oxide and advanced nuclear fuels. Phys. Rev. B 2011, 83, 094104. [Google Scholar] [CrossRef]
- Kato, M.; Oki, T.; Watanabe, M.; Hirooka, S.; Vauchy, R.; Ozawa, T.; Uwaba, T.; Ikusawa, Y.; Nakamura, H.; Machida, M. A science-based mixed oxide property model for develop advanced oxide nuclear fuels. J. Am. Ceram. Soc. 2024, 107, 2998–3011. [Google Scholar] [CrossRef]
- Yamashita, T.; Nitani, N.; Tsuji, T.; Inagaki, H. Thermal expansions of NpO2 and some other actinide dioxides. J. Nucl. Mater. 1997, 245, 72–78. [Google Scholar] [CrossRef]
- Sobolev, V. Thermophysical properties of NpO2, AmO2 and CmO2. J. Nucl. Mater. 2009, 389, 45–51. [Google Scholar] [CrossRef]
- Moore, K.T.; van der Laan, G. Nature of the 5 f states in actinide metals. Rev. Mod. Phys. 2009, 81, 235. [Google Scholar] [CrossRef]
- Freyss, M.; Vergnet, N.; Petit, T. Ab initio modeling of the behavior of helium and xenon in actinide dioxide nuclear fuels. J. Nucl. Mater. 2006, 352, 144–150. [Google Scholar] [CrossRef]
- Cooper, M.W.D.; Rushton, M.J.D.; Grimes, R.W. A many-body potential approach to modelling the thermomechanical properties of actinide oxides. J. Phys. Condens. Matter 2014, 26, 105401. [Google Scholar] [CrossRef]
- Machida, M. Theoretical and Computational Works on Oxide Nuclear Fuel Materials. In Materials Science and Fuel Technologies of Uranium and Plutonium Mixed Oxide; CRC Press: Boca Raton, FL, USA, 2022; pp. 69–106. [Google Scholar]
- Wen, X.D.; Martin, R.L.; Henderson, T.M.; Scuseria, G.E. Density functional theory studies of the electronic structure of solid state actinide oxides. Chem. Rev. 2013, 113, 1063–1096. [Google Scholar] [CrossRef] [PubMed]
- Kuganathan, N.; Arya, A.; Grimes, R.W. Phase stability, electronic structures and elastic properties of (U,Np)O-2 and (Th,Np)O-2 mixed oxides. Phys. Chem. Chem. Phys. 2018, 20, 18707–18717. [Google Scholar]
- Guéneau, C.; Chartier, A.; Brutzel, L.V. Thermodynamic and Thermophysical Properties of the Actinide Oxides. Compr. Nucl. Mater. 2012, 2, 21–59. [Google Scholar]
- Murphy, G.L.; Langer, E.M.; Walter, O.; Wang, Y.; Wang, S.; Alekseev, E.V. Insights into the structural chemistry of anhydrous and hydrous hexavalent uranium and neptunium dinitrato, trinitrato, and tetranitrato complexes. Inorg. Chem. 2020, 59, 7204–7215. [Google Scholar] [CrossRef]
- Yu, A.; Teterin, A.Y.; Ivanov, K.E.; Ryzhkov, M.V.; Maslakov, K.I.; Kalmykov, S.N.; Petrov, V.G.; Enina, D.A. X-ray photoelectron spectra structure and chemical bond nature in NpO2. Phys. Rev. B 2014, 89, 35102. [Google Scholar]
- Xiang, X.; Zhang, G.; Wang, X.; Tang, T.; Shi, Y. A new perspective on the process of intrinsic point defects in α-Al2O3. Phys. Chem. Chem. Phys. 2015, 17, 29134–29141. [Google Scholar] [CrossRef]
- Serizawa, H.; Arai, Y.; Takano, M.; Suzuki, Y. X-ray Debye temperature and Grüneisen constant of NpO2. J. Alloys Compd. 1999, 282, 17–22. [Google Scholar] [CrossRef]
- Singh, S.; Sonvane, Y.; Nekrasov, K.; Kupryazhkin, A.; Gajjar, P.; Gupta, S.K. A first principles investigation of defect energetics and diffusion in actinide dioxides. J. Nucl. Mater. 2024, 591, 154901. [Google Scholar] [CrossRef]
- Bendjedid, A.; Seddik, T.; Khenata, R.; Baltache, H.; Murtaza, G.; Bouhemadou, A.; Omran, S.B.; Azam, S.; Khan, S.A. GGA+ U study on phase transition, optoelectronic and magnetic properties of AmO2 with spin–orbit coupling. J. Magn. Magn. Mater. 2015, 396, 190–197. [Google Scholar] [CrossRef]
- Jin, L.; Li, P.; Lei, X.; Zhou, H.; Wang, C. Mechanical and thermal properties of NpO2 using LSDA+ U approach. Prog. Nat. Sci. Mater. Int. 2014, 24, 373–377. [Google Scholar] [CrossRef]
- Han, X.; Alcock, N.M.; Kaltsoyannis, N. Effect of point defects on water adsorption on the ThO2 {111} surface: A first-principles computational study. J. Nucl. Mater. 2024, 588, 154763. [Google Scholar] [CrossRef]
- McCleskey, T.M.; Bauer, E.; Jia, Q.; Burrell, A.K.; Scott, B.L.; Conradson, S.D.; Mueller, A.; Roy, L.; Wen, X.; Scuseria, G.E.; et al. Optical band gap of NpO2 and PuO2 from optical absorbance of epitaxial films. J. Appl. Phys. 2013, 113, 013515. [Google Scholar] [CrossRef]
- Morée, J.B.; Outerovitch, R.; Amadon, B. First-principles calculation of the Coulomb interaction parameters U and J for actinide dioxides. Phys. Rev. B 2021, 103, 045113. [Google Scholar] [CrossRef]
- Liu, T.; Gao, T. Structural and Energetic Properties for Point Defects in NpO2 from DFT+U Calculations. Chin. J. Comput. Phys. 2025, 42, 84–89. [Google Scholar]
- Bruneval, F.; Crocombette, J.P. Ab initio formation volume of charged defects. Phys. Rev. B 2012, 86, 71–75. [Google Scholar] [CrossRef]
- Dorado, B.; Freyss, M.; Amadon, B.; Bertolus, M.; Jomard, G.; Garcia, P. Advances in first-principles modelling of point defects in UO2: F electron correlations and the issue of local energy minima. J. Phys. Condens. Matter. 2013, 25, 333201. [Google Scholar] [CrossRef]
- Allen, J.P.; Watson, G.W. Occupation matrix control of d- and f-electron localisations using DFT + U. Phys. Chem. Chem. Phys. 2014, 16, 21016. [Google Scholar] [CrossRef] [PubMed]
- Smith, A.L.; Colle, J.Y.; Beneš, O.; Konings, R.J.M.; Sundman, B.; Guéneau, C. Thermodynamic assessment of the neptunium-oxygen system: Mass spectrometric studies and thermodynamic modelling. J. Chem. Thermodyn. 2016, 103, 257–275. [Google Scholar] [CrossRef]
- Tayal, A.; Conradson, S.D.; Baldinozzi, G.; Namdeo, S.; Roberts, K.E.; Allen, P.G.; Shuh, D.K. Identification of NpO2+x in the binary Np-O system. J. Nucl. Mater. 2017, 490, 279–287. [Google Scholar] [CrossRef]
- Neilson, W.D.; Pegg, J.T.; Steele, H.; Murphy, S.T. The defect chemistry of non-stoichiometric PuO2±x. Phys. Chem. Chem. Phys. 2021, 23, 4544–4554. [Google Scholar] [CrossRef]
- Smith, T.; Moxon, S.; Tse, J.S.; Skelton, J.M.; Cooke, D.J.; Gillie, L.J.; da Silva, E.L.; Harker, R.M.; Storr, M.T.; Parker, S.C.; et al. Structural dynamics of Schottky and Frenkel defects in CeO2: A density-functional theory study. J. Phys. Energy 2023, 5, 025004. [Google Scholar] [CrossRef]
- Berthinier, C.; Rado, C.; Chatillon, C.; Hodaj, F. Thermodynamic assessment of oxygen diffusion in non-stoichiometric UO2±x from experimental data and Frenkel pair modeling. J. Nucl. Mater. 2013, 433, 265–286. [Google Scholar] [CrossRef]
- Chollet, M.; Léchelle, J.; Belin, R.C.; Richaud, J.-C. In situ X-ray diffraction study of point defects in neptunium dioxide at elevated temperature. J. Appl. Crystallogr. 2014, 47, 1008–1015. [Google Scholar] [CrossRef]
- Konings, R.J.M.; Beneš, O. The heat capacity of NpO2 at high temperatures: The effect of oxygen Frenkel pair formation. J. Phys. Chem. Solids 2013, 74, 653–655. [Google Scholar] [CrossRef]
- Na-Phattalung, S.; Smith, M.F.; Kim, K.; Du, M.H.; Wei, S.H.; Zhang, S.B.; Limpijumnong, S. First-principles study of native defects in anataseTiO2. Phys. Rev. B 2006, 73, 125205. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, H.; Wang, S.; Li, L.; Qiu, R.; Qian, S.; Yang, S. Energetics of Intrinsic Point Defects in NpO2 from DFT + U Calculations. Materials 2025, 18, 2487. https://doi.org/10.3390/ma18112487
Yu H, Wang S, Li L, Qiu R, Qian S, Yang S. Energetics of Intrinsic Point Defects in NpO2 from DFT + U Calculations. Materials. 2025; 18(11):2487. https://doi.org/10.3390/ma18112487
Chicago/Turabian StyleYu, Huilong, Shuaipeng Wang, Laiyang Li, Ruizhi Qiu, Shijun Qian, and Suolong Yang. 2025. "Energetics of Intrinsic Point Defects in NpO2 from DFT + U Calculations" Materials 18, no. 11: 2487. https://doi.org/10.3390/ma18112487
APA StyleYu, H., Wang, S., Li, L., Qiu, R., Qian, S., & Yang, S. (2025). Energetics of Intrinsic Point Defects in NpO2 from DFT + U Calculations. Materials, 18(11), 2487. https://doi.org/10.3390/ma18112487






