First-Principles Design of Qubits in Charged Carbon Nanomaterials
Abstract
1. Introduction
2. Models and Methods
3. Results and Discussions
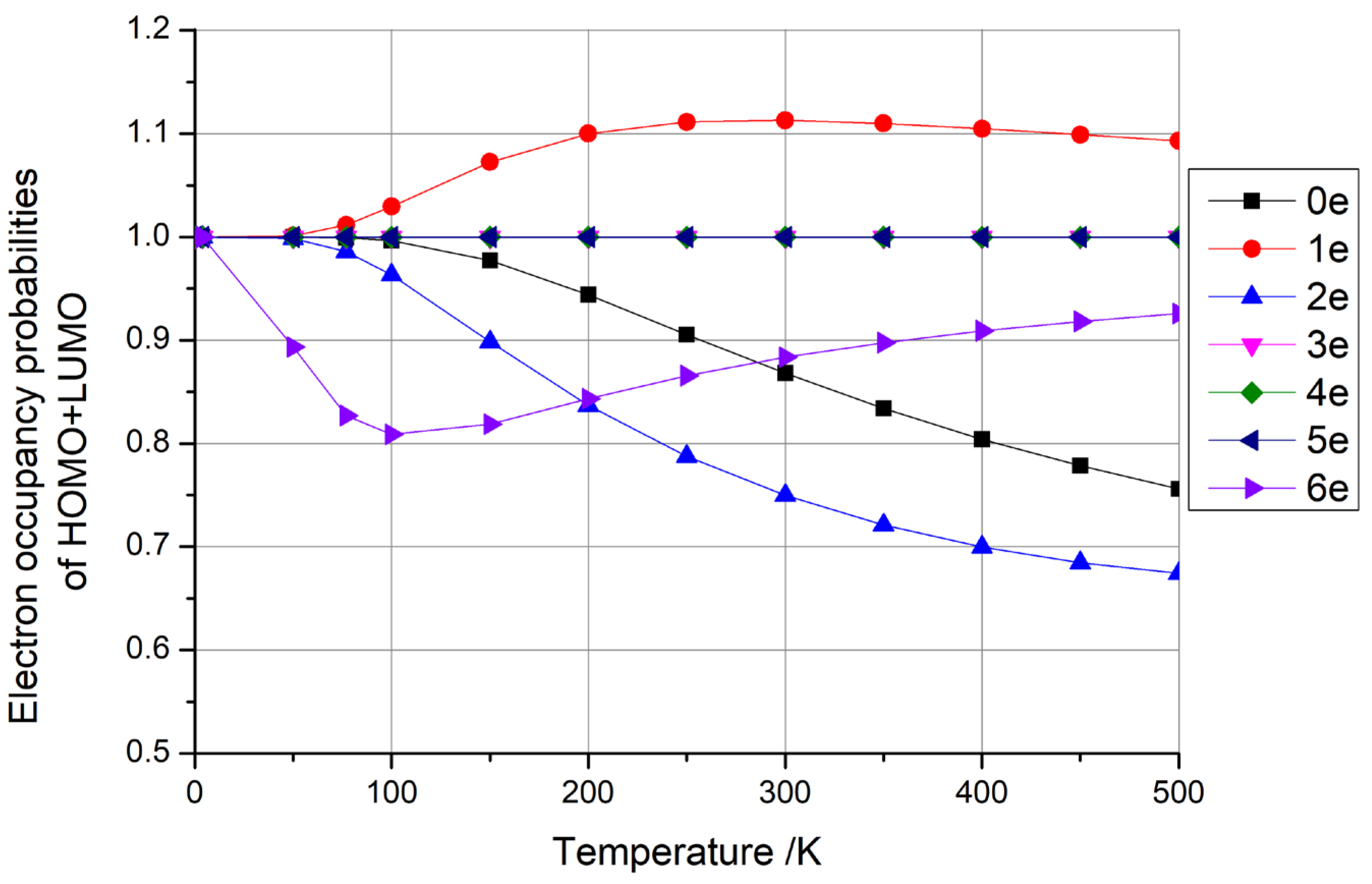
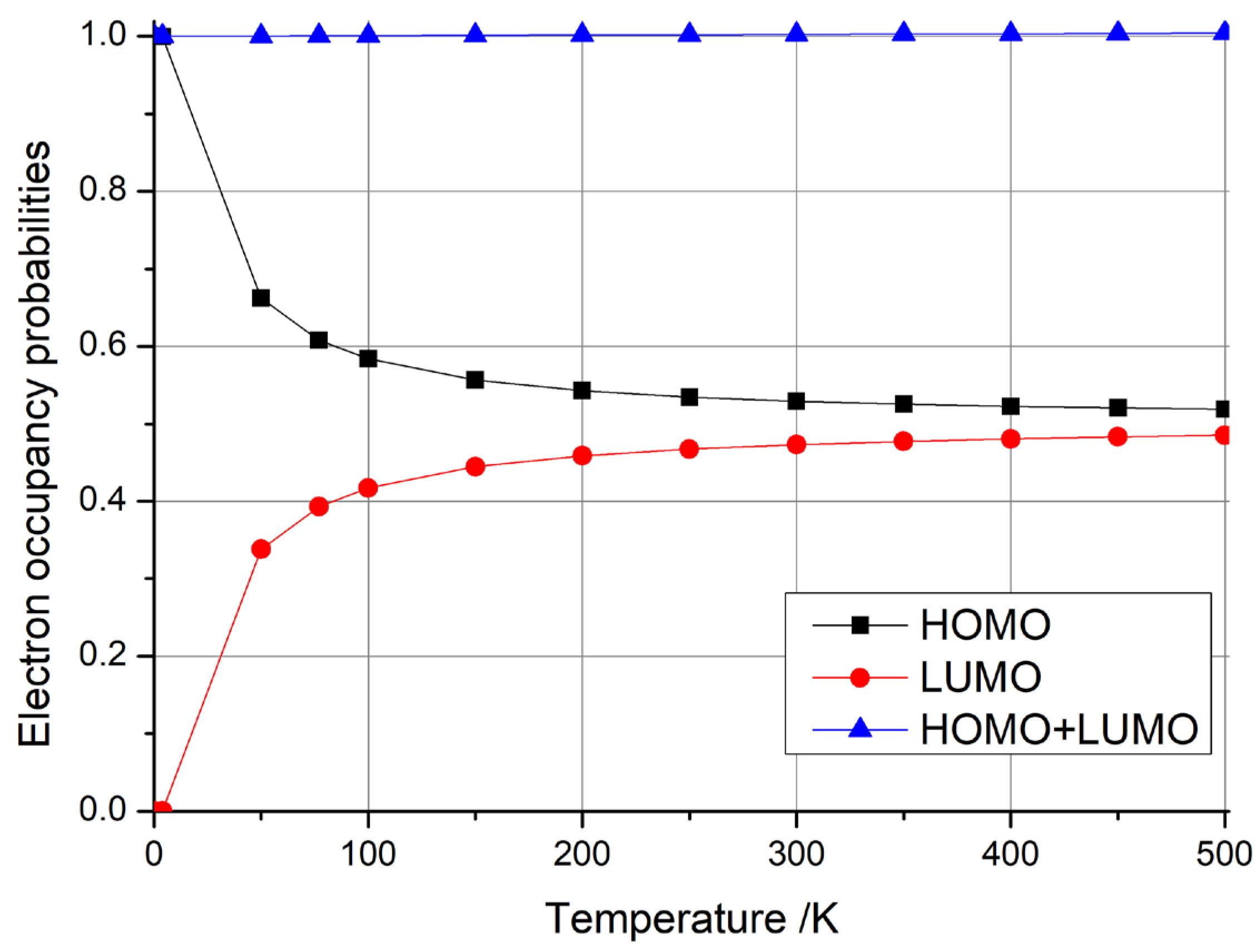
3.1. One-Dimensional Carbon Nanomaterials Containing 100 Carbon Atoms with 0–6 Additional Electrons
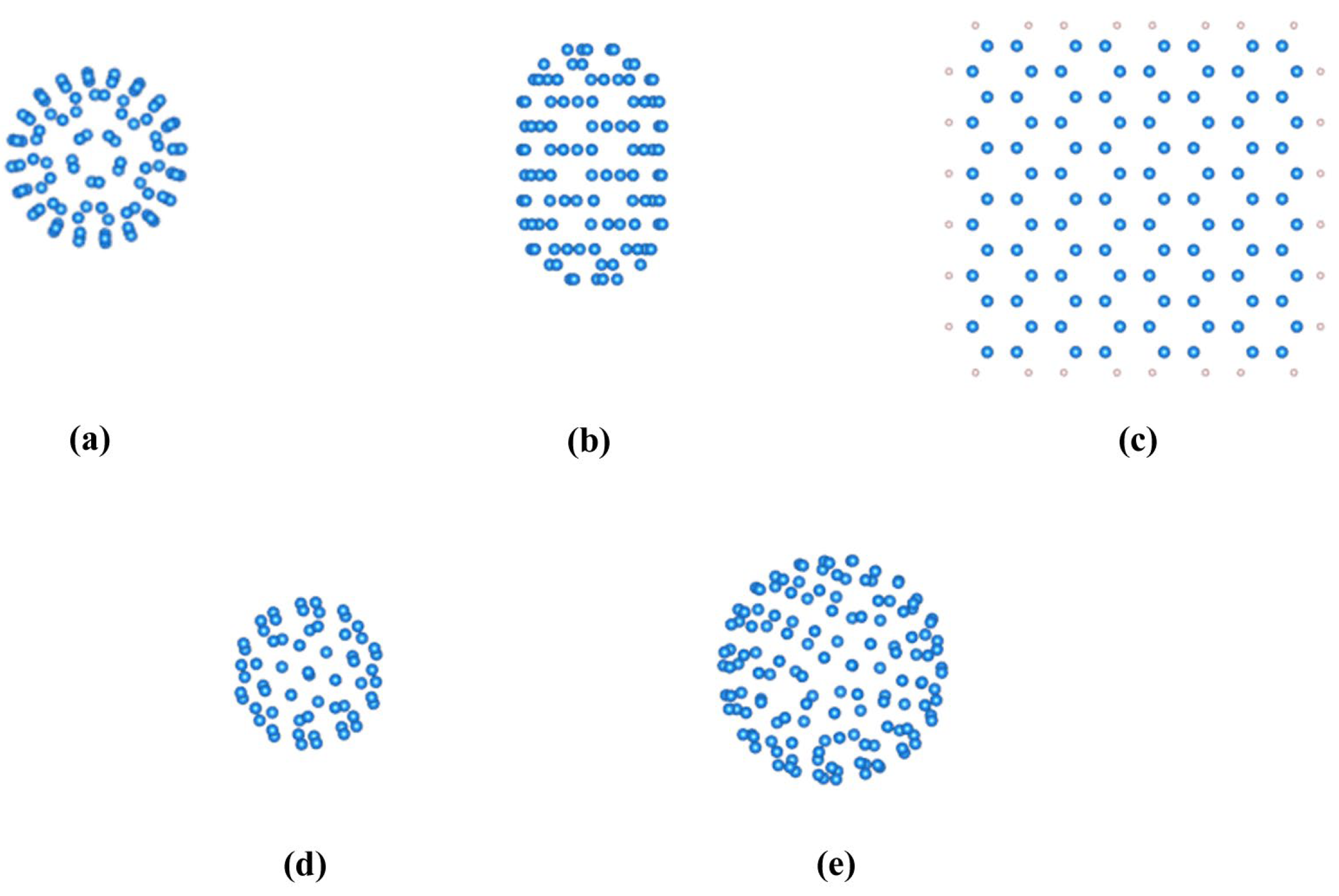
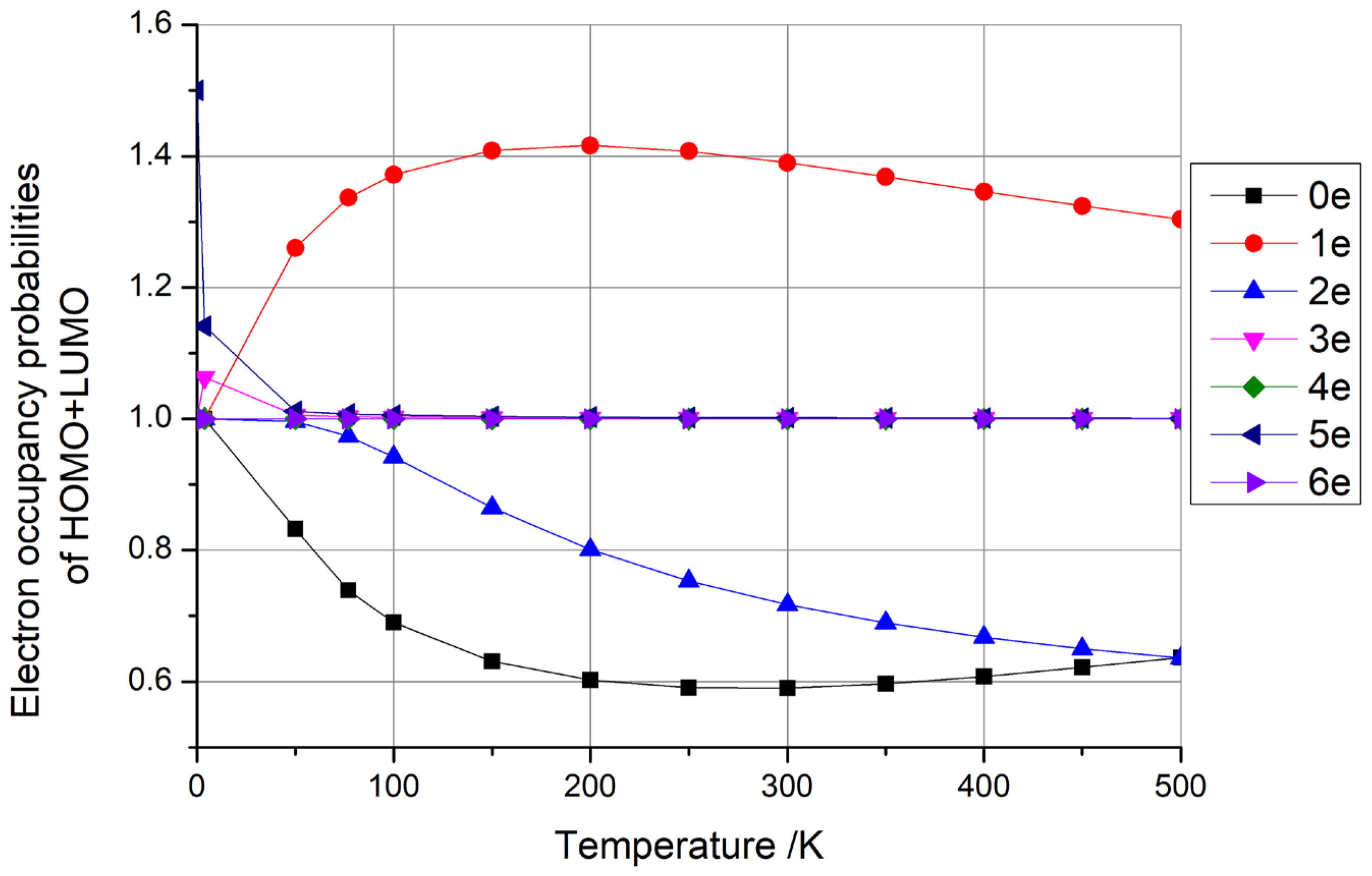
3.2. Zero-Dimensional and Two-Dimensional Carbon Nanomaterials Containing Approximately 100 C Atoms with 0–6 Additional Electrons
3.3. Carbon Nanomaterials Containing 60 and 140 C Atoms with 0–6 Additional Electrons
3.4. Feasibility and Advancement Analysis of Proposed System
3.4.1. Experimental Feasibility
3.4.2. Material Superiority over Conventional Systems
- Quantum Confinement Effects: Discrete HOMO-LUMO energy levels in carbon nanomaterials enable precise charge and spin manipulation, unachievable in bulk semiconductors with continuous bands.
- Weak Spin–Orbit Coupling: The absence of heavy atoms in carbon systems minimizes spin decoherence, enhancing spin lifetime.
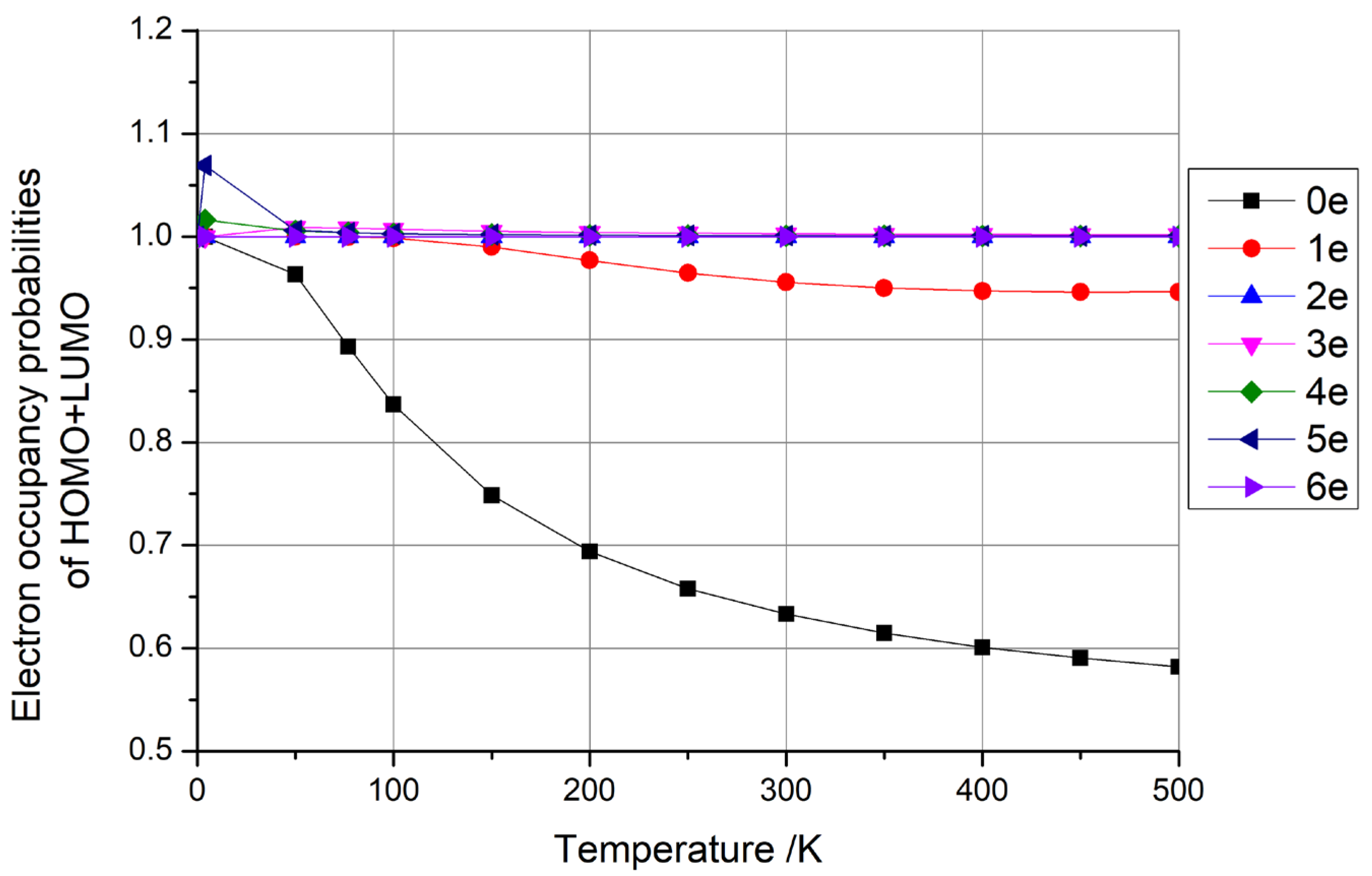
- Thermal Stability: The HOMO + LUMO occupancy remains stable up to 300 K (Figure 5), outperforming temperature-sensitive systems like diamond NV centers.
- Dimensional Flexibility: The diversity of carbon nanomaterials (0D fullerenes, 1D nanotubes, 2D graphene) allows for their tailored selection for specific quantum applications [39].
3.4.3. Technological Advancement
3.4.4. Experimental Precedents
3.4.5. Computational Rationality
3.4.6. Key Challenges, Mitigation Strategies, and Future Directions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- García-Ripoll, J.J.; Zoller, P.; Cirac, J.I. Speed Optimized Two-Qubit Gates with Laser Coherent Control Techniques for Ion Trap Quantum Computing. Phys. Rev. Lett. 2003, 91, 157901. [Google Scholar] [CrossRef] [PubMed]
- Singh, R.; Dutta, M.; Stroscio, M.A. Role of Confined Optical Phonons in Exciton Generation in Spherical Quantum Dot. Materials 2022, 15, 5545. [Google Scholar] [CrossRef] [PubMed]
- Duan, L.-M.; Cirac, J.I.; Zoller, P. Geometric Manipulation of Trapped Ions for Quantum Computation. Science 2001, 292, 1695–1697. [Google Scholar] [CrossRef]
- Preskill, J. Quantum Computing in the NISQ Era and Beyond. Quantum 2018, 2, 79. [Google Scholar] [CrossRef]
- Ryabinkin, I.G.; Yen, T.-C.; Genin, S.N.; Izmaylov, A.F. Qubit Coupled Cluster Method: A Systematic Approach to Quantum Chemistry on a Quantum Computer. J. Chem. Theory Comput. 2018, 14, 6317–6326. [Google Scholar] [CrossRef]
- Kane, B.E. A Silicon-Based Nuclear Spin Quantum Computer. Nature 1998, 393, 133–137. [Google Scholar] [CrossRef]
- Yen, T.-C.; Verteletskyi, V.; Izmaylov, A.F. Measuring All Compatible Operators in One Series of Single-Qubit Measurements Using Unitary Transformations. J. Chem. Theory Comput. 2020, 16, 2400–2409. [Google Scholar] [CrossRef]
- Rivest, R.L.; Shamir, A.; Adleman, L. A Method for Obtaining Digital Signatures and Public-Key Cryptosystems. Commun. ACM 1978, 21, 120–126. [Google Scholar] [CrossRef]
- Shor, P.W. Algorithms for Quantum Computation: Discrete Logarithms and Factoring. In Proceedings of the Proceedings 35th Annual Symposium on Foundations of Computer Science, Santa Fe, NM, USA, 20–22 November 1994; IEEE Computer Society Press: Santa Fe, NM, USA, 1994. [Google Scholar]
- Hey, T. Quantum Computing: An Introduction. Comput. Control Eng. J. 1999, 10, 105–112. [Google Scholar] [CrossRef]
- Laird, E.A.; Kuemmeth, F.; Steele, G.A.; Grove-Rasmussen, K.; Nygård, J.; Flensberg, K.; Kouwenhoven, L.P. Quantum Transport in Carbon Nanotubes. Rev. Mod. Phys. 2015, 87, 703–764. [Google Scholar] [CrossRef]
- Chen, J.; Hu, C.; Stanton, J.F.; Hill, S.; Cheng, H.-P.; Zhang, X.-G. Decoherence in Molecular Electron Spin Qubits: Insights from Quantum Many-Body Simulations. J. Phys. Chem. Lett. 2020, 11, 2074–2078. [Google Scholar] [CrossRef] [PubMed]
- Hendrickx, N.W.; Lawrie, W.I.L.; Petit, L.; Sammak, A.; Scappucci, G.; Veldhorst, M. A Single-Hole Spin Qubit. Nat. Commun. 2020, 11, 3478. [Google Scholar] [CrossRef]
- Qiang, X.; Zhou, X.; Wang, J.; Wilkes, C.M.; Loke, T.; O’Gara, S.; Kling, L.; Marshall, G.D.; Santagati, R.; Ralph, T.C.; et al. Large-Scale Silicon Quantum Photonics Implementing Arbitrary Two-Qubit Processing. Nat. Photonics 2018, 12, 534–539. [Google Scholar] [CrossRef]
- Beke, D.; Valenta, J.; Károlyházy, G.; Lenk, S.; Czigány, Z.; Márkus, B.G.; Kamarás, K.; Simon, F.; Gali, A. Room-Temperature Defect Qubits in Ultrasmall Nanocrystals. J. Phys. Chem. Lett. 2020, 11, 1675–1681. [Google Scholar] [CrossRef]
- Pashkin, Y.A.; Astafiev, O.; Yamamoto, T.; Nakamura, Y.; Tsai, J.S. Josephson Charge Qubits: A Brief Review. Quantum Inf. Process. 2009, 8, 55–80. [Google Scholar] [CrossRef]
- Samkharadze, N.; Zheng, G.; Kalhor, N.; Brousse, D.; Sammak, A.; Mendes, U.C.; Blais, A.; Scappucci, G.; Vandersypen, L.M.K. Strong Spin-Photon Coupling in Silicon. Science 2018, 359, 1123–1127. [Google Scholar] [CrossRef]
- Borjans, F.; Croot, X.G.; Mi, X.; Gullans, M.J.; Petta, J.R. Resonant Microwave-Mediated Interactions between Distant Electron Spins. Nature 2020, 577, 195–198. [Google Scholar] [CrossRef]
- Hsiao, T.-K.; Rubino, A.; Chung, Y.; Son, S.-K.; Hou, H.; Pedrós, J.; Nasir, A.; Éthier-Majcher, G.; Stanley, M.J.; Phillips, R.T.; et al. Single-Photon Emission from Single-Electron Transport in a SAW-Driven Lateral Light-Emitting Diode. Nat. Commun. 2020, 11, 917. [Google Scholar] [CrossRef]
- Hensen, B.; Wei Huang, W.; Yang, C.-H.; Wai Chan, K.; Yoneda, J.; Tanttu, T.; Hudson, F.E.; Laucht, A.; Itoh, K.M.; Ladd, T.D.; et al. A Silicon Quantum-Dot-Coupled Nuclear Spin Qubit. Nat. Nanotechnol. 2020, 15, 13–17. [Google Scholar] [CrossRef]
- Nadj-Perge, S.; Frolov, S.M.; Bakkers, E.P.A.M.; Kouwenhoven, L.P. Spin–Orbit Qubit in a Semiconductor Nanowire. Nature 2010, 468, 1084–1087. [Google Scholar] [CrossRef]
- Pomorski, K. Electrostatically Interacting Wannier Qubits in Curved Space. Materials 2024, 17, 4846. [Google Scholar] [CrossRef] [PubMed]
- Nakamura, Y.; Pashkin, Y.A.; Tsai, J.S. Coherent Control of Macroscopic Quantum States in a Single-Cooper-Pair Box. Nature 1999, 398, 786–788. [Google Scholar] [CrossRef]
- Park, J.-M.; Chong, Z.X.; Kim, R.H.J.; Haeuser, S.; Chan, R.; Murthy, A.A.; Kopas, C.J.; Marshall, J.; Setiawan, D.; Lachman, E.; et al. Probing Non-Equilibrium Pair-Breaking and Quasiparticle Dynamics in Nb Superconducting Resonators under Magnetic Fields. Materials 2025, 18, 569. [Google Scholar] [CrossRef] [PubMed]
- Birge, N.O.; Satchell, N. Ferromagnetic Materials for Josephson π Junctions. APL Mater. 2024, 12, 041105. [Google Scholar] [CrossRef]
- Makhlin, Y.; Schön, G.; Shnirman, A. Quantum-State Engineering with Josephson-Junction Devices. Rev. Mod. Phys. 2001, 73, 357–400. [Google Scholar] [CrossRef]
- Sotirova, A.S.; Sun, B.; Leppard, J.D.; Wang, A.; Wang, M.; Vazquez-Brennan, A.; Nadlinger, D.P.; Moser, S.; Jesacher, A.; He, C.; et al. Low Cross-Talk Optical Addressing of Trapped-Ion Qubits Using a Novel Integrated Photonic Chip. Light Sci. Appl. 2024, 13, 199. [Google Scholar] [CrossRef]
- Brown, K.R.; Wilson, A.C.; Colombe, Y.; Ospelkaus, C.; Meier, A.M.; Knill, E.; Leibfried, D.; Wineland, D.J. Single-Qubit-Gate Error below 10−4 in a Trapped Ion. Phys. Rev. A 2011, 84, 030303. [Google Scholar] [CrossRef]
- Wolfowicz, G.; Heremans, F.J.; Anderson, C.P.; Kanai, S.; Seo, H.; Gali, A.; Galli, G.; Awschalom, D.D. Quantum Guidelines for Solid-State Spin Defects. Nat. Rev. Mater. 2021, 6, 906–925. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient Iterative Schemes for Ab Initio Total-Energy Calculations Using a Plane-Wave Basis Set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector Augmented-Wave Method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Xu, L.-H.; Wu, S.-Q.; Huang, Z.-Q.; Zhang, F.; Chuang, F.-C.; Zhu, Z.-Z.; Ho, K.-M. HOT Graphene and HOT Graphene Nanotubes: New Low Dimensional Semimetals and Semiconductors. Nanoscale Res. Lett. 2020, 15, 56. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.-P.; Bao, H.-H.; Han, L.-L.; Yuan, W.-J.; Luo, J.; Zhu, J. Different Charging-Induced Modulations of Highest Occupied Molecular Orbital Energies in Fullerenes in Comparison with Carbon Nanotubes and Graphene Sheets. Chin. Phys. Lett. 2018, 35, 127301. [Google Scholar] [CrossRef]
- Hu, Y.H.; Ruckenstein, E. Endohedral Chemistry of C60-Based Fullerene Cages. J. Am. Chem. Soc. 2005, 127, 11277–11282. [Google Scholar] [CrossRef]
- Ibach, H.; Lüth, H. Solid-State Physics: An Introduction to Principles of Materials Science; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Huang, K.; Han, R.Q. Solid-State Physics; Higher Education Press: Beijing, China, 1988. [Google Scholar]
- Hu, Z.; Dong, B.-W.; Liu, Z.; Liu, J.-J.; Su, J.; Yu, C.; Xiong, J.; Shi, D.-E.; Wang, Y.; Wang, B.-W.; et al. Endohedral Metallofullerene as Molecular High Spin Qubit: Diverse Rabi Cycles in Gd2@C79N. J. Am. Chem. Soc. 2018, 140, 1123–1130. [Google Scholar] [CrossRef]
- Morton, J.J.L.; Tyryshkin, A.M.; Ardavan, A.; Benjamin, S.C.; Porfyrakis, K.; Lyon, S.A.; Briggs, G.A.D. Bang–Bang Control of Fullerene Qubits Using Ultrafast Phase Gates. Nat. Phys. 2006, 2, 40–43. [Google Scholar] [CrossRef]
- Cao, J.; Wang, Q.; Dai, H. Electron Transport in Very Clean, as-Grown Suspended Carbon Nanotubes. Nat. Mater. 2005, 4, 745–749. [Google Scholar] [CrossRef][Green Version]
- Mananghaya, M.; Yu, D.; Santos, G.N.; Rodulfo, E. Scandium and Titanium Containing Single-Walled Carbon Nanotubes for Hydrogen Storage: A Thermodynamic and First Principle Calculation. Sci. Rep. 2016, 6, 27370. [Google Scholar] [CrossRef]
- Dean, C.R.; Young, A.F.; Meric, I.; Lee, C.; Wang, L.; Sorgenfrei, S.; Watanabe, K.; Taniguchi, T.; Kim, P.; Shepard, K.L.; et al. Boron Nitride Substrates for High-Quality Graphene Electronics. Nat. Nanotechol. 2010, 5, 722–726. [Google Scholar] [CrossRef]
- Shevchenko, E.V.; Talapin, D.V.; Kotov, N.A.; O’Brien, S.; Murray, C.B. Structural Diversity in Binary Nanoparticle Superlattices. Nature 2006, 439, 55–59. [Google Scholar] [CrossRef]




| Model/Charge | 0e | 1e | 2e | 3e | 4e | 5e | 6e |
|---|---|---|---|---|---|---|---|
| C60 | <1 | >1 | <1 | <1 | >1 | >1 | =1 |
| C100 | <1 | >1 | <1 | >1 | =1 | >1 | =1 |
| C140 | <1 | <1 | <1 | <1 | =1 | >1 | <1 |
| CNT | <1 | >1 | <1 | =1 | =1 | =1 | <1 |
| Graphene | <1 | <1 | =1 | >1 | >1 | >1 | =1 |
| Parameter/System | Carbon Nanomaterials | NV Centers | Semiconductor Quantum Dots |
|---|---|---|---|
| Regulation Method | Charge Injection/Temperature | Optical/Microwave | Electric Field/Magnetic Field |
| Scalability | High | Low | Medium |
| Coherence Time | Long | Longest | Shorter |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Wu, M.; Xie, F.; Meng, D.; Luo, J.; Zhu, J. First-Principles Design of Qubits in Charged Carbon Nanomaterials. Materials 2025, 18, 2451. https://doi.org/10.3390/ma18112451
Yang H, Wu M, Xie F, Meng D, Luo J, Zhu J. First-Principles Design of Qubits in Charged Carbon Nanomaterials. Materials. 2025; 18(11):2451. https://doi.org/10.3390/ma18112451
Chicago/Turabian StyleYang, Hongping, Minghui Wu, Fengyan Xie, Dongli Meng, Jun Luo, and Jing Zhu. 2025. "First-Principles Design of Qubits in Charged Carbon Nanomaterials" Materials 18, no. 11: 2451. https://doi.org/10.3390/ma18112451
APA StyleYang, H., Wu, M., Xie, F., Meng, D., Luo, J., & Zhu, J. (2025). First-Principles Design of Qubits in Charged Carbon Nanomaterials. Materials, 18(11), 2451. https://doi.org/10.3390/ma18112451





