Damage Identification of Fiber-Reinforced Composite Thin Plate by Curvature Modal Shape Scanning Method
Abstract
1. Introduction
2. Damage Location Detection Principle of the FCTP Based on the CMSSM
2.1. To Obtain Modal Shape of the FCTP Based on Laser Scanning Method
2.2. Extraction of Curvature Modal Shape of the FCTP
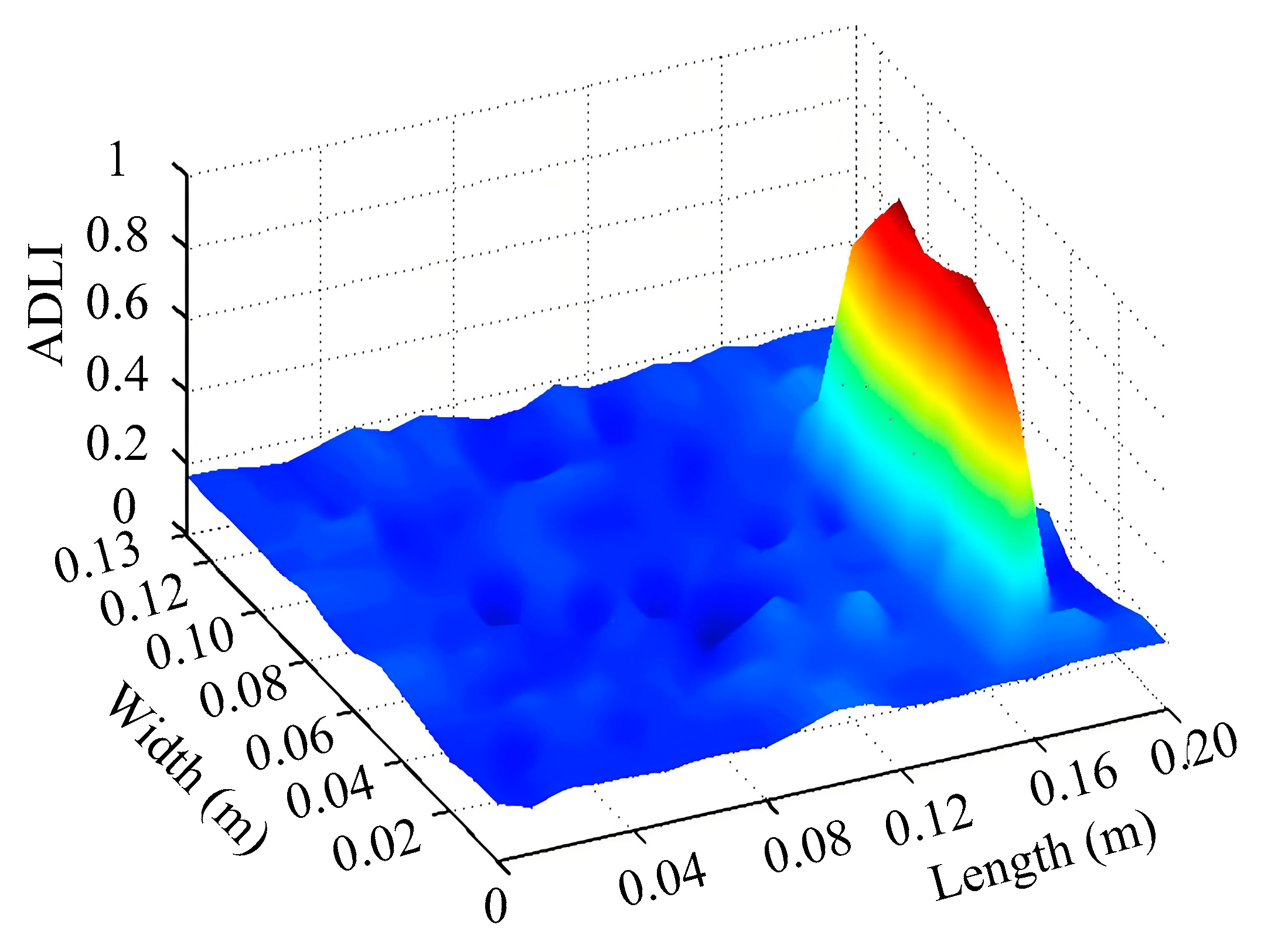
2.3. Determination of Damage Location Based on the Two-Dimensional Five-Spot-Tripling Surface Smoothing Method
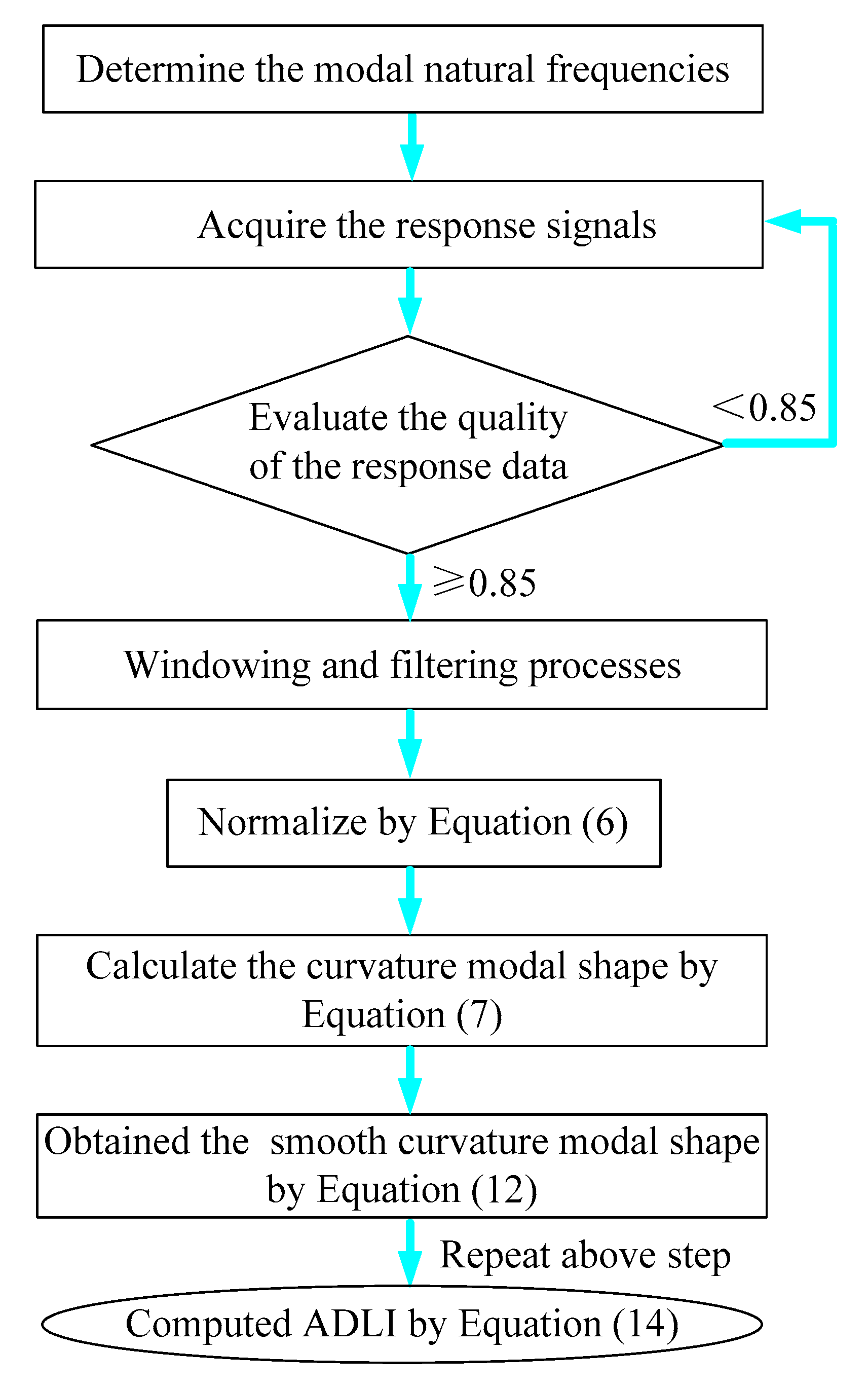
3. Damage Identification Procedure of FCTP
- Accurately determining the modal natural frequencies of the damaged FCTP.
- 2.
- Exciting the FCTP to the resonance state of the sufficiently energized, conducting laser scanning tests and acquiring the response signals.
- 3.
- Evaluating the quality of the resonance response data based on the coherence function.
- 4.
- Applying windowing and filtering processes to the resonance response signals.
- 5.
- Normalization process and obtain the curvature modal shape of some order.
- 6.
- Determine the damage location of the FCTP.
- 7.
- The data processing and analysis steps.
4. Case Study
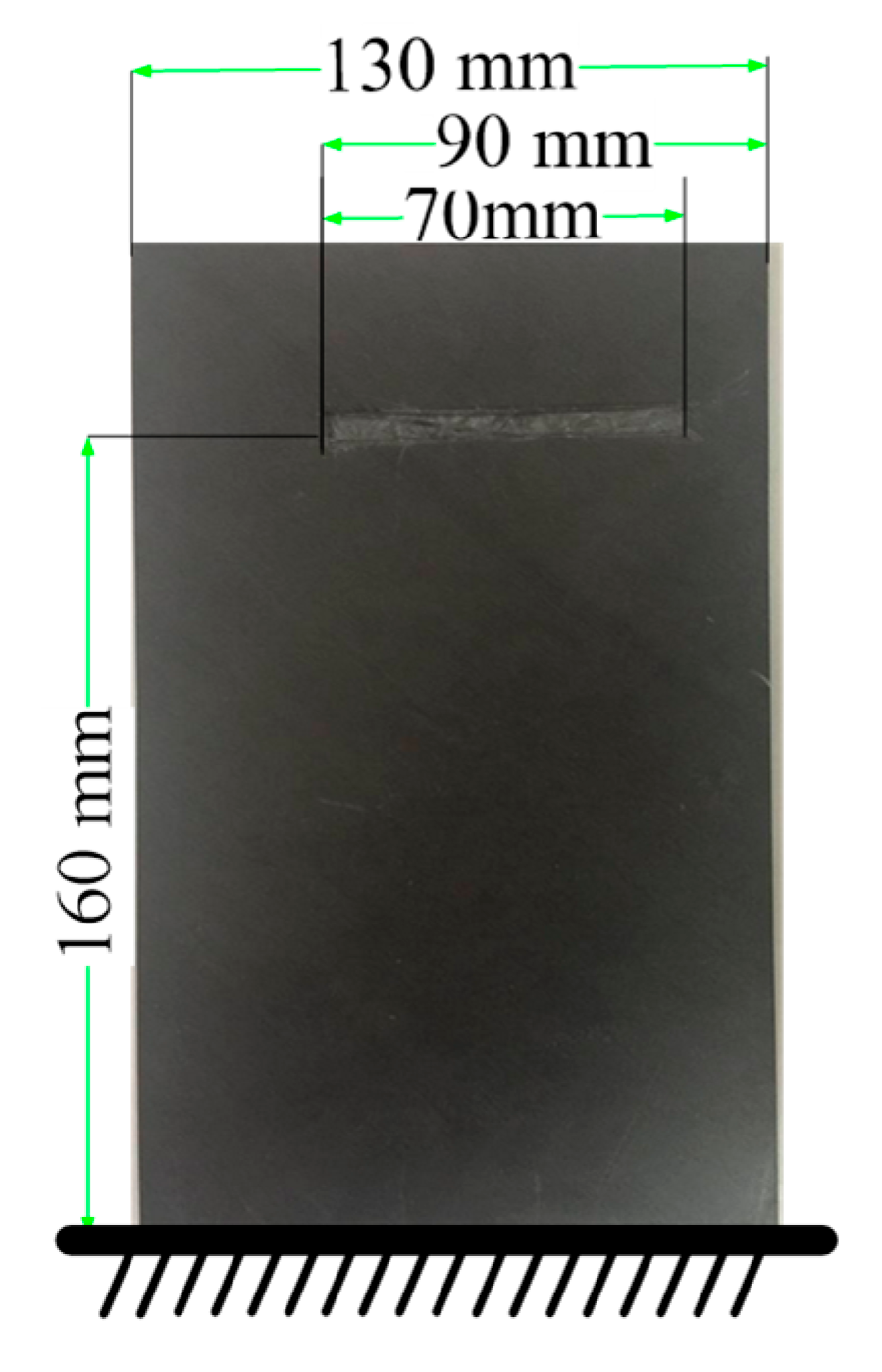
4.1. Experimental Object
4.2. Experimental Equipment
- LMS SCADAS (Siemens, Munich, Germany) 16-channel data acquisition instrument;
- LianNeng JZK-100 (Sinocera Piezotronics Inc., Zhejiang, China) electromagnetic exciter (maximum sinusoidal excitation force: 1000 N) and LianNeng YE5878 power amplifier (rated output power: 1200 W, output voltage: 40 Vrms);
- Polytec PDV-100 (Polytec GmbH, Karlsruhe, Germany) laser vibrometer (frequency range: 0–22 kHz, minimum velocity resolution: 0.05 μm/s; the light source uses a He-Ne laser (λ = 632 nm) with an output power of 1 mW and a beam diameter of 1 mm and does not cause additional damage to TC500);
- A self-developed excitation platform equipped with a B&K4508B accelerometer (Brüel & Kjær, Copenhagen, Denmark) on its surface.
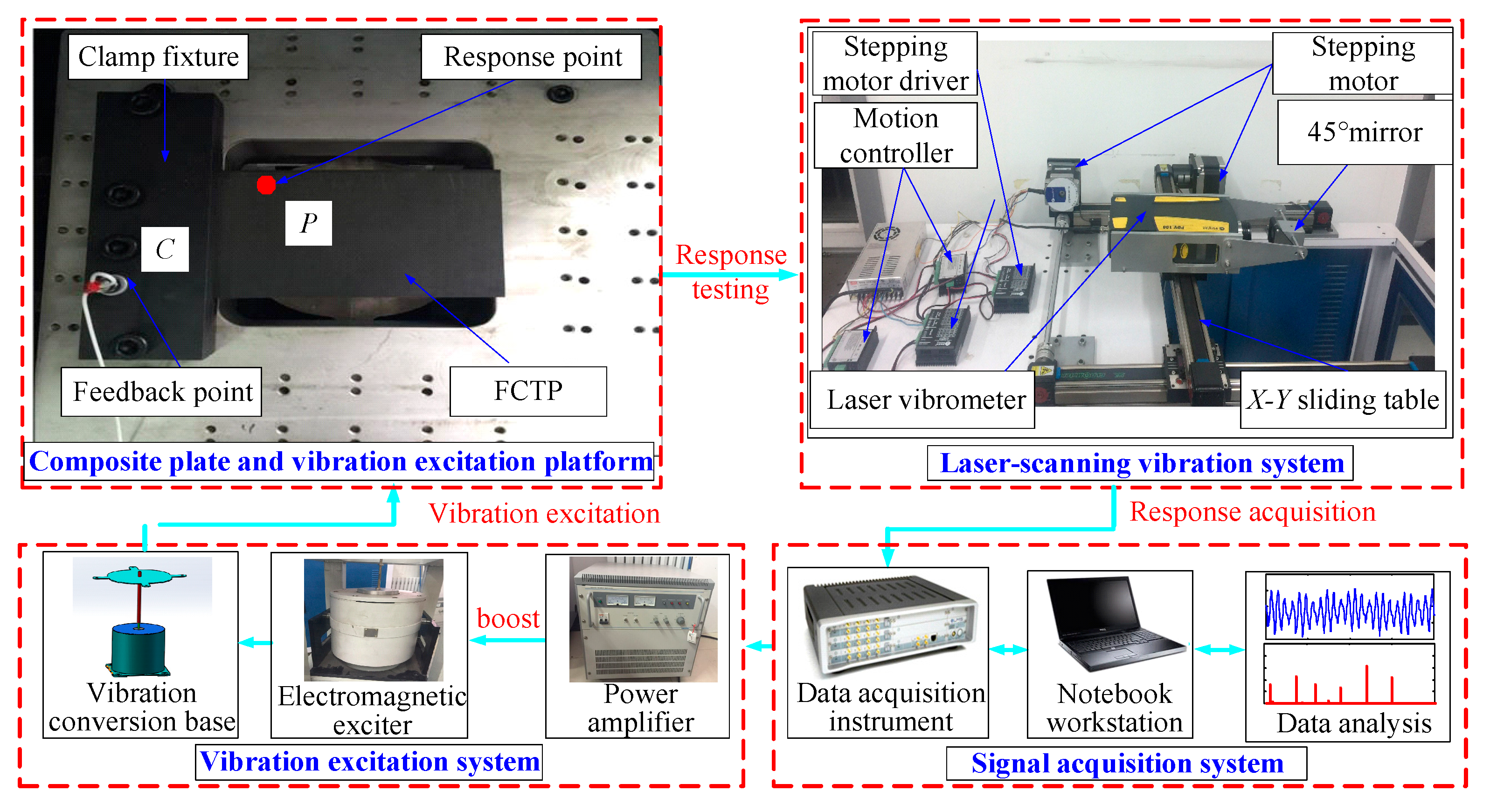
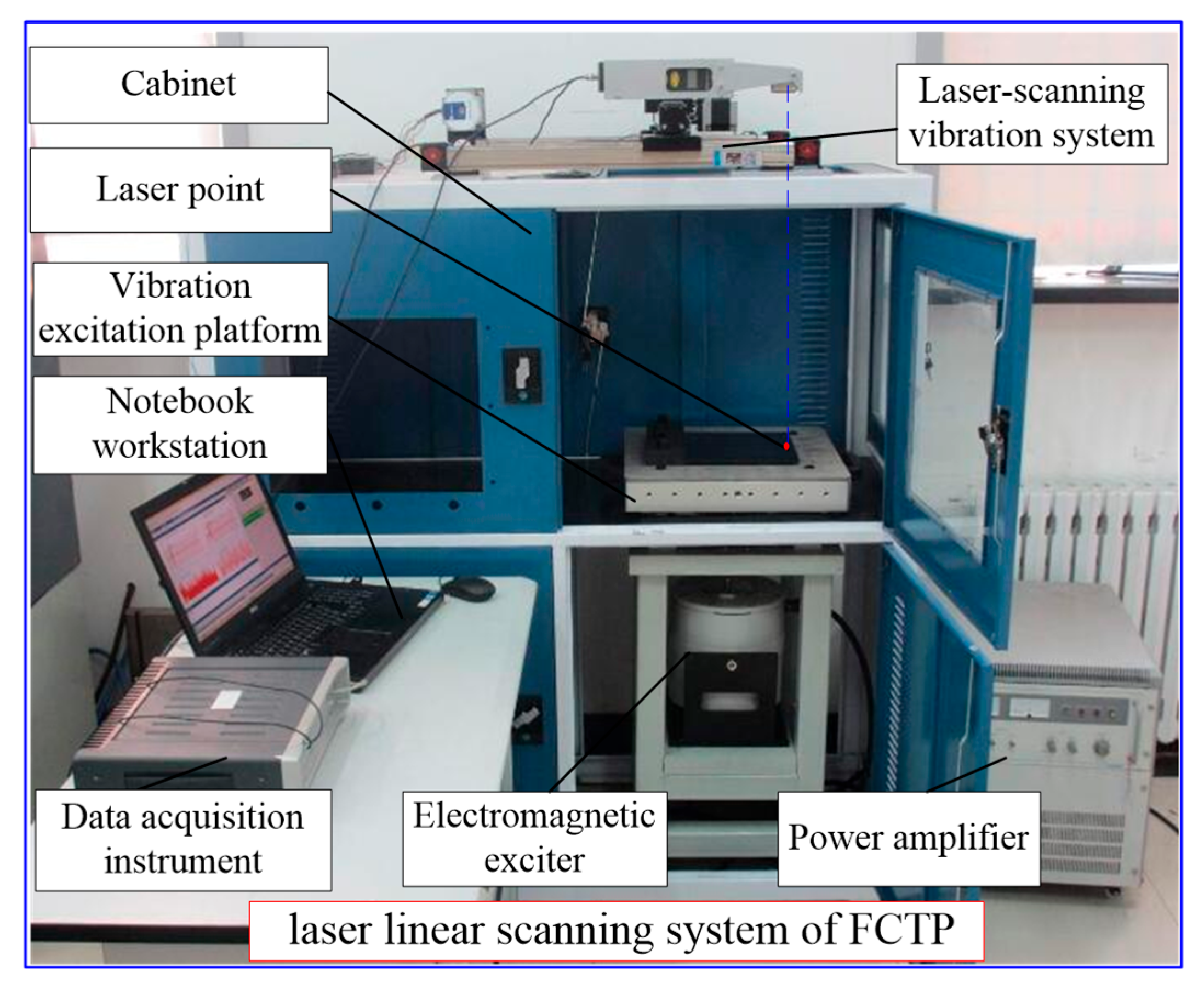
4.3. Test Results
5. Discussion
- Impact assessment of the varied constraint boundary conditions.
- 2.
- Impact assessment of the varied excitation levels.
- 3.
- Impact assessment of the varied scanning rates.
6. Conclusions
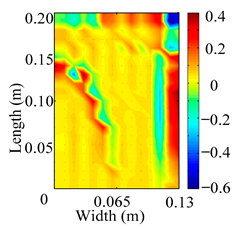
- The TC500 fiber/epoxy composite plate is utilized as an experimental object, and the damage identification experiment is performed based on the established laser scanning vibration testing system. The experimental results shows that the ADLI is significantly higher near the fiber break points than in other parts, proving that this method can indeed accurately detect the fiber breakage position of this FCTP.
- The boundary condition has a large influence on curvature modal shapes and damage localization indexes of the FCTP, so it must be clamped to ensure it is in a good constraint state by enough tightening torque. In this way, the damage localization indexes become clear and easy to identify through the CMSSM.
- When the excitation level is low, it will lead to unsatisfactory curvature modal shape results (e.g., fuzzy damage localization indexes), and with the increase in excitation level, the curvature modal shapes and the damage localization indexes become clearly identified. So, to improve the accuracy of the test, we need use a sufficient excitation level to excite the tested FCTP in the resonance state but also need to avoid too much excitation energy.
- The laser scanning rate has effects on curvature modal shapes and damage localization indexes. It can be found out that when the scanning rate is 3 mm/s, the curvature modal shapes and the damage localization indexes become clearly identified. But with the increase in scanning rate, it will lead to unsatisfactory curvature modal shapes and damage localization indexes results, and we can choose an appropriate laser scanning rate by referring to the proposed selection criterion.
- The two-dimensional laser scanning vibration system is used to accurately detect damage locations of two-dimensional plates. The accuracy of the damage location of the shell structure needs to be further verified. In the future, the application of the three-dimensional testing system for shell structure damage positioning will continue to be studied. In addition, the experimental results show that this method can accurately locate symmetrically orthogonally laid composite materials. The accuracy of using this method to detect the fiber breakage location of woven composite materials will also be studied.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CMSSM | Curvature Modal Shape Scanning Method |
| FCTP | Fiber-reinforced Composite Thin Plate |
| ADLI | Average Damage Localization Index |
References
- Morgan, P. Carbon Fibers and Their Composites; The Chemical Rubber Company Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Ren, R.; Ma, X.; Yue, H.; Yang, F.; Lu, Y. Stiffness enhancement methods for thin-walled aircraft structures: A review. Thin Walled Struct. 2024, 201, 111995. [Google Scholar] [CrossRef]
- Lopes, H.R.; Dos Santos, J.A.; Soares, C.M.; Guedes, R.M.; Vaz, M.P. A numerical-experimental method for damage location based on rotation fields spatial differentiation. Comput. Struct. 2011, 89, 1754–1770. [Google Scholar] [CrossRef]
- Nam, K.W.; Ahn, S.H.; Moon, C.K. Fracture behavior of carbon fiber reinforced plastics determined by the time–frequency analysis method. J. Appl. Polym. Sci. 2003, 88, 1659–1664. [Google Scholar] [CrossRef]
- Zhang, J.; Lin, G.; Vaidya, U.; Wang, H. Past, present and future prospective of global carbon fibre composite developments and applications. Compos. Part B Eng. 2023, 250, 110463. [Google Scholar] [CrossRef]
- Ge, X.; Zhang, P.; Zhao, F.; Liu, M.; Liu, J.; Cheng, Y. Experimental and numerical investigations on the dynamic response of woven carbon fiber reinforced thick composite laminates under low-velocity impact. Compos. Struct. 2022, 279, 114792. [Google Scholar] [CrossRef]
- Maimı, P.; Camanho, P.P.; Mayugo, J.A.; Turon, A. Matrix cracking and delamination in laminated composites. Part I: Ply constitutive law, first ply failure and onset of delamination. Mech. Mater. 2011, 43, 169–185. [Google Scholar] [CrossRef]
- Kumar, D.; Roy, R.; Kweon, J.H.; Choi, J.H. Numerical modeling of combined matrix cracking and delamination in composite laminates using cohesive elements. Appl. Compos. Mater. 2016, 23, 397–419. [Google Scholar] [CrossRef]
- Shah, V.; Zadourian, S.; Yang, C.; Zhang, Z.; Gu, G.X. Data-driven approach for the prediction of mechanical properties of carbon fiber reinforced composites. Mater. Adv. 2022, 3, 7319–7327. [Google Scholar] [CrossRef]
- Doebling, S.W.; Farrar, C.R.; Prime, M.B. A summary review of vibration-based damage identification methods. Shock Vib. Dig. 1998, 30, 91–105. [Google Scholar] [CrossRef]
- Zou, Y.; Tong, L.; Steven, G.P. Vibration-based model-dependent damage (delamination) identification and health monitoring for composite structures—A review. J. Sound Vib. 2000, 203, 357–378. [Google Scholar] [CrossRef]
- Kessler, S.S.; Spearing, S.M.; Atalla, M.J.; Cesnik, C.E.S.; Soutis, C. Damage detection in composite materials using frequency response methods. Compos. Part B Eng. 2002, 33, 87–95. [Google Scholar] [CrossRef]
- Hu, H.; Wang, J. Damage detection of a woven fabric composite laminate using a modal strain energy method. Eng. Struct. 2009, 31, 1042–1055. [Google Scholar] [CrossRef]
- Li, H.; Xu, Z.H.; Wang, D.S.; Zu, X.D. Damage Location of Fiber-Reinforced Composites Based on Lumped Mass Approach. J. Northeast. Univ. (Nat. Sci.) 2020, 41, 234. [Google Scholar]
- Zhu, C.; Li, G.; Yu, X.; Zhao, G.; Yang, J. Vibration power flow based damage detection of fiber-reinforced laminated composite plates. Mech. Adv. Mater. Struct. 2024, 2024, 1–15. [Google Scholar] [CrossRef]
- Roy, K.; Agrawal, S.; Bhattacharya, B.; Ray-Chaudhuri, S. Fundamental mode shape to localize delamination in cantilever composite plates using laser doppler vibrometer. In Advances in Structural Engineering: Materials; Springer Nature: New Delhi, India, 2015; pp. 2621–2633. [Google Scholar]
- Vanlanduit, S.; Guillaume, P.; Schoukens, J.; Parloo, E. Linear and nonlinear damage detection using a scanning laser vibrometer. Shock Vib. 2002, 9, 43–56. [Google Scholar] [CrossRef]
- Lieven, N.A.J.; Ewins, D.J. Spatial correlation of mode shapes, the coordinate modal assurance criterion (COMAC). In Proceedings of the 6th International Modal Analysis Conference, Kissimmee, FL, USA, 1–4 February 1988. [Google Scholar]
- Pandey, A.K.; Biswas, M.; Samman, M.M. Damage detection from changes in curvature mode shapes. J. Sound Vib. 1991, 145, 321–332. [Google Scholar] [CrossRef]
- Peng, H.; You, C.H.; Meng, Y. Damage Diagnosis of Beam Stuctures by Modal Curvature Difference Method. Eng. Mech. 2006, 23, 49–53. [Google Scholar]
- Govindasamy, M.; Kamalakannan, G.; Kesavan, C.; Meenashisundaram, G.K. Damage detection in glass/epoxy laminated composite plates using modal curvature for structural health monitoring applications. J. Compos. Sci. 2020, 4, 185. [Google Scholar] [CrossRef]
- Pacheco-Chérrez, J.; Cárdenas, D.; Probst, O. Experimental detection and measurement of crack-type damage features in composite thin-wall beams using modal analysis. Sensors 2021, 21, 8102. [Google Scholar] [CrossRef]
- Ratcliffe, C.P. Damage detection using a modified Laplacian operator on mode shape data. J. Sound Vib. 1997, 204, 505–517. [Google Scholar] [CrossRef]
- Ratcliffe, C.P.; Bagaria, W.J. Vibration technique for locating delamination in a composite beam. AIAA J. 1998, 36, 1074–1077. [Google Scholar] [CrossRef]
- Ratcliffe, C.P. A frequency and curvature based experimental method for locating damage in structures. J. Vib. Acoust. 2000, 122, 324–329. [Google Scholar] [CrossRef]
- Yang, Z.C.; Dang, X.J.; Wang, L.; Ding, Y. Experimental research of combined damage location method for composite material. J. Vib. Meas. Diagn. 2010, 30, 115–118. [Google Scholar]
- Yoon, M.K.; Heider, D.; Gillespie, J.W., Jr.; Ratcliffe, C.P.; Crane, R.M. Local damage detection using the two-dimensional gapped smoothing method. J. Sound Vib. 2005, 279, 119–139. [Google Scholar] [CrossRef]
- Li, H.; Sun, W.; Xu, Z.; Han, Q.K. An experimental method of laser rotating canning to measure mode shape of constrained thin cylindrical shell. J. Vib. Shock 2014, 33, 155–159. [Google Scholar]
- Qiao, P.; Lu, K.; Lestari, W.; Wang, J. Curvature mode shape-based damage detection in composite laminated plates. Compos. Struct. 2007, 80, 409–428. [Google Scholar] [CrossRef]
- Li, H.; Chang, Y.; Xu, Z.; Zhu, Q.; Wen, B. Modal shape measurement of fiber-reinforced composite plate with high efficiency and precision based on laser linear scanning method. Meas. Control 2018, 51, 470–487. [Google Scholar] [CrossRef]




| Modal Order | Natural Frequency | Modal Shape | Curvature Modal Shapes | Smooth Curvature Modal Shapes |
|---|---|---|---|---|
| 1 | 31.0 Hz | 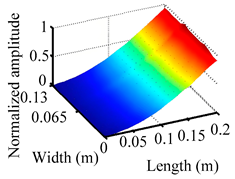 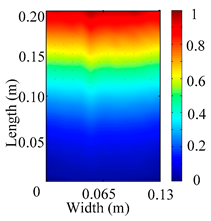 | 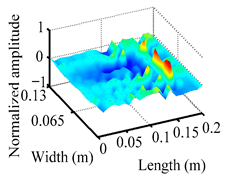 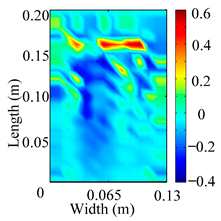 | 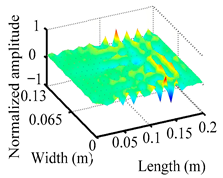  |
| 2 | 175.5 Hz | 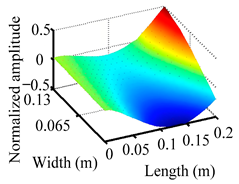 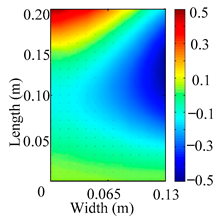 | 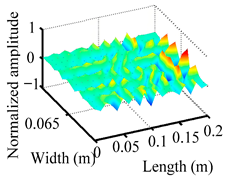 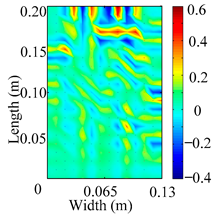 | 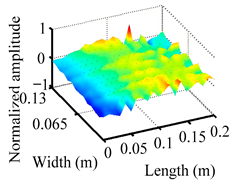 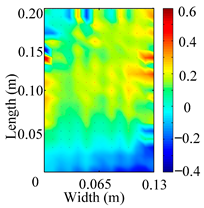 |
| 3 | 193.5 Hz | 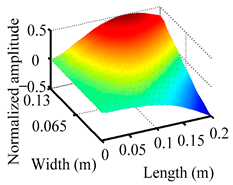 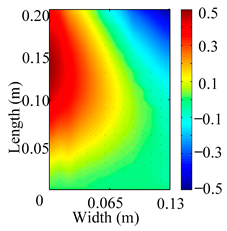 | 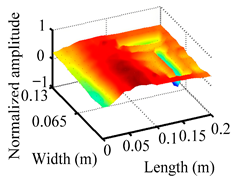  | 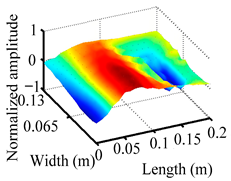  |
| 4 | 499.5 Hz | 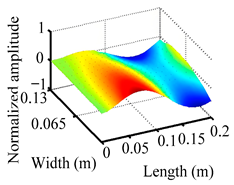 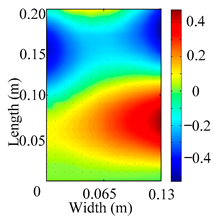 |   | 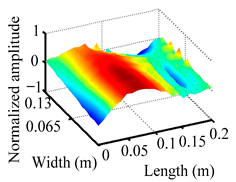 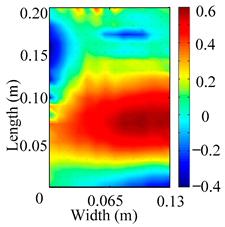 |
| 5 | 580.5 Hz | 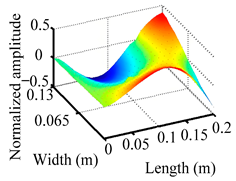 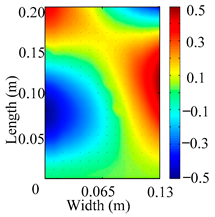 | 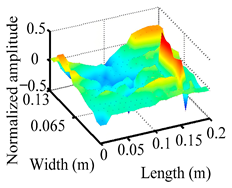 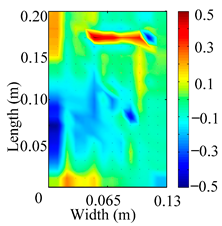 |  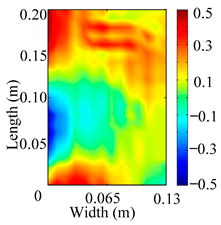 |
| Constraint Boundary I (5 Nm Tightening Torque) | Constraint Boundary II (30 Nm Tightening Torque) | Constraint Boundary III (50 Nm Tightening Torque) |
|---|---|---|
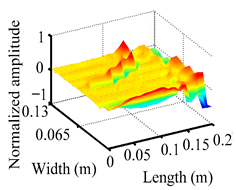  | 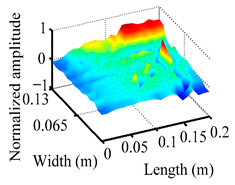 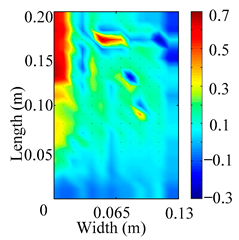 | 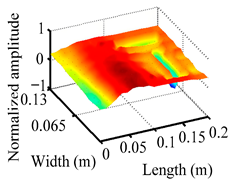 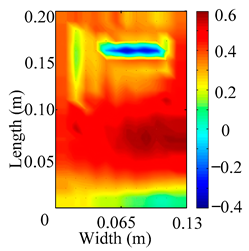 |
| Constraint Boundary I (5 Nm Tightening Torque) | Constraint Boundary II (30 Nm Tightening Torque) | Constraint Boundary III (50 Nm Tightening Torque) |
|---|---|---|
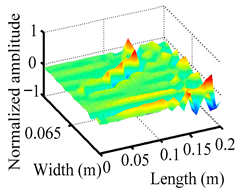 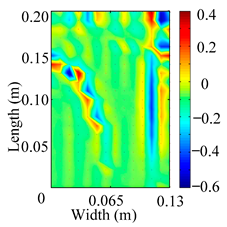 |   | 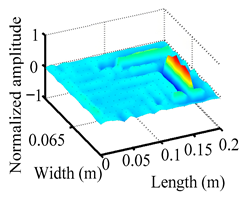 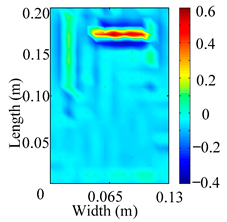 |
| Excitation Level | Natural Frequency (Hz) | Curvature Modal Shapes | Damage Localization Indexes |
|---|---|---|---|
| 200 N | 501.0 |  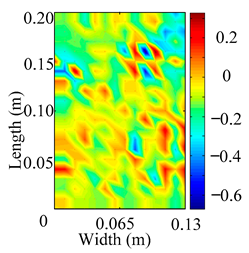 | 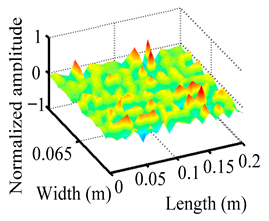  |
| 490 N | 500.2 | 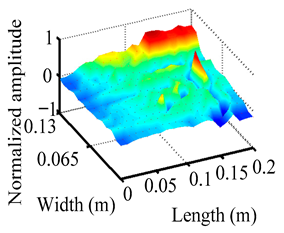 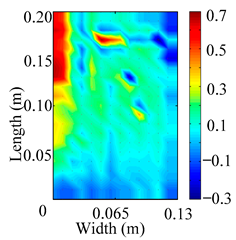 | 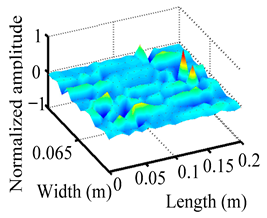 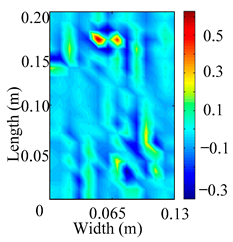 |
| 1100 N | 499.5 | 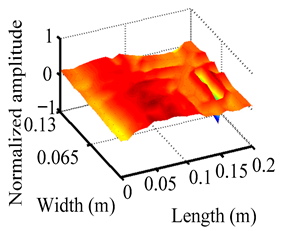 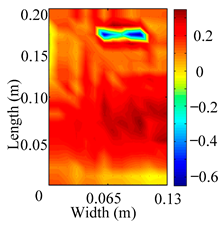 |  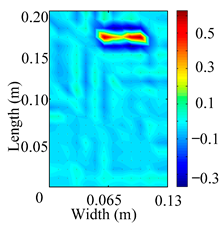 |
| 1600 N | 498.3 | 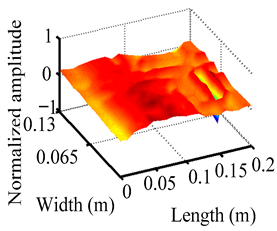  |   |
| Laser Scanning Rate of 3 mm/s | Laser Scanning Rate of 10 mm/s | Laser Scanning Rate of 20 mm/s |
|---|---|---|
 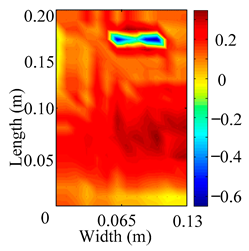 | 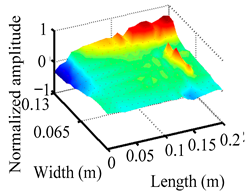 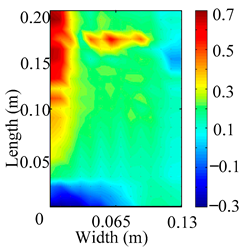 | 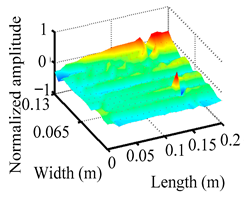 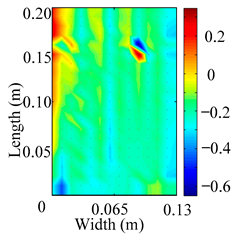 |
| Laser Scanning Rate of 3 mm/s | Laser Scanning Rate of 10 mm/s | Laser Scanning Rate of 20 mm/s |
|---|---|---|
 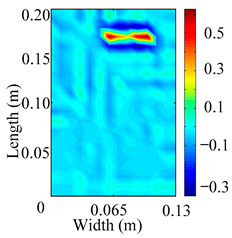 | 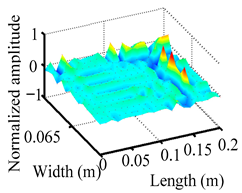  | 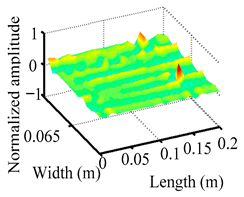  |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chang, Y.; Zhao, Q.; Han, H.; Zhao, X.; Qin, L.; Li, X.; Wu, L.; Li, H. Damage Identification of Fiber-Reinforced Composite Thin Plate by Curvature Modal Shape Scanning Method. Materials 2025, 18, 2431. https://doi.org/10.3390/ma18112431
Chang Y, Zhao Q, Han H, Zhao X, Qin L, Li X, Wu L, Li H. Damage Identification of Fiber-Reinforced Composite Thin Plate by Curvature Modal Shape Scanning Method. Materials. 2025; 18(11):2431. https://doi.org/10.3390/ma18112431
Chicago/Turabian StyleChang, Yougle, Qi Zhao, Hao Han, Xiaodi Zhao, Lingyao Qin, Xiaoye Li, Liyan Wu, and Hui Li. 2025. "Damage Identification of Fiber-Reinforced Composite Thin Plate by Curvature Modal Shape Scanning Method" Materials 18, no. 11: 2431. https://doi.org/10.3390/ma18112431
APA StyleChang, Y., Zhao, Q., Han, H., Zhao, X., Qin, L., Li, X., Wu, L., & Li, H. (2025). Damage Identification of Fiber-Reinforced Composite Thin Plate by Curvature Modal Shape Scanning Method. Materials, 18(11), 2431. https://doi.org/10.3390/ma18112431








