Endogenous–Exogenous Analyses of the Solidification Structure in 475 mm Extra-Thick Slabs: Columnar-to-Equiaxed Positioning and Effect of Strand Electromagnetic Stirring
Abstract
1. Introduction
2. Methodology Descriptions
2.1. Heat Transfer and Solidification Model
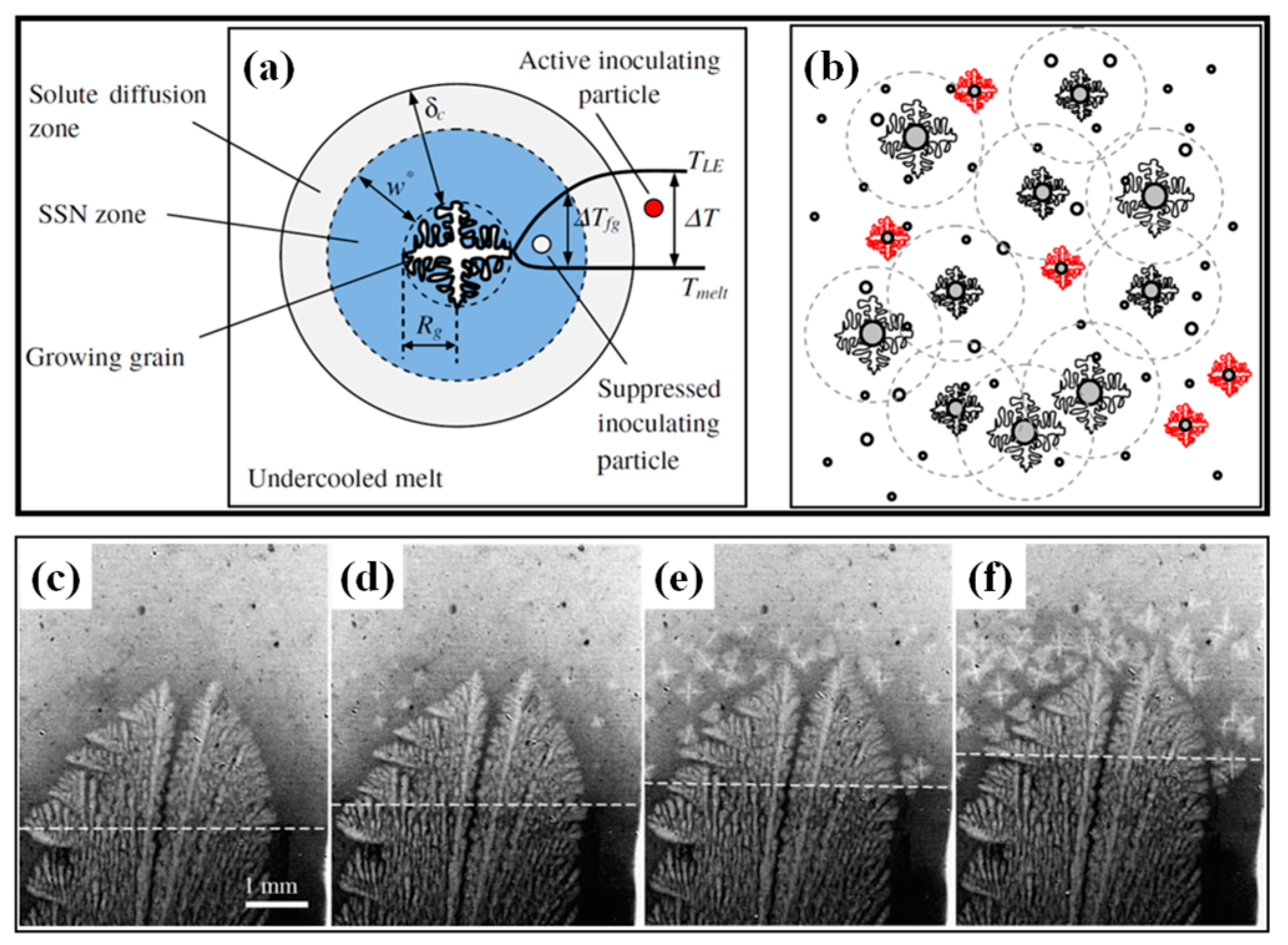
2.2. Grain Nucleation Model
2.3. Dendrite Tip Growth Kinetics Model
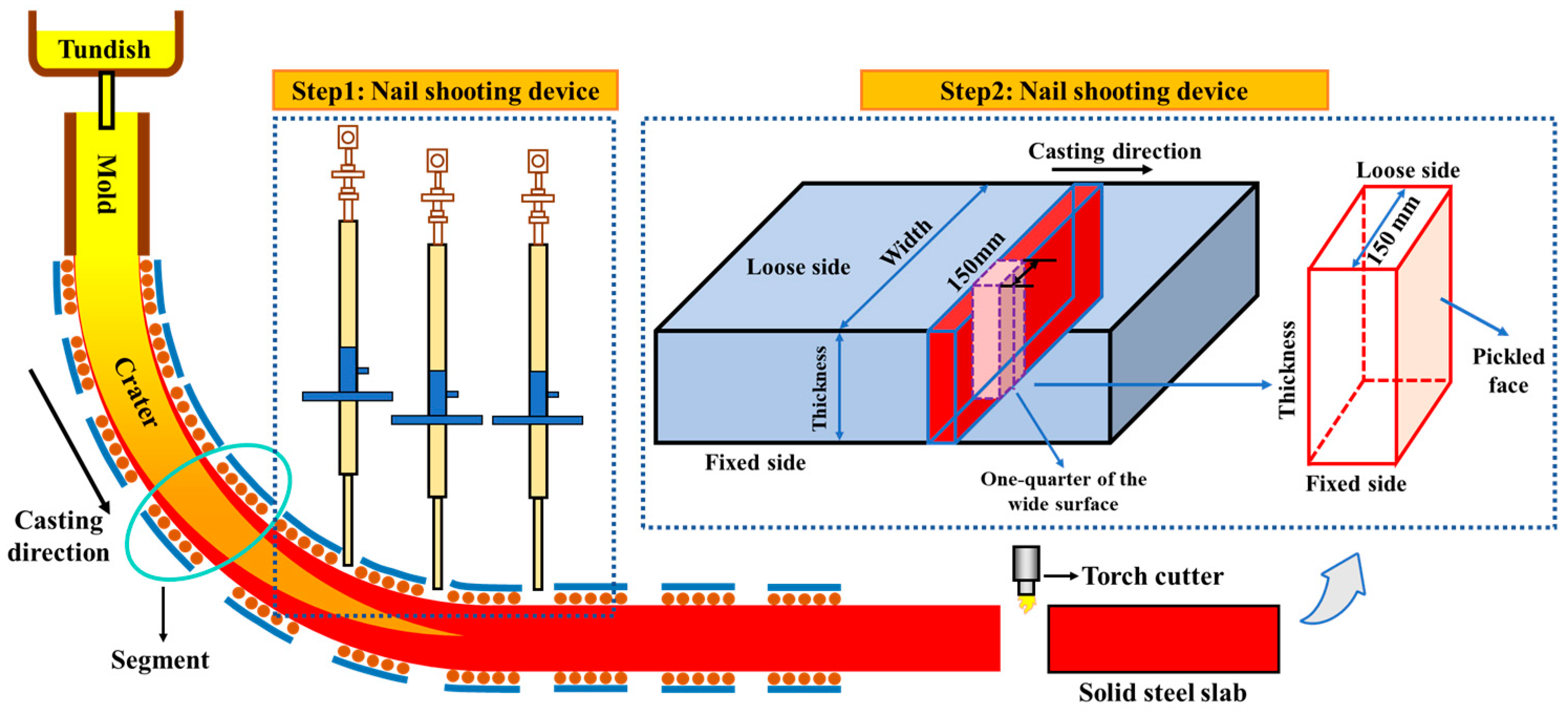
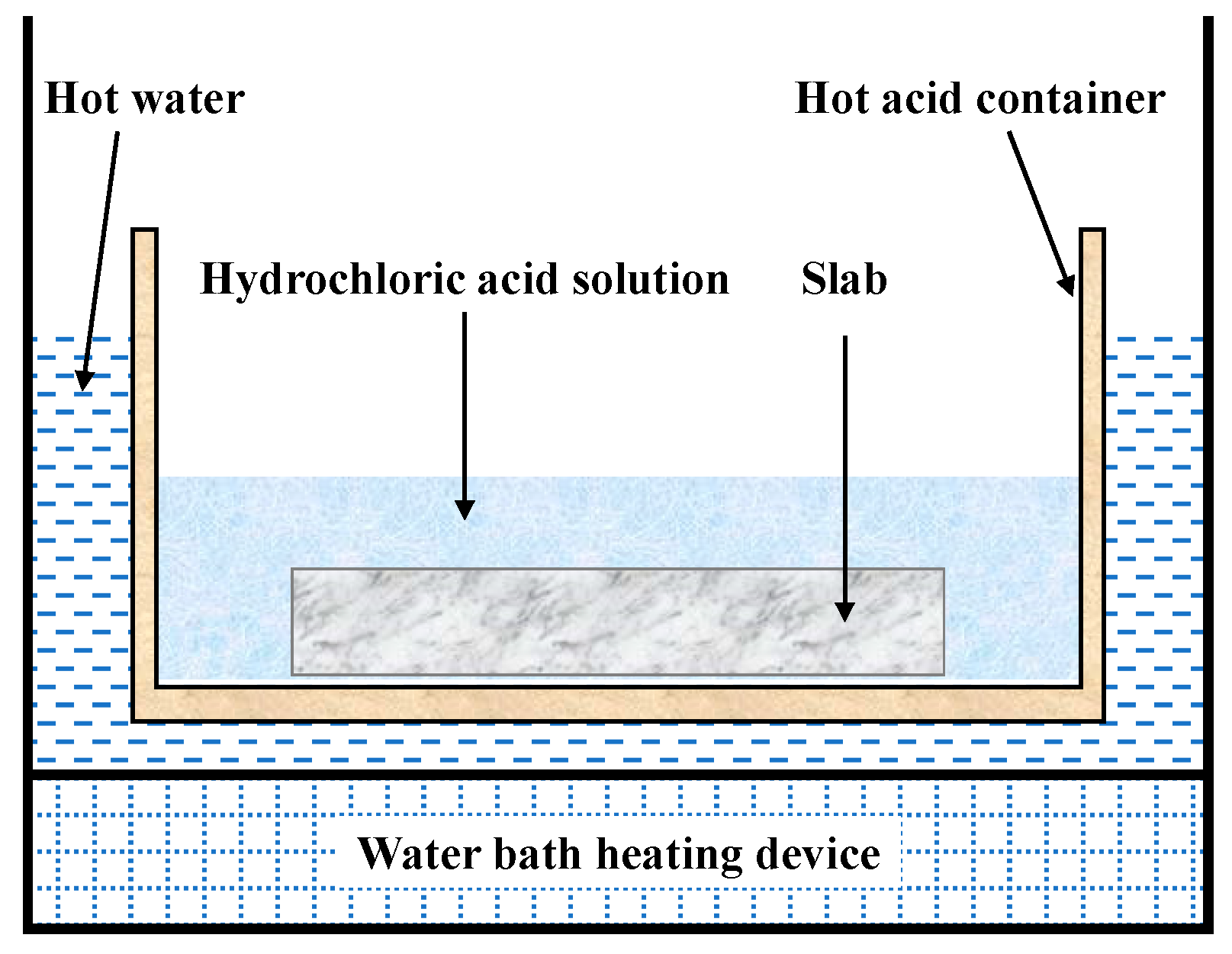
3. Experimental Conditions and Model Verification
3.1. Thermo-Physical Properties of Steel
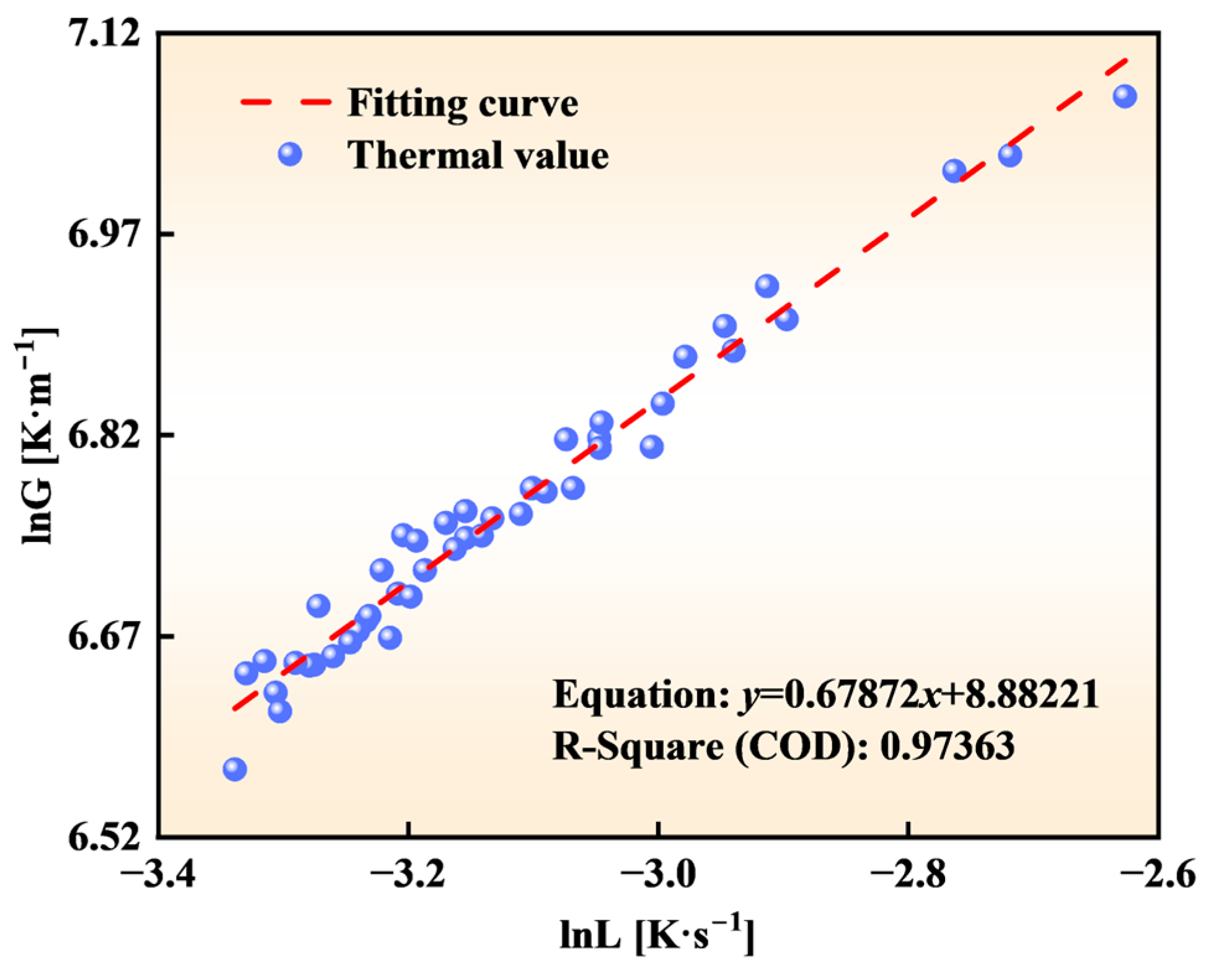
3.2. Verification of the Mathematical Models
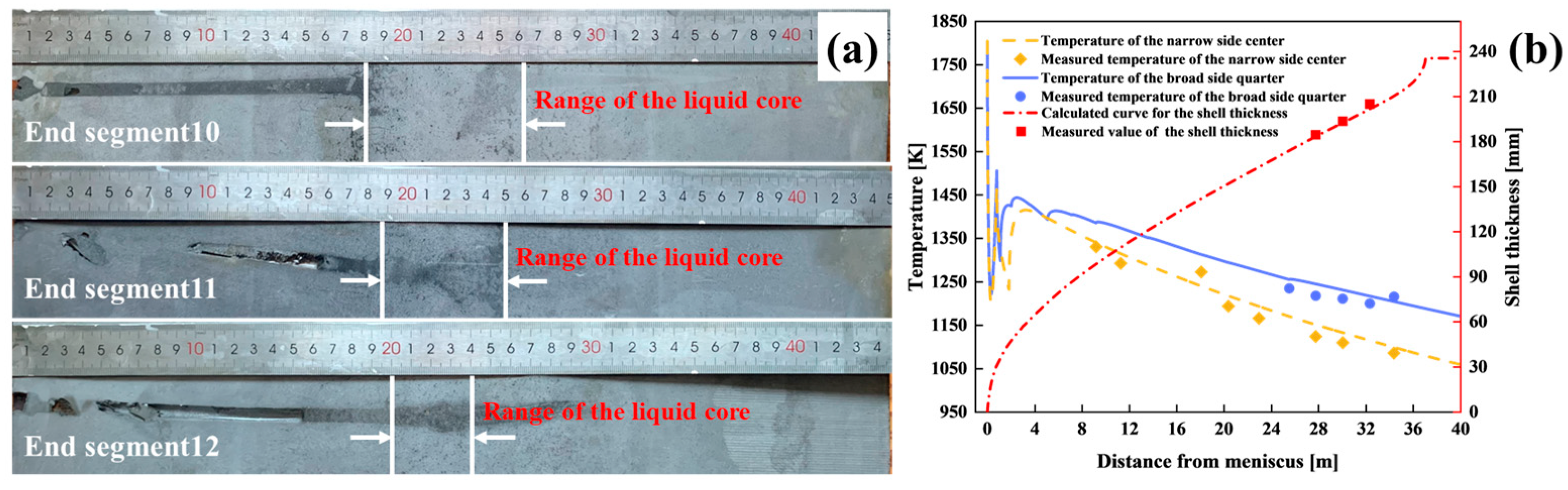
3.2.1. Verification of the Solidification Heat Transfer Model
3.2.2. Validation of the Solidification Structure Model
4. Results and Discussion
4.1. Columnar-to-Equiaxed Crystal Transition Analysis
4.1.1. Temperature Conditions at the CET Initiation Position
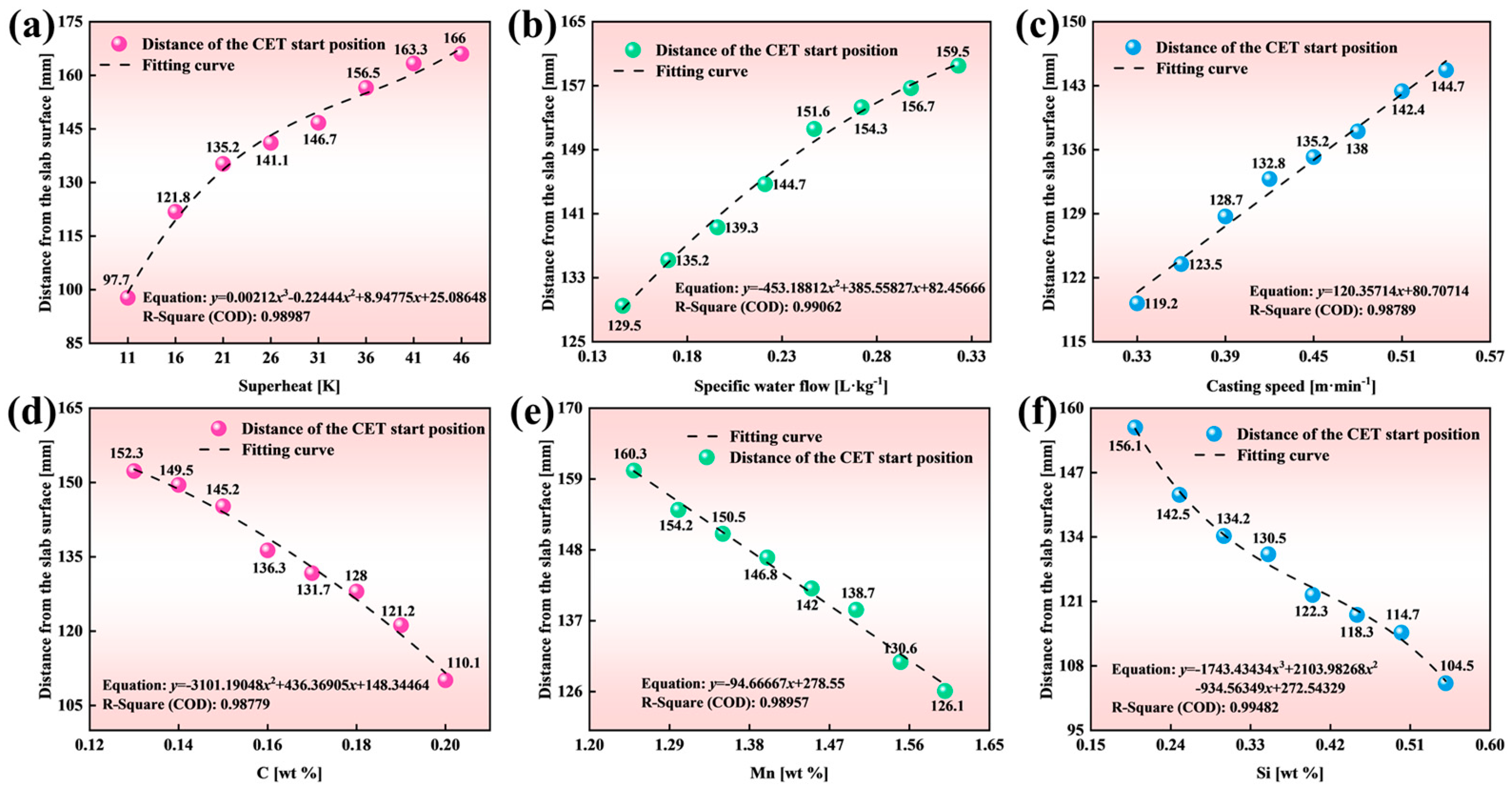
4.1.2. Relationship Between the CET Initiation Position and Parameters
4.2. Analysis of the Effects of S-EMS on the Internal Structure and Quality of the Extra-Thick Slab
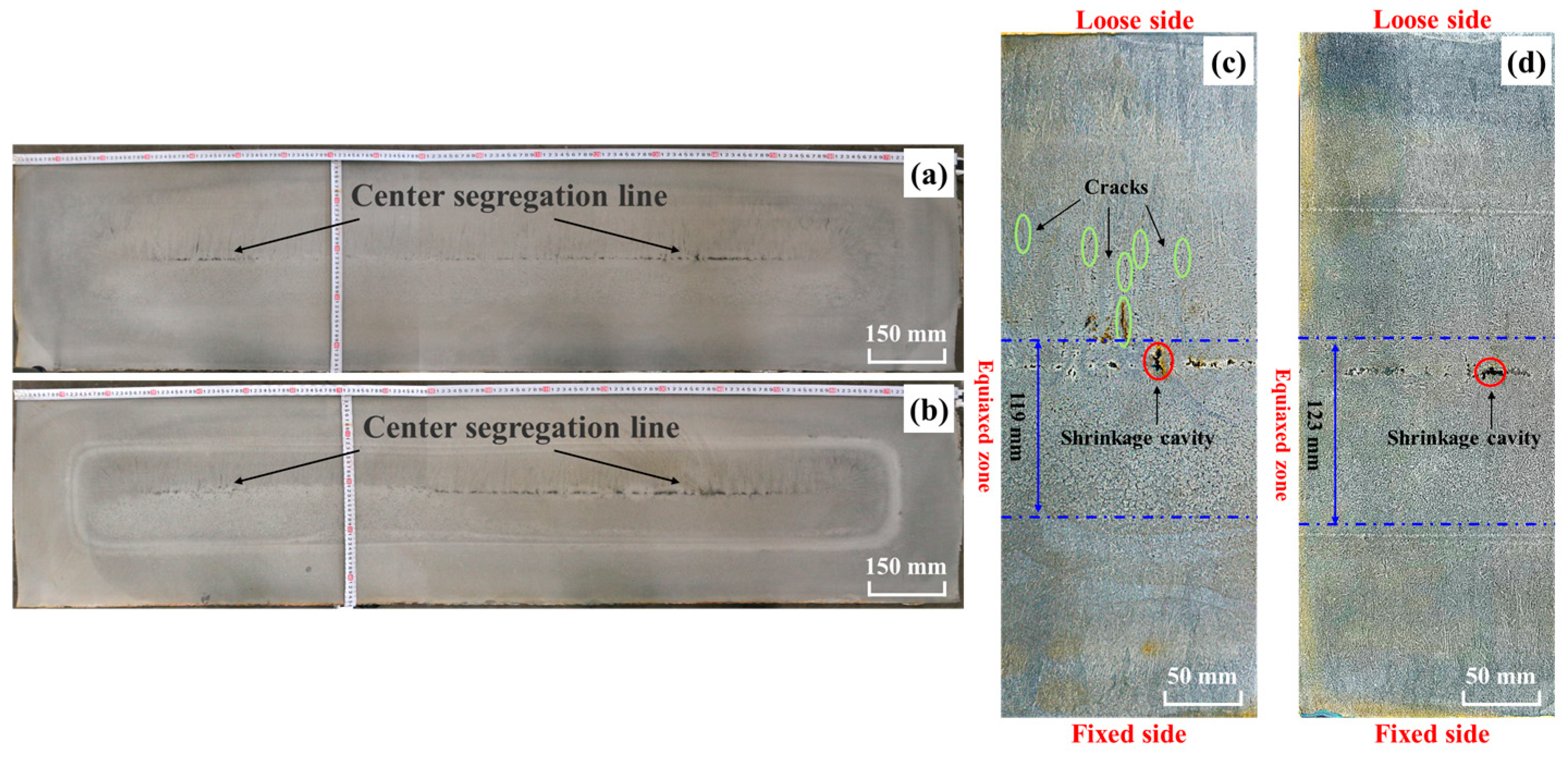
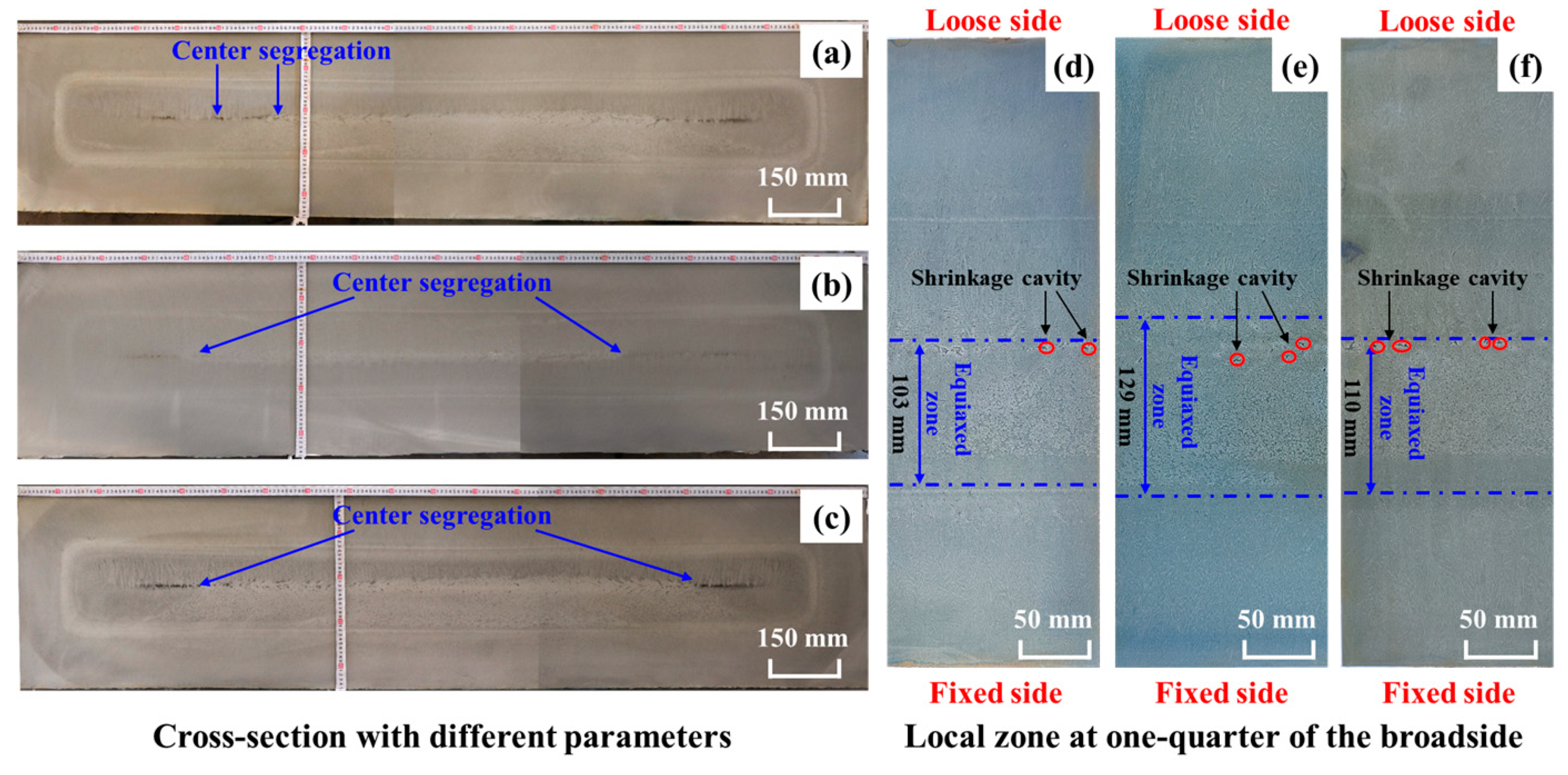
4.2.1. Analysis of the Effect S-EMS on Internal Quality
4.2.2. Analysis of the Effect of S-EMS on the Solidification Structure
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| fS | solid fraction | ΔTv,max | volume nucleation undercooling |
| C | carbon | ΔTv,σ | standard deviation of the volume during undercooling |
| Mn | manganese | nv | volume nucleation density |
| Si | silicon | c0 | composition of molten steel |
| T | local temperature, K | k | equilibrium solute partition coefficient |
| l | thermal conductivity, W·m−1·K−1 | G | Gibbs–Thompson coefficient |
| ρ | density, kg·m−3 | M | liquidus slope |
| H | enthalpy, kJ·kg−1 | D | solute diffusion coefficient |
| LP | latent heat, J·kg−1 | Mmax | maximum value |
| cp | specific heat, J·kg−1·K−1 | Mmin | minimum value |
| T0 | initial temperature, K | G | temperature gradient at the dendrite tip, K·m−1 |
| T1 | casting temperature, K | N0 | heterogeneous nucleation density, m−3 |
| qm | heat flux of the mold, kW·m−2 | ΔTN | heterogeneous nucleation undercooling, K |
| B | coefficient related to mold cooling conditions, kW·m−2·s−1/2 | ΔTC | undercooling at the dendrite tip, K |
| t | residence time of molten steel in the mold, s | V | dendrite tip growth rate, m·s−1 |
| qa | average heat flux, kW·m−2 | ΔT0 | temperature difference between the equilibrium liquid phases, ΔT0 = c0M(k − 1)/k |
| l | length of the mold, m | a1 | constant associated with the alloy system |
| V | casting speed, m·s−1 | L | cooling rate, K·s−1 |
| ΔTn | mean undercooling, K | y | distance from the CET beginning position to the slab surface, mm |
| ΔTσ | standard deviation of undercooling, K | x1 | superheat, K |
| Nmax | maximum nucleation density, m−3 | x2 | specific water flow, L·kg−1 |
| ΔT | undercooling, K | x3 | actual casting speed, m·min−1 |
| ΔTs,max | surface nucleation undercooling | x4 | C content, wt.% |
| ΔTs,σ | standard deviation of the surface during undercooling | x5 | Mn content, wt.% |
| ns | surface nucleation density | x6 | Si content, wt.%. |
References
- Zhong, H.; Wang, R.; Han, Q.; Fang, M.; Yuan, H.; Song, L.; Xie, X.; Zhai, Q. Solidification Structure and Central Segregation of 6Cr13Mo Stainless Steel under Simulated Continuous Casting Conditions. J. Mater. Res. Technol. 2022, 20, 3408–3419. [Google Scholar] [CrossRef]
- Zhang, L.; Shi, Y.; Hu, L.; Wang, L.; Hu, F. Impact of Multi-Level Microstructures on the Strength and Yield Ratio of Extra-Thick Ultra-High-Strength Steel. J. Mater. Eng. Perform. 2023, 32, 10344–10353. [Google Scholar] [CrossRef]
- Lu, Y.; Jiang, J.; Zhang, Q.; Cai, W.; Chen, W.; Ye, J. Experimental and Numerical Investigation on Mechanical Behavior of Q355 Steel and Connections in Fire Conditions. Eng. Struct. 2023, 281, 115765. [Google Scholar] [CrossRef]
- Wang, G.; Liu, Z.; Zhang, D.; Chu, M. Innovation infrastructure and research progress of iron and steel enterprises. Iron Steel 2023, 58, 2–14. [Google Scholar] [CrossRef]
- Xu, L.; Zhang, P.; Shuai, Y.; Shi, P.; Zhan, Z.; Wang, M. Study of Process Parameters on Solidification Structure and Centre Grain Size of 2311 in 420 Mm Extra-Thick Continuously Cast Slabs. Metals 2022, 13, 47. [Google Scholar] [CrossRef]
- Tian, N.; Zhang, G.; Yan, P.; Li, P.; Feng, Z.; Wang, X. Simulation and Experimental Study on the Effect of Superheat on Solidification Microstructure Evolution of Billet in Continuous Casting. Materials 2024, 17, 682. [Google Scholar] [CrossRef]
- Gu, S.; Sun, M.; Wang, B.; Zhang, J. Simulation and Experimental Study of Fluid Flow and Solidification Behavior in Thin Slabs Continuous Casting Process under Secondary Electromagnetic Stirring. Steel Res. Int. 2024, 95, 2300398. [Google Scholar] [CrossRef]
- Li, P.; Zhang, G.; Yan, P.; Zhang, P.; Tian, N.; Feng, Z. Numerical and Experimental Study on Carbon Segregation in Square Billet Continuous Casting with M-EMS. Materials 2023, 16, 5531. [Google Scholar] [CrossRef]
- Pan, Q.; Jin, W.; Huang, S.; Guo, Y.; Jiang, M.; Li, X. Simulation and Study of Influencing Factors on the Solidification Microstructure of Hazelett Continuous Casting Slabs Using CAFE Model. Materials 2024, 17, 1869. [Google Scholar] [CrossRef]
- Hunt, J.D. Steady State Columnar and Equiaxed Growth of Dendrites and Eutectic. Mater. Sci. Eng. 1984, 65, 75–83. [Google Scholar] [CrossRef]
- Feng, S.; Liotti, E.; Grant, P.S. X-Ray Imaging of Alloy Solidification: Crystal Formation, Growth, Instability and Defects. Materials 2022, 15, 1319. [Google Scholar] [CrossRef]
- Martorano, M.A.; Beckermann, C.; Gandin, C.-A. A Solutal Interaction Mechanism for the Columnar-to-Equiaxed Transition in Alloy Solidification. Metall. Mater. Trans. A 2003, 34, 1657–1674. [Google Scholar] [CrossRef]
- Hou, Z.; Yao, S.; Li, T.; Sha, M. Study on Columnar to Equiaxed Transition of Aluminium Alloy. T Mater. Heat Treat. 2013, 34, 120–125. [Google Scholar] [CrossRef]
- Niu, L.; Qiu, S.-T.; Zhao, J.-X.; Chen, Y.-Q.; Yang, S.-F. Processing Parameter Optimization for Continuous Casting of 38CrMoAl Round Bloom Based on a Prediction Model of the Equiaxed Crystal Ratio. Ironmak. Steelmak. 2019, 46, 835–844. [Google Scholar] [CrossRef]
- Luo, S.; Zhu, M.; Louhenkilpi, S. Numerical Simulation of Solidification Structure of High Carbon Steel in Continuous Casting Using Cellular Automaton Method. ISIJ Int. 2012, 52, 823–830. [Google Scholar] [CrossRef]
- Shibata, H.; Itoyama, S.; Kishimoto, Y.; Takeuchi, S.; Sekiguchi, H. Prediction of Equiaxed Crystal Ratio in Continuously Cast Steel Slab by Simplified Columnar-to-Equiaxed Transition Model. ISIJ Int. 2006, 46, 921–930. [Google Scholar] [CrossRef][Green Version]
- Lekakh, S.N.; O’Malley, R.; Emmendorfer, M.; Hrebec, B. Control of Columnar to Equiaxed Transition in Solidification Macrostructure of Austenitic Stainless Steel Castings. ISIJ Int. 2017, 57, 824–832. [Google Scholar] [CrossRef]
- Kardani, A. Strain-Rate-Dependent Plasticity of Ta-Cu Nanocomposites for Therapeutic Implants. Sci. Rep. 2023, 13, 15788. [Google Scholar] [CrossRef]
- Kardani, A. Unraveling the Temperature-Dependent Plastic Deformation Mechanisms of Polycrystalline Ta Implants through Numerical Analysis of Grain Boundary Dynamics. J. Mater. Sci. 2022, 57, 16490–16506. [Google Scholar] [CrossRef]
- Liu, D.; Zhang, G.; Zeng, J.; Xie, X. Numerical Simulation of Segregation in Slabs under Different Secondary Cooling Electromagnetic Stirring Modes. Materials 2024, 17, 2721. [Google Scholar] [CrossRef]
- Yao, C.; Wang, M.; Ni, Y.; Gong, J.; Xing, L.; Zhang, H.; Bao, Y. Effects of Secondary Cooling Segment Electromagnetic Stirring on Solidification Behavior and Composition Distribution in High-Strength Steel 22MnB5. JOM 2022, 74, 4823–4830. [Google Scholar] [CrossRef]
- Wu, S.; Wan, Y.; Yu, Y.; Chen, W. Effect of electromagnetic stirring in secondary cooling zone on quality of continuous casting slab of non-oriented silicon steel. Steelmaking 2012, 28, 11–14+24. [Google Scholar]
- Xiao, Z.; Zhang, G.; Liu, D.; Wu, C. Impact of Electromagnetic Stirring Roller Arrangement Pattern on Magnetic Field Simulation and Solidification Structure of PW800 Steel in the Second Cooling Zone. Materials 2024, 17, 1038. [Google Scholar] [CrossRef]
- Hu, W.; Dong, Z.; Ji, C.; Zhu, M. Influence of External Physical Fields on Grain Distribution in Continuous Cast Round Blooms. Metall. Mater. Trans. B 2024, 55, 745–757. [Google Scholar] [CrossRef]
- Wang, Z.; Luo, S.; Wang, W.; Zhu, M. Numerical Simulation of Solidification Structure of Continuously Cast Billet with Grain Motion. Metall. Mater. Trans. B 2020, 51, 2882–2894. [Google Scholar] [CrossRef]
- Abou-Khalil, L.; Salloum-Abou-Jaoude, G.; Reinhart, G.; Pickmann, C.; Zimmermann, G.; Nguyen-Thi, H. Influence of Gravity Level on Columnar-to-Equiaxed Transition during Directional Solidification of Al–20 wt.% Cu Alloys. Acta Mater. 2016, 110, 44–52. [Google Scholar] [CrossRef]
- Esaka, H.; Wakabayashi, T.; Shinozuka, K.; Tamura, M. Origin of Equiaxed Grains and Their Motion in the Liquid Phase. ISIJ Int. 2003, 43, 1415–1420. [Google Scholar] [CrossRef]
- Fix, C.; Elixmann, S.-M.; Senk, D.G. Design of As-Cast Structures of Continuously Cast Steel Grades: Modeling and Prediction. Steel Res. Int. 2020, 91, 2000235. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, L.; Zhang, H. Simulation of the Macrosegregation in the Gear Steel Billet Continuous Casting Process. Chin. J. Eng. 2021, 43, 561–568. [Google Scholar] [CrossRef]
- Savage, J.; Pritchard, W. The Problem of Rupture of the Billet in the Continuous Casting of Steel. J. Iron Steel Inst. 1954, 178, 269–277. [Google Scholar]
- Zhang, P.; Wang, M.; Shi, P.; Xu, L. Effects of Alloying Elements on Solidification Structures and Macrosegregation in Slabs. Metals 2022, 12, 1826. [Google Scholar] [CrossRef]
- Rappaz, M. Modelling of Microstructure Formation in Solidification Processes. Int. Mater. Rev. 1989, 34, 93–124. [Google Scholar] [CrossRef]
- Gandin, C.-A.; Desbiolles, J.-L.; Rappaz, M.; Thevoz, P. A Three-Dimensional Cellular Automation-Finite Element Model for the Prediction of Solidification Grain Structures. Metall. Mater. Trans. A 1999, 30, 3153–3165. [Google Scholar] [CrossRef]
- Tan, Y.; Wang, H. Modeling Constrained Dendrite Growth in Rapidly Directional Solidification. J. Mater. Sci. 2012, 47, 5308–5316. [Google Scholar] [CrossRef]
- Kurz, W.; Giovanola, B.; Trivedi, R. Theory of Microstructural Development during Rapid Solidification. Acta Metall. 1986, 34, 823–830. [Google Scholar] [CrossRef]
- Li, W. Self-Diffusion Coefficient of Components in Iron-Based Liquid Metal. In Metallurgy and Physical Chemistry of Materials; Metallurgical Industry Press: Beijing, China, 2001; pp. 531–533. [Google Scholar]
- Fang, Q.; Ni, H.; Zhang, H.; Wang, B.; Lv, Z. The Effects of a Submerged Entry Nozzle on Flow and Initial Solidification in a Continuous Casting Bloom Mold with Electromagnetic Stirring. Metals 2017, 7, 146. [Google Scholar] [CrossRef]
- Yu, K.; Wang, M.; Fan, H.; Zhan, Z.; Ren, Z.; Xu, L. Investigation on the Solidification Structure of Q355 in 475 Mm Extra-Thick Slabs Adopting Cellular Automaton-Finite Element Model. Metals 2024, 14, 1012. [Google Scholar] [CrossRef]
- Kurz, W.; Bezençon, C.; Gäumann, M. Columnar to Equiaxed Transition in Solidification Processing. Sci. Technol. Adv. Mater. 2001, 2, 185–191. [Google Scholar] [CrossRef]
- Lin, H.; Yue, F.; Wu, H.; Chen, Z. Simulation of the effect of alloying elements on the solidification structure of 20CrMnTi steel. Iron Steel 2016, 92, 36–42. [Google Scholar] [CrossRef]
- Bai, L.; Liu, H.; Zhang, Y.; Miao, X.; Ruan, X. Numerical simulation of the effect of microstructure and alloying elements on 22CrMoH continuous casting billet. J. Univ. Sci. Technol. Beijing 2011, 33, 1091–1098. [Google Scholar] [CrossRef]
- Zhang, Y.L.; Yang, X.G.; Xu, J.G.; Bai, L.G. Effects of Alloying Elements on the Microstructure of 22CrMoH Gear Steels Billets. Adv. Mat. Res. 2011, 239, 3–14. [Google Scholar] [CrossRef]
- YB/T 4003-2016; Standard Diagram for Macrostructure and Defect in Continuous Casting Slab. Metallurgical Industry Press: Beijing, China, 2016.
- Ding, H.; Zhang, G.; Yang, C.; Li, J.; Zhang, Y. Experimental study on the influence of electromagnetic stirring frequency on slab quality in roller secondary cooling zone. Contin. Cast. 2015, 1, 17–21. [Google Scholar] [CrossRef]
- Asai, S. Birth and Recent Activities of Electromagnetic Processing of Materials. ISIJ Int. 1989, 29, 981–992. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, X. Effect of electromagnetic stirring in secondary cooling zone on central segregation of continuous casting slab. J. Univ. Sci. Technol. Beijing 2007, 590, 582–585. [Google Scholar] [CrossRef]
- Zhao, H.; Li, Z. Formation mechanism of white band on continuous casting billet. J. Iron. Steel Res. Int. 2000, 1, 75–76. [Google Scholar] [CrossRef]
- Sasaki, K.; Sugitani, Y.; Kobayashi, S.; Ishimura, S. The effect of fluid flow on the formation of the negative segregation zone in steel ingots. Tetsu-to-Hagane 1979, 65, 60–69. [Google Scholar] [CrossRef]
- Takahashi, T.; Ohsasa, K.; Katayama, N. Simulation for Progress of Solid-Liquid Coexisting Zone in Continuous Casting of Carbon Steels. Tetsu-to-Hagane 1990, 76, 728–734. [Google Scholar] [CrossRef][Green Version]
- Xia, S.; Wang, P.; Tang, Q.; Li, W.; Hu, K.; Zhang, J. Solidification end characteristics and central quality control of bearing steel bloom. Iron Steel 2023, 59, 1–15. [Google Scholar] [CrossRef]
- Xu, Z.-G.; Wang, X.-H.; Jiang, M.; Li, L.-P. Investigation on Formation of Equiaxed Zone in Low Carbon Steel Slabs. Metall. Res. Technol. 2016, 113, 106. [Google Scholar] [CrossRef]
- Wang, C.; Liu, Z.; Li, B.; Dou, Z.; Rong, W. Effect of Alternate Electromagnetic Stirring on Macrostructure Evolution in Thin-Slab Continuous Casting. Metall. Mater. Trans. B 2024, 55, 2124–2137. [Google Scholar] [CrossRef]





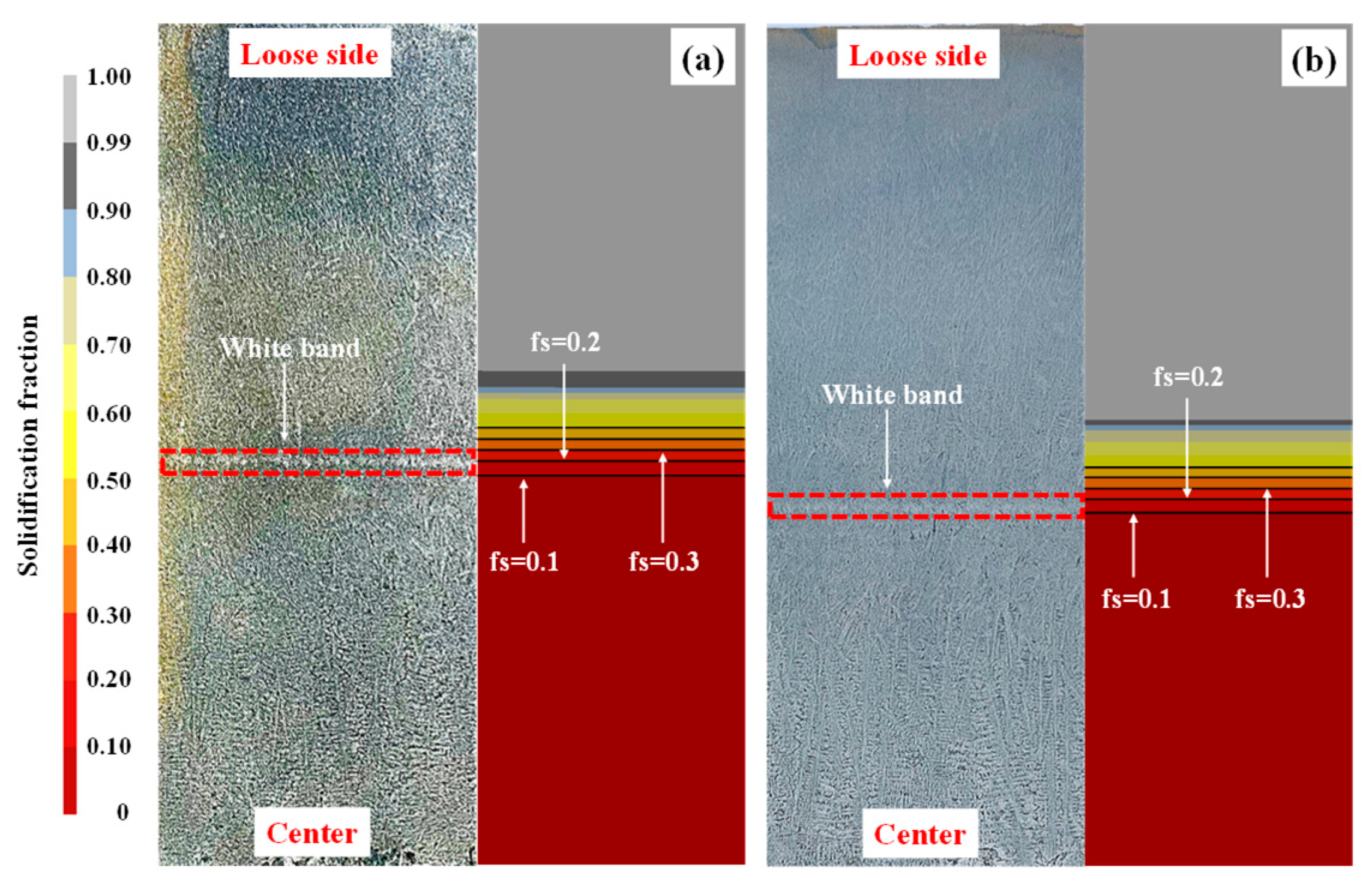
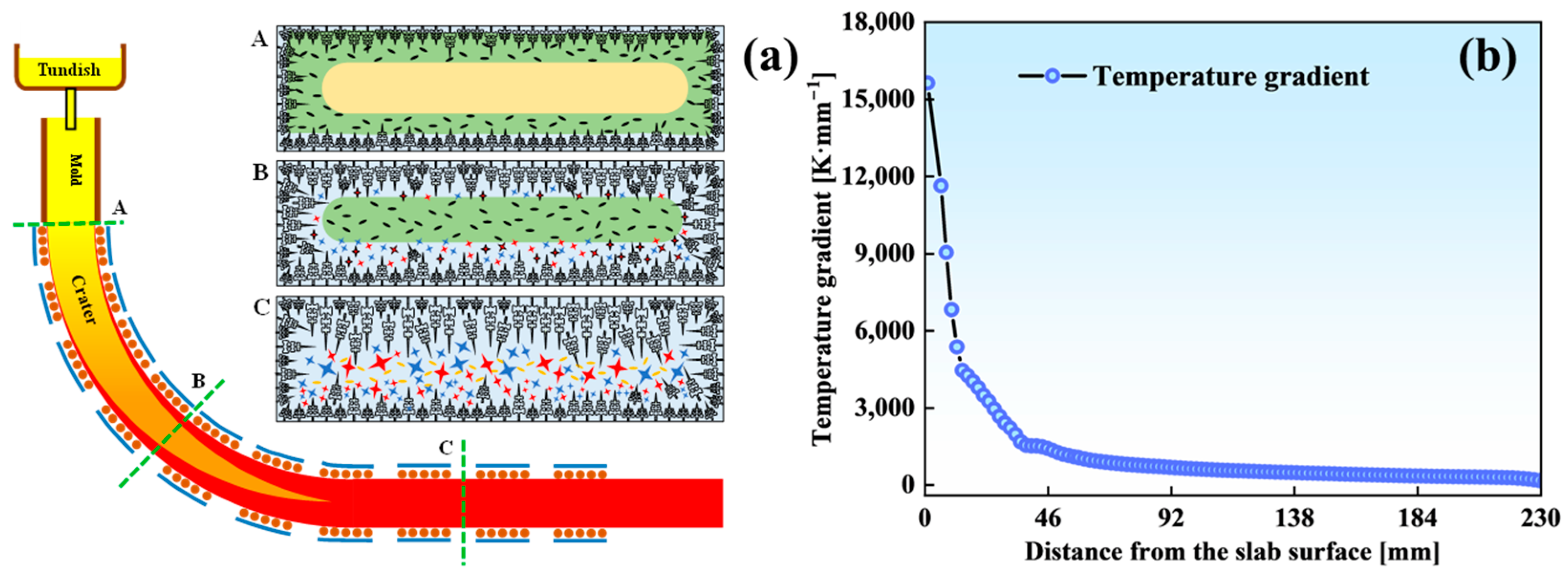
| Cooling Zone | Length [m] | Water Flow Rate [L·min−1] | Computational Formula | |
|---|---|---|---|---|
| Secondary Cooling Zone | Foot roll section (N) | 1.82 | 78.20 | [31] |
| Foot roll section (W) | 1.09 | 126.00 | ||
| II | 2.02 | 76.40 | [5] | |
| III | 3.52 | 75.10 | ||
| IV | 5.08 | 74.70 | ||
| V | 7.13 | 34.80 | ||
| VI | 9.19 | 27.70 | ||
| VII | 13.53 | 29.30 | ||
| VIII | 18.08 | 22.50 | ||
| IX | 20.37 | 9.20 | ||
| X | 25.51 | 18.60 | ||
| Air Cooling Zone | / | |||
| [31] | ||||
| Nucleation Parameter | ΔTS,max [K] | ΔTS,σ [K] | nS | ΔTV,max [K] | ΔTV,σ [K] | nV |
|---|---|---|---|---|---|---|
| Value | 1 | 0.1 | 1 × 108 | 4.3 | 1.1 | 3 × 1010 |
| Composition | c0 [wt.%] | M [K·(wt.%)−1] | K0 | D [m2·s−1] | Γ [m·K] |
|---|---|---|---|---|---|
| C | 0.163 | −80.61 | 0.16 | 1.10 × 10−8 | 3 × 10−7 |
| Si | 0.0286 | −16.64 | 0.55 | 8.50 × 10−9 | |
| Mn | 0.525 | −5.20 | 0.71 | 2.40 × 10−9 | |
| P | 0.021 | −28.65 | 0.26 | 4.60 × 10−9 | |
| S | 0.0042 | −38.23 | 0.03 | 3.50 × 10−9 | |
| Cr | 0.021 | −1.63 | 0.91 | 3.30 × 10−9 | |
| Al | 0.044 | 4.74 | 1.17 | 2.47 × 10−8 | |
| Ti | 0.016 | −13.86 | 0.28 | 4.4 × 10−9 |
| Element | Content [wt.%] | TL [K] | Ts [K] | α3 [m·s−1·K−3] | Content [wt.%] | TL [K] | Ts [K] | α3 [m·s−1·K−3] |
|---|---|---|---|---|---|---|---|---|
| C | 0.13 | 1514 | 1474 | 1.221 × 10−5 | 0.17 | 1510 | 1467 | 8.396 × 10−6 |
| 0.14 | 1513 | 1473 | 1.105 × 10−5 | 0.18 | 1509 | 1465 | 7.703 × 10−6 | |
| 0.15 | 1511 | 1471 | 1.004 × 10−5 | 0.19 | 1509 | 1464 | 7.129 × 10−6 | |
| 0.16 | 1511 | 1469 | 9.161 × 10−6 | 0.20 | 1508 | 1461 | 6.599 × 10−6 | |
| Mn | 1.25 | 1512 | 1470 | 9.735 × 10−6 | 1.45 | 1511 | 1469 | 9.135 × 10−6 |
| 1.30 | 1512 | 1469 | 9.580 × 10−6 | 1.50 | 1511 | 1469 | 8.992 × 10−6 | |
| 1.35 | 1512 | 1470 | 9.427 × 10−6 | 1.55 | 1511 | 1468 | 8.852 × 10−6 | |
| 1.40 | 1512 | 1469 | 9.280 × 10−6 | 1.60 | 1510 | 1468 | 8.715 × 10−6 | |
| Si | 0.20 | 1512 | 1471 | 9.954 × 10−6 | 0.40 | 1509 | 1465 | 7.791 × 10−6 |
| 0.25 | 1511 | 1469 | 9.332 × 10−6 | 0.45 | 1508 | 1463 | 7.365 × 10−6 | |
| 0.30 | 1511 | 1468 | 8.770 × 10−6 | 0.50 | 1507 | 1462 | 6.974 × 10−6 | |
| 0.35 | 1511 | 1466 | 8.257× 10−6 | 0.55 | 1506 | 1460 | 6.615× 10−6 |
| Number | Value | Standard Error | 99% Confidence Interval | R2 (COD) |
|---|---|---|---|---|
| a | 0.96819 | 0.03102 | (0.90544, 1.03095) | 0.98670 |
| b | 0.98189 | 0.04838 | (0.88403, 1.07975) | |
| c | 1.67767 | 0.13735 | (1.39985, 1.95549) | |
| d | 0.97364 | 0.05069 | (0.87111, 1.07617) | |
| e | 0.98738 | 0.05275 | (0.88068, 1.09408) | |
| f | 0.96392 | 0.04055 | (0.88189, 1.04595) | |
| g | −678.9288 | 18.41823 | (−716.1832, −641.67441) |
| Schemes | Casting Speed [m·min−1] | S-EMS Current [A] | S-EMS Frequency [Hz] |
|---|---|---|---|
| Scheme 1 | 0.45 | 0 | 0 |
| Scheme 2 | 0.45 | 570 | 5 |
| Scheme 3 | 0.42 | 570 | 5 |
| Scheme 4 | 0.42 | 570 | 3.5 |
| Scheme 5 | 0.42 | 420 | 3.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, K.; Xu, L.; Zhang, Y.; Zhang, H.; Zhan, Z. Endogenous–Exogenous Analyses of the Solidification Structure in 475 mm Extra-Thick Slabs: Columnar-to-Equiaxed Positioning and Effect of Strand Electromagnetic Stirring. Materials 2025, 18, 2179. https://doi.org/10.3390/ma18102179
Yu K, Xu L, Zhang Y, Zhang H, Zhan Z. Endogenous–Exogenous Analyses of the Solidification Structure in 475 mm Extra-Thick Slabs: Columnar-to-Equiaxed Positioning and Effect of Strand Electromagnetic Stirring. Materials. 2025; 18(10):2179. https://doi.org/10.3390/ma18102179
Chicago/Turabian StyleYu, Kezai, Lijun Xu, Yanling Zhang, Haibo Zhang, and Zhonghua Zhan. 2025. "Endogenous–Exogenous Analyses of the Solidification Structure in 475 mm Extra-Thick Slabs: Columnar-to-Equiaxed Positioning and Effect of Strand Electromagnetic Stirring" Materials 18, no. 10: 2179. https://doi.org/10.3390/ma18102179
APA StyleYu, K., Xu, L., Zhang, Y., Zhang, H., & Zhan, Z. (2025). Endogenous–Exogenous Analyses of the Solidification Structure in 475 mm Extra-Thick Slabs: Columnar-to-Equiaxed Positioning and Effect of Strand Electromagnetic Stirring. Materials, 18(10), 2179. https://doi.org/10.3390/ma18102179







