The Potential of Polymers and Glass to Enhance Hydrogen Storage Capacity: A Mathematical Approach
Abstract
1. Introduction
2. Materials and Methods
2.1. Hydrogen Storage Technology Description
- Type I: These tanks are composed entirely of metal, typically steel or aluminum. They are durable but heavy, limiting their gravimetric efficiency.
- Type II: These tanks have a metal liner with partial composite reinforcement, providing a balance between weight and cost but with limited improvement in storage capacity.
- Type III: A metal liner (often aluminum) fully wrapped with a composite material, these tanks offer improved weight reduction and strength, though they are more expensive.
- Type IV: Tanks that use a plastic (polymer) liner with full composite wrapping, making them the lightest option and superior in gravimetric efficiency. However, they are the costliest and require careful design to ensure the control of hydrogen permeability.
2.2. Material Selection
2.2.1. Selection of Potential Types of Polymers
2.2.2. Selection of Potential Types of Glass
2.3. Storage Capacity Calculation
- Material Properties: The calculations will utilize the previously identified parameters for each selected material, such as density, ultimate tensile strength, and admissible stress, based on Table 2. It is assumed that these values accurately reflect the performance characteristics of the materials under the specified conditions. The values from Table 2 related to tensile strength consider the material in an ideal state without any defects, so our calculated values could be more optimistic compared to results obtained from practical experiments where material defects cannot be avoided but limited by a proper production process.
- Safety Factors: When considering operational safety, a safety factor of 2 will be applied to account for potential variations in material performance and external stressors that may affect the integrity of the storage system.
- Environmental Conditions: The calculations will assume standard environmental conditions for temperature and pressure, allowing for a consistent basis for comparison across different materials and configurations.
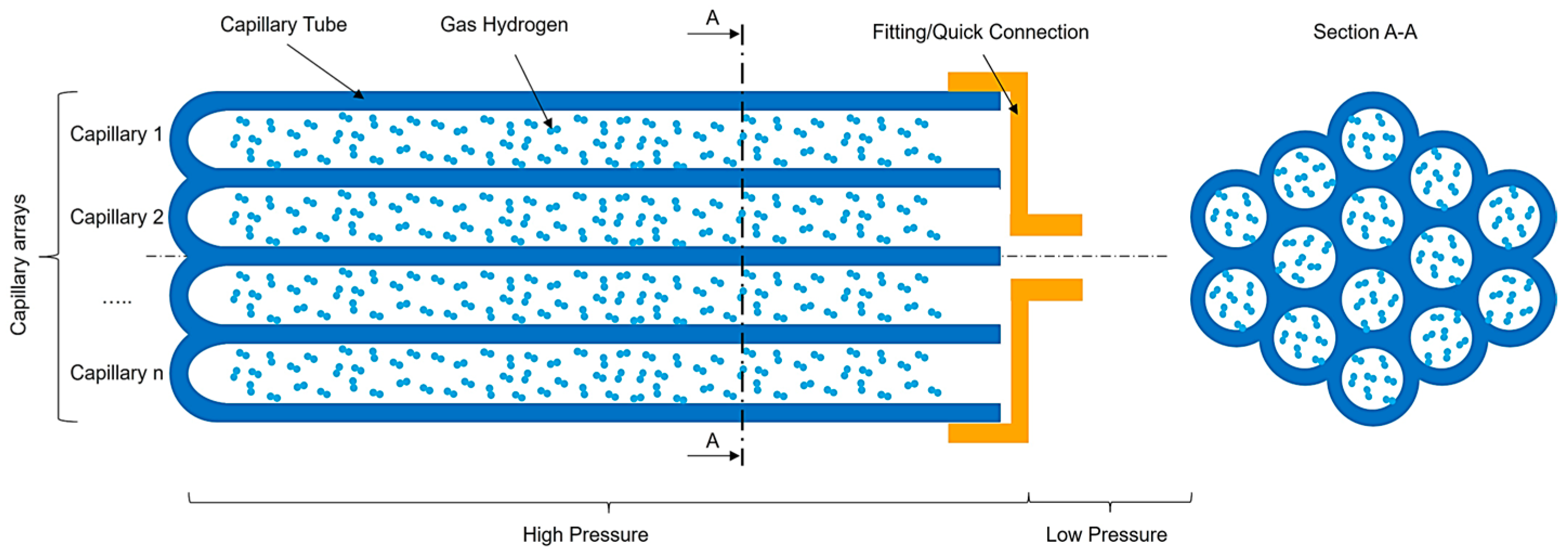
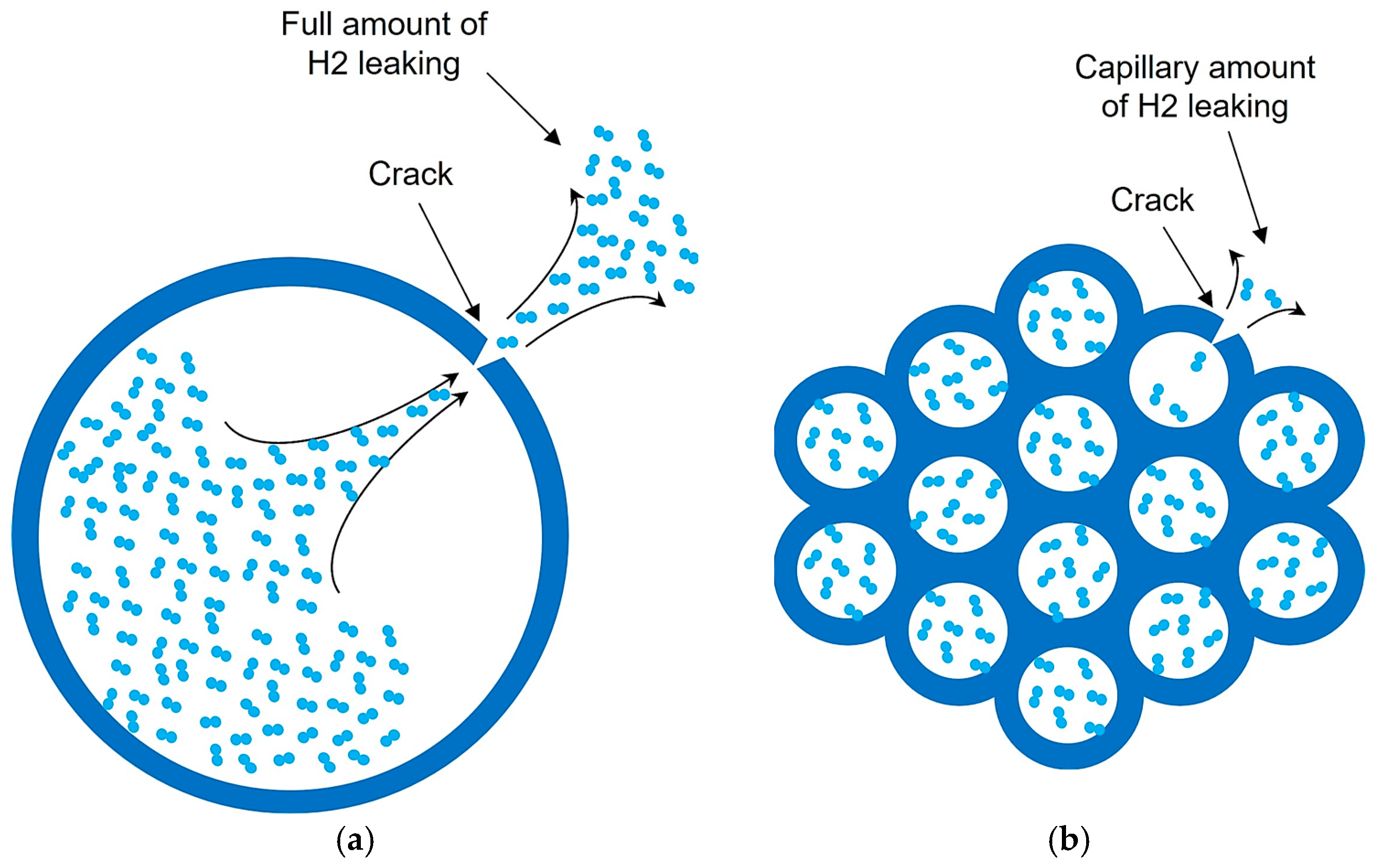
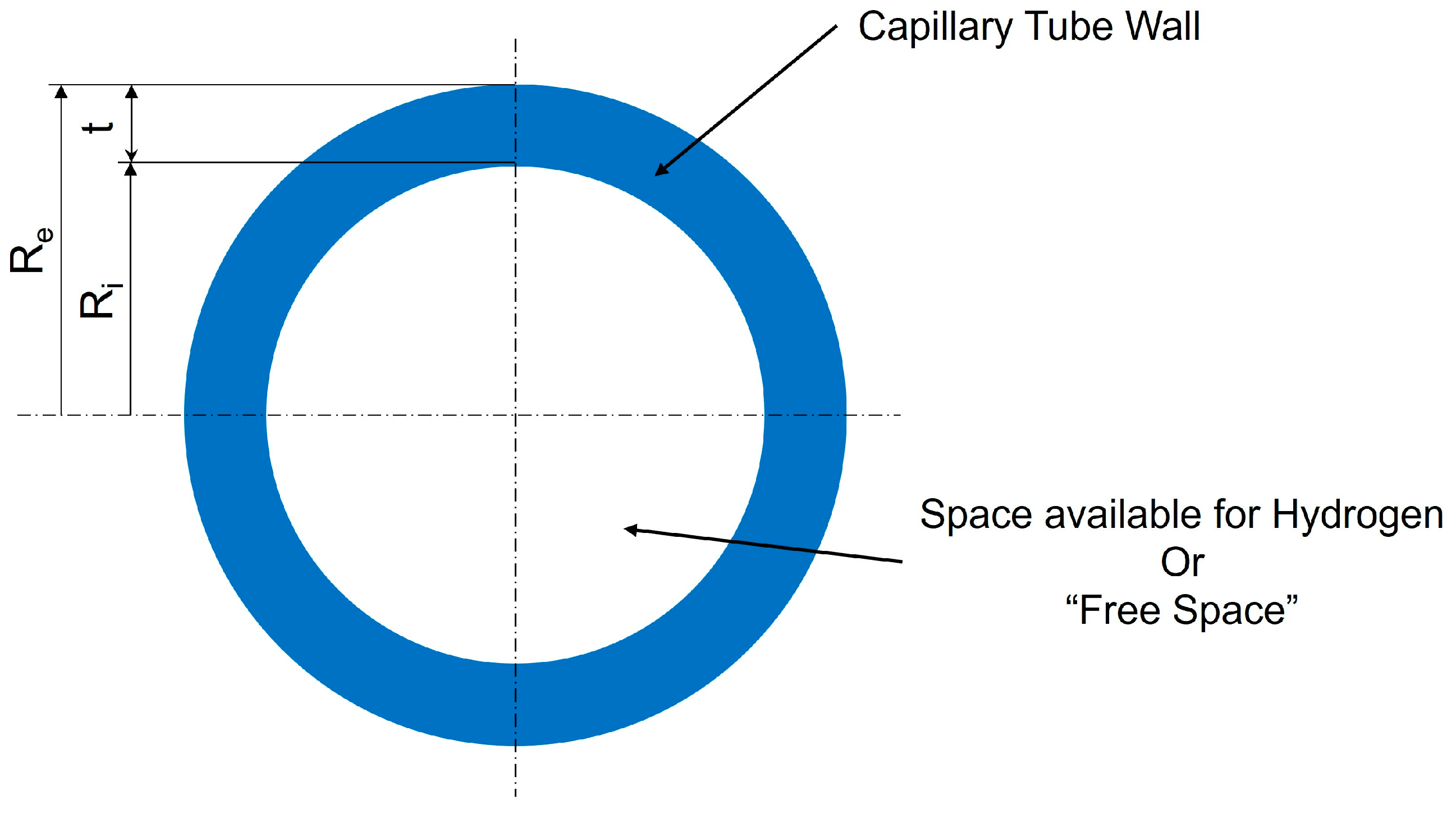
- Geometric Configurations: To simplify the calculations, we will analyze the potential of the chosen materials through a storage system composed of a single capillary tube with a circular section cut, see Figure 4.
2.3.1. Thin-Walled Pressure Vessels
- σh is the hoop stress,
- pi is the internal pressure,
- Ri is the internal radius of the vessel,
- t is the wall thickness.
- σl is the longitudinal stress.
2.3.2. Thick-Walled Pressure Vessels
2.3.3. Gravimetric Capacity Calculation
- mH2 is the mass of hydrogen stored,
- mstorage is the mass of the storage material.
- P is the pressure of hydrogen,
- V is the volume of hydrogen,
- n is the number of moles of hydrogen,
- R is the ideal gas constant (8.314 J/(mol·K)),
- T is the temperature in Kelvin (K).
- is the density of the material used for the storage,
- is the length of the capillary tube.
2.3.4. Volumetric Capacity Calculation
- Vstorage = Vcapillary = πRe2L for a single capillary tube.
3. Results
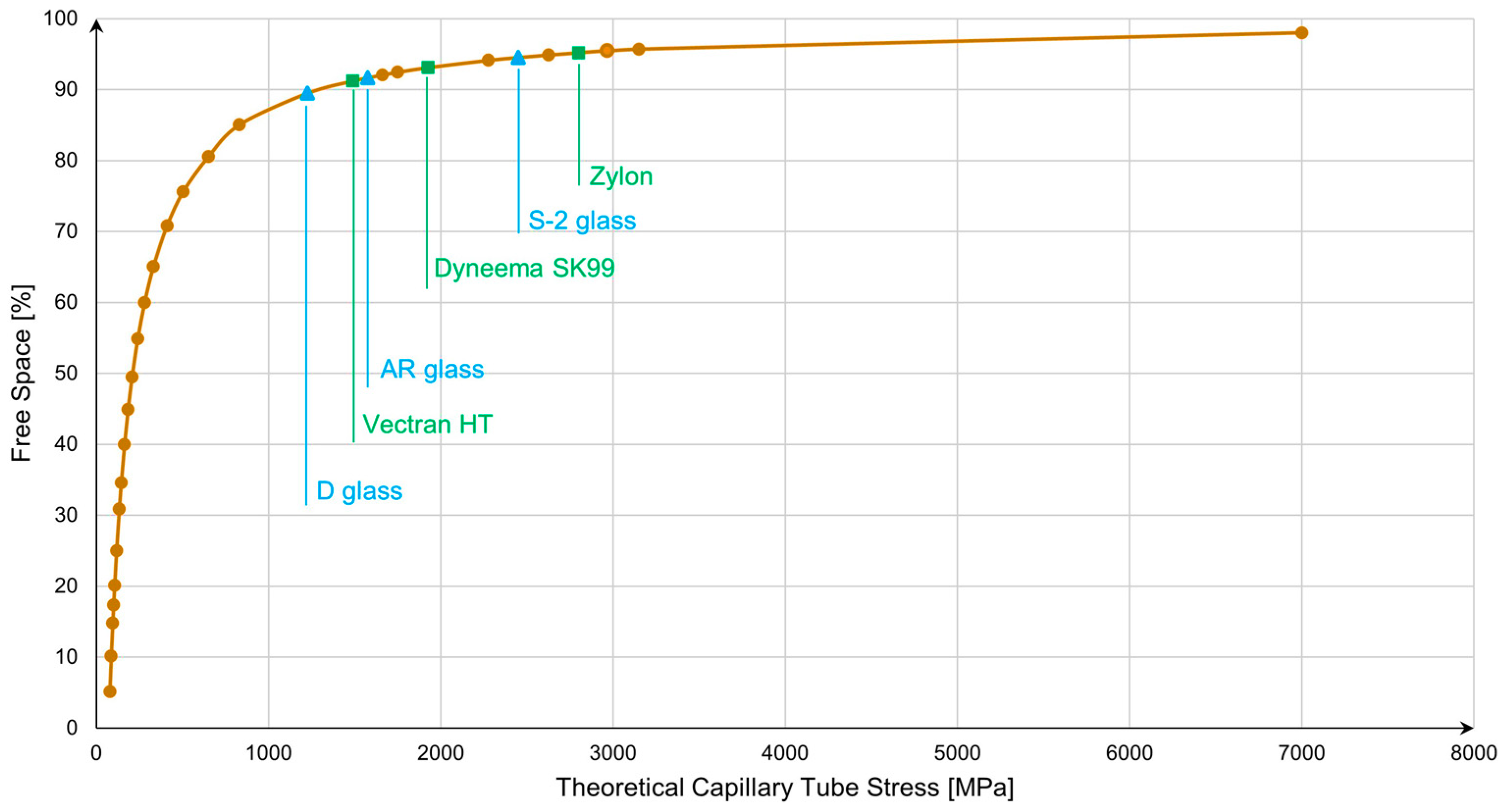
3.1. Impact of Free Space Increase on Capillary Tube Wall Stress
3.2. Gravimetric Capacity Analysis
3.3. Volumetric Capacity Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Abe, J.O.; Popoola, A.P.I.; Ajenifuja, E.; Popoola, O.M. Hydrogen energy, economy and storage: Review and recommendation. Int. J. Hydrog. Energy 2019, 44, 15072–15086. [Google Scholar] [CrossRef]
- Somerday, B.P.; Marchi, S.C. Solid-State Hydrogen Storage; Woodhead Publishing: Sawston, UK, 2008; pp. 51–81. [Google Scholar] [CrossRef]
- DOE Technical Targets for Onboard Hydrogen Storage for Light-Duty Vehicles. Available online: https://www.energy.gov/eere/fuelcells/doe-technical-targets-onboard-hydrogen-storage-light-duty-vehicles (accessed on 12 October 2024).
- Usman, R.M. Hydrogen storage methods: Review and current status. Renew. Sustain. Energy Rev. 2022, 167, 112743. [Google Scholar] [CrossRef]
- Alzohbi, G.; Almoaikel, A.; Alshuhail, L. An overview on the technologies used to store hydrogen. Energy Rep. 2023, 9, 28–34. [Google Scholar] [CrossRef]
- Ekpotu, W.F.; Akintola, J.; Obialor, M.C.; Philemon, U. Historical Review of Hydrogen Energy Storage Technology. World J. Eng. Technol. 2023, 11, 454–475. [Google Scholar] [CrossRef]
- Holtappels, K.; Beckmann-Kluge, M.; Gebauer, M.; Grueneberg, M.; Eliezer, D. Hydrogen Storage in Glass Capillary Arrays for Portable and Mobile Systems. In Proceedings of the 3rd International Conference on Hydrogen Safety, Corsica, France, 16–18 September 2009. [Google Scholar]
- Zuettel, A.; Borgschulte, A.; Schlapbach, L. Hydrogen as a Future Energy Carrier; Wiley-VCH Verlag GmbH & Co. KGa: Berlin, Germany, 2008. [Google Scholar] [CrossRef]
- Zuettel, A. Materials for hydrogen storage. Mater. Today 2004, 6, 24–33. [Google Scholar] [CrossRef]
- Li, Y.; Barzagli, F.; Liu, P.; Xiaoan, Z.; Zhang, X.; Yang, Z.; Xiao, M.; Huang, Y.; Luo, X.; Li, C.; et al. Mechanism and Evaluation of Hydrogen Permeation Barriers: A Critical Review. Ind. Eng. Chem. Res. 2023, 62, 15752–15773. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, J. Advanced lightweight materials for Automobiles: A review. Mater. Des. 2022, 221, 110994. [Google Scholar] [CrossRef]
- Pedicini, R.; Sacca, A.; Carbone, A.; Passalacqua, E. Hydrogen storage based on polymeric material. Int. J. Hydrog. Energy 2011, 36, 906–9068. [Google Scholar] [CrossRef]
- Hearle, J.W.S. High-Performance Fibres; Woodhead Publishing: Sawston, UK, 2001. [Google Scholar]
- Holtappels, K.; Beckmann-Kluge, M.; Gebauer, M.; Eliezer, D. Pressure Resistance of Glass Capillaries for Hydrogen Storage. Mater. Test. 2011, 53, 14–18. [Google Scholar] [CrossRef]
- Li, X.; Huang, Q.; Liu, Y.; Zhao, B.; Li, J. Review of the Hydrogen Permeation Test of the Polymer Liner Material of Type IV On-Board Hydrogen Storage Cylinders. Materials 2023, 16, 5366. [Google Scholar] [CrossRef]
- Prewitz, M.; Gaber, M.; Mueller, R.; Marotztke, C.; Holtappels, K. Polymer coated glass capillaries and structures for high-pressure hydrogen storage: Permeability and hydrogen tightness. Int. J. Hydrog. Energy 2018, 43, 5637–5644. [Google Scholar] [CrossRef]
- Cheng, Q.; Zhang, R.; Shi, Z.; Lin, J. Review of common hydrogen storage tanks and current manufacturing methods for aluminium alloy tank liners. Int. J. Lightweight Mater. Manuf. 2024, 7, 269–284. [Google Scholar] [CrossRef]
- Gómez, J.A.; Santos, D.M.F. The Status of On-Board Hydrogen Storage in Fuel Cell Electric Vehicles. Designs 2023, 7, 97. [Google Scholar] [CrossRef]
- C.En. Available online: https://www.cenh2.com/ (accessed on 13 October 2024).
- Ratoi, A.; Munteanu, C.; Eliezer, D. Maximizing Onboard Hydrogen Storage Capacity by Exploring High-Strength Novel Materials Using a Mathematical Approach. Materials 2024, 17, 4288. [Google Scholar] [CrossRef] [PubMed]
- Beckman, I.P.; Lozano, C.; Freeman, E.; Riveros, G. Fiber Selection for Reinforced Additive Manufacturing. Polymers 2021, 13, 2231. [Google Scholar] [CrossRef]
- Omnexu. The Material Selection Platform. Available online: https://omnexus.specialchem.com/polymer-property/strength-at-break-tensile (accessed on 11 October 2024).
- Laminated Plastics. Material Datasheets. Available online: https://laminatedplastics.com/rod.php?myDestination=http%3A%2F%2Fwww.laminatedplastics.com%2Fabs.pdf (accessed on 10 October 2024).
- ThePlasticShop. Plastic Technical Data Sheets. Available online: https://www.theplasticshop.co.uk/ (accessed on 12 October 2024).
- Plastim. Available online: https://plastim.co.uk/stock-shapes/ (accessed on 14 October 2024).
- Ibrahim, A.; Ryu, Y.; Saidpour, M. Stress Analysis of Thin-Walled Pressure Vessels. Mod. Mech. Eng. 2015, 5, 1–9. [Google Scholar] [CrossRef]
- Tripa, P. Rezistenta Materialelor; Mirton: Timisoara, Romania, 2001. (In Romanian) [Google Scholar]
- Cryo-Compressed Hydrogen Storage. Available online: https://web.archive.org/web/20240521085440/https://www.yumpu.com/en/document/read/26741293/cryo-compressed-hydrogen-storage (accessed on 21 May 2024).
- Hua, T.; Ahluwalia, R.; Peng, J.-K.; Kromer, M.; Lasher, S.; McKenney, K.; Law, K.; Sinha, J. Technical Assessment of Compressed Hydrogen Storage Tank Systems for Automotive Applications. Int. J. Hydrog. Energy 2011, 36, 3037–3049. [Google Scholar] [CrossRef]
- Ahluwalia, R.K.; Hua, T.Q.; Peng, J.-K.; Lasher, S.; McKenney, K.; Sinha, J.; Gardiner, M. Technical Assessment of Cryo-Compressed Hydrogen Storage Tank System for Automotive Applications. Int. J. Hydrog. Energy 2010, 35, 4171–4184. [Google Scholar] [CrossRef]
- Satyapal, S.; Read, C.; Ordaz, G.; Stetson, N.; Thomas, G.; Petrovic, J. The U.S. Department of Energy’s National Hydrogen Storage Project: Goal, Progress, and Future Plans. In Proceedings of the Fourth U.S.-Korea Forum on Nanotechnology: Sustainable Energy, Honolulu, HI, USA, 26–27 April 2007. [Google Scholar]
- Ahmed, A.; Seth, S.; Purewal, J.; Wong-Foy, A.G.; Veenstra, M.; Matzger, A.J.; Siegel, D.J. Exceptional hydrogen storage achieved by screening nearly half a million metal-organic frameworks. Nat. Commun. 2019, 10, 1568. [Google Scholar] [CrossRef]
- Krishna, R.; Titus, E.; Salimian, M.; Okhay, O.; Rajendran, S.; Rajkumar, A.; Sousa, J.M.G.; Ferreira, A.L.C.; Gil, J.C.; Gracio, J. Hydrogen Storage for Energy Application; Intech Open: London, UK, 2012. [Google Scholar]
- Meyer, R. A New Technology for Hydrogen Safety: Glass Structures as Storage System. In Proceedings of the 4th International Conference on Hydrogen Safety (ICHS), San Francisco, CA, USA, 12–14 September 2011. [Google Scholar]
- Moradi, R.; Growth, K.M. Hydrogen storage and delivery: Review of the state-of-the-art technologies and risk and reliability analysis. Int. J. Hydrog. Energy 2019, 44, 12254–12269. [Google Scholar] [CrossRef]
- Yin, L.; Yang, H.; Ju, Y. Review on the key technologies and future development of insulation structure for liquid hydrogen storage tanks. Int. J. Hydrog. Energy 2024, 57, 1302–1315. [Google Scholar] [CrossRef]
- Zhang, X.; Liu, P.; Zhang, Y. The application of MOFs for hydrogen storage. Inorganica Chim. Acta 2023, 557, 121683. [Google Scholar] [CrossRef]
- Flekiewicz, M.; Kubica, G. Hydrogen Onboard Storage Technologies for Vehicles. In Diesel Engines—Current Challenges and Future Perspectives; Intech Open: London, UK, 2023. [Google Scholar]
- Keith, A.; Zlotea, C.; Szilágyi, P.Á. Perspective of interstitial hydrides of high-entropy alloys for vehicular hydrogen storage. Int. J. Hydrog. Energy 2024, 52, 531–546. [Google Scholar] [CrossRef]
- Ley, M.B.; Jepsen, L.H.; Lee, Y.S.; Cho, Y.W.; Von Colbe, J.M.B.; Dornheim, M.; Rokni, M.; Jensen, J.O.; Sloth, M.; Filinchuk, Y.; et al. Complex hydrides for hydrogen storage—New perspectives. Mater. Today 2014, 17, 122–128. [Google Scholar] [CrossRef]
- Akbayrak, S.; Özkar, S. Ammonia borane as hydrogen storage materials. Int. J. Hydrog. Energy 2018, 43, 18592–18606. [Google Scholar] [CrossRef]


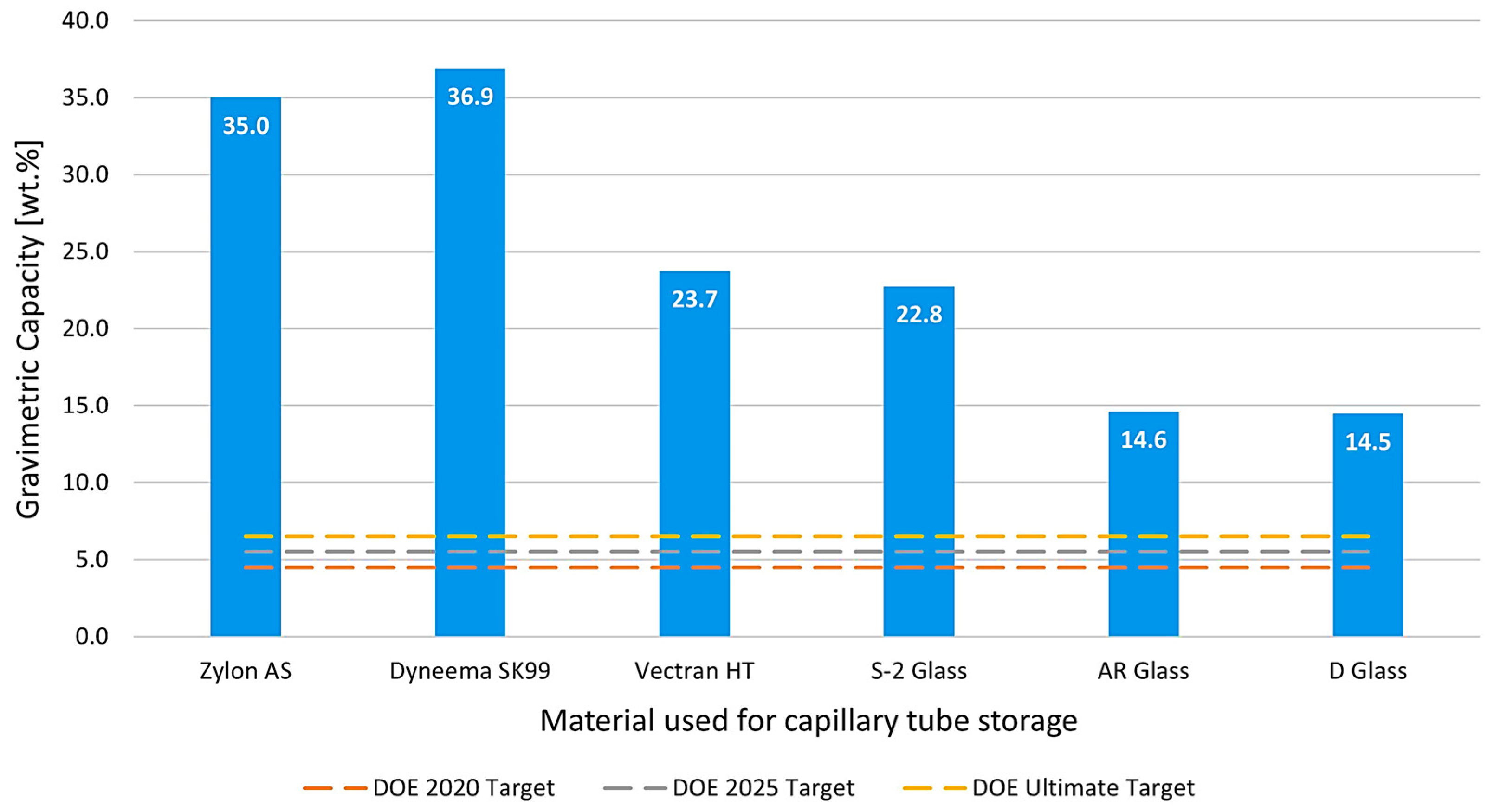
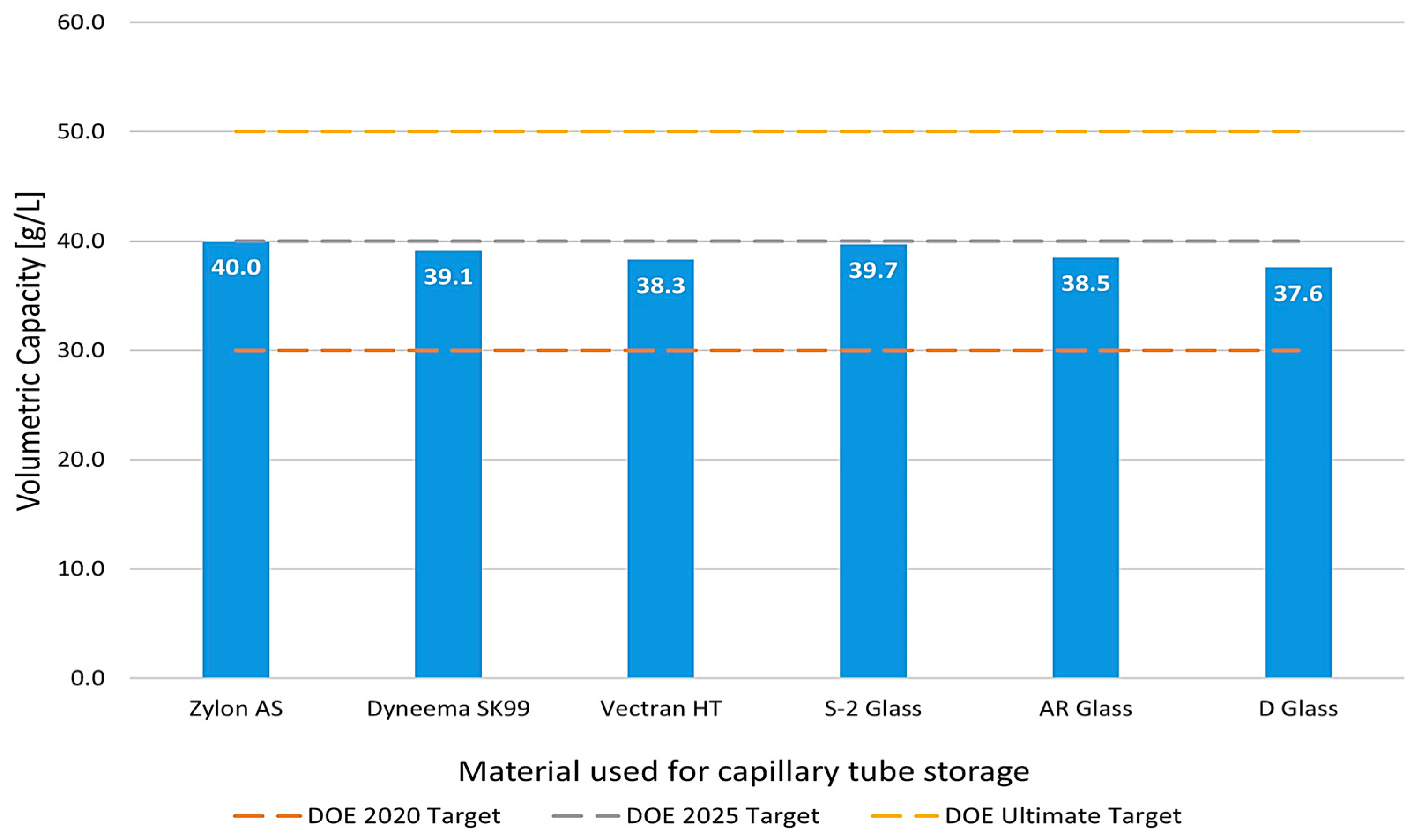
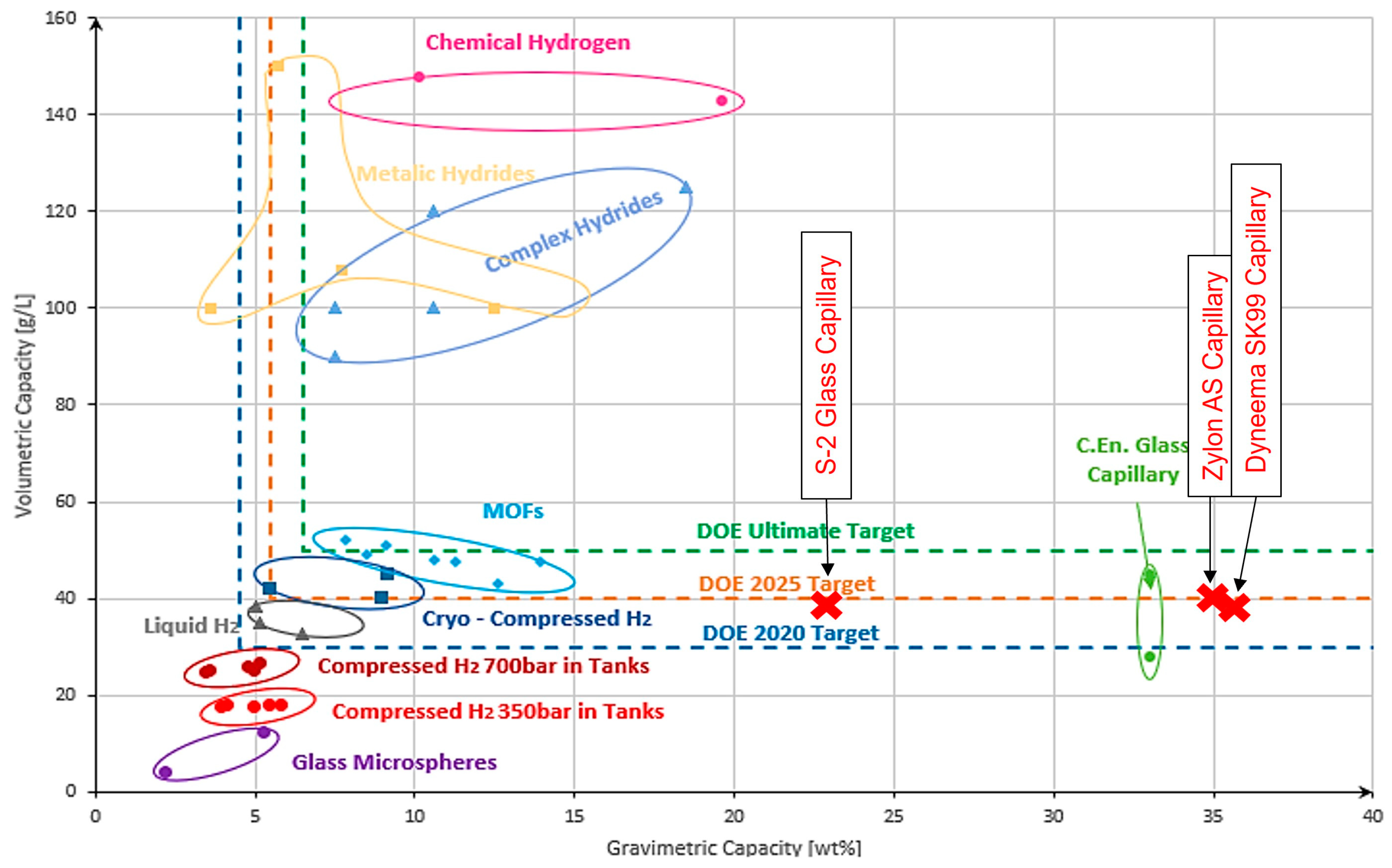
| Type | Material [17] | Gravimetric Capacity [wt.%] [18] | DOE 2020 Target [3] | DOE 2025 Target [3] | DOE Ultimate Target [3] |
|---|---|---|---|---|---|
| I | Steel/Al | 1.7 | |||
| II | Steel/Al + partial composite reinforcement | 2.1 | |||
| III | Steel/Al + full composite reinforcement | 4.2 | 4.5 | 5.5 | 6.5 |
| IV | Polymer + full composite reinforcement | 5.7 |
| Material | Density [g/cm3] | UTS [MPa] | σ Admissible [MPa] * |
|---|---|---|---|
| Polymers | |||
| Zylon AS (PBO fiber) | 1.54 | 5800 | 2900 |
| DSM Dyneema SK99 (UHMWPE fiber) | 0.97 | 4100 | 2050 |
| Vectran HT (LCP fiber) | 1.40 | 3000 | 1500 |
| Glass | |||
| S-2 Glass fiber (aluminosilicate) | 2.46 | 4890 | 2445 |
| AR-Glass fiber (soda-lime) | 2.70 | 3240 | 1620 |
| D-Glass fiber (borosilicate) | 2.11 | 2500 | 1250 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ratoi, A.; Munteanu, C.; Eliezer, D. The Potential of Polymers and Glass to Enhance Hydrogen Storage Capacity: A Mathematical Approach. Materials 2024, 17, 6065. https://doi.org/10.3390/ma17246065
Ratoi A, Munteanu C, Eliezer D. The Potential of Polymers and Glass to Enhance Hydrogen Storage Capacity: A Mathematical Approach. Materials. 2024; 17(24):6065. https://doi.org/10.3390/ma17246065
Chicago/Turabian StyleRatoi, Andrei, Corneliu Munteanu, and Dan Eliezer. 2024. "The Potential of Polymers and Glass to Enhance Hydrogen Storage Capacity: A Mathematical Approach" Materials 17, no. 24: 6065. https://doi.org/10.3390/ma17246065
APA StyleRatoi, A., Munteanu, C., & Eliezer, D. (2024). The Potential of Polymers and Glass to Enhance Hydrogen Storage Capacity: A Mathematical Approach. Materials, 17(24), 6065. https://doi.org/10.3390/ma17246065








