Thermodynamically-Consistent Modeling of Ferromagnetic Hysteresis
Abstract
1. Introduction
Notation
2. Balance Equations
3. Euclidean Invariance and Power Representation
4. Consistency with the Balance of Angular Momentum
5. Thermodynamic Restrictions
Cyclic Processes
6. Hyper-Magnetoelastic Materials
6.1. Linear Magnetoelastic Materials
6.2. Nonlinear Magnetoelastic Materials
7. Hypo-Magnetoelastic Materials
A Simple Hypo-Magnetoelastic Model
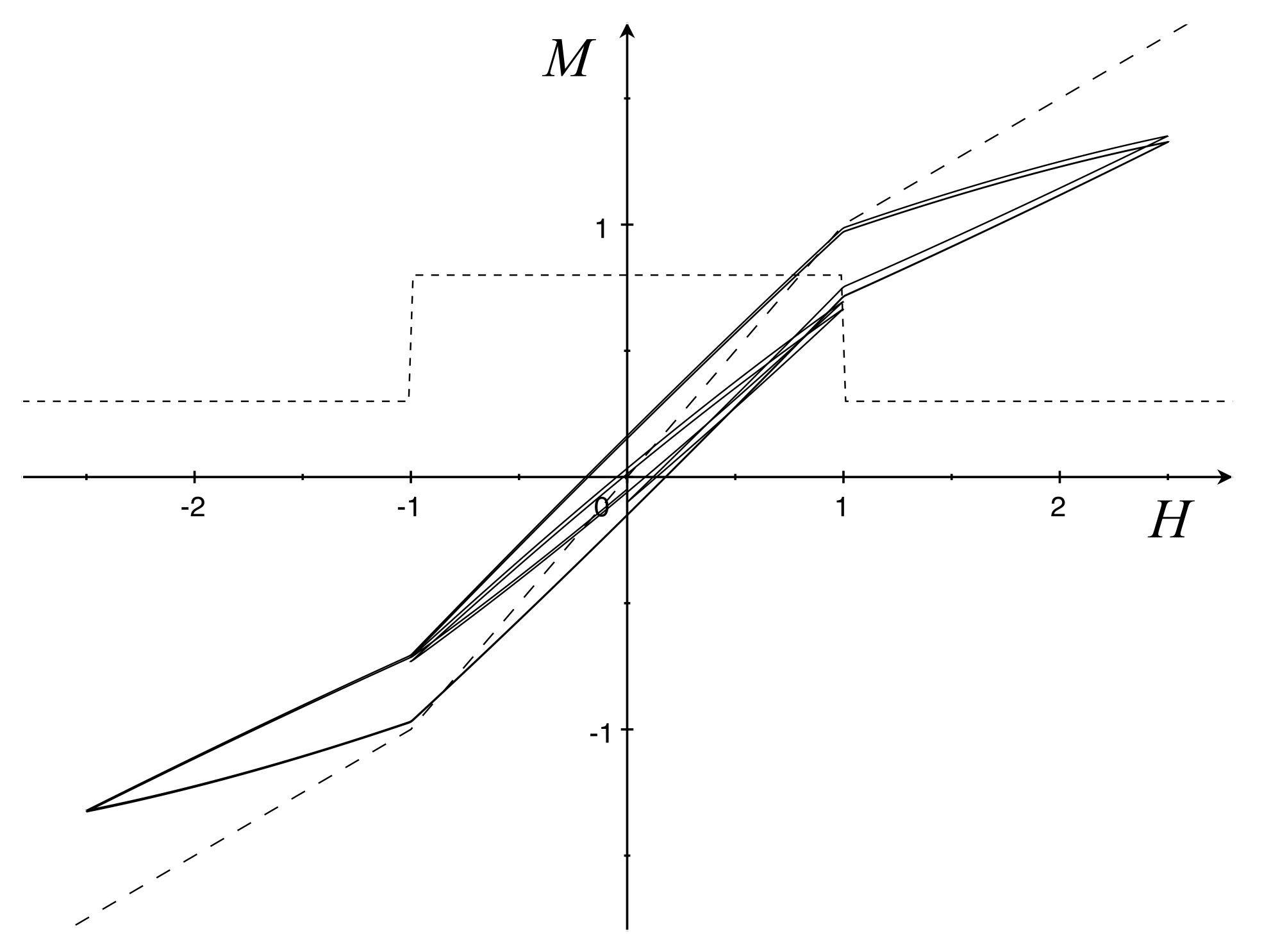
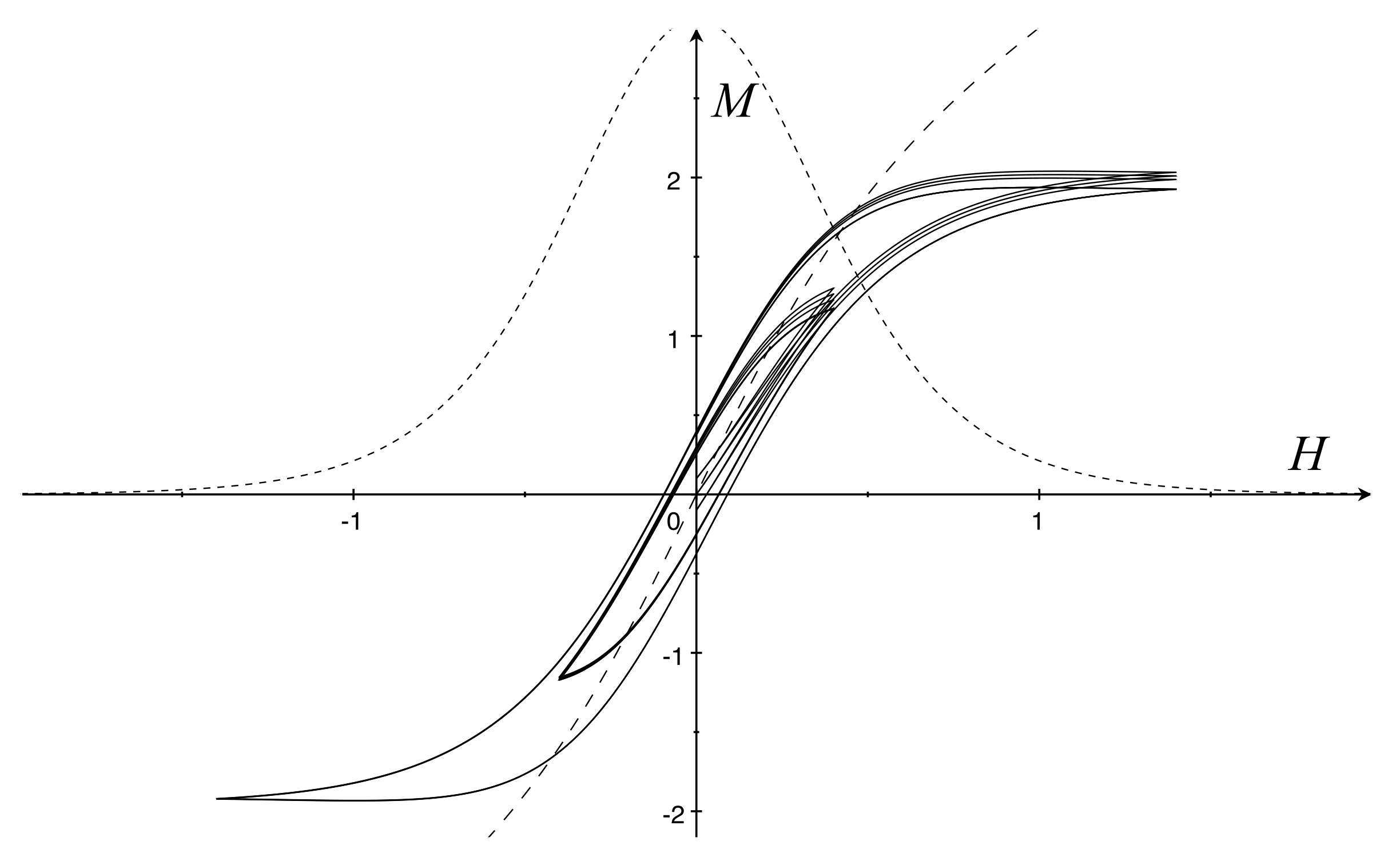
8. Ferromagnetic Hysteresis
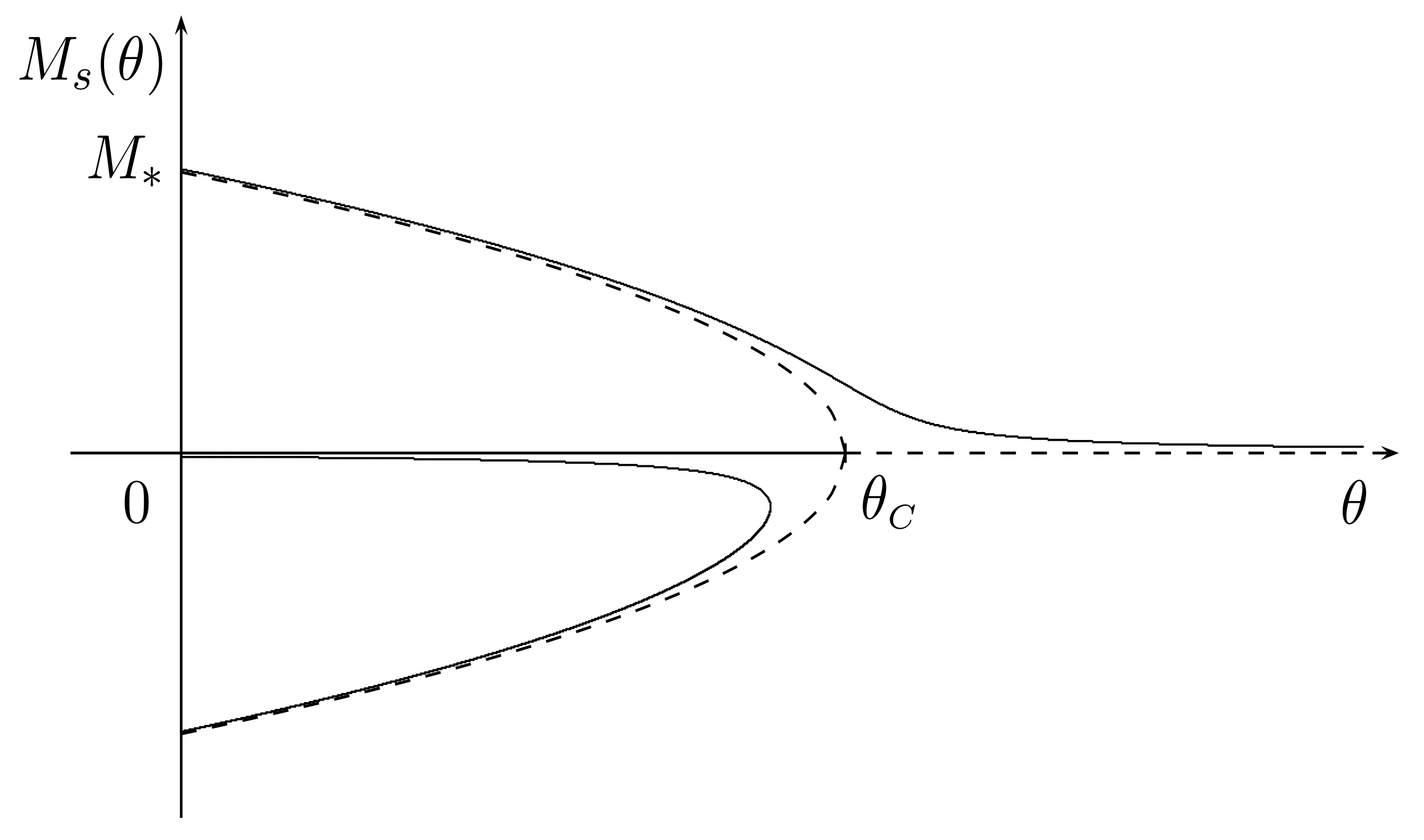
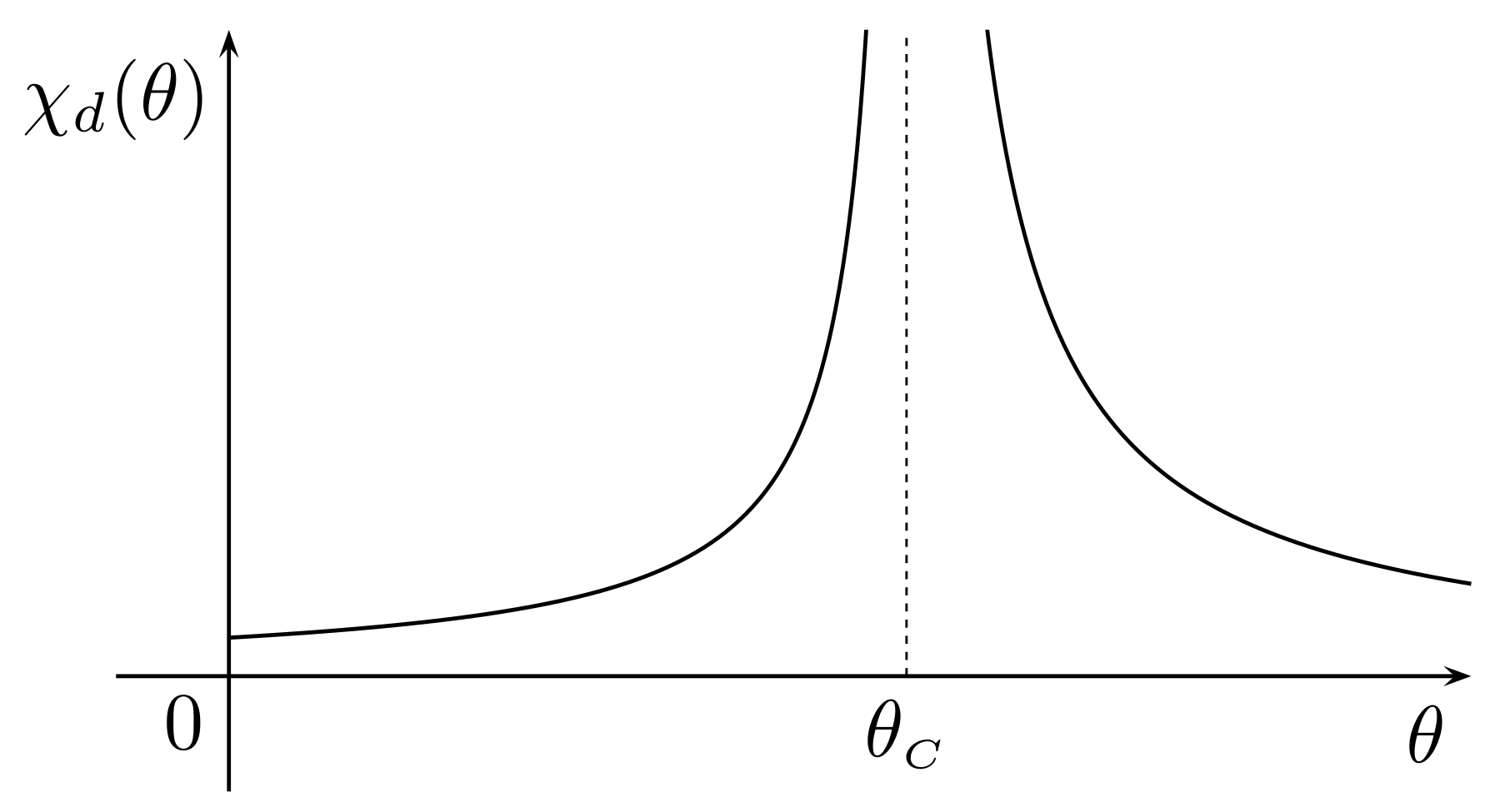
8.1. One-Dimensional Models of Hysteresis
8.2. Soft Iron Models
8.3. Hysteresis Loss
8.4. A Rate-Dependent Generalization
9. Generalization to Materials within Non-Uniform Fields
10. Relation to Other Models
11. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schenck, J.F. The role of magnetic susceptibility in magnetic resonance imaging: MRI magnetic compatibility of the first and second kinds. Med. Phys. 1996, 23, 815–850. [Google Scholar] [CrossRef] [PubMed]
- Ahn, M.C.; Jang, J.; Lee, W.S.; Hahn, S.; Lee, H. Experimental Study on Hysteresis of Screening-Current-Induced Field in an HTS Magnet for NMR Applications. IEEE Trans. Appl. Supercond. 2014, 24, 4301605. [Google Scholar]
- Duhem, P. Die dauernden Änderungen und die Thermodynamik I: Die dauernden Änderungen der Systeme, welche von einer einzingen normalen Veränderlichen abhängen. Z. Phys. Chem. 1897, 22, 543–589. [Google Scholar]
- Chua, L.O.; Stromsmoe, K.A. Lumped circuit models for nonlinear inductors exhibiting hysteresis loops. IEEE Trans. Circuit Theory 1970, CT-17, 564–574. [Google Scholar] [CrossRef]
- Chua, L.O.; Bass, S.C. A generalized hysteresis model. IEEE Trans. Circuit Theory 1972, CT-19, 36–48. [Google Scholar] [CrossRef]
- Coleman, B.D.; Hodgdon, M.L. A constitutive relation for rate-independent hysteresis in ferromagnetically soft materials. Int. J. Eng. Sci. 1986, 24, 897–919. [Google Scholar] [CrossRef]
- Coleman, B.D.; Hodgdon, M.L. On a class of constitutive relations for ferromagnetic hysteresis. Arch. Ration. Mech. Anal. 1987, 99, 375–396. [Google Scholar] [CrossRef]
- Preisach, F. Über the magnetische Natchwirkung. Z. Phys. 1935, 94, 277–302. [Google Scholar] [CrossRef]
- Macki, J.W.; Nistri, P.; Zecca, P. Mathematical models for hysteresis. SIAM Rev. 1993, 35, 94–123. [Google Scholar] [CrossRef]
- Visintin, A. Models of Hysteresis; Pitman Research Notes in Mathematics Series 286; Longman: Burnt Mill, England, UK, 1993. [Google Scholar]
- Jiles, D.C.; Atherton, D.L. Theory of ferromagnetic hysteresis (invited). J. Appl. Phys. 1984, 55, 2115–2120. [Google Scholar] [CrossRef]
- Jiles, D.C.; Atherton, D.L. Theory of ferromagnetic hysteresis. J. Magn. Magn. Mater. 1986, 61, 48–60. [Google Scholar] [CrossRef]
- Ho, K. A thermodynamically consistent model for magnetic hysteresis. J. Magn. Magn. Mater. 2014, 357, 93–96. [Google Scholar] [CrossRef]
- Brokate, M.; Sprekels, J. Hysteresis and Phase Transitions; Springer: New York, NY, USA, 1996. [Google Scholar]
- Visintin, A. Differential Models of Hysteresis; Springer: Berlin, Germany, 1994. [Google Scholar]
- Green, A.E.; Naghdi, P.M. On thermodynamics and the nature of the second law. Proc. R. Soc. Lond. A 1977, 357, 253–270. [Google Scholar]
- Giorgi, C.; Morro, A. A thermodynamic approach to rate-type models of elastic-plastic materials. J. Elast. 2021, 147, 113–148. [Google Scholar] [CrossRef]
- Giorgi, C.; Morro, A. A thermodynamic approach to rate-type models in deformable ferroelectrics. Cont. Mech. Thermodyn. 2021, 33, 727–747. [Google Scholar] [CrossRef]
- Giorgi, C.; Morro, A. Nonlinear models of thermo-viscoelastic materials. Materials 2021, 14, 7617. [Google Scholar] [CrossRef] [PubMed]
- Landau, L.D.; Lifshitz, E.M.; Pitaevskii, L.P. Electrodynamics of Continuous Media; Pergamon Press: Oxford, UK, 1984. [Google Scholar]
- Truesdell, C.; Noll, W. Non-Linear Field Theories of Mechanics. In Encyclopedia of Physics III/3; Flügge, S., Ed.; Springer: Berlin, Germany, 1965. [Google Scholar]
- Verma, S.; Kujur, S.; Sharma, R.; Pathak, D.D. Cucurbit[6]uril-supported Fe3O4 magnetic nanoparticles catalyzed green and sustainable synthesis of 2-substituted benzimidazoles via acceptorless dehydrogenative coupling. ACS Omega 2022, 7, 9754–9764. [Google Scholar] [CrossRef] [PubMed]
- Kittel, C. Introduction to Solid State Physics; Wiley: New York, NY, USA, 1953. [Google Scholar]
- Fabrizio, M.; Giorgi, C.; Morro, A. A thermodynamic approach to non-isothermal phase-field evolution in continuum physics. Physica D 2006, 214, 144–156. [Google Scholar] [CrossRef]
- Liorzou, F.; Phelps, B.; Atherton, D.L. Macroscopic models of magnetization. IEEE Trans. Magn. 2000, 36, 418–427. [Google Scholar] [CrossRef]
- Otténio, M.; Destrade, M.; Ogden, R.W. Incremental magnetoelastic deformations with application to surface instability. J. Elast. 2008, 90, 19–42. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giorgi, C.; Morro, A. Thermodynamically-Consistent Modeling of Ferromagnetic Hysteresis. Materials 2023, 16, 2882. https://doi.org/10.3390/ma16072882
Giorgi C, Morro A. Thermodynamically-Consistent Modeling of Ferromagnetic Hysteresis. Materials. 2023; 16(7):2882. https://doi.org/10.3390/ma16072882
Chicago/Turabian StyleGiorgi, Claudio, and Angelo Morro. 2023. "Thermodynamically-Consistent Modeling of Ferromagnetic Hysteresis" Materials 16, no. 7: 2882. https://doi.org/10.3390/ma16072882
APA StyleGiorgi, C., & Morro, A. (2023). Thermodynamically-Consistent Modeling of Ferromagnetic Hysteresis. Materials, 16(7), 2882. https://doi.org/10.3390/ma16072882






