Hydraulic Characterization of Ceramic Foam Filters Used in Aluminum Filtration
Abstract
1. Introduction
2. Theory
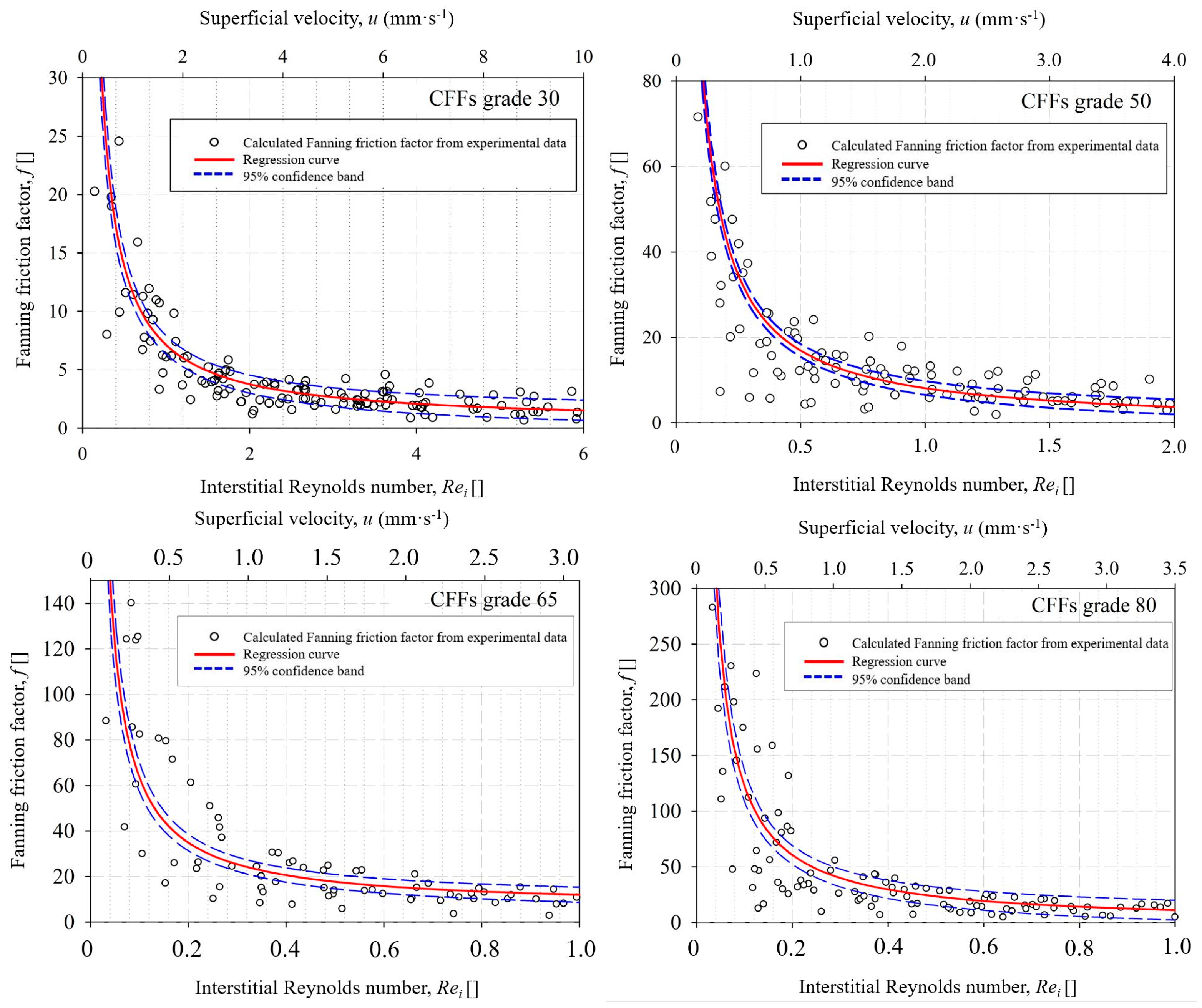
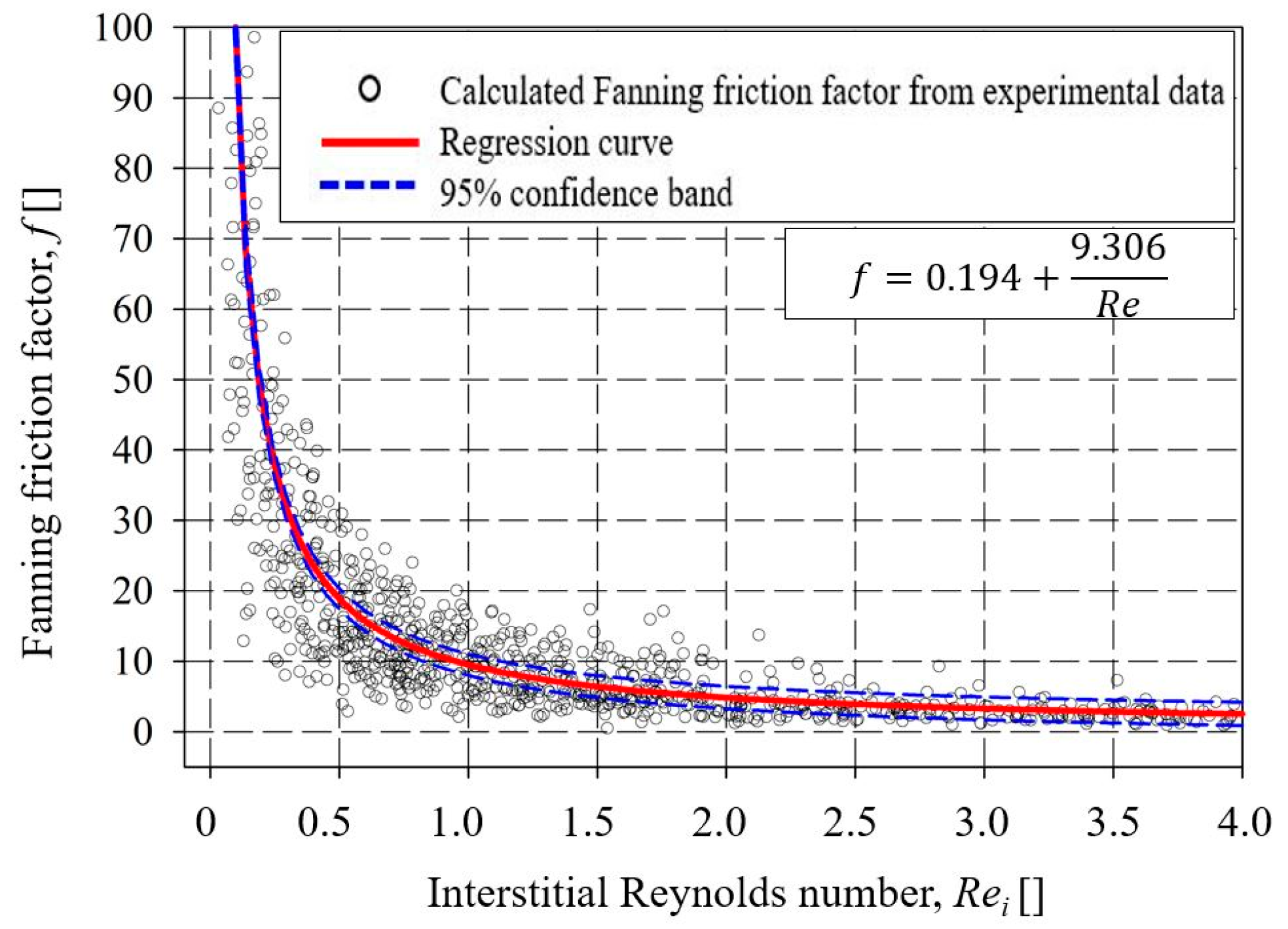
- The first region (Darcy law) is the Darcian flow regime at extremely low (<1 mm·s−1) fluid velocities—there is a linear relationship between the friction factor and Rei, and viscous forces are predominant.
- At the upper end of the Darcian flow regime, the transition flow starts and the linear relation between the friction factor and Rei starts to bend, and the viscous forces are not predominant anymore.
- The third region (Turbulent flow) is the Forchheimer or turbulent flow regime, where the form drag forces are dominant. The Fanning friction factor gets close to zero at the upper end of the transition zone, and the curve becomes almost horizontal.
3. Materials and Methods
3.1. Sample Preparation
3.2. Total Porosity
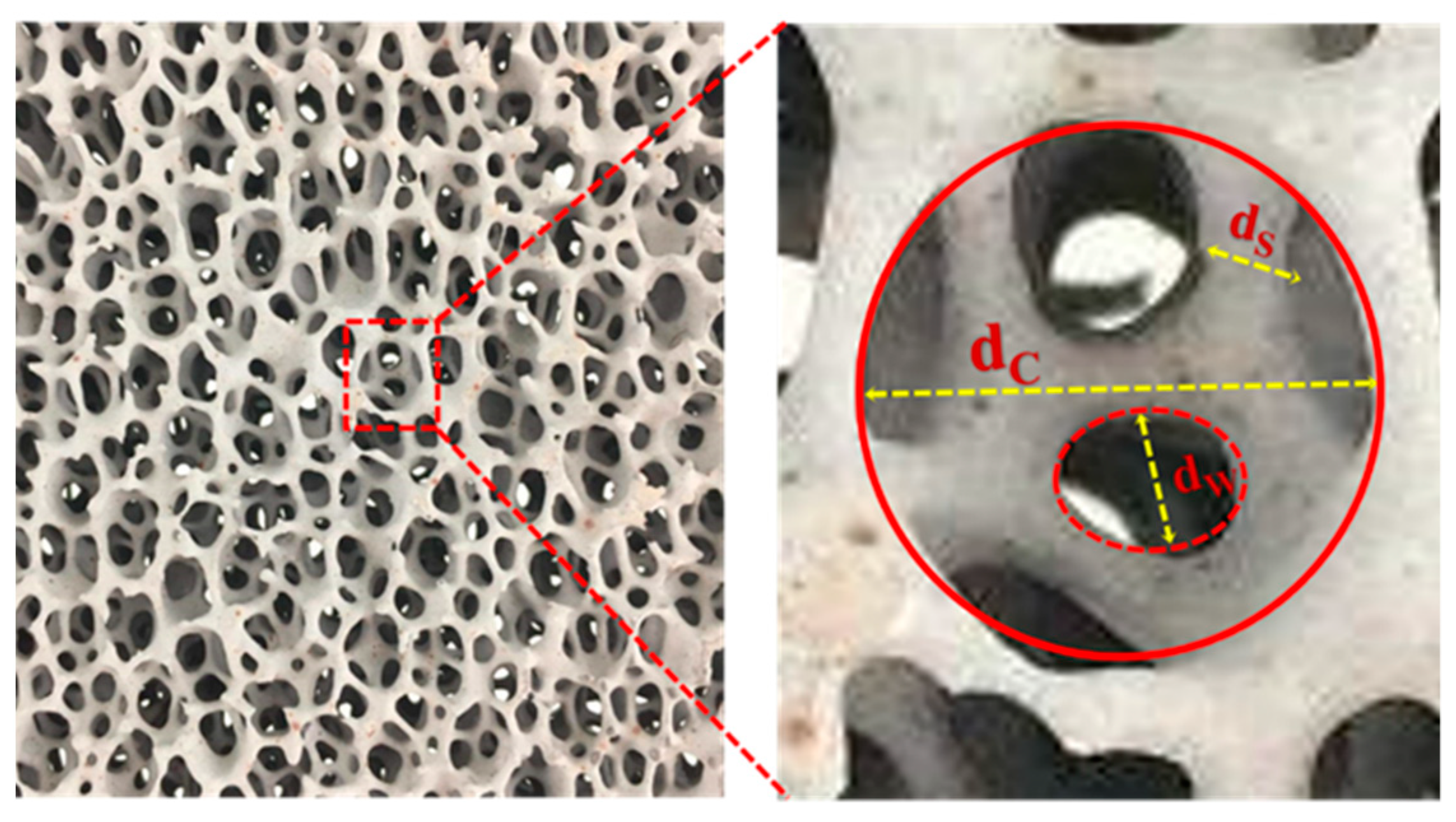
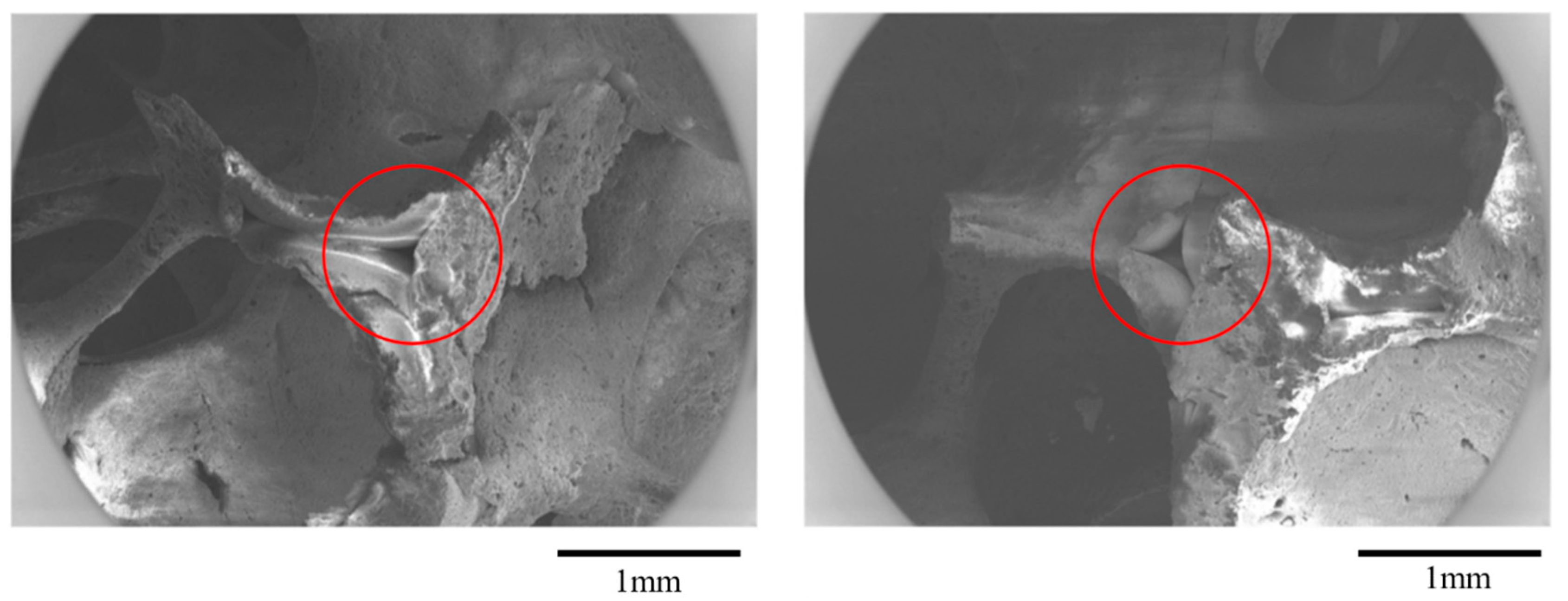
3.3. Physical Morphology
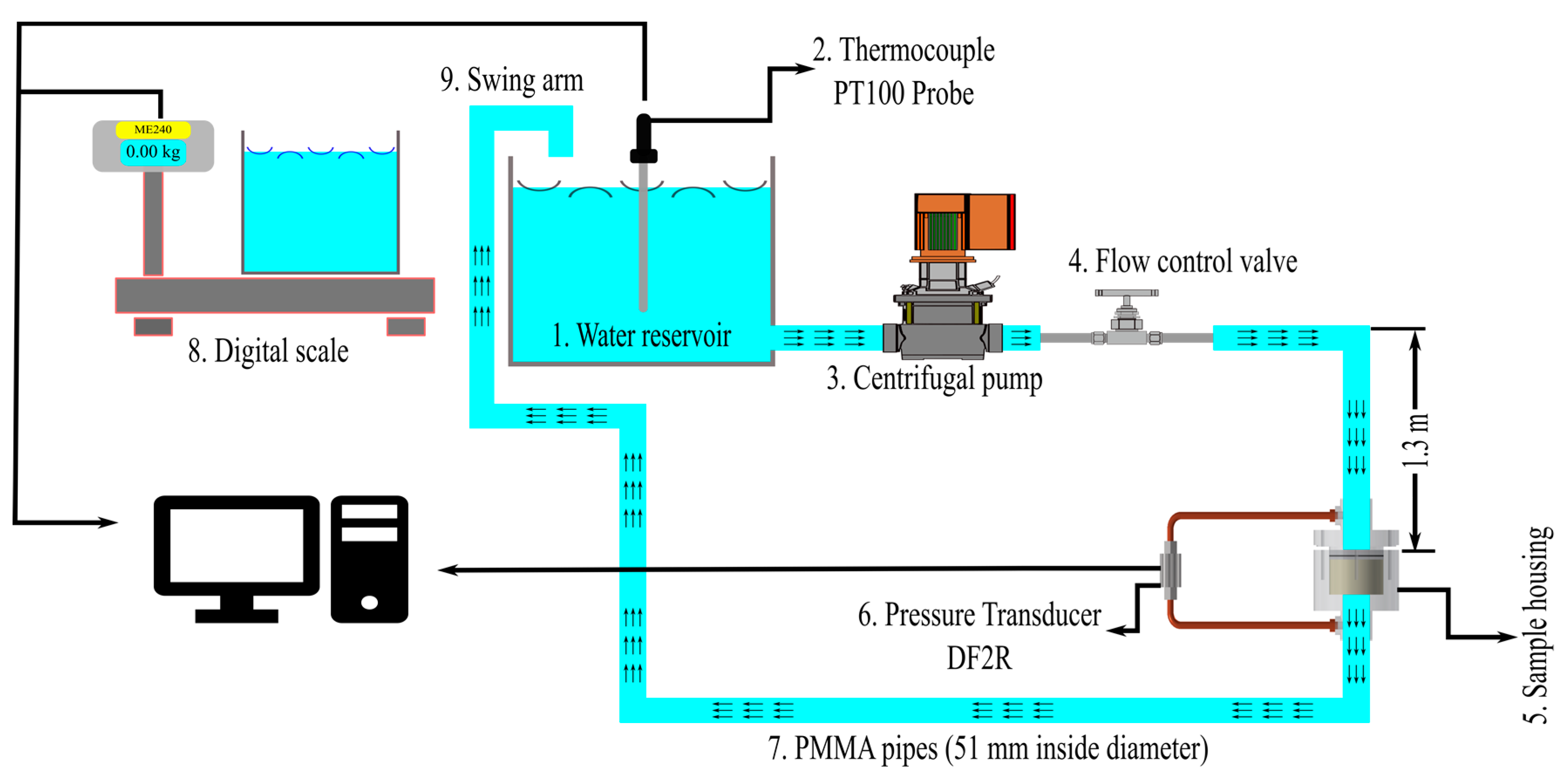
3.4. Pressure Drop Test
- The water reservoir.
- The thermocouple Probe PT100 and data logger GMH3750 from GHM Group, Remscheid, Germany) to measure the water temperature. The thermocouple was factory-calibrated to an expanded uncertainty of ±0.22 °C with a coverage factor of 2. The software (GSOFT 3050, GHM Group, Remscheid, Germany) was used to transfer data from the logger to the PC.
- The vertical multistage centrifugal pump (GRUNDFOS, Bjerringbro, Denmark) with integrated frequency converter, maximum 10 bar pressure and 20.5 m3·h−1.
- The stainless-steel needle valve regulates the flow of water through the system allowing adjustment of the velocity.
- The sample housing.
- The differential pressure transducers DF2R.
- Poly Methyl Methacrylate (PMMA) pipes used to transport the water in the system. The inlet pipe was 1.3 m long to secure a fully developed laminar flow in the low water velocity range. This length was calculated using Equation (10) where D is the pipe diameter (m), LE the entrance length (m), and ReD the Reynolds number of the pipes calculated from the defined flow rate (dimensionless), i.e., 10 mm·s−1,:
- The digital scale model ME204, METTLER TOLEDO, Switzerland, with the readability of 0.1 mg and a maximum capacity of 220 g. The scale was equipped with a LAB-it View software (version 5.0.1), for computer data storage.
- The swingarm that was part of the piping system.
4. Results and Discussion
4.1. Porosity
4.2. Mean Feret Diameter of the Cell and the Window
4.3. Permeability
4.4. Flow Regimes in CFF
5. Conclusions and Future Work
- Comparing the permeability and mean Window Feret diameter of different filters confirmed the need for a systemic method to categorize CFFs.
- Filtration of Al using CFF is performed at the superficial velocity at which the fluid flow in the channels is turbulent.
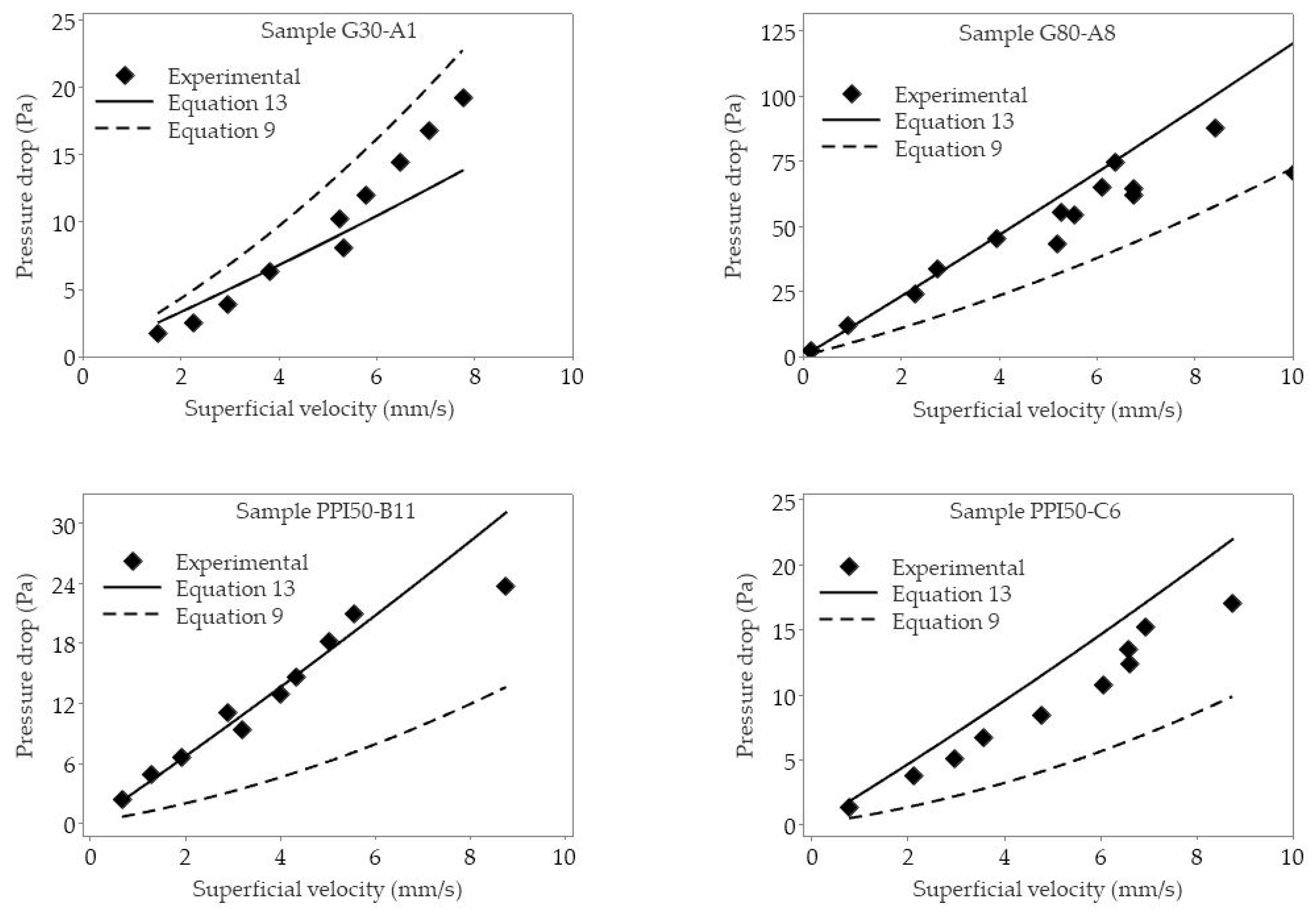
- The derived empirical model for the calculation of the pressure drop of CFFs correlates well with the experimental pressure drop data.
- Empirical expressions for calculating the pressure drop over CFF should be derived based on experimental measurements carried out at the velocity range of the application.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Grade 30 | Grade 50 | |||
|---|---|---|---|---|
| Porosity | Uncertainty | Porosity | Uncertainty | |
| A1 | 8.72 × 10−1 | 3.92 × 10−4 | 8.39 × 10−1 | 8.19 × 10−4 |
| A2 | 8.79 × 10−1 | 7.30 × 10−4 | 8.50 × 10−1 | 1.09 × 10−3 |
| A3 | 8.98 × 10−1 | 8.09 × 10−4 | 8.68 × 10−1 | 1.22 × 10−3 |
| A4 | 8.77 × 10−1 | 1.02 × 10−4 | 8.41 × 10−1 | 1.16 × 10−3 |
| A5 | 8.84 × 10−1 | 9.95 × 10−4 | 8.50 × 10−1 | 7.68 × 10−4 |
| A6 | 8.69 × 10−1 | 6.36 × 10−4 | 8.49 × 10−1 | 8.47 × 10−4 |
| A7 | 8.80 × 10−1 | 8.87 × 10−4 | 8.56 × 10−1 | 2.43 × 10−3 |
| A8 | 8.94 × 10−1 | 8.37 × 10−4 | 8.42 × 10−1 | 8.42 × 10−4 |
| A9 | 8.74 × 10−1 | 1.32 × 10−4 | 8.33 × 10−1 | 1.05 × 10−3 |
| A10 | 8.83 × 10−1 | 7.53 × 10−4 | 8.45 × 10−1 | 9.21 × 10−4 |
| A11 | 8.98 × 10−1 | 1.64 × 10−4 | 8.41 × 10−1 | 7.33 × 10−4 |
| A12 | 8.95 × 10−1 | 4.98 × 10−4 | 8.43 × 10−1 | 1.19 × 10−3 |
| A13 | 8.95 × 10−1 | 1.54 × 10−4 | 8.60 × 10−1 | 1.14 × 10−3 |
| A14 | 8.98 × 10−1 | 1.01 × 10−4 | 8.48 × 10−1 | 1.14 × 10−3 |
| A15 | 8.75 × 10−1 | 6.79 × 10−4 | 8.47 × 10−1 | 1.37 × 10−3 |
| Grade 65 | Grade 80 | |||
|---|---|---|---|---|
| Porosity | Uncertainty | Porosity | Uncertainty | |
| A1 | 8.73 × 10−1 | 9.35 × 10−4 | 8.63 × 10−1 | 1.11 × 10−3 |
| A2 | 8.54 × 10−1 | 1.00 × 10−3 | 8.73 × 10−1 | 3.97 × 10−3 |
| A3 | 8.47 × 10−1 | 9.33 × 10−4 | 8.55 × 10−1 | 4.79 × 10−3 |
| A4 | 8.49 × 10−1 | 7.50 × 10−4 | 8.51 × 10−1 | 1.47 × 10−3 |
| A5 | 8.54 × 10−1 | 1.00 × 10−3 | 8.58 × 10−1 | 2.26 × 10−3 |
| A6 | 8.43 × 10−1 | 1.12 × 10−3 | - | - |
| A7 | 8.42 × 10−1 | 1.41 × 10−3 | 8.63 × 10−1 | 2.72 × 10−3 |
| A8 | 8.45 × 10−1 | 1.19 × 10−3 | 8.44 × 10−1 | 3.12 × 10−3 |
| A9 | 8.77 × 10−1 | 1.89 × 10−3 | 8.40 × 10−1 | 1.75 × 10−3 |
| A10 | 8.58 × 10−1 | 2.01 × 10−3 | 8.57 × 10−1 | 1.38 × 10−3 |
| A11 | 8.47 × 10−1 | 9.59 × 10−4 | 8.55 × 10−1 | 2.63 × 10−3 |
| A12 | 8.46 × 10−1 | 1.82 × 10−3 | 8.58 × 10−1 | 2.42 × 10−3 |
| A13 | 8.76 × 10−1 | 1.67 × 10−3 | 8.59 × 10−1 | 2.02 × 10−3 |
| A14 | 8.55 × 10−1 | 1.34 × 10−3 | 8.42 × 10−1 | 1.76 × 10−3 |
| A15 | 8.60 × 10−1 | 1.24 × 10−3 | 8.49 × 10−1 | 1.02 × 10−2 |
| 30 PPI | 50 PPI | 60 PPI | ||||
|---|---|---|---|---|---|---|
| Porosity | Uncertainty | Porosity | Uncertainty | Porosity | Uncertainty | |
| B1 | 8.83 × 10−1 | 2.89 × 10−4 | - | - | - | - |
| B2 | 8.72 × 10−1 | 3.16 × 10−4 | - | - | - | - |
| B3 | 8.84 × 10−1 | 2.88 × 10−4 | 8.76 × 10−1 | 3.06 × 10−4 | 8.79 × 10−1 | 2.98 × 10−4 |
| B4 | - | - | 8.69 × 10−1 | 3.24 × 10−4 | 8.82 × 10−1 | 2.91 × 10−4 |
| B5 | 8.82 × 10−1 | 2.91 × 10−4 | 8.67 × 10−1 | 3.28 × 10−4 | 8.70 × 10−1 | 3.20 × 10−4 |
| B6 | 8.87 × 10−1 | 2.80 × 10−4 | - | - | - | - |
| B7 | 8.89 × 10−1 | 2.75 × 10−4 | 8.71 × 10−1 | 3.19 × 10−4 | 8.74 × 10−1 | 3.12 × 10−4 |
| B8 | 8.77 × 10−1 | 3.04 × 10−4 | 8.80 × 10−1 | 2.96 × 10−4 | 8.79 × 10−1 | 2.98 × 10−4 |
| B9 | 8.77 × 10−1 | 3.04 × 10−4 | - | - | - | - |
| B10 | 8.77 × 10−1 | 3.04 × 10−4 | 8.73 × 10−1 | 1.78 × 10−3 | 8.67 × 10−1 | 3.28 × 10−4 |
| B11 | - | - | 8.69 × 10−1 | 3.23 × 10−4 | - | - |
| B12 | - | - | - | - | - | - |
| B13 | - | - | 8.79 × 10−1 | 3.00 × 10−4 | 8.67 × 10−1 | 3.29 × 10−4 |
| B14 | - | - | - | - | 8.73 × 10−1 | 3.14 × 10−4 |
| B15 | - | - | 8.70 × 10−1 | 3.22 × 10−4 | 8.70 × 10−1 | 3.22 × 10−4 |
| 30 PPI | 50 PPI | 60 PPI | ||||
|---|---|---|---|---|---|---|
| Porosity | Uncertainty | Porosity | Uncertainty | Porosity | Uncertainty | |
| C1 | 8.88 × 10−1 | 2.30 × 10−4 | - | - | 8.89 × 10−1 | 2.30 × 10−4 |
| C2 | 8.81 × 10−1 | 2.47 × 10−4 | 8.98 × 10−1 | 2.10 × 10−4 | 8.91 × 10−1 | 2.25 × 10−4 |
| C3 | 8.82 × 10−1 | 2.43 × 10−4 | 8.93 × 10−1 | 2.22 × 10−4 | - | - |
| C4 | 8.86 × 10−1 | 2.37 × 10−4 | - | - | - | - |
| C5 | - | - | 8.91 × 10−1 | 2.25 × 10−4 | 8.85 × 10−1 | 2.39 × 10−4 |
| C6 | 8.81 × 10−1 | 2.45 × 10−4 | 8.93 × 10−1 | 2.22 × 10−4 | 8.89 × 10−1 | 2.29 × 10−4 |
| C7 | 8.85 × 10−1 | 2.38 × 10−4 | - | - | - | - |
| C8 | - | - | - | - | - | - |
| C9 | 8.87 × 10−1 | 2.34 × 10−4 | 8.92 × 10−1 | 2.24 × 10−4 | 8.87 × 10−1 | 2.33 × 10−4 |
| C10 | 8.78 × 10−1 | 2.52 × 10−4 | 8.88 × 10−1 | 2.32 × 10−4 | 8.81 × 10−1 | 2.48 × 10−4 |
| C11 | - | - | 8.99 × 10−1 | 2.09 × 10−4 | - | - |
| C12 | - | - | 9.03 × 10−1 | 2.00 × 10−4 | - | - |
| C13 | - | - | - | - | 8.88 × 10−1 | 2.34 × 10−4 |
| C14 | - | - | - | - | 8.84 × 10−1 | 2.39 × 10−4 |
| C15 | - | - | 8.86 × 10−1 | 2.36 × 10−4 | 8.78 × 10−1 | 2.54 × 10−4 |
| G30 | G50 | |||||
|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| A1 | 97 | 252.3 | 853.3 | 240 | 201.1 | 444 |
| A2 | 543 | 257.9 | 685.4 | 183 | 163.1 | 579.7 |
| A3 | 485 | 256.1 | 654.5 | 485 | - | 386.2 |
| A4 | 209 | 226.6 | 960.4 | 191 | 206.9 | 604 |
| A5 | 105 | 270.4 | 768.9 | - | - | - |
| A6 | 212 | 284.2 | 948.6 | 219 | 214.9 | 433.3 |
| A7 | 117 | 254.4 | 759.8 | 158 | 151.3 | 537.1 |
| A8 | 135 | 206.1 | 564.5 | 135 | 130.7 | 471.2 |
| A9 | 83 | 268.2 | 626.5 | 766 | 173.9 | 464.5 |
| A10 | - | - | - | 136 | 109.7 | 430.8 |
| A11 | - | - | - | 146 | 124.4 | 521.7 |
| A12 | 108 | 296.8 | 725.6 | 178 | 121.4 | 539.7 |
| A13 | - | - | - | 129 | 147.4 | 573.4 |
| A14 | 172 | 252.2 | 759.1 | 189 | 184 | 529.1 |
| A15 | 446 | 292.3 | 705.8 | 174 | 133.8 | 534.2 |
| Grade 65 | Grade 80 | |||||
|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| A1 | 167 | 131.2 | 436.4 | 296 | 92.2 | 323.1 |
| A2 | 106 | 108 | 398.8 | 271 | 98.9 | 285.1 |
| A3 | 115 | 87.2 | 443.4 | - | - | - |
| A4 | 217 | 165.7 | 411.1 | 143 | 78.5 | 283.3 |
| A5 | - | - | - | 94 | 67.3 | 305.3 |
| A6 | 169 | 67.8 | 456 | 200 | 147.1 | 427.9 |
| A7 | 168 | 120.9 | 416.1 | 101 | 95 | 397.2 |
| A8 | 131 | 97 | 412.2 | 68 | 89.6 | 319.8 |
| A9 | 195 | 109.3 | 433.3 | 437 | 77.5 | 287.5 |
| A10 | - | - | - | - | - | - |
| A11 | 129 | 93.5 | 437 | 186 | 86.7 | 363.5 |
| A12 | 196 | 97.8 | 425.7 | 230 | 110.4 | 308.7 |
| A13 | - | - | - | 231 | 149.1 | 450.3 |
| A14 | 119 | 62.6 | 237.4 | 121 | 70.2 | 397.6 |
| A15 | 170 | 85.7 | 378.6 | - | - | - |
| 30 PPI | 50 PPI | 60 PPI | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| B1 | 158 | 129 | 556.4 | 159 | 96.1 | 507.2 | 137 | 56.5 | 284.7 |
| B2 | 127 | 190.5 | 733.6 | 78 | 74 | 480 | 140 | 77.8 | 423.2 |
| B3 | 111 | 173.6 | 664.4 | 112 | 82.8 | 442.8 | - | - | - |
| B4 | 100 | 136.4 | 636.3 | - | 119.1 | 591.2 | 117 | 81.9 | 370.9 |
| B5 | 103 | 183 | 763.6 | 118 | 74.2 | 479.1 | 117 | 87 | 413.5 |
| B6 | 131 | 149.2 | 609.8 | 116 | 86.9 | 469 | 115 | 59.3 | 331.4 |
| B7 | 124 | 201.8 | 738.8 | 111 | 105.9 | 519.2 | 153 | 62.1 | 337.8 |
| B8 | 127 | 203.5 | 824.8 | 144 | 102.9 | 563.1 | 124 | 90.3 | 391.5 |
| B9 | 122 | 185 | 716.2 | 133 | 107.1 | 565 | 139 | 72.6 | 392.1 |
| B10 | 138 | 165 | 728.5 | 151 | 88.6 | 461.3 | 92 | 106.5 | 417 |
| B11 | - | - | - | 115 | 107.2 | 580.8 | 182 | 41.6 | 267.2 |
| B12 | - | - | - | 115 | 93.2 | 521.2 | - | 71.4 | 388.4 |
| B13 | - | - | - | 146 | 100.7 | 482.4 | 174 | 71.6 | 391.4 |
| B14 | - | - | - | 174 | 89 | 444.6 | 116 | 57.9 | 337.1 |
| B15 | - | - | - | 133 | 86 | 498.7 | 179 | 43.7 | 249.7 |
| 30 PPI | 50 PPI | 60 PPI | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| C1 | 119 | 250.7 | 991 | 150 | 176.3 | 775.7 | 374 | 82.5 | 450.8 |
| C2 | - | - | - | 167 | 176.3 | 657.5 | - | - | - |
| C3 | 109 | 229 | 846.2 | 143 | 192.9 | 789.7 | 210 | 75.4 | 384.2 |
| C4 | 132 | 218.8 | 779.3 | 174 | 152.7 | 701.8 | 201 | 83.9 | 435.1 |
| C5 | 114 | 226.9 | 928.8 | 129 | 166.3 | 716.5 | - | 80.7 | 436.9 |
| C6 | 123 | 260.7 | 1026.8 | 216 | 163.5 | 703.7 | 232 | 84.8 | 449.2 |
| C7 | 110 | 293.3 | 1044.7 | 160 | 169.3 | 731.3 | 129 | 64.1 | 370 |
| C8 | 111 | 253.3 | 926 | 151 | 188.3 | 825.4 | 206 | 60.5 | 410.3 |
| C9 | 148 | 240.8 | 1083.2 | 165 | 130.7 | 640.2 | 231 | 79.6 | 365.7 |
| C10 | 148 | 218 | 870.5 | 176 | 162.4 | 712.4 | 201 | 60 | 332.7 |
| C11 | 157 | 267.2 | 1065.4 | 198 | 158.5 | 696.1 | 174 | 71.2 | 376.8 |
| C12 | 115 | 288.3 | 1040.6 | 133 | 169.4 | 895.8 | 192 | 79.1 | 410.8 |
| C13 | 131 | 269.4 | 999.9 | 107 | 176.9 | 720.6 | 183 | 63.9 | 359 |
| C14 | 142 | 259.8 | 995.9 | 150 | 146.2 | 644.2 | 238 | 59.2 | 334.6 |
| C15 | 160 | 210.2 | 845.9 | 127 | 190.8 | 667.3 | 173 | 68.1 | 382.1 |
| Grade 30 | Grade 50 | |||||
|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| A1 | 110 | 392.5 | 948.2 | 155 | 459.1 | 972.7 |
| A2 | 348 | 1237.7 | 1836.5 | 149 | 771.3 | 1186.3 |
| A3 | 561 | 755.7 | 1070.7 | 1203 | 336.2 | 565.3 |
| A4 | 94 | 290.6 | 740.1 | 143 | 797.5 | 1370.7 |
| A5 | 134 | 579.2 | 1179.4 | 109 | 722.6 | 1272 |
| A6 | 162 | 345.3 | 1083 | 164 | 469.5 | 879.1 |
| A7 | 143 | 630.6 | 1178.3 | 427 | 635.9 | 957.6 |
| A8 | 180 | 411.2 | 827.5 | 178 | 754.7 | 932.8 |
| A9 | 90 | 802.4 | 1661.8 | 948 | 686.9 | 993.4 |
| A10 | - | - | - | 69 | 599.3 | 1376.1 |
| A11 | - | - | - | 125 | 732.4 | 1571.5 |
| A12 | 107 | 1624 | 1871.9 | 160 | 537.8 | 1129.6 |
| A13 | - | - | - | 156 | 649.7 | 1006.9 |
| A14 | 148 | 360.5 | 983.1 | 225 | 537.5 | 796.9 |
| A15 | 421 | 1195.5 | 1676.2 | 97 | 277.4 | 706.4 |
| Grade 65 | Grade 80 | |||||
|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| A1 | 240 | 412.4 | 729.2 | - | - | - |
| A2 | 137 | 488.8 | 868.2 | 402 | 603.6 | 770 |
| A3 | 62 | 620.6 | 1154.6 | - | - | - |
| A4 | 219 | 612.2 | 1133.6 | 239 | 354.3 | 525.3 |
| A5 | - | - | - | 181 | 435.1 | 713.6 |
| A6 | 222 | 476.6 | 943.2 | 406 | 595.5 | 814.7 |
| A7 | 140 | 444 | 990.9 | 205 | 474.4 | 759.9 |
| A8 | 220 | 568.7 | 833.8 | 194 | 383.5 | 600.2 |
| A9 | 232 | 452.7 | 720.3 | 682 | 401.3 | 596.2 |
| A10 | - | - | - | - | - | - |
| A11 | 158 | 443.7 | 914.9 | 552 | 527.4 | 342.3 |
| A12 | 198 | 687.4 | 1020.8 | 329 | 282.9 | 594.9 |
| A13 | - | - | - | 226 | 343 | 767.9 |
| A14 | 186 | 548.9 | 812.4 | 195 | 310.5 | 739.3 |
| A15 | 509 | 552.7 | 513.3 | - | - | - |
| 30 PPI | 50 PPI | 60 PPI | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| B1 | 196 | 980.1 | 1259.5 | 189 | 734.2 | 1252.2 | 257 | 807.9 | 1244.2 |
| B2 | 146 | 678.2 | 1491.6 | 135 | 440.2 | 1046 | 281 | 444.7 | 878.8 |
| B3 | 253 | 1157.4 | 1397.6 | 313 | 893.8 | 1208.9 | - | - | - |
| B4 | 277 | 1054.5 | 1520.6 | 205 | 505.7 | 1066.2 | 265 | 528.7 | 942.2 |
| B5 | 83 | 491.1 | 1280.1 | 216 | 468.5 | 1003.4 | 336 | 537.9 | 946.3 |
| B6 | 264 | 881 | 1105.2 | 167 | 744.9 | 1208.2 | 244 | 505.2 | 919 |
| B7 | 218 | 545.5 | 1140.6 | 151 | 462.5 | 1202.8 | 258 | 566.7 | 835.4 |
| B8 | 166 | 844.5 | 1382.5 | 409 | 567.9 | 919.3 | 252 | 629.2 | 1016.6 |
| B9 | 88 | 709.3 | 1484.6 | 146 | 595.7 | 1216.7 | 175 | 575.9 | 1139.6 |
| B10 | 182 | 613.4 | 1173.1 | 237 | 507.6 | 918.2 | 235 | 824.8 | 911.1 |
| B11 | - | - | - | 444 | 1162.7 | 1435.3 | 275 | 548.8 | 846.7 |
| B12 | - | - | - | 206 | 484.3 | 1012.3 | 247 | 414.1 | 631.7 |
| B13 | - | - | - | 141 | 684.9 | 1229.8 | 356 | 583 | 721.8 |
| B14 | - | - | - | 178 | 709.4 | 1156.8 | 392 | 346.5 | 559.7 |
| B15 | - | - | - | 205 | 669.8 | 1135.4 | 365 | 576.9 | 742 |
| 30 PPI | 50 PPI | 60 PPI | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | Counts | St. Dev. (µm) | Mean (µm) | |
| C1 | 125 | 1174.6 | 1778.9 | 164 | 991.1 | 1953.7 | 449 | 606.2 | 923.8 |
| C2 | - | - | - | 274 | 657 | 1167.2 | - | - | - |
| C3 | 136 | 1198.9 | 1955.1 | 153 | 1294.8 | 1908 | 236 | 567.4 | 1018.4 |
| C4 | 150 | 1428 | 2017.6 | 192 | 879.8 | 1499.9 | 298 | 509.9 | 960 |
| C5 | 82 | 1738.4 | 2530.5 | 173 | 876.9 | 1545 | 342 | 415.7 | 711.6 |
| C6 | 75 | 1251.1 | 2342.7 | 155 | 1003.1 | 1770.7 | 429 | 660.5 | 1012.6 |
| C7 | 101 | 1158.4 | 2292.3 | 269 | 515.5 | 1066.1 | 233 | 561 | 906.4 |
| C8 | 99 | 1066.9 | 2030.5 | 264 | 646.3 | 1098.3 | 295 | 563.4 | 933.9 |
| C9 | 109 | 1348.2 | 2211.3 | 354 | 582.2 | 1072.4 | 323 | 536.1 | 885.6 |
| C10 | 123 | 942.1 | 1866.3 | 263 | 821.4 | 1278.3 | 307 | 465.5 | 835 |
| C11 | 118 | 928.1 | 2011.2 | 195 | 755.3 | 1386.8 | 290 | 454.5 | 820.9 |
| C12 | 102 | 1054.4 | 2147.1 | 282 | 744.3 | 1229.6 | 308 | 650.1 | |
| C13 | 92 | 1518.3 | 2582.7 | 129 | 815.3 | 1453.6 | 407 | 480.7 | 846.7 |
| C14 | 112 | 709.8 | 1872 | 133 | 750.4 | 1396.7 | 301 | 460.1 | 838.1 |
| C15 | 110 | 1355.4 | 2239.4 | 111 | 842.4 | 1614.1 | 244 | 577.8 | 1012.3 |
| Grade 30 | Grade 50 | |||
|---|---|---|---|---|
| Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | |
| 1 | 8.80 × 10−8 | 6.70 × 10−10 | 1.00 × 10−8 | 8.60 × 10−11 |
| 2 | 4.40 × 10−8 | 6.40 × 10−9 | 2.30 × 10−8 | 3.60 × 10−10 |
| 3 | 2.80 × 10−8 | 3.30 × 10−9 | 2.40 × 10−8 | 3.80 × 10−10 |
| 4 | 4.90 × 10−8 | 1.10 × 10−8 | 4.50 × 10−8 | 1.20 × 10−9 |
| 5 | 4.10 × 10−8 | 5.00 × 10−10 | 2.50 × 10−8 | 1.20 × 10−9 |
| 6 | 7.20 × 10−8 | 7.60 × 10−10 | - | - |
| 7 | 7.40 × 10−8 | 5.10 × 10−9 | 2.50 × 10−8 | 8.60 × 10−10 |
| 8 | 3.90 × 10−8 | 1.10 × 10−9 | 1.30 × 10−8 | 5.50 × 10−10 |
| 9 | * 2.5 × 10−7 | 1.10 × 10−8 | 1.60 × 10−8 | 8.90 × 10−11 |
| 10 | 6.90 × 10−8 | 2.80 × 10−9 | 1.90 × 10−8 | 1.40 × 10−9 |
| 11 | 3.80 × 10−8 | 3.60 × 10−10 | 7.60 × 10−8 | 3.90 × 10−10 |
| 12 | 7.30 × 10−8 | 1.10 × 10−9 | 1.30 × 10−8 | 4.30 × 10−10 |
| 13 | 1.10 × 10−7 | 2.60 × 10−9 | 2.40 × 10−8 | 1.80 × 10−10 |
| 14 | 5.60 × 10−8 | 3.20 × 10−10 | 1.70 × 10−8 | 1.40 × 10−9 |
| 15 | 9.80 × 10−8 | 3.60 × 10−9 | 2.00 × 10−8 | 1.20 × 10−9 |
| Grade 65 | Grade 80 | |||
|---|---|---|---|---|
| Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | |
| 1 | - | - | 1.50 × 10−8 | 1.40 × 10−9 |
| 2 | 9.90 × 10−9 | 9.60 × 10−11 | 8.90 × 10−9 | 3.60 × 10−11 |
| 3 | 8.30 × 10−9 | 5.60 × 10−10 | 3.30 × 10−9 | 2.50 × 10−11 |
| 4 | 1.60 × 10−8 | 7.70 × 10−10 | 1.10 × 10−8 | 5.70 × 10−10 |
| 5 | 2.10 × 10−8 | 7.70 × 10−10 | 1.20 × 10−8 | 7.40 × 10−11 |
| 6 | - | - | - | - |
| 7 | 1.10 × 10−8 | 1.10 × 10−10 | 6.80 × 10−9 | 2.50 × 10−11 |
| 8 | 8.50 × 10−9 | 5.60 × 10−10 | 5.30 × 10−9 | 2.60 × 10−11 |
| 9 | 2.40 × 10−8 | 3.20 × 10−10 | 5.40 × 10−9 | 1.70 × 10−11 |
| 10 | 7.50 × 10−9 | 4.80 × 10−10 | 4.90 × 10−9 | 2.70 × 10−10 |
| 11 | - | - | 2.40 × 10−9 | 4.20 × 10−10 |
| 12 | 9.10 × 10−9 | 1.20 × 10−10 | 5.70 × 10−9 | 2.60 × 10−10 |
| 13 | 9.00 × 10−9 | 2.20 × 10−10 | 9.30 × 10−9 | 8.20 × 10−10 |
| 14 | 9.90 × 10−9 | 1.90 × 10−10 | 4.90 × 10−9 | 4.30 × 10−11 |
| 15 | 1.10 × 10−8 | 1.20 × 10−10 | 1.10 × 10−8 | 6.60 × 10−10 |
| 30 PPI | 50 PPI | 60 PPI | ||||
|---|---|---|---|---|---|---|
| Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | |
| 1 | 4.1 × 10−8 | 1.1 × 10−9 | - | - | - | - |
| 2 | 3.5 × 10−8 | 8.2 × 10−10 | - | - | - | - |
| 3 | 1.7 × 10−7 | 2.9 × 10−9 | 1.0 × 10−8 | 8.7 × 10−11 | 1.1001 × 10−8 | 2 × 10−10 |
| 4 | - | - | 1.8 × 10−8 | 1.4 × 10−10 | 9.4303 × 10−9 | 1 × 10−10 |
| 5 | 9.1 × 10−8 | 8.9 × 10−10 | 2.2 × 10−8 | 2.2 × 10−10 | 5.8 × 10−9 | 8 × 10−11 |
| 6 | 4.5 × 10−8 | 3.7 × 10−10 | - | - | - | - |
| 7 | 1.1 × 10−7 | 9.7 × 10−10 | 1.6 × 10−8 | 2.4 × 10−10 | 1.09 × 10−8 | 1 × 10−10 |
| 8 | 5.9 × 10−8 | 8.3 × 10−10 | 1.3 × 10−8 | 1.3 × 10−10 | 9.5434 × 10−9 | 9 × 10−11 |
| 9 | 1.1 × 10−7 | 1.5 × 10−9 | - | - | - | - |
| 10 | 2.1 × 10−8 | 2.2 × 10−10 | 1.5 × 10−8 | 1.3 × 10−10 | 8.45 × 10−9 | 6 × 10−11 |
| 11 | - | - | 1.6 × 10−8 | 1.8 × 10−10 | - | - |
| 12 | - | - | - | - | - | - |
| 13 | - | - | * 5.5 × 10−8 | 3.1 × 10−10 | 6.16 × 10−9 | 4 × 10−11 |
| 14 | - | - | - | - | 6.06 × 10−9 | 2 × 10−11 |
| 15 | - | - | 1.3 × 10−8 | 1.0 × 10−10 | 6.66 × 10−9 | 4 × 10−11 |
| 30 PPI | 50 PPI | 60 PPI | ||||
|---|---|---|---|---|---|---|
| Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | Permeability (m2) | St. Dev. (m2) | |
| 1 | 1.8 × 10−7 | 2.9 × 10−9 | - | - | 9.1 × 10−9 | 1.9 × 10−10 |
| 2 | 6.6 × 10−8 | 2.5 × 10−9 | 7.0 × 10−8 | 7.1 × 10−10 | 1.2 × 10−8 | 1.8 × 10−10 |
| 3 | 1.5 × 10−7 | 1.6 × 10−9 | 2.7 × 10−8 | 2.1 × 10−10 | - | - |
| 4 | 9.2 × 10−8 | 1.4 × 10−9 | - | - | - | - |
| 5 | - | - | * 9.8 × 10−8 | 2.2 × 10−9 | 1.2 × 10−8 | 1.7 × 10−10 |
| 6 | 8.8 × 10−8 | 8.6 × 10−10 | 3.8 × 10−8 | 5.4 × 10−10 | 9.2 × 10−9 | 1.1 × 10−10 |
| 7 | 2.2 × 10−7 | 2.4 × 10−9 | - | - | - | - |
| 8 | - | - | - | - | - | - |
| 9 | 7.0 × 10−8 | 1.1 × 10−9 | 3.8 × 10−8 | 5.5 × 10−10 | 1.1 × 10−8 | 1.1 × 10−10 |
| 10 | 9.9 × 10−8 | 6.5 × 10−10 | 4.3 × 10−8 | 7.4 × 10−10 | 1.2 × 10−8 | 4.1 × 10−10 |
| 11 | - | - | 3.9 × 10−8 | 3.3 × 10−10 | - | - |
| 12 | - | - | 4.8 × 10−8 | 1.4 × 10−9 | - | - |
| 13 | - | - | - | - | 1.7 × 10−8 | 4.5 × 10−10 |
| 14 | - | - | - | - | 1.0 × 10−8 | 6.8 × 10−11 |
| 15 | - | - | 3.2 × 10−8 | 5.0 × 10−10 | 1.2 × 10−8 | 8.7 × 10−11 |
References
- Scheffler, M. Cellular Ceramics: “Structure, Manufacturing, Properties and Applications”; Wiley-VCH; John Wiley Distributor: New York, NY, USA, 2005. [Google Scholar]
- Engh, T.A.; Sigworth, G.K.; Kvithyld, A. Principles of Metal Refining and Recycling; Oxford University Press: New York, NY, USA, 2021. [Google Scholar]
- Fritzsch, R.; Kennedy, M.W.; Akbarnejad, S.; Aune, R.E. Effect of Electromagnetic Fields on the Priming of High-Grade Ceramic Foam Filters (CFF) with Liquid Aluminium; Light Metals 2015; Springer: Berlin/Heidelberg, Germany, 2015; pp. 929–935. [Google Scholar]
- Wu, J.; Djavanroodi, F.; Gode, C.; Attarilar, S.; Ebrahimi, M. Melt Refining and Purification Processes in Al alloys: A comprehensive study. Mater. Res. Express 2022, 9, 3. [Google Scholar] [CrossRef]
- Apelian, D.; Mutharasan, R. Filtration: A Melt Refining Method. JOM 1980, 32, 14–19. [Google Scholar] [CrossRef]
- Engh, T.A.; Rasch, B.; Bathen, E. Deep Bed Filtration Theory Compared with Experiments, in Essential Readings in Light Metals: Volume 3 Cast Shop for Aluminium Production; Grandfield, J.F., Eskin, D.G., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 263–270. [Google Scholar]
- Grandfield, J.F.; Eskin, D.G.; Bainbridge, I.F. Direct-Chill Casting of Light Alloys: Science and Technology; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2013. [Google Scholar]
- Bao, S.; Engh, T.A.; Syvertsen, M.; Kvithyld, A.; Tangstad, M. Inclusion (Particle) Removal by Interception and Gravity in Ceramic Foam Filters. J. Mater. Sci. 2012, 47, 7986–7998. [Google Scholar] [CrossRef]
- Kocaefe, D.; Murray-Chiasson, A.; Kocaefe, Y.; Waite, P. Modelling of Inclusion Re-entrainment During Filtration. Can. J. Chem. Eng. 2008, 82, 1191–1201. [Google Scholar] [CrossRef]
- Kennedy, M.W.; Zhang, K.X.; Fritzsch, R.; Akhtar, S.; Bakken, J.A.; Aune, R.E. Characterization of Ceramic Foam Filters Used for Liquid Metal Filtration. Met. Mater. Trans. B 2013, 44, 671–690. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Saffari Pour, M.; Jonsson, L.T.I.; Jönsson, P.G. Effect of Fluid Bypassing on the Experimentally Obtained Darcy and Non-Darcy Permeability Parameters of Ceramic Foam Filters. Met. Mater. Trans. B 2016, 48, 197–207. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Tilliander, A.; Sheng, D.-Y.; Jönsson, P.G. Effect of Batch Dissimilarity on Permeability of Stacked Ceramic Foam Filters and Incompressible Fluid Flow: Experimental and Numerical Investigation. Metals 2022, 12, 1001. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Jonsson, L.T.I.; Kennedy, M.W.; Aune, R.E.; Jönsson, P.G. Analysis on experimental investigation and mathematical modeling of incompressible flow through ceramic foam filters. Met. Mater. Trans. B 2016, 47, 2229–2243. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Kennedy, M.W.; Fritzsch, R.; Aune, R.E. An Investigation on Permeability of Ceramic Foam Filters (CFF). Light Met. 2015, 949–954. [Google Scholar] [CrossRef]
- Akbarnejad, S.; Saffari Pour, M.; Jonsson, L.; Jönsson, P. Significance of Fluid Bypassing Effect on Darcy and Non-Darcy Permeability Parameters of Ceramic Foam Filters. 2016. Available online: http://urn.kb.se/resolve?urn=urn:nbn:se:kth:diva-183073 (accessed on 22 March 2023).
- Milligan, B.; Ray, S.F. Recent Improvements in the Measurement and Control of Ceramic Foam Filter Quality. In Proceedings of the International Conference Advances in Production and Processing of Aluminium, (APPA 2001), Manama, Bahrain, 12–15 February 2001; Elsevier: Amsterdam, The Netherlands, 2001; p. 15. [Google Scholar]
- Bear, J. Dynamics of Fluids in Porous Media; Dover Publications: New York, NY, USA, 1988; p. 764. [Google Scholar]
- Bağcı, Ö.; Dukhan, N.; Özdemir, M. Flow Regimes in Packed Beds of Spheres from Pre-Darcy to Turbulent. Transp. Porous Media 2014, 104, 501–520. [Google Scholar] [CrossRef]
- Innocentini, M.D.; Salvini, V.R.; Macedo, A.; Pandolfelli, V.C. Prediction of Ceramic Foams Permeability Using Ergun’s Equation. Mater. Res. 1999, 2, 283–289. [Google Scholar] [CrossRef]
- Dukhan, N.; Bagci, O.; Ozdemir, M. Experimental Flow in Various Porous Media and Reconciliation of Forchheimer and Ergun Relations. Exp. Therm. Fluid Sci. 2014, 57, 425–433. [Google Scholar] [CrossRef]
- Ingham, D.B.; Pop, I.I. Transport Phenomena in Porous Media; Kidlington: Oxford, UK, 1998. [Google Scholar]
- Nield, D.A.; Bejan, A. Convection in Porous Media, 3rd ed.; Springer: New York, NY, USA, 2006. [Google Scholar]
- Wilson, L.; Narasimhan, A.; Venkateshan, S.P. Permeability and Form Coefficient Measurement of Porous Inserts with Non-Darcy Model Using Non-Plug Flow Experiments. J. Fluids Eng. 2005, 128, 638–642. [Google Scholar] [CrossRef]
- Ergun, S.; Orning, A.A. Fluid Flow Through Randomly Packed Columns and Fluidized Beds. Ind. Eng. Chem. 1949, 41, 1179–1184. [Google Scholar] [CrossRef]
- Dietrich, B.; Schabel, W.; Kind, M.; Martin, H. Pressure Drop Measurements of Ceramic Sponges-Determining the Hydraulic Diameter. Chem. Eng. Sci. 2009, 64, 3633–3640. [Google Scholar] [CrossRef]
- Fourie, J.G.; Plessis, J.P.D. Pressure Drop Modelling in Cellular Metallic Foams. Chem. Eng. Sci. 2002, 57, 2781–2789. [Google Scholar] [CrossRef]
- Viswanath, D.S. Viscosity of Liquids: Theory, Estimation, Experiment; Springer: Cham, The Netherlands, 2007. [Google Scholar]
- Ji, Z.G. Hydrodynamics and Water Quality: Modelling Rivers, Lakes, and Estuaries; Wiley-Interscience: Hoboken, NJ, USA, 2008. [Google Scholar]
- Tundal, U.; Steen, I.; Strmsvg, G.; Haugen, T.; Hkonsen, A. Drain Free Filtration (DFF)—A New CFF Technology; Springer International Publishing: Cham, The Netherlands, 2019. [Google Scholar]





| CFF Supplier | Dimensions (Length × Width × Height) (mm) | Grade | PPI |
|---|---|---|---|
| A | 584.2 × 584.2 × 51 | 30, 50, 65 and 80 | - |
| B | 584.2 × 584.2 × 51 | - | 30, 50, and 60 |
| C | 508 × 508 × 50 | - | 30, 50, and 60 |
| CFF Supplier | True Particle Density (kg·m−3) | Std. Dev. (kg·m−3) | Average True Particle Density (kg·m−3) | |
|---|---|---|---|---|
| A | Grade 30 | 3413.6 | 9 | 3426.3 |
| Grade 50 | 3431.5 | 3.8 | ||
| Grade 65 | 3434.4 | 6.2 | ||
| Grade 80 | 3425.8 | 4 | ||
| B | 30 PPI | 3427.8 | 7.8 | 3427.9 |
| 50 PPI | 3417.3 | 8.3 | ||
| 60 PPI | 3438.8 | 4.4 | ||
| C | 30 PPI | 3398.3 | 8.1 | 3388.8 |
| 50 PPI | 3391.7 | 7.3 | ||
| 60 PPI | 3376.5 | 3 |
| Supplier A | Supplier B | Supplier C | |||||||
|---|---|---|---|---|---|---|---|---|---|
| G * 30 | G 50 | G 65 | G 80 | 30 PPI | 50 PPI | 60 PPI | 30 PPI | 50 PPI | 60 PPI |
| 0.88 | 0.85 | 0.86 | 0.85 | 0.88 | 0.87 | 0.87 | 0.88 | 0.89 | 0.89 |
| * Grade | |||||||||
| Supplier and Grade/PPI Number | Counts (Number of Samples) | Cell | Window | ||
|---|---|---|---|---|---|
| Mean Feret Diameter (µm) | Pooled Std. Dev. (µm) | Mean Feret Diameter (µm) | Pooled Std. Dev. (µm) | ||
| A Grade 30 | 12 | 1254.7 | 893.1 | 751.0 | 262.9 |
| A Grade 50 | 14 | 1047.8 | 577.8 | 503.5 | 388.6 |
| A Grade 65 | 12 | 886.3 | 531.4 | 407.2 | 109.3 |
| A Grade 80 | 12 | 655.9 | 460.2 | 345.8 | 102.2 |
| B 30 PPI | 10 | 1323.5 | 872.0 | 697.2 | 172.8 |
| B 50 PPI | 15 | 1134.1 | 722.6 | 501.0 | 93.7 |
| B 60 PPI | 14 | 881.1 | 568.3 | 354.4 | 69.7 |
| C 30 PPI | 14 | 2134.1 | 1219.1 | 960.3 | 249.1 |
| C 50 PPI | 15 | 1429.3 | 793.5 | 725.2 | 167.7 |
| C 60 PPI | 13 | 900.4 | 535.3 | 389.3 | 73.4 |
| Equality of the Sample Variances (F-Test) | Significance of the Means (t-Test, Equal Variances) | |||
|---|---|---|---|---|
| Mean Permeability (m2) | A30 = 6.29 × 10−8 | B30 = 7.5 × 10−8 | A30 = 6.29 × 10−8 | B30 = 7.5 × 10−8 |
| Observation Number | A30 = 14 | B30 = 9 | A30 = 14 | B30 = 9 |
| Degrees of Freedom (df) | A30 = 13 | B30 = 8 | 21 | |
| F or T-critical (One tail) | 0.36 | 1.72 | ||
| Test Statistic | 0.28 | 0.82 | ||
| Null (H0) | S *A302 = SB302 | |||
| Reject Null | No | No | ||
| * “S” is the sample standard deviation. ** “” is the sample mean. | ||||
| Supplier B | Supplier C | ||||||
|---|---|---|---|---|---|---|---|
| PPI 30 | PPI 50 | PPI 60 | PPI 30 | PPI 50 | PPI 60 | ||
| Supplier A | Grade 30 | ||||||
| Grade 50 | |||||||
| Grade 65 | |||||||
| Grade 80 | |||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassanabadi, M.; Berto, T.; Akhtar, S.; Aune, R.E. Hydraulic Characterization of Ceramic Foam Filters Used in Aluminum Filtration. Materials 2023, 16, 2805. https://doi.org/10.3390/ma16072805
Hassanabadi M, Berto T, Akhtar S, Aune RE. Hydraulic Characterization of Ceramic Foam Filters Used in Aluminum Filtration. Materials. 2023; 16(7):2805. https://doi.org/10.3390/ma16072805
Chicago/Turabian StyleHassanabadi, Massoud, Thomas Berto, Shahid Akhtar, and Ragnhild E. Aune. 2023. "Hydraulic Characterization of Ceramic Foam Filters Used in Aluminum Filtration" Materials 16, no. 7: 2805. https://doi.org/10.3390/ma16072805
APA StyleHassanabadi, M., Berto, T., Akhtar, S., & Aune, R. E. (2023). Hydraulic Characterization of Ceramic Foam Filters Used in Aluminum Filtration. Materials, 16(7), 2805. https://doi.org/10.3390/ma16072805





