An Approximately Isotropic Origami Honeycomb Structure and Its Energy Absorption Behaviors
Abstract
:1. Introduction
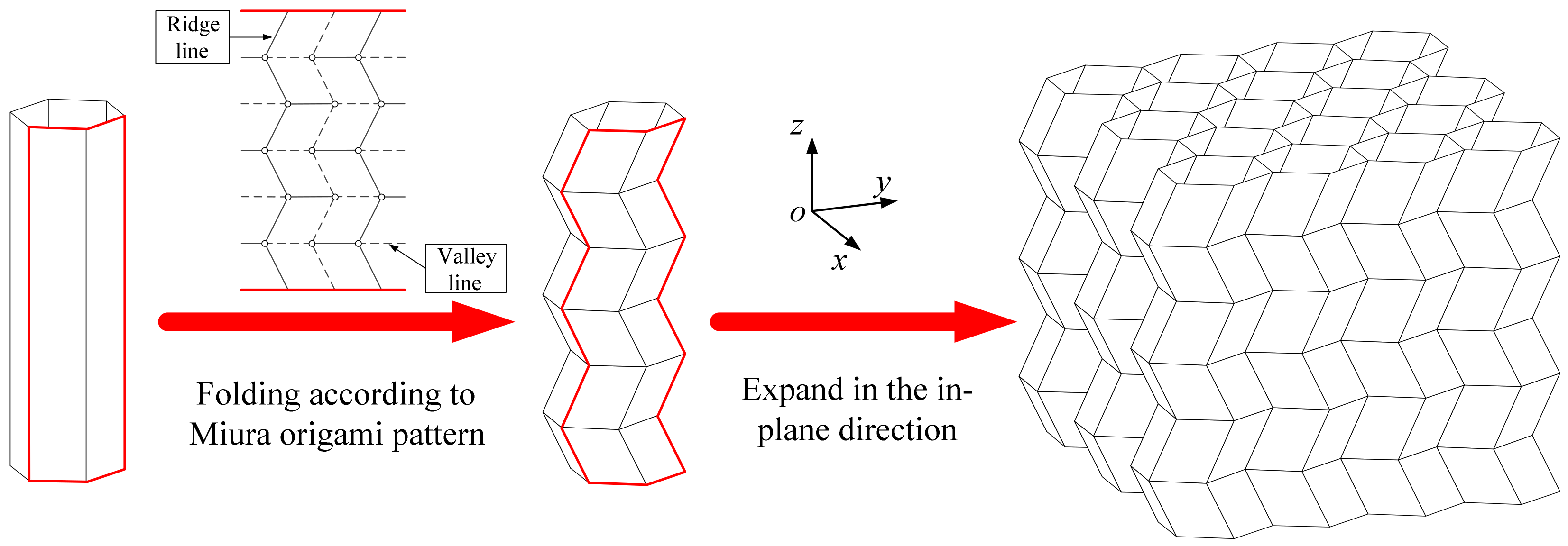
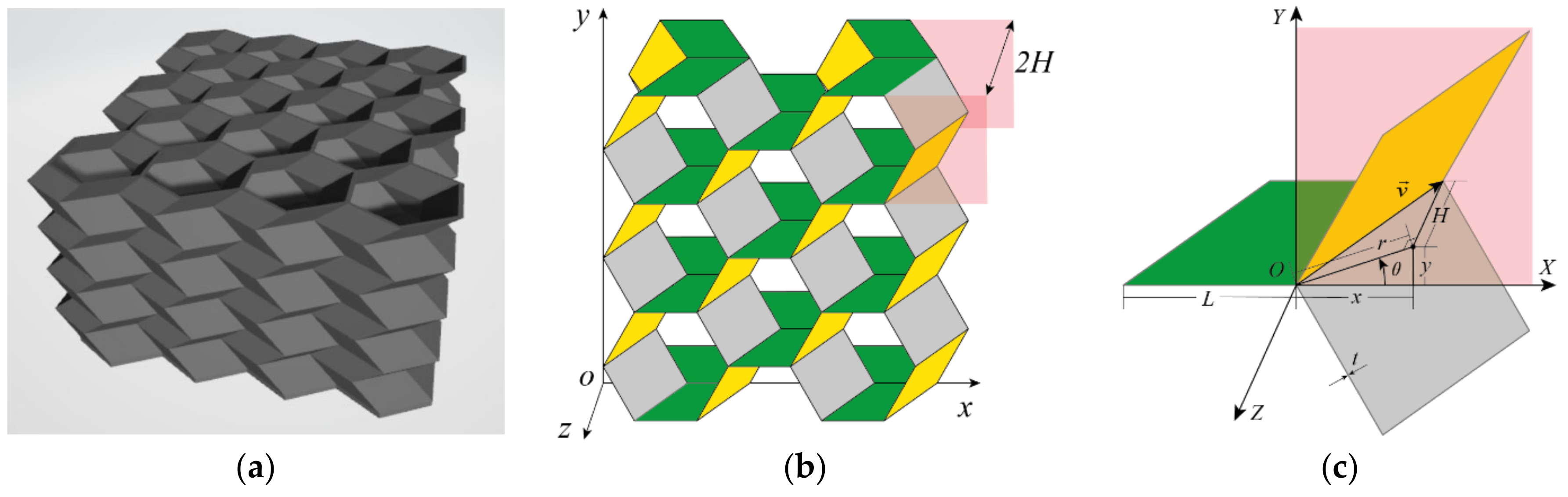
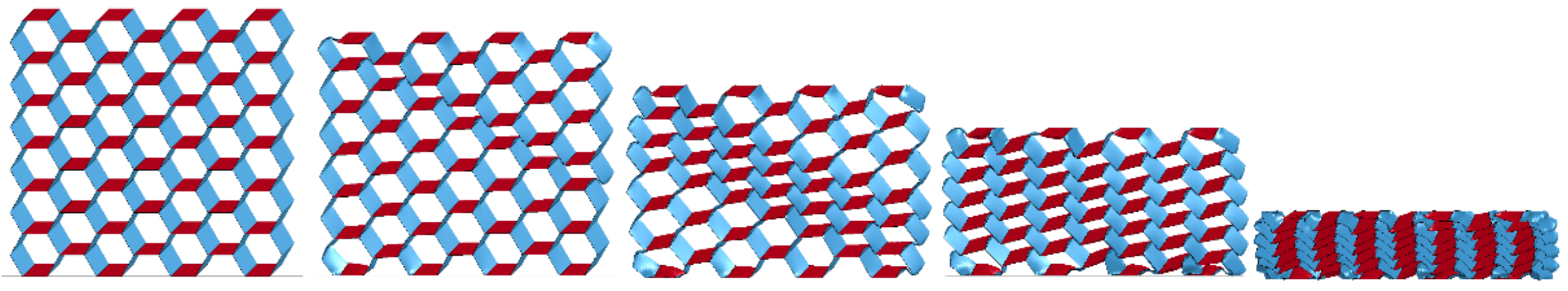
2. Geometry of the Origami Honeycomb
3. Numerical Simulation of Origami Honeycomb
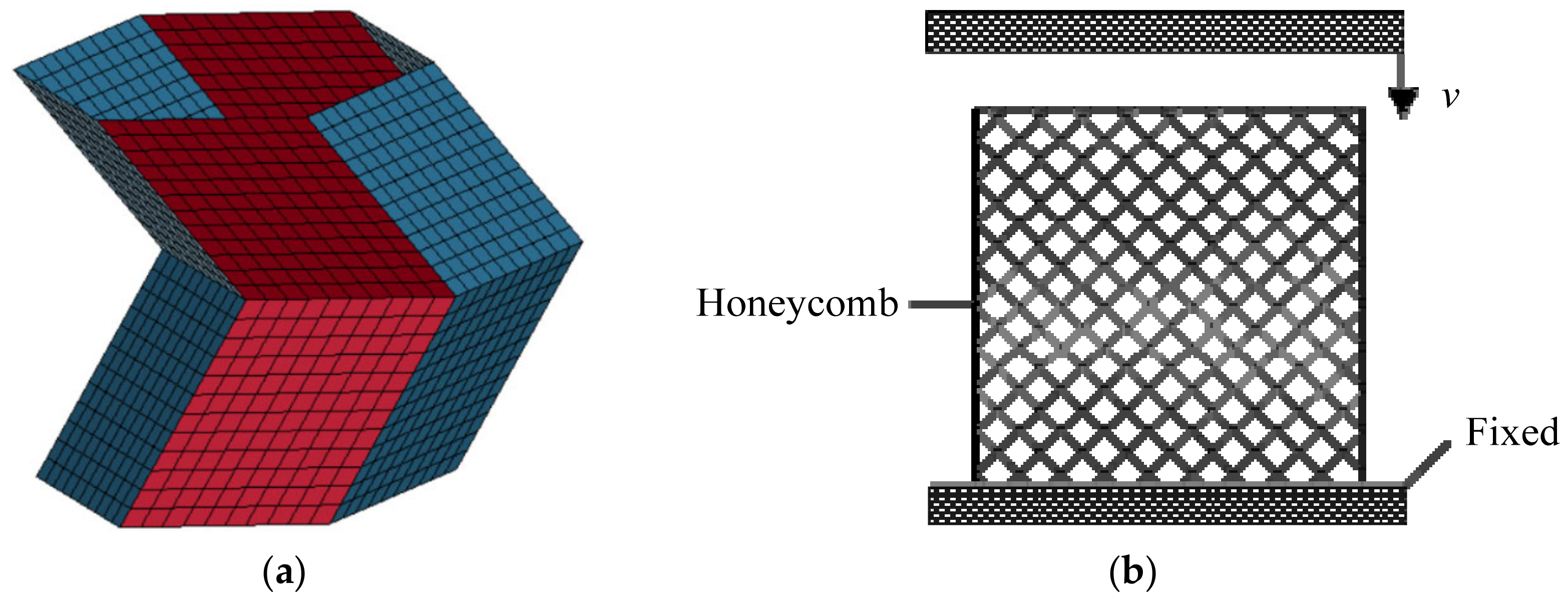
3.1. Numerical Model
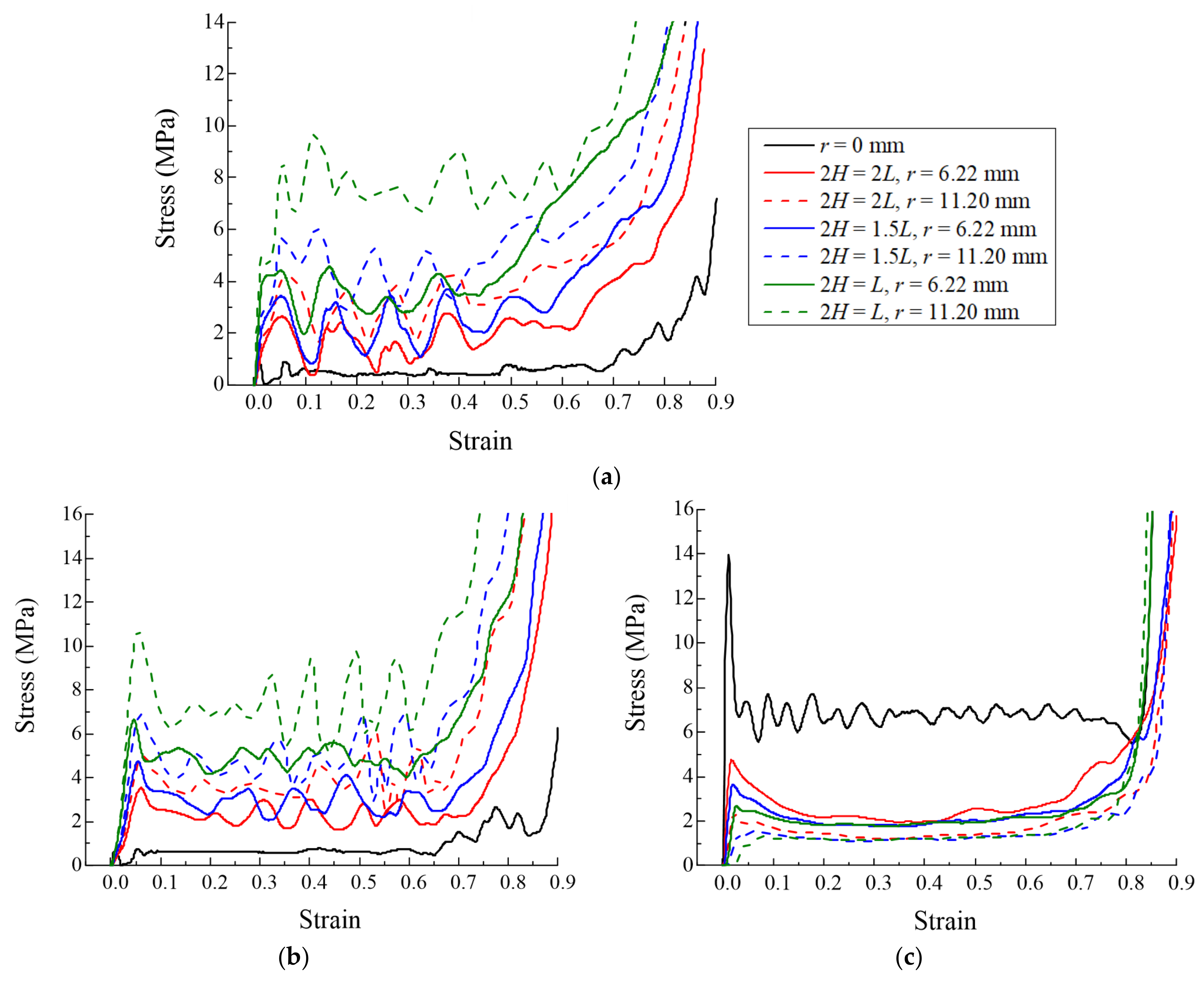
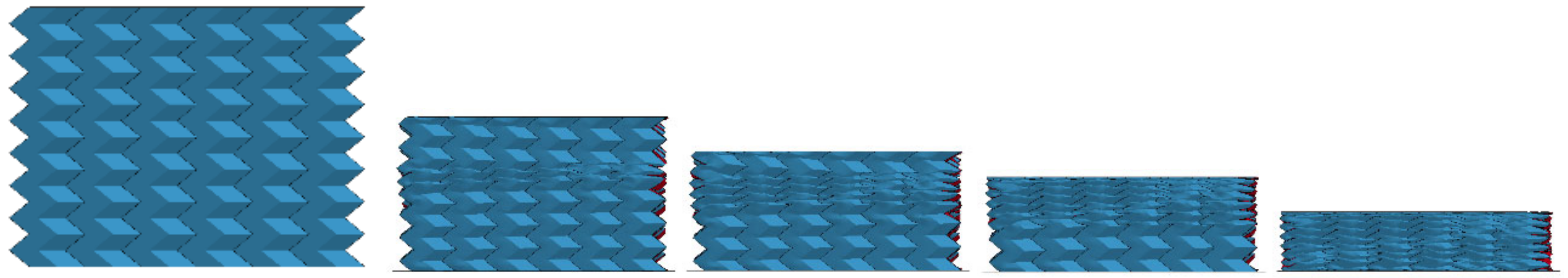
3.2. Numerical Results and Analysis
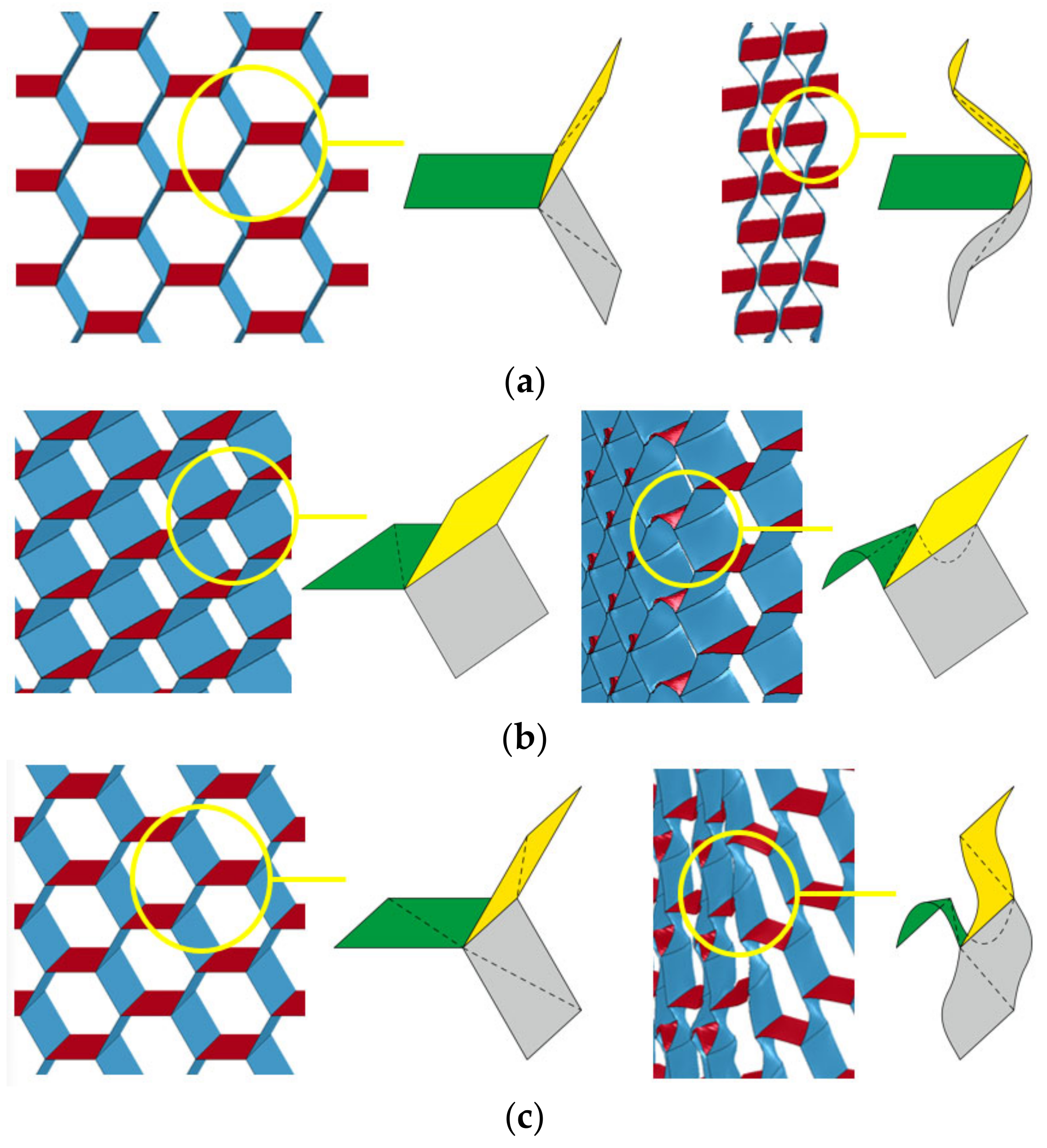
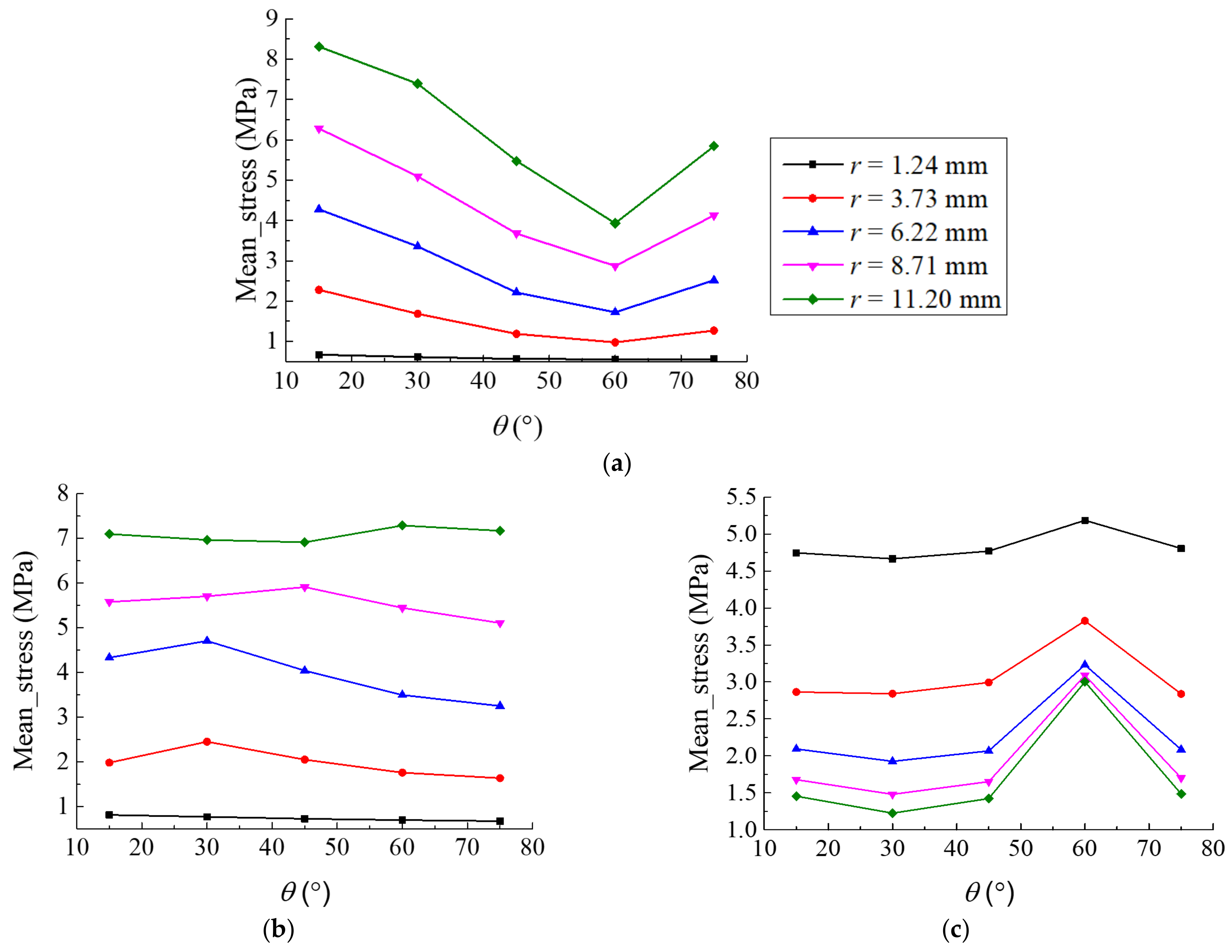
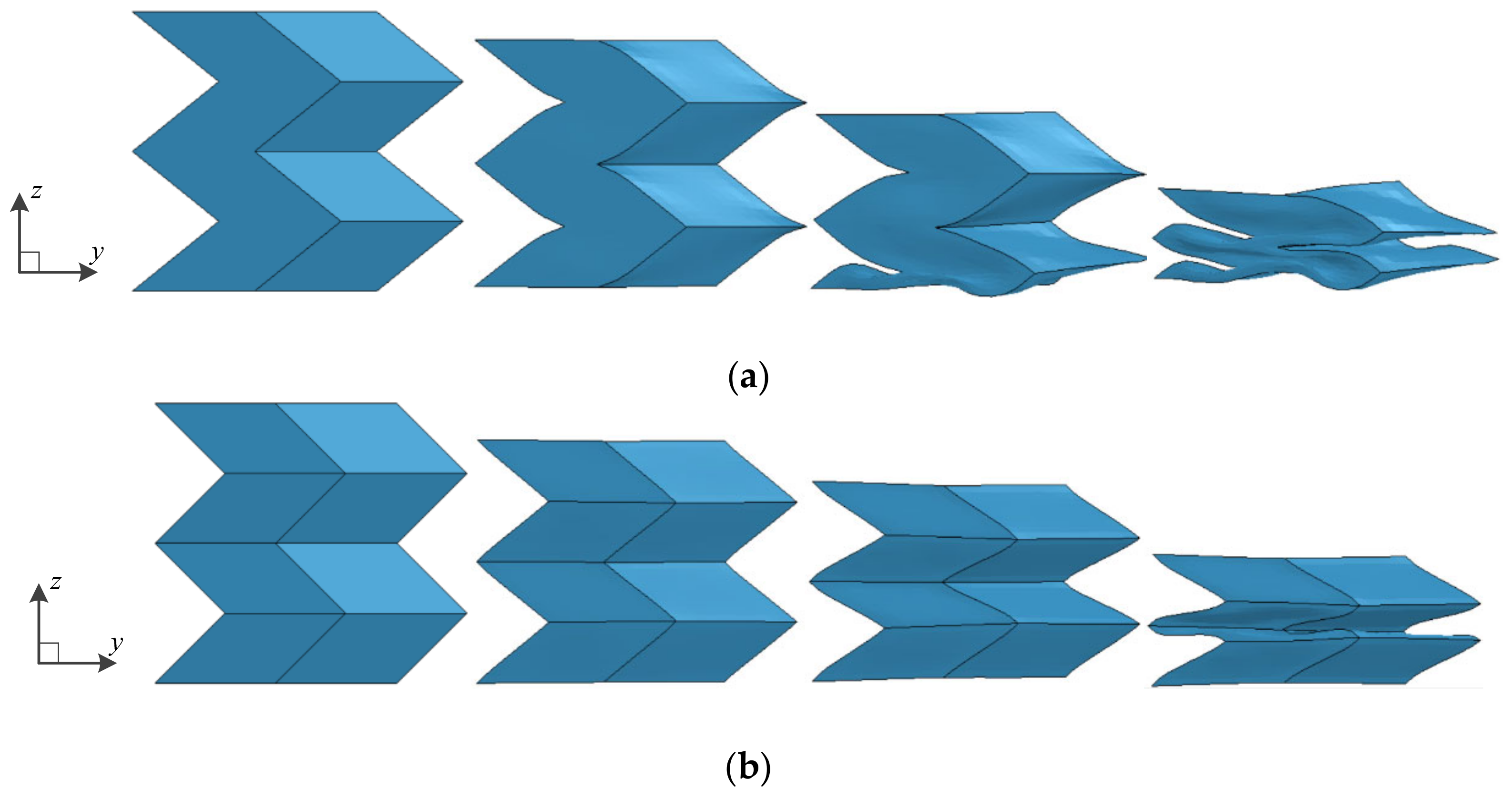
3.3. Deformation Mode and Mean Stress of Origami Honeycomb
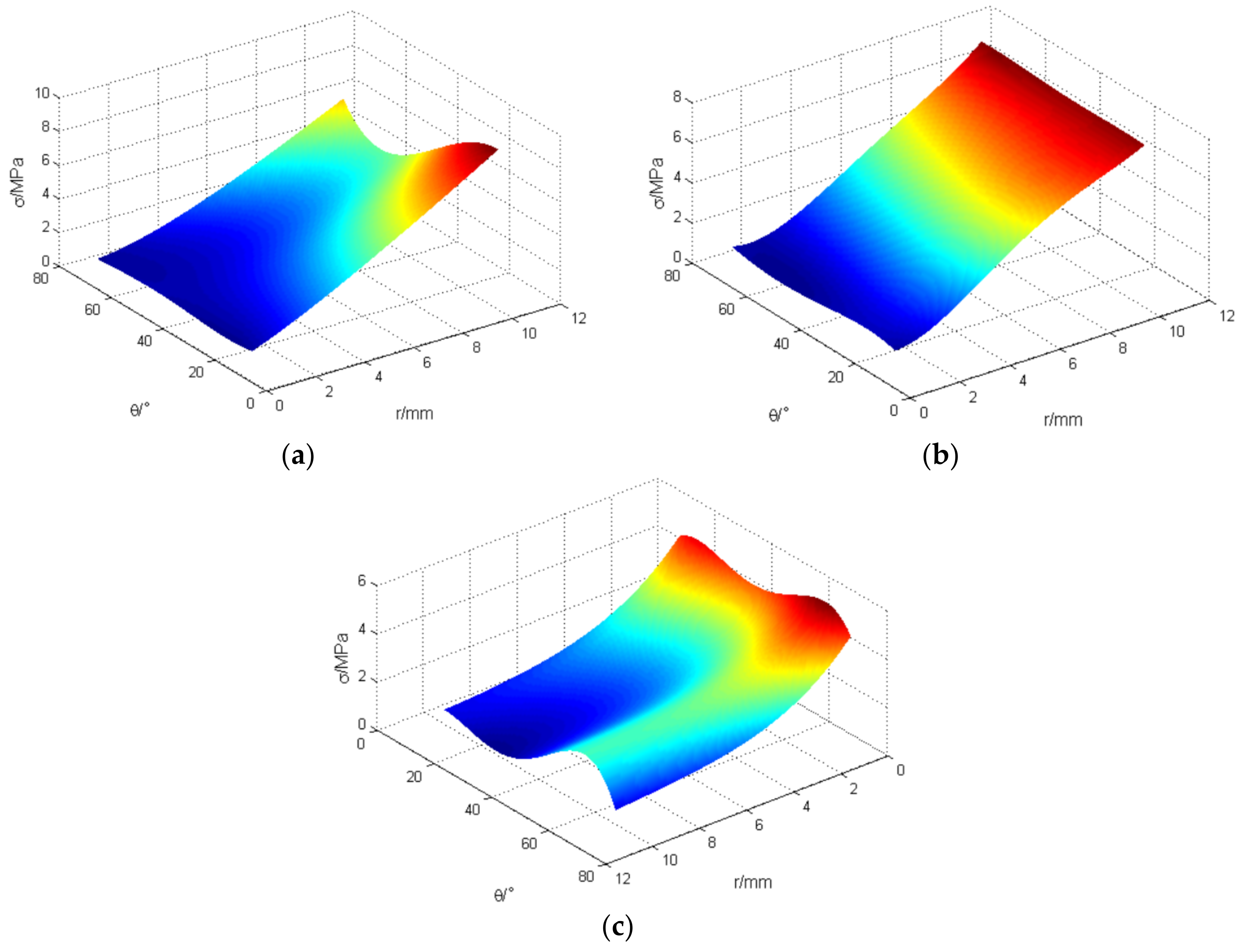
3.4. Response Surface Analysis of Mean Stress
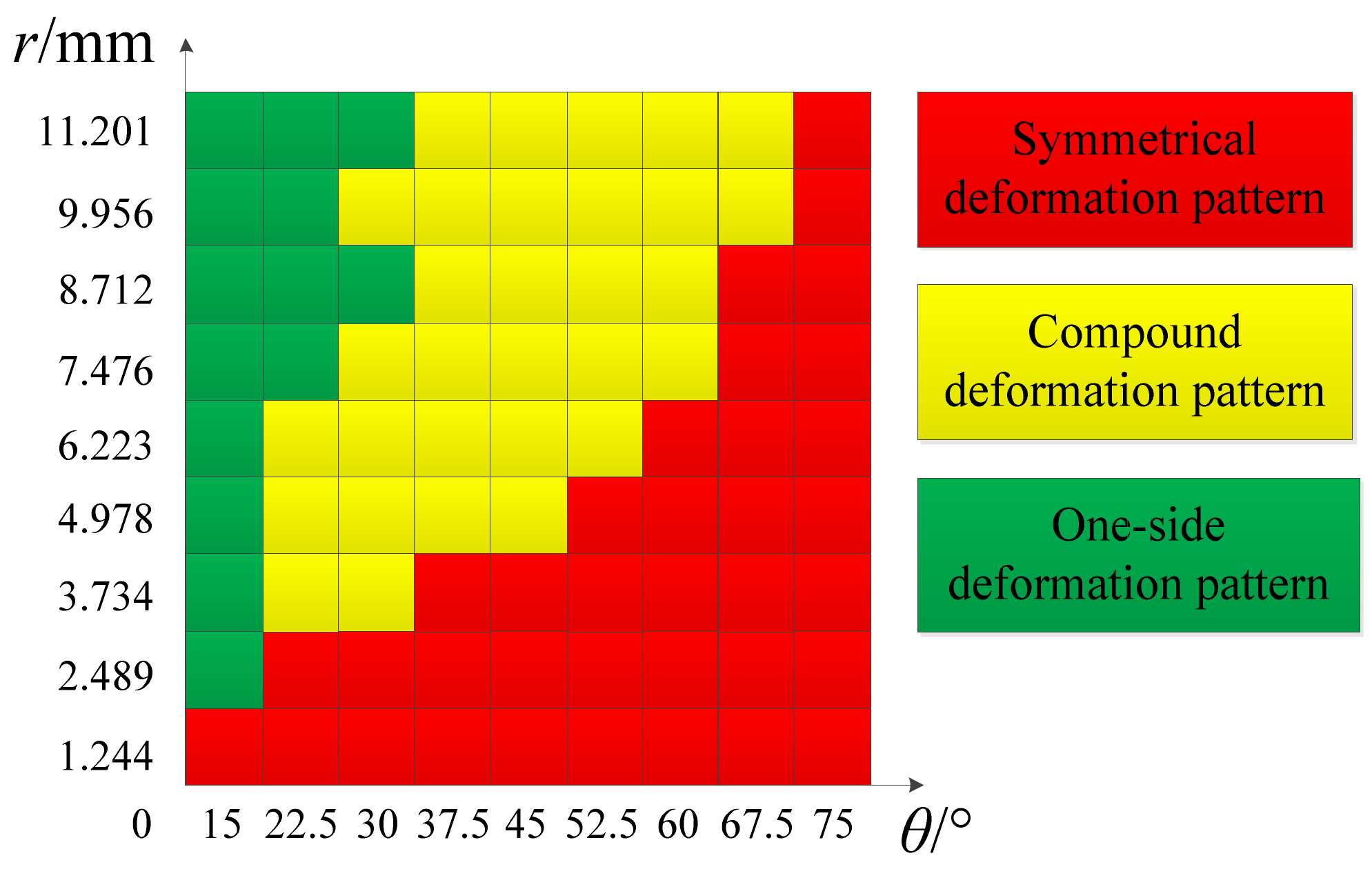
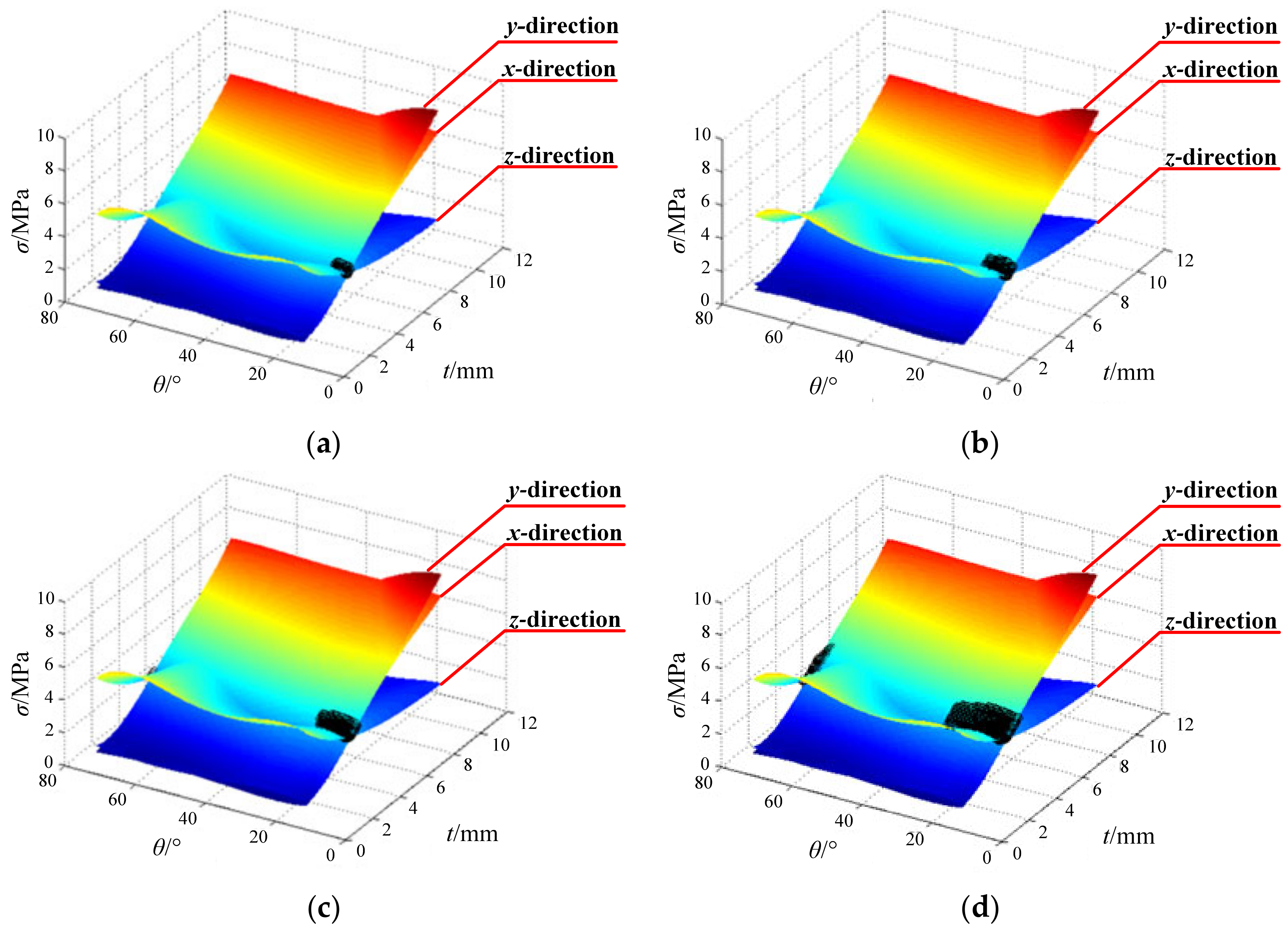
4. Approximate Isotropy Analysis of Origami Honeycomb
5. Experiment
5.1. Experimental Equipment and Specimen
5.2. Experimental Results
6. Results
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mc Farland, R.K. Hexagonal cell structures under post-buckling axial load. AIAA J. 1963, 1, 1380–1385. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Abramowicz, W. On the Crushing Mechanics of Thin-Walled Structures. J. Appl. Mech. 1983, 50, 727–734. [Google Scholar] [CrossRef]
- Tachi, T.; Miura, K. Rigid-Foldable Cylinders and Cells. J. Int. Assoc. Shell Spat. Struct. 2012, 53, 217–226. [Google Scholar]
- Chen, W.; Wierzbicki, T. Relative merits of single-cell, multi-cell and foam-filled thin-walled structures in energy absorption. Thin-Walled Struct. 2001, 39, 287–306. [Google Scholar] [CrossRef]
- Harris, J.; Winter, R.; McShane, G. Impact response of additively manufactured metallic hybrid lattice materials. Int. J. Impact Eng. 2017, 104, 177–191. [Google Scholar] [CrossRef]
- Slimane, S.; Kebdani, S.; Boudjemai, A. Effect of position of tension-loaded inserts on honeycomb panels used for space applications. Int. J. Interact. Des. Manuf. 2018, 12, 393–408. [Google Scholar] [CrossRef]
- Slimane, S.A.; Slimane, A.; Guelailia, A.; Boudjemai, A.; Kebdani, S.; Smahat, A.; Mouloud, D. Hypervelocity impact on honeycomb structure reinforced with bi-layer ceramic/aluminum facesheets used for spacecraft shielding. Mech. Adv. Mater. Struct. 2022, 29, 4487–4505. [Google Scholar] [CrossRef]
- Zheng, Z.; Yu, J.; Li, J. Dynamic crushing of 2D cellular structures: A finite element study. Int. J. Impact Eng. 2005, 32, 650–664. [Google Scholar] [CrossRef]
- Li, K.; Gao, X.-L.; Wang, J. Dynamic crushing behavior of honeycomb structures with irregular cell shapes and non-uniform cell wall thickness. Int. J. Solids Struct. 2007, 44, 5003–5026. [Google Scholar] [CrossRef]
- Zhang, J.; Ashby, M. The out-of-plane properties of honeycombs. Int. J. Mech. Sci. 1992, 34, 475–489. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, X.-C. The influence of cell micro-topology on the in-plane dynamic crushing of honeycombs. Int. J. Impact Eng. 2009, 36, 98–109. [Google Scholar] [CrossRef]
- Zhang, X.; Cheng, G.; Zhang, H. Theoretical prediction and numerical simulation of multi-cell square thin-walled structures. Thin-Walled Struct. 2006, 44, 1185–1191. [Google Scholar] [CrossRef]
- Wang, A.-J.; Kumar, R.S.; McDowell, D.L. Mechanical Behavior of Extruded Prismatic Cellular Metals. Mech. Adv. Mater. Struct. 2005, 12, 185–200. [Google Scholar] [CrossRef]
- Yu, J.; Zheng, Z. Dynamic crushing of 2D cellular metals: Microstructure effects and rate-sensitivity mechanisms. Acta Mech. Solida Sin. 2010, 23, 45–55. [Google Scholar]
- Zhang, Y.; He, N.; Song, X.; Chen, T.; Chen, H. On impacting mechanical behaviors of side fractal structures. Thin-Walled Struct. 2020, 146, 106490. [Google Scholar] [CrossRef]
- Neville, R.M.; Scarpa, F.; Pirrera, A. Shape morphing Kirigami mechanical metamaterials. Sci. Rep. 2016, 6, 31067. [Google Scholar] [CrossRef]
- Yu, X.; Zhou, J.; Liang, H.; Jiang, Z.; Wu, L. Mechanical metamaterials associated with stiffness, rigidity and compressibility: A brief review. Prog. Mater. Sci. 2018, 94, 114–173. [Google Scholar] [CrossRef]
- Xiao, D.; Dong, Z.; Li, Y.; Wu, W.; Fang, D. Compression behavior of the graded metallic auxetic reentrant honeycomb: Experiment and finite element analysis. Mater. Sci. Eng. A 2019, 758, 163–171. [Google Scholar] [CrossRef]
- Li, Y.; Chen, Z.; Xiao, D.; Wu, W.; Fang, D. The Dynamic response of shallow sandwich arch with auxetic metallic honeycomb core under localized impulsive loading. Int. J. Impact Eng. 2020, 137, 103442. [Google Scholar] [CrossRef]
- Silverberg, J.L.; Evans, A.A.; McLeod, L.; Hayward, R.C.; Hull, T.; Santangelo, C.D.; Cohen, I. Using origami design principles to fold reprogrammable mechanical metamaterials. Science 2014, 345, 647–650. [Google Scholar] [CrossRef]
- Nicolaou, Z.G.; Motter, A.E. Mechanical metamaterials with negative compressibility transitions. Nat. Mater. 2012, 11, 608–613. [Google Scholar] [CrossRef] [PubMed]
- Boatti, E.; Vasios, N.; Bertoldi, K. Origami Metamaterials for Tunable Thermal Expansion. Adv. Mater. 2017, 29, 1700360. [Google Scholar] [CrossRef] [PubMed]
- Overvelde, J.T.; de Jong, T.A.; Shevchenko, Y.; Becerra, S.A.; Whitesides, G.M.; Weaver, J.C.; Hoberman, C.; Bertoldi, K. A three-dimensional actuated origami-inspired transformable metamaterial with multiple degrees of freedom. Nat. Commun. 2016, 7, 10929. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Yang, Q.; Fang, R.; Chen, W.; Hao, H. Origami metamaterial with two-stage programmable compressive strength under quasi-static loading. Int. J. Mech. Sci. 2020, 189, 105987. [Google Scholar] [CrossRef]
- Kamrava, S.; Mousanezhad, D.; Ebrahimi, H.; Ghosh, R.; Vaziri, A. Origami-based cellular metamaterial with auxetic, bistable, and self-locking properties. Sci. Rep. 2017, 7, srep46046. [Google Scholar] [CrossRef]
- Tao, R.; Ji, L.; Li, Y.; Wan, Z.; Hu, W.; Wu, W.; Liao, B.; Ma, L.; Fang, D. 4D printed origami metamaterials with tunable compression twist behavior and stress-strain curves. Compos. Part B Eng. 2020, 201, 108344. [Google Scholar] [CrossRef]
- Yang, K.; Xu, S.; Zhou, S.; Shen, J.; Xie, Y.M. Design of dimpled tubular structures for energy absorption. Thin-Walled Struct. 2017, 112, 31–40. [Google Scholar] [CrossRef]
- Yang, K.; Xu, S.; Shen, J.; Zhou, S.; Xie, Y.M. Energy absorption of thin-walled tubes with pre-folded origami patterns: Numerical simulation and experimental verification. Thin-Walled Struct. 2016, 103, 33–44. [Google Scholar] [CrossRef]
- Song, J.; Chen, Y.; Lu, G. Axial crushing of thin-walled structures with origami patterns. Thin-Walled Struct. 2012, 54, 65–71. [Google Scholar] [CrossRef]
- Zhang, X.; Cheng, G.; You, Z.; Zhang, H. Energy absorption of axially compressed thin-walled square tubes with patterns. Thin-Walled Struct. 2007, 45, 737–746. [Google Scholar] [CrossRef]
- Ma, J.; You, Z. Energy absorption of thin-walled beams with a pre-folded origami pattern. Thin-Walled Struct. 2013, 73, 198–206. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, H. Experimental and numerical investigation on crush resistance of polygonal columns and angle elements. Thin-Walled Struct. 2012, 57, 25–36. [Google Scholar] [CrossRef]
- Ma, J.; Le, Y.; You, Z. Axial Crushing Tests of Steel Thin-Walled Square Tubes with Pyramid Pattern. In Proceedings of the 51st AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Orlando, FL, USA, 12–15 April 2010. [Google Scholar]
- Ma, J.; You, Z. Energy Absorption of Thin-Walled Square Tubes with a Prefolded Origami Pattern—Part I: Geometry and Numerical Simulation. J. Appl. Mech. 2013, 81, 011003. [Google Scholar] [CrossRef]
- You, Z.; Williams, M. Thin-Walled Tubes with Pre-Folded Origami Patterns as Energy Absorption Devices; University of Oxford: Oxford, UK, 2011. [Google Scholar]
- Aranda-Ruiz, J. Analysis of Specific Energy Absorption in Multi-layered Origami Plates. In Proceedings of the 1st Virtual Iberian Conference on Structural Integrity, Virtual, 16 January 2020. [Google Scholar]
- Schenk, M.; Guest, S.D. Geometry of Miura-folded metamaterials. Proc. Natl. Acad. Sci. USA 2013, 110, 3276–3281. [Google Scholar] [CrossRef]
- Harris, J.; McShane, G. Impact response of metallic stacked origami cellular materials. Int. J. Impact Eng. 2021, 147, 103730. [Google Scholar] [CrossRef]
- Xiang, X.; Qiang, W.; Hou, B.; Tran, P.; Lu, G. Quasi-static and dynamic mechanical properties of Miura-ori metamaterials. Thin-Walled Struct. 2020, 157, 106993. [Google Scholar] [CrossRef]
- Yuan, L.; Dai, H.; Song, J.; Ma, J.; Chen, Y. The behavior of a functionally graded origami structure subjected to quasi-static compression. Mater. Des. 2020, 189, 108494. [Google Scholar] [CrossRef]
- Zhang, J.; Karagiozova, D.; You, Z.; Chen, Y.; Lu, G. Quasi-static large deformation compressive behaviour of origami-based metamaterials. Int. J. Mech. Sci. 2019, 153, 194–207. [Google Scholar] [CrossRef]
- Karagiozova, D.; Zhang, J.; Lu, G.; You, Z. Dynamic in-plane compression of Miura-ori patterned metamaterials. Int. J. Impact Eng. 2019, 129, 80–100. [Google Scholar] [CrossRef]
- Ma, J.; Song, J.; Chen, Y. An origami-inspired structure with graded stiffness. Int. J. Mech. Sci. 2017, 136, 134–142. [Google Scholar] [CrossRef]
- Zhai, J.; Liu, Y.; Geng, X.; Zheng, W.; Zhao, Z.; Cui, C.; Li, M. Energy absorption of pre-folded honeycomb under in-plane dynamic loading. Thin-Walled Struct. 2019, 145, 106356. [Google Scholar] [CrossRef]
- Li, M.; Liu, R.Q.; Guo, H.W.; Deng, Z.Q. A Study on Out-of-Plane Compressive Properties of Metal Honeycombs by Numerical Simulation. Adv. Mater. Res. 2011, 217, 723–727. [Google Scholar] [CrossRef]
- Zhai, J.; Zhang, D.; Li, M.; Cui, C.; Cai, J. Out-of-plane energy absorption and crush behavior of origami honeycomb. Thin-Walled Struct. 2022, 181, 109966. [Google Scholar] [CrossRef]
- Wei, W.; Zhang, F.; Xing, Y.; Wang, H.; Liu, R. Research on Mechanical Properties of Origami Aluminum Honeycomb for Automobile Energy Absorbing Box. Materials 2023, 16, 141. [Google Scholar] [CrossRef]
- Fang, H.; Rais-Rohani, M.; Liu, Z.; Horstemeyer, M. A comparative study of metamodeling methods for multiobjective crashworthiness optimization. Comput. Struct. 2005, 83, 2121–2136. [Google Scholar] [CrossRef]
- Lindman, H.R. Analysis of Variance in Experimental Design; Springer: New York, NY, USA, 1992. [Google Scholar]






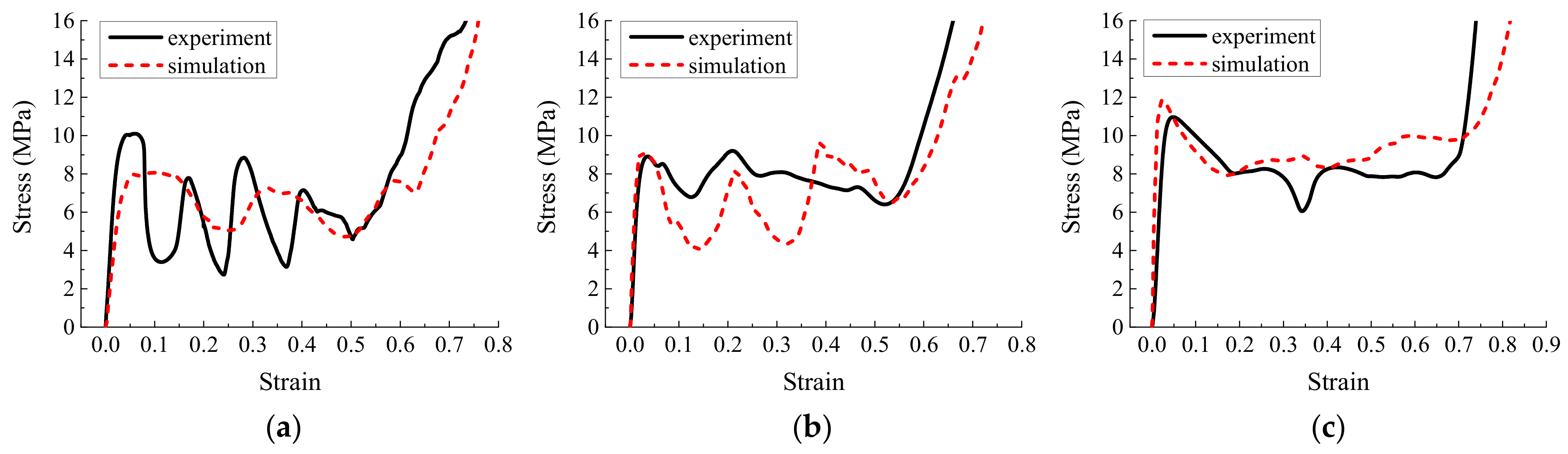
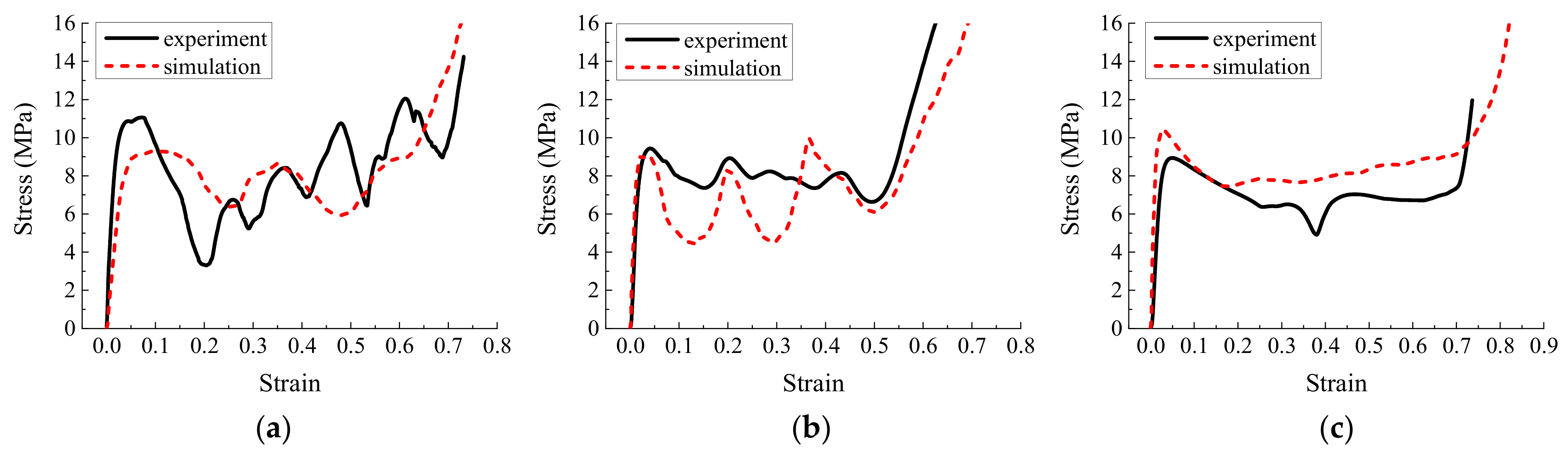
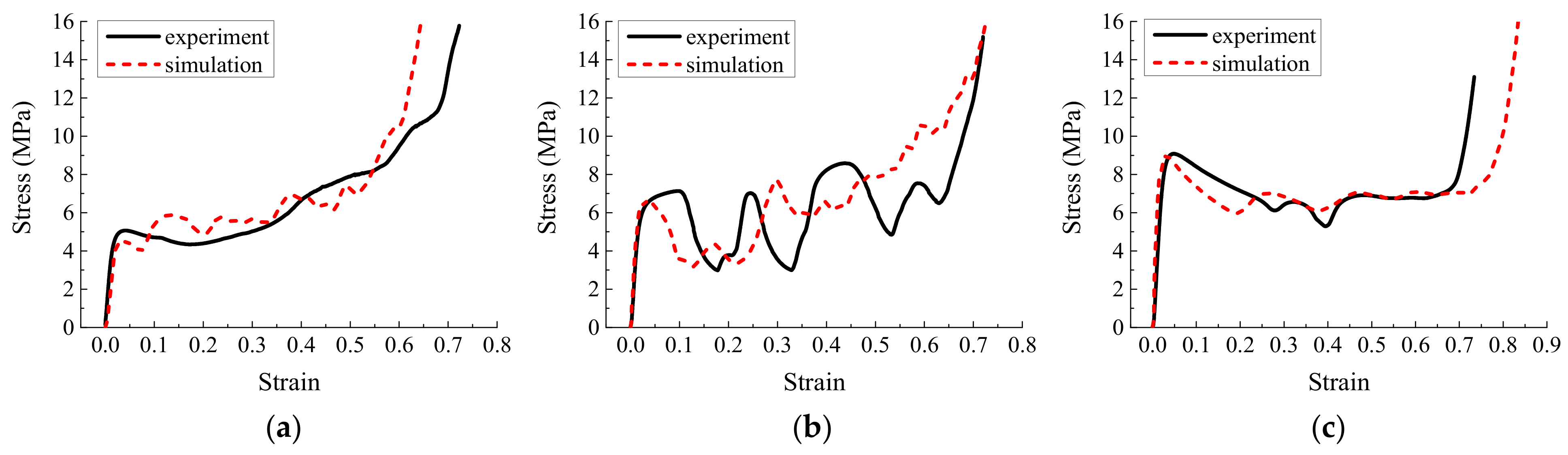
| Model | H | r/mm | σy/MPa | Relative Value | σx/MPa | Relative Value | σz/MPa | Relative Value |
|---|---|---|---|---|---|---|---|---|
| 0-1 | 0 | 0.488 | 1 | 0.582 | 1 | 6.784 | 1 | |
| 1-1 | 2H = 2L | 6.22254 | 1.540 | 3.16 | 2.229 | 3.83 | 2.458 | 0.36 |
| 1-2 | 2H = 2L | 11.20057 | 2.867 | 5.88 | 3.462 | 5.95 | 1.436 | 0.21 |
| 2-1 | 2H = 1.5L | 6.22254 | 2.173 | 4.45 | 2.895 | 4.97 | 2.128 | 0.31 |
| 2-2 | 2H = 1.5L | 11.20057 | 4.193 | 8.59 | 4.675 | 8.03 | 1.247 | 0.18 |
| 3-1 | 2H = L | 6.22254 | 3.356 | 6.88 | 4.705 | 8.08 | 1.927 | 0.28 |
| 3-2 | 2H = L | 11.20057 | 7.389 | 15.14 | 6.963 | 11.96 | 1.226 | 0.18 |
| Model | H | r/mm | Simulation Value | RSM Approximate Solution | ||||
|---|---|---|---|---|---|---|---|---|
| σy/MPa | σx/MPa | σz/MPa | σry/MPa | σrx/MPa | σrz/MPa | |||
| 1 | 1.244508 | π/12 | 0.672 | 0.813 | 4.746 | 0.699 | 0.713 | 4.710 |
| 2 | 1.244508 | π/6 | 0.613 | 0.771 | 4.664 | 0.505 | 0.927 | 4.773 |
| 3 | 1.244508 | π/4 | 0.568 | 0.727 | 4.768 | 0.623 | 0.689 | 4.575 |
| 4 | 1.244508 | π/3 | 0.557 | 0.698 | 5.184 | 0.627 | 0.564 | 5.384 |
| 5 | 1.244508 | 5π/12 | 0.561 | 0.675 | 4.805 | 0.517 | 0.790 | 4.725 |
| 6 | 3.733524 | π/12 | 2.280 | 1.981 | 2.864 | 2.322 | 2.142 | 2.892 |
| 7 | 3.733524 | π/6 | 1.686 | 2.451 | 2.841 | 1.711 | 2.385 | 2.890 |
| 8 | 3.733524 | π/4 | 1.189 | 2.050 | 2.993 | 1.191 | 2.106 | 2.877 |
| 9 | 3.733524 | π/3 | 0.978 | 1.759 | 3.826 | 0.875 | 1.762 | 3.808 |
| 10 | 3.733524 | 5π/12 | 1.270 | 1.635 | 2.838 | 1.304 | 1.482 | 2.895 |
| 11 | 6.22254 | π/12 | 4.277 | 4.330 | 2.094 | 4.215 | 4.214 | 2.051 |
| 12 | 6.22254 | π/6 | 3.356 | 4.705 | 1.927 | 3.345 | 4.418 | 1.953 |
| 13 | 6.22254 | π/4 | 2.216 | 4.041 | 2.067 | 2.277 | 4.151 | 2.123 |
| 14 | 6.22254 | π/3 | 1.723 | 3.495 | 3.230 | 1.666 | 3.762 | 3.198 |
| 15 | 6.22254 | 5π/12 | 2.518 | 3.248 | 2.085 | 2.588 | 3.274 | 2.078 |
| 16 | 8.711556 | π/12 | 6.282 | 5.576 | 1.679 | 6.231 | 5.728 | 1.674 |
| 17 | 8.711556 | π/6 | 5.094 | 5.702 | 1.481 | 5.235 | 5.848 | 1.439 |
| 18 | 8.711556 | π/4 | 3.678 | 5.909 | 1.652 | 3.686 | 5.670 | 1.773 |
| 19 | 8.711556 | π/3 | 2.871 | 5.443 | 3.090 | 2.776 | 5.434 | 3.004 |
| 20 | 8.711556 | 5π/12 | 4.126 | 5.105 | 1.701 | 4.121 | 5.055 | 1.714 |
| 21 | 11.20057 | π/12 | 8.313 | 7.094 | 1.456 | 8.356 | 6.999 | 1.511 |
| 22 | 11.20057 | π/6 | 7.389 | 6.963 | 1.226 | 7.342 | 7.013 | 1.084 |
| 23 | 11.20057 | π/4 | 5.477 | 6.910 | 1.424 | 5.351 | 7.020 | 1.556 |
| 24 | 11.20057 | π/3 | 3.930 | 7.285 | 3.002 | 4.115 | 7.157 | 2.939 |
| 25 | 11.20057 | 5π/12 | 5.842 | 7.167 | 1.486 | 5.788 | 7.230 | 1.503 |
| RMSE | |||
|---|---|---|---|
| σy | 0.1251 | 0.9989 | 0.9973 |
| σx | 0.2240 | 0.9967 | 0.9920 |
| σz | 0.1462 | 0.9949 | 0.9878 |
| Model | r/mm | θ/rad | Approximate Solution | k | ||
|---|---|---|---|---|---|---|
| σry/MPa | σrx/MPa | σrz/MPa | ||||
| 1 | 3.9 | 1/9π | 2.22 | 2.46 | 3.02 | 0.27 |
| 2 | 4.0 | 4/45π | 2.79 | 2.59 | 4.00 | 0.35 |
| 3 | 4.2 | 1/9π | 2.43 | 2.72 | 2.88 | 0.16 |
| 4 | 4.3 | 1/6π | 2.05 | 2.86 | 2.62 | 0.28 |
| 5 | 4.4 | 2/15π | 2.39 | 2.95 | 2.85 | 0.19 |
| 6 | 4.5 | 1/9π | 2.80 | 3.35 | 3.61 | 0.23 |
| 7 | 4.6 | 1/8π | 2.60 | 3.11 | 2.69 | 0.16 |
| 8 | 4.7 | 1/10π | 2.89 | 3.09 | 2.63 | 0.15 |
| 9 | 4.8 | 1/9π | 2.87 | 3.23 | 2.63 | 0.19 |
| 10 | 5 | 5/12π | 2.74 | 2.68 | 3.20 | 0.16 |
| Model | r/mm | θ/rad | nx | ny | nz | H/mm | L/mm | t/mm |
|---|---|---|---|---|---|---|---|---|
| Specimen 1 | 4 | 4/45π | 3 | 4 | 4 | 4.4 | 8.8 | 0.5 |
| Specimen 2 | 4.5 | 1/9π | 3 | 4 | 4 | 4.4 | 8.8 | 0.5 |
| Specimen 3 | 5 | 5/12π | 3 | 4 | 4 | 4.4 | 8.8 | 0.5 |
| σry/MPa | σrx/MPa | σrz/MPa | k | ||
|---|---|---|---|---|---|
| Specimen 1 | Simulation value | 6.41 | 6.21 | 8.92 | 0.30 |
| Experimental value | 7.34 | 5.74 | 8.25 | 0.30 | |
| Relative error (%) | −14.51 | 7.57 | 7.51 | ||
| Specimen 2 | Simulation value | 6.63 | 7.78 | 8.32 | 0.21 |
| Experimental value | 7.41 | 8.26 | 7.13 | 0.14 | |
| Relative error (%) | −11.76 | −6.17 | 14.30 | ||
| Specimen 3 | Simulation value | 6.1 | 5.96 | 6.85 | 0.13 |
| Experimental value | 6.01 | 5.71 | 6.96 | 0.18 | |
| Relative error (%) | 1.48 | 4.19 | −1.61 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhai, J.; Zhang, D.; Li, M.; Cui, C.; Cai, J. An Approximately Isotropic Origami Honeycomb Structure and Its Energy Absorption Behaviors. Materials 2023, 16, 1571. https://doi.org/10.3390/ma16041571
Zhai J, Zhang D, Li M, Cui C, Cai J. An Approximately Isotropic Origami Honeycomb Structure and Its Energy Absorption Behaviors. Materials. 2023; 16(4):1571. https://doi.org/10.3390/ma16041571
Chicago/Turabian StyleZhai, Jiayue, Dingguo Zhang, Meng Li, Chengbo Cui, and Jianguo Cai. 2023. "An Approximately Isotropic Origami Honeycomb Structure and Its Energy Absorption Behaviors" Materials 16, no. 4: 1571. https://doi.org/10.3390/ma16041571
APA StyleZhai, J., Zhang, D., Li, M., Cui, C., & Cai, J. (2023). An Approximately Isotropic Origami Honeycomb Structure and Its Energy Absorption Behaviors. Materials, 16(4), 1571. https://doi.org/10.3390/ma16041571





