1. Introduction
Currently, the growing consumption and the increase in the number of individual shipments make it necessary to ensure the safe storage and transport of goods. At the same time, more emphasis is placed on ecology, which is why companies quickly began to abandon plastic, which not only harms the environment, but also has a negative impact on the reputation of enterprises. For these reasons, corrugated cardboard has begun to conquer the packaging market. It owes its huge popularity to numerous advantages that largely meet the needs of today. Cardboard packaging is recyclable, easy to dispose of, biodegradable, durable under appropriate conditions, and takes up little space before folding. In addition to the positives for the environment, there are also useful properties for companies. Corrugated cardboard products can be easily shaped by adding holes, ventilation holes, or by printing a brand logo on them. It is also possible to create boxes with perforations, which are often used in the food industry. Such packages are opened by tearing off a part of the material along the previously designed perforations, and then immediately put on the shelf. Such a procedure allows for significant time savings, which brings profits for large companies.
In addition to all the above-mentioned advantages, packaging should, above all, effectively protect the goods. For this reason, the load-bearing capacity of packaging has been studied by scientists since the 1950s. The first methods were analytical methods determined for boxes with a rectangular base. In 1952, Kellicutt and Landt introduced an approach based on box perimeter, overall ring crush strength, and paper and box constants [
1]. Four years later, Maltenfort presented the box compressive strength depending on the critical force, paper parameters, box dimensions, and empirical constants [
2]. In 1963, McKee et al. proposed a relationship that has been commonly used in the packaging industry for many years to estimate the compressive strength of cardboard boxes [
3]. The popular McKee formula is based on the edge crush resistance (ECT) value, flexural stiffnesses, box perimeter, and correction factors. Due to the simplicity of the formula, it is possible to obtain a result quickly, but it can only be used for simple standard boxes. In the following years, scientists tried to extend and modify the McKee formula. In 1985, Allerby et al. changed the constants and exponents [
4] and, in 1987, Schrampfer et al. extended the approach to new cardboard cutting methods and equipment [
5]. In 1993, Batelka and Smith included the dimensions of the packaging in the formula [
6].
In practice, the compressive strength of the packaging depends on many factors related to the construction and storage conditions [
7]. From the construction point of view, attention should be paid to aspects, such as openings, ventilation holes, perforations, and offsets [
8,
9,
10,
11,
12], because they significantly affect the behavior of the box under pressure. Load-bearing capacity is also influenced by moisture content [
13,
14], stacking load [
15], storage time and conditions [
16], and many other factors.
With the development of technology, numerical computations have become popular. The well-known finite element method (FEM) is often used to determine the compressive strength of packaging. Urbanik and Frank compared the compressive strength of boxes calculated using FEM with the results of the McKee formula extended by Poisson’s ratio [
17]. Furthermore, FEM simulations were used by Nordstrand and Carlsson to compare analytical, experimental, and numerical values of transverse shear moduli [
18,
19]. The issue of transverse shear was studied by Aviles et al. [
20] and Garbowski et al. [
21,
22], where the role of transversal shear stiffness in orthotropic sandwich material is presented. Urbanik and Saliklis used FEM to observe buckling and post-buckling phenomena in cardboard boxes [
23]. Maneengam et al. used the FE model to study the vibration and damping characteristics of honeycomb sandwich structures reinforced with carbon nanotubes [
24]. Corrugated board reinforced with metal liners has also been investigated by Gu et al., where uniaxial compression tests were conducted to analyze the damage and failure modes [
25]. Sohrabpour and Hellström presented a review of analytical and numerical methods for estimating box compressive strength [
26].
Due to the orthotropy of the paper and layered structure, corrugated board is quite difficult to analyze numerically. To facilitate the computations, the homogenization process is used, which consists of replacing a complicated cardboard cross-section with a homogeneous board with equivalent parameters. Homogenization methods can be divided into analytical and numerical. In analytical approaches, the equations of the classical theory of material strength and the classical theory of laminates are used [
27]. Numerical homogenization is based on a finite element method framework. In this study, a method based on strain energy equivalence between a 3D cardboard model and a flat plate is used. This homogenization procedure was introduced by Biancolini [
28] and later extended by Garbowski and Gajewski to include transversal shear stiffnesses [
29]. In 2003, Hohe presented a representative element of heterogeneous and homogenized structures derived from the strain energy equations [
30]. Homogenization was also used to determine substitute panel parameters, such as membrane and bending characteristics [
31] and torsional stiffness [
32]. In 2018, a multiscale asymptotic homogenization was used by Ramírez-Torres et al. for layered hierarchical structure analysis [
33,
34]. Suarez et al. used numerical homogenization to analyze seating made of multi-wall corrugated cardboard [
35]. Garbowski et al. presented the influence of different creasing and perforation on cardboard parameters [
36]. In 2022, Mrówczyński et al. presented non-local sensitivity analysis in the optimal design of three- [
37] and five-layered [
38] corrugated cardboard. A review of homogenization methods and their accuracy was made by Nguyen-Minh et al., based on analysis of corrugated panels [
39].
During the production process, the corrugated board may be deformed due to changes in temperature and humidity. Two types of emerging imperfections can be distinguished, namely global and local. A model describing systematic, large-scale deviation from the intended flat shape of cardboard was presented by Beck and Fischerauer [
40]. However, more attention was paid to local imperfections. In 1995, Nordstrand presented the effect of the size of imperfections on the compressive strength of cardboard boxes [
41], and in 2004 extended the nonlinear buckling analysis of Rhodes and Harvey orthotropic plates to include local imperfections [
42]. Lu et al. investigated the effect of imperfections on the mechanical properties of cardboard during compression [
43]. The problem of non-ideal shape during bending of double-walled corrugated cardboard was analyzed analytically by Garbowski and Knitter-Piątkowska [
44]. Mrówczyński et al. presented a method of including the initial imperfections in the analysis of single-walled cardboard [
45]. Recently Cillie and Coetzee presented an experimental and numerical study on the in-plane compression of corrugated paperboard panels with global and local imperfections [
46].
In the article, the authors focused on the issue of local imperfections in double-walled corrugated board. The method presented here allows us to quickly and easily obtain the effective cardboard stiffnesses reduction due to the imperfect shape of its component layers. The described approach is an extension of the homogenization method proposed in the authors’ previous works. Due to the specificity of the production process and very thin layers of paper, cardboard always has some imperfections, so it is important to be able to include this aspect in determining its mechanical parameters. The approach proposed in this paper extends the discussion carried out in our previous work [
45], where the influence of imperfections on the stiffness of three-ply cardboard was investigated. The mechanics of both types differ significantly and, therefore, in this work other techniques, adequate to solve this problem, were used. The extension consists of taking into account the influence of the geometrical imperfections of individual layers on the calculated cross-section’s effective stiffness. These imperfections were taken into account in the model by appropriate modeling of geometrical imperfections, which were determined in a priori buckling analysis and were appropriately included using the innovative technique based on numerical homogenization of double-walled corrugated board. Thanks to this approach, it is possible not only to quickly obtain homogenized stiffnesses data for complex cross-sections, but also to easily take into account the effects of geometrical imperfections.
3. Results
The first step was to properly create the corrugated cardboard RVE model in FE software. In the cases of samples with imperfections, the geometry of the liners was changed and replaced with the correct buckling shape (see
Figure 3). Then, the material parameters contained in
Table 1 were assigned to both liners and waves. Following the homogenization procedure described in
Section 2.3, stiffness matrices were determined for all analyzed cases. In
Table 3, an example of the stiffness matrix
is presented for a model without imperfections and with 0% offset. No buckling mode is assigned to this case, because the imperfection value is equal to zero; therefore, it was marked with the XX-0-00 symbol.
As expected, non-zero components of the
matrix appeared in the stiffness matrix
, which results from the asymmetry of the double-walled corrugated board sample.
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10 show the main diagonal components of the stiffness matrix for all analyzed cases. The components
are omitted, but this does not create an error in the analysis because these elements are related to the components
and
in each stiffness matrix. The following tables do not list the elements of matrix
, but its effect on matrix
has been taken into account by applying Equation (13). The components of the
matrix were also omitted. The data contained in
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10 are also presented in the form of graphs in
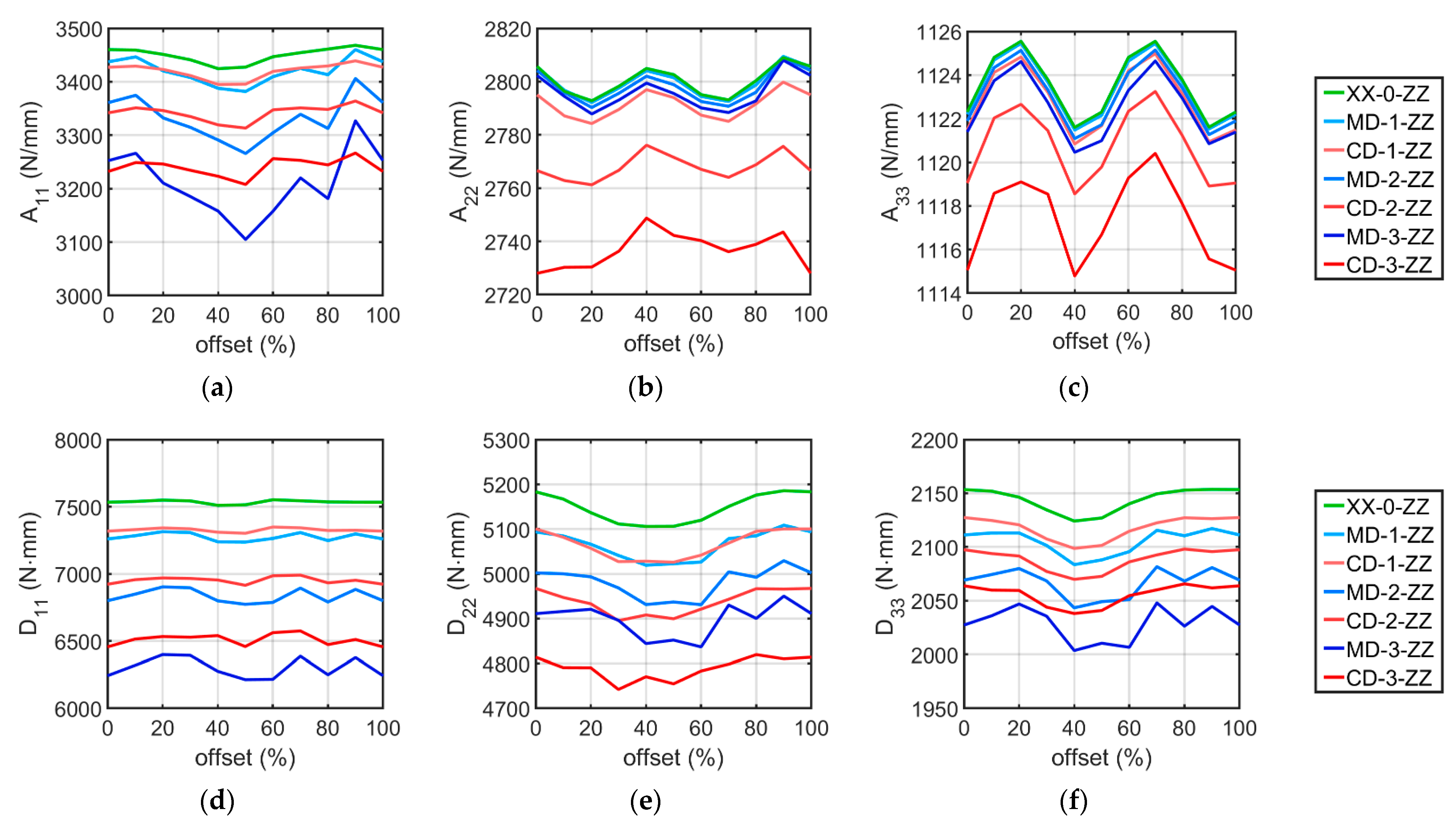
Figure 7.
For each shape and level of imperfection, the average stiffness values from all offset cases were calculated. Then, the obtained values for models with imperfections were compared to the average stiffnesses of perfectly shaped cardboard. In
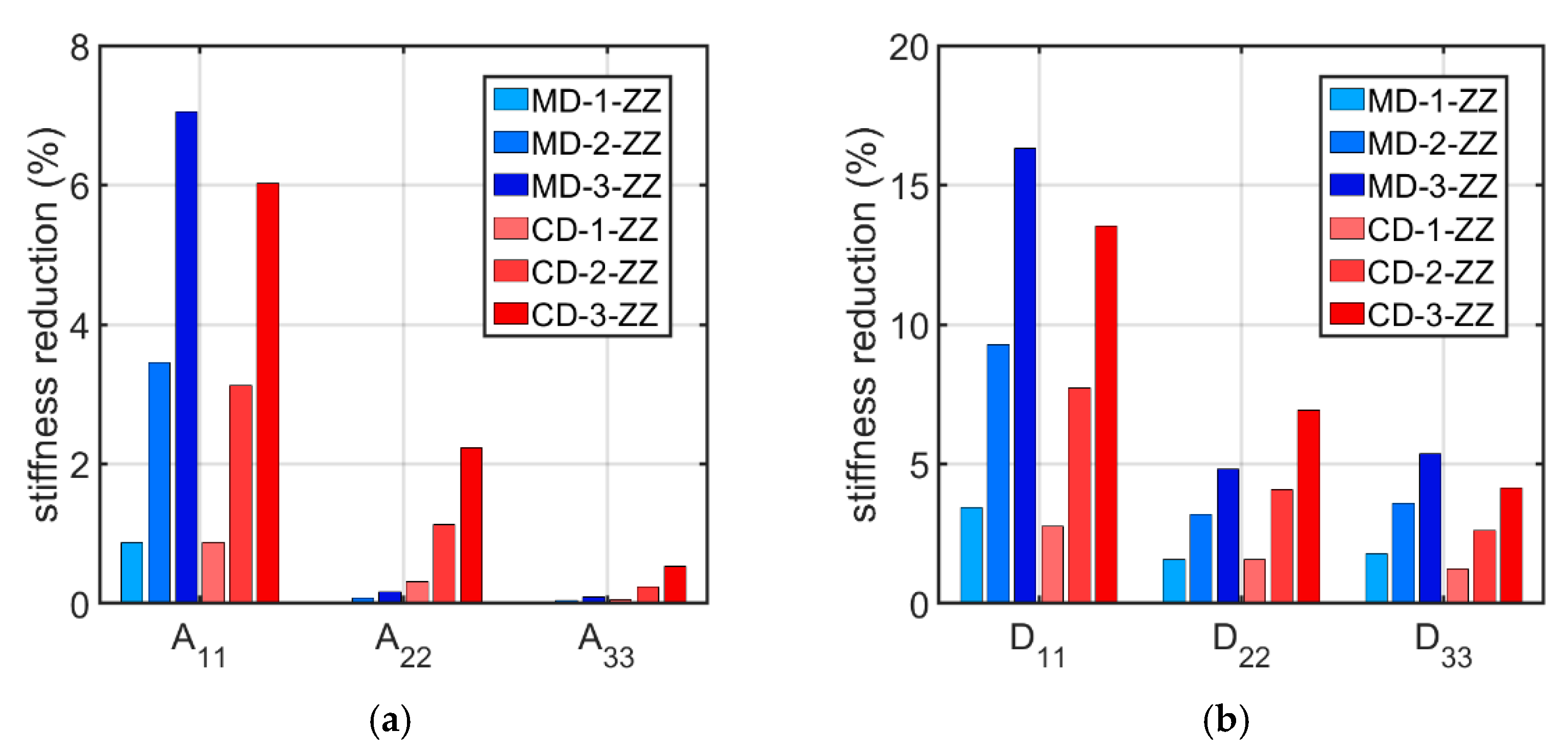
Figure 8, the tensile and bending stiffness reduction due to geometrical imperfections is shown.
As can be seen in
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10 and
Figure 7, the stiffnesses vary depending on the offset. Considering each set of shape and level of imperfection, the difference between the maximum and minimum stiffness values was calculated. Then, the obtained difference was related to the average stiffness obtained from all 10 offset cases, obtaining a percentage relative spread. In
Table 11, the relative spread for all analyzed sets of imperfection is presented.
4. Discussion
All crucial results have been shown in
Table 3,
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9,
Table 10 and
Table 11 and
Figure 7 and
Figure 8. In
Table 3, an example of a stiffness matrix determined for the XX-0-00 model is presented, in which the submatrices
,
,
, and
are distinguished. The
matrix contains non-zero components because the XX-0-00 case cross-section, as in all other models, is asymmetric. For this reason, Equation (13) was used in all corrugated board cases to calculate the uncoupled matrix
, whose values are gathered in
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10. Comparing the stiffness values
,
, and
from
Table 3 and
Table 4, it can be seen that the values in
Table 4 are smaller, which results from the uncoupling of the
matrix.
Considering
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10 and
Figure 7, where the stiffness results for all models are presented, it can be seen that as the level of imperfection increases, the cardboard stiffness decreases, as expected. The line diagrams also show that depending on the applied wave offset, the stiffnesses change their value. This is shown in more detail in
Table 11, which shows the relative spread of the extreme stiffnesses. The greatest fluctuations occur for the
stiffnesses, are slightly smaller for all bending stiffnesses, and are negligibly small for the
and
stiffnesses. In most cases, it is clear that the relative spread increases as the amount of imperfection increases. In addition, the data in this table show that the stiffnesses of the models with the MD imperfection shape are more sensitive to offset changes than the cases with the CD buckling mode.
Based on
Table 4,
Table 5,
Table 6,
Table 7,
Table 8,
Table 9 and
Table 10 and
Figure 7 and
Figure 8, it can be concluded that geometric imperfections have a huge influence on the bending stiffness
. Large decreases were also noted for the stiffnesses
,
, and
. The effect of imperfections on the stiffness
is small, and the effect is negligible on the
stiffness. These results are very similar to those obtained from the analysis of the effect of imperfections on the stiffness of single-walled corrugated board conducted by Mrówczyński et al. [
45], where the largest decreases were also obtained for
, and the smallest were obtained for
. The implementation of the MD buckling mode results in a greater average reduction in stiffnesses
,
, and
compared to the CD imperfection shape, for which a higher average reduction occur for the stiffnesses of
,
, and
. These differences are not that significant and amount to a maximum of 2.75 % (for the stiffness
between the MD-3-ZZ and CD-3-ZZ models).
To simplify computations and save computational time, it is desirable to select one case that should be computed as representative instead of carrying out an entire numerical study. To determine this, several observations from the conducted analyses should be considered. The imperfection shape of the compression in the CD has smaller relative spread values than the buckling mode of compression in the MD, so the change in the offset does not affect the stiffnesses so significantly. In addition, considering the MD shape does not significantly reduce the stiffnesses
and
, which seems to be an unfavorable effect. Based on the calculations, it can be concluded that a reasonable solution is to choose the CD imperfection shape as a representative model. Due to the quite small relative spread, the choice of a representative offset value is not so important, so its value can be chosen arbitrarily. The conclusions drawn from the presented numerical study are consistent with the work of Mrówczyński et al. [
45], in which the authors showed that the CD imperfection shape is also the most representative buckling mode for single-walled corrugated cardboard.
All the observations discussed above prove that there is a direct influence of imperfections on the stiffnesses
A,
B, and
D. However, the basic question arises whether it is possible to verify the presented theoretical approach with experimental data. Although testing corrugated board is not very laborious or particularly expensive, nevertheless validating the theoretical analysis presented here is not an easy task, because, firstly, it is practically impossible to produce corrugated board with a given level of imperfection and, secondly, it is also very laborious to check the actual level of imperfection in the cardboard produced. Nevertheless, below, we present a comparison (validation) of the results obtained using the method presented here and the method presented in paper [
44] in comparison with the results of laboratory tests conducted by Czechowski et al. and presented in paper [
48].
All experimental details are described in [
48], while analytical assumptions, in which imperfections are also included, are given in [
44]. Here, only the results of the experimental campaign (namely, results of 4-point bending tests of various asymmetric double-walled boards) are shown together with the results obtained using numerical and analytical models. All these results are presented in
Table 12, together with the results obtained using the methods presented here with the same amount of imperfection of flat layers as assumed in [
44]. It is worth noting that only one of all stiffnesses is checked, namely the bending stiffness in MD. The obtained results do not differ by more than 5% from the results obtained using the analytical model, while the average absolute error (compared to the experimental data) was 7.5%.