A Finite/Spectral Element Hybrid Method for Modeling and Band-Gap Characterization of Metamaterial Sandwich Plates
Abstract
1. Introduction
- (1)
- The first one is the innovation of the research method, namely the FE-SEHM, developed for the structural modeling and band-gap characteristics analysis of metamaterial plates.
- (2)
- The second one is that an elastic metamaterial sandwich plate with the axially deformed Timoshenko beam cores, considering both the out-of-plane and in-plane deformations of the face plates, is designed and investigated.
- (3)
- The third one is that the metamaterial plate has flexural vibration band-gaps appearing near the flexural vibration natural frequencies of the beams, which uncouple with the axial vibration band-gaps.
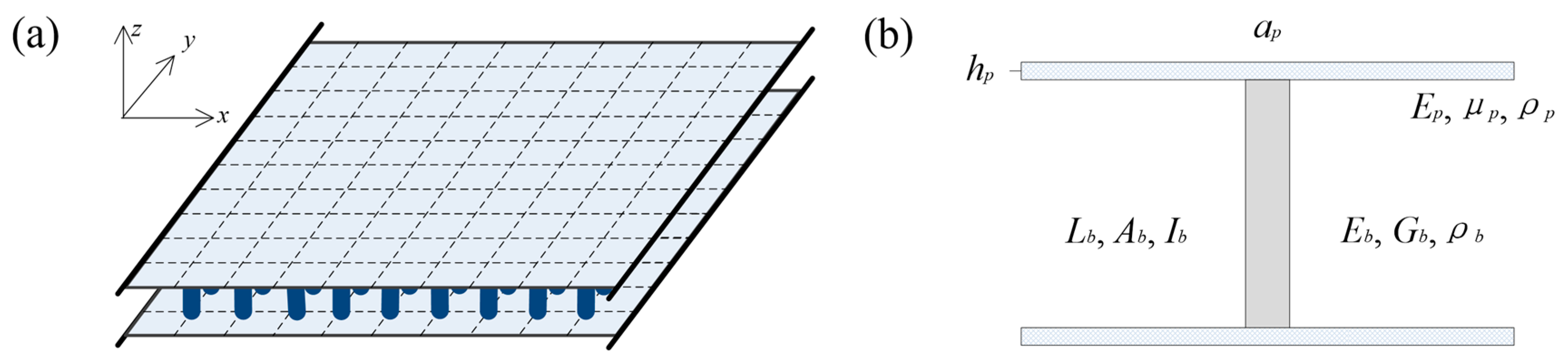
2. Derivation of the Dynamic Stiffness Matrix
2.1. Spectral Element Formulation of the Beam with Axial Deformation
2.2. Finite Element Formulation of Thin Plates
2.3. Dynamic Stiffness Matrix of the Metamaterial Sandwich Plate
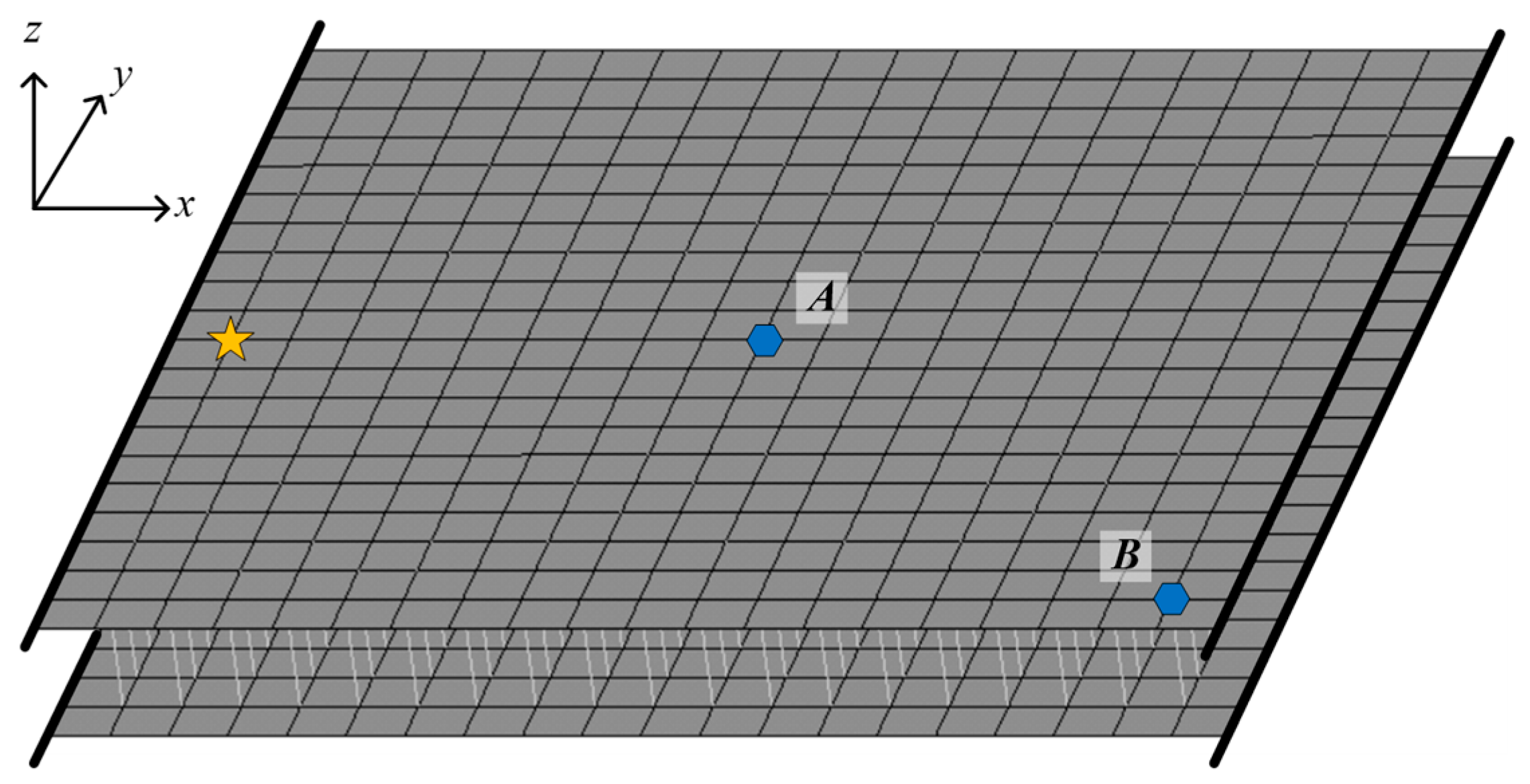
3. Numerical Results and Discussions
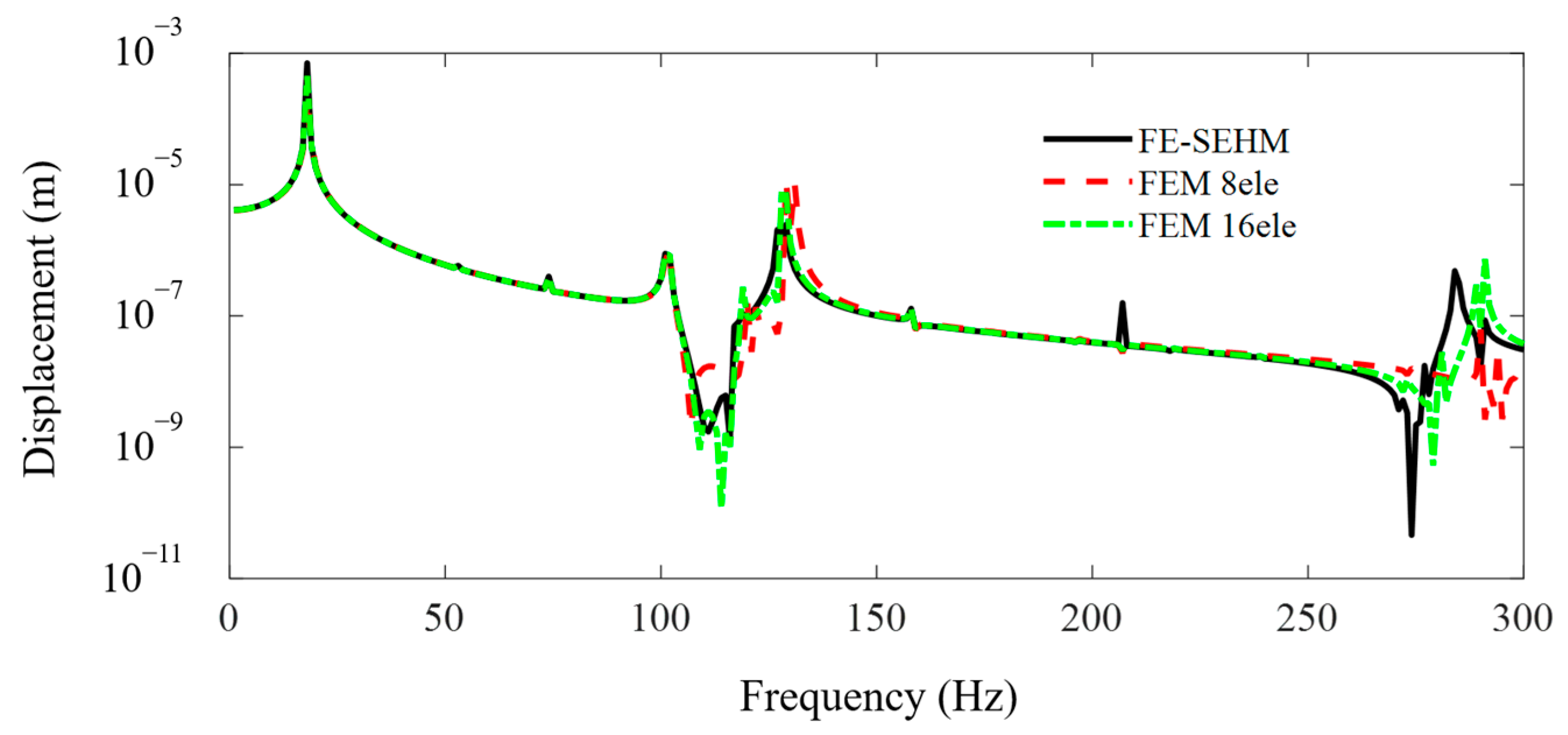
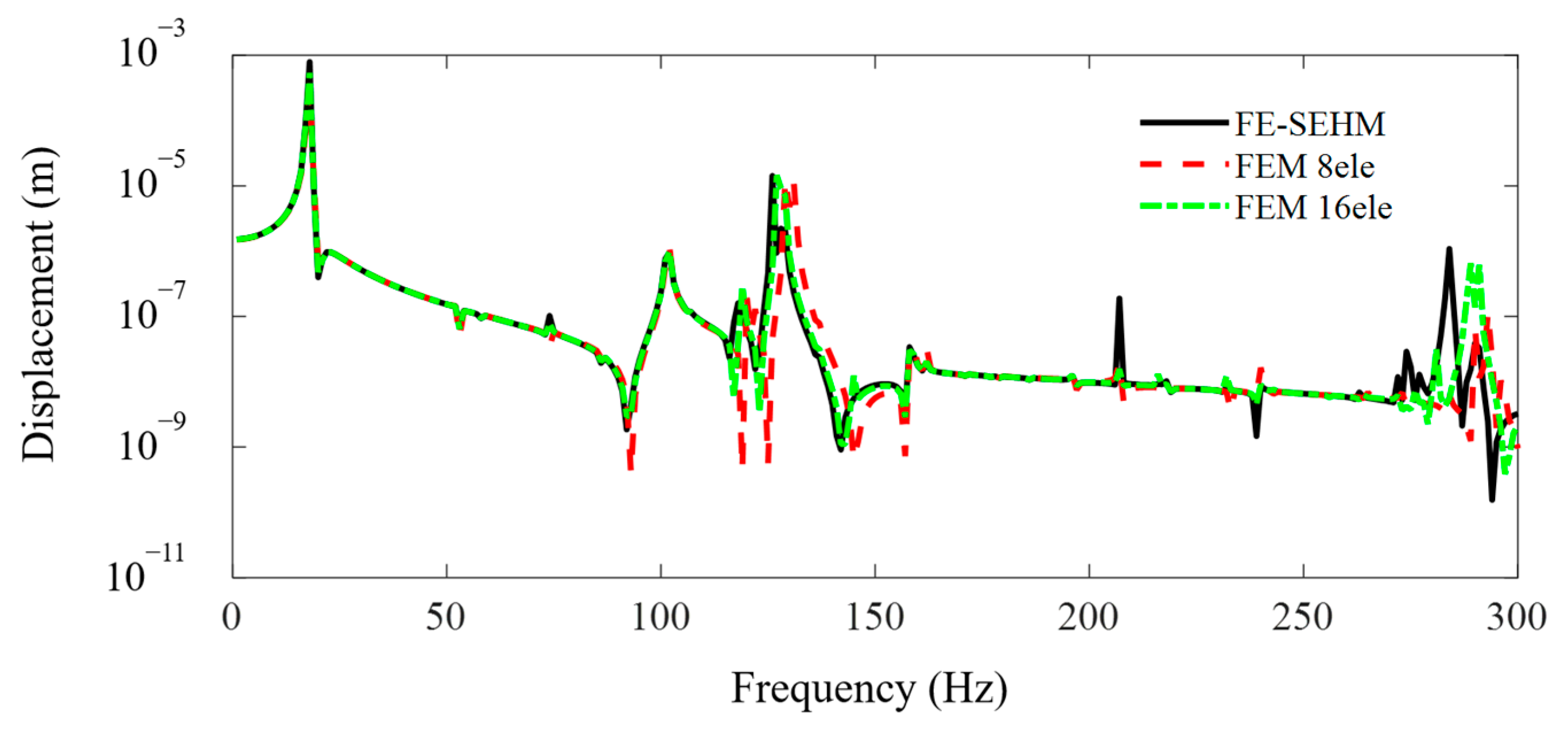
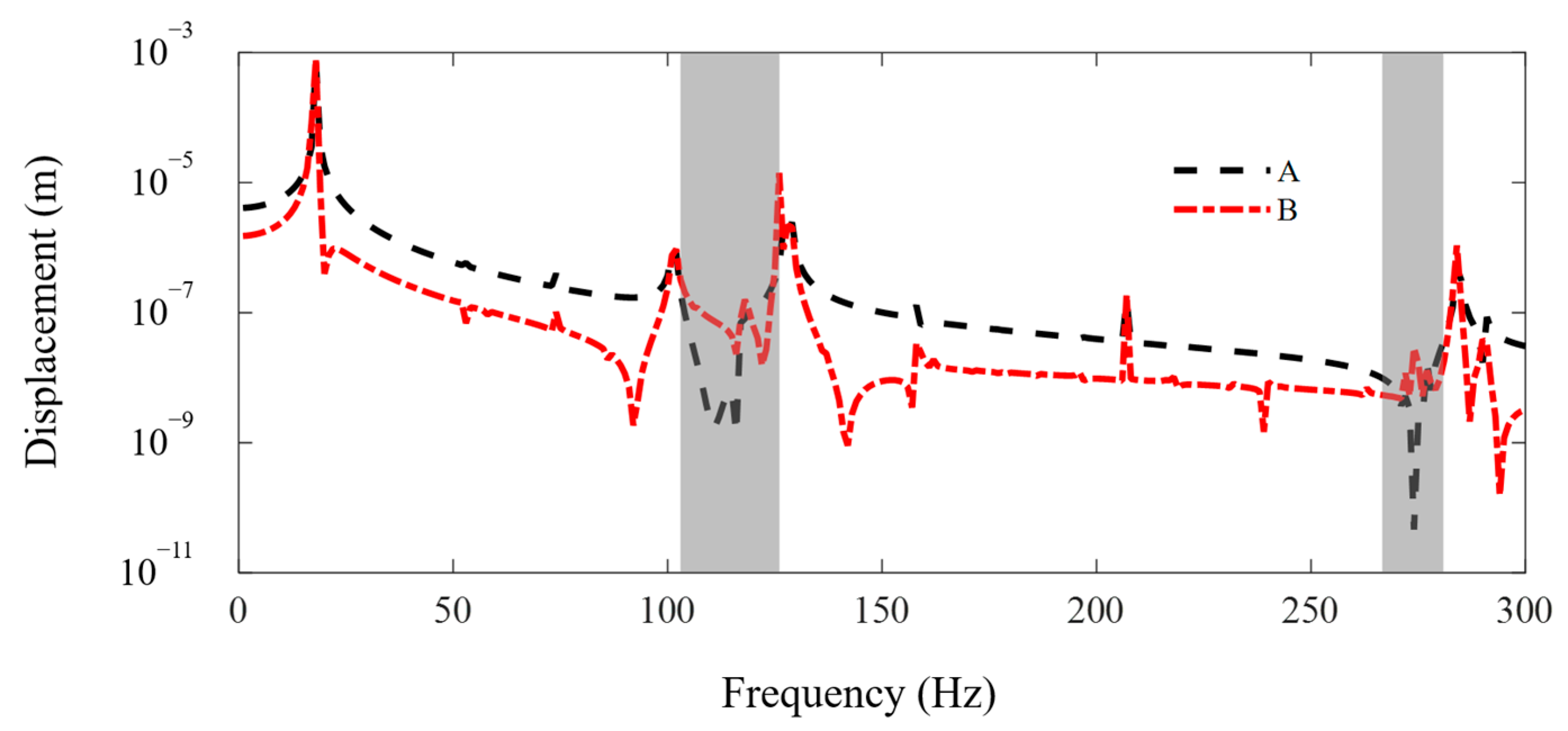
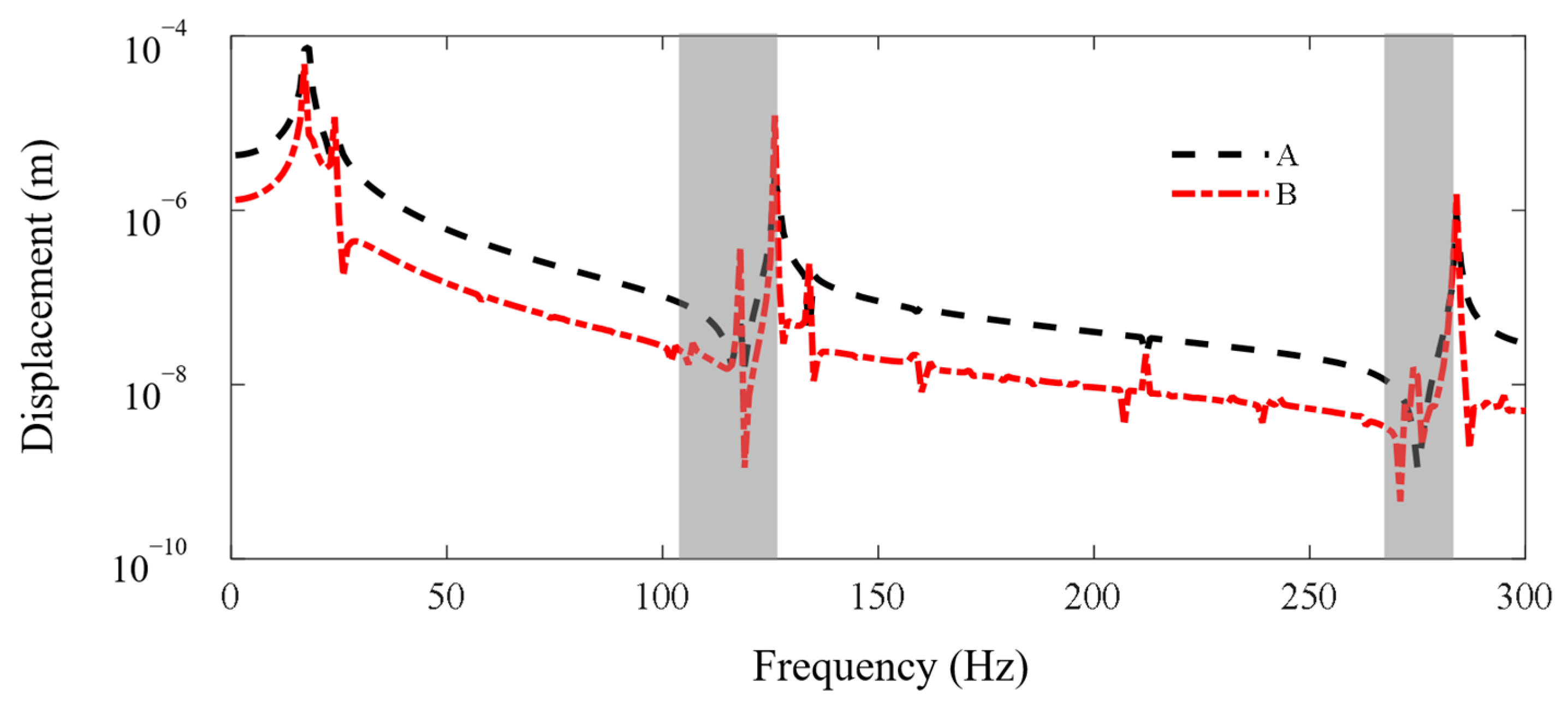
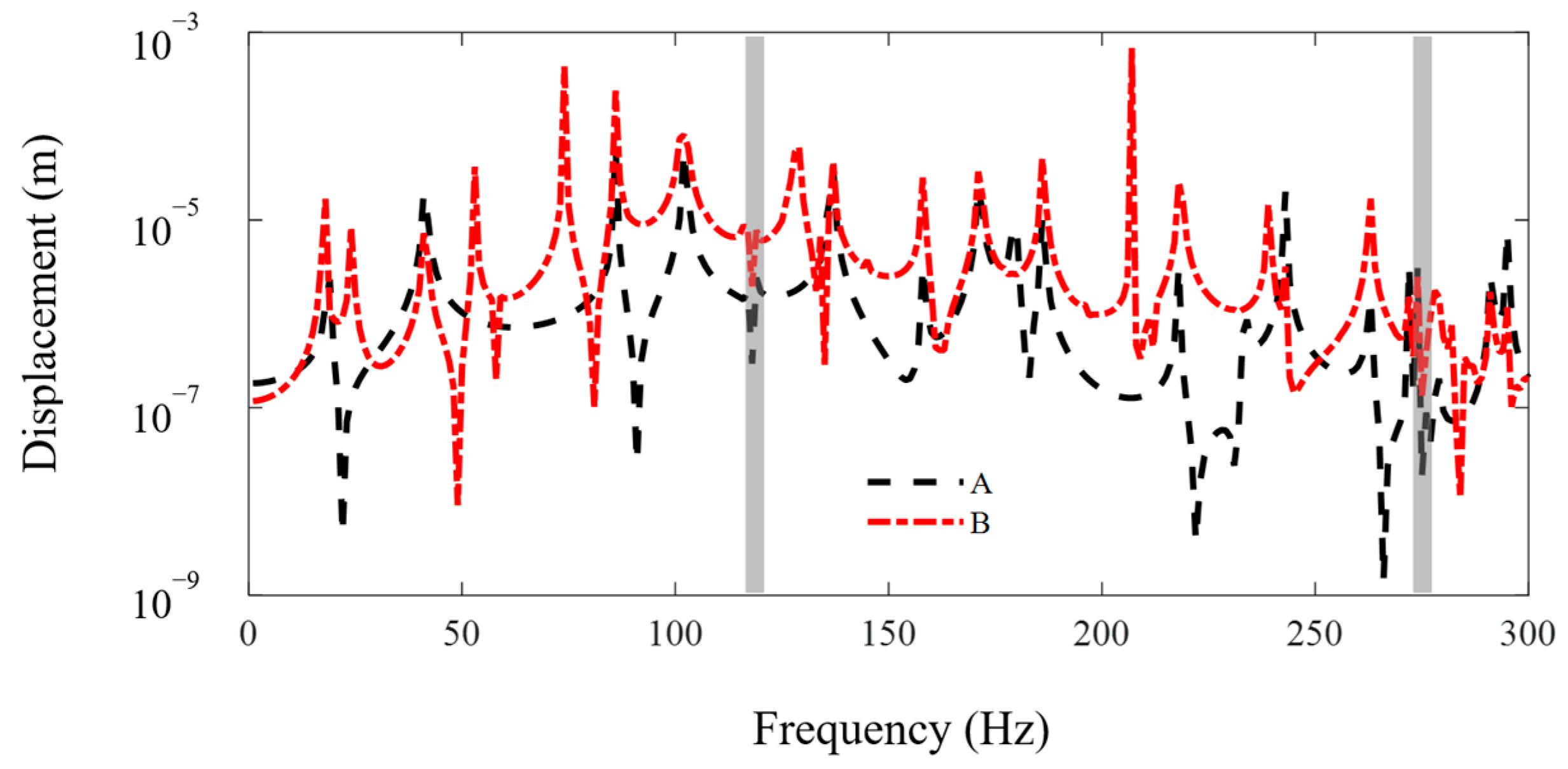
3.1. Validations
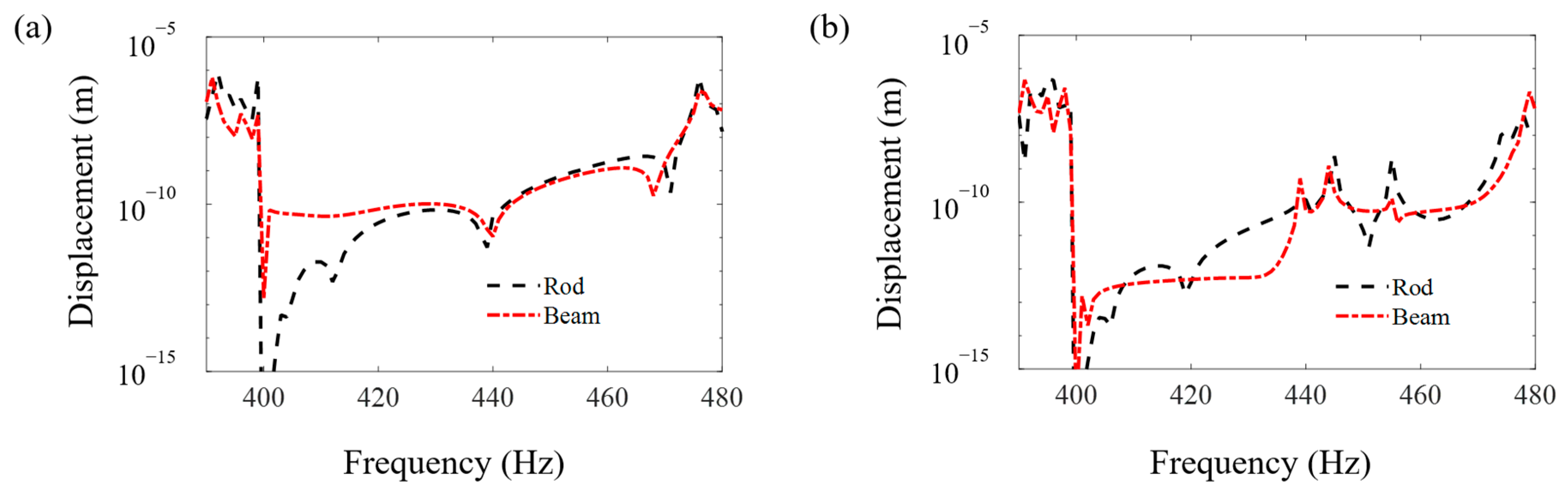
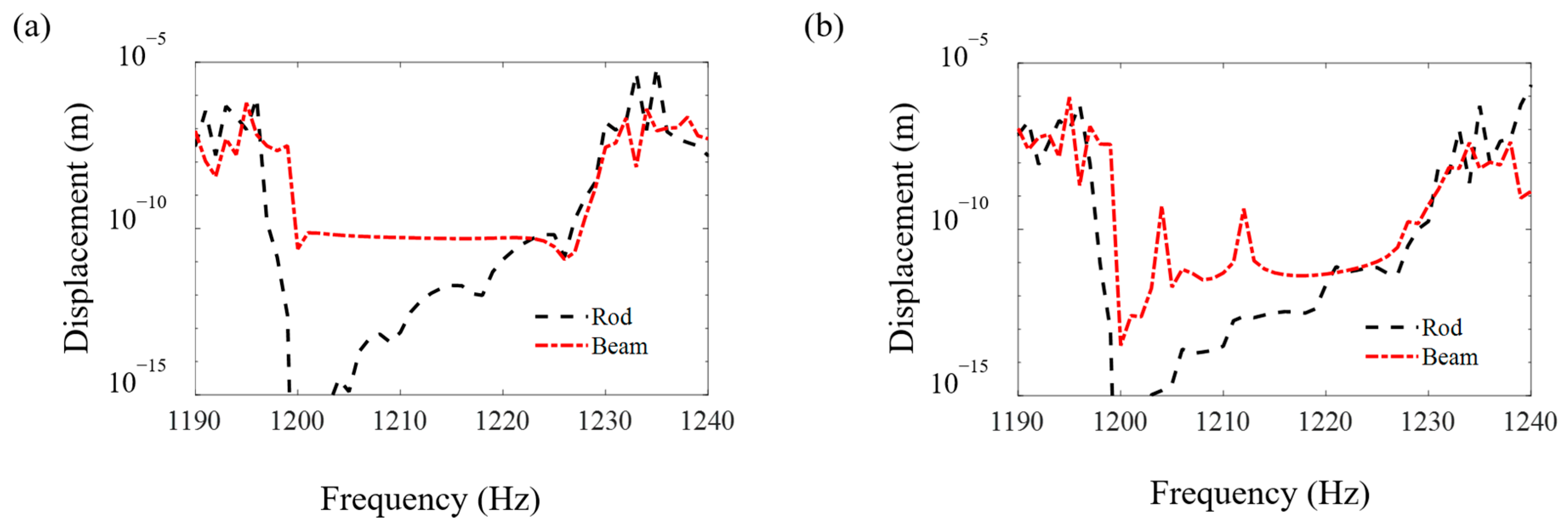
3.2. Band-Gap Characteristics Analysis
4. Conclusions
- (1)
- The FE-SEHM is developed to obtain the dynamic stiffness matrix of the metamaterial sandwich plate with axially deformed beam cores, and the vibration band-gap characteristics can be efficiently characterized by calculating the FRFs of the structure.
- (2)
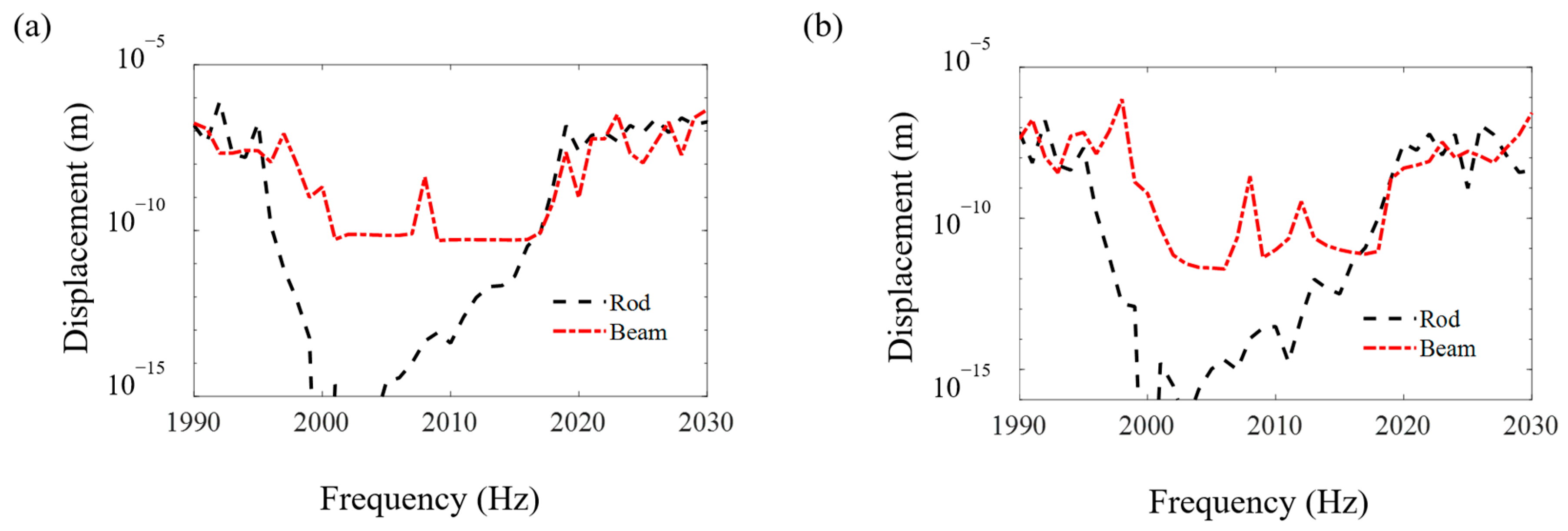
- Compared with the metamaterial plate with rod cores, the positions and widths of the axial vibration band-gaps of the beams for the metamaterial plate with axially deformed beam cores are the same, but the vibration reduction effect is declined, which should be noticed in the practical applications.
- (3)
- The flexural vibration band-gaps of the beams for the metamaterial sandwich plate along the x and y axes appear near the flexural vibration natural frequencies of the axially deformed beams, and the lower order band-gaps are wider than the higher order ones.
- (4)
- The flexural vibration band-gaps of the metamaterial sandwich plate can be found uncoupling with the axial vibration band-gaps. The two kinds of band-gaps reduce the vibrations of the plate along the z axis and the x and y axes independently.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Fabro, A.T.; Beli, D.; Ferguson, N.S.; Arruda, F., Jr.; Mace, B.R. Wave and vibration analysis of elastic metamaterial and phononic crystal beams with slowly varying properties. Wave Motion 2021, 103, 102728. [Google Scholar] [CrossRef]
- Wei, W.; Chronopoulos, D.; Meng, H. Broadband vibration attenuation achieved by 2D elasto-acoustic metamaterial plates with rainbow stepped resonators. Materials 2021, 14, 4759. [Google Scholar] [CrossRef] [PubMed]
- Xiao, X.; He, Z.C.; Li, E.; Cheng, A.G. Design multi-stopband laminate acoustic metamaterials for structural-acoustic coupled system. Mech. Syst. Signal Process. 2019, 115, 418–433. [Google Scholar] [CrossRef]
- Wu, X.; Li, Y.G.; Cai, W.; Guo, K.L.; Zhu, L. Dynamic responses and energy absorption of sandwich panel with aluminium honeycomb core under ice wedge impact. Int. J. Impact Eng. 2022, 162, 104137. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Arabi, E.; Masoodi, A.R. Nonlinear analysis of FG-sandwich plates and shells. Aerosp. Sci. Technol. 2019, 87, 178–189. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Mokhtari, M.; Masoodi, A.R. Stability and free vibration analysis of tapered sandwich columns with functionally graded core and flexible connections. CEAS Aeronaut. J. 2018, 9, 629–648. [Google Scholar] [CrossRef]
- Chen, J.S.; Huang, Y.J.; Chien, I.T. Flexural wave propagation in metamaterial beams containing membrane-mass structures. Int. J. Mech. Sci. 2017, 131–132, 500–506. [Google Scholar] [CrossRef]
- Ren, F.G.; Wang, L.; Liu, H.T. Low frequency and broadband vibration attenuation of a novel lightweight bidirectional re-entrant lattice metamaterial. Mater. Lett. 2021, 299, 130133. [Google Scholar] [CrossRef]
- Banerjee, A. Flexural waves in graded metabeam lattice. Phys. Lett. A 2021, 388, 127057. [Google Scholar] [CrossRef]
- Chen, D.K.; Zi, H.; Li, Y.G.; Li, X.Y. Low frequency ship vibration isolation using the band gap concept of sandwich plate-type elastic metastructures. Ocean. Eng. 2021, 235, 109460. [Google Scholar] [CrossRef]
- Basta, E.E.; Ghommem, M.; Emam, S.A. Vibration suppression and optimization of conserved-mass metamaterial beam. Int. J. Non-Linear Mech. 2020, 120, 103360. [Google Scholar] [CrossRef]
- Yang, M.; Sheng, P. Sound absorption structures: From porous media to acoustic metamaterials. Annu. Rev. Mater. Res. 2017, 47, 83–114. [Google Scholar] [CrossRef]
- Li, Y.G.; Zi, H.; Wu, X.; Zhu, L. Flexural wave propagation and vibration isolation characteristics of sandwich plate-type elastic metamaterials. J. Vib. Control 2021, 27, 1443–1452. [Google Scholar] [CrossRef]
- Santo, D.R.; Mencik, J.M.; Gonçalves, P.J.P. On the multi-mode behavior of vibrating rods attached to nonlinear springs. Nonlinear Dyn. 2020, 100, 2187–2203. [Google Scholar] [CrossRef]
- Lu, K.; Tian, Y.J.; Gao, N.S.; Li, L.Z.; Lei, H.X.; Yu, M.R. Propagation of longitudinal waves in the broadband hybrid mechanism gradient elastic metamaterials rods. Appl. Acoust. 2021, 171, 107571. [Google Scholar] [CrossRef]
- Zhou, J.X.; Dou, L.L.; Wang, K.; Xu, D.L.; Ouyang, H.J. A nonlinear resonator with inertial amplification for very low-frequency flexural wave attenuations in beams. Nonlinear Dyn. 2019, 96, 647–665. [Google Scholar] [CrossRef]
- Guo, Z.K.; Hu, G.B.; Sorokin, V.; Tang, L.H.; Yang, X.D.; Zhang, J. Low-frequency flexural wave attenuation in metamaterial sandwich beam with hourglass lattice truss core. Wave Motion 2021, 104, 102750. [Google Scholar] [CrossRef]
- Mi, Y.Z.; Yu, X. Sound transmission of acoustic metamaterial beams with periodic inertial amplification mechanisms. J. Sound Vib. 2021, 499, 116009. [Google Scholar] [CrossRef]
- Miranda, E.J.P., Jr.; Nobrega, E.D.; Ferreira, A.H.R.; Dos Santos, J.M.C. Flexural wave band gaps in a multi-resonator elastic metamaterial plate using Kirchhoff-Love theory. Mech. Syst. Signal Process. 2019, 116, 480–504. [Google Scholar] [CrossRef]
- Sugino, C.; Ruzzene, M.; Erturk, A. An analytical framework for locally resonant piezoelectric metamaterial plates. Int. J. Solids Struct. 2020, 182–183, 281–294. [Google Scholar] [CrossRef]
- Li, Q.J.; Sheng, M.P.; Qin, Q.; Han, Y.Y.; Wang, S. The merging of bandgaps based on locally resonant plate with periodically attached stepped-frequency resonators. J. Appl. Phys. 2022, 131, 025103. [Google Scholar] [CrossRef]
- Alam, Z.; Sharma, A.K. Functionally graded soft dielectric elastomer phononic crystals: Finite deformation, electro-elastic longitudinal waves, and band gaps tunability via electro-mechanical loading. Int. J. Appl. Mech. 2022, 14, 2250050. [Google Scholar] [CrossRef]
- Sharma, A.K.; Joglekar, M.M.; Joglekar, D.M.; Alam, Z. Topology optimization of soft compressible phononic laminates for widening the mechanically tunable band gaps. Compos. Struct. 2022, 289, 115389. [Google Scholar] [CrossRef]
- Sharma, A.K.; Kosta, M.; Shmuel, G.; Amir, O. Gradient-based topology optimization of soft dielectrics as tunable phononic crystals. Compos. Struct. 2022, 280, 114846. [Google Scholar] [CrossRef]
- Zouari, S.; Brocail, J.; Génevaux, J.M. Flexural wave band gaps in metamaterial plates: A numerical and experimental study from infinite to finite models. J. Sound Vib. 2018, 435, 246–263. [Google Scholar] [CrossRef]
- Dal Poggetto, V.F.; Serpa, A.L. Elastic wave band gaps in a three-dimensional periodic metamaterial using the plane wave expansion method. Int. J. Mech. Sci. 2020, 184, 105841. [Google Scholar] [CrossRef]
- Jiang, H.; Zhang, M.; Liu, Y.; Pei, D.; Chen, M.; Wang, Y. Band gaps and vibration isolation of a three-dimensional metamaterial with a star structure. Materials 2020, 13, 3812. [Google Scholar] [CrossRef] [PubMed]
- Huang, K.X.; Shui, G.S.; Wang, Y.Z.; Wang, Y.S. Meta-arrest of a fast propagating crack in elastic wave metamaterials with local resonators. Mech. Mater. 2020, 148, 103497. [Google Scholar] [CrossRef]
- Wang, H.; Cheng, S.L.; Wang, C.; Sun, Y.T.; Xin, Y.J. Tunable band gaps and double-negative properties of innovative acoustic metamaterials. Appl. Phys. A 2021, 127, 495. [Google Scholar] [CrossRef]
- Fan, L.; He, Y.; Chen, X.A.; Zhao, X. A frequency response function-based optimization for metamaterial beams considering both location and mass distributions of local resonators. J. Appl. Phys. 2021, 130, 115101. [Google Scholar] [CrossRef]
- Dal Poggetto, V.F.; Serpa, A.L. Flexural wave band gaps in a ternary periodic metamaterial plate using the plane wave expansion method. J. Sound Vib. 2021, 495, 115909. [Google Scholar] [CrossRef]
- Yao, D.H.; Xiong, M.K.; Luo, J.Y.; Yao, L.Y. Flexural wave mitigation in metamaterial cylindrical curved shells with periodic graded arrays of multi-resonator. Mech. Syst. Signal Process. 2022, 168, 108721. [Google Scholar] [CrossRef]
- Nanda, A.; Karami, M.A. Tunable bandgaps in a deployable metamaterial. J. Sound Vib. 2018, 424, 120–136. [Google Scholar] [CrossRef]
- Hu, B.; Zhang, Z.F.; Yu, D.L.; Liu, J.W.; Zhu, F.L. Broadband bandgap and shock vibration properties of acoustic metamaterial fluid-filled pipes. J. Appl. Phys. 2020, 128, 205103. [Google Scholar] [CrossRef]
- Ren, T.; Li, F.M.; Chen, Y.N.; Liu, C.C.; Zhang, C.Z. Improvement of the band-gap characteristics of active composite laminate metamaterial plates. Compos. Struct. 2020, 254, 112831. [Google Scholar] [CrossRef]
- Hu, G.B.; Austin, A.C.M.; Sorokin, V.; Tang, L.H. Metamaterial beam with graded local resonators for broadband vibration suppression. Mech. Syst. Signal Process. 2021, 146, 106982. [Google Scholar] [CrossRef]
- Hao, S.M.; Wu, Z.J.; Li, F.M.; Zhang, C.Z. Enhancement of the band-gap characteristics in disordered elastic metamaterial multi-span beams: Theory and experiment. Mech. Res. Commun. 2021, 113, 103692. [Google Scholar] [CrossRef]
- Jung, J.; Kim, H.G.; Goo, S.; Chang, K.J.; Wang, S.Y. Realisation of a locally resonant metamaterial on the automobile panel structure to reduce noise radiation. Mech. Syst. Signal Process. 2019, 122, 206–231. [Google Scholar] [CrossRef]
- Fan, L.; He, Y.; Chen, X.A.; Zhao, X. Elastic metamaterial shaft with a stack-like resonator for low-frequency vibration isolation. J. Phys. D Appl. Phys. 2020, 53, 105101. [Google Scholar] [CrossRef]
- Zhang, Y.Y.; Gao, N.S.; Wu, J.H. New mechanism of tunable broadband in local resonance structures. Appl. Acoust. 2020, 169, 107482. [Google Scholar] [CrossRef]
- E, L.; Wu, Z.; Zou, G.; Li, F.; Zhang, C.; Sun, A.; Du, Q. Band-gap characteristics of elastic metamaterial plate with axial rod core by the finite element and spectral element hybrid method. Mech. Adv. Mater. Struct. 2022, 29, 2405–2422. [Google Scholar]
- E, L.; Chen, Z.; Li, F.; Zou, G. Band-gap properties of elastic sandwich metamaterial plates with composite periodic rod core. Acta Mech. Solida Sin. 2022, 35, 51–62. [Google Scholar]
- Wu, Z.J.; Li, F.M.; Zhang, C. Vibration band-gap properties of three-dimensional Kagome lattices using the spectral element method. J. Sound Vib. 2015, 341, 162–173. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
E, L.; Wu, Z.; Li, F.; Zou, G. A Finite/Spectral Element Hybrid Method for Modeling and Band-Gap Characterization of Metamaterial Sandwich Plates. Materials 2023, 16, 1098. https://doi.org/10.3390/ma16031098
E L, Wu Z, Li F, Zou G. A Finite/Spectral Element Hybrid Method for Modeling and Band-Gap Characterization of Metamaterial Sandwich Plates. Materials. 2023; 16(3):1098. https://doi.org/10.3390/ma16031098
Chicago/Turabian StyleE, Linzhongyang, Zhijing Wu, Fengming Li, and Guangping Zou. 2023. "A Finite/Spectral Element Hybrid Method for Modeling and Band-Gap Characterization of Metamaterial Sandwich Plates" Materials 16, no. 3: 1098. https://doi.org/10.3390/ma16031098
APA StyleE, L., Wu, Z., Li, F., & Zou, G. (2023). A Finite/Spectral Element Hybrid Method for Modeling and Band-Gap Characterization of Metamaterial Sandwich Plates. Materials, 16(3), 1098. https://doi.org/10.3390/ma16031098






