Effect of Latent Heat by Phase Transformation on the Thermal Behavior of Steel Billet during Heating
Abstract
:1. Introduction
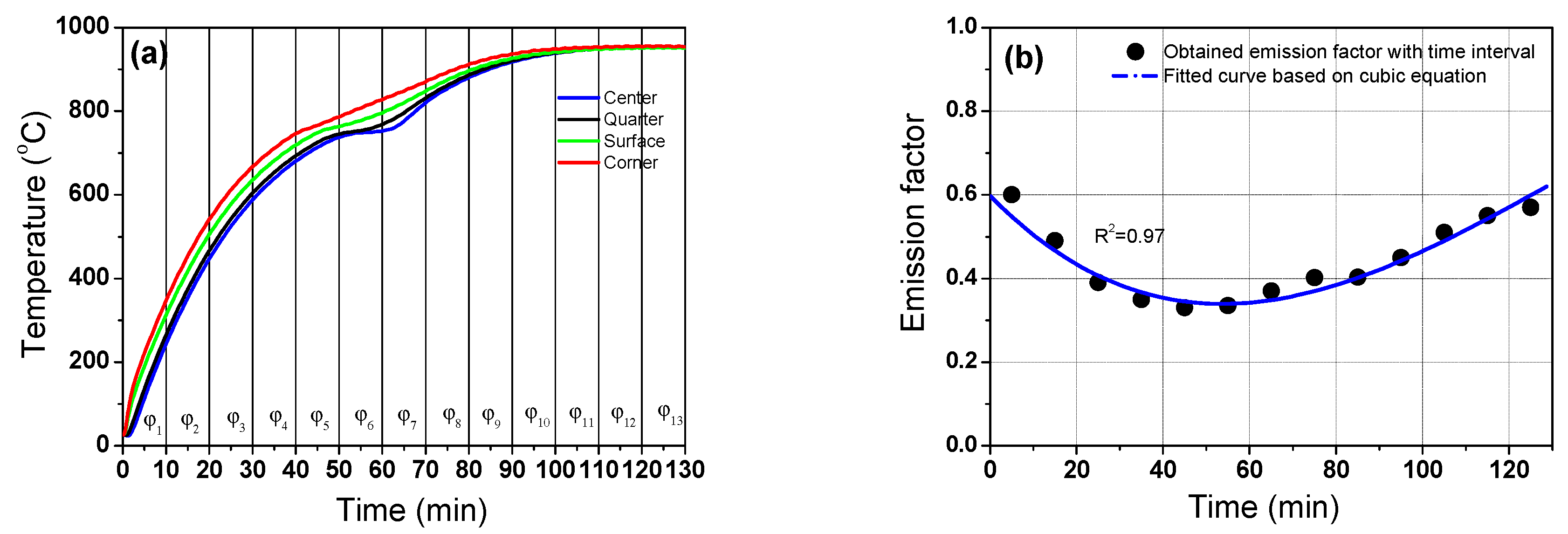
2. Experimental Procedures for Heating
3. Numerical Model
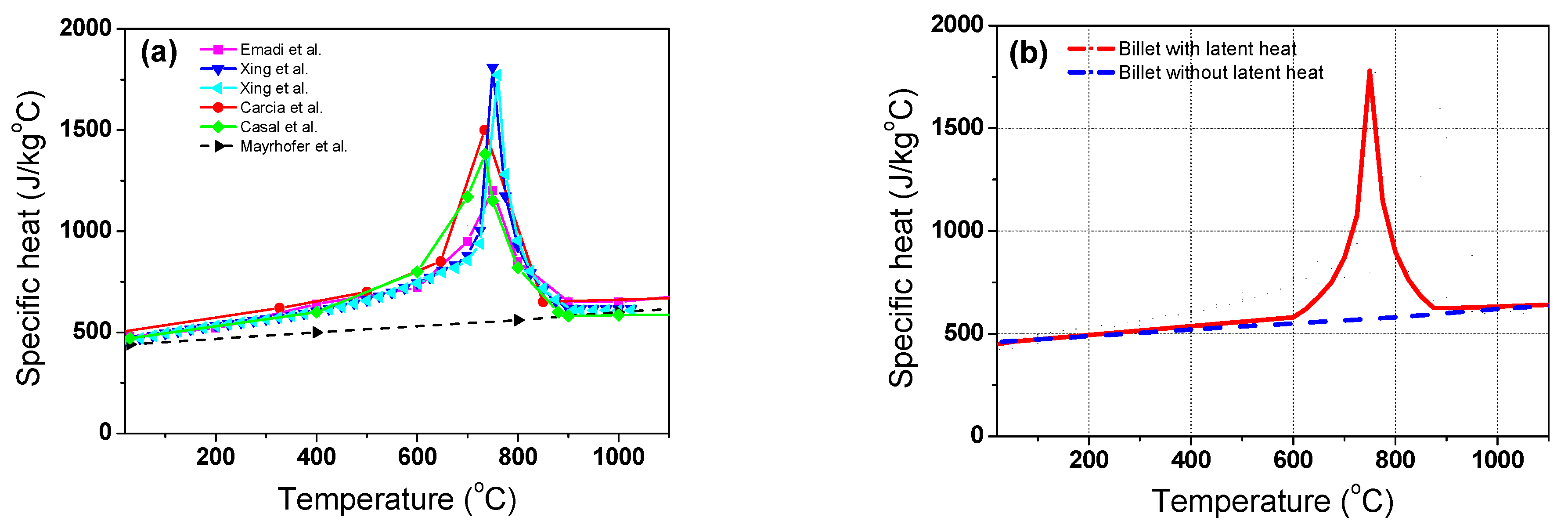
3.1. Physical Problem and Materials
3.2. Governing Equations and Boundary Conditions
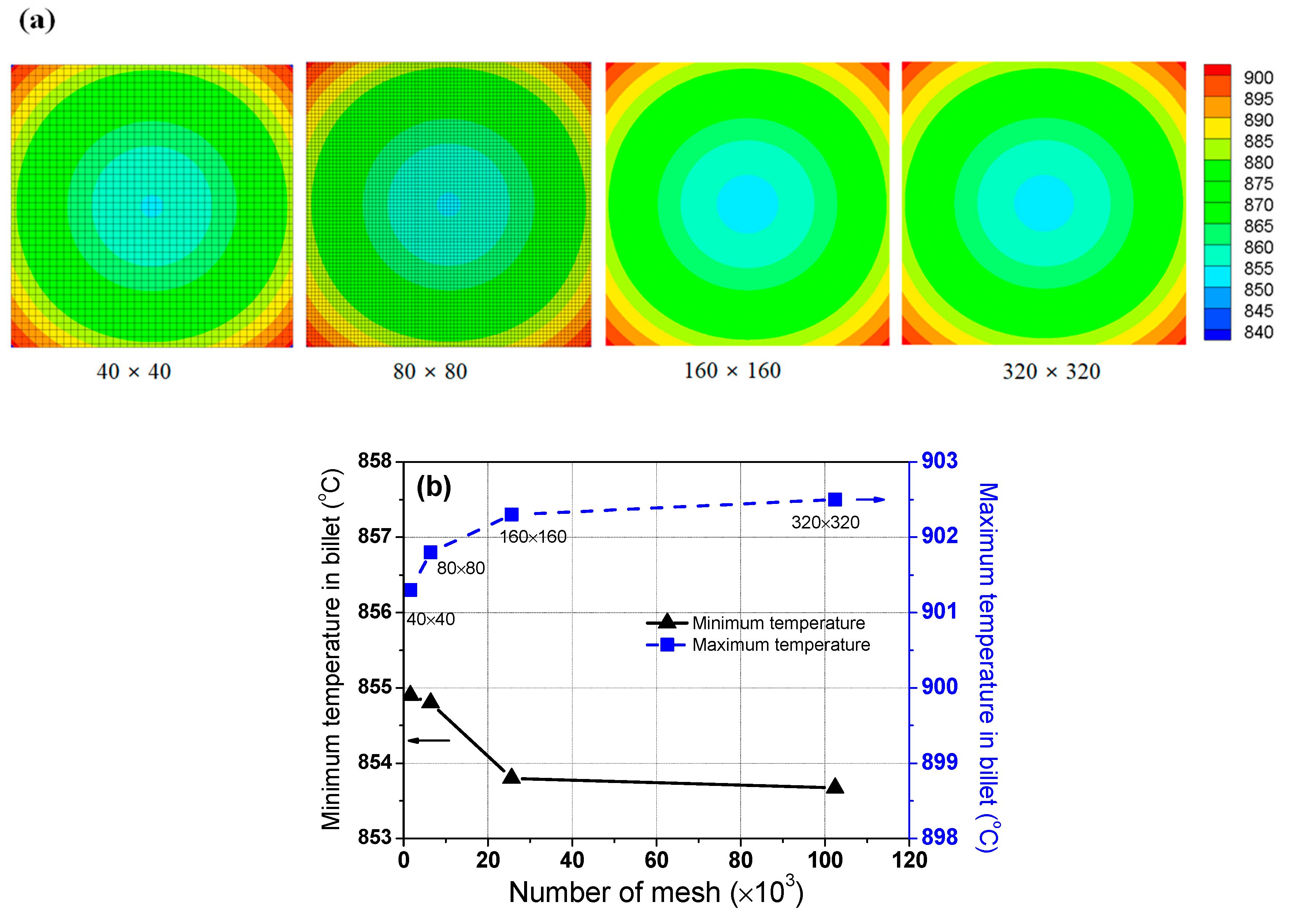
3.3. Numerical Methods and Grid Independence
4. Results and Discussion
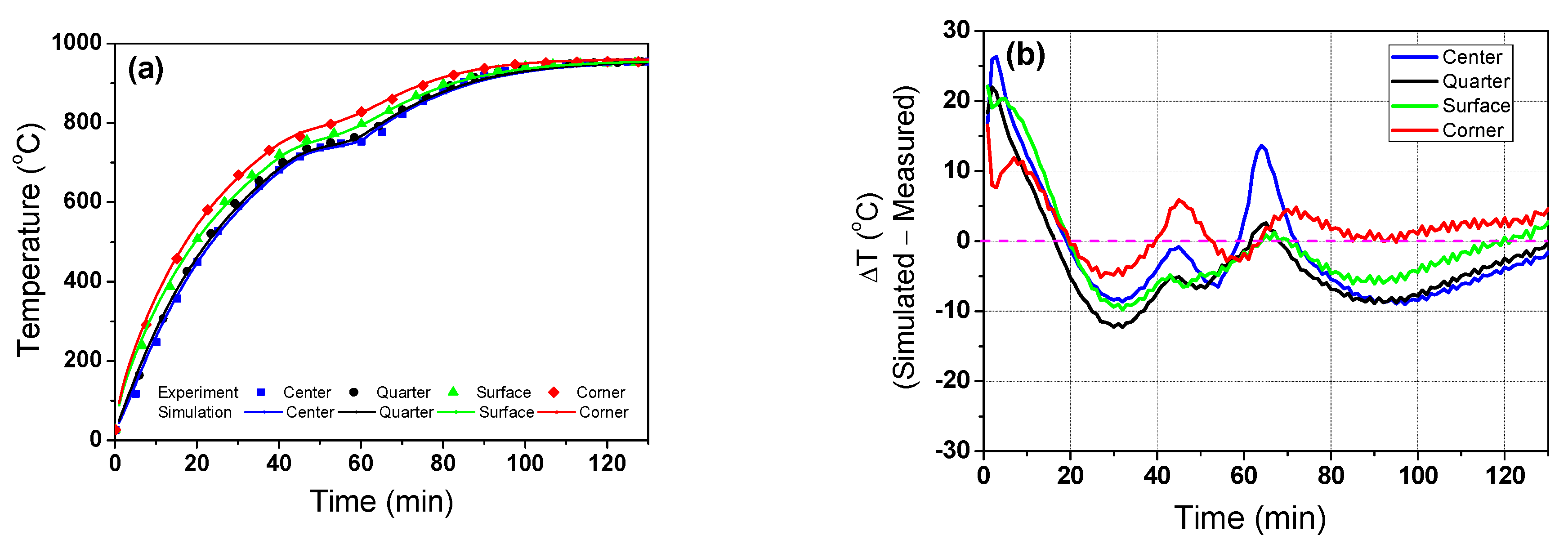
4.1. Model Parameter and Validation
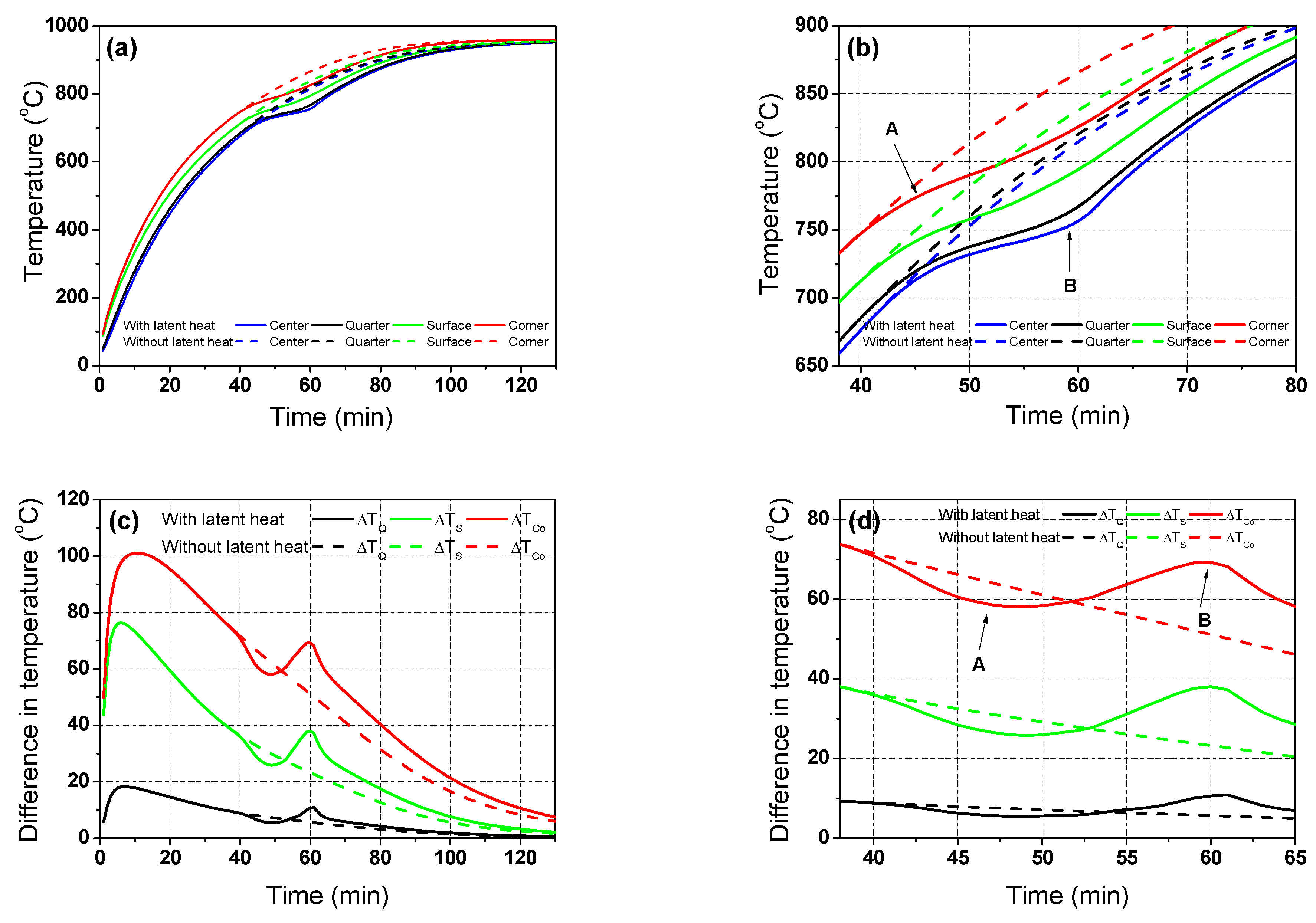
4.2. Influence of Latent Heat by Phase Transform
5. Conclusions
- The latent heat by phase transformation strongly affected the temperature distribution of the billet during the heating process, although the heating rate of the billet was not high. The temperature profile of the center region of the steel with latent heat had a strong flat shape compared with the other regions, as the heat supply to the center region was limited during the heating process owing to the finite thermal conductivity and mass effect of the billet.
- The latent heat by phase transformation typically occurred in the middle stage of heating, and the latent heat increased the temperature deviation of the billet during heating owing to the delay in the temperature rise at the center region of the billet.
- Industrial hot rolling mills are required to consider the latent heat by phase transformation of the billet to properly design the heating pattern for the billet, that is, the heating pattern in the reheating furnace should be varied with the materials to obtain a high heating quality for the billet.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Bi | Biot number |
| cp | specific heat (J/kgK) |
| ∆H | latent heat (kJ/kg) |
| h | heat transfer coefficient (W/m2K) |
| k | thermal conductivity of the billet (W/mK) |
| L | billet width (m) |
| q | heat flux (W/m2) |
| R | heating rate (K/s) |
| S | objective function |
| T | temperature (K) |
| t | time (s) |
| x, y | space coordinates (m) |
| Greek symbols | |
| φ | emission factor |
| ρ | density (kg/m3) |
| σ | Stefan–Boltzmann constant (5.56 × 10−8 (W/m2K4)) |
| ε | emissivity |
| Subscript | |
| C | center |
| Co | corner |
| cv | convection |
| i | initial |
| f | final |
| g | gas in the reheating furnace |
| m | measured |
| p | predicted |
| Q | quarter |
| r | radiation |
| S | surface |
| t | total |
References
- Zhao, J.; Ma, L.; Zayed, M.E.; Elsheikh, A.H.; Li, W.; Yan, Q.; Wang, J. Industrial reheating furnaces: A review of energy efficiency assessments, waste heat recovery potentials, heating process characteristics and perspectives for steel industry. Process Saf. Environ. Prot. 2021, 147, 1209–1228. [Google Scholar] [CrossRef]
- Wusatowski, Z. Fundamentals of Rolling; Pergamon Press: London, UK; New York, NY, USA, 1969. [Google Scholar]
- Kim, S.Y.; Im, Y.T. Three-dimensional finite element analysis of non-isothermal shape rolling. J. Mater. Process Technol. 2002, 127, 57–63. [Google Scholar] [CrossRef]
- Serajzadeh, S.; Taheri, A.K.; Nejati, M.; Izadi, J.; Fattahi, M. An investigation on strain inhomogeneity in hot strip rolling process. J. Mater. Process Technol. 2002, 128, 88–99. [Google Scholar] [CrossRef]
- Hwang, J.K. Thermal Behavior of a Rod during Hot Shape Rolling and Its Comparison with a Plate during Flat Rolling. Processes 2020, 8, 327. [Google Scholar] [CrossRef]
- Kim, S.J.; Lee, T.; Hwang, J.K. High-strength bolt manufactured by an extrusion-based forming process using twinning-induced plasticity steel. J. Manuf. Process 2020, 59, 33–42. [Google Scholar] [CrossRef]
- Kim, J.G.; Huh, K.Y. Prediction of transient slab temperature distribution in the re-heating furnace of a walking-beam type for rolling of steel slabs. ISIJ Int. 2000, 40, 1115–1123. [Google Scholar] [CrossRef]
- Hsieh, C.T.; Huang, M.J.; Lee, S.T.; Wang, C.H. Numerical modeling of a walking-beam-type slab reheating furnace. Numer. Heat Transf. Part A Appl. 2008, 53, 966–981. [Google Scholar] [CrossRef]
- Wang, J.; Liu, Y.; Sundén, B.; Yang, R.; Baleta, J.; Vujanovic, M. Analysis of slab heating characteristics in a reheating furnace. Energy Convers. Manag. 2017, 149, 928–936. [Google Scholar] [CrossRef]
- Kim, J.G.; Huh, K.Y.; Kim, I.T. Three-dimensional analysis of the walking-beam-type slab reheating furnace in hot strip mills. Numer. Heat Transf. Part A Appl. 2014, 38, 589–609. [Google Scholar]
- Tang, G.; Wu, B.; Bai, D.; Wang, Y.; Bodnar, R.; Zhou, C. CFD modeling and validation of a dynamic slab heating process in an industrial walking beam reheating furnace. Appl. Therm. Eng. 2018, 132, 779–789. [Google Scholar] [CrossRef]
- Han, S.H.; Chang, D.; Kim, C.Y. A numerical analysis of slab heating characteristics in a walking beam type reheating furnace. Int. J. Heat Mass Transf. 2010, 53, 3855–3861. [Google Scholar] [CrossRef]
- Han, S.H.; Baek, S.W.; Kang, S.H.; Kim, C.Y. Numerical analysis of heating characteristics of a slab in a bench scale reheating furnace. Int. J. Heat Mass Transf. 2007, 50, 2019–2023. [Google Scholar] [CrossRef]
- Prieler, R.; Mayr, B.; Demuth, M.; Holleis, B.; Hochenauer, C. Numerical analysis of the transient heating of steel billets and the combustion process under air-fired and oxygen enriched conditions. Appl. Therm. Eng. 2016, 103, 252–263. [Google Scholar] [CrossRef]
- Kim, M.Y. A heat transfer model for the analysis of transient heating of the slab in a direct-fired walking beam type reheating furnace. Int. J. Heat Mass Transf. 2007, 50, 3740–3748. [Google Scholar] [CrossRef]
- Han, S.H.; Baek, S.W.; Kim, M.Y. Transient radiative heating characteristics of slabs in a walking beam type reheating furnace. Int. J. Heat Mass Transf. 2009, 52, 1005–1011. [Google Scholar] [CrossRef]
- Tan, C.K.; Jenkins, J.; Ward, J.; Broughton, J.; Heeley, A. Zone modelling of the thermal performances of a large-scale bloom reheating furnace. Appl. Therm. Eng. 2013, 50, 1111–1118. [Google Scholar] [CrossRef]
- Emadi, A.; Saboonchi, A.; Taheri, M.; Hassanpour, S. Heating characteristics of billet in a walking hearth type reheating furnace. Appl. Therm. Eng. 2014, 63, 396–405. [Google Scholar] [CrossRef]
- Barr, P.V. The development, verification, and application of a steady-state thermal model for the pusher-type reheat furnace. Metall. Mater. Trans. B 1995, 26, 851–869. [Google Scholar] [CrossRef]
- Švantner, M.; Študent, J.; Veselý, Z. Continuous walking-beam furnace 3D zonal model and direct thermal-box barrier based temperature measurement. Case Stud. Therm. Eng. 2020, 18, 100608. [Google Scholar] [CrossRef]
- Chen, D.; Xu, H.; Lu, B.; Chen, G.; Zhang, L. Solving the heat transfer boundary condition of billet in reheating furnace by combining “black box” test with mathematic model. Case Stud. Therm. Eng. 2022, 40, 102486. [Google Scholar] [CrossRef]
- Li, G.J.; Li, X.T.; Chen, H.G. The simplified method to calculate two-dimensional heat conduction equations of heating slab. Appl. Mech. Mater. 2011, 79, 105–110. [Google Scholar] [CrossRef]
- Ji, W.; Li, G.; Wei, L.; Yi, Z. Modeling and determination of total heat exchange factor of regenerative reheating furnace based on instrumented slab trials. Case Stud. Therm. Eng. 2021, 24, 100838. [Google Scholar] [CrossRef]
- Gang, D.H.; Kim, G.H.; Lee, Y.G. Prediction of Billet Temperature by the Total Heat Exchange Factor on the Reheating Furnace. Trans. Korean Soc. Mech. Eng. B 2000, 24, 1549–1554. [Google Scholar]
- Luo, X.; Yang, Z. A new approach for estimation of total heat exchange factor in reheating furnace by solving an inverse heat conduction problem. Int. J. Heat Mass Transf. 2017, 112, 1062–1071. [Google Scholar] [CrossRef]
- Jang, Y.J.; Kim, S.W. An estimation of a billet temperature during reheating furnace operation. Int. J. Control. Autom. 2007, 5, 43–50. [Google Scholar]
- Edalatpour, S.; Saboonchi, A.; Hassanpour, S. Effect of phase transformation latent heat on prediction accuracy of strip laminar cooling. J. Mater. Process Technol. 2011, 211, 1776–1782. [Google Scholar] [CrossRef]
- Hwang, J.K. Effect of Ring Configuration on the Deviation in Cooling Rate and Mechanical Properties of a Wire Rod during the Stelmor Cooling Process. J. Mater. Eng. Perform. 2020, 29, 1732–1740. [Google Scholar] [CrossRef]
- Xing, Y.; Wang, W.; Al-azzani, H. Assessment of thermal properties of various types of high-strength steels at elevated temperatures. Fire Saf. J. 2021, 122, 103348. [Google Scholar] [CrossRef]
- Huiping, L.; Guoqun, Z.; Shanting, N.; Chuanzhen, H. FEM simulation of quenching process and experimental verification of simulation results. Mater. Sci. Eng. A 2007, 452, 705–714. [Google Scholar] [CrossRef]
- Hsieh, C.T.; Huang, M.J.; Lee, S.T.; Wang, C.H. A numerical study of skid marks on the slabs in a walking-beam type slab reheating furnace. Numer. Heat Transf. Part A Appl. 2010, 57, 1–17. [Google Scholar] [CrossRef]
- Jang, J.H.; Lee, D.E.; Kim, C.; Kim, M.Y. Prediction of furnace heat transfer and its influence on the steel slab heating and skid mark formation in a reheating furnace. ISIJ Int. 2008, 48, 1325–1330. [Google Scholar] [CrossRef]
- Dubey, S.K.; Srinivasan, P. Development of three dimensional transient numerical heat conduction model with growth of oxide scale for steel billet reheat simulation. Int. J. Therm. Sci. 2014, 84, 214–227. [Google Scholar] [CrossRef]
- Hwang, J.K. The temperature distribution and underlying cooling mechanism of steel wire rod in the Stelmor type cooling process. Appl. Therm. Eng. 2018, 142, 311–320. [Google Scholar] [CrossRef]
- Arkhazloo, N.B.; Bouissa, Y.; Bazdidi-Tehrani, F.; Jadidi, M.; Morin, J.B.; Jahazi, M. Experimental and unsteady CFD analyses of the heating process of large size forgings in a gas-fired furnace. Case Stud. Therm. Eng. 2019, 14, 100428. [Google Scholar] [CrossRef]
- García, A.M.; Colorado, A.F.; Obando, J.E.; Arrieta, C.E.; Amell, A.A. Effect of the burner position on an austenitizing process in a walking-beam type reheating furnace. Appl. Therm. Eng. 2019, 153, 633–645. [Google Scholar] [CrossRef]
- Casal, J.M.; Porteiro, J.; Míguez, J.L.; Vázquez, A. New methodology for CFD three-dimensional simulation of a walking beam type reheating furnace in steady state. Appl. Therm. Eng. 2015, 86, 69–80. [Google Scholar] [CrossRef]
- Mayrhofer, M.; Koller, M.; Seemann, P.; Prieler, R.; Hochenauer, C. CFD investigation of a vertical annealing furnace for stainless steel and non-ferrous alloys strips—A comparative study on air-staged & MILD combustion. Therm. Sci. Eng. Prog. 2022, 28, 101056. [Google Scholar]
- Wang, K.Y.; Jin, Y.J.; Xu, M.J.; Chen, J.S.; Lu, H. Estimation of heat transfer coefficient and phase transformation latent heat by modified pattern search method. Int. Commun. Heat Mass Transf. 2015, 68, 14–19. [Google Scholar] [CrossRef]
- Hwang, J.K. Effect of Air Temperature on the Thermal Behavior and Mechanical Properties of Wire Rod Steel during Stelmor Cooling. ISIJ Int. 2022, 62, 2343–2354. [Google Scholar] [CrossRef]
- Steau, E.; Mahendran, M.; Poologanathan, K. Elevated temperature thermal properties of carbon steels used in cold-formed light gauge steel frame systems. J. Build. Eng. 2020, 28, 101074. [Google Scholar] [CrossRef]
- Yang, Z.; Luo, X. Optimal set values of zone modeling in the simulation of a walking beam type reheating furnace on the steady-state operating regime. Appl. Therm. Eng. 2016, 101, 191–201. [Google Scholar] [CrossRef]
- García, A.M.; Amell, A.A. A numerical analysis of the effect of heat recovery burners on the heat transfer and billet heating characteristics in a walking-beam type reheating furnace. Int. J. Heat Mass Transf. 2018, 127, 1208–1222. [Google Scholar] [CrossRef]
- Hwang, J.K. Comparison of Temperature Distribution between TWIP and Plain Carbon Steels during Wire Drawing. Materials 2022, 15, 8696. [Google Scholar] [CrossRef] [PubMed]
- Singh, V.K.; Talukdar, P. Comparisons of different heat transfer models of a walking beam type reheat furnace. Int. Commun. Heat Mass Transf. 2013, 47, 20–26. [Google Scholar] [CrossRef]
- Wikström, P.; Weihong, Y.; Blasiak, W. The influence of oxide scale on heat transfer during reheating of steel. Steel Res. Int. 2008, 79, 765–775. [Google Scholar] [CrossRef]
- Jang, J.Y.; Huang, J.B. Optimization of a slab heating pattern for minimum energy consumption in a walking-beam type reheating furnace. Appl. Therm. Eng. 2015, 85, 313–321. [Google Scholar] [CrossRef]
- Lindholm, D. A finite element method for solution of the three-dimensional time-dependent heat-conduction equation with application for heating of steels in reheating furnaces. Numer. Heat Transf. Part A Appl. 1999, 35, 155–172. [Google Scholar] [CrossRef]
- Jang, J.H.; Lee, D.E.; Kim, M.Y.; Kim, H.G. Investigation of the slab heating characteristics in a reheating furnace with the formation and growth of scale on the slab surface. Int. J. Heat Mass Transf. 2010, 53, 4326–4332. [Google Scholar] [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; Tayor & Francis: Boca Raton, FL, USA, 1980. [Google Scholar]
- Agarwal, P.K.; Brimacombe, J.K. Mathematical model of heat flow and austenite-pearlite transformation in eutectoid carbon steel rods for wire. Metall. Mater. Trans. B 1981, 12, 121–133. [Google Scholar] [CrossRef]
- Liu, C.; He, A.; Qiang, Y.; Guo, D. Effect of Phase Transformation and Latent Heat on Hot Rolling Deformation Behavior of Non-oriented Electrical Steel. ISIJ Int. 2017, 57, 857–865. [Google Scholar] [CrossRef]
- Johnson, W.A.; Mehl, R.F. Reduction kinetics in processes of nucleation and growth. Trans. AIME 1939, 135, 416–458. [Google Scholar]
- Kang, D.H.; Kwag, D.S.; Kim, W.S. An Inverse Analysis on the Estimation of Two-dimensional Overall Heat Absorptance on the Slab in the Reheating Furnace. Trans. KSME(B) 2005, 29, 1257–1264. (In Korean) [Google Scholar]




| Approach | Strength | Weakness |
|---|---|---|
| 3D CFD analysis in furnace including mass, momentum, energy, and species |
|
|
| Temperature prediction based on analysis of billet and furnace gas/wall interaction |
|
|
| Temperature analysis of billet based on total heat transfer coefficient |
|
|
| C | Mn | Si | Cr | Mo | Fe |
|---|---|---|---|---|---|
| 0.35 | 0.7 | 0.25 | 1.05 | 0.22 | Balance |
| Condition | Parameter | Value |
|---|---|---|
| Billet | Material | Medium-carbon steel (AISI 4137) |
| Dimension | 160 mm × 160 mm × 600 mm | |
| Furnace | Type | Laboratory electric heating furnace |
| Overall dimension | 3.0 m × 1.8 m × 2.8 m | |
| Gas condition | N2 | |
| Billet support method | Conveyor rollers with oscillation | |
| Heating conditions | Initial billet temperature | 26 °C |
| Gas temperature in furnace | 960 °C | |
| Residence time | 130 min | |
| Ambient air temperature | 25 °C | |
| Measurement of temperature | Number of measurement points | 4 (center, quarter, surface, and corner) |
| Type of thermocouple | K-type thermocouple with 3.2 mm-diameter | |
| Data recorder apparatus | GL 820 (Graphtec Corporation, Yokohama, Japan) with sampling interval of 10 s |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, J.-K. Effect of Latent Heat by Phase Transformation on the Thermal Behavior of Steel Billet during Heating. Materials 2023, 16, 7598. https://doi.org/10.3390/ma16247598
Hwang J-K. Effect of Latent Heat by Phase Transformation on the Thermal Behavior of Steel Billet during Heating. Materials. 2023; 16(24):7598. https://doi.org/10.3390/ma16247598
Chicago/Turabian StyleHwang, Joong-Ki. 2023. "Effect of Latent Heat by Phase Transformation on the Thermal Behavior of Steel Billet during Heating" Materials 16, no. 24: 7598. https://doi.org/10.3390/ma16247598
APA StyleHwang, J.-K. (2023). Effect of Latent Heat by Phase Transformation on the Thermal Behavior of Steel Billet during Heating. Materials, 16(24), 7598. https://doi.org/10.3390/ma16247598





