Long-Term Shrinkage Measurements on Large-Scale Specimens Exposed to Real Environmental Conditions
Abstract
:1. Introduction
2. Materials and Methods
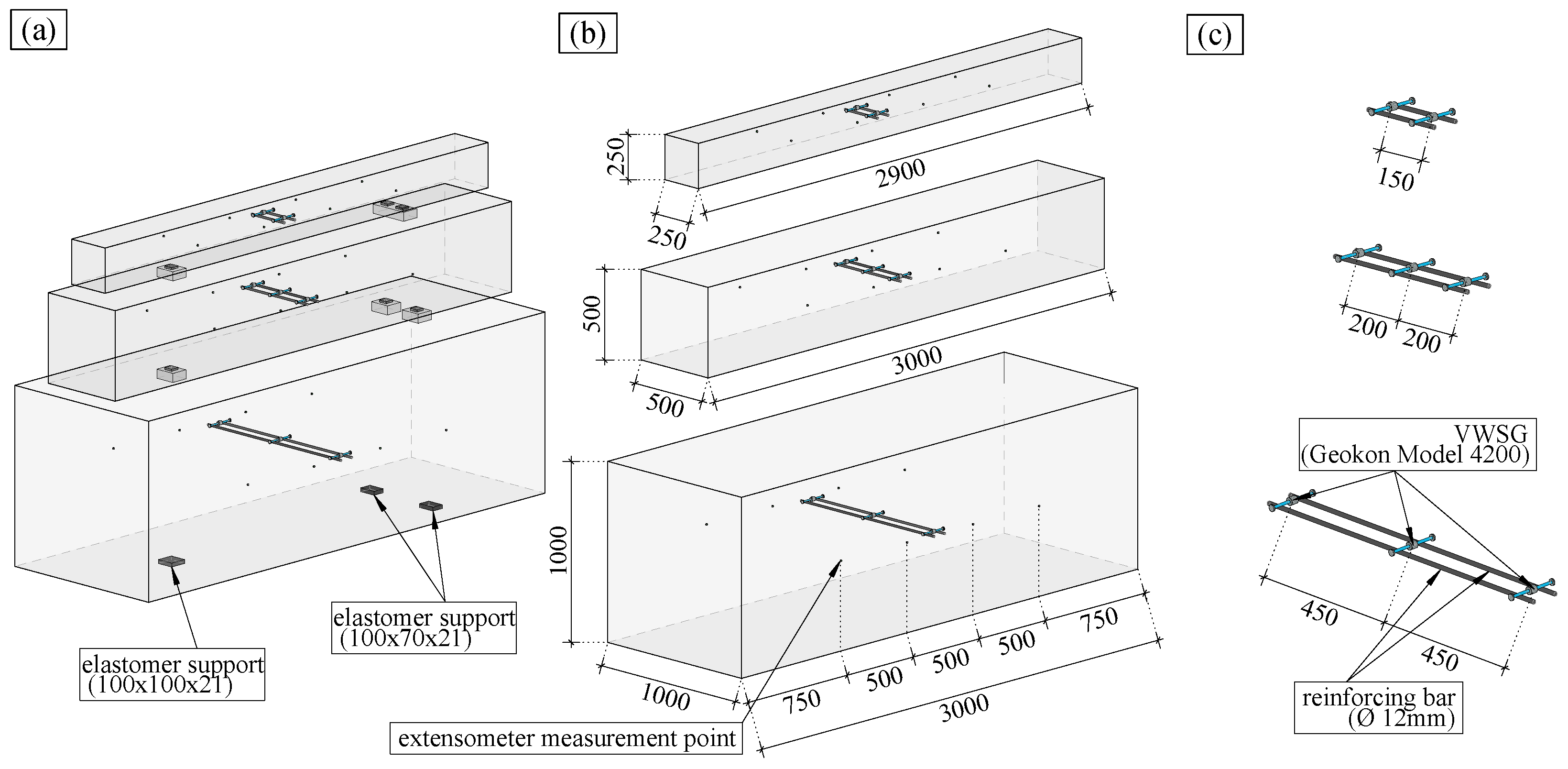
2.1. Experimental Setup
2.2. Production and Storage of the Specimens
2.3. Concrete Properties
2.4. Vibrating Wire Strain Gauges (VWSGs)
3. Theory/Analysis
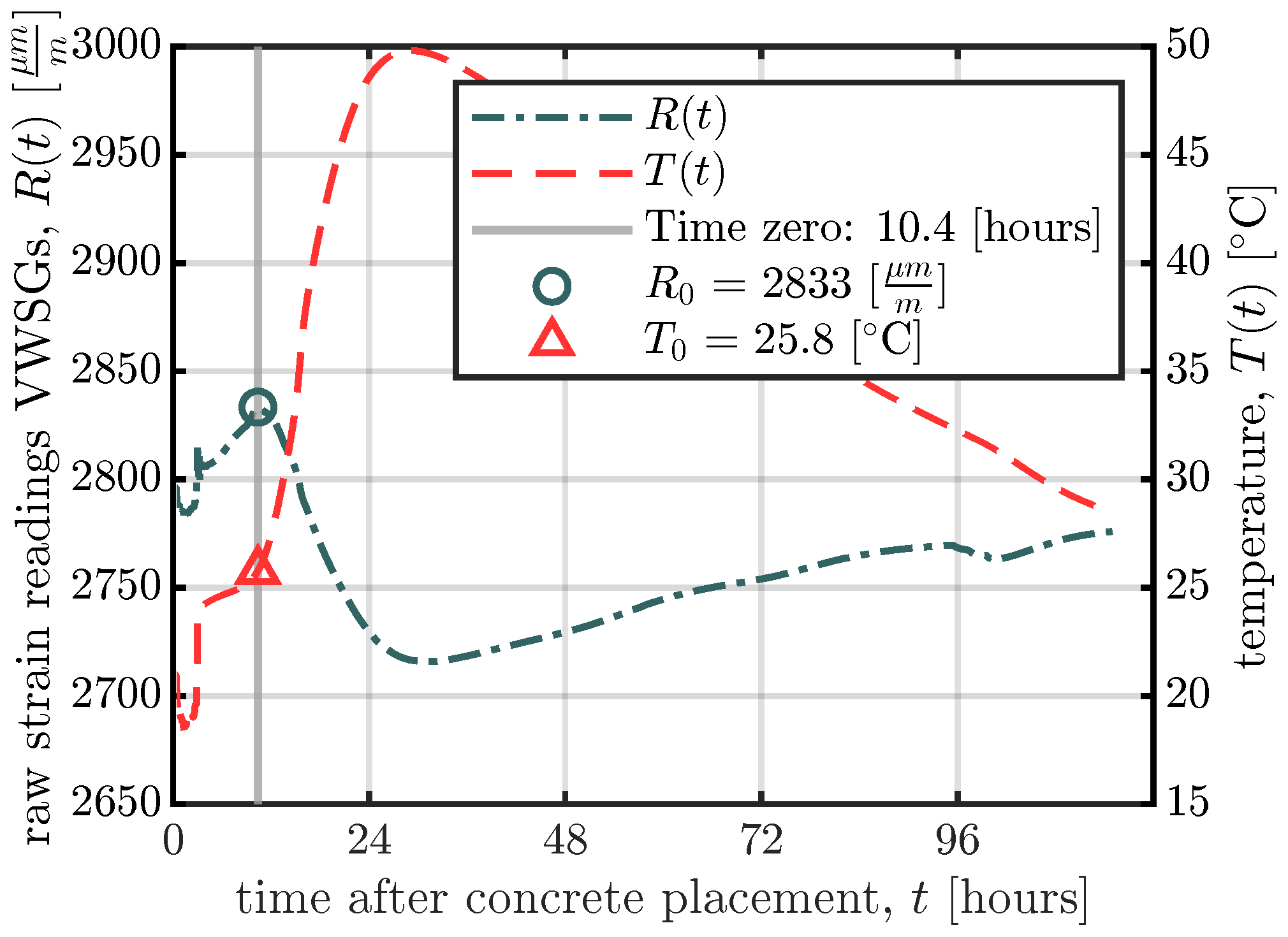
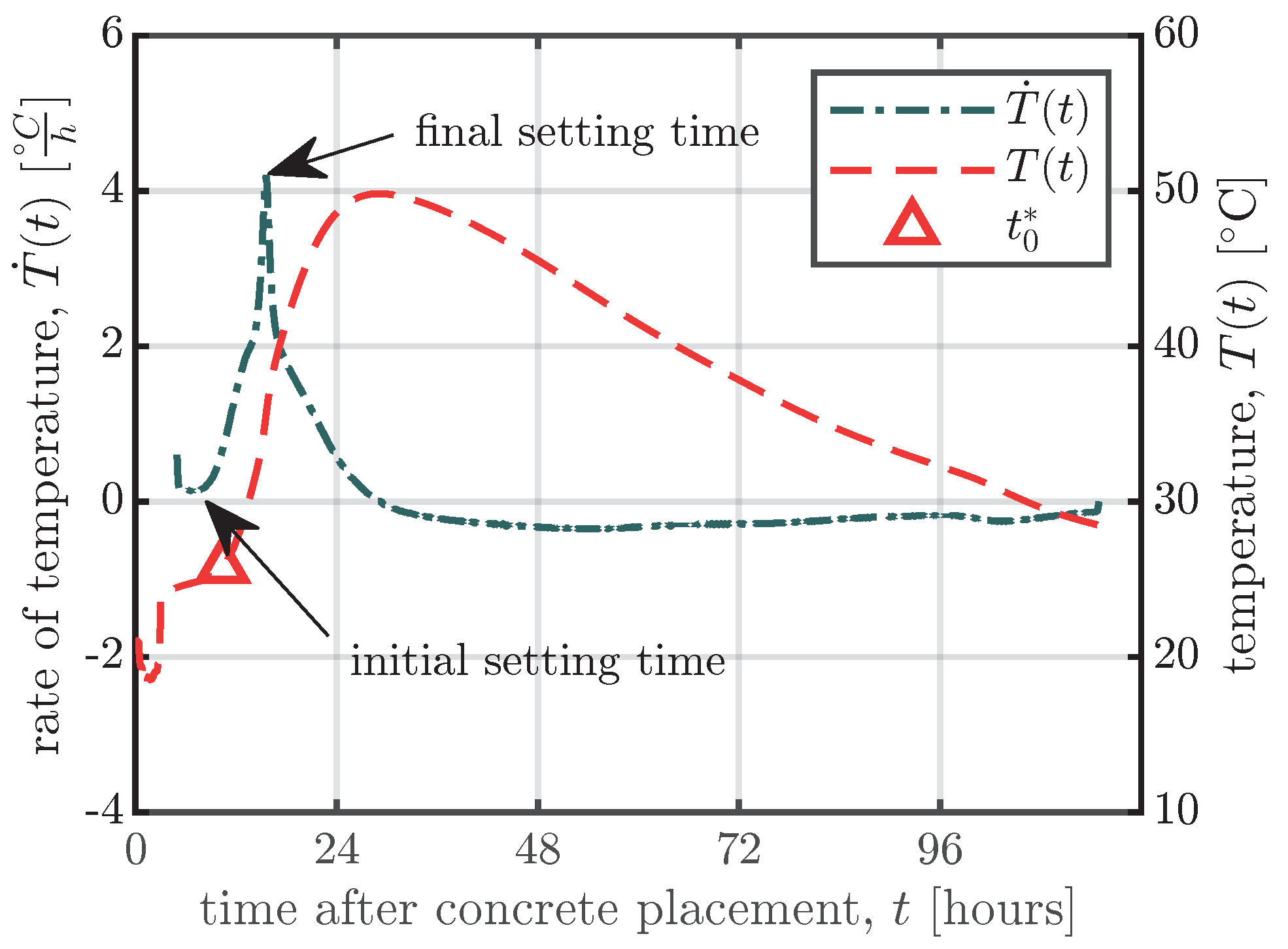
3.1. Determination of Time Zero and Temperature Compensation of the VWSGs
3.2. Separation of the Thermal Strain
4. Results
4.1. Strain Measurements
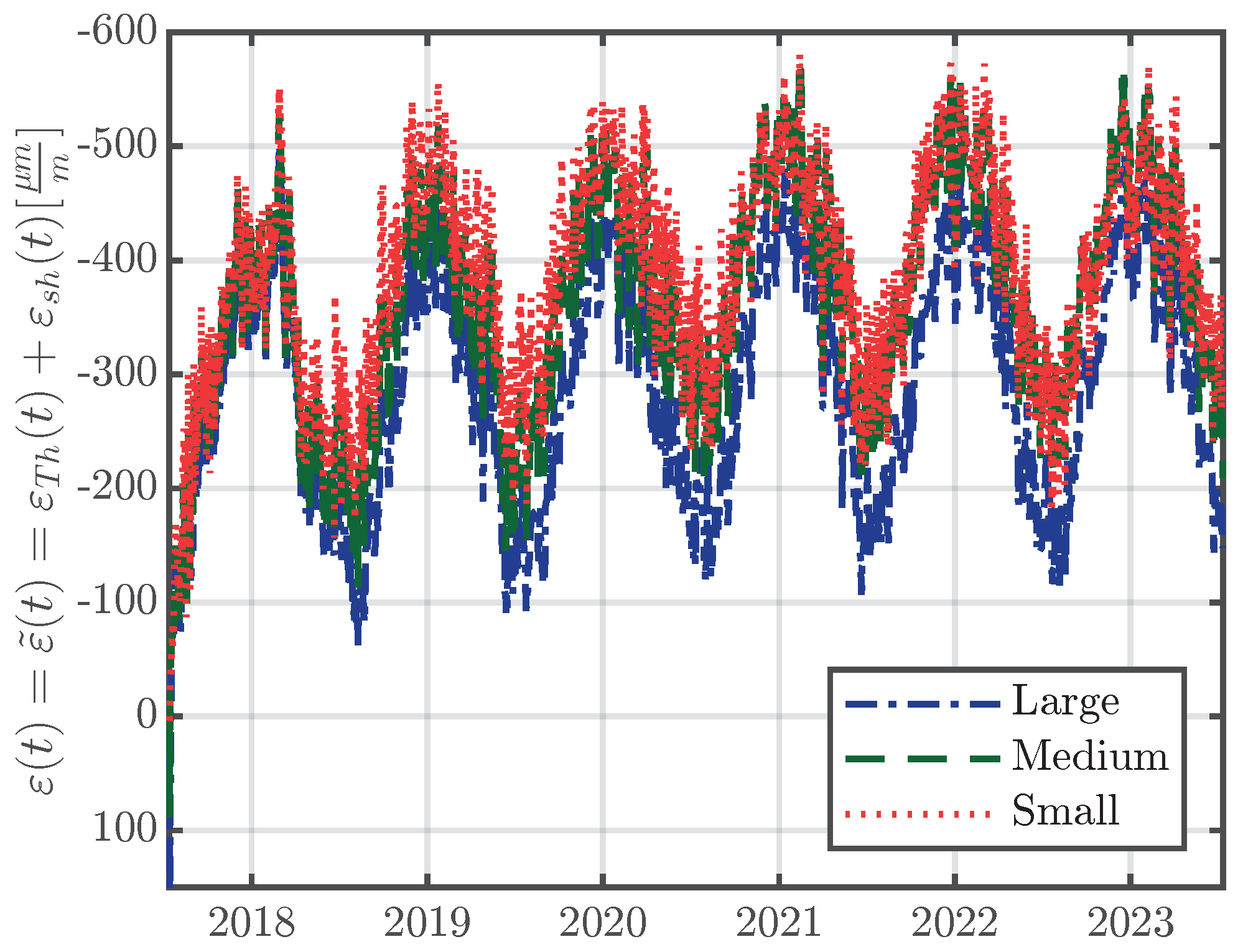
4.2. Shrinkage Strain
5. Discussion
5.1. Measurements and Calculations
5.1.1. Evaluation of Time Zero of the VWSGs
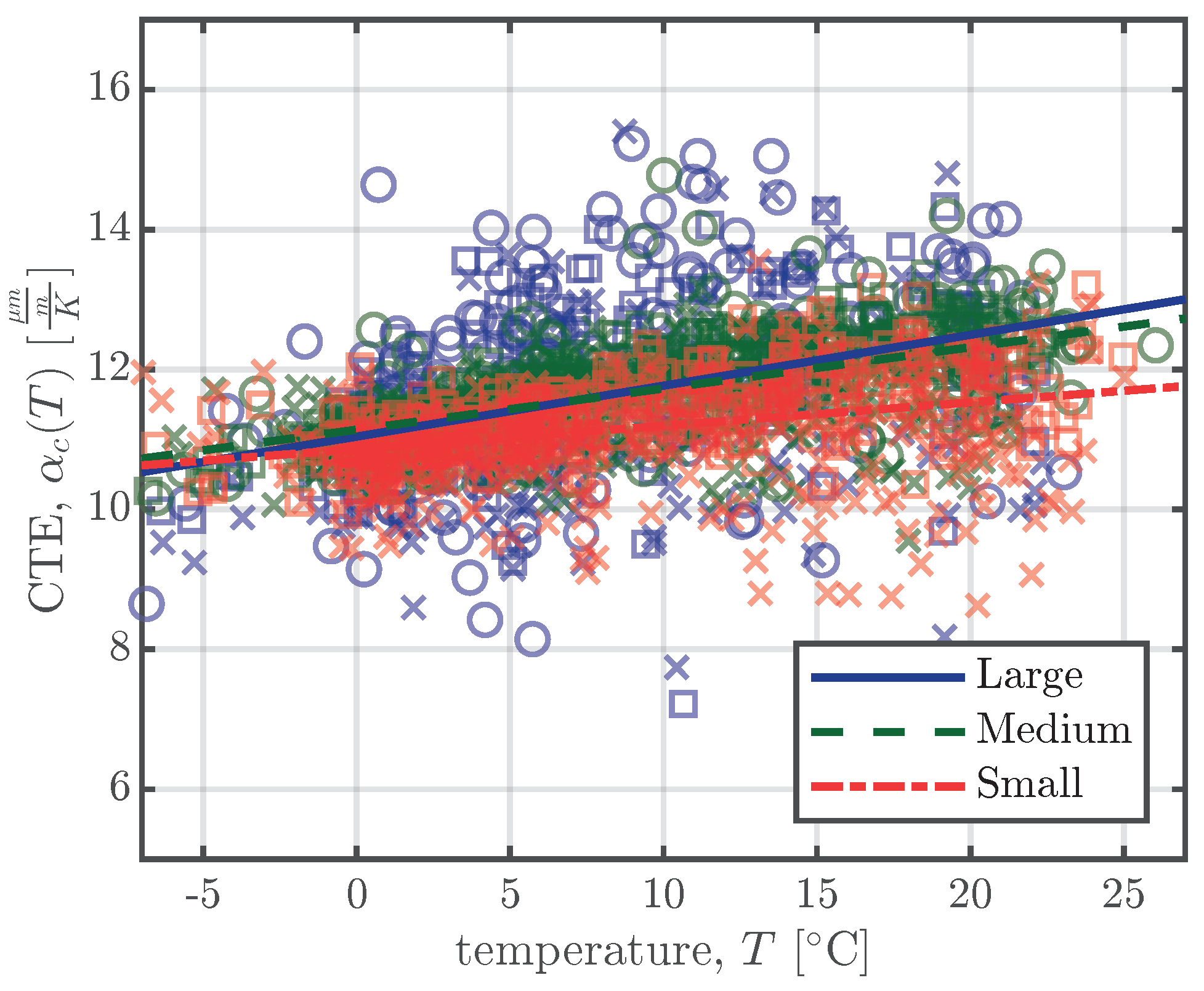
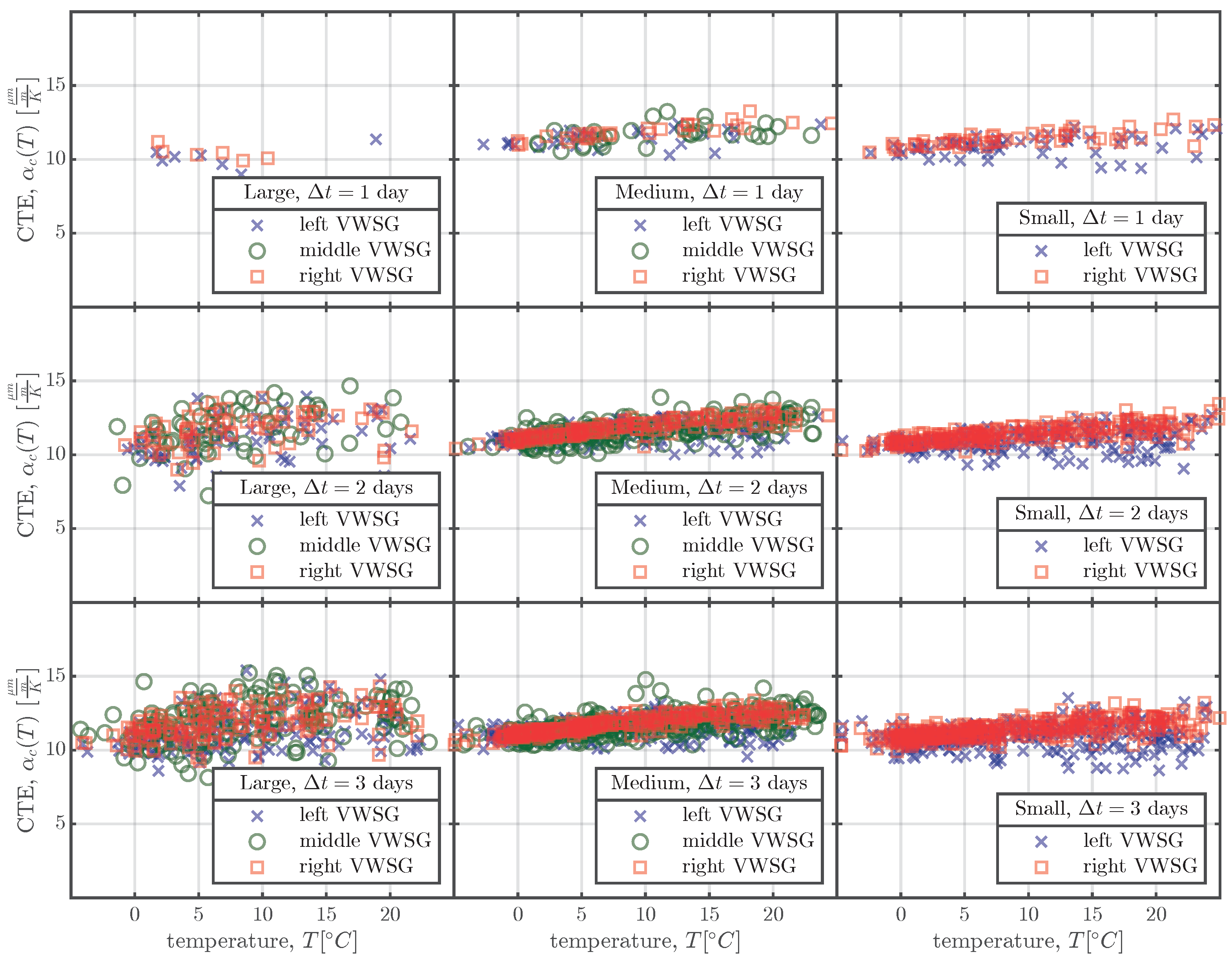
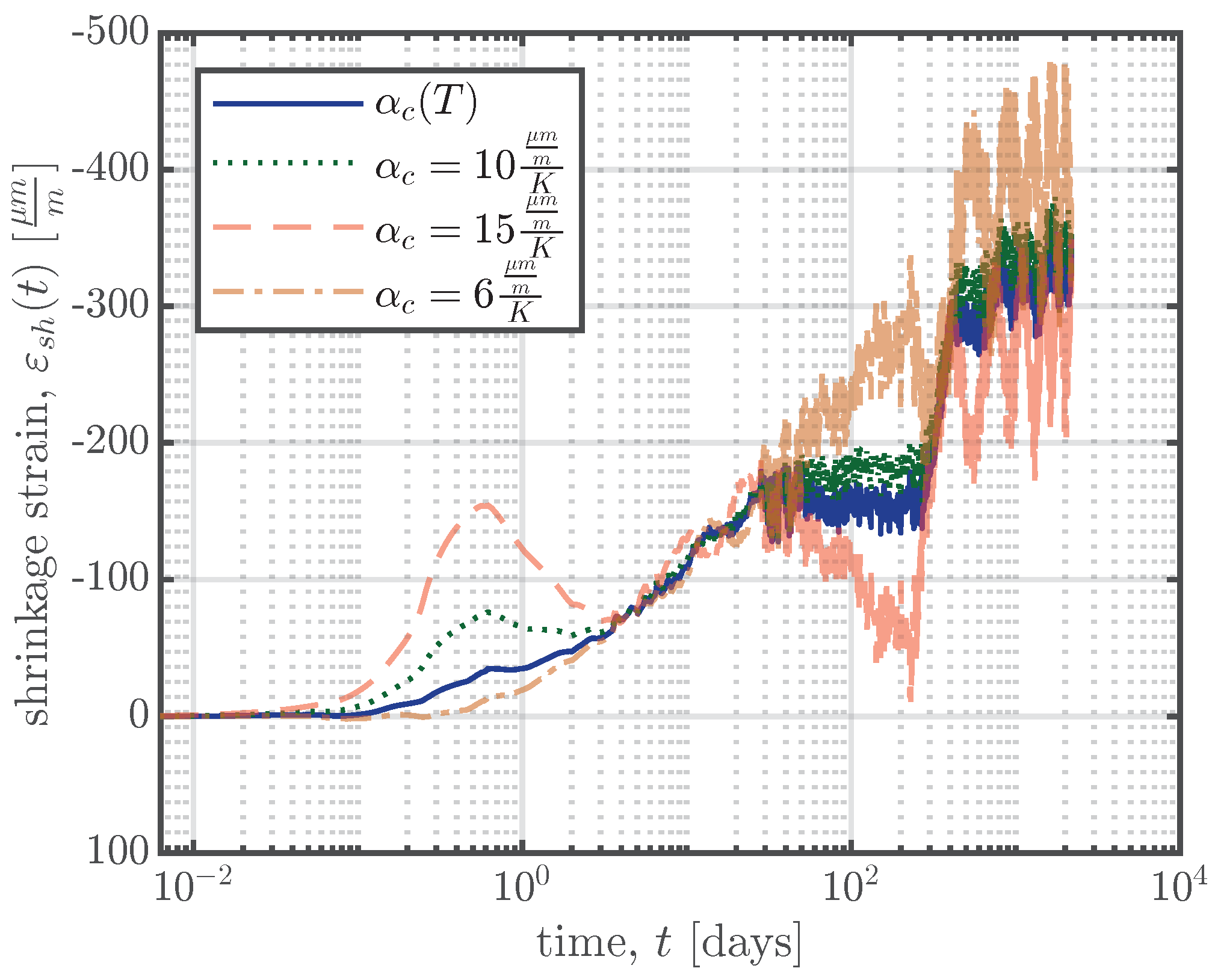
5.1.2. Coefficient of Thermal Expansion
5.1.3. Extensometer Measurements
5.2. Comparison of the Observed Time-Dependent Behaviour with the Predictions of Models from Engineering Societies
- The laboratory measurements, which were performed at a constant temperature and humidity, agree well with the results of both models, with , as indicated in Table 6.
- In the large-scale specimens produced in the summer (S1 and S2), the influence of the specimen size on the measured shrinkage strain was relatively small, contrary to the estimates from the considered models.
- The varying environmental conditions over the first 100 days significantly influenced the rate of shrinkage (decreased shrinkage rate for the summer series (S1 and S2) and increased rate for the winter series S4). This is not reflected by either model.
- As can be seen in Table 6, the results from both models yield unsatisfactory results for the large-scale specimens (especially for series S1), due to the reasons mentioned above, i.e. because the effects of seasonal changes in environmental conditions are not captured by the models.
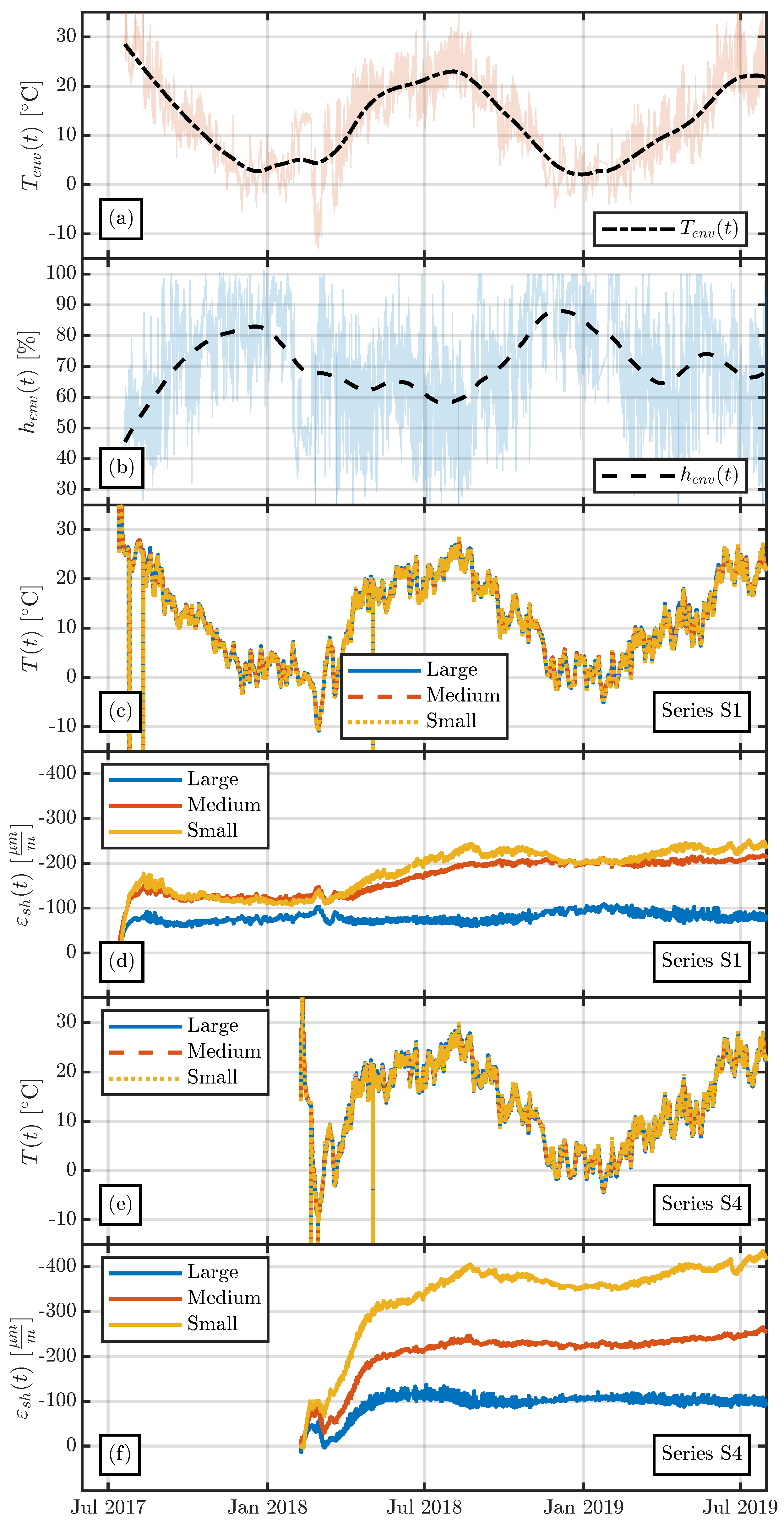
5.3. Seasonal Effects and Influence of the Production Date
- Contrary to the observations of Vandewalle [18], the shrinkage strain of the large-scale specimens did not reach the same shrinkage strain after 2000 days in all specimens, no matter what the production date. The difference between the strains measured in the summer and winter series may be due to the cold winter months during the first year of measurements; see Figure 22.
6. Conclusions
- VWSGs can be used for long-term measurements. In the presented study, VWSGs took strain measurements for over six years and the accuracy of the measurements is confirmed by additional measurements carried out with an extensometer.
- VWSGs allow for early-age concrete strain measurements as soon as the concrete and the sensor start acting compositely (which is some time between the initial and final setting times).
- The coefficient of thermal expansion (CTE) of concrete was back calculated from the measurements of the VWSGs. Two different calculation procedures were used to determine the CTE at an early age and during the whole measurement period.
- The CTE calculated for the whole measurement period shows a dependence on the measured concrete temperature. The calculated CTE values increase with increasing temperature.
- The measured shrinkage strains of the large-scale specimens and the results from analytical models provided by engineering societies (fib and RILEM) did not agree well with each other. Since the models do not capture the influence of changing environmental conditions, the measured shrinkage strains of the large-scale specimens were not predicted accurately by the models. Shrinkage strains from the tests carried out in the laboratory agreed well with the results from the models, since the shrinkage tests were performed under constant environmental conditions ( °C and ).
- The influence of the production date on the shrinkage strains of the large-scale specimens was investigated. One test series was produced during the summer and a second series with the same concrete mixture was produced in the winter. The measurements show that the evolution of the shrinkage strains significantly differs because of the different production dates. During the first measurement year, the shrinkage strains of the specimens which were produced in winter developed faster and achieved nearly four times the value of the measured shrinkage strains of the specimens which were produced in summer.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Shrinkage Data
| Composition I | Composition II | ||
|---|---|---|---|
| (day) | () | (day) | () |
| 0.02 | 1.06 | 0.02 | 0.06 |
| 0.04 | 1.84 | 0.04 | 0.27 |
| 0.08 | −0.59 | 0.08 | −0.56 |
| 0.17 | −0.42 | 0.17 | −2.00 |
| 0.34 | −5.23 | 0.34 | −4.44 |
| 0.70 | −12.66 | 0.69 | −10.54 |
| 1.41 | −26.41 | 1.41 | −22.02 |
| 2.87 | −51.09 | 2.85 | −42.69 |
| 5.82 | −92.09 | 5.79 | −77.09 |
| 11.82 | −151.62 | 11.74 | −128.11 |
| 23.98 | −225.27 | 23.81 | −192.08 |
| 48.65 | −297.38 | 48.30 | −256.48 |
| 98.72 | −362.03 | 97.98 | −314.49 |
| 200.33 | −418.16 | 198.75 | −370.78 |
| 406.50 | −450.37 | 403.16 | −409.74 |
| 824.87 | −478.49 | 817.79 | −442.78 |
| Series S1 | Series S2 | Series S4 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| L | M | S | L | M | S | L | M | S | |||
| (day) | () | () | () | (day) | () | () | () | (day) | () | () | () |
| 0.06 | −1.71 | −2.59 | −3.43 | 0.06 | −0.31 | −0.55 | −0.79 | 0.06 | −1.00 | −1.20 | −0.30 |
| 0.08 | −1.94 | −4.42 | −7.82 | 0.08 | −0.51 | −0.94 | −1.74 | 0.08 | −1.60 | −1.46 | −2.80 |
| 0.11 | −2.45 | −4.26 | −8.43 | 0.11 | −0.71 | −1.37 | −2.65 | 0.11 | −2.04 | −1.44 | −2.76 |
| 0.15 | 0.04 | −4.61 | −9.77 | 0.15 | −1.27 | −2.09 | −3.66 | 0.14 | −1.14 | −2.77 | −3.69 |
| 0.20 | −0.68 | −5.56 | −10.97 | 0.20 | −3.58 | −4.80 | −5.56 | 0.19 | 0.19 | −3.16 | −4.51 |
| 0.26 | −3.79 | −7.95 | −14.10 | 0.26 | −2.64 | −6.65 | −6.19 | 0.25 | −0.64 | −3.92 | −5.50 |
| 0.35 | −5.82 | −8.74 | −15.11 | 0.35 | −3.52 | −7.80 | −14.99 | 0.34 | −1.44 | −4.86 | −7.37 |
| 0.47 | −7.00 | −9.55 | −16.69 | 0.47 | −5.02 | −9.37 | −18.50 | 0.45 | −2.35 | −6.07 | −10.27 |
| 0.63 | −8.22 | −10.67 | −18.35 | 0.63 | −5.17 | −9.61 | −22.97 | 0.60 | −5.03 | −7.68 | −14.07 |
| 0.84 | −9.35 | −13.13 | −22.18 | 0.84 | −0.80 | −11.49 | −27.02 | 0.80 | −6.51 | −9.54 | −17.90 |
| 1.13 | −11.05 | −16.76 | −27.54 | 1.12 | −6.30 | −14.71 | −33.94 | 1.07 | −8.14 | −11.86 | −21.72 |
| 1.51 | −15.27 | −18.15 | −26.94 | 1.50 | −8.26 | −21.32 | −41.42 | 1.43 | −11.49 | −14.05 | −27.58 |
| 2.02 | −15.55 | −23.09 | −38.20 | 2.01 | −10.06 | −24.31 | −47.94 | 1.91 | −13.66 | −17.77 | −34.64 |
| 2.70 | −18.76 | −27.47 | −44.22 | 2.69 | −14.13 | −31.59 | −58.49 | 2.56 | −16.97 | −22.10 | −43.00 |
| 3.61 | −22.55 | −33.01 | −51.83 | 3.60 | −15.36 | −36.01 | −64.49 | 3.41 | −21.51 | −27.31 | −53.79 |
| 4.83 | −25.15 | −40.32 | −63.89 | 4.82 | −23.79 | −41.72 | −76.98 | 4.56 | −25.48 | −34.23 | −67.34 |
| 6.46 | −30.03 | −50.08 | −78.96 | 6.45 | −27.64 | −50.11 | −94.89 | 6.09 | −30.50 | −43.45 | −82.84 |
| 8.64 | −35.83 | −64.05 | −97.91 | 8.63 | −34.65 | −58.78 | −111.78 | 8.38 | −34.73 | −58.02 | −100.20 |
| 11.57 | −40.31 | −70.27 | −104.22 | 11.54 | −33.71 | −63.80 | −127.70 | 10.85 | −30.02 | −50.32 | −86.07 |
| 15.48 | −46.10 | −75.80 | −114.42 | 15.44 | −40.83 | −78.32 | −152.13 | 14.49 | −29.48 | −60.39 | −88.29 |
| 20.71 | −42.80 | −83.04 | −129.16 | 20.66 | −37.84 | −73.45 | −131.92 | 19.34 | −18.32 | −34.04 | −74.28 |
| 27.71 | −43.37 | −87.00 | −129.30 | 27.65 | −48.90 | −86.31 | −158.87 | 25.83 | 6.48 | −24.28 | −91.25 |
| 37.07 | −41.07 | −91.99 | −130.56 | 36.99 | −55.10 | −95.10 | −184.18 | 34.49 | −6.02 | −33.70 | −113.15 |
| 49.60 | −30.51 | −75.27 | −109.35 | 49.49 | −54.72 | −90.46 | −181.28 | 46.06 | −22.40 | −69.08 | −161.64 |
| 66.37 | −33.05 | −64.25 | −93.47 | 66.21 | −46.89 | −77.62 | −167.55 | 61.50 | −65.69 | −128.76 | −240.69 |
| 88.81 | −29.43 | −70.75 | −103.35 | 88.59 | −64.87 | −89.36 | −179.60 | 82.12 | −87.71 | −174.24 | −299.64 |
| 118.83 | −40.15 | −68.57 | −88.32 | 118.53 | −69.63 | −84.70 | −170.93 | 109.65 | −98.57 | −188.51 | −317.50 |
| 159.00 | −36.89 | −63.18 | −85.82 | 158.59 | −76.96 | −89.01 | −170.37 | 146.41 | −105.99 | −202.09 | −353.06 |
| 212.74 | −51.33 | −73.25 | −89.66 | 212.18 | −87.10 | −90.23 | −166.94 | 195.50 | −89.79 | −211.16 | −389.34 |
| 284.66 | −35.29 | −86.81 | −133.86 | 283.89 | −72.12 | −94.60 | −211.60 | 261.04 | −77.92 | −212.04 | −377.70 |
| 380.88 | −35.94 | −137.32 | −191.31 | 379.83 | −109.89 | −119.14 | −268.84 | 348.57 | −100.26 | −207.42 | −353.53 |
| 509.63 | −54.48 | −141.85 | −172.29 | 508.19 | −154.28 | −131.19 | −251.79 | 465.44 | −96.20 | −218.70 | −388.45 |
| 681.91 | −47.20 | −154.92 | −200.18 | 679.94 | −129.73 | −123.60 | −269.33 | 621.49 | −103.95 | −243.43 | −397.52 |
| 912.41 | −89.54 | −174.74 | −188.47 | 909.72 | −185.47 | −144.83 | −270.50 | 829.86 | −90.43 | −259.70 | −438.50 |
| 1220.84 | −91.71 | −165.43 | −166.61 | 1217.17 | −195.27 | −159.00 | −297.28 | 1108.10 | −110.09 | −255.96 | −375.40 |
| 1633.53 | −93.04 | −178.57 | −189.24 | 1628.51 | −206.05 | −185.20 | −305.44 | 1479.62 | −124.93 | −285.69 | −421.24 |
| 2185.73 | −69.07 | −183.58 | −230.96 | 2178.86 | −194.98 | −185.11 | −339.78 | 1975.71 | −109.30 | −311.60 | −466.18 |
References
- Müller, H.S.; Anders, I.; Breiner, R.; Vogel, M. Concrete: Treatment of types and properties in fib Model Code 2010. Struct. Concr. 2013, 14, 320–334. [Google Scholar] [CrossRef]
- Müller, H.S.; Kvitsel, V. Kriechen und Schwinden von Beton. Beton Stahlbetonbau 2002, 97, 8–19. [Google Scholar] [CrossRef]
- Müller, H.S.; Acosta, F.; Kvitsel, V. Modelle zur Vorhersage des Schwindens und Kriechens von Beton—Teil 1: Analyse des Schwindmodells in DIN EN 1992-1-1:2011 und neuer Ansatz im Eurocode 2 prEN 1992-1-1:2020. Beton Stahlbetonbau 2021, 116, 2–18. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Baweja, S. Creep and Shrinkage Prediction Model for Analysis and Design of Concrete Structures: Model B3. Mater. Constr. 1995, 28, 357–365. [Google Scholar]
- Bažant, Z.P.; Hubler, M.H.; Wendner, R. Model B4 for creep, drying shrinkage and autogenous shrinkage of normal and high-strength concretes with multi-decade applicability. Mater. Struct. 2015, 48, 753–770. [Google Scholar] [CrossRef]
- CEN (European Committee for Standardization). Eurocode 2: Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings; CEN: Brussels, Belgium, 2014. [Google Scholar]
- ACI (American Concrete Institut). Guide for Modeling and Calculating Shrinkage and Creep in Hardened Concrete; ACI: Farmington Hills, MI, USA, 2008. [Google Scholar]
- NU Database of Laboratory Creep and Shrinkage Data. 2021. Available online: http://www.civil.northwestern.edu/people/bazant/downloads.html (accessed on 20 July 2023).
- Bažant, Z.P.; Li, G.H. Comprehensive Database on Concrete Creep and Shrinkage. Aci Mater. J. 2008, 105, 635–637. [Google Scholar] [CrossRef]
- Hubler, M.H.; Wendner, R.; Bažant, Z.P. Comprehensive Database for Concrete Creep and Shrinkage: Analysis and Recommendations for Testing and Recording. Aci Mater. J. 2015, 112, 547–558. [Google Scholar] [CrossRef]
- Šmilauer, V.; Havlásek, P.; Dohnalová, L.; Wan-Wendner, R.; Bažant, Z.P. Revamp of Creep and Shrinkage NU Database. In Proceedings of the The Biot-Bažant Conference, Evanston, IL, USA, 1–3 June 2021. [Google Scholar] [CrossRef]
- Hubler, M.H.; Wendner, R.; Bažant, Z.P. Statistical justification of Model B4 for drying and autogenous shrinkage of concrete and comparisons to other models. Mater. Struct. 2015, 48, 797–814. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Nguyen, H.T.; Dönmez, A.A. Scaling in size, time and risk—The problem of huge extrapolations and remedy by asymptotic matching. J. Mech. Phys. Solids 2022, 170, 105094. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Donmez, A. Extrapolation of Test Data in Time, Size and Risk: A Challenge for Concrete Design Codes. In Proceedings of the IABSE Symposium Prague, 2022: Challenges for Existing and Oncoming Structures, Prague, Czech Republic, 25–27 May 2022; pp. 54–66. [Google Scholar]
- Kolínský, V.; Vítek, J.L. Verification of numerical creep and shrinkage models in an arch bridge analysis. Struct. Concr. 2019, 20, 2030–2041. [Google Scholar] [CrossRef]
- Herbers, M.; Wenner, M.; Marx, S. A 576 m long creep and shrinkage specimen—Long-term deformation of a semi-integral concrete bridge with a massive solid cross-section. Struct. Concr. 2023, 24, 3558–3572. [Google Scholar] [CrossRef]
- Barr, B.I.G.; Vitek, J.L.; Beygi, M.A. Seasonal shrinkage variation in bridge segments. Mater. Struct. 1997, 30, 106–111. [Google Scholar] [CrossRef]
- Vandewalle, L. Concrete creep and shrinkage at cyclic ambient conditions. Cem. Concr. Compos. 2000, 22, 201–208. [Google Scholar] [CrossRef]
- Ge, Y.; Elshafie, M.Z.E.B.; Dirar, S.; Middleton, C.R. The response of embedded strain sensors in concrete beams subjected to thermal loading. Constr. Build. Mater. 2014, 70, 279–290. [Google Scholar] [CrossRef]
- Jeong, J.H.; Zollinger Dan, G.; Lim, J.S.; Park, J.Y. Age and Moisture Effects on Thermal Expansion of Concrete Pavement Slabs. J. Mater. Civ. Eng. 2012, 24, 8–15. [Google Scholar] [CrossRef]
- Yeon, J.H.; Choi, S.; Won, M.C. In situ measurement of coefficient of thermal expansion in hardening concrete and its effect on thermal stress development. Constr. Build. Mater. 2013, 38, 306–315. [Google Scholar] [CrossRef]
- Yeon, J.H.; Choi, S.; Won, M.C. Evaluation of zero-stress temperature prediction model for Portland cement concrete pavements. Constr. Build. Mater. 2013, 40, 492–500. [Google Scholar] [CrossRef]
- Guo, T.; Chen, Z.; Lu, S.; Yao, R. Monitoring and analysis of long-term prestress losses in post-tensioned concrete beams. Measurement 2018, 122, 573–581. [Google Scholar] [CrossRef]
- Choi, S. Internal relative humidity and drying shrinkage of hardening concrete containing lightweight and normal-weight coarse aggregates: A comparative experimental study and modeling. Constr. Build. Mater. 2017, 148, 288–296. [Google Scholar] [CrossRef]
- Suza, D. Einfluß des Maßstabseffekts und der Umgebungsbedingungen auf das Kriechen und Schwinden von Beton. Ph.D. Thesis, TU Wien, Vienna, Austria, 2020. [Google Scholar] [CrossRef]
- Fédération internationale du béton (fib). fib Model Code for Concrete Structures 2010; Ernst & Sohn: Berlin, Germany, 2013. [Google Scholar] [CrossRef]
- EN 12390-13:2014; CEN (European Committee for Standardization). Testing Hardened Concrete—Part 13: Determination of Secant Modulus of Elasticity in Compression. CEN: Brussels, Belgium, 2014.
- EN 12390-3:2009; CEN (European Committee for Standardization). Testing Hardened Concrete—Part 3: Compressive Strength of Test Specimens. CEN: Brussels, Belgium, 2009.
- EN 12390-7:2009; CEN (European Committee for Standardization). Testing Hardened Concrete—Part 7: Density of Hardened Concrete. CEN: Brussels, Belgium, 2009.
- Geokon, Model 4200 Vibrating Vire Strain Gauges Instruction Manual. 2019. Available online: https://www.geokon.com/4200-Series (accessed on 20 July 2023).
- Nam, J.H.; Kim, D.H.; Choi, S.; Won, M.C. Variation of Crack Width over Time in Continuously Reinforced Concrete Pavement. Transp. Res. Rec. 2007, 2037, 3–11. [Google Scholar] [CrossRef]
- Mang, H.A.; Hofstetter, G. Festigkeitslehre, 5th ed.; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Jirásek, M. Creep and hygrothermal effects in concrete structures. In Solid Mechanics and its Applications; Springer: Dordrecht, The Netherlands, 2018; Volume 225, pp. 1–921. [Google Scholar] [CrossRef]
- ASTM (American Society for Testing and Materials). Standard Test Method for Time of Setting of Concrete Mixtures by Penetration Resistance; ASTM: Philadelphia, PA, USA, 2008. [Google Scholar]
- Cusson, D.; Hoogeveen, T. An experimental approach for the analysis of early-age behaviour of high-performance concrete structures under restrained shrinkage. Cem. Concr. Res. 2007, 37, 200–209. [Google Scholar] [CrossRef]
- EN 196-3:2005+A1:2008; CEN (European Committee for Standardization). Methods of Testing Cement—Part 3: Determination of Setting Times and Soundness. CEN: Brussels, Belgium, 2008.
- Meyer, S.L. Thermal Expansion Characteristics of Hardened Cement Paste and of Concrete. In Proceedings of the Thirtieth Annual Meeting of the Highway Research Board, Washington, DC, USA, 9–12 January 1951; Volume 30, pp. 193–203. [Google Scholar]
- Grasley, Z.C.; Lange, D.A. Thermal dilation and internal relative humidity of hardened cement paste. Mater. Struct. 2007, 40, 311–317. [Google Scholar] [CrossRef]
- Aili, A.; Maruyama, I.; Vandamme, M. Thermal Expansion of Cement Paste at Various Relative Humidities after Long-term Drying: Experiments and Modeling. J. Adv. Concr. Technol. 2023, 21, 151–165. [Google Scholar] [CrossRef]
- Bjøntegaard, Ø.; Sellevold, E.J. Interaction between thermal dilation and autogenous deformation in high performance concrete. Mater. Struct. 2001, 34, 266–272. [Google Scholar] [CrossRef]
- Sellevold, E.J.; Bjøntegaard, Ø. Coefficient of thermal expansion of cement paste and concrete: Mechanisms of moisture interaction. Mater. Struct. 2006, 39, 809–815. [Google Scholar] [CrossRef]
- Wyrzykowski, M.; Lura, P. Moisture dependence of thermal expansion in cement-based materials at early ages. Cem. Concr. Res. 2013, 53, 25–35. [Google Scholar] [CrossRef]
- Ulm, F.J.; Coussy, O. What is a “massive” concrete structure at early ages? Some dimensional arguments. J. Eng. Mech. 2001, 127, 512–522. [Google Scholar] [CrossRef]
- Wyrzykowski, M.; Lura, P. Controlling the coefficient of thermal expansion of cementitious materials—A new application for superabsorbent polymers. Cem. Concr. Compos. 2013, 35, 49–58. [Google Scholar] [CrossRef]
- Zahabizadeh, B.; Edalat-Behbahani, A.; Granja, J.; Gomes, J.G.; Faria, R.; Azenha, M. A new test setup for measuring early age coefficient of thermal expansion of concrete. Cem. Concr. Compos. 2019, 98, 14–28. [Google Scholar] [CrossRef]
- Loser, R.; Münch, B.; Lura, P. A volumetric technique for measuring the coefficient of thermal expansion of hardening cement paste and mortar. Cem. Concr. Res. 2010, 40, 1138–1147. [Google Scholar] [CrossRef]
- Kada, H.; Lachemi, M.; Petrov, N.; Bonneau, O.; Aïtcin, P.C. Determination of the coefficient of thermal expansion of high performance concrete from initial setting. Mater. Struct. 2002, 35, 35–41. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Baweja, S. Justification and refinements of model B3 for concrete creep and shrinkage 1. statistics and sensitivity. Mater. Struct. 1995, 28, 415–430. [Google Scholar] [CrossRef]
- Barr, B.; Hoseinian, S.B.; Beygi, M.A. Shrinkage of concrete stored in natural environments. Cem. Concr. Compos. 2003, 25, 19–29. [Google Scholar] [CrossRef]
- Kockal, N.U.; Turker, F. Effect of environmental conditions on the properties of concretes with different cement types. Constr. Build. Mater. 2007, 21, 634–645. [Google Scholar] [CrossRef]
- Asamoto, S.; Ohtsuka, A.; Kuwahara, Y.; Miura, C. Study on effects of solar radiation and rain on shrinkage, shrinkage cracking and creep of concrete. Cem. Concr. Res. 2011, 41, 590–601. [Google Scholar] [CrossRef]
- Müller, H.S.; Pristl, M. Creep and shrinkage of concrete at variable ambient conditions. In Proceedings of the Fifth International RILEM Symposium on Creep and Shrinkage of Concrete (ConCreep 5), Barcelona, Spain, 6–9 September 1993; Bažant, Z.P., Carol, I., Eds.; E & FN Spon: London, UK, 1993; pp. 15–26. [Google Scholar]
 ) with the large-scale specimens presented in this paper (
) with the large-scale specimens presented in this paper ( ) with respect to test duration, specimen size, and specimen shape.
) with respect to test duration, specimen size, and specimen shape.
 ) with the large-scale specimens presented in this paper (
) with the large-scale specimens presented in this paper ( ) with respect to test duration, specimen size, and specimen shape.
) with respect to test duration, specimen size, and specimen shape.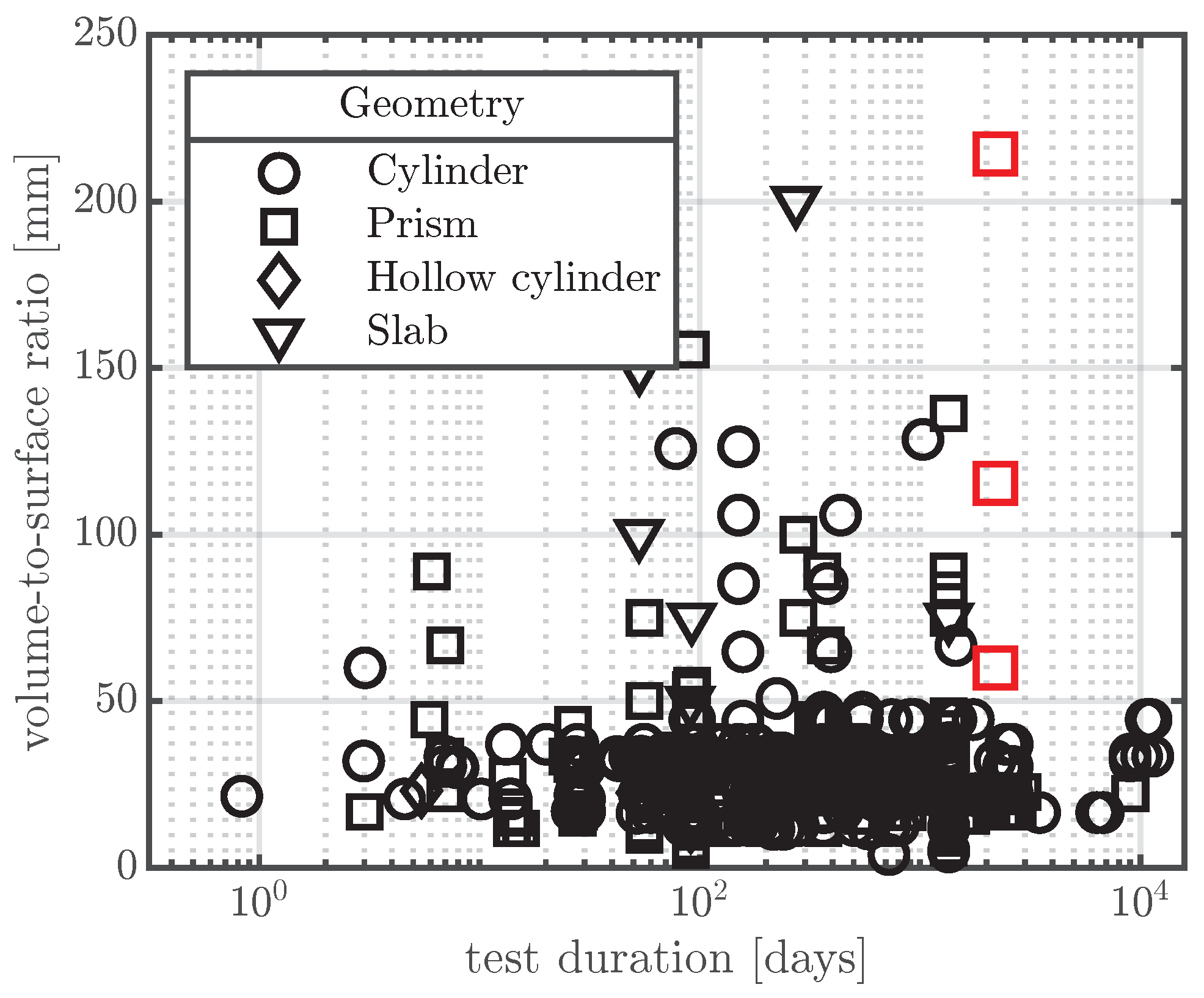

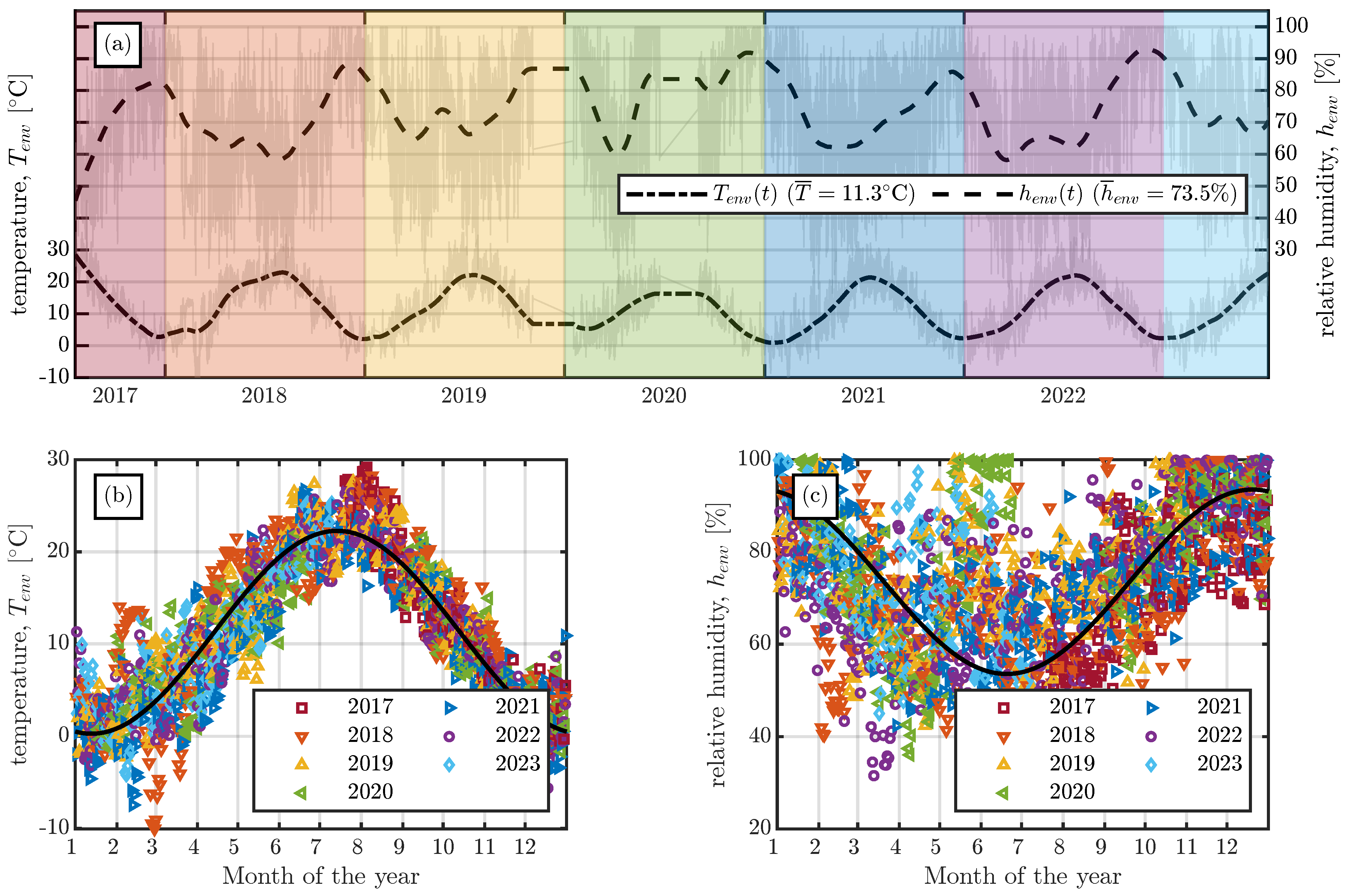


 ) and temperatures (
) and temperatures ( ) inside the specimens of series S1. The time of formwork removal and the time of transportation to their final storage are represented by the vertical dashed (
) inside the specimens of series S1. The time of formwork removal and the time of transportation to their final storage are represented by the vertical dashed ( ) and dash-dotted (
) and dash-dotted ( ) lines, respectively.
) lines, respectively.
 ) and temperatures (
) and temperatures ( ) inside the specimens of series S1. The time of formwork removal and the time of transportation to their final storage are represented by the vertical dashed (
) inside the specimens of series S1. The time of formwork removal and the time of transportation to their final storage are represented by the vertical dashed ( ) and dash-dotted (
) and dash-dotted ( ) lines, respectively.
) lines, respectively.
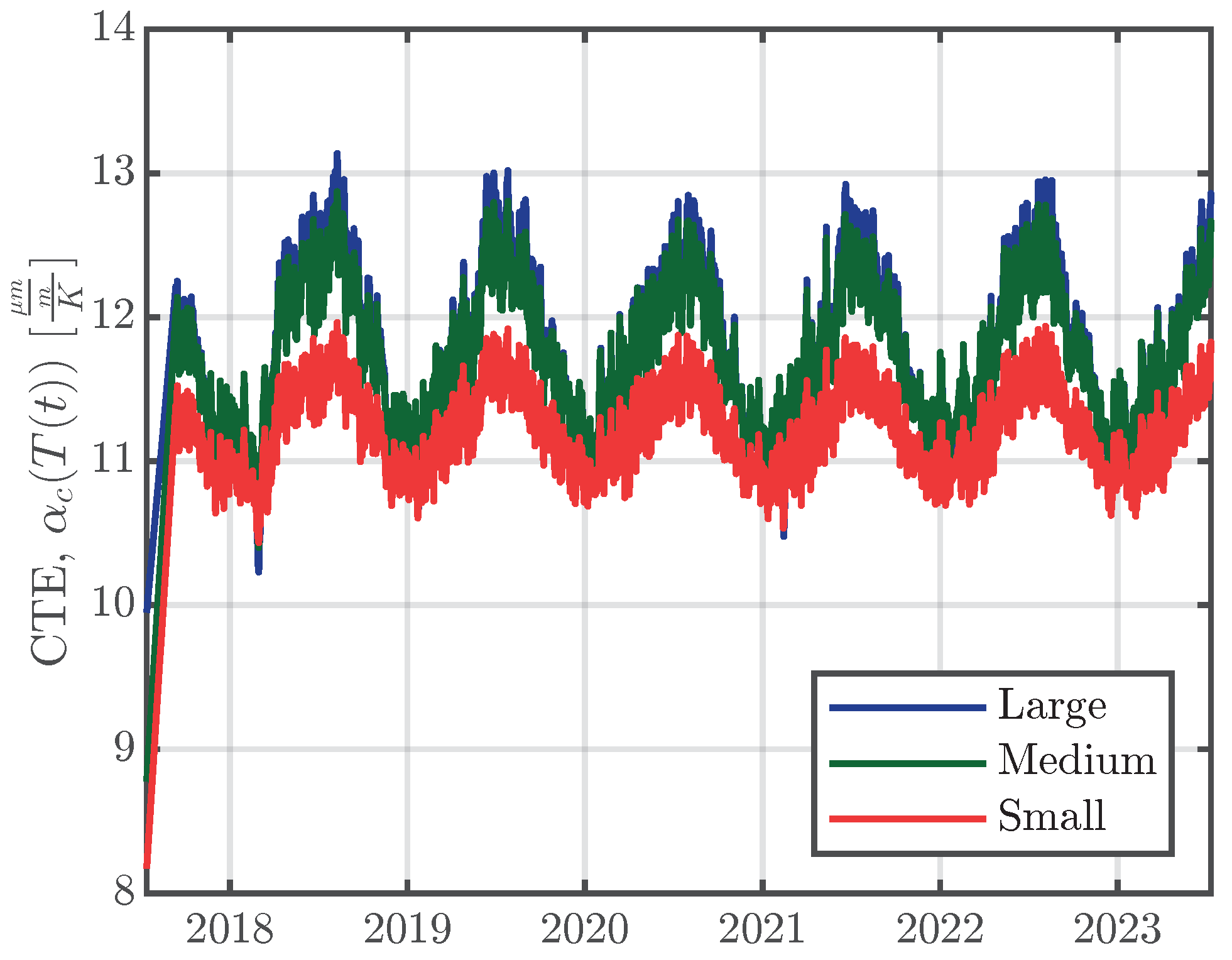

 ), middle (
), middle ( ), and right-hand (
), and right-hand ( ) VWSGs of all specimens.
) VWSGs of all specimens.
 ), middle (
), middle ( ), and right-hand (
), and right-hand ( ) VWSGs of all specimens.
) VWSGs of all specimens.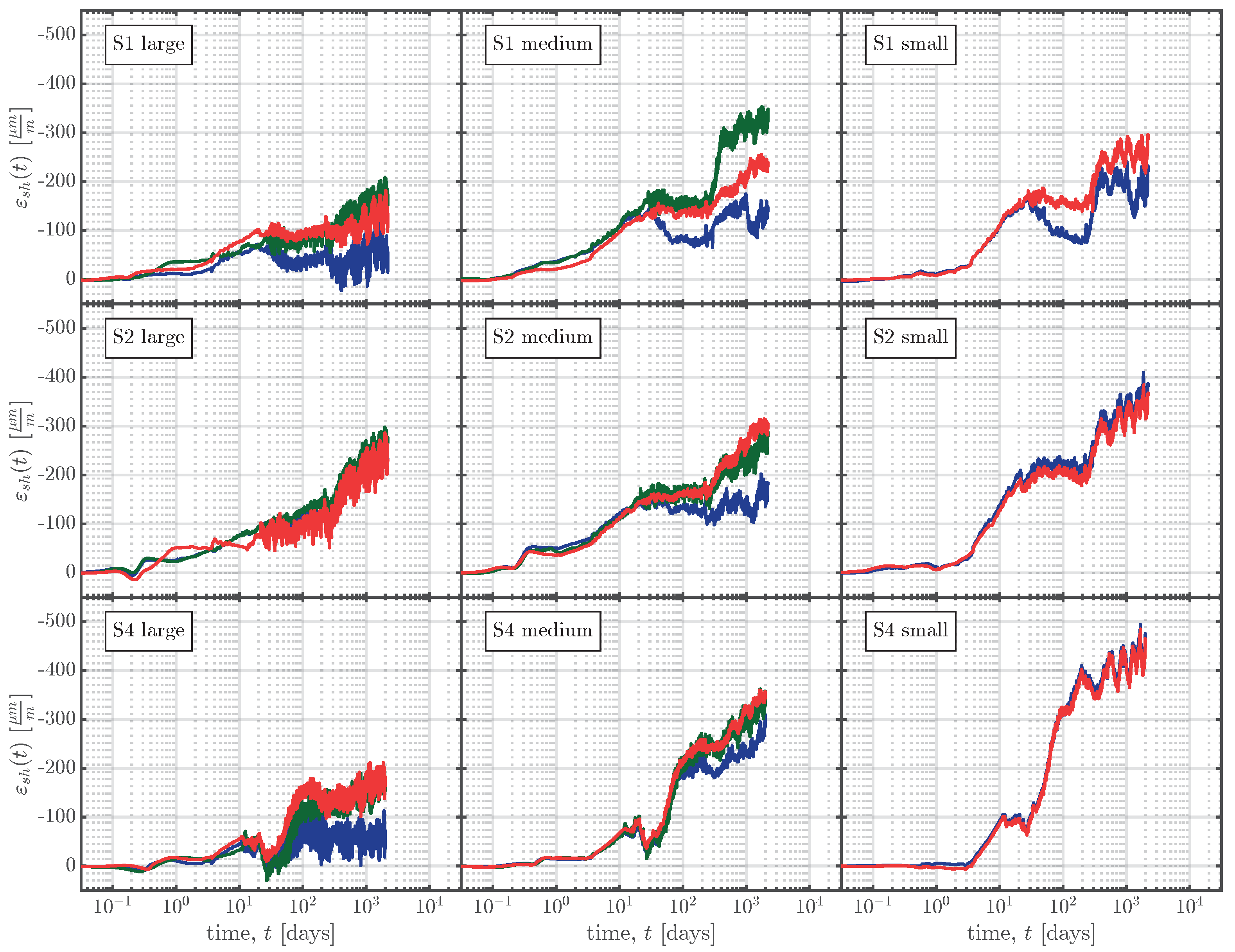

 ) represent the measurements of the VWSG/extensometer on the left side/surface of each specimen and the red measurements (
) represent the measurements of the VWSG/extensometer on the left side/surface of each specimen and the red measurements ( ) represent the measurements on the right side/surface of each specimen, as shown in Figure 2. (a) Large specimens of series S1. (b) Medium specimens of series S1. (c) Small specimens of series S1.
) represent the measurements on the right side/surface of each specimen, as shown in Figure 2. (a) Large specimens of series S1. (b) Medium specimens of series S1. (c) Small specimens of series S1.
 ) represent the measurements of the VWSG/extensometer on the left side/surface of each specimen and the red measurements (
) represent the measurements of the VWSG/extensometer on the left side/surface of each specimen and the red measurements ( ) represent the measurements on the right side/surface of each specimen, as shown in Figure 2. (a) Large specimens of series S1. (b) Medium specimens of series S1. (c) Small specimens of series S1.
) represent the measurements on the right side/surface of each specimen, as shown in Figure 2. (a) Large specimens of series S1. (b) Medium specimens of series S1. (c) Small specimens of series S1.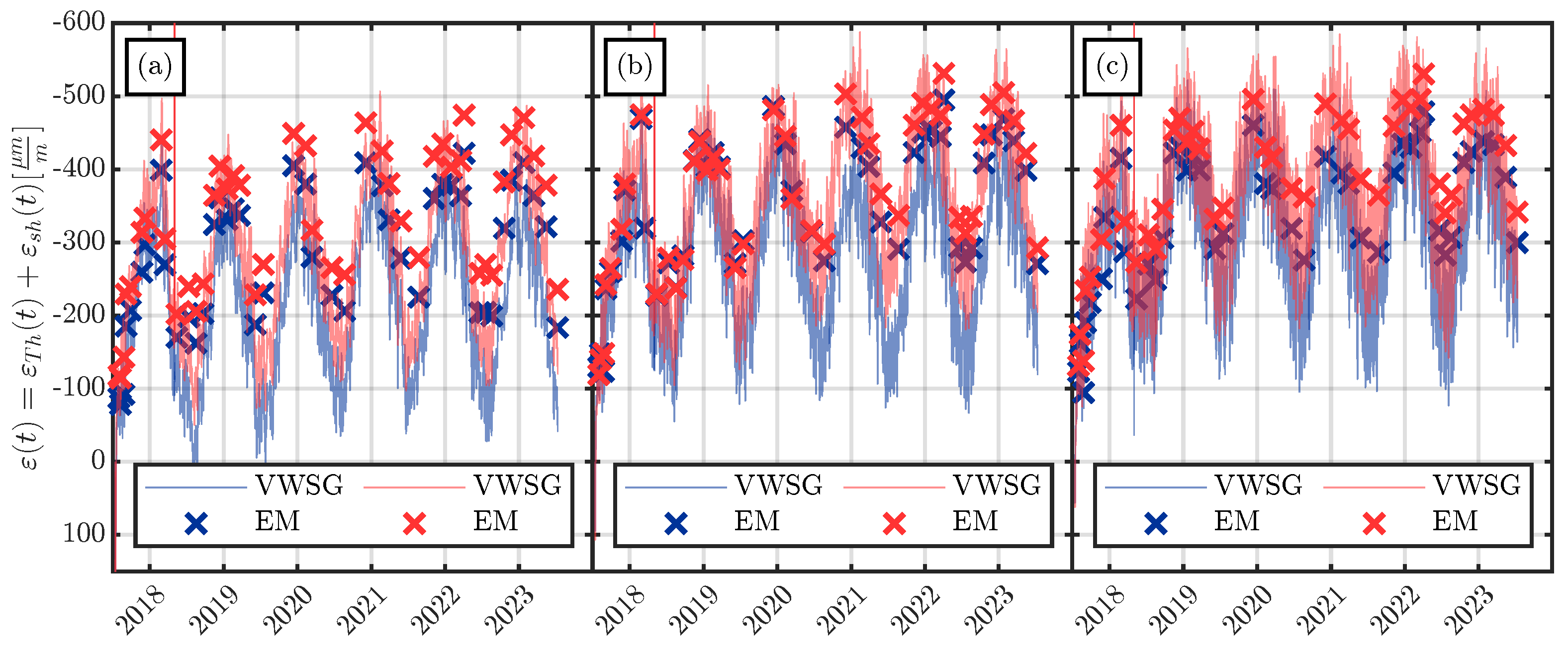
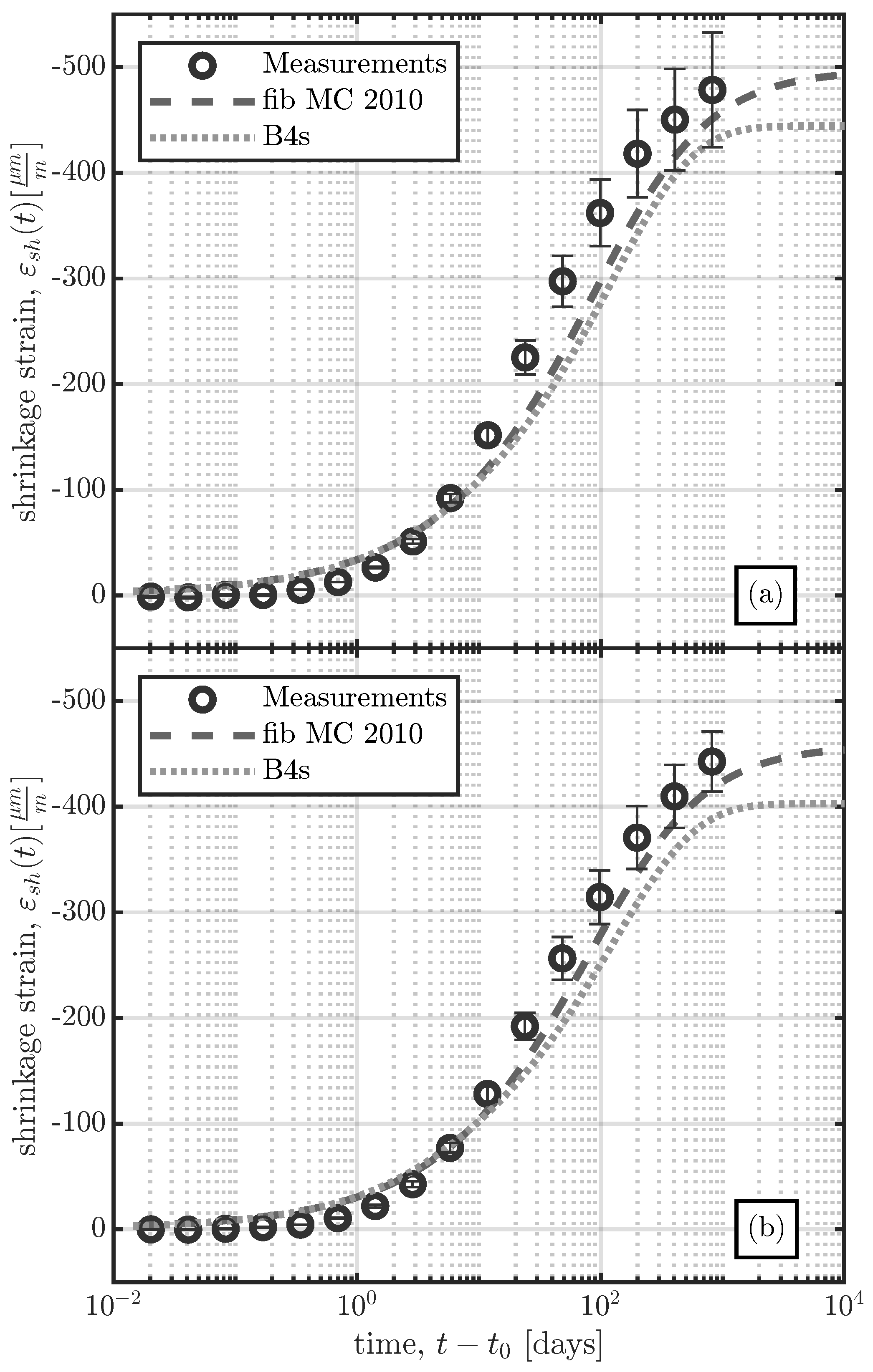
 ) compared with the results of the fib MC 2010 [26] (
) compared with the results of the fib MC 2010 [26] ( ) and B4s [5] (
) and B4s [5] ( ) models. The comparison is fulfilled for all the specimens. The specimen size is indicated by different colours; therefore, the large-sized specimens in blue (
) models. The comparison is fulfilled for all the specimens. The specimen size is indicated by different colours; therefore, the large-sized specimens in blue ( ), the medium-sized specimens in orange (
), the medium-sized specimens in orange ( ), and the small-sized specimens in yellow (
), and the small-sized specimens in yellow ( ). (a) Series S1. (b) Series S2. (c) Series S4.
). (a) Series S1. (b) Series S2. (c) Series S4.
 ) compared with the results of the fib MC 2010 [26] (
) compared with the results of the fib MC 2010 [26] ( ) and B4s [5] (
) and B4s [5] ( ) models. The comparison is fulfilled for all the specimens. The specimen size is indicated by different colours; therefore, the large-sized specimens in blue (
) models. The comparison is fulfilled for all the specimens. The specimen size is indicated by different colours; therefore, the large-sized specimens in blue ( ), the medium-sized specimens in orange (
), the medium-sized specimens in orange ( ), and the small-sized specimens in yellow (
), and the small-sized specimens in yellow ( ). (a) Series S1. (b) Series S2. (c) Series S4.
). (a) Series S1. (b) Series S2. (c) Series S4.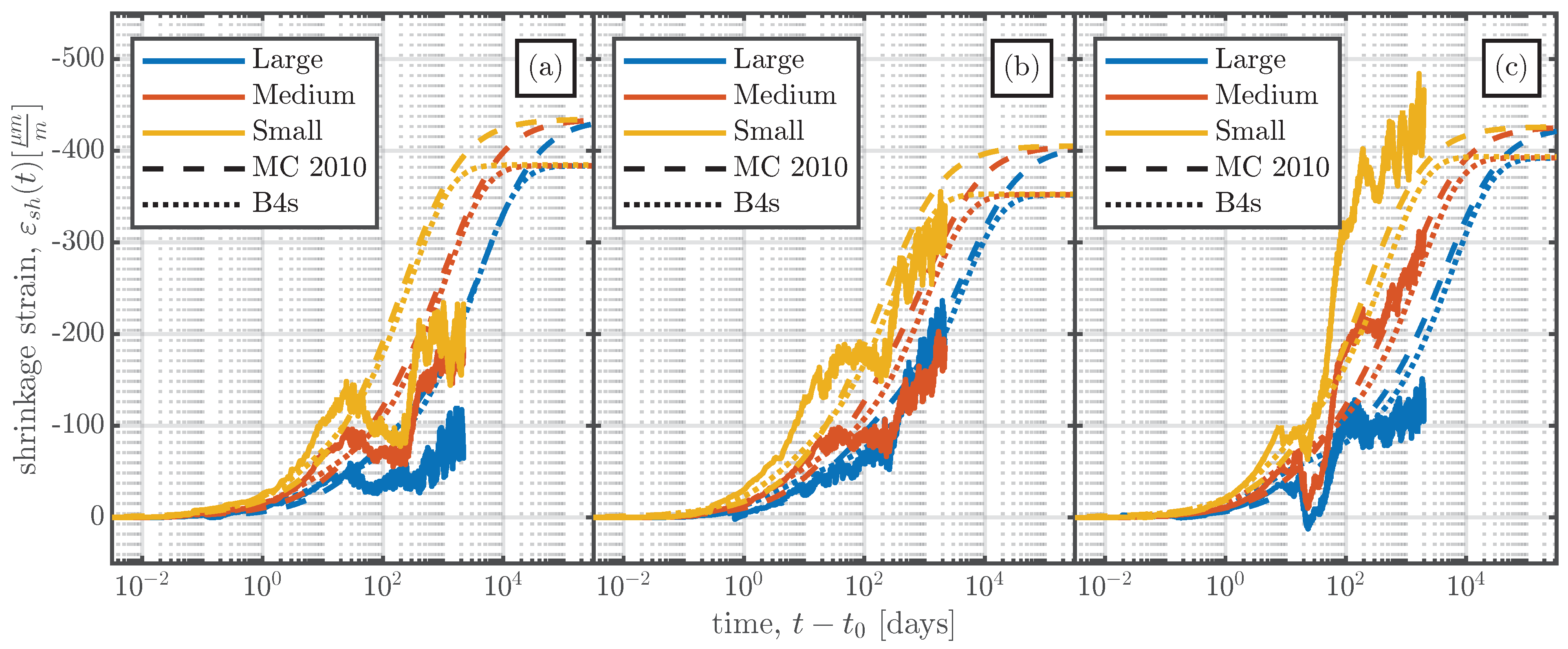

| Series | S1 | S2 | S4 |
|---|---|---|---|
| Production date | 13 July 2017 | 20 July 2017 | 8 February 2018 |
| Stripping date | 17 July 2017 | 24 July 2017 | 12 February 2018 |
| Transportation date | 10 August 2017 | 10 August 2017 | 20 February 2018 |
| Concrete composition | I | II | I |
| Concrete Composition | I | II |
|---|---|---|
| Cement CEM II A-LL 42.5 N | 292 | - |
| Cement CEM II A-LL 42.5 R | - | 450 |
| Processed hydraulic additions | 73 | 70 |
| Water | 167 | 185 |
| Aggregate (45% fine, 55% coarse) | 1794 | 1619 |
| Superplasticiser dynamiQ flow L01 | 2.56 | 3.9 |
| Air-entraining agent dynamiQ air S-01 | 0.55 | 0.78 |
| Property | Unit | S1 | S2 | S4 |
|---|---|---|---|---|
| Young’s modulus, E | GPa | 31.6 ± 0.9 | 32.2 ± 1.1 | 31.0 ± 0.4 |
| Compressive strength, | MPa | 41.4 ± 0.4 | 52.9 ± 0.6 | 44.4 ± 0.1 |
| Density, | kg/dm³ | 2.30 ± 0.01 | 2.31 ± 0.02 | 2.28 ± 0.02 |
| Specimen Size | S1 | S2 | S4 |
|---|---|---|---|
| Large | 9.69 | 10.48 | 10.02 |
| Medium | 8.21 | 10.36 | 9.14 |
| Small | 8.15 | 10.27 | 10.59 |
| S1 | S2 | S4 | ||
|---|---|---|---|---|
| Large | 11.08 | 11.73 | 11.83 | |
| 0.07 | 0.10 | 0.01 | ||
| Medium | 11.14 | 12.05 | 11.68 | |
| 0.06 | 0.07 | 0.06 | ||
| Small | 10.85 | 11.58 | 11.00 | |
| 0.03 | 0.05 | 0.03 |
| Test Data j | Model | ||
|---|---|---|---|
| MC 2010 | B4s | ||
| 1. | CC I | 25.6 | 33.6 |
| 2. | CC II | 19.0 | 31.8 |
| 3. | S1 large | 271.3 | 251.6 |
| 4. | S1 medium | 136.8 | 123.3 |
| 5. | S1 small | 163.6 | 148.8 |
| 6. | S2 large | 28.5 | 30.2 |
| 7. | S2 medium | 125.5 | 98.0 |
| 8. | S2 small | 35.9 | 23.9 |
| 9. | S4 large | 146.0 | 113.0 |
| 10. | S4 medium | 23.1 | 35.5 |
| 11. | S4 small | 38.9 | 49.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bachofner, W.; Suza, D.; Müller, H.S.; Kollegger, J. Long-Term Shrinkage Measurements on Large-Scale Specimens Exposed to Real Environmental Conditions. Materials 2023, 16, 7305. https://doi.org/10.3390/ma16237305
Bachofner W, Suza D, Müller HS, Kollegger J. Long-Term Shrinkage Measurements on Large-Scale Specimens Exposed to Real Environmental Conditions. Materials. 2023; 16(23):7305. https://doi.org/10.3390/ma16237305
Chicago/Turabian StyleBachofner, Wolfgang, Dominik Suza, Harald S. Müller, and Johann Kollegger. 2023. "Long-Term Shrinkage Measurements on Large-Scale Specimens Exposed to Real Environmental Conditions" Materials 16, no. 23: 7305. https://doi.org/10.3390/ma16237305
APA StyleBachofner, W., Suza, D., Müller, H. S., & Kollegger, J. (2023). Long-Term Shrinkage Measurements on Large-Scale Specimens Exposed to Real Environmental Conditions. Materials, 16(23), 7305. https://doi.org/10.3390/ma16237305







