Concrete Cover Cracking and Reinforcement Corrosion Behavior in Concrete with New-to-Old Concrete Interfaces
Abstract
:1. Introduction
2. Experimental Program
2.1. Preparation of Specimens and Mixed Proportions
2.2. Accelerated Corrosion Tests
2.3. Measurement of Corrosion Level
2.4. Tensile Tests
3. Test Results and Discussion
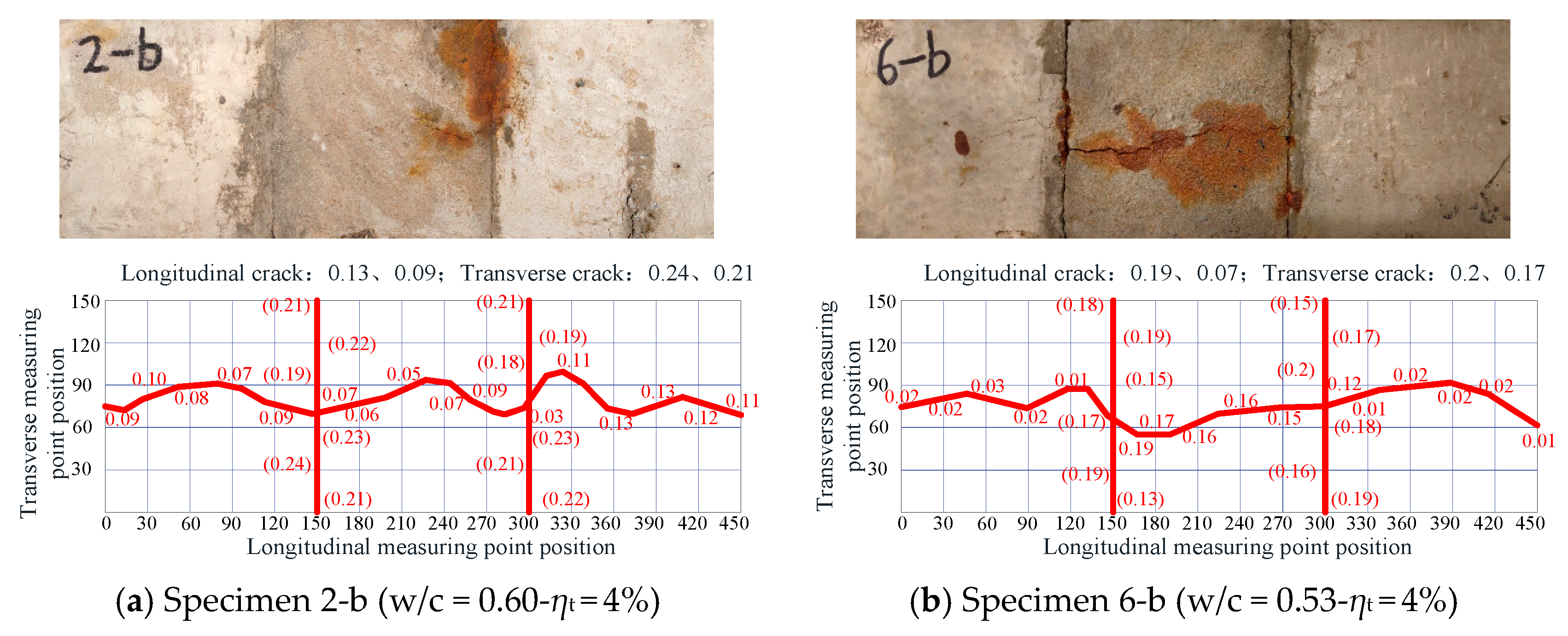
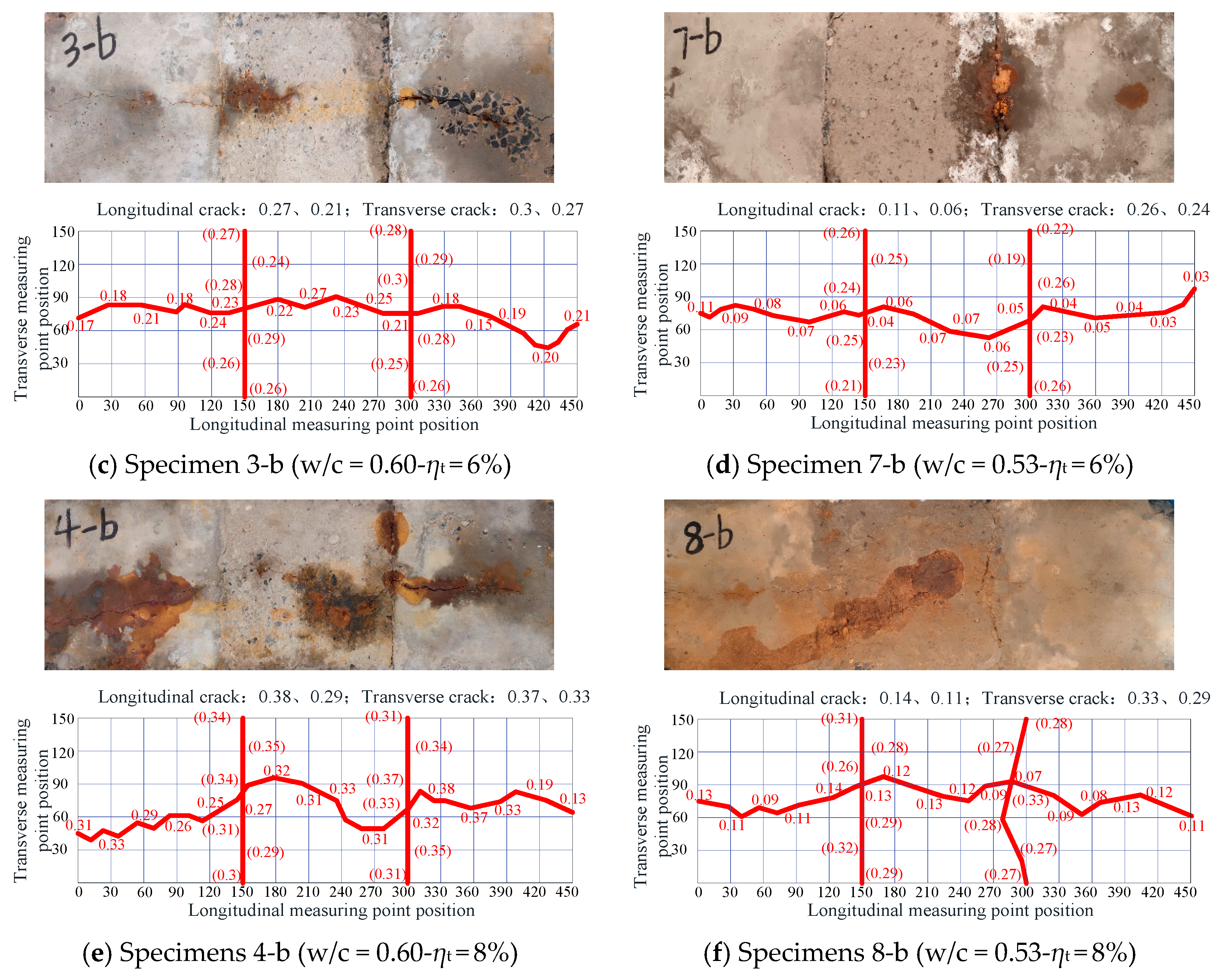
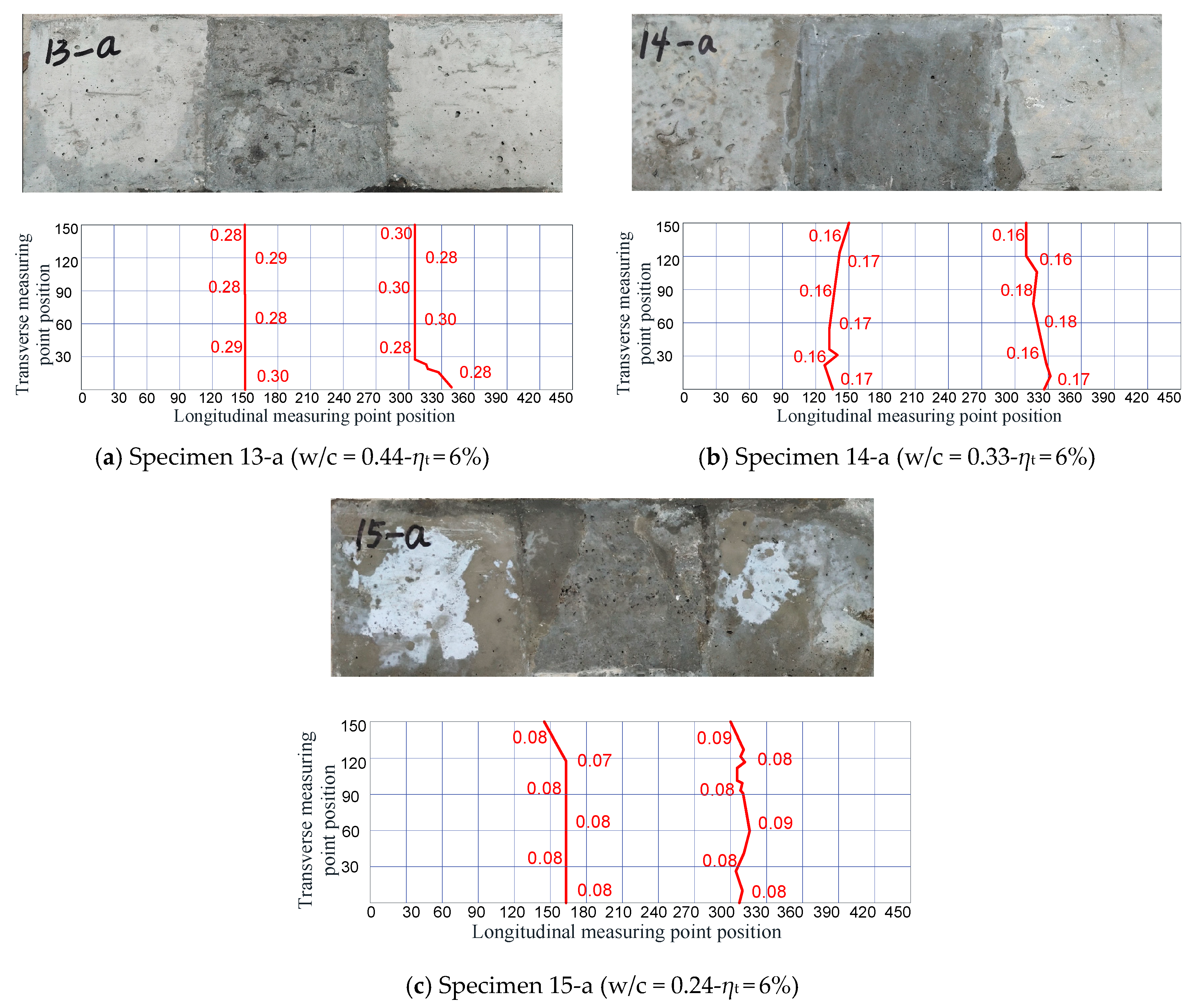
3.1. Corrosion Crack Development
3.1.1. Monolithic Specimens
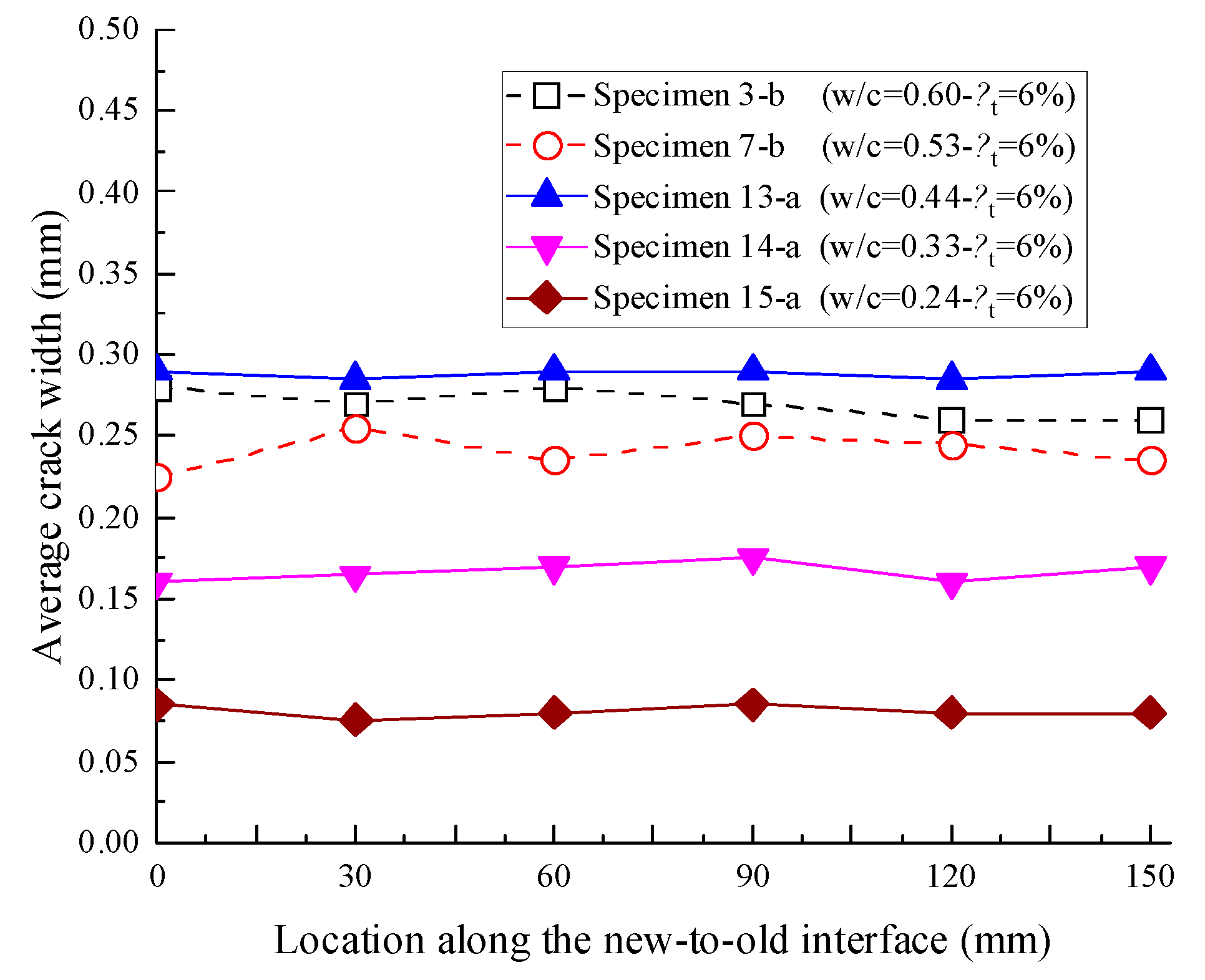
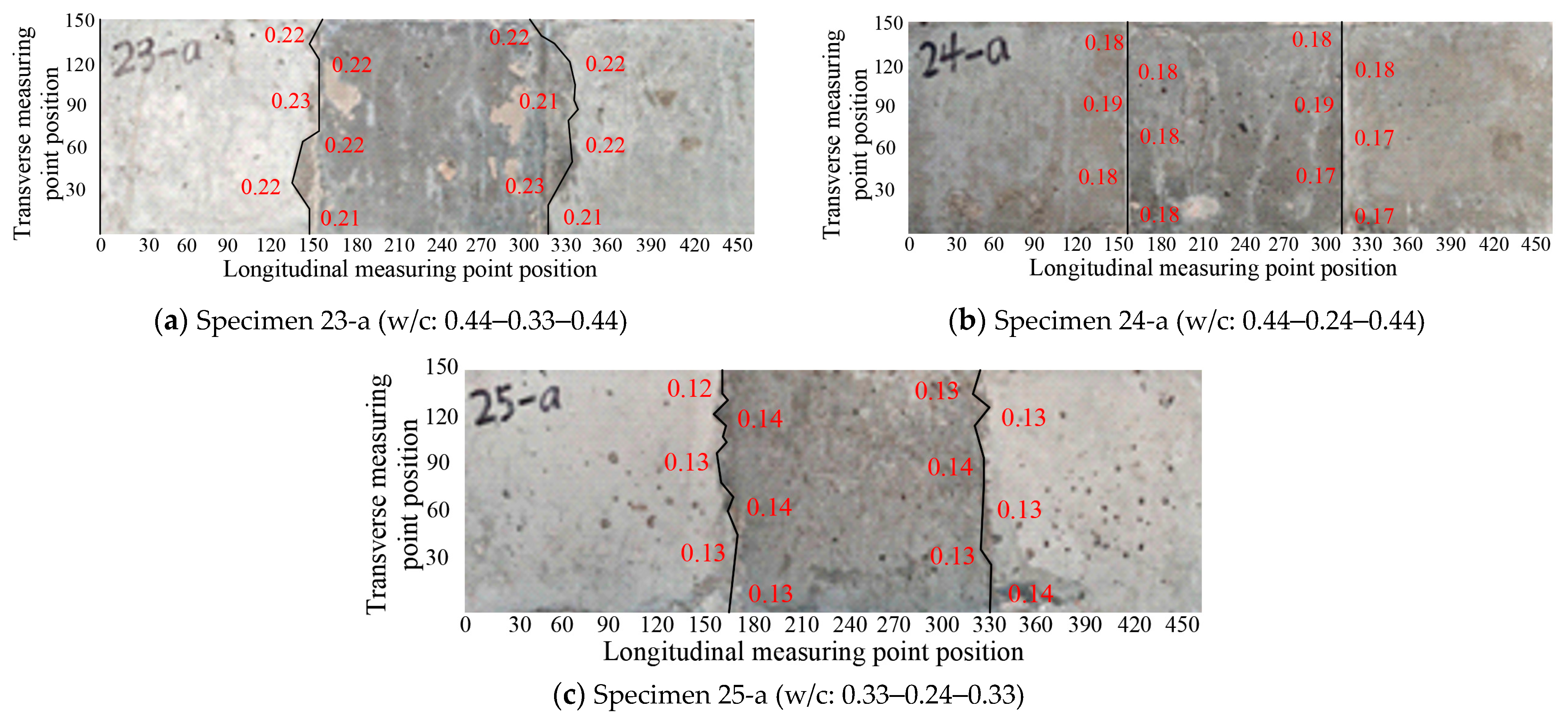
3.1.2. Segmental Specimens with New-to-Old Concrete Interface
3.2. Corrosion Level of Reinforcement
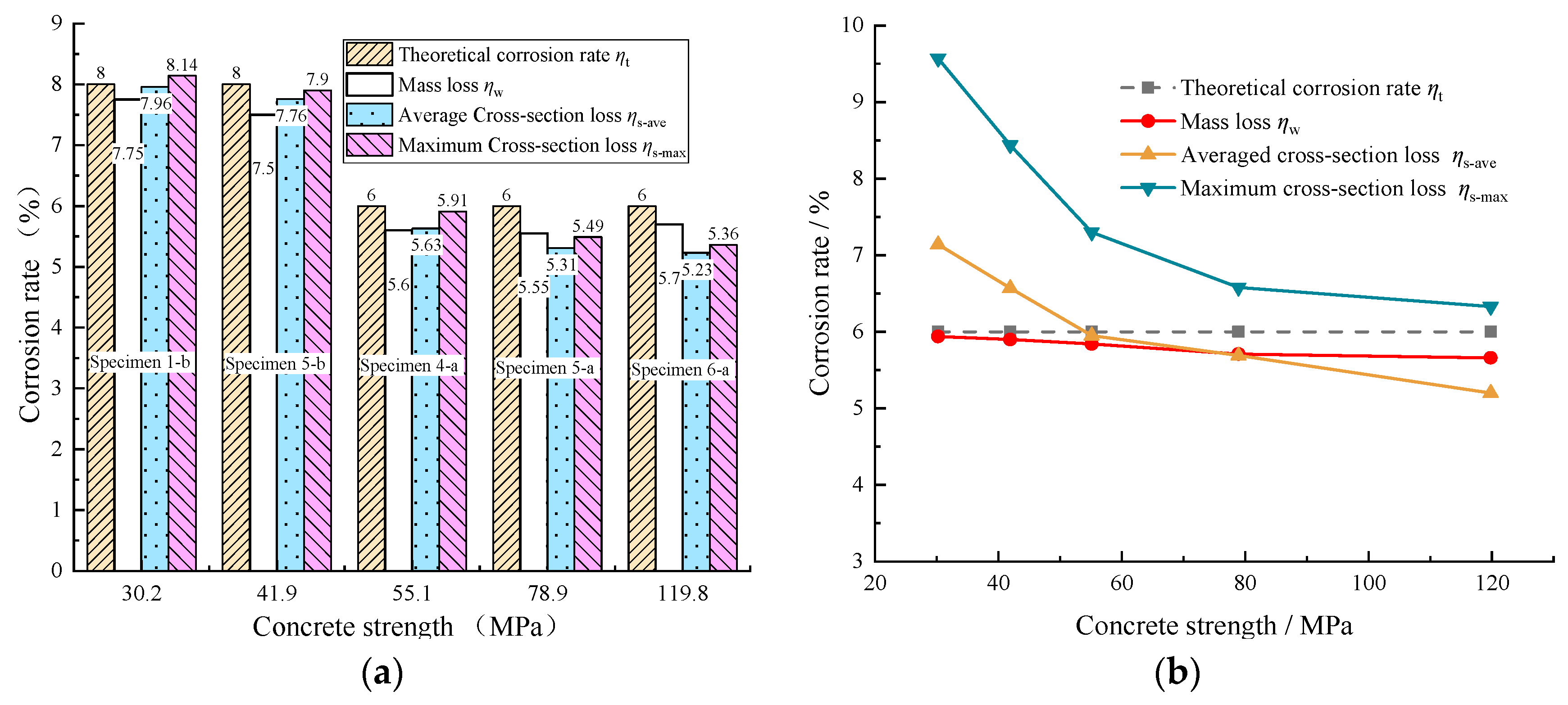
3.2.1. Sectional Loss of Reinforcement
3.2.2. Comparison between Actual and Theoretical Corrosion Levels of Steel
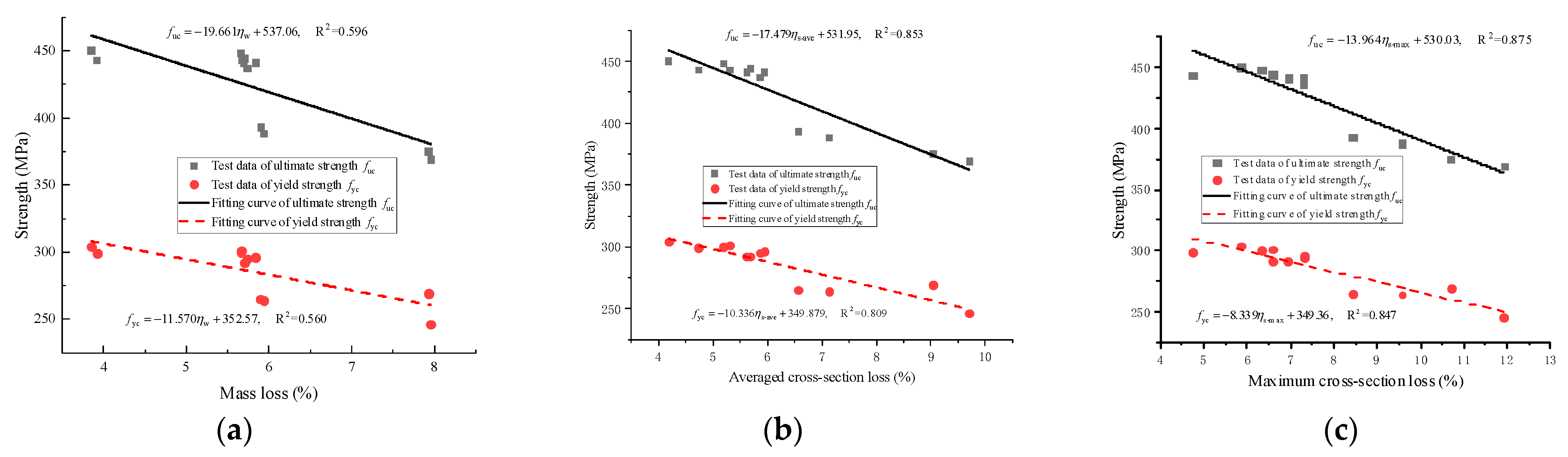
3.2.3. Mechanical Properties of Corroded Reinforcement
3.3. Prediction of Concrete Cracking Time
4. Conclusions
- (1)
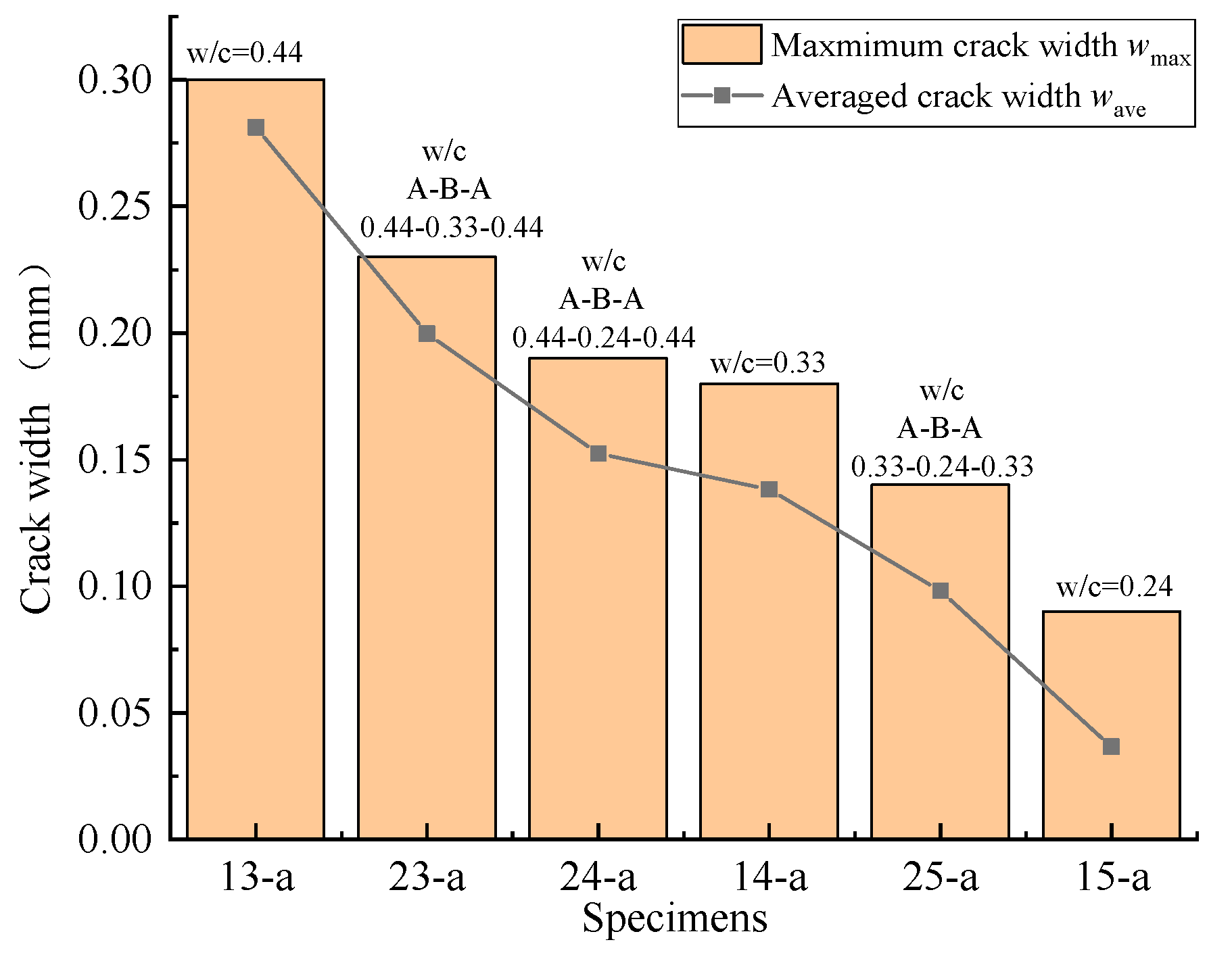
- The steels embedded in segmental specimens suffered higher cross-sectional loss at the new-to-old concrete interfaces, with the maximum sectional loss of the steel sharply decreasing as the concrete strength increased. An influence coefficient based on the section loss of the rebar was proposed to evaluate the influence of interfaces on the rust uniformity of rebars. When there were differences in strength between new and old concrete, the influence of the interface on the uniformity of the steel bar cross-section loss slightly increased.
- (2)
- Both the mass loss and sectional loss could be used to describe the steel uniform corrosion in the monolithic specimens, but for the segmental specimens, it was more appropriate to use cross-sectional loss to evaluate steel corrosion, due to the steel at the new-to-old concrete interface suffering worse sectional loss.
- (3)
- The corroded steels embedded in the monolithic specimens fractured at the middle section, while those embedded in the segmental specimens fractured at the new-to-old concrete interface. The corroded steel bars in the segmental specimens showed worse tensile behavior compared with those in the monolithic specimens.
- (4)
- Based on available theoretical analysis for uniform corrosion, the concrete cracking time of the monolithic specimens was predicted, which was basically consistent with experimental phenomena. However, further research is needed to predict the service life of segmental specimens.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviation
| fy | characteristic steel yield strength | kj | influence coefficient of construction joints |
| ΔmL | mass loss of iron (g) | m2 | rust amount of the rebar in joint section (g) |
| M | molar mass of iron (56 g/mol for Fe) | m1 | rust amount of the rebar in non-joint new concrete section (g) |
| Z | valency of the iron element (i.e., 2.5) | m3 | rust amount of the rebar in non-joint old concrete section (g) |
| F | Faraday constant (i.e., 96,500 C/mol) | ks | influence coefficient based on section loss of steel bars |
| t | duration of electrochemical corrosion(s) | Si | section loss of the rebar at the new-to-old concrete interface |
| I | magnitude of the electric current (A) | Sn, So | average section loss of the rebar at each measuring point in the new and old concrete sections, respectively. |
| S | surface area of reinforcement (m2) | ηw | mass loss of the corroded steel |
| ηt | theoretical corrosion rate | ηs-ave | average cross-section loss of the corroded steel |
| effective mass loss of the rebar | ηs-max | maximum cross-section loss of the corroded steel | |
| mo | measured weight before the corrosion process | q | uniform corrosion-induced stress |
| mc | measured weight after the corrosion process | c | net concrete cover thickness |
| sectional loss | d | rebar diameter | |
| Sl | uncorroded cross-sectional area of reinforcement (m2) | ftk | standard value of axial tensile strength. |
| S2 | corroded cross-sectional area of reinforcement (m2) | δ | thickness of the rust layer |
| dl | uncorroded rebar diameter (m2) | n | volume expansion ratio of the corroded rebar |
| d2 | corroded rebar diameter (m2) | ρ | corrosion rate of the rebar, evaluated using the cross-sectional loss percentages |
| ηs-max | maximum cross-section loss of the corroded steel |
References
- Zhang, J.; Ling, X.; Guan, Z. Finite element modeling of concrete cover crack propagation due to non-uniform corrosion of reinforcement. Constr. Build. Mater. 2017, 132, 487–499. [Google Scholar] [CrossRef]
- Xi, X.; Yang, S. Time to surface cracking and crack width of reinforced concrete structures under corrosion of multiple rebars. Constr. Build. Mater. 2017, 155, 114–125. [Google Scholar] [CrossRef]
- Hu, Z.; Xia, L.; Cheng, C.; Li, B.; Xu, B. Investigation on corrosion-induced cracking and corrosion expansive pressure in reinforced concrete members. J. Harbin Inst. Technol. 2020, 52, 99–105. (In Chinese) [Google Scholar]
- Andrade, C.; Alonso, C.; Molina, F.J. Cover cracking as a function of bar corrosion: Part I-Experimental test. Mater. Struct. 1993, 26, 453–464. [Google Scholar] [CrossRef]
- Alonso, C.; Andrade, C.; Rodriguez, J.; Diez, J.M. Factors controlling cracking of concrete affected by reinforcement corrosion. Mater. Struct. 1998, 31, 435–441. [Google Scholar] [CrossRef]
- Vidal, T.; Castel, A.; François, R. Analyzing crack width to predict corrosion in reinforced concrete. Cem. Concr. Res. 2004, 34, 165–174. [Google Scholar] [CrossRef]
- Zhang, R.; Castel, A.; François, R. Concrete cover cracking with reinforcement corrosion of RC beam during chloride-induced corrosion process. Cem. Concr. Res. 2010, 40, 415–425. [Google Scholar] [CrossRef]
- Malumbela, G.; Alexander, M.; Moyo, P. Interaction between corrosion crack width and steel loss in RC beams corroded under load. Cem. Concr. Res. 2010, 40, 1419–1428. [Google Scholar] [CrossRef]
- Malumbela, G.; Moyo, P.; Alexander, M. Influence of corrosion crack patterns on the rate of crack widening of RC beams. Constr. Build. Mater. 2011, 25, 2540–2553. [Google Scholar] [CrossRef]
- Zhao, Y.; Wu, Y.; Jin, W. Distribution of millscale on corroded steel bars and penetration of steel corrosion products in concrete. Corros. Sci. 2013, 66, 160–168. [Google Scholar] [CrossRef]
- Rivera-Corral, J.; Fajardo, G.; Arliguie, G.; Orozco-Cruz, R.; Deby, F.; Valdez, P. Corrosion behavior of steel reinforcement bars embedded in concrete exposed to chlorides: Effect of surface finish. Constr. Build. Mater. 2017, 147, 815–826. [Google Scholar] [CrossRef]
- Wu, H.; Pan, K.; Dai, Y.; Jin, H.; Li, F. Influence of Chloride Ions on Corrosion Behavior of Steel Bars in a Simulated Concrete Pore Solution. Int. J. Electrochem. Sci. 2019, 14, 4995–5007. [Google Scholar] [CrossRef]
- Cai, Y.; Zhang, W.; Yu, L.; Chen, M.; Yang, C.; François, R.; Yang, K. Characteristics of the steel-concrete interface and their effect on the corrosion of steel bars in concrete. Constr. Build. Mater. 2020, 253, 119162. [Google Scholar] [CrossRef]
- Wang, X.; Yuan, J.; Wei, P.; Zhu, M. Effects of fly ash microspheres on sulfate erosion resistance and chlorion penetration resistance in concrete. J. Therm. Anal. Calorim. 2020, 139, 3395–3403. [Google Scholar] [CrossRef]
- Oliveira, R.L.; Bragança, M.O.; Medeiros-Junior, R.A. Effect of coarse aggregate size on corrosion of reinforced concrete exposed to carbonation and chloride ingress by electrochemical measurements. Constr. Build. Mater. 2022, 361, 129665. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, C.; Zhang, P.; Sun, M.; Geng, Y.; Zhao, M.; Fan, L.; Liu, N.; Duan, J. Chloride binding of monosulfate hydrate (AFm) and its effect on steel corrosion in simulated concrete pore solution. J. Build. Eng. 2023, 67, 105945. [Google Scholar] [CrossRef]
- Zhang, L.; Niu, D.; Li, H.; Wen, B.; Wang, Y. Rust expansion cracking behaviour of new Cr alloy-reinforced coral aggregate concrete. Constr. Build. Mater. 2023, 395, 132048. [Google Scholar] [CrossRef]
- Zhao, J.; Li, F. Chloride Transport in Interfacial Zone of New–Old Concrete Joints of Precast Prestressed Concrete Bridges under Fatigue Load. J. Mater. Civ. Eng. 2022, 34, 04022263. [Google Scholar] [CrossRef]
- Zhang, X.H.; Wang, H.; Zhang, Y.; Wang, L. Corrosion of Steel Rebars across UHPC Joint Interface under Chloride Attack. Constr. Build. Mater. 2023, 387, 131591. [Google Scholar] [CrossRef]
- Li, G.; Hu, H.; Ren, C. Resistance of Segmental Joints to Chloride Ions. ACI Mater. J. 2016, 113, 471. [Google Scholar] [CrossRef]
- Liu, J.; Huang, H.; Ma, Z.J.; Chen, J. Effect of shear reinforcement corrosion on interface shear transfer between concretes cast at different times. Eng. Struct. 2021, 232, 111872. [Google Scholar] [CrossRef]
- Sung-Won, Y.; Seung-Jun, K. Effects of cold joint and loading conditions on chloride diffusion in concrete containing GGBFS. Constr. Build. Mater. 2016, 115, 247–255. [Google Scholar]
- Yang, H.M.; Lee, H.S.; Yang, K.H.; Ismail, M.A.; Kwon, S.J. Time and cold joint effect on chloride diffusion in concrete containing GGBFS under various loading conditions. Constr. Build. Mater. 2018, 167, 739–748. [Google Scholar] [CrossRef]
- Li, G.P.; Hu, H.; Ren, C.; Zhou, S.Y.; Li, J.X. Study on durability of joints in concrete bridge structures. China Civ. Eng. Journa 2018, 51, 98–103. (In Chinese) [Google Scholar]
- Huang, G.; Wu, B.; Shen, Y.; Wang, L.; Li, G. Comparative experiment of steel bar corrosion at concrete construction joints. Front. Mater. 2022, 9, 1094696. [Google Scholar] [CrossRef]
- GB/T 50081-2019; Standard for Test Methods of Concrete Physical and Mechanical Properties. China Academy of Building Research: Beijing, China, 2019.
- GB/T 228.1-2021; Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature. China Standard Press: Beijing, China, 2021.
- Al-Mehthel, M.; Al-Dulaijan, S.; Al-Idi, S.H.; Shameem, M.; Ali, M.R.; Maslehuddin, M. Performance of generic and proprietary corrosion inhibitors in chlo-ride-contaminated silica fume cement concrete. Constr. Build. Mater. 2009, 23, 1768–1774. [Google Scholar] [CrossRef]
- Gan, W.Z.; Jin, W.L.; Gao, M.Z. Applicability study on accelerated corrosion methods of steel bars in concrete structure. J. Build. Struct. 2011, 32, 41–47. (In Chinese) [Google Scholar]
- Tondolo, F. Bond behaviour with reinforcement corrosion. Constr. Build. Mater. 2015, 93, 926–932. [Google Scholar] [CrossRef]
- Ma, Y.; Guo, Z.; Wang, L.; Zhang, J. Experimental investigation of corrosion effect on bond behavior between reinforcing bar and concrete. Constr. Build. Mater. 2017, 152, 240–249. [Google Scholar] [CrossRef]
- ASTM G1–03; Standard Practice for Preparing, Cleaning, and Evaluating Corrosion Test Specimens. American Society for Testing and Materials (ASTM): West Conshohocken, PA, USA, 2011.
- Lu, C.; Yuan, S.; Cheng, P.; Liu, R. Mechanical properties of corroded steel bars in pre-cracked concrete suffering from chloride attack. Constr. Build. Mater. 2016, 123, 649–660. [Google Scholar] [CrossRef]
- François, R.; Khan, I.; Dang, V.H. Impact of corrosion on mechanical properties of steel embedded in 27-year-old corroded reinforced concrete beams. Mater. Struct. 2013, 46, 899–910. [Google Scholar] [CrossRef]
- Zhang, W.P.; Chen, H.; Gu, X.L. Tensile behavior of corroded steel bars under different strain rates. Mag. Concr. Res. 2016, 68, 127–140. [Google Scholar] [CrossRef]
- Tang, F.; Lin, Z.; Chen, G.; Yi, W. Three-dimensional corrosion pit measurement and statistical mechanical degradation analysis of deformed steel bars subjected to accelerated corrosion. Constr. Build. Mater. 2014, 70, 104–117. [Google Scholar] [CrossRef]
- Ou, Y.-C.; Susanto, Y.T.T.; Roh, H. Tensile behavior of naturally and artificially corroded steel bars. Constr. Build. Mater. 2016, 103, 93–104. [Google Scholar] [CrossRef]
- Jin, W.L.; Zhao, Y.X.; Yan, F. The mechanism of corroded expansion force of reinforced concrete members. J. Hydraul. Eng. 2001, 7, 57–62. (In Chinese) [Google Scholar]
- Ji, Y.S.; Yan, Y.S.; Li, F.M. Corrosion-induced expansive force and time-dependent change characteristics of corrosion rate of steel bars in concrete. J. Hohai Univ. Nat. Sci. 2009, 37, 430–436. (In Chinese) [Google Scholar]
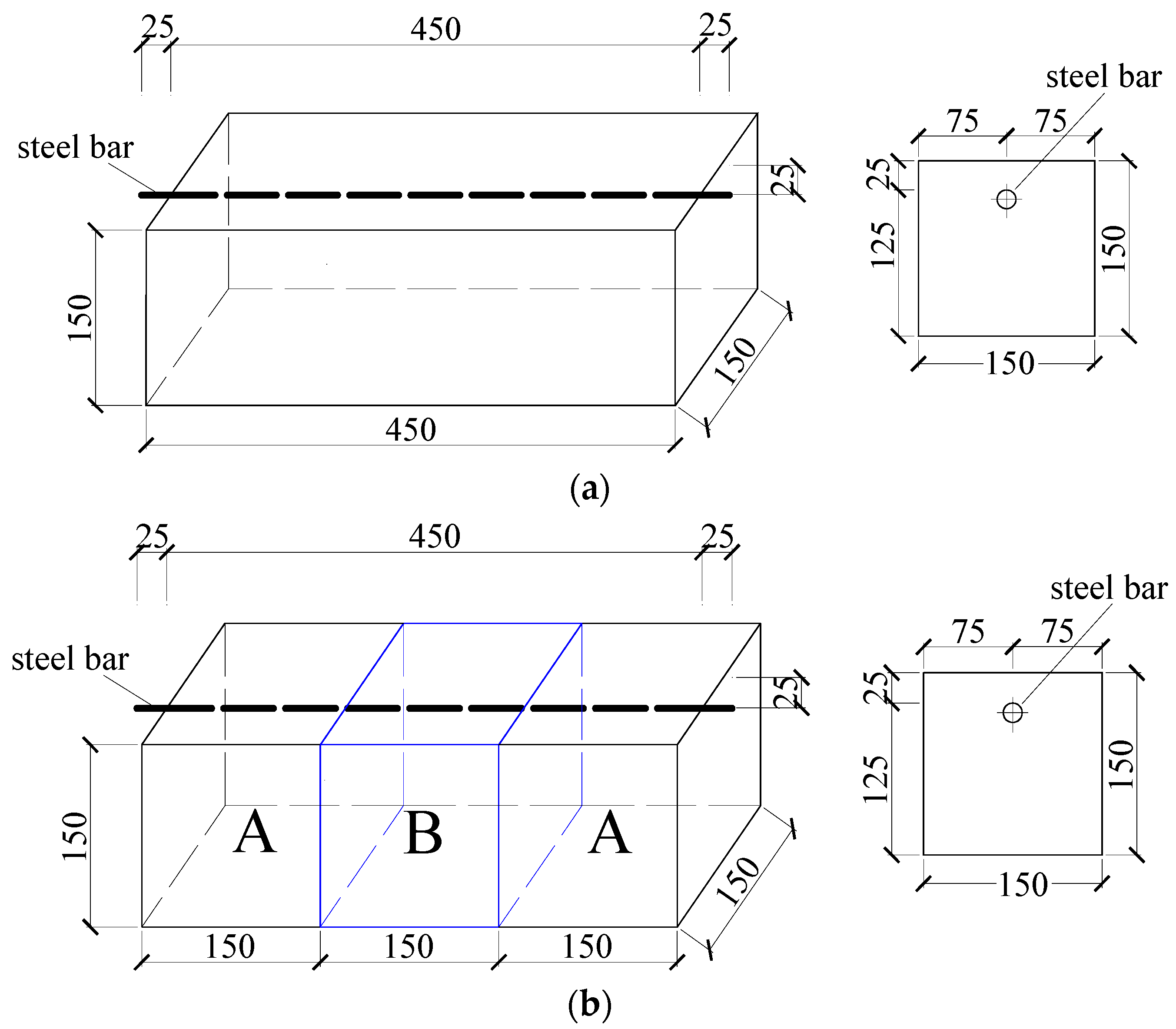










| Group | Specimens | Casting Method | Theoretical Corrosion Rate ηt (%) | Water–Cement Ratio | Concrete Number | |
|---|---|---|---|---|---|---|
| Monolithic | Segmental Casting (A–B–A) | |||||
| Ordinary concrete | 1-b | Monolithic | 8 | 0.6 | No.1 | |
| 2-b | Segmental casting | 4 | / | 0.60–0.60–0.60 | No.1 | |
| 3-b | Segmental casting | 6 | 0.60–0.60–0.60 | No.1 | ||
| 4-b | Segmental casting | 8 | 0.60–0.60–0.60 | No.1 | ||
| 5-b | Monolithic | 8 | 0.53 | No.2 | ||
| 6-b | Segmental casting | 4 | / | 0.53–0.53–0.53 | No.2 | |
| 7-b | Segmental casting | 6 | 0.53–0.53–0.53 | No.2 | ||
| 8-b | Segmental casting | 8 | 0.53–0.53–0.53 | No.2 | ||
| High-strength concrete | 4-a | Monolithic | 6 | 0.44 | No.3 | |
| 5-a | Monolithic | 6 | 0.33 | No.4 | ||
| 6-a | Monolithic | 6 | 0.24 | No.5 | ||
| 13-a | Segmental casting | 6 | / | 0.44–0.44–0.44 | No.3 | |
| 14-a | Segmental casting | 6 | 0.33–0.33–0.33 | No.4 | ||
| 15-a | Segmental casting | 6 | 0.24–0.24–0.24 | No.5 | ||
| 23-a | Segmental casting | 6 | 0.44–0.33–0.44 | No.3 and No.4 | ||
| 24-a | Segmental casting | 6 | 0.44–0.24–0.44 | No.3 and No.5 | ||
| 25-a | Segmental casting | 6 | 0.33–0.24–0.33 | No.4 and No.5 | ||
| Number | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Cement type | P.O42.5 | P.O42.5 | P.O42.5 | P.II52.5 | P.II52.5 |
| Cement quantity (kg/m3) | 350 | 300 | 350 | 440 | 550 |
| Slag type | / | S95 | S95 | S95 | S95 |
| Slag quantity (kg/m3) | / | 60 | 70 | 60 | 60 |
| Fly ash type | / | F-I | F-I | F-I | F-I |
| Fly ash quantity (kg/m3) | / | 60 | 70 | 60 | 70 |
| Fine aggregate (medium sand) quantity (kg/m3) | 719 | 895 | 867 | 855 | 795 |
| Coarse aggregate (size: 5–10 mm) quantity (kg/m3) | 1170 | 564 | 542 | 513 | 477 |
| Coarse aggregate (size: 10–20 mm) quantity (kg/m3) | 376 | 361 | 342 | 318 | |
| Admixture Type | / | DSZ-1 | DSZ-1 | DSZ-1 | DSZ-1 |
| Admixture quantity (kg/m3) | / | 2.80 | 3.92 | 10.08 | 18.9 |
| Water quantity (kg/m3) | 210 | 160 | 155 | 145 | 130 |
| w/c ratio | 0.60 | 0.53 | 0.44 | 0.33 | 0.24 |
| Compressive strength (MPa) | 30.2 | 41.9 | 55.1 | 78.9 | 119.8 |
| Specimens | 3-b | 7-b | 13-a | 14-a | 15-a | 25-a | 24-a | 23-a |
|---|---|---|---|---|---|---|---|---|
| w/c | 0.60 | 0.53 | 0.44 | 0.33 | 0.24 | 0.44–0.33–0.44 | 0.44–0.24–0.44 | 0.33–0.24–0.33 |
| ks | 1.401 | 1.322 | 1.249 | 1.180 | 1.227 | 1.316 | 1.295 | 1.299 |
| Specimens | Water–Cement Ratio | Casting Method | Theoretical Corrosion Rate η/% | Mass Loss Ratio ηw/% | Averaged Sectional Loss Ratio ηs-ave/% | Maximum Sectional Loss Ratio ηs-max/% | Yield Strength fyc/MPa | Ultimate Strength fuc/MPa |
|---|---|---|---|---|---|---|---|---|
| 1-b | 0.6 | Monolithic | 8 | 7.75 | 7.96 | 8.14 | 274 | 394 |
| 5-b | 0.53 | Monolithic | 8 | 7.5 | 7.76 | 7.9 | 276 | 395 |
| 4-a | 0.44 | Monolithic | 6 | 5.6 | 5.63 | 5.91 | 305 | 449 |
| 5-a | 0.33 | Monolithic | 6 | 5.55 | 5.31 | 5.49 | 305 | 452 |
| 6-a | 0.24 | Monolithic | 6 | 5.7 | 5.23 | 5.36 | 294 | 454 |
| 2-b | 0.6–0.6–0.6 | Segmental | 4 | 3.92 | 4.74 | 6.82 | 299 | 443 |
| 3-b | 0.6–0.6–0.6 | Segmental | 6 | 5.94 | 7.14 | 9.57 | 264 | 388 |
| 4-b | 0.6–0.6–0.6 | Segmental | 8 | 7.96 | 9.71 | 11.93 | 246 | 369 |
| 6-b | 0.53–0.53–0.53 | Segmental | 4 | 3.85 | 4.19 | 5.85 | 304 | 450 |
| 7-b | 0.53–0.53–0.53 | Segmental | 6 | 5.9 | 6.57 | 8.44 | 265 | 393 |
| 8-b | 0.53–0.53–0.53 | Segmental | 8 | 7.93 | 9.04 | 10.7 | 269 | 375 |
| 13-a | 0.44–0.44–0.44 | Segmental | 6 | 5.84 | 5.95 | 7.3 | 296 | 441 |
| 14-a | 0.33–0.33–0.33 | Segmental | 6 | 5.71 | 5.69 | 6.58 | 292 | 444 |
| 15-a | 0.24–0.24–0.24 | Segmental | 6 | 5.66 | 5.20 | 6.33 | 300 | 448 |
| 23-a | 0.44–0.33–0.44 | Segmental | 6 | 5.74 | 5.87 | 7.30 | 295 | 437 |
| 24-a | 0.44–0.24–0.44 | Segmental | 6 | 5.70 | 5.62 | 6.94 | 292 | 441 |
| 25-a | 0.33–0.24–0.33 | Segmental | 6 | 5.67 | 5.31 | 6.58 | 301 | 443 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Li, J.; Zhao, Y.; Wang, S.; Guan, Z. Concrete Cover Cracking and Reinforcement Corrosion Behavior in Concrete with New-to-Old Concrete Interfaces. Materials 2023, 16, 5969. https://doi.org/10.3390/ma16175969
Zhang J, Li J, Zhao Y, Wang S, Guan Z. Concrete Cover Cracking and Reinforcement Corrosion Behavior in Concrete with New-to-Old Concrete Interfaces. Materials. 2023; 16(17):5969. https://doi.org/10.3390/ma16175969
Chicago/Turabian StyleZhang, Juhui, Jing Li, Yuchuan Zhao, Shikun Wang, and Zhongguo Guan. 2023. "Concrete Cover Cracking and Reinforcement Corrosion Behavior in Concrete with New-to-Old Concrete Interfaces" Materials 16, no. 17: 5969. https://doi.org/10.3390/ma16175969
APA StyleZhang, J., Li, J., Zhao, Y., Wang, S., & Guan, Z. (2023). Concrete Cover Cracking and Reinforcement Corrosion Behavior in Concrete with New-to-Old Concrete Interfaces. Materials, 16(17), 5969. https://doi.org/10.3390/ma16175969





