Effect of Contact Pressure on Strain Distribution during Compression-Type Bulk Forming Processes
Abstract
1. Introduction
2. Experiment and Deformation Analysis
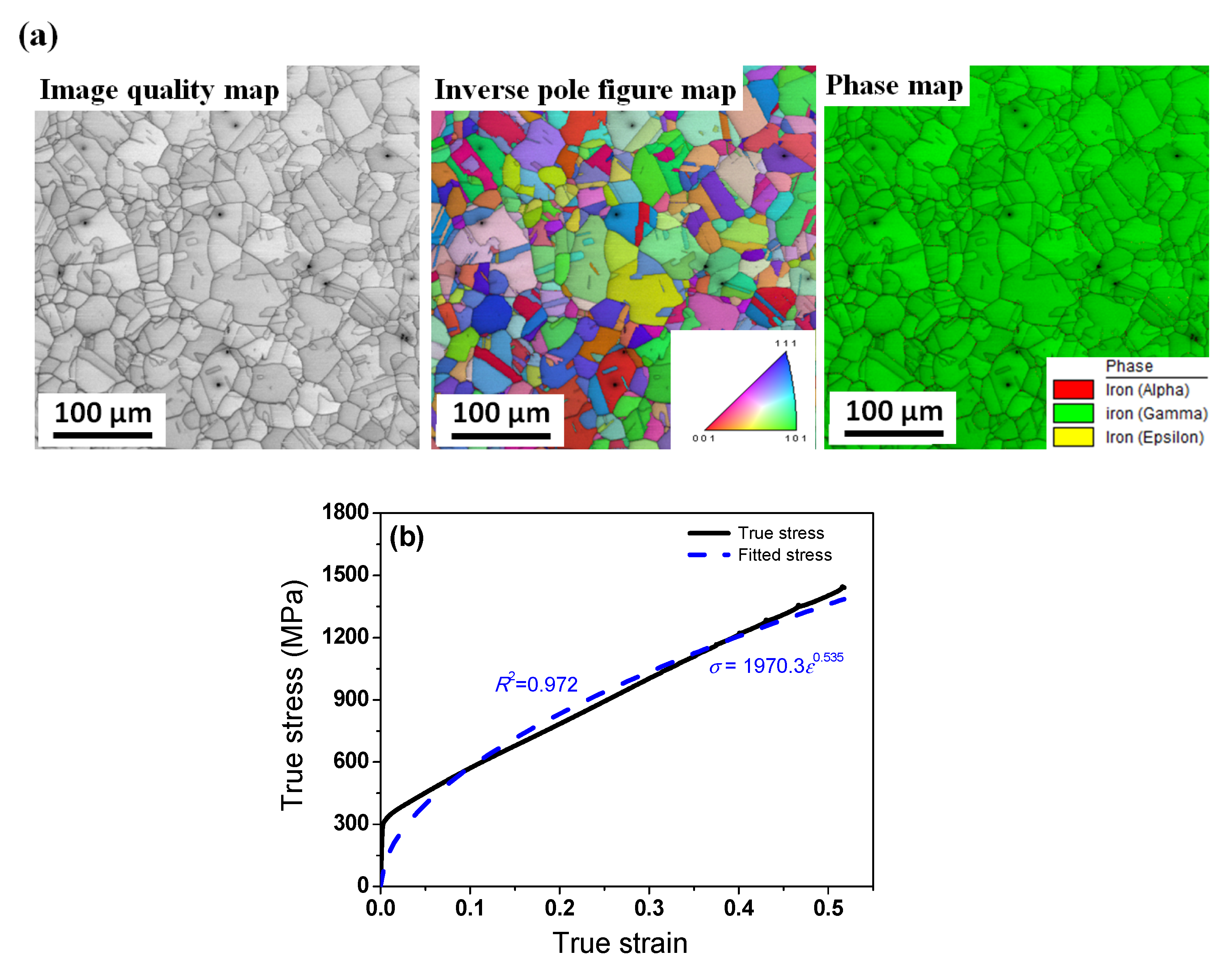
2.1. Experiments
2.2. Deformation Analysis
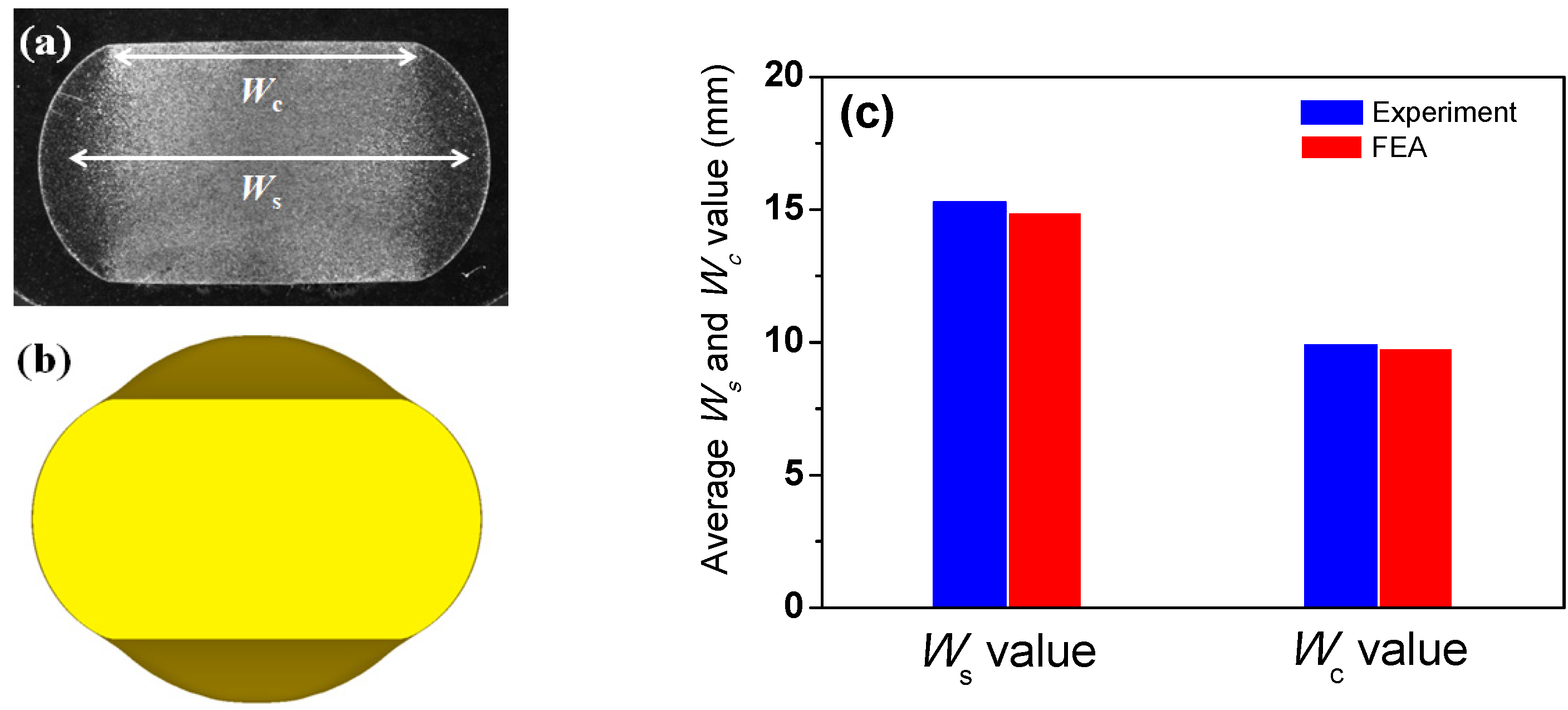
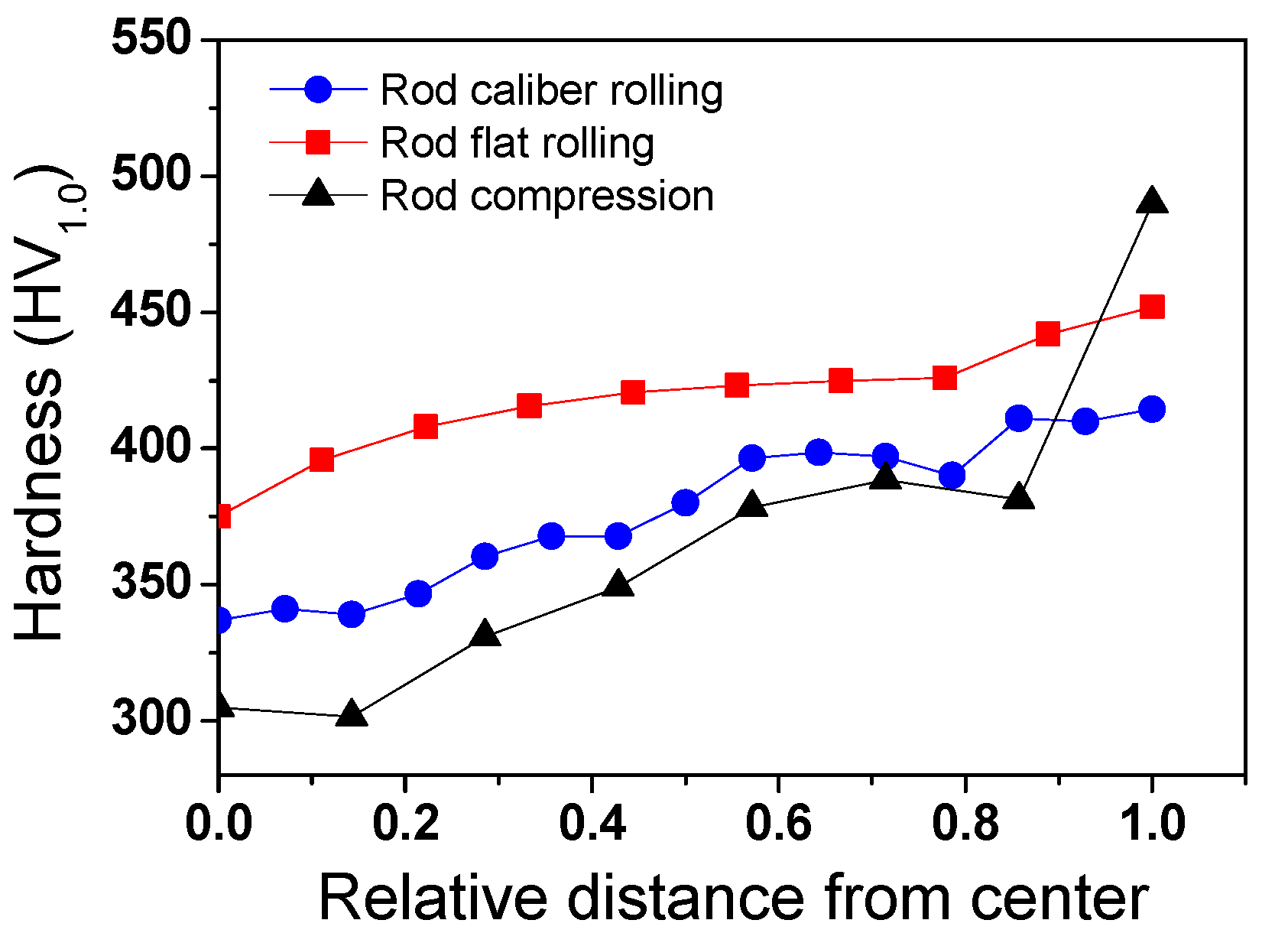
3. Validation
4. Results and Discussion
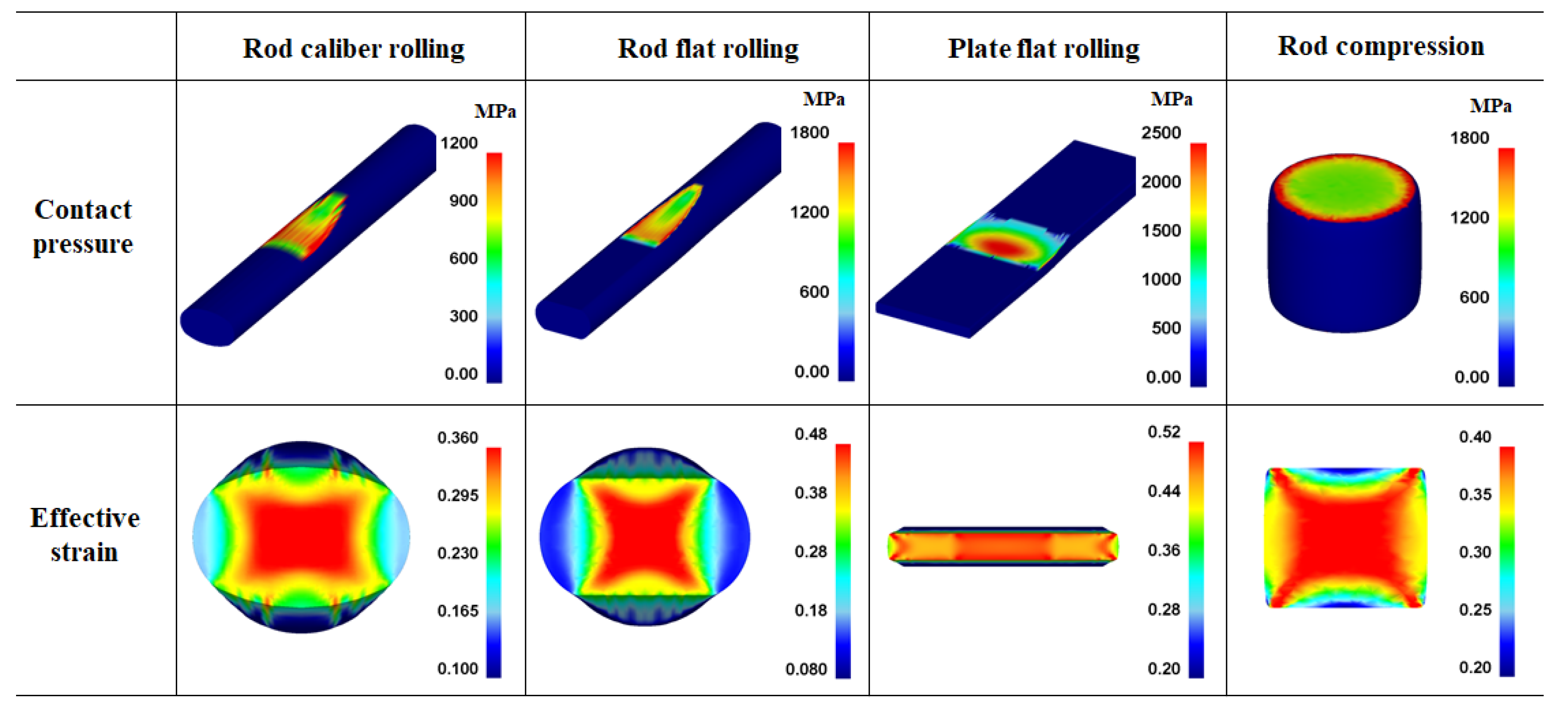
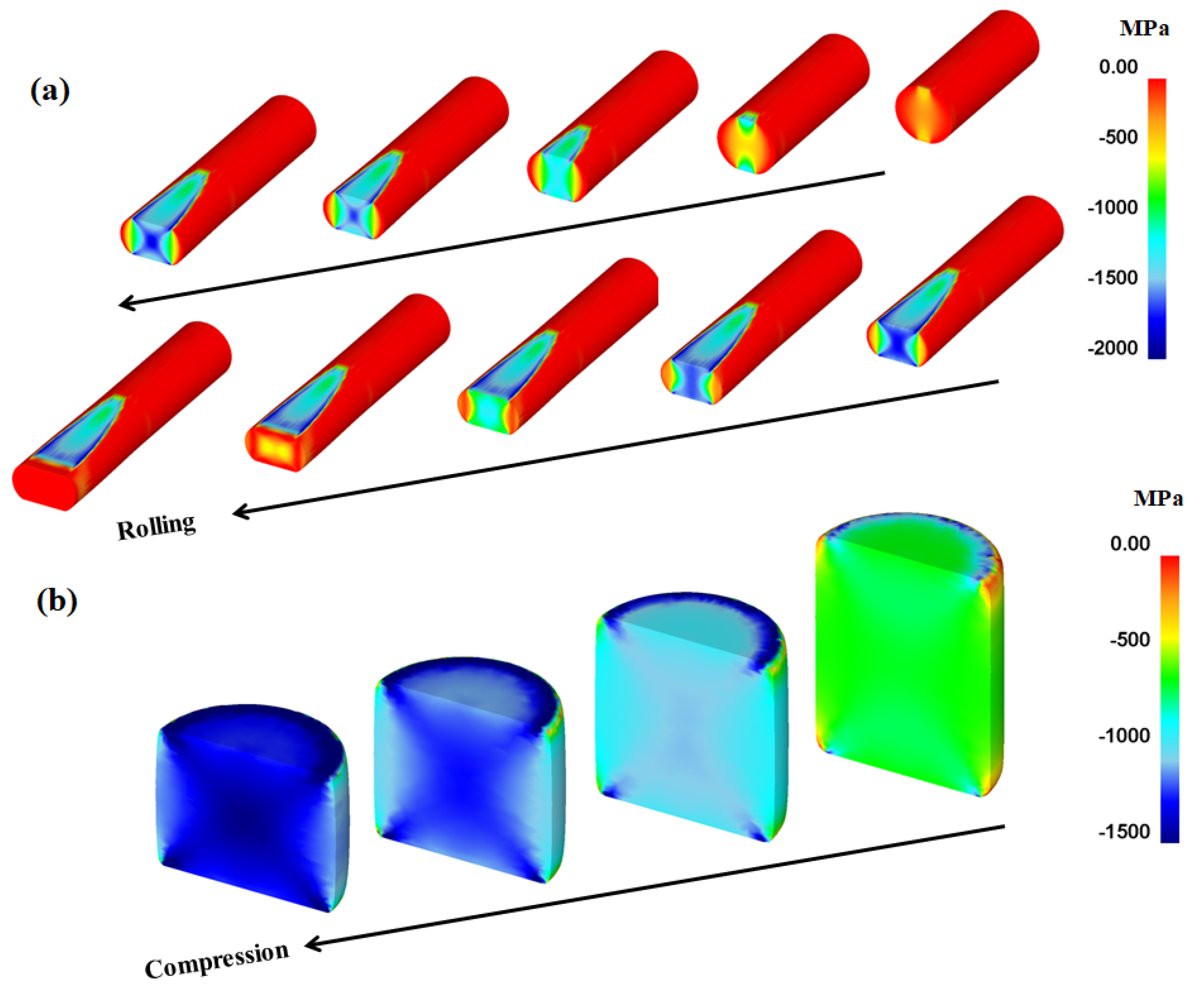
4.1. Strain Distribution in Workpiece
4.2. Contact Pressure Distribution on Workpiece
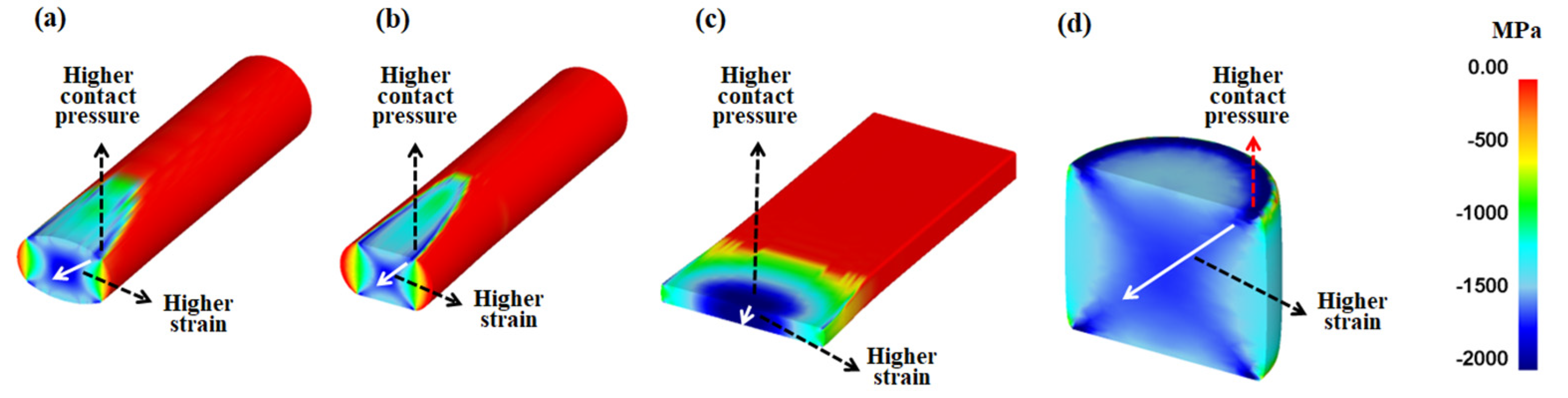
4.3. Effect of Contact Pressure on Strain Distribution
5. Conclusions
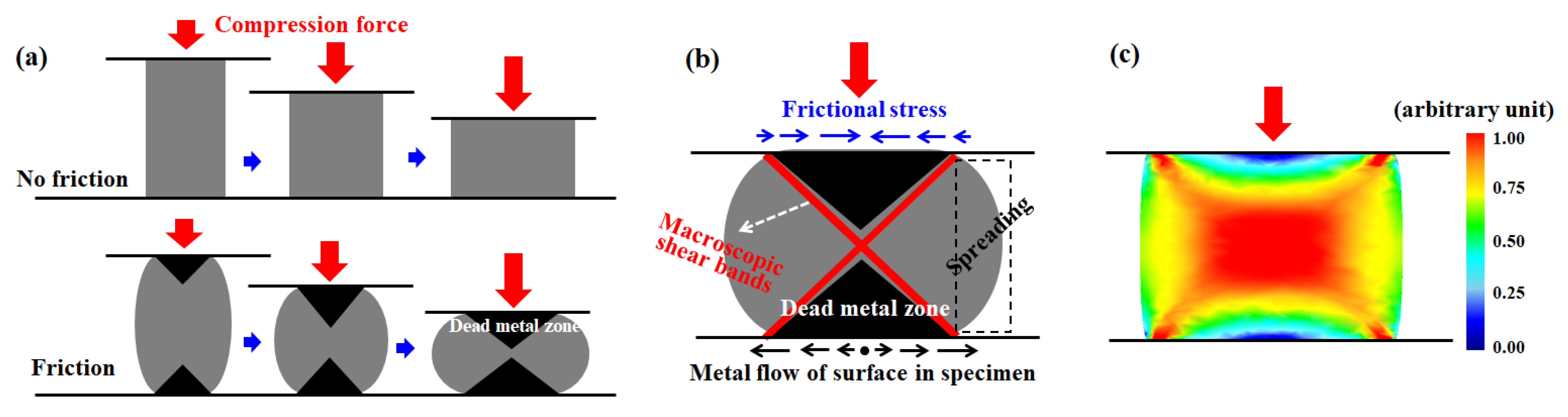
- Strain inhomogeneity or MSBs appeared in the workpiece during the CBFPs. Except for the flat-rolled plate, the core region of all specimens showed peak εeff, and the free surface region exhibited the lowest value. The regions along the diagonal direction showed a relatively high εeff, resulting in the occurrence of MSBs. However, the level of strain inhomogeneity in the workpiece was different from the forming process, because the formation of MSBs was dependent on the initial workpiece shape and tool design. The flat-rolled rod exhibited the maximum strain inhomogeneity, whereas the flat-rolled plate showed the minimum strain inhomogeneity.
- The occurrence of MSBs is related to the distribution of Pc or compression stress. The MSBs were stronger when the Pc was higher in the edge region of the workpiece because the sound metal flow in the width direction of the workpiece was disturbed as the Pc increased in the edge region. For example, the flat-rolled plate had weaker MSBs due to the relatively uniform Pc or higher Pc value on the central region. By contrast, strong MSBs appeared in the flat-rolled rod and compressed rod because the Pc value in the edge region of these two processes was high.
- The strain homogeneity in a workpiece fabricated via CBFP can be improved by tailoring the distribution of Pc on the surface of the workpiece. To improve the strain homogeneity of the workpiece, the Pc on the workpiece surface should be homogeneous by controlling the initial shape of the workpiece, reduction ratio, lubricants, tool design, and strain hardening rate of material.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Semiatin, S.L.; Jonas, J.J. Formability and Workability of Metals: Plastic Instability and Flow Localization; American Society for Metals USA: Russell Township, OH, USA, 1984. [Google Scholar]
- Paul, H.; Driver, J.H.; Tarasek, A.; Wajda, W.; Miszczyk, M.M. Mechanism of macroscopic shear band formation in plane strain compressed fine-grained aluminium. Mater. Sci. Eng. A 2015, 642, 167–180. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Lahoti, G.D. The occurrence of shear bands in isothermal, hot forging. Metall. Mater. Trans. A 1982, 13, 275–288. [Google Scholar] [CrossRef]
- Lambiase, F.; Ilio, A.D. Deformation inhomogeneity in roll drawing process. J. Manuf. Process. 2012, 14, 208–215. [Google Scholar] [CrossRef]
- Jia, N.; Eisenlohr, P.; Roters, F.; Raabe, D.; Zhao, X. Orientation dependence of shear banding in face-centered-cubic single crystals. Acta Mater. 2012, 60, 3415–3434. [Google Scholar] [CrossRef]
- Cheng, L.; Xue, X.; Tang, B.; Liu, D.; Li, J.; Kou, H.; Li, J. Deformation behavior of hot-rolled IN718 superalloy under plane strain compression at elevated temperature. Mater. Sci. Eng. A 2014, 606, 24–30. [Google Scholar] [CrossRef]
- Tang, B.; Xiang, L.; Cheng, L.; Liu, D.; Kou, H.; Li, J. The formation and evolution of shear bands in plane strain compressed nickel-base superalloy. Metals 2018, 8, 141. [Google Scholar] [CrossRef]
- Lee, D.J.; Yoon, E.Y.; Park, L.J.; Kim, H.S. The dead metal zone in high-pressure torsion. Scr. Mater. 2012, 67, 384–387. [Google Scholar] [CrossRef]
- Eom, J.G.; Son, Y.H.; Jeong, S.W.; Ahn, S.T.; Jang, S.M.; Yoon, D.J.; Joun, M.S. Effect of strain hardening capability on plastic deformation behaviors of material during metal forming. Mater. Des. 2014, 54, 1010–1018. [Google Scholar] [CrossRef]
- Hor, A.; Morel, F.; Lebrun, J.L.; Germain, G. An experimental investigation of the behaviour of steels over large temperature and strain rate ranges. Int. J. Mech. Sci. 2013, 67, 108–122. [Google Scholar] [CrossRef]
- Kazeminezhad, M.; Karimi Taheri, A. Deformation inhomogeneity in flattened copper wire. Mater. Des. 2007, 28, 2047–2053. [Google Scholar] [CrossRef]
- Hwang, J.K. Deformation behaviors of flat rolled wire in twinning-induced plasticity steel. Met. Mater. Int. 2020, 26, 603–616. [Google Scholar] [CrossRef]
- Hwang, J.K. Enhanced homogeneity of a flat-rolled wire in twinning-induced plasticity steel using the pass schedule design. ISIJ Int. 2020, 60, 2493–2502. [Google Scholar] [CrossRef]
- Hwang, J.K. Effects of caliber rolling on microstructure and mechanical properties in twinning-induced plasticity (TWIP) steel. Mater. Sci. Eng. A 2018, 711, 156–164. [Google Scholar] [CrossRef]
- Kim, S.J.; Lee, T.; Hwang, J.K. High-strength bolt manufactured by an extrusion-based forming process using twinning-induced plasticity steel. J. Manuf. Process. 2020, 59, 33–42. [Google Scholar] [CrossRef]
- Hwang, J.K. Inhomogeneity of microstructure and mechanical properties in compression-type bulk formed specimens. Proc. IMechE Part B J. Eng. Manuf. 2023, 237, 1485–1496. [Google Scholar] [CrossRef]
- Orowan, E. The calculation of roll pressure in hot and cold flat rolling. Proc. Inst. Mech. Eng. 1943, 150, 140–167. [Google Scholar] [CrossRef]
- Carlsson, B. The contact pressure distribution in flat rolling of wire. J. Mater. Process. Technol. 1998, 73, 1–6. [Google Scholar] [CrossRef]
- Li, G.J.; Kobayashi, S. Rigid-plastic finite-element analysis of plane strain rolling. J. Eng. Ind. 1982, 104, 55–63. [Google Scholar] [CrossRef]
- Rudnytskyj, A.; Vorlaufer, G.; Leimhofer, J.; Jech, M.; Gachot, C. Estimating the real contact area in lubricated hot rolling of aluminium. Tribol. Int. 2023, 180, 108283. [Google Scholar] [CrossRef]
- Inoue, T.; Yin, F.; Kimura, Y. Strain distribution and microstructural evolution in multi-pass warm caliber rolling. Mater. Sci. Eng. A 2007, 466, 114–122. [Google Scholar] [CrossRef]
- Ghosh, A.K. The influence of strain hardening and strain-rate sensitivity on sheet metal forming. J. Eng. Mater. Technol. 1977, 99, 264–274. [Google Scholar] [CrossRef]
- Wusatowski, Z. Fundamentals of Rolling; Pergamon Press: London, UK; New York, NY, USA, 1969. [Google Scholar]
- Spuzic, S.; Strafford, K.N.; Subramanian, C.; Savage, G. Wear of hot rolling mill rolls: An overview. Wear 1994, 176, 261–271. [Google Scholar] [CrossRef]
- Duan, X.; Sheppard, T. Three dimensional thermal mechanical coupled simulation during hot rolling of aluminium alloy 3003. Int. J. Mech. Sci. 2002, 44, 2155–2172. [Google Scholar] [CrossRef]
- Kazeminezhad, M.; Karimi Taheri, A. Calculation of the rolling pressure distribution and force in wire flat rolling process. J. Mater. Process. Technol. 2006, 171, 253–258. [Google Scholar] [CrossRef]
- Vallellano, C.; Cabanillas, P.A.; Garcia-Lomas, F.J. Analysis of deformations and stresses in flat rolling of wire. J. Mater. Process. Technol. 2008, 195, 63–71. [Google Scholar] [CrossRef]
- Hwang, J.K. Strain hardening rate dependency of deformation shape, strain distribution, and contact pressure during wire flat rolling. J. Iron Steel Res. Int. 2023, 30, 475–486. [Google Scholar] [CrossRef]
- Hartley, P.; Sturgess, C.E.N.; Liu, C.; Rowe, G.W. Experimental and theoretical studies of workpiece deformation, stress, and strain during flat rolling. Int. Mater. Rev. 1989, 34, 19–34. [Google Scholar] [CrossRef]
- Cavaliere, M.A.; Goldschmit, M.B.; Dvorkin, E.N. Finite element analysis of steel rolling processes. Comput. Struct. 2001, 79, 2075–2089. [Google Scholar] [CrossRef]
- Liu, X.; Shi, X.; Li, S.; Xu, J.; Wang, G. FEM analysis of rolling pressure along strip width in cold rolling process. J. Iron Steel Res. Int. 2007, 14, 22–26. [Google Scholar] [CrossRef]
- Tieu, A.K.; Liu, Y.J. Friction variation in the cold-rolling process. Tribol. Int. 2004, 37, 177–183. [Google Scholar] [CrossRef]
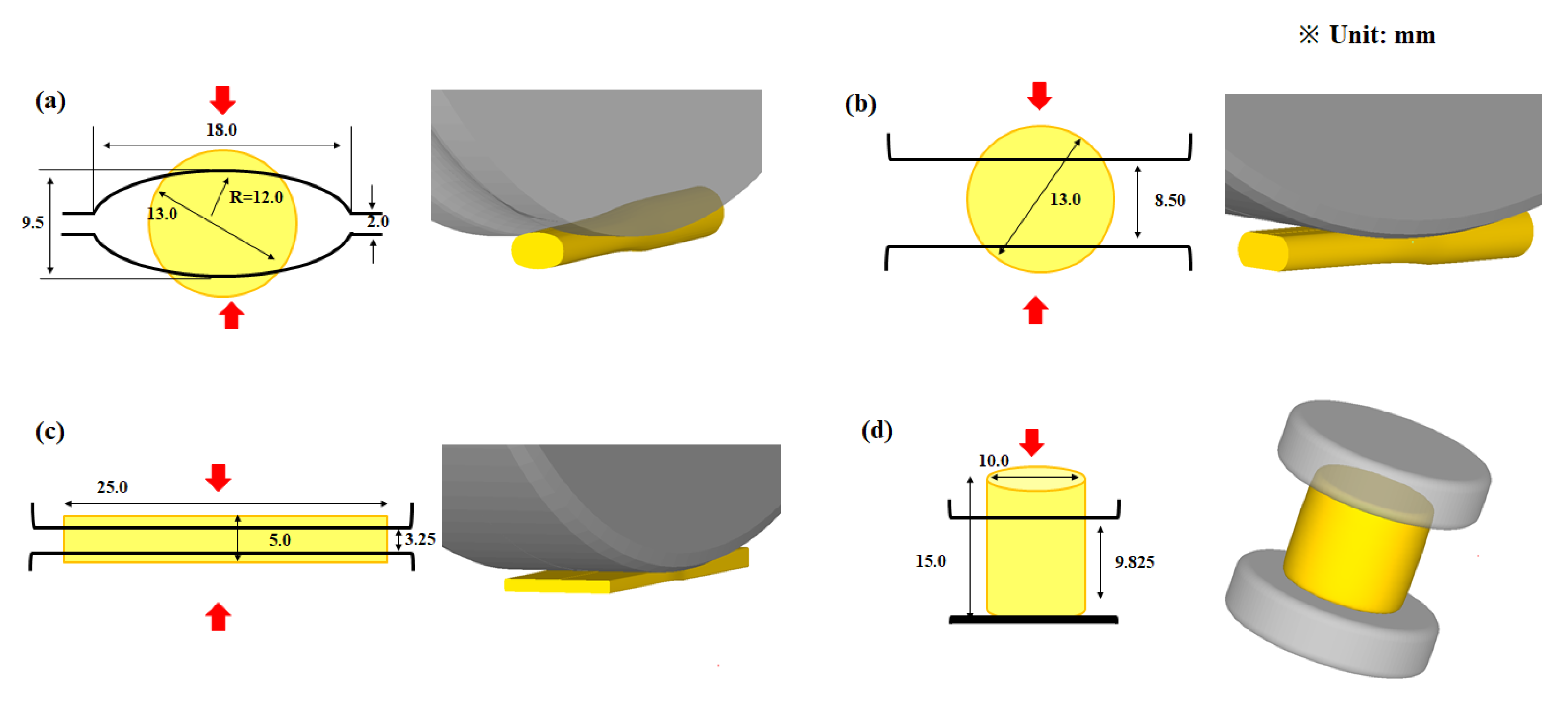


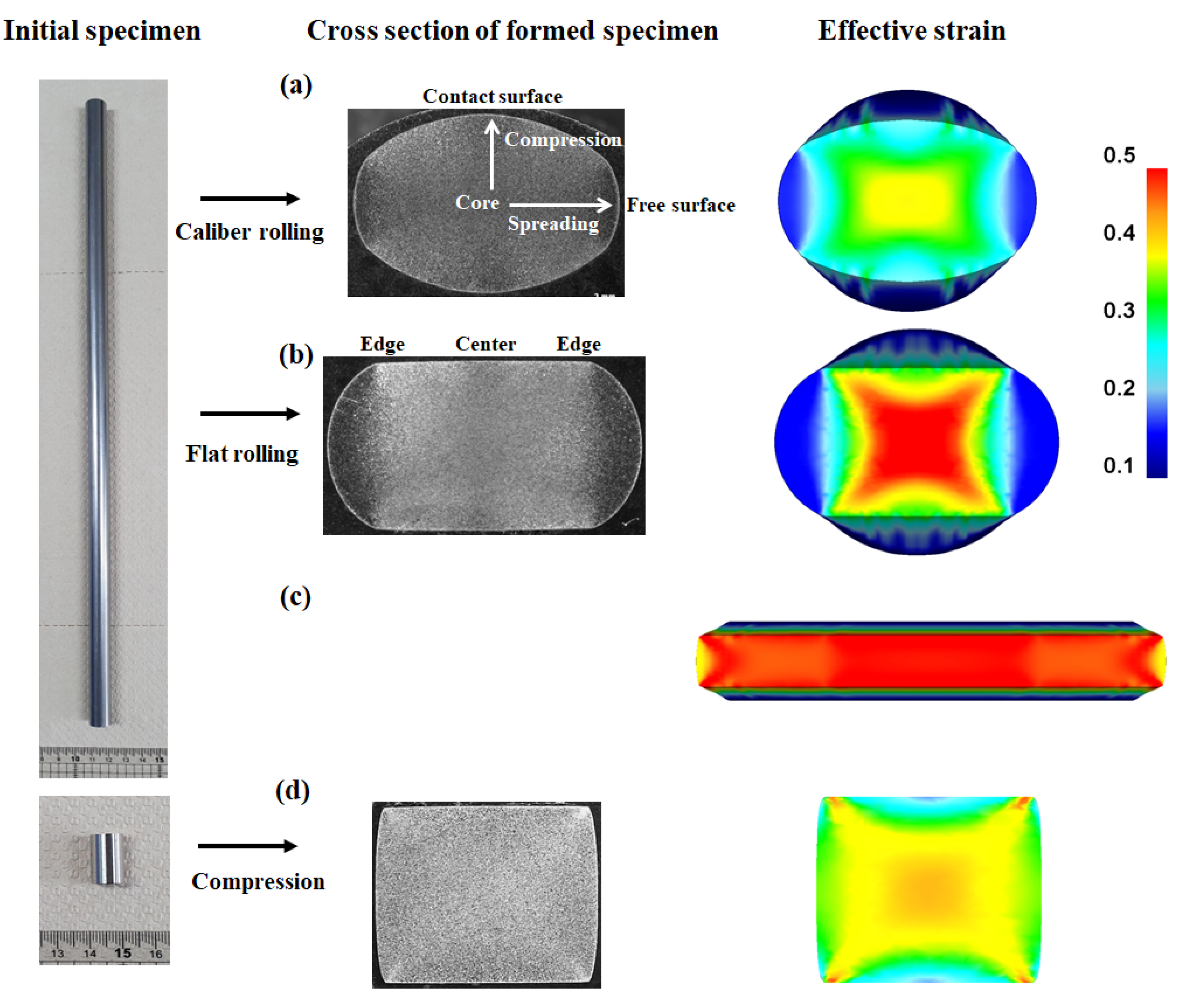
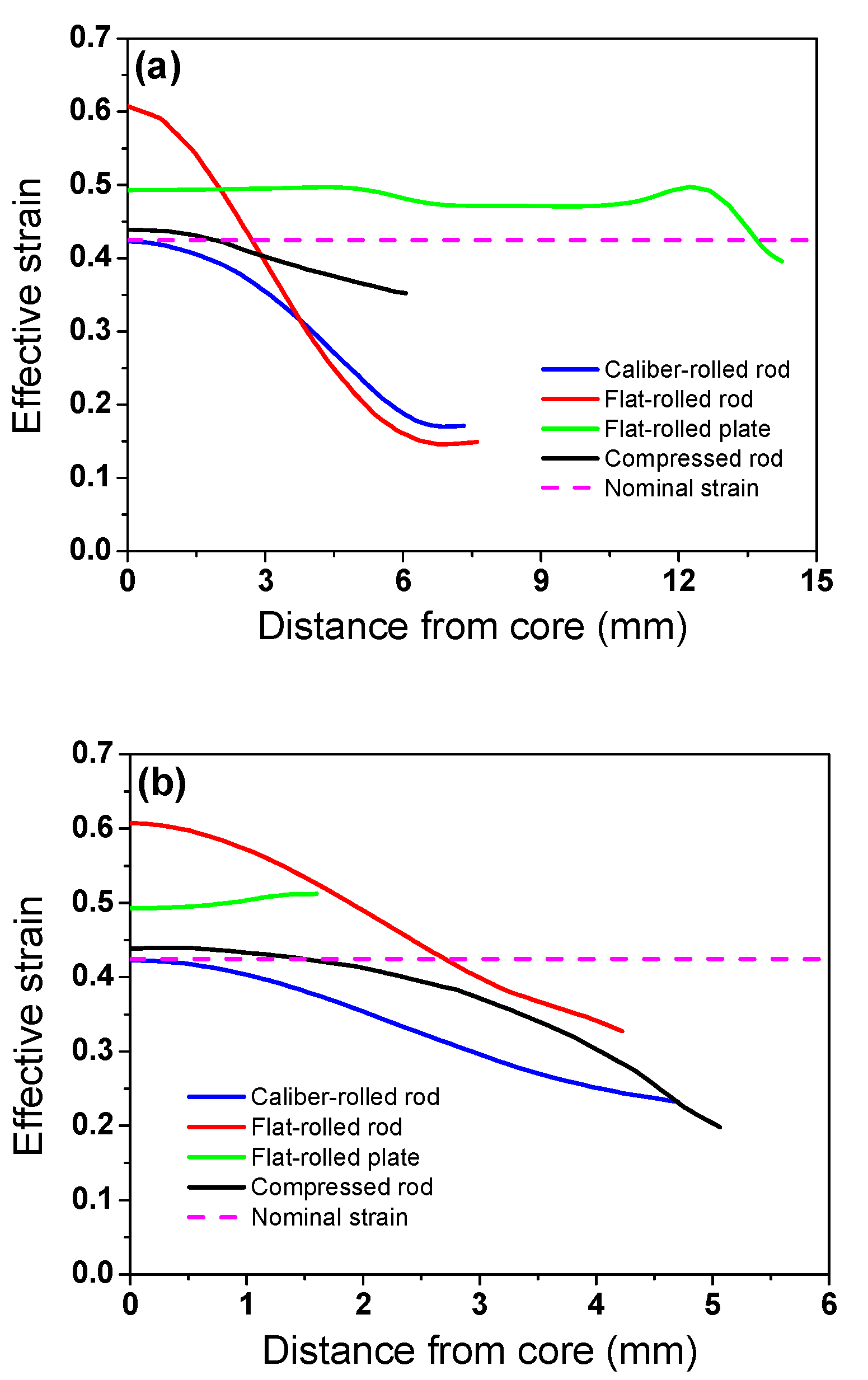
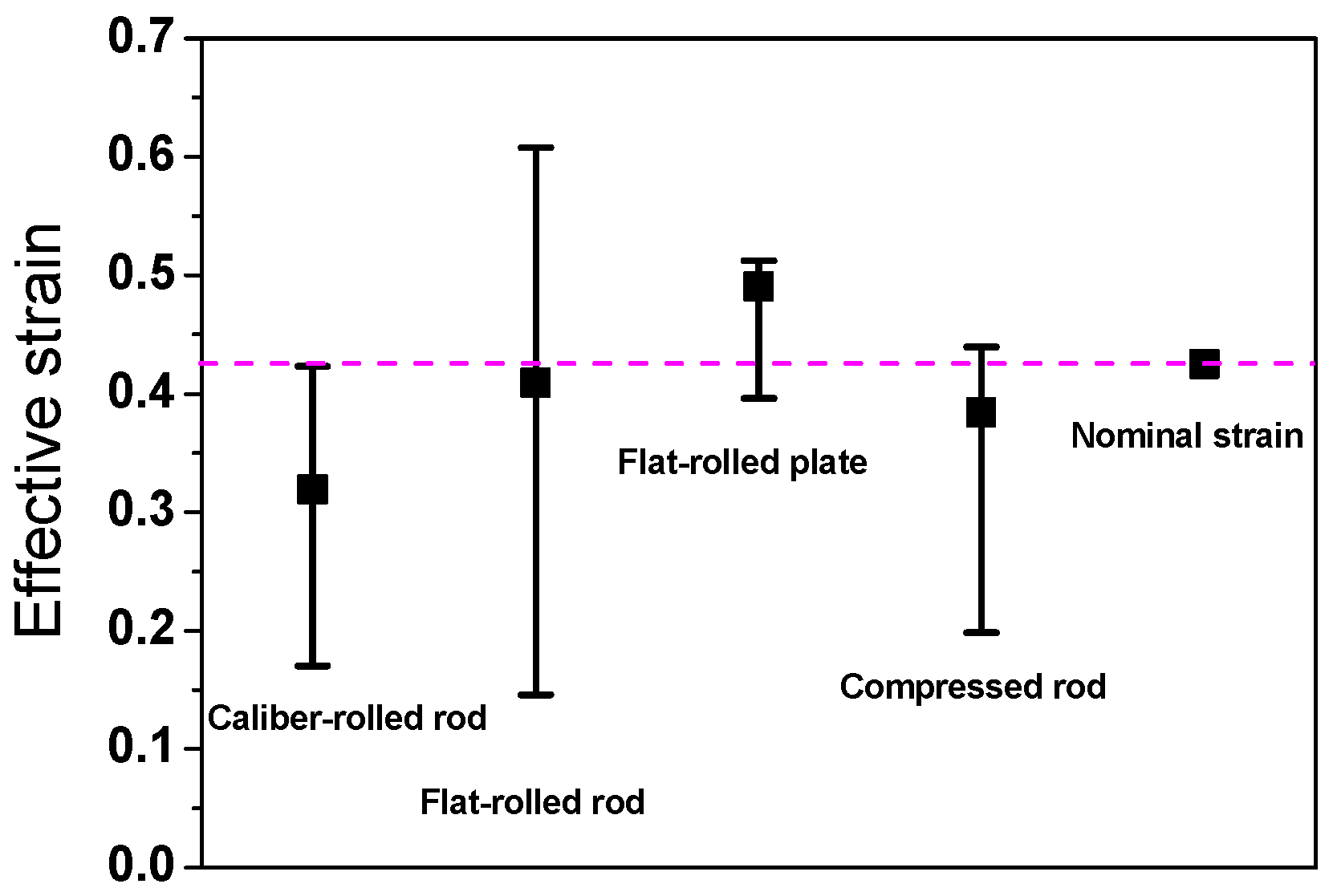
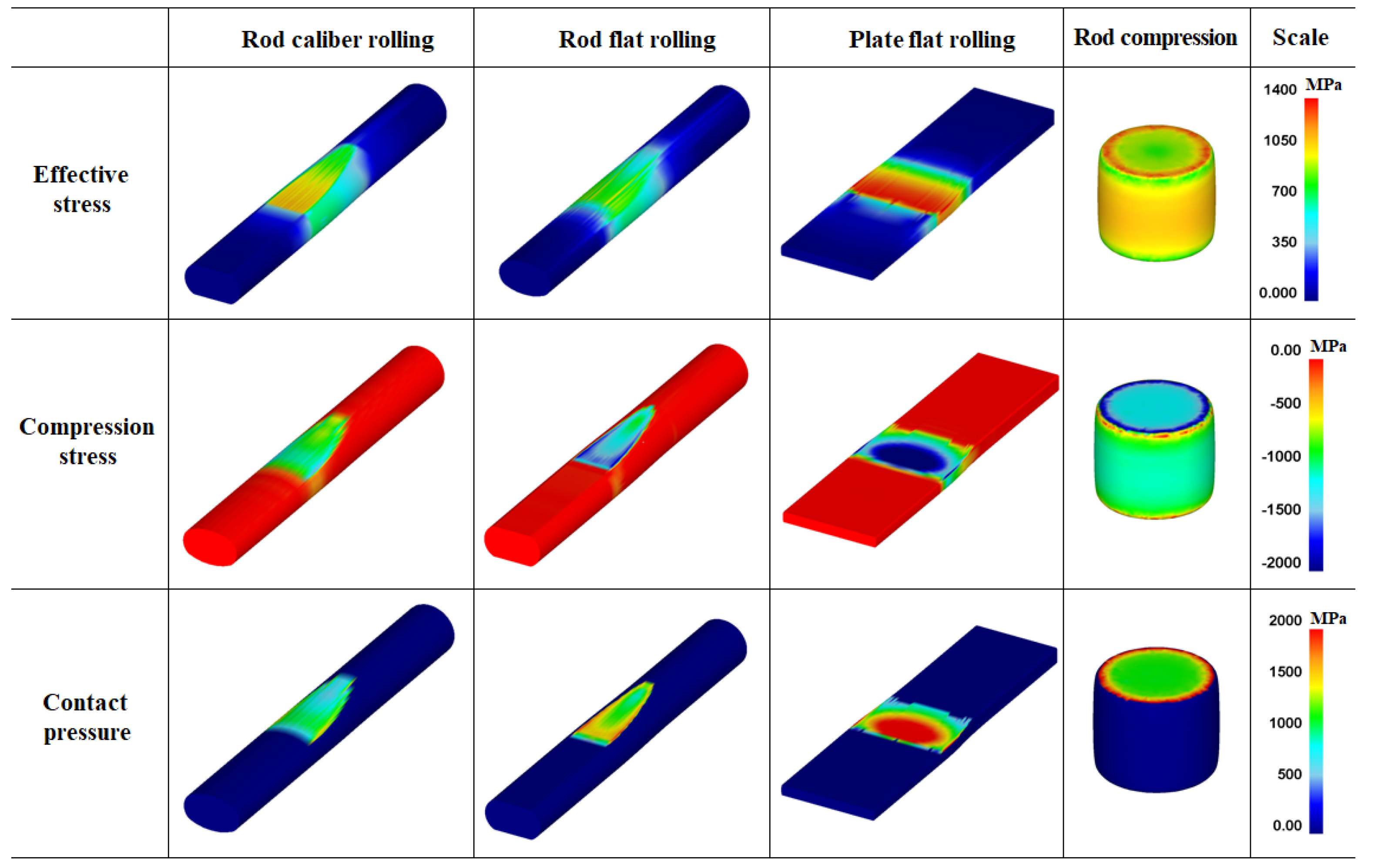


| CBFP | Height Reduction (Rh, %) | Nominal Strain (εn) | Evaluation Method |
|---|---|---|---|
| Rod caliber rolling | 35.2 | 0.43 | Experiment and FEA |
| Rod flat rolling | 34.6 | 0.42 | Experiment and FEA |
| Plate flat rolling | 35.0 | 0.43 | FEA |
| Rod compression | 34.5 | 0.42 | Experiment and FEA |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hwang, J.-K. Effect of Contact Pressure on Strain Distribution during Compression-Type Bulk Forming Processes. Materials 2023, 16, 5041. https://doi.org/10.3390/ma16145041
Hwang J-K. Effect of Contact Pressure on Strain Distribution during Compression-Type Bulk Forming Processes. Materials. 2023; 16(14):5041. https://doi.org/10.3390/ma16145041
Chicago/Turabian StyleHwang, Joong-Ki. 2023. "Effect of Contact Pressure on Strain Distribution during Compression-Type Bulk Forming Processes" Materials 16, no. 14: 5041. https://doi.org/10.3390/ma16145041
APA StyleHwang, J.-K. (2023). Effect of Contact Pressure on Strain Distribution during Compression-Type Bulk Forming Processes. Materials, 16(14), 5041. https://doi.org/10.3390/ma16145041






