A Novel Ultrasonic Fatigue Test and Application in Bending Fatigue of TC4 Titanium Alloy
Abstract
1. Introduction
2. Specimen Design for Very High Cycle Bending Fatigue
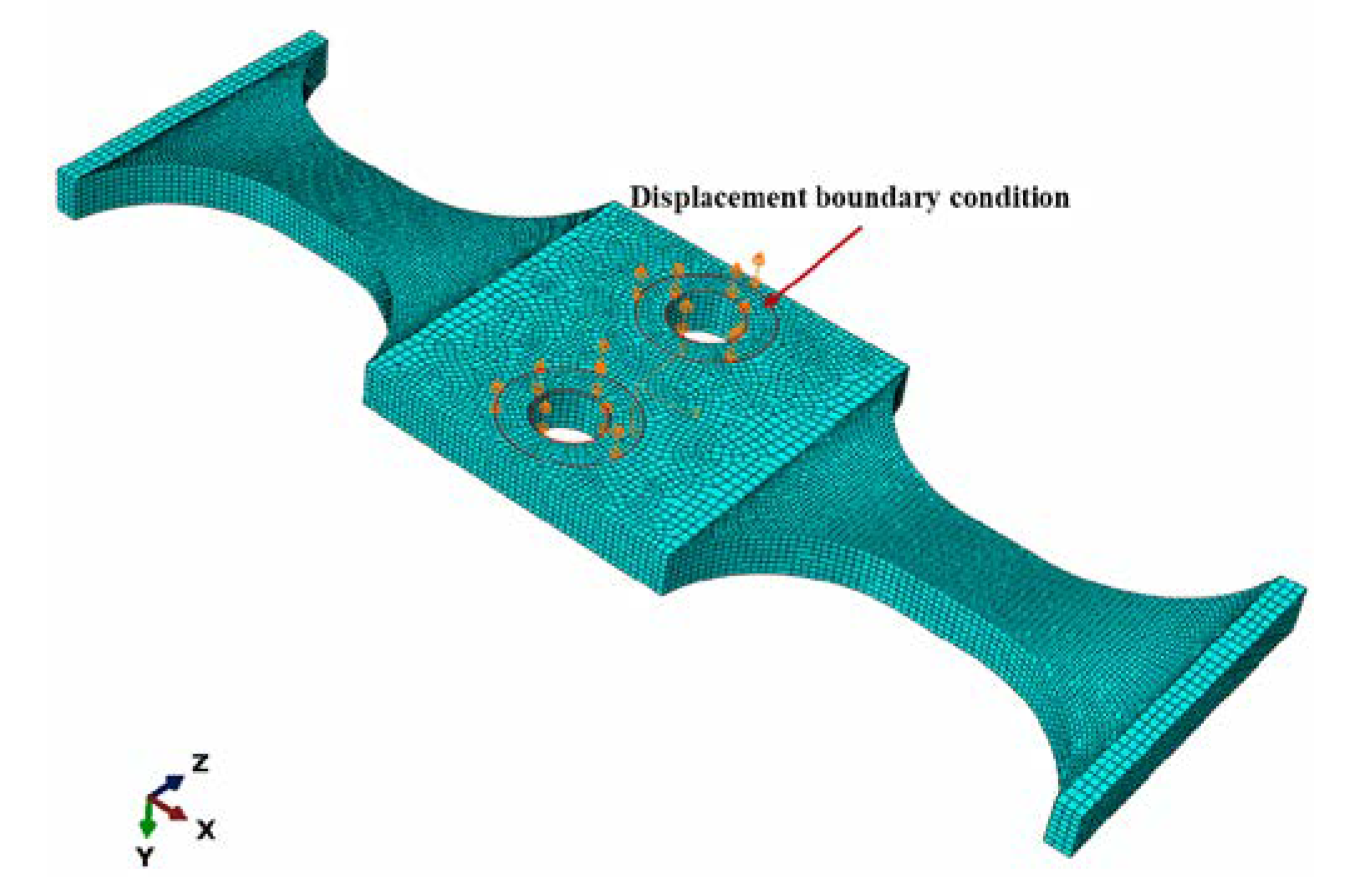
2.1. Specimen Design and Analysis
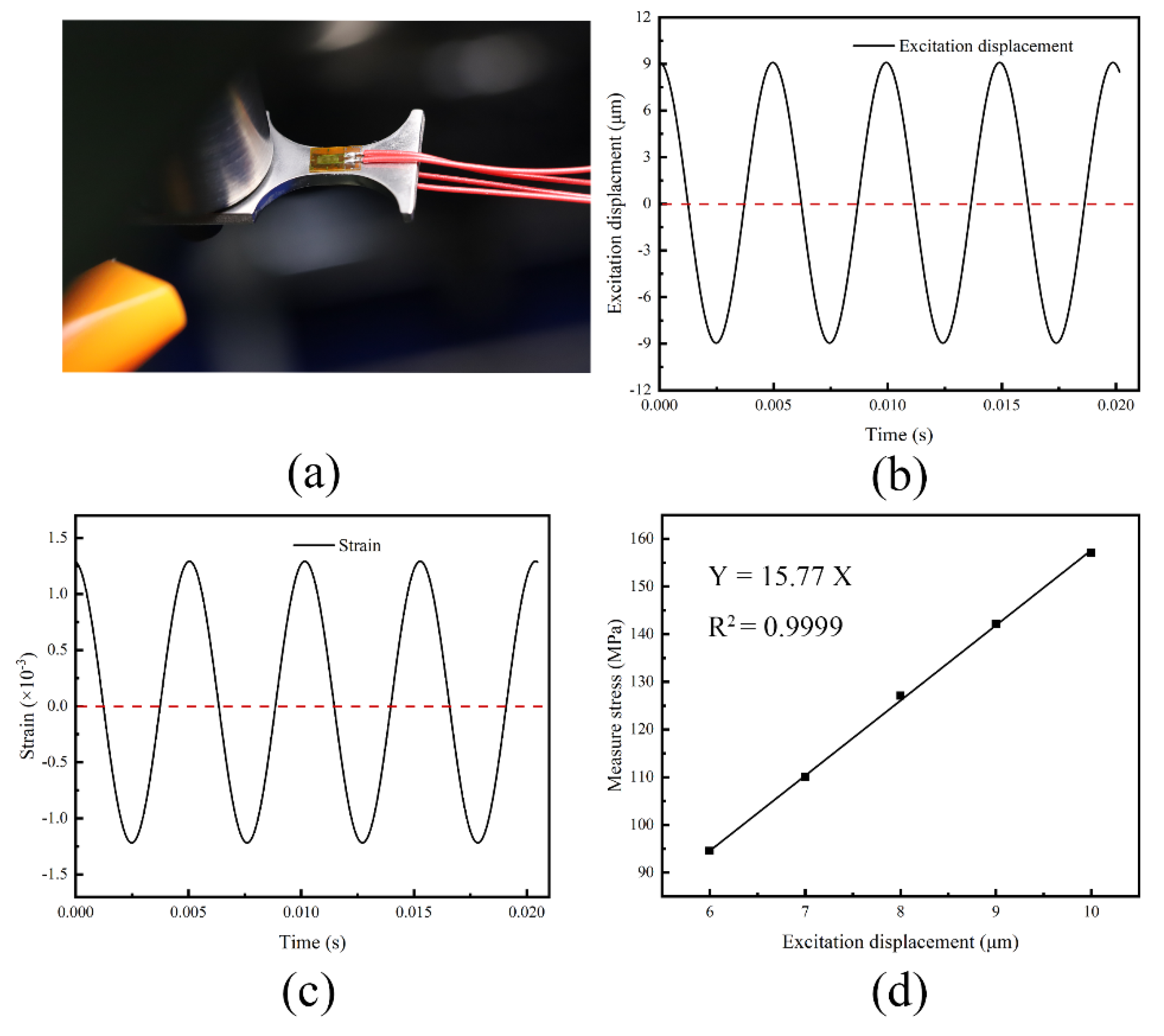
2.2. Stress Calibration
3. Experiment Materials and Methods
3.1. Materials
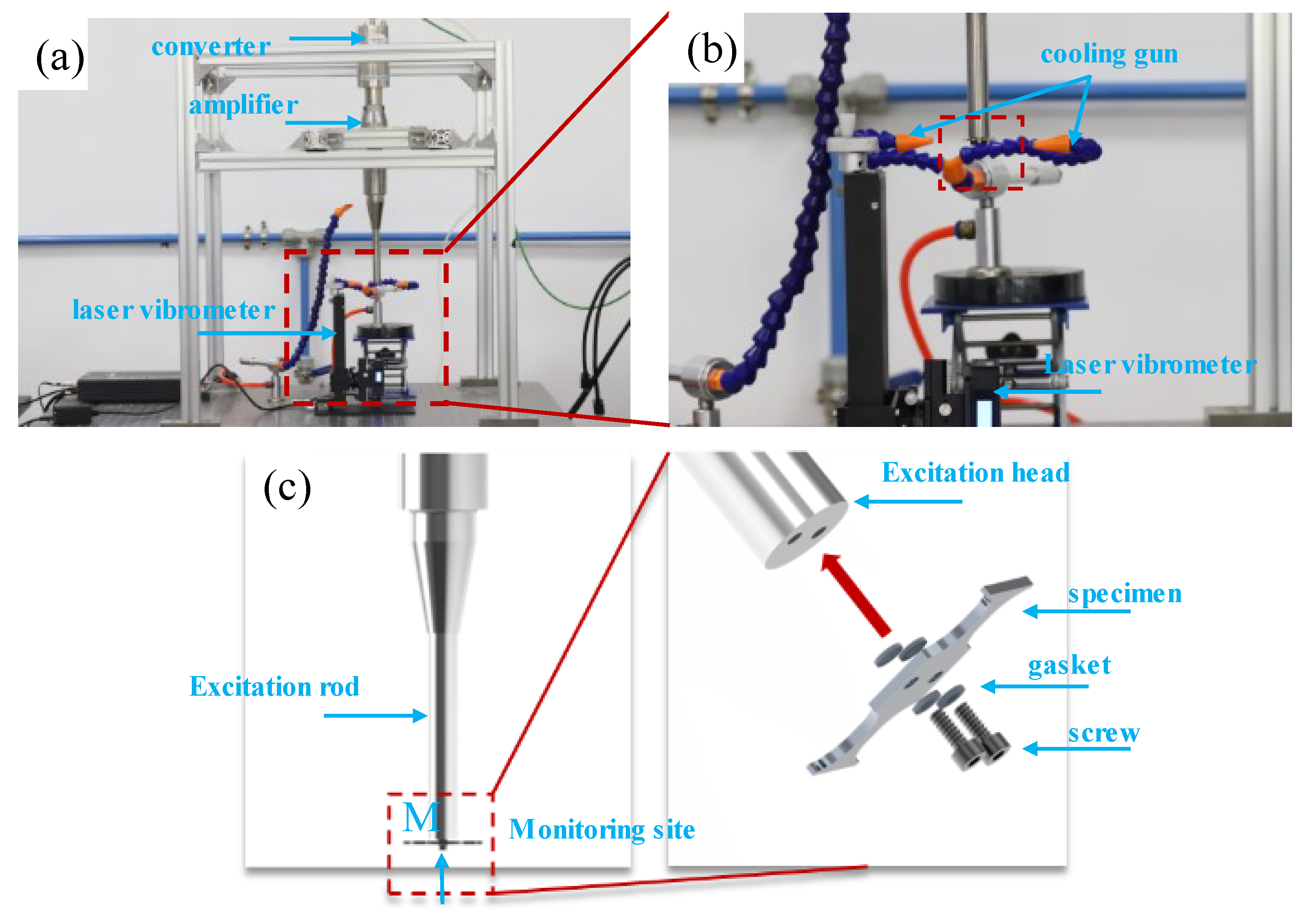
3.2. Ultrasonic Fatigue Test
3.3. Comparison Fatigue Test
4. Results and Discussion
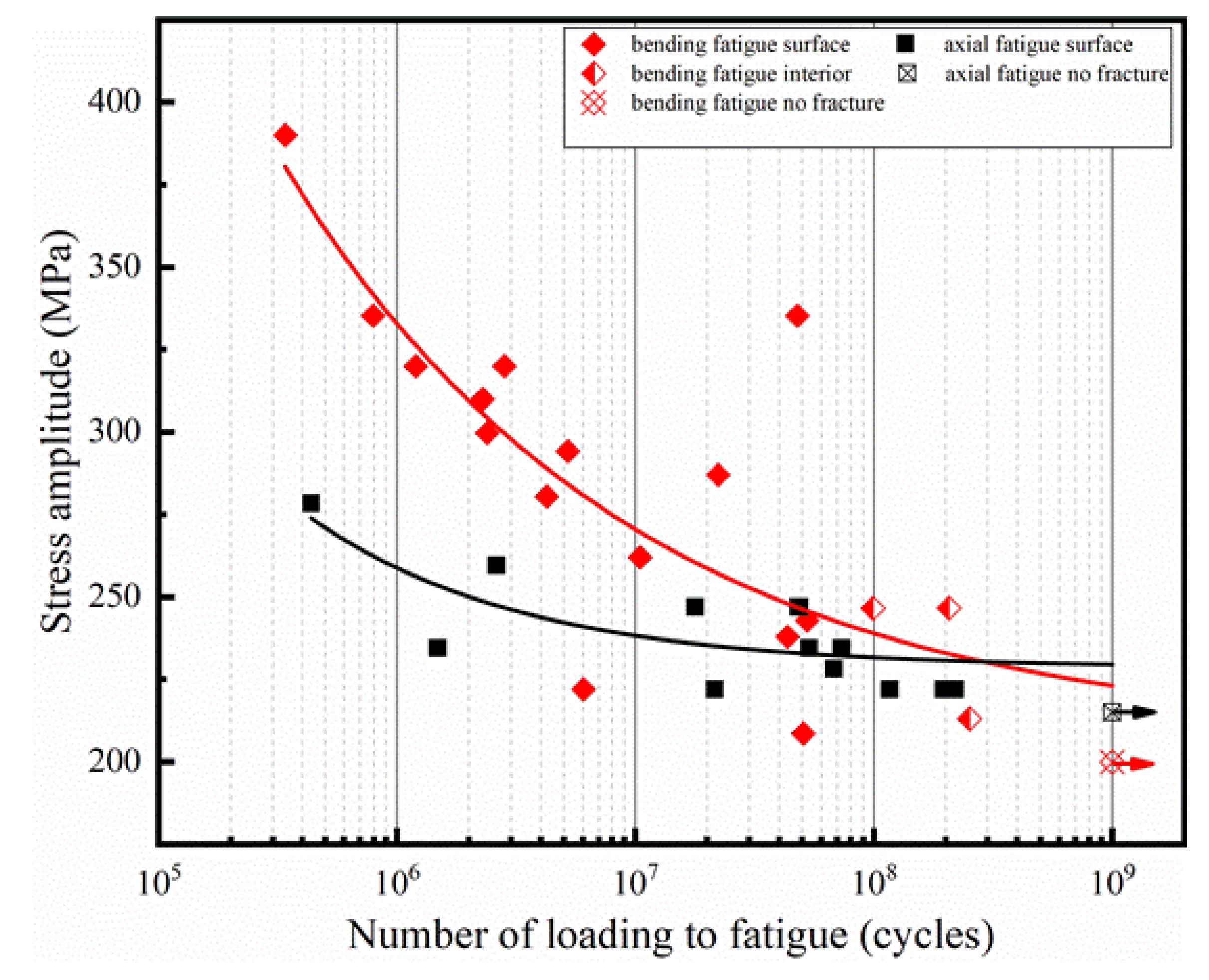
4.1. S-N Curve
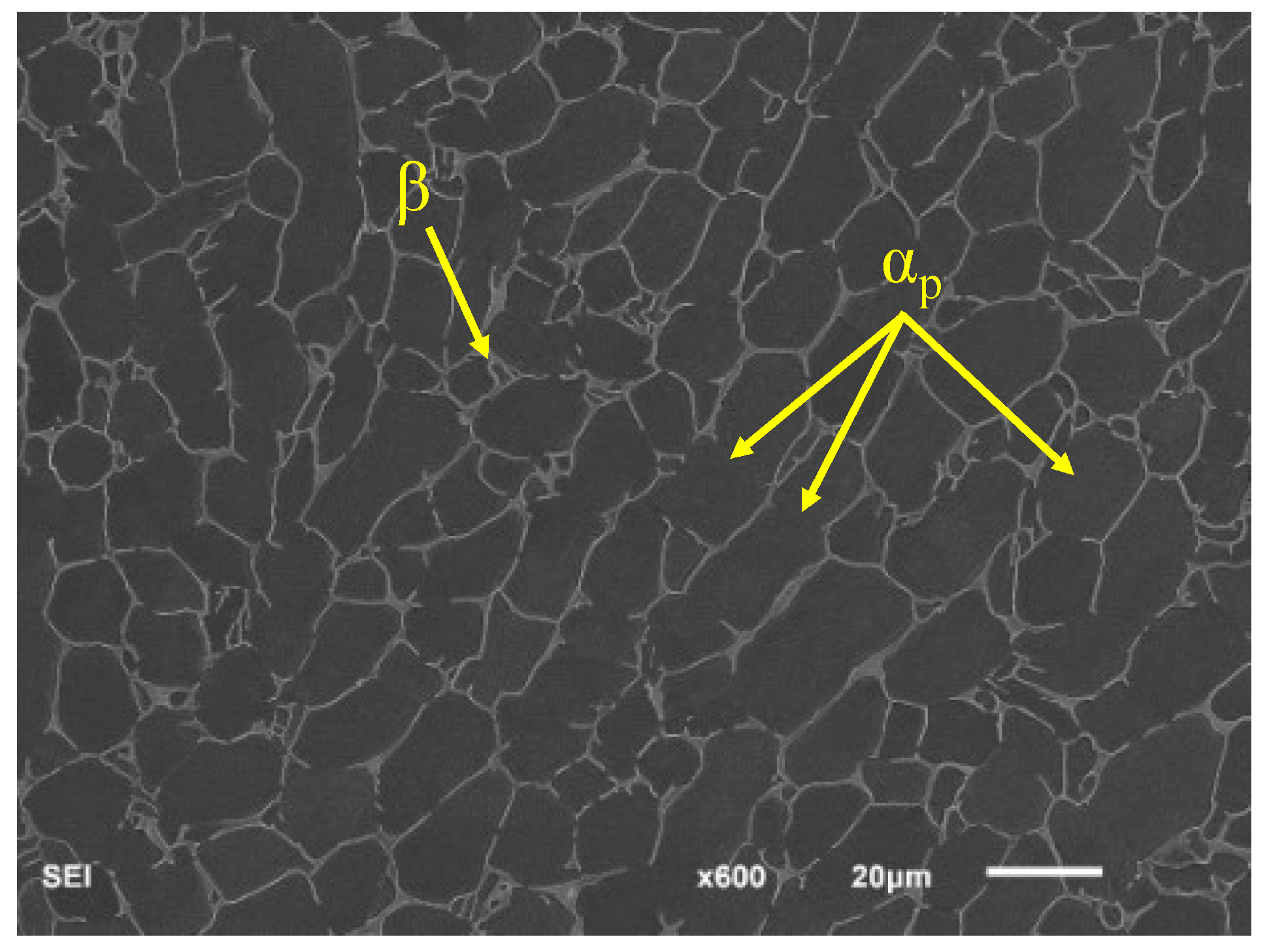
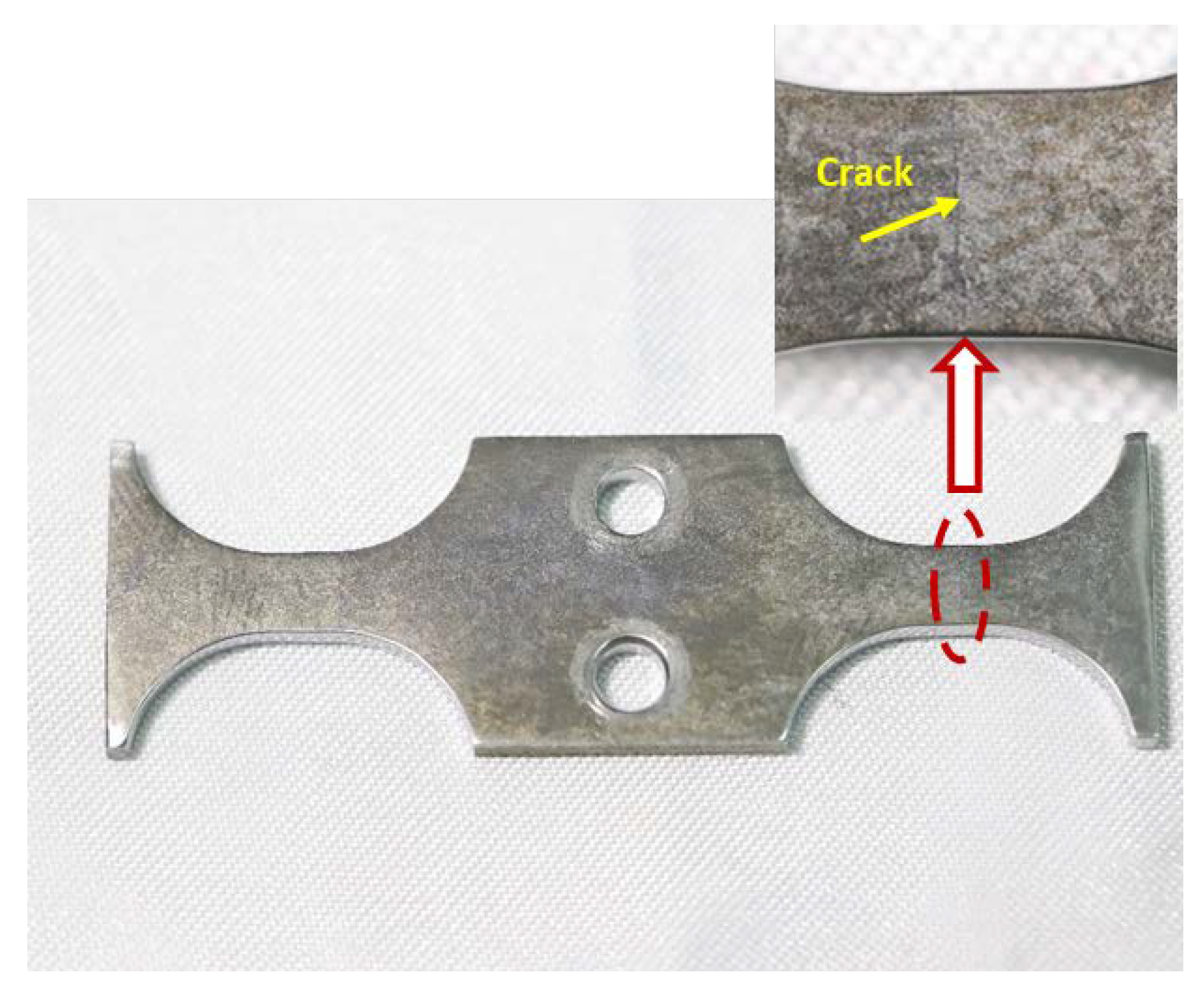
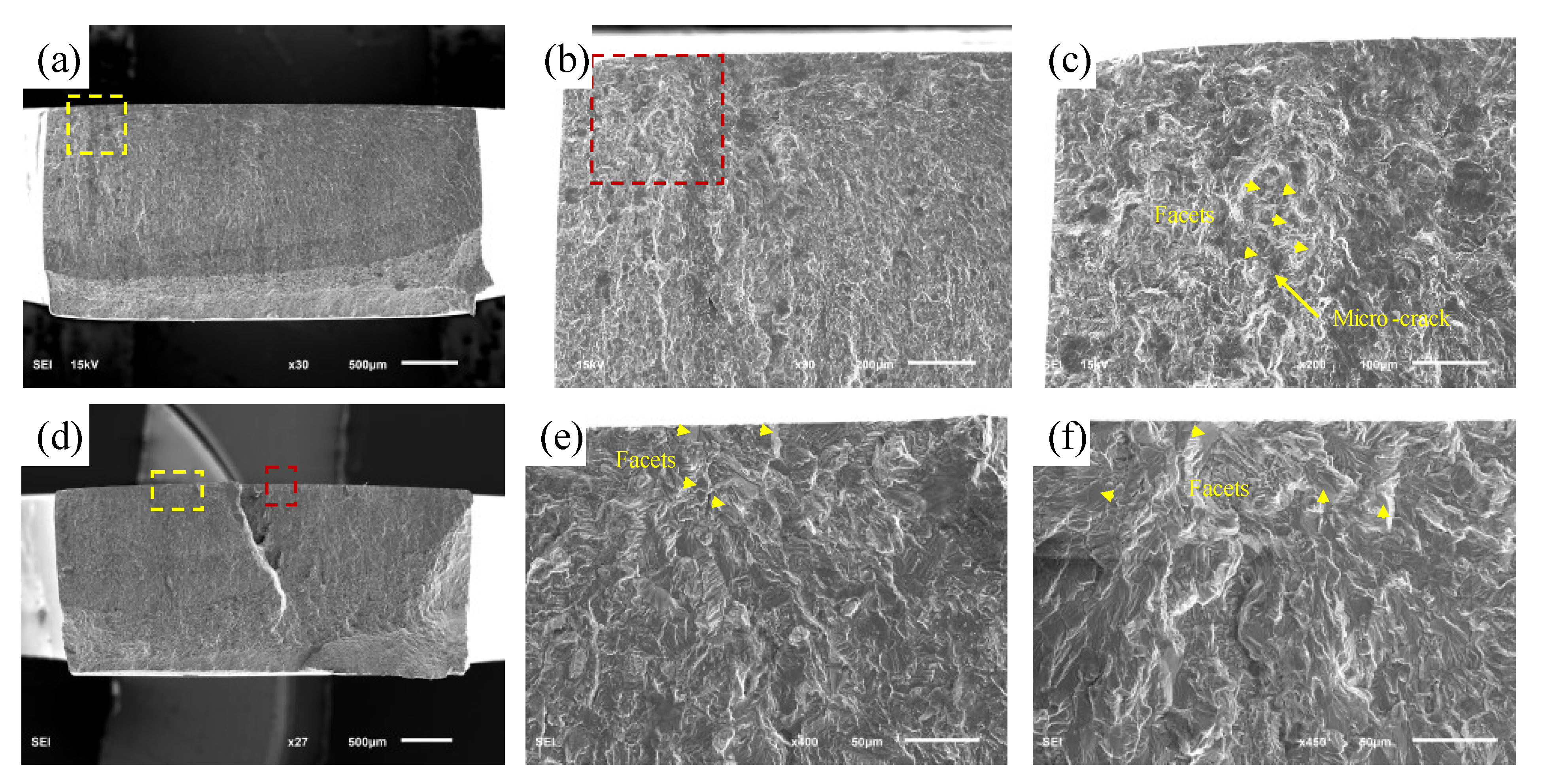
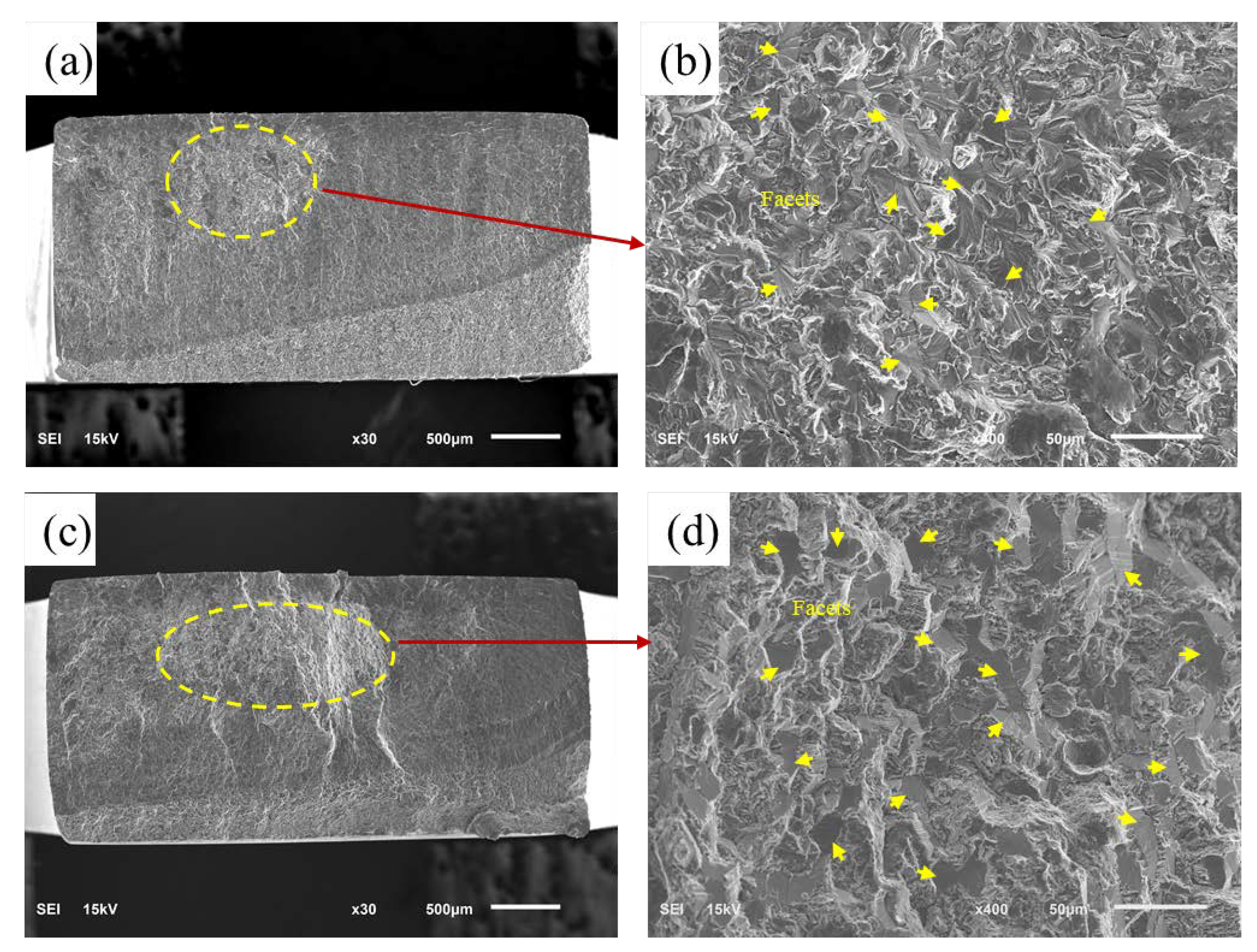
4.2. Fracture Morphology
5. Conclusions
- A new bending fatigue model in the resonant state under ultrasonic loading was developed in which the accelerated bending frequency reached 20 kHz and the symmetrical stress distribution at the gauge section was optimized compared with the conventional cantilever model.
- The S-N curve for the studied material showed that the fatigue data of the bending loading and axial loading intended to have an intersection point when the cycles went beyond 108. Re-discussion of the conservative design strategy may be required regarding bending loading and axial loading in the VHCF regime.
- There were two types of bending fatigue loading—surface crack initiation and internal crack initiation. As the cycle life increased, the possibility of an internal crack occurring also increased.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| R | Stress ratio (minimum stress/maximum stress) |
| Stress amplitude | |
| Material constants | |
| Number of cycles to failure | |
| Coefficient of displacement to stress | |
| A0 | Excitation displacement of the system |
| R2 | Coefficient of determination |
| HCF | High cycle fatigue ( > 105) |
| VHCF | Very high cycle fatigue ( > 107) |
| FEA | Finite element analysis |
References
- Takeuchi, E.; Furuya, Y.; Nagashima, N.; Matsuoka, S. The effect of frequency on the giga-cycle fatigue properties of a Ti-6Al-4V alloy. Fatigue Fract. Eng. Mater. Struct. 2008, 31, 599–605. [Google Scholar] [CrossRef]
- Hong, Y.; Sun, C. The nature and the mechanism of crack initiation and early growth for very-high-cycle fatigue of metallic materials—An overview. Theor. Appl. Fract. Mech. 2017, 92, 331–350. [Google Scholar] [CrossRef]
- Yang, K.; He, C.; Huang, Q.; Huang, Z.Y.; Wang, C.; Wang, Q.; Liu, Y.J.; Zhong, B. Very high cycle fatigue behaviors of a turbine engine blade alloy at various stress ratios. Int. J. Fatigue 2017, 99, 35–43. [Google Scholar] [CrossRef]
- Bathias, C. Piezoelectric fatigue testing machines and devices. Int. J. Fatigue 2006, 28, 1438–1445. [Google Scholar] [CrossRef]
- Pan, X.; Qian, G.; Wu, S.; Fu, Y.; Hong, Y. Internal crack characteristics in very-high-cycle fatigue of a gradient structured titanium alloy. Sci. Rep. 2020, 10, 4742. [Google Scholar] [CrossRef]
- Lesperance, X.; Ilie, P.; Ince, A. Very high cycle fatigue characterization of additively manufactured AlSi10Mg and AlSi7Mg aluminium alloys based on ultrasonic fatigue testing. Fatigue Fract. Eng. Mater. Struct. 2020, 44, 876–884. [Google Scholar] [CrossRef]
- Sharma, A.; Oh, M.C.; Ahn, B. Recent Advances in Very High Cycle Fatigue Behavior of Metals and Alloys—A Review. Metals 2020, 10, 1200. [Google Scholar]
- Stanzl-Tschegg, S. Very high cycle fatigue measuring techniques. Int. J. Fatigue 2014, 60, 2–17. [Google Scholar] [CrossRef]
- Oguma, H.; Nakamura, T. Fatigue crack propagation properties of Ti–6Al–4V in vacuum environments. Int. J. Fatigue 2013, 50, 89–93. [Google Scholar] [CrossRef]
- Sakai, T. Review and Prospects for Current Studies on Very High Cycle Fatigue of Metallic Materials for Machine Structural Use. J. Solid Mech. Mater. Eng. 2009, 3, 425–439. [Google Scholar] [CrossRef]
- Hong, Y.; Liu, X.; Lei, Z.; Sun, C. The formation mechanism of characteristic region at crack initiation for very-high-cycle fatigue of high-strength steels. Int. J. Fatigue 2016, 89, 108–118. [Google Scholar] [CrossRef]
- Nie, B.; Zhao, Z.; Chen, D.; Liu, S.; Lu, M.; Zhang, J.; Liang, F. Effect of Basketweave Microstructure on Very High Cycle Fatigue Behavior of TC21 Titanium Alloy. Metals 2018, 8, 401. [Google Scholar] [CrossRef]
- Jebieshia, T.R.; Kim, J.M.; Kang, J.W.; Son, S.W.; Kim, H.D. Microstructural and Very High Cycle Fatigue (VHCF) Behavior of Ti6Al4V-A Comparative Study. Materials 2020, 13, 1948. [Google Scholar] [CrossRef] [PubMed]
- Guennec, B.; Ueno, A.; Sakai, T.; Takanashi, M.; Itabashi, Y. Effect of the loading frequency on fatigue properties of JIS S15C low carbon steel and some discussions based on micro-plasticity behavior. Int. J. Fatigue 2014, 66, 29–38. [Google Scholar] [CrossRef]
- Macek, W.; Owsiński, R.; Trembacz, J.; Branco, R. Three-dimensional fractographic analysis of total fracture areas in 6082 aluminium alloy specimens under fatigue bending with controlled damage degree. Mech. Mater. 2020, 147, 103410. [Google Scholar] [CrossRef]
- Tomaszewski, T.; Strzelecki, P.; Mazurkiewicz, A.; Musial, J. Probabilistic Estimation of Fatigue Strength for Axial and Bending Loading in High-Cycle Fatigue. Materials 2020, 13, 1148. [Google Scholar] [CrossRef]
- Nadot, Y. Fatigue from Defect: Influence of Size, Type, Position, Morphology and Loading. Int. J. Fatigue 2022, 154, 106531. [Google Scholar] [CrossRef]
- Kawai, M. 2D woven fabric composites under fatigue loading of different types and in different environmental conditions. In Fatigue of Textile Composites; Woodhead Publishing: Cambridge, UK, 2015; pp. 149–191. [Google Scholar]
- Cao, X.; Zhu, J.; Gao, F.; Gao, Z. Influence of Fine Grains on the Bending Fatigue Behavior of Two Implant Titanium Alloys. Materials 2020, 14, 171. [Google Scholar] [CrossRef]
- Sakai, T.; Oguma, N.; Morikawa, A. Microscopic and nanoscopic observations of metallurgical structures around inclusions at interior crack initiation site for a bearing steel in very high-cycle fatigue. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 1305–1314. [Google Scholar] [CrossRef]
- Shrestha, R.; Simsiriwong, J.; Shamsaei, N. Fatigue behavior of additive manufactured 316L stainless steel under axial versus rotating-bending loading: Synergistic effects of stress gradient, surface roughness, and volumetric defects. Int. J. Fatigue 2021, 144, 106063. [Google Scholar] [CrossRef]
- Olmi, G.; Freddi, A. A new method for modelling the support effect under rotating bending fatigue: Application to Ti-6Al-4V alloy, with and without shot peening. Fatigue Fract. Eng. Mater. Struct. 2013, 36, 981–993. [Google Scholar] [CrossRef]
- Bao, X.; Cheng, L.; Ding, J.; Chen, X.; Lu, K.; Cui, W. The Effect of Microstructure and Axial Tension on Three-Point Bending Fatigue Behavior of TC4 in High Cycle and Very High Cycle Regimes. Materials 2019, 13, 68. [Google Scholar] [CrossRef] [PubMed]
- Macek, W. Fracture surface formation of notched 2017A-T4 aluminium alloy under bending fatigue. Int. J. Fract. 2021, 234, 141–157. [Google Scholar] [CrossRef]
- Wang, C.; Chen, X.; Cheng, L.; Ding, J. High-cycle and very-high-cycle fatigue properties of CFRP-aramid honeycomb sandwich structure in three-point bending. Int. J. Fatigue 2022, 155, 106576. [Google Scholar] [CrossRef]
- Hu, Y.; Chen, Y.; He, C.; Liu, Y.; Wang, Q.; Wang, C. Bending Fatigue Behavior of 316L Stainless Steel up to Very High Cycle Fatigue Regime. Materials 2020, 13, 4820. [Google Scholar] [CrossRef]
- Ghadimi, H.; Jirandehi, A.P.; Nemati, S.; Guo, S. Small-sized specimen design with the provision for high-frequency bending-fatigue testing. Fatigue Fract. Eng. Mater. Struct. 2021, 44, 3517–3537. [Google Scholar] [CrossRef]
- Jemii, H.; Bahri, A.; Taktak, R.; Guermazi, N.; Lebon, F. Mechanical behavior and fracture characteristics of polymeric pipes under curved three point bending tests: Experimental and numerical approaches. Eng. Fail. Anal. 2022, 138, 106352. [Google Scholar] [CrossRef]
- Yang, D.; Tang, S.; Hu, Y.; Nikitin, A.; Wang, Q.; Liu, Y.; Li, L.; He, C.; Li, Y.; Xu, B.; et al. A Novel Model of Ultrasonic Fatigue Test in Pure Bending. Materials 2022, 15, 4864. [Google Scholar] [CrossRef]
- Ding, K. Three-dimensional Dynamic Finite Element Analysis of Multiple Laser Shock Peening Processes. Surf. Eng. 2013, 19, 351–358. [Google Scholar] [CrossRef]
- Vasu, A.; Grandhi, R.V. Effects of curved geometry on residual stress in laser peening. Surf. Coat. Technol. 2013, 218, 71–79. [Google Scholar] [CrossRef]
- Jiao, S.; Gao, C.; Cheng, L.; Li, X.; Feng, Y. A Very High-Cycle Fatigue Test and Fatigue Properties of TC17 Titanium Alloy. J. Mater. Eng. Perform. 2016, 25, 1085–1093. [Google Scholar] [CrossRef]
- Wang, B.; Cheng, L.; Li, D. Study on very high cycle fatigue properties of forged TC4 titanium alloy treated by laser shock peening under three-point bending. Int. J. Fatigue 2022, 156, 106668. [Google Scholar] [CrossRef]
- Yang, Z.; Li, H.; Wen, J.; Huang, F.; Wang, Z.; Yi, Z.; Xie, Y.; Dong, H. The microstructure evolution of ballastless track high-strength concrete exposed to compressive and flexural fatigue loads. Int. J. Fatigue 2023, 166, 107247. [Google Scholar] [CrossRef]
- Li, W.; Sakai, T.; Li, Q.; Lu, L.T.; Wang, P. Effect of loading type on fatigue properties of high strength bearing steel in very high cycle regime. Mater. Sci. Eng. A 2011, 528, 5044–5052. [Google Scholar] [CrossRef]
- Wu, G.Q.; Shi, C.L.; Sha, W.; Sha, A.X.; Jiang, H.R. Effect of microstructure on the fatigue properties of Ti–6Al–4V titanium alloys. Mater Des. 2013, 46, 668–674. [Google Scholar] [CrossRef]
- Rack, H.J.; Qazi, J.I. Titanium alloys for biomedical applications. Mater. Sci. Eng. C 2006, 26, 1269–1277. [Google Scholar] [CrossRef]
- Bathias, B. There is no infinite fatigue life in metallic materials. Fatigue Fract. Eng. Mater. Struct. 1999, 22, 559–565. [Google Scholar] [CrossRef]
- Liu, F.; Peng, H.; Liu, Y.; Wang, C.; Wang, Q.; Chen, Y. Crack initiation mechanism of titanium alloy in very high cycle fatigue regime at 400℃ considering stress ratio effect. Int. J. Fatigue 2022, 163, 107012. [Google Scholar] [CrossRef]
- Nie, X.; He, W.; Cao, Z.; Song, J.; Li, X.; Pang, Z.; Yan, X. Experimental study and fatigue life prediction on high cycle fatigue performance of laser-peened TC4 titanium alloy. Mater. Sci. Eng. A 2021, 822, 141658. [Google Scholar] [CrossRef]
- Xu, W.; Chen, X.; Gao, Z.; Li, Y.; He, Y.; Tao, C. Fatigue behaviors of a titanium alloy in the VHCF regime based on a vibration-based bending fatigue test. Fatigue Fract. Eng. Mater. Struct. 2022, 45, 2549–2562. [Google Scholar] [CrossRef]
- Hong, Y.; Zhao, A.; Qian, G.; Zhou, C. Fatigue Strength and Crack Initiation Mechanism of Very-High-Cycle Fatigue for Low Alloy Steels. Metall. Mater. Trans. A 2011, 43, 2753–2762. [Google Scholar] [CrossRef]
- Xu, W.; Zhao, Y.; Chen, X.; Zhong, B.; Yu, H.; He, Y.; Tao, C. An Ultra-High Frequency Vibration-Based Fatigue Test and Its Comparative Study of a Titanium Alloy in the VHCF Regime. Metals 2020, 10, 1415. [Google Scholar] [CrossRef]
- Pan, X.; Su, H.; Sun, C.; Hong, Y. The behavior of crack initiation and early growth in high-cycle and very-high-cycle fatigue regimes for a titanium alloy. Int. J. Fatigue 2018, 115, 67–78. [Google Scholar] [CrossRef]
- Huang, Z.Y.; Liu, H.Q.; Wang, H.M.; Wagner, D.; Khan, M.K.; Wang, Q.Y. Effect of stress ratio on VHCF behavior for a compressor blade titanium alloy. Int. J. Fatigue 2016, 93, 232–237. [Google Scholar] [CrossRef]
- Chai, G.; Forsman, T.; Gustavsson, F.; Wang, C. Formation of fine grained area in martensitic steel during very high cycle fatigue. Fatigue Fract. Eng. Mater. Struct. 2015, 38, 1315–1323. [Google Scholar] [CrossRef]
- Bridier, F.; McDowell, D.; Villechaise, P.; Mendez, J. Crystal plasticity modeling of slip activity in Ti–6Al–4V under high cycle fatigue loading. Int. J. Plast. 2009, 25, 1066–1082. [Google Scholar] [CrossRef]
- McDowell, D.; Dunne, F. Microstructure-sensitive computational modeling of fatigue crack formation. Int. J. Fatigue 2010, 32, 1521–1542. [Google Scholar] [CrossRef]
- Liu, F.; He, C.; Chen, Y.; Zhang, H.; Wang, Q.; Liu, Y. Effects of defects on tensile and fatigue behaviors of selective laser melted titanium alloy in very high cycle regime. Int. J. Fatigue 2020, 140, 105795. [Google Scholar] [CrossRef]



| Young’s Modulus (GPa) | Yield Strength (MPa) | Tensile Strength (MPa) | Poisson’s Ratio | Hardness (Hv) |
|---|---|---|---|---|
| 113 | 915 | 954 | 0.33 | 330 |
| Bending Load | Axial Load | ||||
|---|---|---|---|---|---|
| Specimen No. | Stress Level (MPa) | Fatigue Cycle (Cycles) | Specimen No. | Stress Level (MPa) | Fatigue Cycle (Cycles) |
| 1 | 390.14 | 3.39 × 105 | 1 | 278.29 | 4.38 × 105 |
| 2 | 335.41 | 4.78 × 107 | 2 | 259.49 | 2.61× 106 |
| 3 | 335.41 | 7.96 × 105 | 3 | 246.96 | 1.79 × 107 |
| 4 | 320.00 | 1.20 × 106 | 4 | 246.96 | 4.90 × 107 |
| 5 | 320.00 | 2.82 × 106 | 5 | 246.96 | 4.84 × 107 |
| 6 | 310.00 | 2.29 × 106 | 6 | 234.43 | 1.49 × 106 |
| 7 | 299.75 | 2.39 × 106 | 7 | 234.43 | 5.35 × 107 |
| 8 | 294.25 | 5.20 × 106 | 8 | 234.43 | 7.36 × 107 |
| 9 | 287.07 | 2.23 × 107 | 9 | 228.16 | 6.80 × 107 |
| 10 | 280.50 | 4.25 × 106 | 10 | 221.90 | 1.17 × 108 |
| 11 | 262.10 | 1.05 × 107 | 11 | 221.90 | 2.16 × 107 |
| 12 | 246.77 | 2.07 × 108 | 12 | 221.90 | 2.20 × 108 |
| 13 | 246.66 | 9.91 × 107 | 13 | 221.90 | 1.98 × 108 |
| 14 | 243.02 | 5.26 × 107 | 14 | 215.00 | 1.00 × 109 |
| 15 | 238.03 | 4.35 × 107 | |||
| 16 | 222.01 | 6.05 × 106 | |||
| 17 | 213.10 | 2.53 × 108 | |||
| 18 | 208.59 | 5.06 × 107 | |||
| 19 | 200.00 | 1.00 × 109 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, S.; Wang, X.; Huang, B.; Yang, D.; Li, L.; He, C.; Xu, B.; Liu, Y.; Wang, C.; Wang, Q. A Novel Ultrasonic Fatigue Test and Application in Bending Fatigue of TC4 Titanium Alloy. Materials 2023, 16, 5. https://doi.org/10.3390/ma16010005
Tang S, Wang X, Huang B, Yang D, Li L, He C, Xu B, Liu Y, Wang C, Wang Q. A Novel Ultrasonic Fatigue Test and Application in Bending Fatigue of TC4 Titanium Alloy. Materials. 2023; 16(1):5. https://doi.org/10.3390/ma16010005
Chicago/Turabian StyleTang, Sen, Xinyu Wang, Beihai Huang, Dongtong Yang, Lang Li, Chao He, Bo Xu, Yongjie Liu, Chong Wang, and Qingyuan Wang. 2023. "A Novel Ultrasonic Fatigue Test and Application in Bending Fatigue of TC4 Titanium Alloy" Materials 16, no. 1: 5. https://doi.org/10.3390/ma16010005
APA StyleTang, S., Wang, X., Huang, B., Yang, D., Li, L., He, C., Xu, B., Liu, Y., Wang, C., & Wang, Q. (2023). A Novel Ultrasonic Fatigue Test and Application in Bending Fatigue of TC4 Titanium Alloy. Materials, 16(1), 5. https://doi.org/10.3390/ma16010005







