A Frequency-Adjustable Tuning Fork Electromagnetic Energy Harvester
Abstract
:1. Introduction
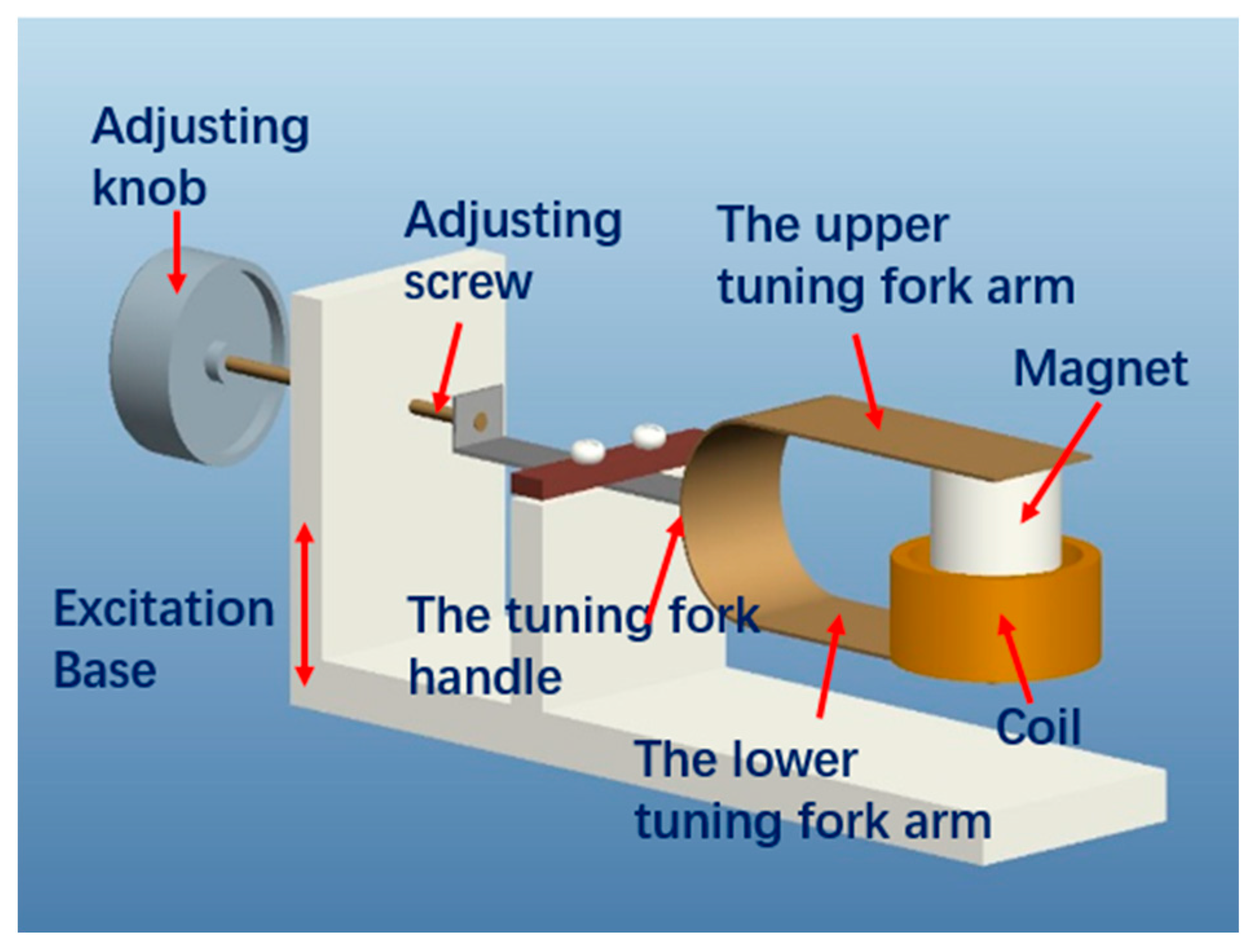
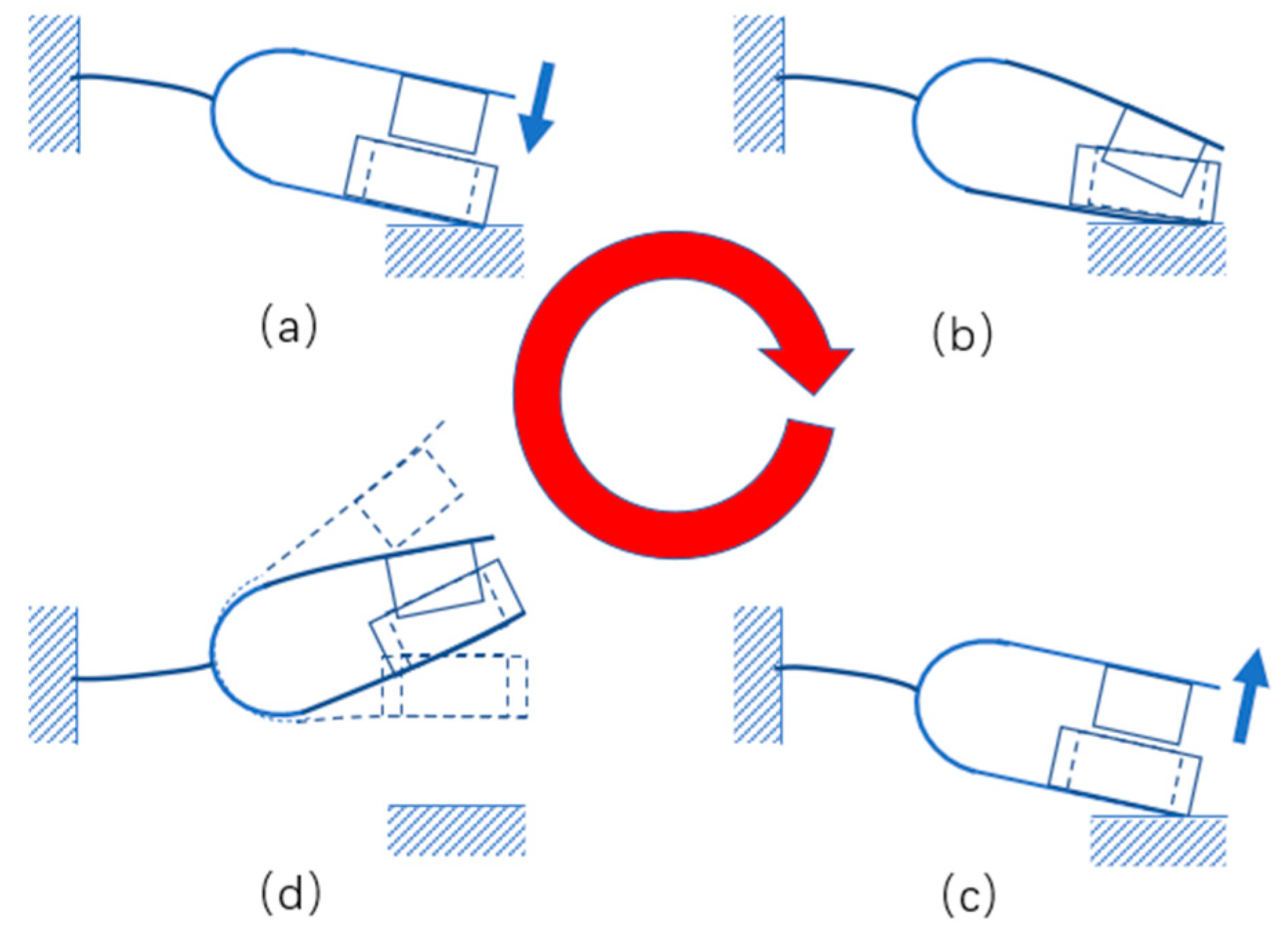
2. Design and Working Principle
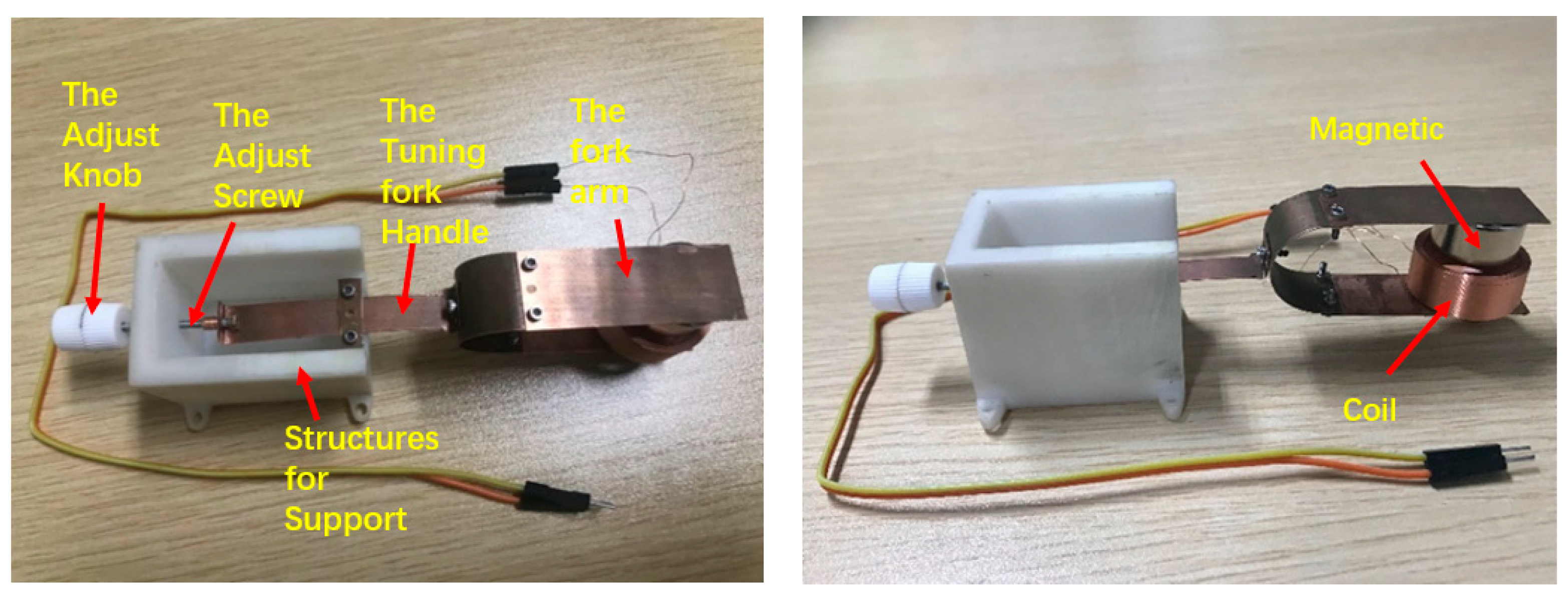
2.1. The Structure Design
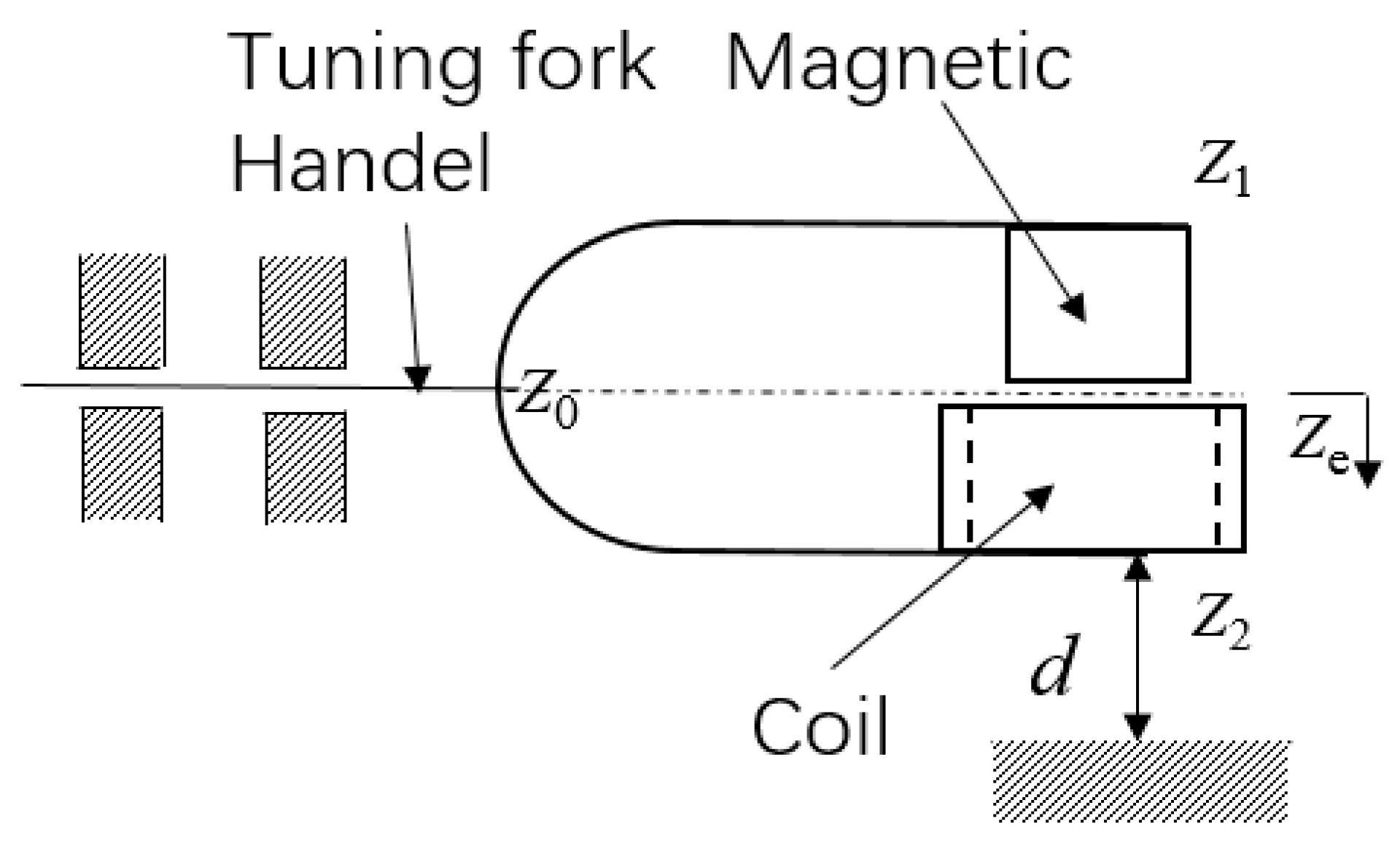
2.2. Electromechanical Coupling Dynamics Model
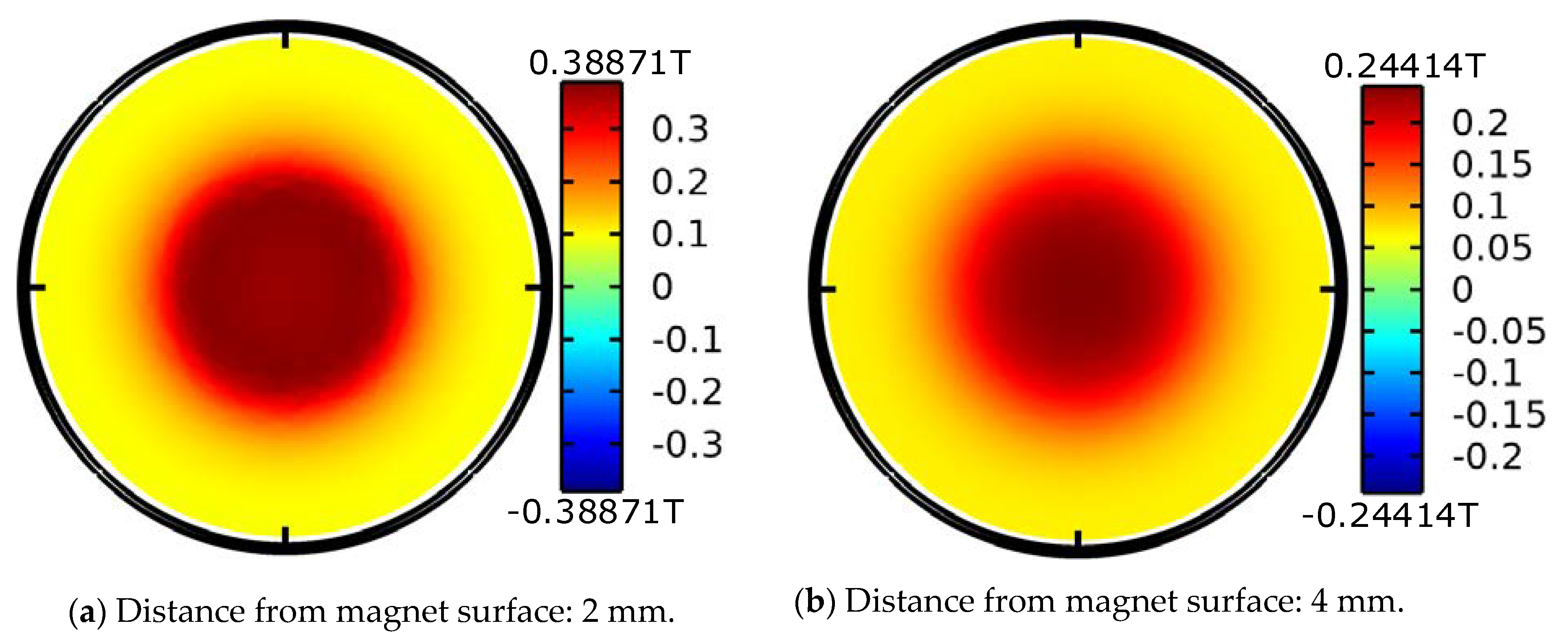
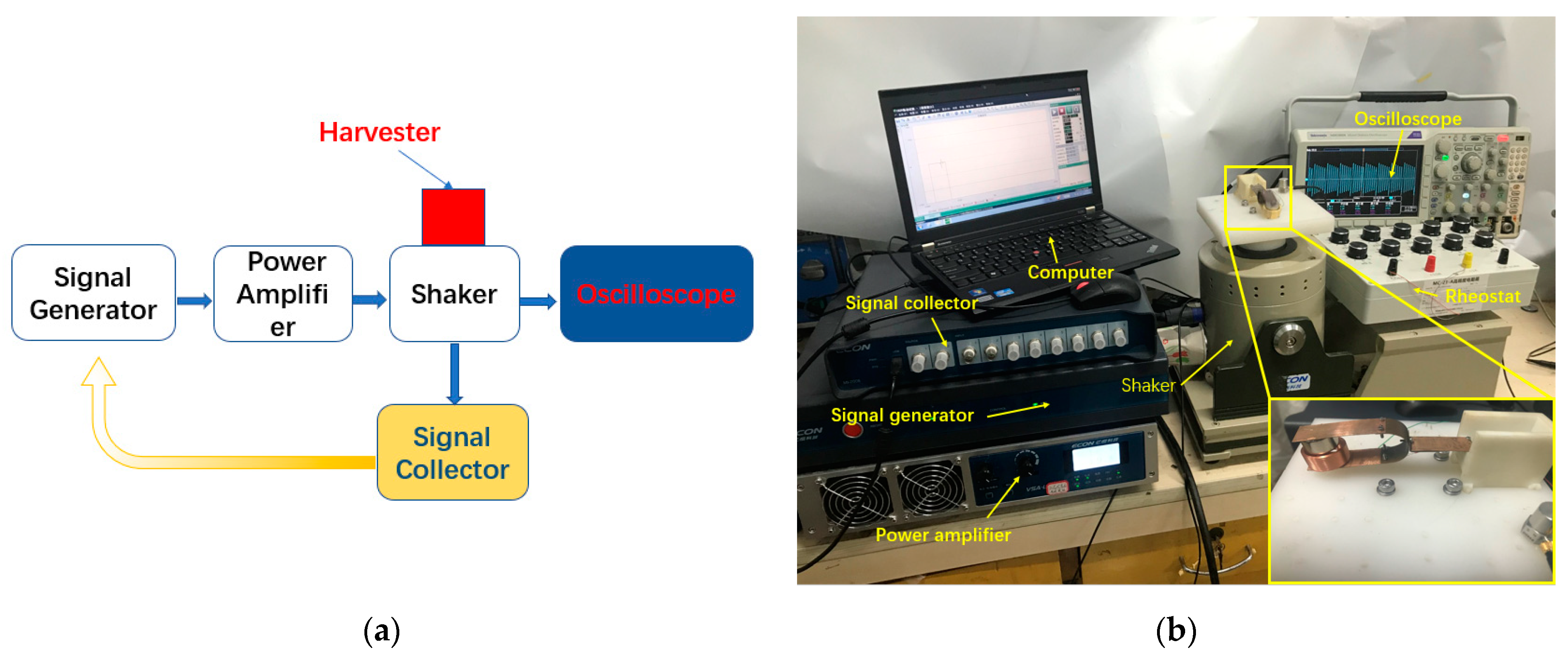
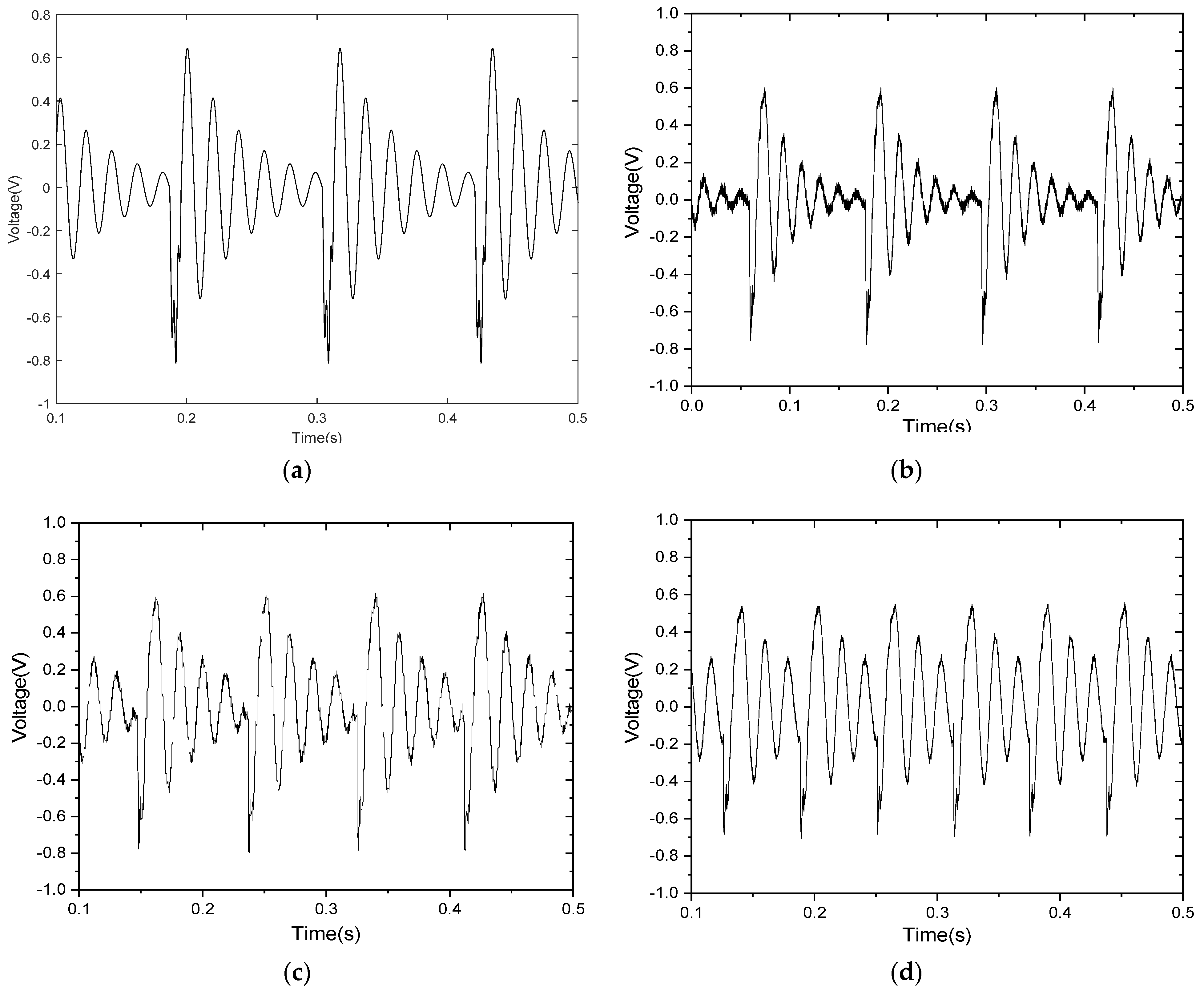
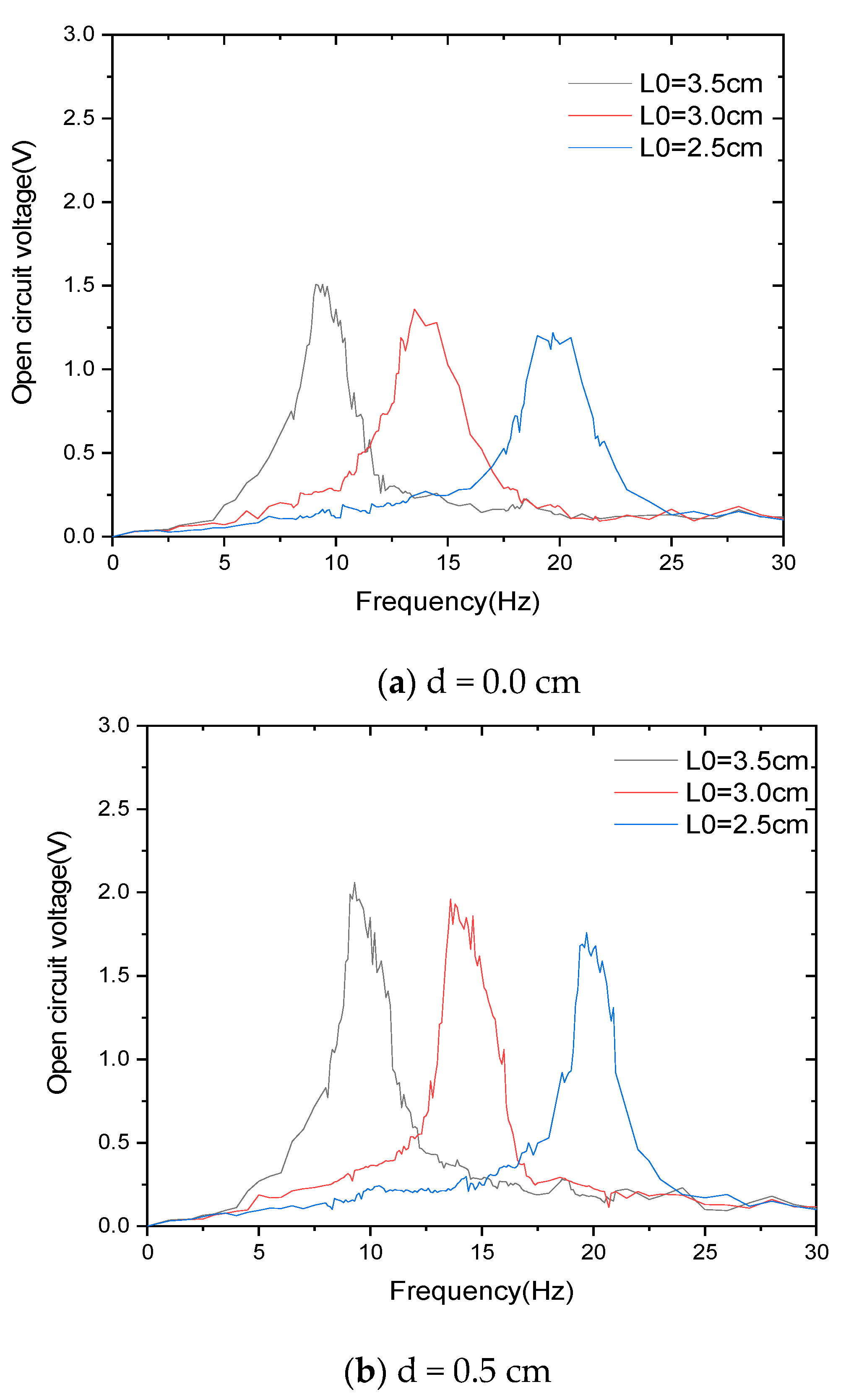
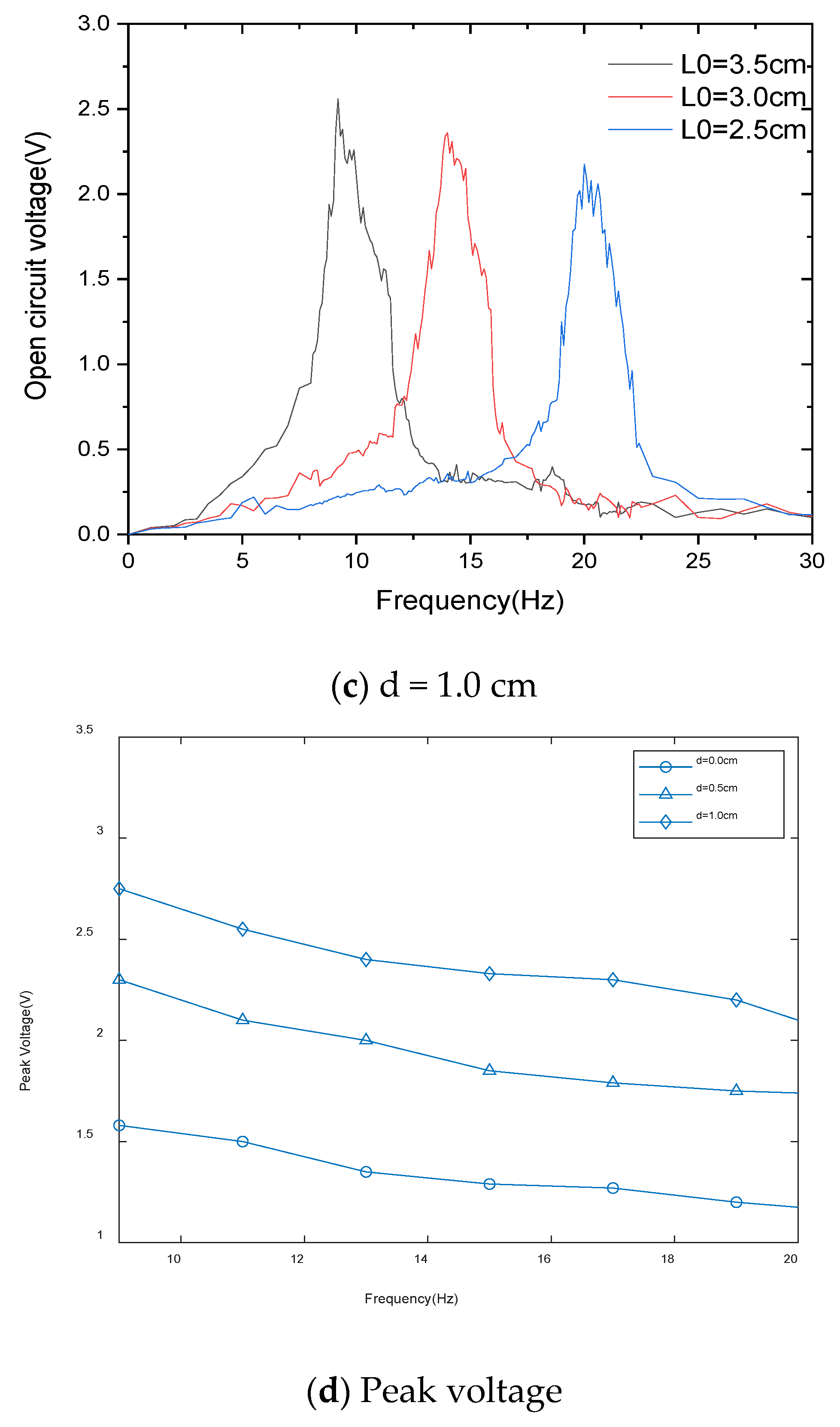
3. Simulation and Experiment
4. Power Supply Experiment for Electrical Appliances
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elvin, N.G.; Elvin, A.A. An experimentally validated electromagnetic energy harvester. J. Sound Vib. 2011, 10, 2314–2324. [Google Scholar] [CrossRef]
- Foisal, A.; Hong, C.; Chung, G.S. Multi-frequency electromagnetic energy harvester using a magnetic spring cantilever. Sens. Actuators A Phys. 2012, 182, 106–113. [Google Scholar] [CrossRef]
- Zhang, Y.; Zheng, R.; Shimono, K.; Kaizuka, T.; Nakano, K. Effectiveness Testing of a Piezoelectric Energy Harvester for an Automobile Wheel Using Stochastic Resonance. Sensors 2016, 10, 1727. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, X.; Chen, C.; Wang, N.; San, H.; Yu, Y.; Halvorsen, E.; Chen, X. A frequency and bandwidth tunable piezoelectric vibration energy harvester using multiple nonlinear techniques. Appl. Energy 2017, 190, 368–375. [Google Scholar] [CrossRef]
- Liu, F.R.; Zhang, W.M.; Zhao, L.C.; Zou, H.X.; Tan, T.; Peng, Z.K.; Meng, G. Performance enhancement of wind energy harvester utilizing wake flow induced by double upstream flat-plates. Appl. Energy 2020, 257, 114034. [Google Scholar] [CrossRef]
- Febbo, M.; Machado, S.P.; Gatti, C.D.; Ramirez, J.M. An out-of-plane rotational energy harvesting system for low frequency environments. Energy Convers. Manag. 2017, 152, 166–175. [Google Scholar] [CrossRef]
- Johar, M.A.; Kang, J.H.; Hassan, M.A.; Ryu, S.W. A scalable, flexible and transparent GaN based heterojunction piezoelectric nanogenerator for bending, air-flow and vibration energy harvesting. Appl. Energy 2018, 222, 781–789. [Google Scholar] [CrossRef]
- Sun, Y.; Gao, X.; Wang, H.; Chen, Z.; Yang, Z. A wideband ultrasonic energy harvester using 1–3 piezoelectric composites with non-uniform thickness. Appl. Phys. Lett. 2018, 4, 043903. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, L.; Cheng, T.; Song, Z.; Qin, F. Sealed piezoelectric energy harvester driven by hyperbaric air load. Appl. Phys. Lett. 2016, 3, 033902. [Google Scholar] [CrossRef]
- Iqbal, M.; Nauman, M.M.; Khan, F.U.; Abas, E.; Cheok, Q.; Aissa, B. Nonlinear multi-mode electromagnetic insole energy harvester for human-powered body monitoring sensors: Design, modeling, and characterization. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2021, 22, 6415–6426. [Google Scholar] [CrossRef]
- Wei, C.; Jing, X. A comprehensive review on vibration energy harvesting: Modelling and realization. Renew. Sustain. Energy Rev. 2017, 74, 1–18. [Google Scholar] [CrossRef]
- Halim, M.A.; Rantz, R.; Zhang, Q.; Gu, L.; Yang, K.; Roundy, S.J.A.E. An electromagnetic rotational energy harvester using sprung eccentric rotor, driven by pseudo-walking motion. Appl. Energy 2018, 217, 66–74. [Google Scholar] [CrossRef]
- Wu, X.; Li, G.; Lee, D.W. A novel energy conversion method based on hydrogel material for self-powered sensor system applications. Appl. Energy 2016, 173, 103–110. [Google Scholar] [CrossRef]
- Rasel, M.; Park, J.Y. A sandpaper assisted micro-structured polydimethylsiloxane fabrication for human skin based triboelectric energy harvesting application. Appl. Energy 2017, 206, 150–158. [Google Scholar] [CrossRef]
- Pondrom, P.; Sessler, G.M.; Boes, J.; Melz, T. Compact electret energy harvester with high power output. Appl. Phys. Lett. 2016, 109, 1457. [Google Scholar] [CrossRef]
- Zhang, S.L.; Xu, M.; Zhang, C.; Wang, Y.C.; Zou, H.; He, X.; Wang, Z.; Wang, Z.L. Rationally Designed Sea Snake Structure Based Triboelectric Nanogenerators for Effectively and Efficiently Harvesting Ocean Wave Energy with Minimized Water Screening Effect. Nano Energy 2018, 48, 421–429. [Google Scholar] [CrossRef]
- Siddique, A.R.M.; Mahmud, S.; Van Heyst, B. A comprehensive review on vibration based micro power generators using electromagnetic and piezoelectric transducer mechanisms. Energy Convers. Manag. 2015, 106, 728–747. [Google Scholar] [CrossRef]
- Jiang, X.Y.; Zou, H.X.; Zhang, W.M. Design and analysis of a multi-step piezoelectric energy harvester using buckled beam driven by magnetic excitation. Energy Convers. Manag. 2017, 145, 129–137. [Google Scholar] [CrossRef]
- Dai, X.; Wen, Y.; Li, P.; Yang, J.; Li, M. Energy harvesting from mechanical vibrations using multiple magnetostrictive/piezoelectric composite transducers. Sens. Actuators A Phys. 2011, 166, 94–101. [Google Scholar] [CrossRef]
- Zhou, Z.; Qin, W.; Zhu, P. Improve efficiency of harvesting random energy by snap-through in a quad-stable harvester. Sens. Actuators A Phys. 2016, 243, 151–158. [Google Scholar] [CrossRef] [Green Version]
- Toyabur, R.M.; Salauddin, M.; Park, J.Y. Design and Experiment of Piezoelectric Multimodal Energy Harvester for Low Frequency Vibration. Ceram. Int. 2017, 43, S675–S681. [Google Scholar] [CrossRef]
- Wu, Y.; Qiu, J.; Zhou, S.; Ji, H.; Chen, Y.; Li, S. A piezoelectric spring pendulum oscillator used for multi-directional and ultra-low frequency vibration energy harvesting. Appl. Energy 2018, 231, 600–614. [Google Scholar] [CrossRef]
- Arrieta, A.F.; Hagedorn, P.; Erturk, A.; Inman, D.J. A piezoelectric bistable plate for nonlinear broadband energy harvesting. Appl. Phys. Lett. 2010, 10, 174103. [Google Scholar] [CrossRef] [Green Version]
- Fu, H.; Yeatman, E.M. A methodology for low-speed broadband rotational energy harvesting using piezoelectric transduction and frequency up-conversion. Energy 2017, 125, 152–161. [Google Scholar] [CrossRef] [Green Version]
- Halim, M.A.; Cho, H.; Park, J.Y. Design and experiment of a human-limb driven, frequency up-converted electromagnetic energy harvester. Energy Convers. Manag. 2015, 106, 393–404. [Google Scholar] [CrossRef]
- Pillatsch, P.; Yeatman, E.M.; Holmes, A.S. A piezoelectric frequency up-converting energy harvester with rotating proof mass for human body applications. Sens. Actuators A Phys. 2014, 206, 178–185. [Google Scholar] [CrossRef]
- Zhang, H.; Jiang, S.; He, X. Impact-based piezoelectric energy harvester for multidimensional, low-level, broadband, and low-frequency vibrations. Appl. Phys. Lett. 2017, 22, 223902. [Google Scholar] [CrossRef]
- Wang, Y.J.; Chen, C.D.; Sung, C.K. Design of a frequency-adjusting device for harvesting energy from a rotating wheel. Sens. Actuators A Phys. 2010, 159, 196–203. [Google Scholar] [CrossRef]
- Wu, Q.; Gao, S.; Jin, L.; Zhang, X.; Yin, Z.; Wang, C. A tuning fork frequency up-conversion energy harvester. Sensors 2021, 21, 7285. [Google Scholar] [CrossRef] [PubMed]
- Li, P.; Xu, N.; Gao, C. A Multi-Mechanisms Composite Frequency Up-Conversion Energy Harvester. Int. J. Precis. Eng. Manuf. 2020, 21, 1781–1788. [Google Scholar] [CrossRef]
- Dallago, E.; Marchesi, M.; Venchi, G. Analytical Model of a Vibrating Electromagnetic Harvester Considering Nonlinear Effects. IEEE Trans. Power Electron. 2010, 25, 1989–1997. [Google Scholar] [CrossRef]







| Parameter | Value |
|---|---|
| Diameter of magnet (NdFeB) | 17 mm |
| Thickness of magnet (NdFeB) | 10 mm |
| Remanent (Br) | 1.2 T |
| Coil (copper) number of turns | 500 |
| Outer diameter of coil (copper) | 24 mm |
| Inner diameter of coil | 20 mm |
| Young’s modulus of beryllium bronze (Ya) | 133 GPa |
| Size of the handle (l0 × b0 × h0) | 35~25 mm × 8 mm × 0.6 mm |
| Size of the arm (la × ba × ha) | 30 mm × 16 mm × 0.5 mm |
| The radius of the arc (R) | 10 mm |
| Stiffness coefficient of the arm (k) | 1420 N/m |
| Stiffness coefficient of the handle (ka) | 65 N/m |
| 0.015 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, Q.; Gao, S.; Jin, L.; Guo, S.; Yin, Z.; Fu, H. A Frequency-Adjustable Tuning Fork Electromagnetic Energy Harvester. Materials 2022, 15, 2108. https://doi.org/10.3390/ma15062108
Wu Q, Gao S, Jin L, Guo S, Yin Z, Fu H. A Frequency-Adjustable Tuning Fork Electromagnetic Energy Harvester. Materials. 2022; 15(6):2108. https://doi.org/10.3390/ma15062108
Chicago/Turabian StyleWu, Qinghe, Shiqiao Gao, Lei Jin, Shengkai Guo, Zuozong Yin, and He Fu. 2022. "A Frequency-Adjustable Tuning Fork Electromagnetic Energy Harvester" Materials 15, no. 6: 2108. https://doi.org/10.3390/ma15062108
APA StyleWu, Q., Gao, S., Jin, L., Guo, S., Yin, Z., & Fu, H. (2022). A Frequency-Adjustable Tuning Fork Electromagnetic Energy Harvester. Materials, 15(6), 2108. https://doi.org/10.3390/ma15062108







