Orientation Dependent Hardening by <001> Rod-Shaped Misfitting Precipitates in Aluminium Alloys
Abstract
1. Introduction
2. Theory
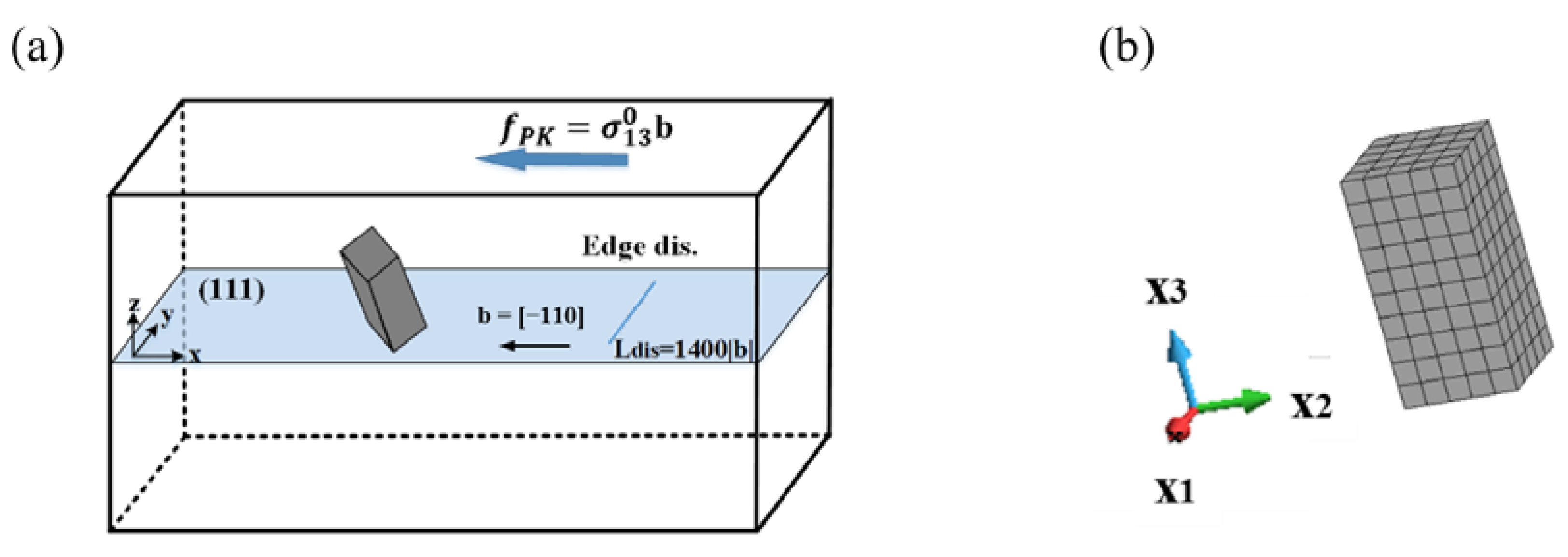
2.1. Geometry of Dislocation Interaction with a <001> Rod Precipitate
2.2. Computation of Dislocation Motion among Internal Stress of a <001> Rod
3. Results
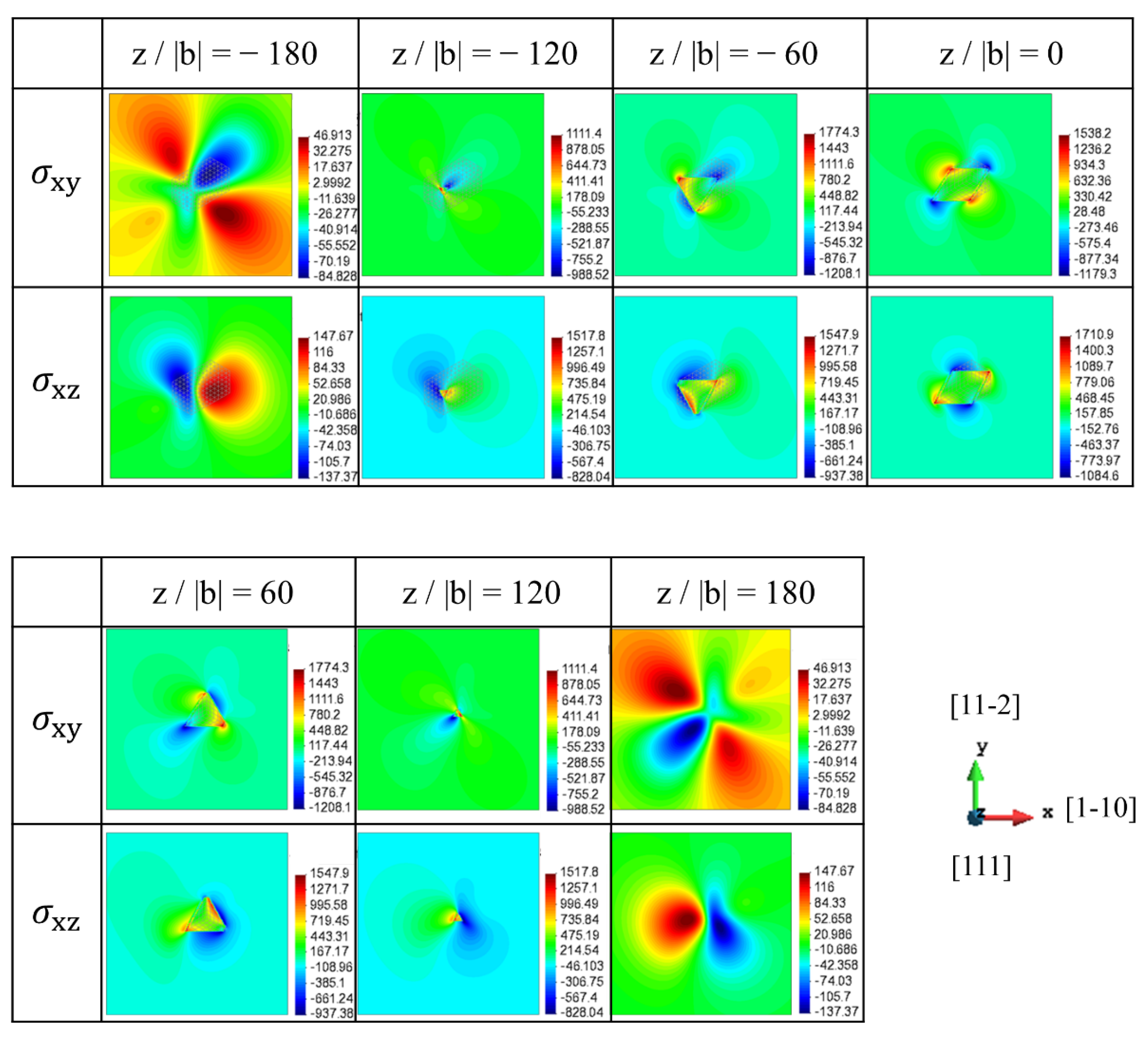
3.1. Stress Field of <001> Rod Precipitate on the (111) Dislocation Slip Plane
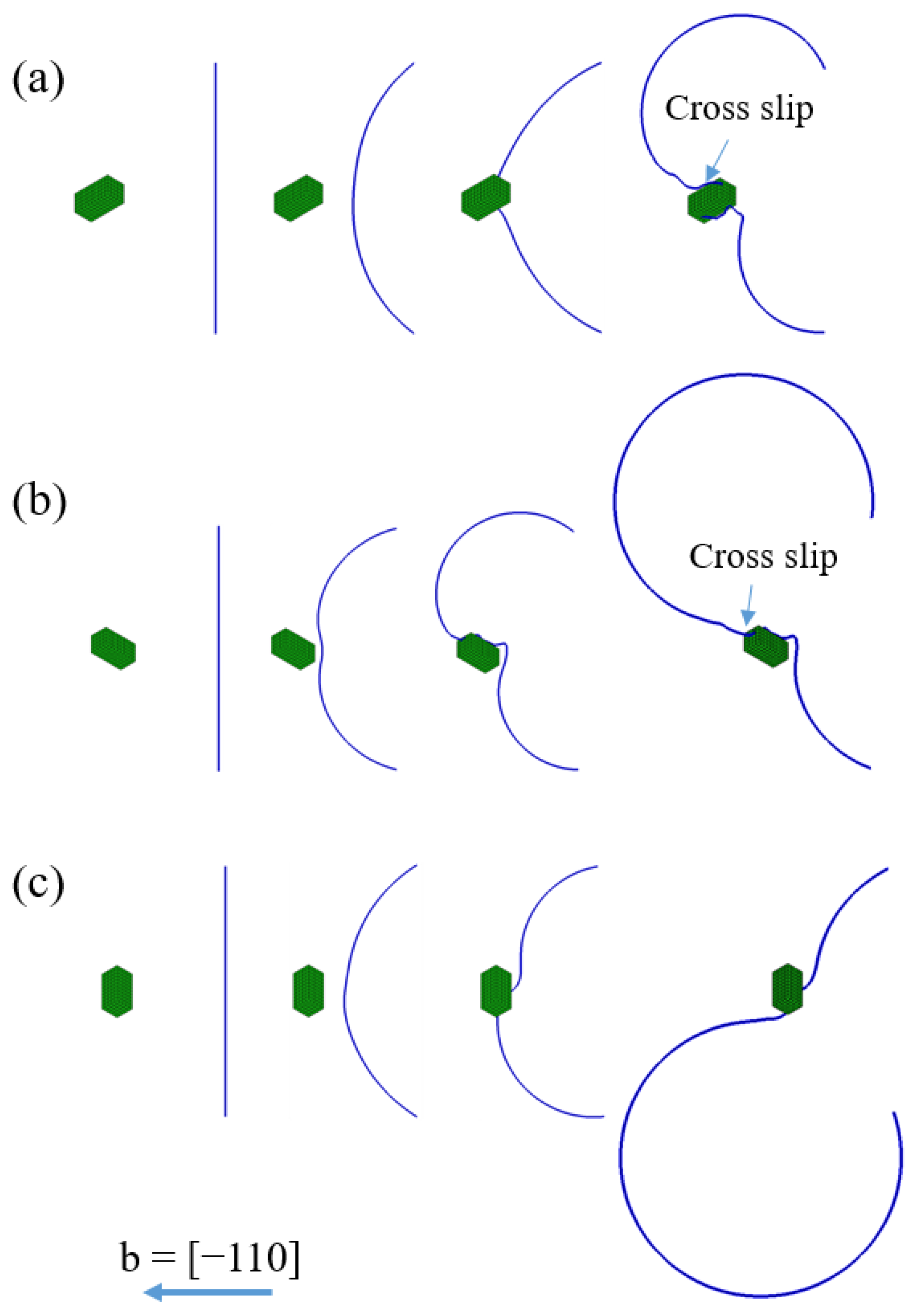
3.2. Dislocation Motion Behaviours around <001> Rod Precipitates
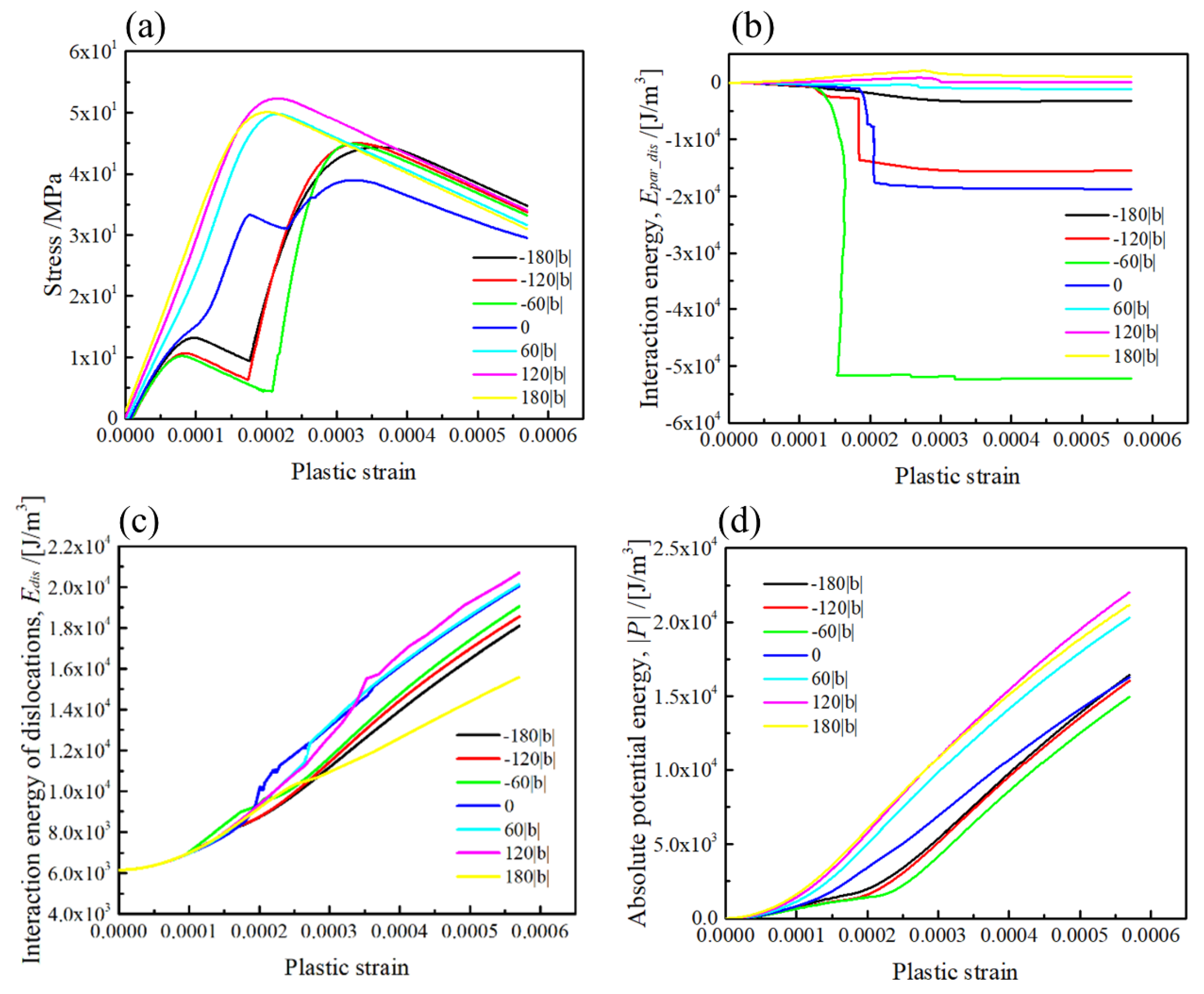
3.3. Orientation Dependent Hardening by <001> Rod Precipitates
4. Discussion
5. Conclusions
- (1)
- The stress field of the <001> variants on the dislocation slip plane was greatly changed with the orientation of the rod, which influenced the topological changes of dislocation motion and the resultant stress–strain curves.
- (2)
- Medium strengthening was observed when the Burger’s vector was normal to the longitudinal direction of the [001] rod (Type-A variant), while strong and weak hardening effects were found when Burger’s vector intersected the [100] and [010] rods with the inclined angle of 60 degrees (Type-B variants).
- (3)
- The maximum strengthening and slope of the stress–strain curves for overcoming dislocation in the cases of Type-A and Type-B variants are in good agreement with the prediction of the average interaction energy of the external stress and the misfit strain.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Byrne, J.G.; Fine, M.E.; Kelly, A. Precipitate hardening in an aluminium-copper alloy. Philos. Mag. 1961, 6, 1119–1145. [Google Scholar] [CrossRef]
- Russell, K.G.; Ashby, M. Slip in aluminum crystals containing strong, plate-like particles. Acta Met. 1970, 18, 891–901. [Google Scholar] [CrossRef]
- Naumenko, K.; Gariboldi, E.; Nizinkovskyi, R. Stress-regime-dependence of inelastic anisotropy in forged age-hardening aluminium alloys at elevated temperature: Constitutive modeling, identification and validation. Mech. Mater. 2020, 141, 103262. [Google Scholar] [CrossRef]
- Mura, T. Micromechanics of Defects in Solids, 2nd ed.; Kluwer Academic Publishers: Boston, MA, USA, 1987. [Google Scholar]
- Park, S.-J.; Muraishi, S. Micromechanical analysis of residual stress around coarse precipitates under cold rolling conditions. Mech. Mater. 2021, 157, 103841. [Google Scholar] [CrossRef]
- Tanaka, K.; Mori, T. The hardening of crystals by non-deforming particles and fibres. Acta Met. 1970, 18, 931–941. [Google Scholar] [CrossRef]
- Bellón, B.; Haouala, S.; Llorca, J. An analysis of the influence of the precipitate type on the mechanical behavior of Al-Cu alloys by means of micropillar compression tests. Acta Mater. 2020, 194, 207–223. [Google Scholar] [CrossRef]
- Milligan, B.; Ma, D.; Allard, L.; Clarke, A.; Shyam, A. Crystallographic orientation-dependent strain hardening in a precipitation-strengthened Al-Cu alloy. Acta Mater. 2021, 205, 116577. [Google Scholar] [CrossRef]
- Zandbergen, H.W.; Andersen, S.J.; Jansen, J. Structure Determination of Mg 5 Si 6 Particles in Al by Dynamic Electron Diffraction Studies. Science 1997, 277, 1221–1225. [Google Scholar] [CrossRef]
- Ehlers, F.J.; Holmestad, R. Ab initio based interface modeling for fully coherent precipitates of arbitrary size in Al alloys. Comput. Mater. Sci. 2013, 72, 146–157. [Google Scholar] [CrossRef][Green Version]
- Wenner, S.; Holmestad, R. Accurately measured precipitate–matrix misfit in an Al-Mg-Si alloy by electron microscopy. Scr. Mater. 2016, 118, 5–8. [Google Scholar] [CrossRef]
- Nicholson, R.B.; Nutting, J. Direct observation of the strain field produced by coherent precipitated particles in an age-hardened alloy. Philos. Mag. 1958, 3, 531–535. [Google Scholar] [CrossRef]
- Dahlgren, S.D. Coherency stresses, composition and dislocation interactions for θ″ precipitates in age-hardened Al-Cu. Met. Mater. Trans. A 1976, 7, 1401–1405. [Google Scholar] [CrossRef]
- Muddle, B.; Polmear, I. The precipitate Ω phase in Al-Cu-Mg-Ag alloys. Acta Met. 1989, 37, 777–789. [Google Scholar] [CrossRef]
- Suh, I.; Park, J. Influence of the elastic strain energy on the nucleation of Ω phase in Al-Cu-Mg(-Ag) alloys. Scr. Met. Mater. 1995, 33, 205–211. [Google Scholar] [CrossRef]
- Tanaka, Y.; Sato, A.; Mori, T. Stress assisted nucleation of α″ precipitates in Fe-N single crystals. Acta Met. 1978, 26, 529–540. [Google Scholar] [CrossRef]
- Eto, T.; Sato, A.; Mori, T. Stress-oriented precipitation of G.P. Zones and θ′ in an Al-Cu alloy. Acta Met. 1978, 26, 499–508. [Google Scholar] [CrossRef]
- Muraishi, S.; Kumai, S.; Sato, A. Stress-oriented nucleation of ω-phase plates in an Al-Cu-Mg-Ag alloy. Philos. Mag. A 2002, 82, 415–428. [Google Scholar] [CrossRef]
- Eto, T. Hardening due to oriented G.P. zones in Al-Cu single crystals. Scr. Met. 1980, 14, 133–135. [Google Scholar] [CrossRef]
- Muraishi, S.; Niwa, N.; Maekawa, A.; Kumai, S.; Sato, A. Strengthening of Al-Cu single crystals by stress-oriented Guinier-Preston zones. Philos. Mag. A 2002, 82, 2755–2771. [Google Scholar] [CrossRef]
- Singh, C.V.; Mateos, A.; Warner, D. Atomistic simulations of dislocation–precipitate interactions emphasize importance of cross-slip. Scr. Mater. 2011, 64, 398–401. [Google Scholar] [CrossRef]
- microMegas (mM) Code. Available online: http://zig.onera.fr/mm_home_page/ (accessed on 18 August 2021).
- Tridis: The Edge-Screw 3D Discrete Dislocation Dynamics (3D4) Code. Available online: http://www.numodis.fr/tridis/ (accessed on 18 August 2021).
- ParaDis: Parallel Dislocation Simulator Code. Available online: http://paradis.stanford.edu/ (accessed on 18 August 2021).
- Sills, R.B.; Kuykendall, W.P.; Aghaei, A.; Cai, W. Fundamentals of dislocation dynamics simulations. In Multiscale Materials Modeling for Nanomechanics; Weinberger, C.R., Tucker, G.J., Eds.; Springer International Publishing: Berlin/Heidelberg, Germany, 2016; pp. 53–88. [Google Scholar]
- Ghoniem, N.M. Curved Parametric Segments for the Stress Field of 3-D Dislocation Loops. J. Eng. Mater. Technol. 1999, 121, 136–142. [Google Scholar] [CrossRef][Green Version]
- Takahashi, A.; Ghoniem, N.M. A computational method for dislocation–precipitate interaction. J. Mech. Phys. Solids 2008, 56, 1534–1553. [Google Scholar] [CrossRef]
- Liu, H.; Gao, Y.; Qi, L.; Wang, Y.; Nie, J.-F. Phase-Field Simulation of Orowan Strengthening by Coherent Precipitate Plates in an Aluminum Alloy. Met. Mater. Trans. A 2015, 46, 3287–3301. [Google Scholar] [CrossRef]
- Santos-Güemes, R.; Esteban-Manzanares, G.; Papadimitriou, I.; Segurado, J.; Capolungo, L.; Llorca, J. Discrete dislocation dynamics simulations of dislocation-θ′ precipitate interaction in Al-Cu alloys. J. Mech. Phys. Solids 2018, 118, 228–244. [Google Scholar] [CrossRef]
- Hu, Y.; Curtin, W. Modeling peak-aged precipitate strengthening in Al-Mg-Si alloys. J. Mech. Phys. Solids 2021, 151, 104378. [Google Scholar] [CrossRef]
- Liu, J.; Muraishi, S. Energy analysis of misfit hardening by parametric dislocation dynamics simulation. Comput. Mater. Sci. 2020, 178, 109630. [Google Scholar] [CrossRef]
- Liu, J.; Muraishi, S. Orientation dependent hardening of {111} plate precipitate by parametric dislocation dynamics. Mech. Mater. 2021, 160, 103968. [Google Scholar] [CrossRef]




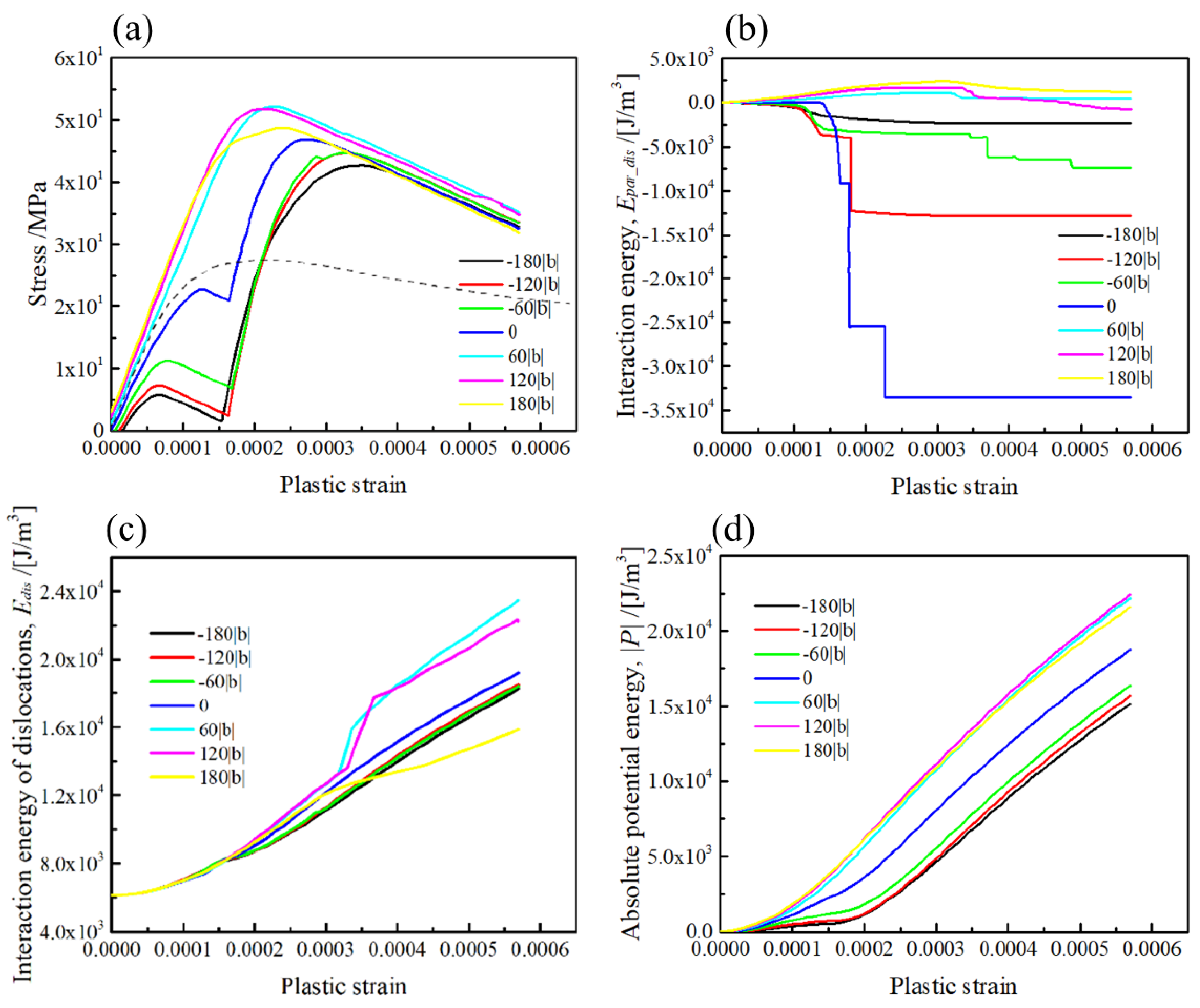
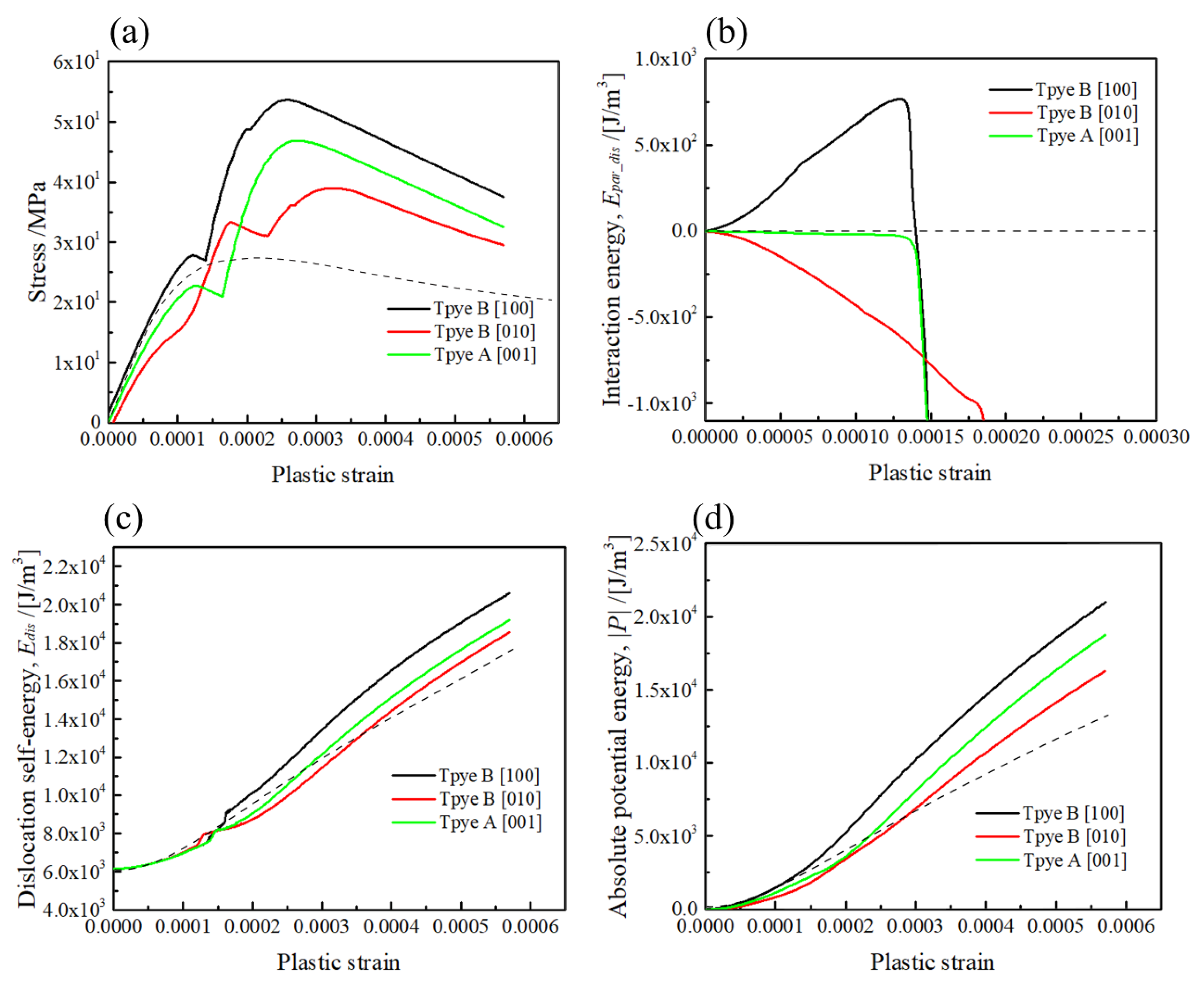
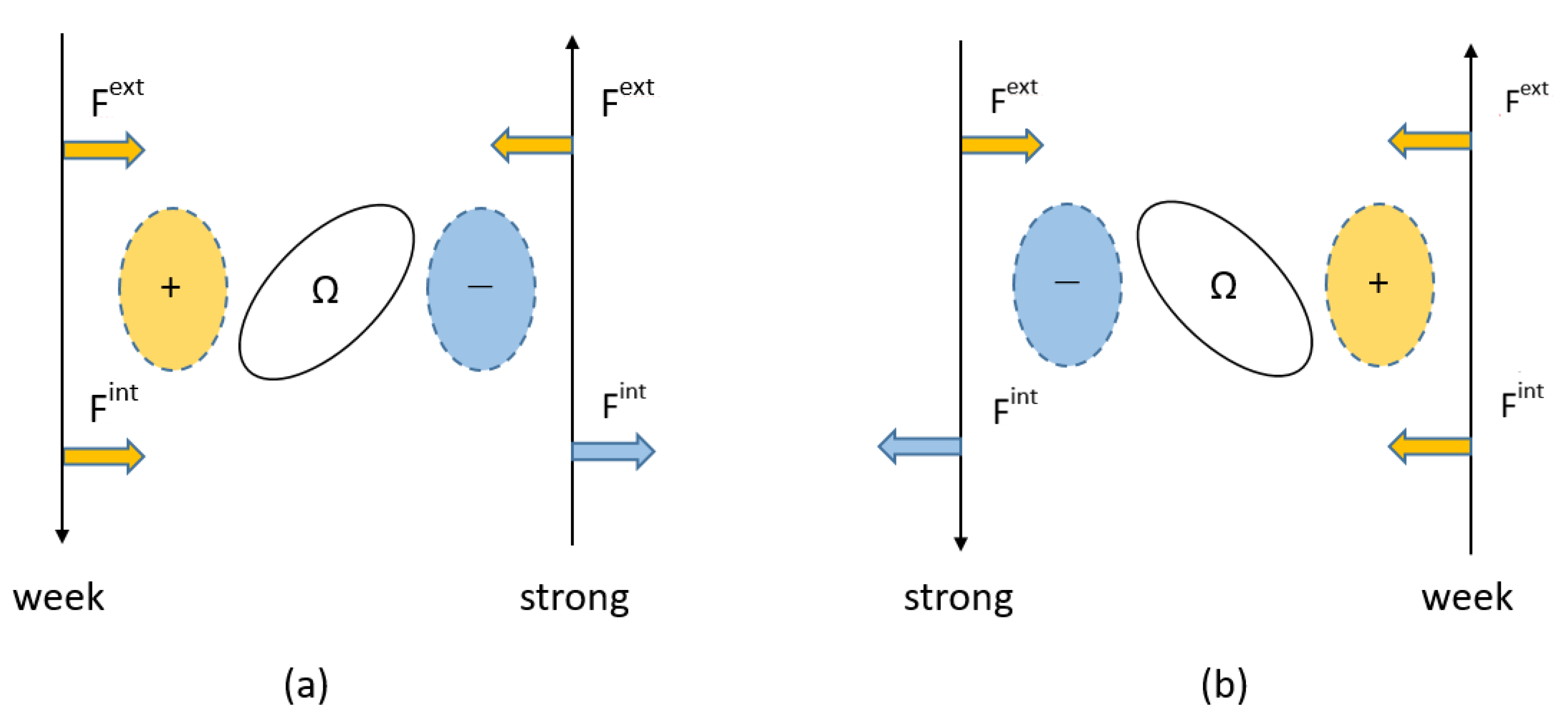
| Parameters | Magnitude |
|---|---|
| 27 GPa | |
| 0.3 | |
| 70 GPa | |
| 0.286 nm | |
| Burgers vector b | [−110] |
| Slip system | (111) [−110] |
| Precipitate size | (34 nm) (68 nm) |
| Initial length of dislocation | (400.4 nm) |
| Constant strain rate | |
| ) |
| Type of Interaction | Orientation | Average Interaction Energy | |
|---|---|---|---|
| Type-B | [100] | 54 | >0 |
| Type-B | [010] | 39 | <0 |
| Type-A | [001] | 46 | =0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Muraishi, S. Orientation Dependent Hardening by <001> Rod-Shaped Misfitting Precipitates in Aluminium Alloys. Materials 2022, 15, 1380. https://doi.org/10.3390/ma15041380
Liu J, Muraishi S. Orientation Dependent Hardening by <001> Rod-Shaped Misfitting Precipitates in Aluminium Alloys. Materials. 2022; 15(4):1380. https://doi.org/10.3390/ma15041380
Chicago/Turabian StyleLiu, Jianbin, and Shinji Muraishi. 2022. "Orientation Dependent Hardening by <001> Rod-Shaped Misfitting Precipitates in Aluminium Alloys" Materials 15, no. 4: 1380. https://doi.org/10.3390/ma15041380
APA StyleLiu, J., & Muraishi, S. (2022). Orientation Dependent Hardening by <001> Rod-Shaped Misfitting Precipitates in Aluminium Alloys. Materials, 15(4), 1380. https://doi.org/10.3390/ma15041380







